Stefanica D. Solutions Manual: A Primer For The Mathematics Of Financial Engineering
Подождите немного. Документ загружается.

114
CHAPTER
5.
TAYLOR'S
FORMULA.
TAYLOR
SERIES.
5.1.
SOLUTIONS
TO
CHAPTER
5
EXERCISES
115
Solution: Recall
that
the
Taylor series expansion of
the
function f(x)
=
♂
around
0 converges
to
eX
at
all points
♂
ε
JR.,
i.
e.
,
∞巾
k
♂=三示,
\i
xE
JR.
l
1-
x
2
L:
x
2k
= 1+ x
2
+
x
4
忖
6+...
,
\i
xE(-l
,
l)
口
Problem
4: Let
已
0.25
ι
(0.25)k
w _
ι(_l)k+l
x
k
T(x) =
):
be
the
Taylor series expansion of f (
x)
= In
(1
十
x).
Our
goal is
to
show
that
T(x) = f(x) for all x such
that
Ixl
<
1.
Let
已
(_l)k+l
x
k
Pn(x)
= )
~
be
the
Taylor polynomial of degree
ηcorrespondi
吨
to
f(x). Since T(x) =
limn
→∞
Pn(x)
,
it
follows
that
f(x) = T(x) for all
Ixl
< 1
if
and
only if
J
兰在
If(x)
一凡
(x)1
= 0,
\i
lxl<
1.
(5.3)
For x = 0.25
we
find
that
l.
e.
,
eO.
25
= lim x
n
, where x
n
=
安哗
fii7h
三
0
n
→
3
二--
"'.,
k=O
Note
that
the
sequence
{xn}n=O:
∞
is
increasing.
It
is
then
enough
to
compute
Xo
,
Xl
,
x2
,…, until
the
first seven decimal
digit~
_<!f
these
terms
are
the
same,
in
order
to
find
the
first six decimal digits of
e
U
'
均.
We find
that
Xo
=
1;
Xl
=
1.
25;
X2
=
1.
28125;
X3 =
1.
28385417; X4 =
1.
28401698; X5 =
1.
28402507;
X6
=
1.
28402540;
X7
=
1.
28402541,
and
conclude
that
(i) Show
that
, for any x ,
eO.
25
自
1.
284025.
口
f(x) -
Pn(x)
,
G
n
…
z-m
f\iz
,
1
1-x
L:
x
k
= 1 +x +x
2
+
x
3
十
,
\i
xE(-l
,l)
(ii) Show
that
,for any
0
三
x
< 1,
If(x) -
Pn(x)1
:三
x
n
In(l
十
x)
and
prove
that
(5.3) holds for all x such
that
0
三
x<
1.
(iii) Assume
that
-1
< x <
O.
Show
tl
时
If(x) -
Pn(x)1
三(
-x)nlln(l
十
x)1
and
conclude
that
(5.3) holds
true
for all x such
that
-1
< x <
O.
Solution: (i) From
the
integral formula for
the
Taylor approximation error
we
know
that
Problem
3:
Find
the
Taylor series expansion of
the
functions
叶泸)
and
击
around
the
point 0, using
the
Taylor series expansions of
In(l
-
x)
and
占
Solution: Recall
that
。c
z,.
.;:::.......
x
k
x
2
x
3
x
4
In (1 -
x)
=
一〉
;-z-z
一一一一一一一…,
V x E
[-1
,1);
乞
t
k 2 3 4
By
substituting
x
2
for x in
the
Taylor expansions above, where
Ixl
< 1,
we
find
that
。
c
一-
In (1 - x
2
)
=
一了
zffz-z2-21-Ef-zf-
7V
Z
ε(-1
,
1);
仨
t
k
234
{X
(x
-
t)n
An+l)
f(x) -
Pn(x)
= I
一石「
ftmj(t)dt.
Since f(x) = In
(1
+
x)
,
we
obtain
by
inductio
旦出
at
the
de
巾
atives
of
f(x) are
(5
.4)
(k) (
-l)k十
l(k
- 1)!
fwj(z)=
』
V
k
>1
(1
+
x)k
(5.5)

116
CHAPTER
5.
TAYLOR'S
FORMULA.
TAYLOR
SERIES.
5.1.
SOLUTIONS
TO
CHAPTER
5
EXERCISES
117
(5.6)
recall
that
, by definition 8 =
-x.
Note
that
,for
a
叮
Z
ε(-1
,
0)
,
卫鬼
(-x)nlln(l
+
x)1
= 0
From (5
.4)
and (5.5) it follows
that
{X
(x -
t)
n(-1
)7川
n!
J
f(x) - Pn(x) = I
一一~
\
-/
. d
Jo
n!
(1
十
t)
叫山
{X
(
-1)η
十
2(X
- t)n A
Jo
(1
+
t)
叫一
(ii) Let x
ε[0
,
1).
By taking absolute values in
(5.6)
and using
the
fact
that
We conclude
that
J
豆豆
If(x)
一凡
(x)1
0, V x
ε(-1
,
0).
(5.8)
主二!-
<
ι
\7
0<t<x<1
、
1
+t
一'
一一
,
we
obtain
that
lf(x)
一凡
(x)1
f(EY
T41dt
三
42Ifzdt
From (5.7)
and
(5.8)
we
obtain
that
Jl
忠
If(x)
一凡
(x)1
= 0, V x E
(-1
,
1)
,
and
co
叫
ude
t~at
the
Taylor series expansion of
the
function f(x) =
ln(l
十
x)
converges
to
f
(x)
for
a
叮
x
with
Ixl
<
1.口
We conclude
that
lim If(x) - Pn(x) I
0, V x
巳
[0
,
1).
(5.7)
Problem
5:
In
the
Cox-Ross-Rubinstein parametrizationfor a binomial tree,
the
up and down factors u and d, and
the
risk-neutral probability p of the
price going up during one time step are
主二三
<8
‘
\7
0<Z<8<
1.
1-z
一'
一一
'
{S
(8
-
z)n
f(x) -
Pn(x)
= - I
By taking absolute values
and
using
the
fact
that
(iii) Assume
that
x
巳
(-1
,
0)
and let 8 =
-x.
From
(5.6)
,
it
follows
that
{X(_1)n+2(x -
t)n
J
-I-
r-
s
(
-1)η
十 2(-8
- t)n J
f(x) - Pn(x) = I \
~~
I
~~-n+l
-/
dt
= I
。
(1
+
t)
肿
1
~V
Jo
(1
+
t)
叫山
{-s
(
-1?n+2(8
十
t)n
J
-I-
r-
s
(8
十
t)n
h
Jo
(1
+
t)
叫…。
(1
十
t)
叫…
Using
the
substitution t =
-z
,
we
obtain
that
A =
~
(川十川附
Use Taylor expansions
to
show
that
,for a small time step
8t
,u,d and p may
be approximated by
(5.12)
(5.13)
(5.14)
d
u
u =
A+VA
亡
I
(5.9)
d =
A-V
万
1·
(\
(5.10
\)
e
r8t
- d
(5.11)
p
一-
u-d'
where
e
CJ
Vlt
:
e
一
σJ
瓦
1 1
(T'
σ\~
p
-
一十一
i
一一~
I v8t.
2 '
2\σ2/
. --
In other words,write
the
Taylor expansion for (5.9-5.11) and for (5.12-5.14)
and show
that
they
are identical if all
the
terms of order 0 (
8t)
and smaller
are neglected.
Solution: We will show
that
the
Taylor expansion for (5.9-5.11)
and
for
(5.12-5.14) are identical if all
the
terms of order 0 ((
8t)
3
/2)
and smaller are
{S
(8
-
z)
η{
I:i
8
n
J_.
l a z < I
一一…
dz
Jo
(1
-
z)
卅
1
厅
-
Jo
1-
z
内
8
n
(一
l
叫
1
-
z))I;
二;
-8
叫
n(1-8)
=
8
n
ll
叫
1
-
8)1
(
-x)nlln(l
十
x)l;
If(x) - P
n
(x)1
we
find
that

118
CHAPTER
5.
TAYLOR'S
FORMULA.
TAYLOR
SERIES.
5.1.
SOLUTIONS
TO
CHAPTER
5
EXERCISES
neglected.
In
other words,
we
will show
that
U
eu
!8i
十
0((6t)3/2);
e
一
σ
!8i
十
0((6t)3/2).
1 1 , a
2
一十一
(γ
一三
)v6t十
0((
出
)3/2)
,
2
2σ2
d
p
Recall
the
following Taylor approximations:
x
e
l+x
十二十州
7
川→
0;
1+j
十
O(
此
as
x •
0;
l-j+0(
向
as
x • 0
In
particular,note
that
l
十
?+0
川)
飞
/i
十
O(M)
Then
,
A
=
~
(e-
r
<5
t
+
e(r+u
2
)
<5t)
;(1-dt+0((
们
)2)
+
1
十
(r
忖协
0((
们
)2))
1+
宁
十
O
叫仰州(仅仰阳(仔仰州
6
们
t
户=
(1+
平。
((ot)'))'
-
1
σ26t
+
0((
们
)2;
d
亡工工作
26t
+
0((6t)2)
=
σ
币、Ii十
0(6t)
σ
v6t
.(1
。但
!l
+
0((6t)2))
(
十一叫一
+
0((6t)2)
I
σ
v6t
+
0((
们
)3/2)
and
u
-
A 十
J
万
τ
丁
1
十字
+
0((M)2)
+
a
v6t
+
0((M)3
(5.15)
(5.16)
(5.17)
1
+
a
05t
+
宁
+
叫创仰州(付仰川
6
缸
t
A
一
J
万丁
I
1-
a
05t
+
字+
O(
川
d
Since
eU
V&t
而
(σ
yft;i)2
十一门一+
0((σ
05t
)3)
l+a
v6t
十
字
+0
叭咐州(仅
ω
阳
(θ
仰川
6
缸
t
1
一
d
十宁+叫川
e
σ
v&t
we
conclude
that
u
=
e
U
V&t
+0((M)3/2);
d
=
e-
u
V&t
+0((6t)3/2).
Therefore
,
(5.15) and
(5.16) are established.
Finally
,
e
r
<5t
-
d
p
工
u
-
d
(1
+
r6t
+
0((
圳))一
(1
一
σ
yft;i
十字
+
0((6t)3/2))
(1
+
a
yft;i
十字+
0((
们
)3/2)
)一
(1
-
a
yft;i
十字
+
0((6t)3/
升
σ
yft;i
+
(γ-
~)们十
0((
们)附)一
σ
十
(γ
一号
)yft;i
+
O(6t)
2σ
yft;i
+
0((6t)3/2)
2σ
十
O(
们)
1 1 ,
a
2
一十一
(γ
一巳
)v6t十
0((6t)3
j
2)
,
2
2σ2
wh
Problem
6:
(i)
What
is
the
approximate
value
~凡
αp
附
prox
money
put
option on
a
nor
丑
1
一
di
忖飞
vider
丑
ld
一
paying
underlying asset with
s
印
po
创
t
price
S
=
60
,
volatility
σ=
0.25
,
and
maturity
T
=
1 year
,
if
the
constant
risk-free
interest
rate
is
r
=
07
(ii)
Compute
the
Black-Scholes value
PB
归
S
氏向仲仲如?卢
r
俨户
r
伫严叫咆气
mate
the
relative
approximate error
IPBS
户闪
=0
-~α
即叫
r=O
,
q=ol
PBS
,
r=O
,
俨
O

120
CHAPTER
5.
TAYLOR'S
FORMULA.
TAYLOR
SERIES.
5.1.
SOLUTIONS
TO
CHAPTER
5
EXERCISES
(iii)Assume
thatT=0.06and
q=0.03Compute
the
approximate value
Pα
.p
prox
,
r=0.06
俨
0.03
of an ATM
put
option and estimate
the
relative approxi-
日
late
error
u
jPBS
,
r=0.06
,
俨
0.03
一凡
pprox
,
r=0.06
俨
0.031
P
B
S
,r=0.06,q=0.03 '
where P
B
S
,r=0.06,q=0.03
is
the
Black-Scholes value of
the
put
option.
Solution: (i) Using
the
approximate formula
_ / T
Pα
附町
=O
,
q=O
- asV2
1r'
for ATM call and
put
options do
not
satis
有
T
the
Put-Call
parity:
P
十
Se-
qT
- C S
(e-
qT
一
(γ
-
q)T)
并
Se-
rT
Ke-
r
(5.18)
Based on
the
linear Taylor expansion
e-
x
自
1
一凯
new
approximation
formulas for
the
price of AT
lV
I options which satisfy
the
Put-Call
parity can
be obtained by replacing
rT
and
qT
by 1 -
e-
rT
and 1 -
e-
qT
, respectively.
The
resulting formulas are
Pα
.p
prox
,
r=O
,
q=O
- 5.984134.
(ii) From
the
Black-Scholes formula,
we
find
that
PBS
,r=O,
q=O
= 5.968592,
JZehedSM-frT)
(5.19)
C
用
σ
Sy
;1r
-
2
十
jz
qT+
叮
S
(e-
qT
-
e-
rT
)
P 自
σ
S1/.!.-e
(5.20)
2
2
we
obtain
that
(iii)
Usi
吨
the
approximate formula
IT
(
(γ
+q)T
\
P
appr
叫附
=
aSy
;1r
\
1
一
j
we
obtain
that
Pα
.p
prox
,
r=0.06
,
q=0.03
= 4.814848.
From
the
Black-Scholes formula,
we
自 nd
that
PBS
,r=0.06,q=0.03 - 4.886985,
(i) Show
that
the
Put-Call
parity
is
satisfied by
the
approximations (5.19)
and (5.20).
(ii) Estimate how good
the
new
approximatio
丑
(5.20)
is
, for an ATM
put
with S =
60
, q
二二
0.03,
σ
= 0.25, and T = 1, if r = 0.06, by computing
the
corresponding relative approximate erro
r.
Compare this error with
the
relative approximate error (5.18) found in
the
previous exercise.
Solution: (i) From (5.19) and (5.20), it
is
easy
to
see
that
and therefore
IPBS
,
r=O
俨
0
一凡
pprox
,
r=O
,
q=O
1=
0.002604 = 0.26%.
PBS
,
r=O
,
俨
O
7
QU
p-c
十川=
-2
S
(寸
e-
rT
)
十
Se-
qT
_
Se-
rT
kfrT7
since K
=
S for ATM options.
(ii)
Using
the
new approximation formula (5.20)
,
we
obtain
that
and
therefore
IP
BS
,
r=0.06
,
俨
0.03
-
~α
pprox
,
r=0.06
,
q=0.031
=
0.014761
.r
BS
,
r=0.06
,
q=0.03
1
.4
761
%.口
凡
,p
prox_new
,
r=0.06
,
俨
0.03
-
4.86103
1.
The
Black-Scholes
value of
the
put
option
is
P
B
S
,
r=0.06
,
q=0.03
=
4.886985
,
and
therefore
IP
BS
,
问
0.06
,俨
0.03
-
~α
.p
prox_new
,
r=0.06
俨
0.031
工
0.005311
=
0.5311%.
PBS
,
r=0.06
,
q=0.03
Recall from Problem
7
that
the
approximation error corresponding
to
the
original approximation formula is
1.
4761%.
We
conclude
that
,
for this
particular example
,
the
new
approximation formula
is
more
accurate.
口
Problem
7:
It
is
interesting
to
note
that
the
approximate formulas
C
臼
σ
S
占
(ν1
卜
υJ
一
(r
价巳叮
γ
丁
γlγγγqω
旷
)T
P
自
σ
s
f[;
(1JTlq)T
十
(r
-
q)TS:
2
(γ
-
q)T
σ
2
Problem
8: Consider
an
ATM
put
option
with
strike
40
on
a
non-dividend
paying
asset
with volatility
30%
,
and
assume zero interest rates.
122
CHAPTER
5.
TAYLOR'S
FORMULA.
TAYLOR
SERIES.
5.1.
SOLUTIONS
TO
CHAPTER
5
EXERCISES
123
Compute
the
relative approximation error of
the
approximation
P
臼
σ
币汪叫叫…
p
川
1
,
3, 5,
10
, and
20
years
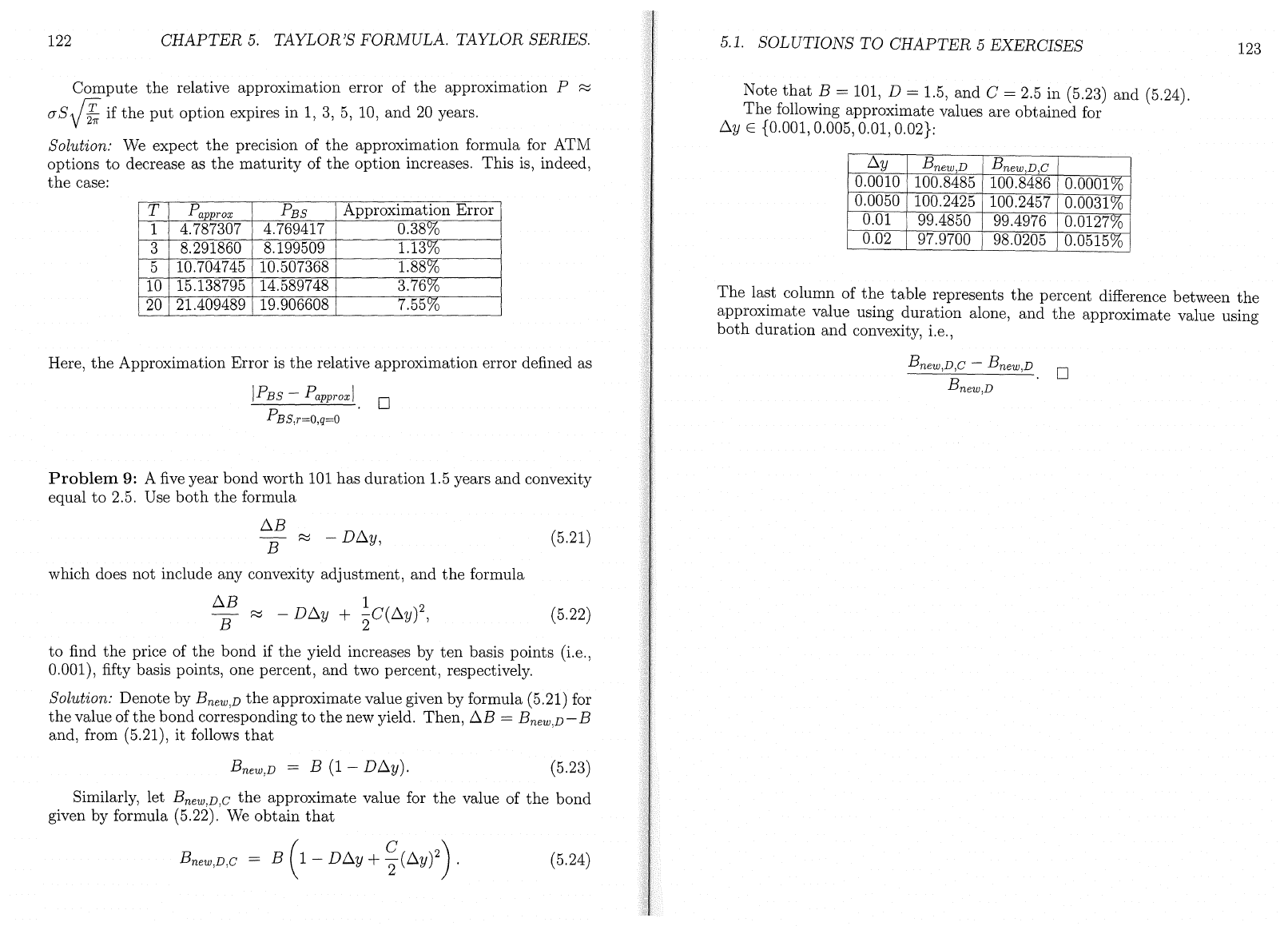
Solution: We expect
the
precision of
the
approximation formula for
ATJVI
options to decrease as
the
maturity
of
the
option increases. This
is
, indeed,
the
case:
T
Pα
pprox
PBS
Approximation Error
1 4.787307 4.769417 0.38%
3
8.291860
8.199509
1.
13%
5
10.704745 10.507368 1.88%
10
15.138795 14.589748 3.76%
20
21
.4
09489 19.906608
7.55%
Here
,
the
Approximation Error
is
the
relative approximation error defined as
IP
BS
一凡
pproxl
[
PBS
,
r=O
,
q=
。一
Problem
9:
A
在
ve
year bond worth
101
has duration
1.
5 years and convexity
equal
to
2.5. Use
both
the
formula
b:..
B
王一用一
D
b:..
y
,
(5.21)
which does
not
include any convexity adjustment, and
the
formula
Note
that
pz1017D
=157and
C
=25i
口
(5.23)
and (5.24).
The
following approximate values are obtained for
b:..
y
ε{0.001
,
0.005,0.01,0.02}:
b:..
y
B
ne
ω
,
D
1B0η0ε.ω8
,
4D8
,G
6
0.0010
100.8485
0.0001%
0.0050
100.2425
100.2457
0.0031%
0.01
99
.4
850
99
.4
976
0.0127%
0.02
97.9700
98.0205
0.0515%
The
last column of
the
table represents
the
percent difference between
the
approximate value miIlg d11ratioa
done?and
ttle approximate value usiIIg
both
duration and convexity, i.e.,
B
ne
ω
,
D
,G
-
B
ne
叽
D
〔
B
ne
ω
,
D
一
b:..
B
E
一目
-
D
b:..
y +
~C(
b:..
y)2
,
(5.22)
to
自
nd
the
price of
the
bond if
the
yield increases by
ten
basis points (i.e.,
0.001), fifty basis points, one
perce
风
and
two
perce
风
respectively.
Solution: Denote by
B
n
毗
D
the
approximate value given by formula (5.21) for
the
value of
the
bond corresponding
to
the
new yield.
Then
,
b:..
B =
B
ne
ω
.D-B
a
叫
from
(5.21), it follows
that
Bnew
,D = B
(1
- D
b:..
y).
(5.23)
Similarly, let Bnew.D.C
the
approximate value for
the
value of
the
bond
given by formula (5.22).
We
obtain
that
Bn
,w,D,C = B
(1
- D
t>
y
斗叫)
. (5.24)
124
CHAPTER
5.
TAYLOR'S
FORMULA.
TAYLOR
SERIES.
5.2.
SUPPLEMENTAL
EXERCISES
125
5.2
Supplemental
Exercises
1.
(i) Let g(x) be an
i
凶
nitely
differentiable function.
Find
the
linear and
q~adrati~
Taylor approximations of eg(x) around
the
point
O.
(ii) Use
the
result
~bove
to compute
the
quadratic Taylor approximation
around
0 of e(x+I)2
(iii) Compute
the
quadratic Taylor
approxi
可
lation
around 0 of e(x+I)2
by using Taylor approximations of
eX
and
eX·.
6.
The
goal of this exercise
is
to
compute
11In(1-
x)
In
例
dx
(5.26)
(i) Show
that
:地(同
-
x)
ln(
♂))
= li
m-
(问
-
x)
ln(x)) = 0,
x/I
2. Show
that
e-
X
一
-L=0(
内,
as
x
•
o
l
十
Z
and
conclude
that
the
integral
(5.26)
can
be
regarded
as
a definite
integra
l.
(ii) Use
the
Taylor
series
expansion
of
1
叫
1-
x)
for
jxj
<
1
to show
that
l'扑
μl1
〉」
1h
凶丑叫
(1
一
功训巾叫
ln
以坤口叫巾
(μ
川
Z
仨
IηJ
叫
O
3.
Compute
the
Taylor
series
expansion of
叫;号~)
(iii) Prove
that
around
the
point
0
,
and
find its radius
of
convergence.
[
In(l
一叫
立
1
4.
Recall
that
41·&
>-
Z
VV
14
Z
1-z
+
4i
//111\
<
<
z
\111J/'
1-z
/JIl--\
(iv) Use
that
fact
that
立主
=Z
>-
Z
Vv
l-2
+
1-z
/fiI\
<
<
-b
l-2
+
\1111/
1-z
4ti
/Iit\
4EU
a
h
4L
e
v
O
VA
PA
to
obtain
that
才叶到
ln(x
5.
(i)
Find
the
radius of
convergence
of
the
series
~4
、、
8
~12
1
十
L
十
L
十二一+.
2!
'
4!
'
6!
(5.25)
7.
Consider
an
ATM
put
option
with
strike
40
on
an
asset with volatility
30%
and paying
2%
dividends continuously.
Assume
that
the
interest
rates
are
constant
at
4.5%. Compute
the
relative
approximation
error
to
the
Black-Scholes
value of
the
option of
the
approximate
value
.IT
(
(7'十
q)T
\(
7'
-
q)T
Pα
附
oX
,
r
手
O
,
q
并
o
-σ
BV
;1T
\
1
一
FJ
-
if
the
put
option expires
in 1
,
3
,
5
,
10
,
and
20
years.
(ii)
Show
that
the
series from
(5.25)
is
the
Taylor
series expansion of
the
function
ez2
十
e-
X2
2

126
CHAPTER
5.
TAYLOR'S
FORMULA.
TAYLOR
SERIES.
5.3
Solutions
to
Supplemental
Exercises
Problem
1: (i)
Let
g(x)
be
an
infinitely differentiable function.
Find
the
linear
and
quadratic
Taylor approximations of
eg(x)
around
the
point
O.
(ii) Use
the
result above
to
compute
the
quadratic
Taylor
approximation
around
0 of
e(x+1?
(iii)
Compute
the
quadratic
Taylor
斗
pproximation
around
0 of
e(x
札
)2
by using
Taylor approximations of
eX
and
e
X
*.
Solution: (i)
Let
j(x)
=
eg(x).
Then
f'(x) = g'(x)eg(x)
and
j"(x)
= (g"(x) +
(g'
(x))2)e
g
(X).
The
linear Taylor
approximation
j(x)
=
j(O)
十
x
f' (O)
+
O(x
2
)
, as x • 0,
can
be
written
as
eg(x)
=
eg(O)
十
xe
到
O)g'(O)
+
O(x
2
)
, as x •
O.
The
quadratic
Taylor
approximation
j(x)
••
_,
x
2
_"
。
j(O) +
x
j'
(O)
十五
f
气。)
+
O(x
J
)
, as x • 0,
becomes
eg(x)
=
e
g
(
川
(5.27)
as
x •
O.
(ii)
By
letting
g(x) =
(x
十
I?
in
(5.27)
,we find
that
e(
叫
)2
工
e
+
2ex
+
3ex
2
+ 0
(川),
as x •
O.
(iii)
Usi
吨
the
quadratic
Taylor approximations
(2X)2
I
"f
~3\
1 I
(L
I (2x)2
e""x
= 1 + 2x + \
-;一
+
O(x
J
)
, = 1 +
2x
十一
γ
+
O(x
3
)
, as x •
0;
e
x2
_ 1+
x
2
十
O(x
气
as
x • 0,
it
follows
that
e(x
十
1)2=e
-dz-et=e(l
十
2x
十
2x
2
)(1
十
x
2
)
十
O(x
3
)
e
十
2ex
+
3ex
2
+
O(x
3
)
, as x
→
O.
口
5.3.
SOLUTIONS
TO
SUPPLEMENTAL
EXERCISES
127
Problem
2:
Show
that
e-
x
一
-L=0(z2)7aS
Z
•
0
1
十
Z
Solution:
The
qu
础
atic
Taylor
approximations of
e-
x
and
l~X
are
I-x
十二十州,
as
x
•
0;
1-
x
+
x
2
十。但
3)
,
as x
•
O.
1
1
十
Z
Therefore
,
e-
x
一
-L=
一主十
O(
泸)
l+x
Note
that
we implicitly proved
that
。但
2)
,
as x •
O.
1
e-
x
一-
l+x
一二十
O(x
3
)
,
as x
-t
0
口
Problem
3:
Compute
the
Taylor series expansion of
In
(1
+纠
飞
1-
x J
around
the
point
0,
and
find
its
radius
of convergence.
Solution:
Note
that
the
function
叫
;52)=l
中川川
is
not
defined for x =
-lor
x =
1.
Therefore,
the
largest possible radius of
convergence of
its
Taylor series expansion
around
0 is
1.
The
Taylor series expansions
of
the
functions
In(l
十
x)
and
In(l
-
x)
are
In
(1
+
x)
立
fz2z3z4
(-OMJZZ
一一十一一一+...
, V
♂
ε(-1
,
1];
k 2
'3
4
军空气
x
k
x
2
x
3
x
4
一予:二
=
-x
一一一一一一一...
, V x
ε[-1
,
1)
,
乞
fk
234
In
(1
-
x)
128
CHAPTER
5.
TAYLOR'S
FORMULA.
TAYLOR
SERIES.
and
have radius of convergence equal
to
1.
We conclude
that
the
Taylor
s~ries
expansion of In
(i~~)
is
叫;占~)
In(1 +
x)
一
l
叫
1
-
x)
( • ,
I.
, 1 x
k
x
k
\λ
2x
2j
+
1
t;
((一叫+讨
=
至
271
=
2
叶子+车十
,\I
川
xEε
川)
and
has radius of
c
∞
O
∞
I
口
1
飞
vergence
equal
tω0
1.口
Problem
4: Prove
that
(1+D
忖
<
e <
(1
十
~r\
\l
x?1
Sol
州
on:
Recall from (5.28)
that
(]斗"\
2
y
3
,
2ν5
In (
~一立)
=
2y
十一+一十...
,
\I
y E
(-1
,1).
飞
1
-
y)
-0'
3 ' 5
For any
x
三
1
,
substitute
y
=
古
i
口
(5.30)
and
obtain
that
(5.28)
(5.29)
(5.30)
咔
+D
=击+…
2
Jwn2
4
、民十
,
\I
x > 1,
which
can
also
be
written
as
(什)咔
+1)=1+
。
J
川十川一
1
亏\d
+.
.,
,
\I
x >
1.
(5
出)
XI
From (5.31),
we
find
th
创
1 <
(→)咔
+:)7VQ17
which is equivalent
to
/
...\
x+~
e <
(1
十二
I
,\I
x
二三1.
飞
X I
The
right inequality of (5.29) is therefore established.
(5.32)
5.3.
SOLUTIONS
TO
SUPPLE
l\
1ENTAL
EXERCISES
129
Fr
。因
(5.31)
,
we
also find
that
\liI/
1-z
+
414
/II11\
n
-EA
\liI/
Z
/IIl-\
1
十
1y
一」
3(2x
十
1)2
位
(2x
+
1)2k
1 1
1
十
3(2z+1)21-tF
一
1+
一」龟
12x(x
十
1)'
and
therefore
that
(1+~时
1
十一)
X+
j <
e
1
吨
\l
x?1
Z
(5.33)
Recall
that
e<(l+:yl
Using (5.34),
we
丑
nd
from (5.33)
that
\l
x>
1.
(5.34)
(1
十
~r
十二
e
卢
<
e(1+:)?
VG17
and
we
conclude
that
(1
十
D
叫一百
< e,
\I
x
二三
1.
The
left inequality of (5.29) is therefore
established.
口
Problem
5: (i)
Find
the
radius of
converge
肌
e
of
the
series
+
t-2
4t4
(5.35)
(ii) Show
that
the
series from (5.35) is
the
Taylor series expansion of
the
function
ex2 +
e-
x2
2
Solution: (i)
The
series (5.35) can
be
written
as a power series as follows:
>-
DA
Vv
-n
w
z
hv
Z
T
5.3.
SOLUTIONS
TO
SUPPLEMENTAL
EXERCISES
130
CHAPTER
5.
TAYLOR'S
FORMULA.
TAYLOR
SERIES.
131
Problem
6:
The
goal of this exercise
is
to
compute
From Stirling's formula
we
know
that
lim
__
k!
1
日
1
一一----,一
k
→
χ(~)κd
在
,.
(
2p
\
1/
2
1
Ll吨
1
...."
-
11
日
1
I
,'~
,,,.
In
I
一…一一一
τ;:;-
P
叫
((2p)
!)1/
4
p -
p~
叩\
((2p)!
)l /2
p
)
(2p)1/
e
1/2
lim
~
= 0
p
→
x
飞
/2p
Therefore,
the
radius of convergence of
the
power series T(x) is
ll
R
一
-
lims
叩
k
→况
|α
kl
1/k
limp
→∞
|α
4p\1
/
4
p
~~,
which means
that
the
series
(5.35)
is
co
盯
ergent
for all x E
JR
(ii) Using
the
Taylor series expansion
;倍
;21+
主
i
二
;11)
1
王三产+
(_1)k
x
2k
f
工
x
4j
- -
---
2
位
k!
但
(2j)!
1+z4z8212
37+ZI+
石「+
which is
the
same as
the
series
(5.35).
口
It
is
then
easy
to
see
that
and therefore
that
Using (5.36),
we
自
nd
tl
川
J
坦
lα
年
111
年
it
is easy
to
see
that
ez2
十
e-
X2
2
[
In(1
一叫)
(5.37)
1.
们
(k!)l
/
k
..…-
k
二二
x
k
(i) Show
that
:地(问-
x)
In
(x)) = lim
(叫-
x)
In(x)) = 0,
x/1
1
e
p
n4
,,,,,,,,
25
lp
a
时
co
肌
lude
that
the
integral
(5.37)
can be regarded as a
de
直
nite
integral.
(ii) Use
the
Taylor series
expansio
口
of
ln(l
-
x)
for
Ixl
< 1
to
show
that
=ε
(5.36)
才
1ln
川
h
川
(iii) Prove
that
[ln(1
一叫)
dx
立
1
(iv) Use
that
fact
that
立去
z
z
to
obtain
that
ez=57Vzd?
才
112(1
一叫)
dx
工
2-Z
Sol
毗
on:
(i) First of all,note
that
?V
口
(1
-
x)
In(x)) = lim
(同
-
x)
ln(x))
x/1
(5.38)
We compute
the
left
hand
side limit of
(5.38)
by changing it
to
a limit to
infinity corresponding
to
y =
~
as follows:
:与(l
n(l
一仙
(x
))
vhu
UU
n
-ai
1-U"1
1/fIi1\/JI--\
/i\Bh
132
CHAPTER
5.
TAYLOR'S
FORMULA.
TAYLOR
SERIES.
5.3.
SOLUTIONS
TO
SUPPLE
1V
IENTAL
EXERCISES
133
丑
ecall
that
From
(5
.4
1)
and
(5
.4
2)
,
it
follows
that
[忡
立
1
(5
.4
3)
主
G
一击)
zA
兰
G
一击)
From
(5
.4
3)
and
(5
.4
4)
,
we
conclude
that
[I
中叫
l
川
Problem
7: Consider
a
口
ATIVI
put
option with strike
40
on an asset with
volatility 30%
and
paying
2%
dividends continuously. Assume
that
the
in-
terest rates are constant
at
4.5%. Compute
the
relative approximation error
of
the
approximation
IT
(
(γ
十
q)T
\(γ
-
q)T
凡
,
pprox
,
呐
J41-j)=:
and therefore
J
坦咐
-D")
=
-1
From (5.39) and
(5
.4
0)
it follows
that
ln(ν)
l
地
(ln(1-z)lm(z))=J
马亏一=
0
We conclude
that
(5
.4
0)
(iv) Note
that
l
n(
η+
1)
Then
,
it
is easy
to
see
that
1
η(η
十
1)2
1 1
η(η
十
1)η+1
111
n
n+
1
(η+1
)2
炖(川
-
x)
ln(x))
=
li~
(ln
(1-
x)
ln(x))
= 0
x/I
The
integral (5.37)
is
equal
to
the
de
自缸
fin
丑旧
l
让
it
怡
e
in
丑挝
t
怡
egra
址
1
be
创
tweer
丑
1
0 and 1 of
the
C
∞
O
∞
I
丑毗
1
g(O
创)
=
g(l)
=
0;
g(x)
=
ln(l
一
x)l
叫
x)
,
VO<x<
1.
(ii)
The
Taylor series expansion of
ln(l
x)
,i.
e.
,
V
「
ZK
M
川
lι
一
Z
叫
)
=
一
L
忑
Vx
托叫
ε
叫(
一→
-1
,
1υ
,
1
均)
i
坦
s
absolutely
c
∞
or
盯
erge
旧
to
1
口
(1
-
x).
Then
,
[ln(1-X)ln(x)
dx
=
-
[信
nl:(x)
)
ln(
1
-
x)
ln(
x)
dx
=
-
I
I)
~
~一一
l
In
\....-
n
I
-Zifznln(Z)dz
Therefore
,
汇
1
\III-/
/IIlI\
Here
,
we
used
the
fact
that
立主
=Z
and
the
telescoping
series
(5
.4
1)
(iii)
Using
integration
by
parts
,
it
is
easy
to
see
that
f
x
n
+
I
ln(x)
x
n
+
I
znln(z)dzzd-r
Then
1
1
xnln
仪
(
x
n
+
I
l
日
(x)
一
x
n
+
I
\[
η
+
1
(η
十
1
)2
)
10
L
一一
~~.~~(x
肿
Il
n
(x)
)
(η+
1)2
dF
VGl
(5
.4
2)
η(η+
1)
(η+
1)2
一汇去
(5
.4
4)
lN
1.
