Stefanica D. Solutions Manual: A Primer For The Mathematics Of Financial Engineering

Подождите немного. Документ загружается.

CHAPTER

PROBABILITY.

BLACK-SCHOLES

FORlvIULA.

3.1.

SOLUTIONS

CHAPTER

EXERCISES

where

since d

十

VTτ

and

therefore

θKθK

differentiati

吨

(3.10)

with

respect

K ,

find

that

空气工

e-r(T-t)

N'(d

)

纽工

e-r(T-t)

τLe

手

252

叫

三作

θK

Note

that

lim

∞

and

lim

仇=一∞.

(T-t)

→∞

(T-t)

→∞

Then

li~

N(d

) = 1 and

，

~li~

N(d

) = 0

(T-t)

→∞

(T-t)

→∞

and

therefore

!γ

(N(d

)

N(d

))

一

σe-4i

∞

(T-t)

→∞

\2y2

作

)

We conclude

that

, for T - t large enough,

8(C)

Ke-

叫

(γ

(N(d

)

出))一

σJ}

飞

2y2

作

- t) )

will

positive. We note

that

the

positive value of

C) is nonetheless small,

丑

lim(T-t)

→∞

8(C)

口

Note

that

(圣)

(γ

-q-~)(T-t)

JT-t

In(K)

州十

(γ

-q

一手)

- t)

JT-t

σVT

丁

Then

θKσ

KJT-t'

and

,from (3.11)

and

(3.12),

conclude

that

(3.12)

Problem

12:

Show

that

the

price of a plain vanilla European call option is

a convex function of

the

strike of

the

option, i.e.,show

that

θ2C

一一=-=-

一

θ2C

e-r(T-t)

二

Ji>O

口

作

- t)

Solution: Recall

that

Se-q(T-t)

N'(d

) -

Ke-r(T-t)

N'(d

By differentiating

the

Black-Scholes formula

C -

Se-q(T

一忖

T(d

)

Ke-r(T-t)

N(d

)

with

respect

K ,

obtain

that

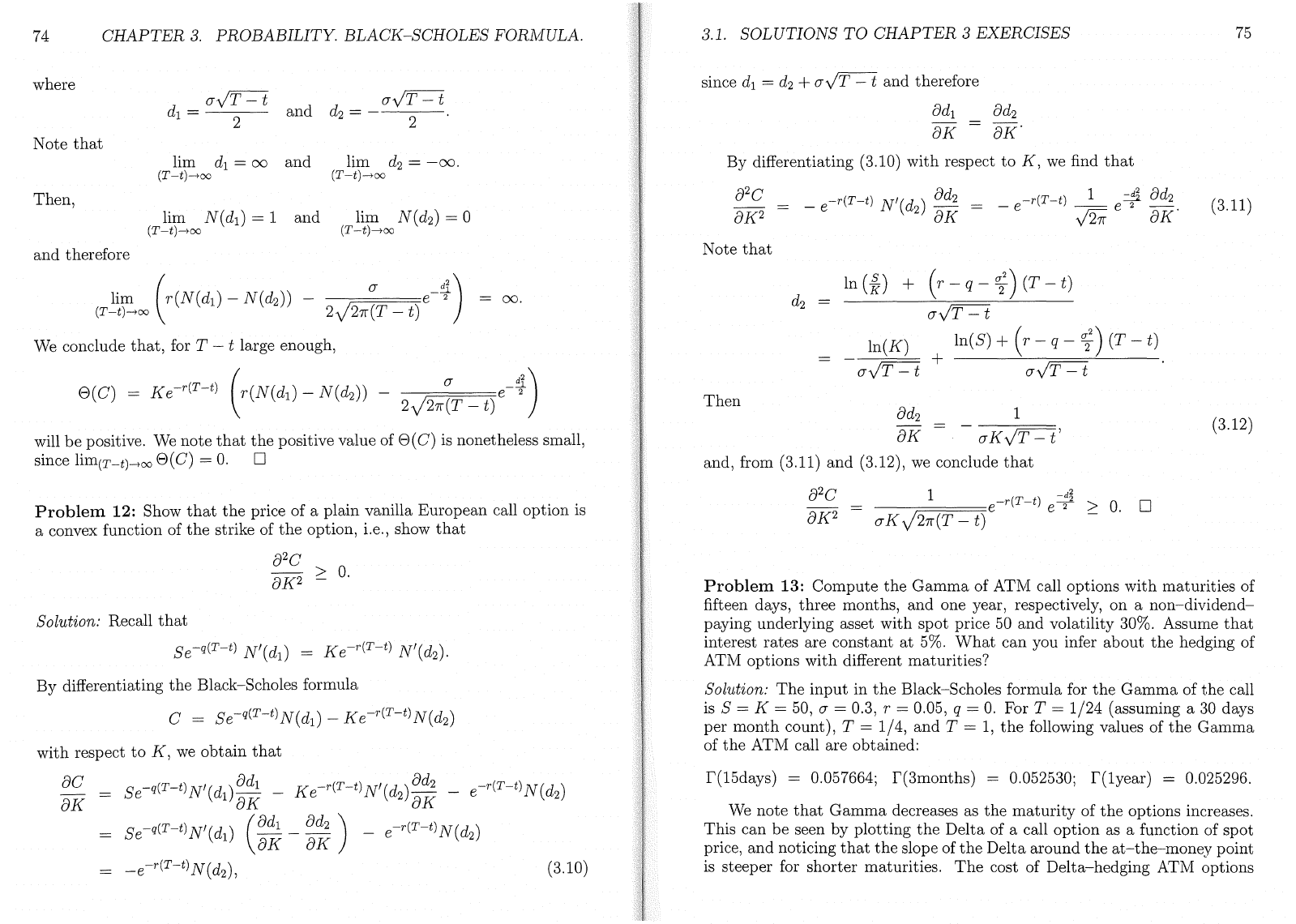
Problem

13:

Compute

the

Gamma

of ATl\!I call options

with

maturities of

fifteen days

, three months, and one year, respectively, on a

non-dividend-

paying underlying asset with spot price

and

volatility 30%. Assume

that

interest rates are constant

5%.

What

can you infer

about

the

hedging of

l\!I options with different maturities?

Solution:

The

input

the

Black-Scholes formula for

the

Gamma

the

call

S = K =

，

σ= 0.3, r = 0.05, q =

For T =

1/24

(assuming a

days

per

month

count), T =

1/4

and

T = 1,

the

following values of

the

Gamma

the

ATM call are obtained:

Se-q(T

一叫鞋一

Ke-r(T

一川也)在一

e-r(T

一切

)

Se-q(T-t)

N'(d

)

(

坐

-252}

e-T(T

一切(出)

飞

θKθ

}

_e-r(T-t)

N(d

) , (3.10)

r(15days)

- 0.057664;

f(3months)

= 0.052530;

r(1year)

= 0.025296.

note

that

Gamma

decreases as

the

maturity

the

options increases.

This can

seen

plotting

the

Delta

of a call option as a function of spot

price

and

noticing

that

the

slope of

the

Delta around

the

at-the-money

point

is steeper for shorter maturities.

The

cost of Delta-hedging ATl\!I options

CHAPTER

PROBABILITY. BLACK-SCHOLES

FOR

IULA.

may

higher for short

dated

options, since small changes

口

the

price of

the

underlying asset lead

higher changes

the

Delta

the

option,

and

therefore

may

require more often hedge

rebalancing.

口

Problem

14:

(i)

The

vega of a plain vanilla

European

call or

put

is positive,

smce

vega(

- vega(P) -

Se-q(T

一明习气

一手

VL/

(3.13)

Can

you give a financial explanation for this?

(ii)

Compute

the

vega of ATM Call options

with

maturities

自

fteen

days,

three

months,

and

one year, respectively, on a non-dividend-paying under-

lying asset with spot price

and

volatility 30%. For simplicity, assume zero

interest

rates

i.e.

，

γ=0.

(iii)

r = q = 0,

the

vega of

ATJVI

call

and

put

options is

吨

a(C)

工吨

a(P)

SVT

习气

LJ7

飞/三'Tr

巾

叫

巳

Cωom

川附

山

仰

叩

丑毗丑

吨叩&叫圳州

(ρ

阴

川

让凶

ime

江

一

，

i.e.,

θ(vega(

C))

θ(T

and

explain

the

results from

part

(ii) of

the

problem.

Solution:

(i)

The

fact

the

vega of a

plai

口

vanilla

European call or

put

positive means

that

,all other things being equal,options on underlying assets

with

higher volatility are more valuable (or more expensive,

dependi

吨

日

whether you have a long or short options position). This could

understood

as follows:

the

higher

the

volatility of

the

underlying asset,

the

higher

the

risk associated with writing options

日

the

asset. Therefore,

the

premium

charged for selling

the

option will

higher.

you have a long position in either

put

or call options you are essentially

"10

日

volatility" .

(ii)

The

即

the

Black-Scholes formula for

the

Gamma

the

call is

S = K =

，

σ=

0.3

，

q =

For T =

1/24

, T =

1/4

and

T = 1,

the

following values of

the

vega of

the

ATJVI

call are obtained:

vega(15days)

vega(3months)

vega(lyear)

4.069779;

9.945546;

19.723967.

3.1.

SOLUTIONS

CHAPTER

EXERCISES

(iii) For clarity, let 7 = T -

For r = q = 0,

obtain

from (3.13)

that

vega(C) =

豆坦豆

VL1

汀

「

since,for

ATJVI

option

with

r = q = 0,

= In

(主)十

(γ

-q

十号

_aft

σJ

于一

By direct computation,

find

that

…

/'1\-

nu-

一旦

〉宇

-z;

工

2)2

'Tr

石

忐

一宁

牛

For

σ=

0.3

and

for time

maturity

less

than

one year, i.e., for

三

，

丑

that

vhu

414

and

therefore

θ(vega(C))

θ;

三

We conclude

that

, for options with moderately large time

maturity

the

vega

mcreasmg as time

maturity

increωes.

Therefore

expect

that

vega(1year) > vega(3months) > vega(15days

which is

what

previously obtained by direct

computation.

口

Problem

15: Assume

that

interest rates are constant and equal

toγ.

Show

tflat7unless tfle price C of a ca11optiOIlwith strike k

amd

mat11rity T

OIl

non-dividend paying asset with spot price S satisfies

the

inequality

Se-

三

SfqT7

(3.14)

arbitrage

opportunities

arise.

Show

that

the

value P

the

corresponding

put

option

must

satisfy

the

following

no-arbitrage

condition:

Se-

(3.15)

CHAPTER

PROBABILITY.

BLACK-SCHOLES

FORMULA.

3.1.

SOLUTIONS

CHAPTER

EXERCISES

V(T)

S(T)

C(T)

S(T)

max(S(T)

- K ,

三

，

Solution: One way

prove these bounds on

the

prices of European options

is by using

the

Put-Call

parity, i.e,

十

Se-

二

Ke-

To establish

the

bounds (3.14) on

the

price of

the

call, no

A more insightful way

prove these bounds is

use arbitrage arguments

and

the

Law of One Price.

Consider a portfolio made of a short position in one call option with strike

and

maturity

and

a long position

units of

the

underlying asset.

The

value of

time 0 of this portfolio is

V(O)

Se-

- C.

the

dividends received on

the

long asset position are invested continuously

buying more units of

the

underlying asset,

the

size of

the

asset position

time

T will

1 unit of

the

asset.

Thus

The

payoff of

the

put

time T

即以

S(T)

which is less

叩

the

strike

The

value P of

the

put

time 0 cannot

than

the

present value

time 0 of K

time

Also,

the

value P of

the

put

option

lllust

greater

than

Thus

三

(3.17)

and

, from (3.16)

and

(3.17),

obtain

that

Se-

Ke-

Se-

Ke-

+ P - C < Se-qT.

To establish

the

bounds (3.15) on

the

price of

the

put

, note

that

Ke-

Se-

十

(3.18)

The

payoff of

the

call

time T is

max(S(T)

- K ,

which is

l~s

than

S(T).

The

value C of

the

call

time 0 cannot

than

Se-

the

present

value

time

0 of one

unit

the

underlying asset

time T ,if

the

dividends

paid

the

asset

rate

q are continuously reinvested in

the

asset. Also,

the

value C of

the

call option must be greater

than

Thus

。三

三

Se-qT.

(3.19)

From (3.18) and (3.19)

follows

that

Ke-

Se-

< P <

Ke-

Problem

16:

A portfolio containing derivative securities on only one asset

has

Delta

5000

and

Gamma

-200.

A call on

the

asset

with

(C)

= 0

and

f(C)

= 0.05,

and

put

the

same asset,

with

(P) -

-0.5

and

f(P)

= 0.07 are currently traded. How do you make

the

portfolio Delt

neutral and

Gamma-neutral?

Solution: Take positions of size

and

, respectively, in

the

call and

put

options

speci

五

above.

The

value II of

the

new portfolio

II = V +

C +

归

，

where V is

the

value of

the

original portfolio. This portfolio will be

Delta-

and

Gamma-neutral

, provided

that

and

are chosen such

that

(II) =

(V) +

6.(

C) +

(P) =

5000

十

0.5X2

f(II)

f(V)

十

xlf(C)

十

X2f(P)

= -

200

十

05X

十

0.07X2

The

solution of this linear system is

250, 000 ._.

330, 000

一一一

ι...::....::..

-4716.98

and x')

一」一-=-=-

= 6226

53 -. - - --

.---.-

To make

the

initial portfolio as close

Delt

and

Gamma-neutral

possible by only

trading

the

given call

and

put

options, 4717 calls must

sold and 6226

put

options must

bought.

The

Delta

and

Gamma

the

new portfolio are

6.(江)

(V) - 4717

6.(

十

6226

(P)

= 0.2;

口)

f(V)+4717f(C)+6226f(P)

一

0.03.

since,if

S(T)

> K ,

then

V(T)

S(T)

一

(S(T)

= K ,

and

,if

S(T)

三

，

then

V(T)

S(T)

三

From

the

Generalized Law of One Price

conclude

that

V(O)

Se-

三

Ke-y-T?

and therefore

Se-

- K

三

，

which is

the

left inequality from (3.14).

All

the

other inequalities can be proved similarly:

• To establish

that

~二

Se-

，

show

that

the

payoff

maturity

T of a

portfolio made of a long position

丑

units of

the

underlying asset

time

and

a short position in

the

call option

nonnegative for any possible values

S(T);

• To establish

that

Se-

~二

，

show

that

the

payoff at

maturity

of a portfolio

made

of a long position in

units of

the

underlying asset

time 0

and

a long position in

the

put

option is greater

than

K for any

possible values of

S(T);

• To establish

that

~二

, show

that

the

payoff

maturity

T of a

portfolio made of a short position in

the

put

option

and

a long cash position

Ke-

time

0 is nonnegative for any possible values of

S(T).

口

(3.16)

C -

Se-

Ke-

十

CHAPTER

PROBABILITY.

BLACK-SCHOLES

FOR

IULA.

3.1.

SOLUTIONS

CHAPTER

EXERCISES

understand

how well balanced

the

hedged portfolio II is, recall

that

the

initial portfolio

had

~(V)

= 5000

and

r(V)

-200.

口

(iii)

The

new spot price and

maturity

the

option are 8

= 98

and

12~

/252

(there are 252

trading

days

one year).

The

value of

the

call option

53134

and

the

value of

the

por

毛

folio

(10000

5898

)

一

(1000C

5898

)

=一

$3.74.

1000C

5898

= - 49268.87.

1000(C

=一

$118

74.

For

the

Delta-hedged portfolio,

the

loss incurred is

(iv)

the

long call position

not

Delta-hedged,

the

loss incl

decrease in

the

spot

price of

the

underlying asset is

As expected

, this loss is much smaller

than

the

loss incurred if

the

options

positions is

not

hedged

("naked").

口

1000~(

= 1000e-

) = 653.50

units of

the

underlying asset, where

(圣)

(1'

吟

二一

-σ

飞

Problem

17:

You are long 1000 call options

with

strike 90

and

three months

maturity. Assume

that

the

underlying asset has a lognormal distribution

with

drift

μ=

0.08

and

volatility

σ=

0.2,

and

that

the

spot price of

the

asset is 92.

The

risk-free

rate

= 0.05. vVhat Delta-hedging position do

you need

take?

Solutio

η:

A long call position is Delta-hedged by a short position

the

underlying asset. Delta-hedging

the

long position

1000 calls is done by

shorting

with

8 = 92, K = 90,T =

1/4

，

σ=

0.2

，

γ=

0.05, q =

Note

that

, for Delta-hedging purposes,

is not necessary

know

the

driftμof

the

underlying asset,since

(C)

does

口

depend on

μ.

口

Problem

18:

You

buy

1000 six months ATM Call options on a

non-

dividend-paying asset

with

spot price 100, following a lognormal process

with

volatility 30%. Assume

the

interest rates are constant

5%.

(i) How much money do you pay for

the

options?

(ii)

What

Delta-hedgi

吨

position

do you have

take?

(iii)

the

next trading day,

the

asset opens

98.

What

the

value of

your position (the option and shares

positio

时?

(iv) Had you

not

Delt

hedged, how

旧

would you have lost due

the

increωe

the

price of

the

asset?

Solution: (i)

Usi

吨

the

Black-Scholes

forml

出

with

input

= K = 100,

T =

1/2

，

σ=

0.3,

= 0.05, q = 0,

we 自

时

the

value of one call option

= 9.634870. Therefore,$9,634.87 must

paid for 1000 calls.

(ii)

The

Delta-hedgi

吨

position

for long 1000 calls is short

1000~(

C) = 1000e-

) = 588.59

units

the

underlying. Therefore, 589 units of

the

underlying must

shorted.

CHAPTER

PROBABILITY.

BLACK-SCHOLES FORNIULA.

3.3.

SOLUTIONS

SUPPLEMENTAL EXERCISES

3.2

Supplemental

Exercises

What

the

expected number of coin tosses of a fair coin in order

get two heads in a row?

What

the

coin is biased

and

the

probability

of getting heads is

What

the

expected number of tosses

order

get k heads in a row

for a biased coin

with

probability of getting heads equal

Calculate

the

mean

and variance of

the

uniform distribution

the

interval

Let X be a normally distributed

random

variable

with

meanμand

standard

deviation

σ>

Compute

IXI

]

and

E[X

Compute

the

expected value

and

variance of

the

Poisson distribution,

i.e., of a

random

variable X taking only positive integer values

with

probabilities

一入飞

P(X=k)

气卡，

三

，

whereλ>

0 is a fixed positive number.

Show

that

the

values of a plain vanilla

put

option

and

of a

plai

且

vanilla

call option

with

the

same

maturity

and

strike,

and

the

same under-

lying asset

, are equal if

and

only if

the

strike is equal

the

forward

pnce.

You hold a portfolio

made

of a long position in 1000

put

options

with

strike price

and

maturity

of six

months

, on a

non-dividend-paying

stock

with

lognormal distribution

with

volatility 30%, a long position

400 shares of

the

same stock,which has

spot

price $20,

and

$10,000

如

cash.

Assume

that

the

risk

自

free

rate

is constant

4%.

(i) How much is

the

portfolio worth?

(ii) How do you adjust

the

stock position

make

the

portfolio

Delta-

neutral?

(iii) A

month

later

the

spot

price of

the

underlying asset is $24.

时

is new value of your portfolio,

and

how do you

adjust

the

stock position

make

the

portfolio

Delta-neutral?

You hold a portfolio

with

6.(口)

= 300,

f(II)

= 100,

and

vega(II) = 89.

You can

trade

the

underlying asset, in a call option

with

(C)

= 0.2;

f(C)

= 0.1; vega(C) = 0.1,

and

in a

put

option

with

(P) =

-0.8;

f(P)

= 0.3;

vega(P)

= 0.2.

What

trades

do you make

obtain

a 6.-, f

and

vega-neutral

port-

folio?

3.3

Solutions

Supplemental

Exercises

Problem

What

the

expected number of coin tosses of a fair coin in

order

get two heads in a row?

What

the

coin is biased

and

the

probability

of getting heads is

Solution:

p is

the

probability of

the

coin toss resulting in heads,

then

the

probability of

the

coin toss resulting in tails is 1 - p.

The

outcomes of

the

first two tosses are as follows:

•

the

first toss is tails, which happens

with

probability 1 - p,

then

the

process resets

and

the

expected number of tosses increases by

•

the

趾

toss is

he 告

，

and

the

second toss is also heads, which hap-

pens

with

probability

气

then

two consecutive heads were obtained after two

tosses.

·If

the

first toss is

heads?and

the

second toss is

tails?which

happens

with

probability

p(l

then

the

process resets

and

the

expected number

of tosses increases by

E[X]

denotes

the

expected number of tosses in order

get two heads

in a row

conclude

that

E[X]

(1-

p)(l

E[X])

十

p(l

p)(2 +

E[X]).

(3.20)

We solve (3.20) for

E[X]

and

obtain

that

牛竹

E[X]

寸主.

For

u~biase_d

coi

r:,

i.e.,for p =

~，

find

that

E[X]

= 6,

and

therefore

the

expected number of coin tosses to-

obtain

two heads

a row is

口

CHAPTER

PROBABILITY.

BLACK-SCHOLES

FOR

IULA.

Problem

What

the

expected number of tosses

order

get n heads

a row for a biased coin

with

probability of

getting

heads equal

Solution:

The

probability

that

the

first n throws are all heads is

pn.

the

且

rst

k throws

are

heads

and

the

(k +

l)-th

throw is tails, which

happen

让

吐

probability

pk(

οl

一

，

then

the

process resets after

the

十

steps; here,

k =

(η-1).

Then

,if

x( η)

denotes

the

expected number of tosses

order

get

ηheads

a row,

follows

that

'hh

-iJSStt-

Recall

that

二

kpk

npn

十

(η

l)pn

十

p)2

l-p7

Then

, we find

that

η)

一

(η

十

l)pn

十

npn+1

，

十

x(n)(l

_ pn)

1-p

-一一十

η)(1

_ pn) ,

1-p

and

therefore

1-p

(η)

=一一一-

(1-

p)'

We conclude

that

the

expected number of tosses in order

get n

heads

a row for a biased coin

with

时

ability

getti

昭

leads

叩

pis

元

the

∞

丑

were

unbia

时，

i.e., for p =

~，

the

expected number of tosses

order

get n heads

a row is

2η+1

口

Problem

3: Calculate

the

mean

and

variance of

the

uniform distribution

the

interval

[价].

Solution:

The

probability density function of

the

uniform distribution U

the

interval

the

∞

stant

functi

∞

j(x)

b~a'

for all x E

b].

Then

• 1 r

E[U]

= I

xj(x)

一~

xdx

一

jαb

一

αjα2

3.3.

SOLUTIONS

SUPPLEMENTAL

EXERCISES

var(U) = E

[eu

E[U])2]

= E [

一引

二

f(z-132)dz=

击

~(X_b;a)'

一

Problem

4: Let X

a normally distributed

random

variable

with

meanμ

and

standard

deviation

σ>

Compute

IXI

]

and

E[X

Solution: We

compute

IXI

]in

terms

the

cumulative

dist

由

ution

N(t)

工

言

巾

the

standard

normal variable Z.

Note

that

μ+σ

Then

E[IX

门

言汇

lμ+

的

一手

τ~

(

一

μ/σ

一

(μ

十的)

一句+气

(μ

一手

马丁

J-x

一

μ/σ

-tl:Vdz

一元

zlJNJdz

+μ

一言

一

r.::=

一

μ/σ-

，.

~在

一

μ/σ

山

easy

see

that

JUJu

ft7

1-du

N(-~)

1-N(~);

(-e

一手)

二

一叫一￡);

在[~"

e-'; dy N

(;)

;

(-e-~)

J:二

/σ=

叫一￡)

CHAPTER

PROBABILITY.

BLACK-SCHOLES

FORJVIULA.

3.3.

SOLUTIONS

SUPPLEMENTAL

EXERCISES

We conclude

that

Then

follows

that

E[\X\]

一

(l-N(;))

十元叫一出

十叫;)

+在叫一￡)

(2N

(;)

-1)

+岳叫一出

二气

k-l

、

)

一一一一一一一

…

二-:-;

eλ

(3.22)

(3.23)

From

(3.21)

and

(3.22)

丑

that

E[X]

λ.

Similarly

One

way

compute

E[X

]

would

compute

the

followi

吨

integral:

E[X

]

E[(

μ+σ

Z)2]

仨/∞

(μ

十

M)2Jdz

V'<""

J-OO

E[X

]

。

~一

飞

汇

P(X

k)·

汇气产

一

已

1)λk

~一

亏

λk

- -

..一

台

(k-1)!

~但

飞

k-2

飞

k-l

d2EtrA

罢工一

λ2

十

λ7

兰川

While

this

would

provide

the

correct

result

easier

way is

recall

that

var(X)

E[X

]

一

(E[X]?

Since

E[X]

μand

var(X)

=σ2

，

concl

时

that

E[X

]

var(X)

(E[X])2

μ2

十

σ2

where

(3.22)

and

(3.23)

were used

for

the

last

equality.

We conclude

that

Problem

Compute

the

expected

value

and

variance

the

Poisson

distri-

bution

i.e.

random

variable

taking

only

positive

integer

values

with

probabilities

var(X)

E[X

]

(E[X])2

口

Problem

6: Show

that

the

values

of a

plain

vanilla

put

option

and

plai

丑

vanilla

call

option

with

the

same

maturity

and

strike

and

the

same

underlying asset

are

equal

and

only

the

strike is equal

the

forward

pnce.

Solution: Recall

that

the

forward

price

is F

一

(r-q)T

From

the

Put-Call

parity

know

that

Be-

Ke-

(3.24)

一入飞

P(X

气!'

三

，

where

λ>

fixed

positive

numbe

Solution:

show

that

E[X]

λand

var(X)

=λ

definition

Recall

that

the

Taylor

expansion

the

function

call

and

put

with

the

same

strike

K have

the

same

value

i.e.

(3.24)

then

Be-

Ke-

Thu

=z;

一

(r-q)T7

i.e.

the

strike of

the

options is

equal

the

forward

price.

口

CHAPTER

PROBABILITY. BLACK-SCHOLES

FOR

IULA.

3.3.

SOLUTIONS

SUPPLEMENTAL

EXERCISES

Problem

7: You hold a portfolio

made

of a long position in 1000

put

options

with

strike price

and

maturity

of six

months

, on a

non-dividend-paying

stock

with

lognormal distribution

with

volatility 30%, a long position

400

shares of

the

same stock, which

has

spot

price $20,

and

$10,000 in cash.

Assume

that

the

risk-free

rate

is constant

4%.

(i) How

时

the

portfolio worth?

(ii) How do you

adjust

the

stockposition

make

the

portfolio

Delta-neutral?

(iii) A

month

later

the

spot price of

the

underlying asset is $24.

What

is new

value ofyour portfolio

and

how do you

adjust

the

stock position

make

the

portfolio

Delta-neutral?

Solution: (i)

The

value of

the

portfolio is

1000P(0) +

4008(0)

十

10000

= 22927,

where

8(0)

二

the

spot

price of

the

underlyi

吨

asset

and

the

value

P(O)

= 4.9273 of

the

put

option is

obtained

using

the

Black-Scholes formula.

(ii)

The

Delta

the

put

option position is

-1000N(

-d

) =

-803.

(Here

and

the

rest of

the

problem,

the

values of

Delta

are rounded

the

nearest

integer.)

The

Delta

the

portfolio is

-803

+400 = - 403.

obtain

Delta-neutral

portfolio,403 shares must

purchased for $8,060.

The

Delta-neutral

portfolio will

made

of a long position in 1000

put

op-

tions a long position

803 shares of

the

underlying stock,

and

,940

cash.

(iii) A

month

later

the

spot

price of

the

und

凹

lying asset is S (

古剖)

= 24

丑

the

put

opt

悦

ions

have

直

飞

months

lef

丘

时

垃削

挝

让

maturity.

The

Black-Scholes value

the

put

option is P

(古)

= 2.1818.

The

cash position has accrued interest

and

its

current value is 1940 exp

(呼)

= 1946.

The

portfolio is

巾

(

1\(

1000P I

~-_

I +

8038

~-_

十

1946

= 23400.

飞

)

飞

}

The

new

Delta

the

portfolio is

-1000N(

-d

)

+ 803 = 292.

To make

the

portfolio

Delta-neutral

, you should sell 292

shares.

口

Problem

8: You hold a portfolio

with

i:::..

(II) = 300,

r(II)

工

100

and

vega(II) =

89.

You

can

trade

the

underlyi

吨

asset

，

a call option

with

i:::..

(C)

工

0.2;

r(C)

= 0.1; vega(C) = 0.1,

and

in a

put

option

with

i:::..

(P) =

-0.8;

r(p)

= 0.3;

vega(P)

= 0.2.

What

trades

do you make

obtain

a i:::..-, r

and

vega-neutral

portfolio?

Soltdi07ZJ

You

can

make

the

portfolio

T-and

Vega-neutral

takiIlg posi-

tions

the

call

and

put

optiOI17respectively.By

trading

iII

the

underlying

asset7the

aad

Vega

of tfle portfolio would

not

change7and

the

portfolio

can

made

i:::..

-neutra

Formally7let z17Z27and

hbe

the

positions

i2the

underlying

asset7the

Cali

option?and

the

put

option7respectively.The

value of

the

zlew

portfolio

旧

w=II

十

+ X2

句

and

therefore

i:::..(丑

nω)

i:::..

(II)

十

i:::..

(C)

十

i:::..

(P);

r(II

nω

)

口)

+ X2

(C)

+ X3

(P);

vega(

口

new)

vega(II)

十

(C)

十

(P).

Then

i:::..(丑

nω)

丑

ηω)

vega(II

叫

0 if

and

only if

(们

0.2X2-

0.8

•

-300;

十

一

100;

十

-89.

The

solution (rounded

the

nearest

integer)is

Zl=-254

向饥=

-670

、

均

=-1101n

othr

words,

make

the

portfolio

A-7T-and

Vega

一丑

eut

叫:

one

must

short

254units

ofthe

underlying asset

and

sell

670call

options

&创

口

110

put

options.

口

Chapter

Lognormal

random

variables.

Risk-neutral

prIcIng.

4.1

Solutions

Chapter

Exercises

Problem

1: Let

= Z and X

-Z

two independent random vari-

ables

, where Z is

the

standard

normal variable. Show

that

+ X

is a

normal variable

mean 0

and

variance 2, i.e.,

十

Solution:

丑

ecall

that

and X

are

independent normal random variables

with mean and variance

μ1

and

σ?7aMμ2

and

，

respectively,

then

+ X

is a normal variable

with

mean

μ1+μ2

and

varia

肌

eσr+σ27and

+ X

f.t

1 +

f.t

2 +

;;;f.丐

For

= Z

and

-Z

follows

that

μ1=

问=

and

σ1=

句=

vVe

conclude

that

E[X]=

μ1

十问=

and

var(X)

=σ?+σ~

= 2,

and

therefore

X =

口

Problem

2: Assume

that

the

normal random variables

, X

, … , X

meanμand

variance

σ2

陀

uncorrelated

，

飞

cov(X

，

Xj)

- 0, for all

三

并

三

η.

(This happens, e.g., if

, X

, … , X

are independent.)

~=1

the

average of

the

variables Xi, i = 1

:风

show

that

E[Sn]

μand

var

问

Solution: Recall

that

,for Ci

ε~

，

斗[~←

呐凡斗]

~吝辛←←

叫

剧刷

町耶啊啊[仄问阳

几均

斗]

CHAPTER

LOGNORMAL VARIABLES.

PRICING.

SOLUTIONS

CHAPTER 4 EXERCISES

Problem

the

price S(t) of

∞

n-dividend

paying asset has

lognor

口时

distribution

with

drift r

and

volatility

风

show

that

刊主

Therefore,

crvar(X

)

+ 2

~二呐∞，

v(X

，

Xj )

川

S(t

c5t)]

[S2(t

十

c5t)]

S(t);

e(2r+

S2(t).

(4.3)

(4.4)

Solution 1:

the

price S

(t)

the

non-dividend

payi

吨

asset

has lognormal

distrib

叫

川

ith

drift

川

叫

创削

址

til

过

lity

川

given by

丑〈俨(了俨

贝(川

J = I

一一

+ σ)

圳

叼

S(t

例

t均)

)\/

Recall

时，

if ln(Y)

=μ

十歹

is a lognormal

random

variable

with

parameters

μand

歹，

the

expected value

and

variance of

Yare

=μ;

一

·ημ

一

·n

σ

血

:土贝

Xi]

主

zmE

间十二三二

-nCOVM)

[~字]

咔字)

主

二

var(Xi)

E[Sn]

var(Sn)

Solution: We can regard

S(t

十们)

as a

random

variable over

the

probability

space{U7D}ofttle

possible moves

ofthe

price

ofthe

asset

fromtimet

time

.L+

~ndo~ed

with-

the

risk-neutral

probability function P :

,D} •

with

P(U) = p

and

P(D) = 1 -

Then

S(t +

8t)

is given by

Problem

3: Assume

have a one period binomial model for

the

evolution

the

price of

underlying asset between

time

and

time

十

8t:

S(t) is

the

price of

the

asset

time

t~en

the

p:~ce

S.(t

十

8t)

the

asset

time

十

will

either

S(t)

叽

with

(risk-ne

飞巾

al)

probability p,or S(t)d,

with

probability 1 -

Assume

that

u > 1

and

d <

Show

that

since

COV(Xi

)

= 0 for all

三

泸

三

η.

(pu

- p)d) S(t);

(pu

十

)

(t).

(4.5)

(

4.7)

(4.8)

(

4.9)

=eTSt;

」一

·E[S(

仆

8t)]

S(t)

-L-Var

(S(t+60)-

S2(t)

叫

μ+

三);

优

(2μ

)

(i:

- 1)

叫

((γ_

~2)

十叩

仪

(γ_

~2)

们+州

)2)

(e(

而

)2-

1J4

)

(产

-1)

E[Y]

var(Y)

E[tft)]

mr(tf))

E[tft)j

vaT

(吐了))

盯=需在

叫

=(γ

一手

)

and

歹工

v5i

and

切向

Note

that

(4.1)

(4.2)

S(t)d.

口

S(t

十们

)(D)

= S(t)u;

ERN[S2(t

十

缸创

均)刀

S(t

十们

)(U)

Then

,by definition,

From

(4.5)

and

(4.8)

and

from

(4.7)

and

(4.9)

,respectively,

conclude

that

N[S2(t

8t)]

P(U) .

S(t

十们

)(U)

P(D)·

S(t

十

8t)(D)

(pu

- p)d) S(t);

P(U) .

(S(t

十们

)(U)?

十

P(D)

(S(t

十们

)(D))2

(pu

十

)

(t)

.口

E[S(t

十

8t)]

var (S(t + 8t))

t);

叮产-

S2(t)

(4.10)