Stefanica D. Solutions Manual: A Primer For The Mathematics Of Financial Engineering

94

CHAPTER

4.

LOGNOR

1V

IAL

VARIABLES.

RN

PRICING.

Note

that

var

(S(t

十们))

= E[S2(t +

8t)]

一

(E[S(t

十们

)])2

=

E[S2(t

十

6t)]

-

e2r6ts2(t).

From (4.10) and (4.11)

it

follows

that

E[S2(t

十

6t)]

= var

(S(t

十

8t))

+e

2r

6t

S2(

t)

(4.11)

e

2r6t

十

σ

26t

S2(t) ,

which is

what

we

wanted

to

show.

Solution 2: Note

that

S(t

十归)

can

be

written

as a function of

the

standard

normal variable Z as follows:

I

σ2

飞

r\

S(t

十们)

=

S(t)

仰~

~

r

一言)

6t

十

σ

训

tZ)

Then

,

刁卖

i

七言

fι:S

附

(tω

川

t

均)

m

左

ι

叫

6

们

ιt

←一宁

+o-V5t

♂卜斗一

ζ

x;)

ν

dx

μ1

r

∞/

(x

一

σ

Vti?

飞

J

S(t)

矿

Ot

苟言儿仪

p

\一

-2

-')α

仰附)汩

)e

r

旷沪

T

S(t

阶

t

均

)e

旷

T5"t?

where we used

the

substitution y =

x

一

σ

vti

and

the

fact

that

E[S(t +

6t)]

viz

汇巾

ν-

1,

since

÷eJ

兰

is

the

density function of

the

standard

normal variable.

SimiLr1377mobtaizltbM

E[S2

忻州]=去

(00

S2(

均叫

2

(γ_

~2)

6t +

2σ

vti

x)

e-~

dx

\I

L,7r

J

一∞\\

I

/

1

f

∞

I

n

r

τ

-

x-

\

嘈

去

=

I S2(t) exp t2r6t -

o-:.:I

6t +

2σ

yot

x -

2)

dx

\I

L,7r

J

一∞\/

4.1.

SOLUTIONS

TO

CHAPTER

4

EXERCISES

95

fχ(.L£

J-

I

_2£

J-

(X-2

o-V5t

)2\

S2(t)

可言=

/ exp

12γ

们十

σ

2

6

t

- n i

V

L-7r

J-x

飞.<.

I

fχ

(

(x

-

2σ

V5t

)2

飞

S2(t)e(2r+

e>

2)

6t

~

I exp

{一门)

V

L,7r

J

-x

\

.<.

I

S2

(t)e

(2r+

e>

2)

6t

↓/沉

e-4ds

V

L,7r

J-x

S2(t)e(2r+

σ2)6t;

the

substituti

∞

S

可

-2σ

v5t

was used

above

口

Problem

5:

The

results of

the

previous two exercises can be used

to

calibrate

a binomial tree model

to

a lognormally distributed process. This means

在

nding

the

up

and

down factors u

and

d,

and

the

risk-neutral probability p

(of

goi

鸣

up)

such

that

the

values of

ERN[S(t

十

6t)]

and

ERN[S2(t+6t)] given

by

(4.1) and (4.2) coincide with

the

values (4.3)

and

(4

.4)

for

the

lognormal

mode

l.

In

other

words,

we

are looking for u,d,

and

p such

that

pu

十

(1

- p)d = e

r

6t

;

pu2

+

(1

_ p)d

2

=

e(2r

十

σ

2)

6t

(4.12)

(4.13)

Since there are two constraints

and

three unknowns,

the

solution will not

be

umque.

(i) Show

that

(4.12-4.13) are

equivale

时

to

p

(e

r

6t

-

d)

(u

- e

r

6t

)

aTb

d-r

Ml-ub

e-e

(4.14)

(4.15)

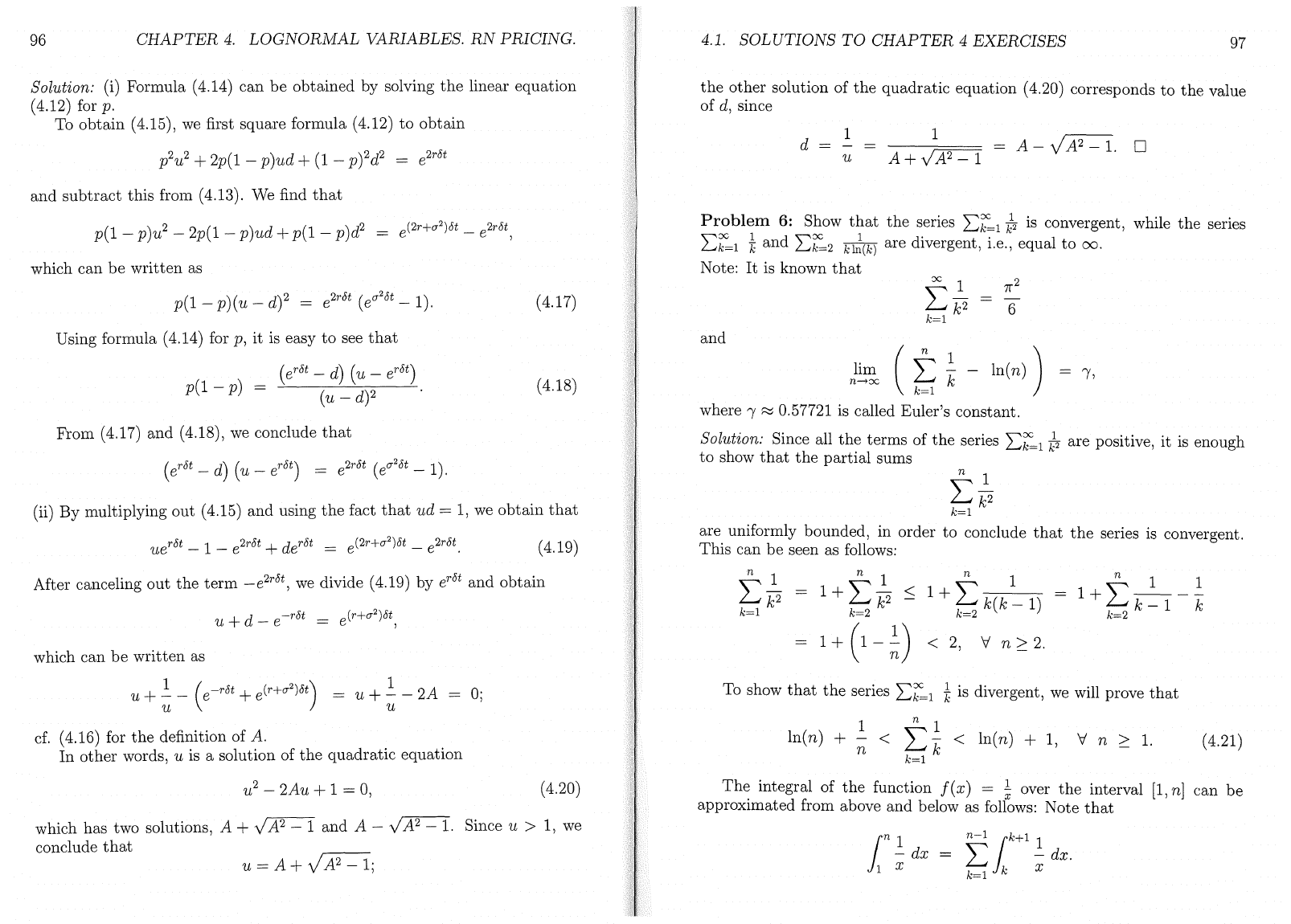
(ii) Derive

the

Cox

一

RoωS

臼卧

Sεs-

一

-Rubi

阳

ns

时

teir

丑

1

paramet

忧

ri

坦

za

创

tion

for a binomial tree, by

solving

(4.14-4.15) with

the

additional condition

that

ud

-

1.

Show

that

the

solution can

be

written

as

p

=

主二

f;

也

=

A+

YA2才

;

d=A-VA

可

7

u

一

α

where

A =

~

(川十

e(r+

e>

2)

6t)

. (4.16)

96

CHAPTER

4.

LOGNOR

Iv

IAL

VARIABLES.

RN

PRICING.

4.1.

SOLUTIONS

TO

CHAPTER

4

EXERCISES

97

Solution: (i) Formula (4.14) can

be

obtained by solving

the

linear equation

(4.12) for

p.

To

obtain

(4.15),

we 在

rst

square formula (4.12)

to

obtain

p2

U

2+2p(1 - p)ud +

(1-

p)2d

2

- e

2r8t

and

subtract

this from (4.13).

We

缸

d

that

the

other solution of

the

quadratic equation (4.20) corresponds

to

the

value

of

d,since

d = 1

L

二

=A

一州

-1

口

u

A

十飞!

A2

_ 1

v

哥…

-

p(l

- p)u

2

- 2p(1 -

p)ud

十

p(l

- p)d

2

已

(2r

十

σ

2)8t

_ e

2r

8t

Prabl

巳

ill

6: Show

that

the

series

1

二;二

l

击

is

conve

带风

while

the

series

2:%二

1i

and

E

二;二

2

世而

are

diverge

风

i.e.

，

equal

to

∞

Note:

It

is known

that

which can

be

written

as

p(l

- p)(u -

d)2

=

e

2r

们

(e

o-

2

8t

_

1).

Using formula (4.14) for p,

it

is easy

to

see

that

(

e

r

8t

一份

(u-e

r

8t

)

p(l

-

p)

=

(u -

d)2

From (4.17)

and

(4.18),

we

conclude

that

(e

r

8t

-

d)

(u -

e

r

们

)

= e

2r

8t

(e

o-

2

8t

-

1)

(4.17)

汇丰

2

1r

6

and

(4.18)

战(兰

;-h(η))

=

~

where

γ

自

0.57721

is called Euler's constant.

Solution: Since all

the

terms

of

the

series

2:%二

l

击

are

positive,

it

is

eno

吨

h

to

show

that

the

partial

sums

(ii)

By

mt

山

iplyi

吨

out

(4.15)

and

usi

吨

the

fact

that

ud

= 1,

we

obtain

that

ue

r

8t

- 1 _

e

2r

8t

十

de

r

8t

=

e(2r

十

σ

2)

8t

_

e

2

叫

(

4.19)

After canceling

out

the

term

_e

2r8t

,

we

divide (4.19) by

e

耐

and

obtain

~

1

·一

仨

k

2

are uniformly bounded, in order

to

conclude

that

the

series

is

convergent.

This can

be

seen as follows:

U

十

d

-

e-

r

8t

-

e(r

十泸州

7

汇丰

L-41

++

1+

各

(kll)

工

1

十二击

-i

which can

be

written

as

U

十

1

一

(

e-

r

8t

+

e(r

十

σ2)

叫

z

U

+1-2A=0;

u \ / U

< 2, V n

~三 2.

d.

(4.16) for

the

de

自

nition

of A.

In

other words,u is a solution of

the

quadratic equation

u

2

-

2Au

十

1

= 0,

To show

that

the

series

1

二;二

1

t is

diverge

风

we

will prove

that

4η4

i

丑

(η)

十二<

)~二<

In

(n) + 1, V

n

三

1

乞

fk

(4.21)

(4.20)

The

integral of

the

function

f(x)

-

~

over

the

interval

[1

,

n]

can

be

approximated from above and below as follows: Note

that

fjdzzzf+1:dz

which has two solutions, A +

VA

亡

landA-VA

亡

1.

Since u > 1, we

conclude

that

u=A

十

J

万丁

I;

98

CHAPTER

4.

LOGNORNIAL

VARIABLES.

RN

PRICING.

Since j (x) =

~

is

a decreasing function,

it

is easy

to

see

that

-L<f(z)<17V

Z

巳

(k

，

k

十

1).

k +1 -

OJ

\--

/ - k

Then

,

Z

1-z

flJI

ZfV

>

ZfzLzdz

z

击=

-1

十

zi

Zfl:dz

<

Zfidz

zi=

一;十主;

The

inequality (4.21) follows from (4.22) and (4.23), since

f:dz=1

日

(η

1-z

n

p''''''1i

(4.22)

(4.23)

In

a similar fashion,by considering

the

integral of x

l~(

百

over

the

interval

[2

,

n]

,

we

can show

that

川)

-ln(

叩门十

L

<土 -L

vn>2;

(424)

内

(η)

仨

k

ln(k)'

立

-L<ln(ln(η))

-

In(ln(2))

十

1

…(

4.25)

kd

klB(k)21

口

(2)'

and

co

肌

lude

that

the

series

'2二;二

2

时而

is

divergent

For example

, (4.24) can be proved as follows:

川

1

~

r

k

+

1

1η~

r

k

+

1

主~

1

I-~-_-_dx-)'I

-

一二

~dx<

亨

l

一一一一

7"

dx

=

予一一-一

儿

xln(x)ω

中台儿

xln

仙

台儿

k

ln(k)

山仨

k

ln(k)'

which is equivalent

to

I".'

二二

'一…一‘'

儿土豆(幻川

I

nln(n)\

仨

kln(k)'

(4.26)

4.1.

SOLUTIONS

TO

CHAPTER

4

EXERCISES

99

Since

/-l-dz=ln(ln(η))

- In

(l

n(2)),

J2

x ln(x)

we

conell

陇

from

(4.26) and (4.27)

that

(4.27)

坦

(lB(n))-h(ln(2))+

」一<安

1

nln(η)

仨

kln(k)'

which

is

the

same as

(4.24).

口

Problem

7:

Find

the

radius of convergence R of

the

power series

三气

x

k

.二

位

k

ln(k)'

and investigate

what

happens

at

the

points x where

Ixl

= R.

Solution:

It

is

easy

to

see

that

2

二 αJ?

2

二

x

k

with

句:时可

，

k

三

2.

Note

that

lim

Iα

川

=lim(

」

-YK=1(429)

k

→∞

k

→∞\

k

ln(k))

(4.28)

Recall

that

,if

li

问→∞|时

11k

exists,

the

radius of

co

盯

erge

肌

e

of

the

series

E

二;二

2α

kXk

is

given by

R

一一」

一

-

li

叫→∞

|α k

1

1/k

'

From (4.29) and (4.30)

the

radius of

co

盯

ergence

of

the

series (4.28)

is

R = 1

We

co

肌

lude

that

the

series is

co

盯

ergent

if

Ixl

< 1, and not

co

盯

ergent

if

Ixl

>

1.

If

x=

工

-1.

the

series becomes

~∞

ζ

且

Since

the

terms

ζ

且

have

S

~k=l

kln(k)'

i:)!ll

ce

'L

ne

'L

erms kln(k)

alternating signs and decrease in absolute value

to

0,

the

series

is

co

丑

vergent.

If

x = 1,

the

series becomes

'2二;二

1

kl~(k)'

which was

show

口

to

be

divergent

in Problem 6 of this

chapter.

口

(4.30)

Problem

8: Consider a

put

option with strike

55

and

maturity

4 months

on a non-dividend paying asset with spot price

60

which follows a lognormal

100

CHAPTER

4.

LOGNORMAL

VARIABLES.

RN

PRICING.

4.1.

SOLUTIONS

TO

CHAPTER

4

EXERCISES

101

model

with

drift

μ=

0.1 and volatility

σ=

0.3. Assume

that

the

risk-free

rate

is constant equal

to

0.05.

(i)

Find

the

probability

that

the

put

will expire

i

口

the

money

(ii) Find

the

risk

一时的

ral

probability

that

the

put

will expire in

the

money.

(iii) Compute

N(

-d

2

).

Solution: (i)

The

probability

that

the

put

option will expire in

the

money

is equal

to

the

probability

that

the

spot price

at

maturity

is

lower

than

the

strike price,i.e.,

to

P(S(T)

< K). Recall

that

The

凡

d

2

= 0.511983, and

N(

-d

2

) = 0.304331,

which

is

the

same as

the

risk-neutral probability

that

the

put

option will

expire in

the

money; c

f.

(4.31)

To

understand this result, note

that

z

d

T

\Ill-/

q

/JIll\

\111/

/111\

n

PRN(S(T)

<

K)

= P

(z

<

一叫到

+(γ-q-4)

气

1σ

VT

J

d.

(4.31) and

(4.32).

口

=

N(-d

2

);

Then

,

P(5(T)

<

K)

P(;2<

忐)

= P

(叫

:2)<

叫忐))

P(

(μ-q-DT+dZ<

叫录))

)

Problem

9: (i) Consider

an

at

拍

e-money

call on a non-dividend paying

asset; assume

the

Black-Scholes framewor

k.

Show

that

the

Deltaof

the

option

is

always greater

than

0.5.

(ii)

If

the

underlyi

吨

asset

pays dividends

at

the

continuous rate q,when

is

the

Delta of an at-the-money call1ess

than

0.5?

Note: For most cases,

the

Delta of

an

at-the-money call option

is

close

to

0.5.

Sol

毗

on:

(i) Recall

that

the

Delta of a call option is given by

-qT

刷=严

N(

咄

+(γ

-q+

号

)T

}

1σ

〉于

1

For 5 = 60, K = 55, T =

1/3

，

μ=

0.1, q =

0

，

σ=

0.3, and

7'

= 0.05,

we

obtain

that

the

probability

that

the

put

will expire in

the

money

is

0.271525,

i.e., 27.1525%.

(ii)

The

risk-neutral probability

that

the

put

option will expire in

the

money

is obtained

just

like

the

probability

that

the

put

expires

i

丑

the

money, by

substituting

the

risk-free rate

7'

for

μ

，

i.e.,

For an

at-th

e-

money call

on

a non-dividend paying asset, i.e., for K = S

and q = 0,

we

find

that

=N

(

(r

十

j)

♂)三

P

RN

(5(T)

<

K)

P

(z

叫蒜)

-

(γ

-q

一手)寸

1σVT

J

(ii)

If

the

underlyi

吨

asset

pays dividends

at

the

c

∞

or

巾

of

ar

口

1

ATM call

i

恒

S

(4.31)

TN(d

1

)

=

e

刊

γ

-q+

叮

(iii) Recall

that

d

2

叫到十

(7'

-

q

一主

)T

σ

叮

(4.32)

For a fixed risk-free

rateγand

自

xed

maturity

T,we conclude

that

~(C)

< 0.5

if and only if

the

dividend yield q

and

the

volatility

σof

the

underlying asset

102

CHAPTER

4.

LOGNORMAL

VARIABLES.

RN

PRICING.

4.1.

SOLUTIONS

TO

CHAPTER

4

EXERCISES

103

When pricing a plain vanilla call using ris

k-

neutrality,

we

proved

that

n-rT

r

∞

II

_2

\9\

CBS(O)

=

8(0)

二言

=

I exp (

(γ

一二)

T+

σdz-4:}dz

V

二7i

J

-d

2

\\

L,

)

~

)

一

Ke

盯旷

e-

r

一→

T

古

/ιL

仆;〉〉

ef

一勾手幻

d

S

贝

(0

创

)N(

何

d

出均

1)

一

Ke-

…

T

叮

TN(

μd

也

ω2

公).

In

other

words

,

we

showed

that

产去/∞

Adz=e

一叩

(d

2

);

V

L,7i

J

-d

2

(4.34)

satis

句

T

the

following

condition:

This happens

,

for example

,

if

r

=

q

and T is

large enough

,

si

丑

ce

lim N

(

(jV!

1

-

1 and lim

0.5

严=∞口

T

•

00

\

~

J

T

•

00

z

d

T

qr-2

/ft--\

/III-\

DA

x

e

nu

cu

--

T

5

二汇叫

(r-

~2)

T

十

σdz-2)dz=

川

d

1

)

(4.35)

From

(4.33)

,

(4.34)

,

and

(4.35)

,

we

conclude

that

V(O)

=

K

2

e-

rT

N(d

2

)

-

2K8(0)N(d

1

)

同一

rT

r

∞

/

?\

+8

2

(0)

~

J-d2

叫

(2

…协

2σ

VTx

-

~-

)

dx.

(4.36)

The

integral

from

(4.36)

is computed

by

co

皿

pleti

吨

the

square

as

follows:

~-rT

r

∞/哎\

的。)

e

/c\

/叫(

(2γ

-

(j

2)T

+

2σdz-Z}dz

V

L,7i

J

-d

2

\

L,

)

-rT

r

∞

(

(x

-

2σ

VT)2

、

的

O)e~

/

侃

p

(一

η

十

(2γ+σ

2)T

1

dx

v

L,7f

J

一出

飞

L,

I

的

0)

川

)T

牛/∞叫{一

(x

一

2

(j

VT?

}

V

L,7i

J

-d

2

飞

L，

I

8

2

(0)

户

2)T

↓/∞

叫(一白

dy

飞!"l，7i

J

一

(d

2

+2

σ

-IT)

\

L，

J

S

俨的州

2

气泪栩(仰例

0

创印)诈

e

户(价

T

we

used

the

substitution

y

=

x

-

2σ

-IT

and

the

fact

that

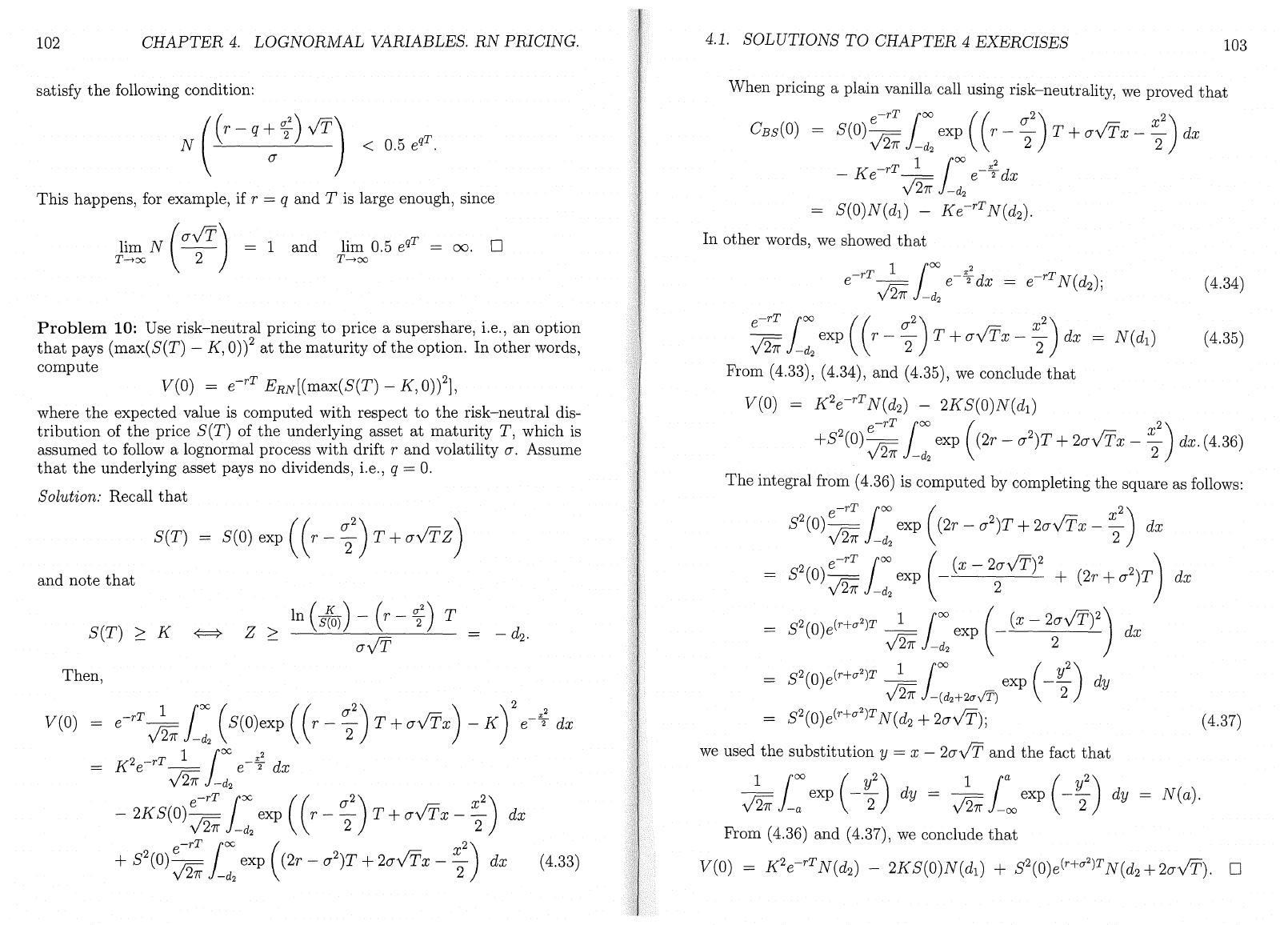
Problem

10: Use

risk-neutral pricing

to

price a supershare

,

i.e.

,

an option

that

pays

(max(8(T)

-

K

,

0))2

at

the

maturity

of

the

option.

In

other words

,

compute

V(O)

=

e-

rT

E

RN

[(max(8(T)

-

K

,

0))2

],

where

the

expected

value

is

computed with respect

to

the

risk-neutral

dis-

tribution

of

the

price

8(T)

of

the

underlyi

吨

asset

at

maturity

T

,

which

is

assumed

to

follow a

lognormal process

with

drift

r

and

volatility

σ.

Assume

that

the

underlying asset pays no dividends

,

i.e.

,

q

=

O.

Solution:

Recall

that

and note

that

8(T)

三

K

白

z

>

叫茄)一

(γ

一手

)

T

z

一也

一们

IT

Then

,

m

工

ff

「

7T

汀咽

'1

K

2

的阶

2

与乍

e-

一

TTV

左

i

七言

l

兀;

巾

Z

一叫

+刊

d

咐呻

S

俨的内

2

气协(仰

0

恬

lι:

〉仪叫叫叫

P(

均巾巾((←价

(ρ

伪

2

衍

T

…一→

σ

巾

2

巧)泞

T+2

川

Z

卜一

ζ

x;)

ν

dx

(4.33)

V

布

i

七言

l

仆:〉

e

叫

X

Fr

￥

'rorαm

(4.36) and

(4.37)

,

we

conclude

that

V(O

恒的

-rT

N(

d

2

)

-

2K

8(0)N(

d

1

)

+

8

2

(0)

户

)TN(d

2

十

2σ

叮

)

.口

104

CHAPTER

4.

LOGNORMAL

VARIABLES.

RN

PRICING.

4.1.

SOLUTIONS

TO

CHAPTER

4

EXERCISES

105

Problem

11:

If

the

price of

an

部

set

follows a normal process, i.e.,

d8

=

μ

dt+

σ

dX

，

then

8(t2) =

8(tl)

十

μ

(t2

- t

1

)

+

σd

产石

Z

，

V 0 <

tl

<

t2·

Assume

that

the

risk free

rate

is constant

and

equal

to

r.

(i) Use risk neutrality

to

且

nd

the

value of a call option with strike K

and

maturity

T.

(ii) Use

the

Put-Call

parity

to

find

the

y~~ue

of a

put

~ption

with

s~rike

K

~n'

d

maturity

T ,if

the

underlying asset follows a normal process as above.

Sol

仰

on:

(i) Using risk-neutral

prici

吗，

it

follows

that

C(O) =

e-

rT

ER

川

max(8(T)

- K ,

0)]

,

where

the

expected value is computed

with

respect

to

8(T)

given by

8(T)

= 8(0)

+γT

十

σ

VTZ.

(ii) Regardless of

the

model used for

describi

吨

the

evolution of

the

price of

the

underlying asset,

the

Put-Call

parity

says

that

a portfolio made of a long

position in a plain vanilla European call option

and

a short position in a plain

vanilla European

put

option on

the

same asset and

with

the

same strike

and

maturity

as

the

call option has

the

same payoff

at

maturity

as a long position

in a unit of

the

underlying asset

and

a short cash position equal

to

the

strike

of

the

options. Using

risk-neutral

pricing, this can

be

written

as

(4.38)

0(0)

-

P(O)

=

e-

rT

E

RN

[8(T) - K] =

e-

rT

(8(0)

+

γ

T-K)

，

(4

.4

0)

since

ER

From

(但

4.3ω9

创)

and

(任

4

.4钊

0

创)，

we

obtain

that

P(O)

=

0(0)

一

(8(0)

十

γ

T)e-

rT

十

Ke-

rT

K e

-rT

(1

- N (

-d))

一

(8(0)

十

rT)

ε

-rT(l

-

N(

-d))

已

-rT

σ

vT

d

2

+卢

J

e

一

τ

飞

/2

汀

K - 8(0) -

rT

-

;[

8(T)

> K iff Z

>

一

-

d

~-rT

_.

/币刀

Ke-rTN(d)

一

(8(0) +

rT)e-

rT

N(d)

十

b

二

V

.L

e

一专

since 1 -

N(

-d)

=

N(d).

口

Note

that

Then

,

0(0)

严

V

在

i

七言

[(卡归仰贝仰(仰仲

O

(问贝喇

O

的如)忖十忖州叫

rT

们巾町

Tη

叩旷)诈

γγ

巳

e-

r

严一

-r

叮

T

「

÷L

l

∞

汁

dx

-

Ke-

rT

去

f

e-hz

〉三1r

Jd

y

'21r

Jd

+

Note

tl

挝

lat

zc

J!!

叫

tm

一切

=

it(-e

一句

I:

1-vi

言

f:

e

九

=

1

-

N

(d)

=

N

(

-d)

2

d

e

2;

where

N(t)

is

the

cumulative

distribution

of

the

standard

normal

variable.

We

conclude

that

m

e-

rT

厅、

IT

0(0)

工

(8(0)

+

rT)e-

rT

N(-d)

-

K

e-

rT

N(-d)

+

v

~.L

e

一

τ.

(4.39)

106

CHAPTER

4.

LOGNORMAL

VARIABLES.

RN

PRICING.

4.3.

SOLUTIONS

TO

SUPPLENIENTAL

EXERCISES

107

1.

Show

that

the

sequence

X

n

=

(言。-

I

日

(η)

is

convergent

to

a limit between 0

and

1.

Note:

The

limit of this sequence is

γ

臼

0.57721

，

the

Euler's constant.

4.3

Solutions

to

Supplemental

Exercises

Problem

1: Show

that

the

sequence

4.2

Supplemental

Exercises

2.

Assume

that

an asset with spot price

50

paying dividends continuously

at

rate

q = 0.02 has lognormal distribution with mean

μ=

0.08 and

volatility

σ=

0.3. Assume

that

the

risk-free rates are constant and

equal

to

r = 0.05.

(i)

Find

95%

and

99%

confidence intervals for

the

spot price of

the

asset

in

15

days, 1 month, 2 months, 6 months, and 1 year.

(ii)

Find

95% and

99%

risk-neutral

con

在

dence

intervals for

the

spot

price of

the

asset in

15

days, 1 month,2 months, 6 months,

and

1 year,

i.

e.

, assuming

that

the

drift of

the

asset is equal

to

the

risk-free rate.

X

n

=

(剖-

ln(η)

is

convergent

to

a limit between 0 and

1.

Solution: Recall from (4.21)

that

ln(η)+j<zi<ln(η)

+ 1,

\;/

η

三

1

，

which can be written as

42···b

>

n

vv

<

n

z

<

It

is

easy

to

see

that

3.

If

you play (American)

1

roulette 100 times

, betting $100

on

black each time,

what

is the prob-

ability of winning

at

least $1000,

and

what

is

the

probability of losing

at

least $10007

X

n

+1

一句

:-L-ln(η+

1)

+

ln(η)

.

η

十

l

Therefore,

X

n

十

1

< X

n

if and only if

5.

Find a binomial tree

parame~rization

for a risk-neutral probability (of

going up or

dow

时

equal

to

~.

In

other words, find

the

up

and

down

factors

u and d such

that

tI<lnh+1)

一

ln(η)=

叫丁)

(n+

1\

T

且

is is

叫

uivalent

to

1 <

(η

十

1)

I

口(平)，

and therefore

to

/η+1\η+1

(~1

\η+1

e <

I

一一

-I

=

11

十一

i

飞

η/

飞

η/

which holds for

anyη;

三

1

，

from

the

definition of

e.

We showed

that

the

sequence

(x

讪

=1:

∞

is

decreasi

吨

and

bounded from

below by 0 and from above by 1

,

The

seque

口

ce

is therefore convergent

to

a

limit between 0 and

1.

口

4.

Use risk-neutral pricing to find

the

value of

an

option on a

口。且一

dividend-paying asset with lognormal distribution if

the

payoff of

the

option

at

maturity

is

equal

to

max((S(T))

α-

K ,

O).

Here

，

α>

0

is

a

fixed constant.

pu +

(l-p)d

pu2

十

(1

-

p)d

2

'?L

)

T(

Problem

2: Assume

that

an asset with spot price

50

paying dividends

continuously

at

rate

q = 0.02 has lognormal distribution with mean μ= 0.08

and volatility

σ=

0.3. Assume

that

the

risk-free rates are constant and equal

to

r = 0.05.

(i) Find

95%

and

99%

confidence intervals for

the

spot price of

the

asset in

15

days, 1 month, 2 months, 6 months, and 1 yea

r.

ifp=i

1American roulette has

18

red slots:

18

black slots: and two green slots (correspond-

ing

to

0 and 00). European roulette: also called

Fr

ench roulette: has only one green slot

corresponding to

O.

108

CHAPTER

4.

LOGNORMAL

VARIABLES.

RN

PRICING.

4.3.

SOLUTIONS

TO

SUPPLEMENTAL

EXERCISES

109

(ii)

Find

95% and

99%

risk-neutral

con

且

dence

intervals for

the

spot price of

theωset

in

15

days, 1 month,2 months,6 months,and 1 year,i.e.,assuming

that

the

drift of

the

asset

is

equal

to

the

risk-free rate.

Solution:

If

the

asset has lognormal distribution,

then

S(t)

=

S(O)

叫(

(μ-q-f)t

十

σ

0z

)

(4

.4

1)

飞飞

2 } }

=

50

exp(O.Ol

日十

0.30z).

Recall

that

the

95%

and

99%

con

直

dence

intervals for

the

standard

normal

distribution

Z are [-1.95996

,1.

95996]

and [-2.57583,2.57583

],

i.e.,

P(

-1.95996 < Z <

1.

95996) = 0.95;

P(

-2.57583

< Z < 2.57583) = 0.99.

Therefore

,

the

95%

and

99%

con

直

dence

intervals for S(t) are

[50

exp(O.Ol

日一

0.30

.

1.

95996),

50

exp(O.Ol

日十

0.30·

1.

95996)];

[50

exp(O.Ol

日-

0.30

·2.57583),

50

exp(0.015t +

0.30

·2.57583)

],

respectively.

The

risk-neutr

，

α

l

confidence intervals for

the

spot price of

the

asset are

found by substituting

the

risk-free

rate

r for

μin

(4

.4

1)

to

obtain

SRN(t)

=

50

exp(

-0.015t

+

0.30Z).

Therefor

飞

the

95%

and

99%

confidence intervals of SRN(t) are

[50

exp(-O.Ol

日一

0.30

.

1.

95996),

50

exp(-O.Ol

日十

0.30

.

1.

95996)]

[50

exp(

-0.01

日-

0.30

·2.57583),

50

exp(

-0.01

日十

0.30

.

2.57583

月

7

respectively.

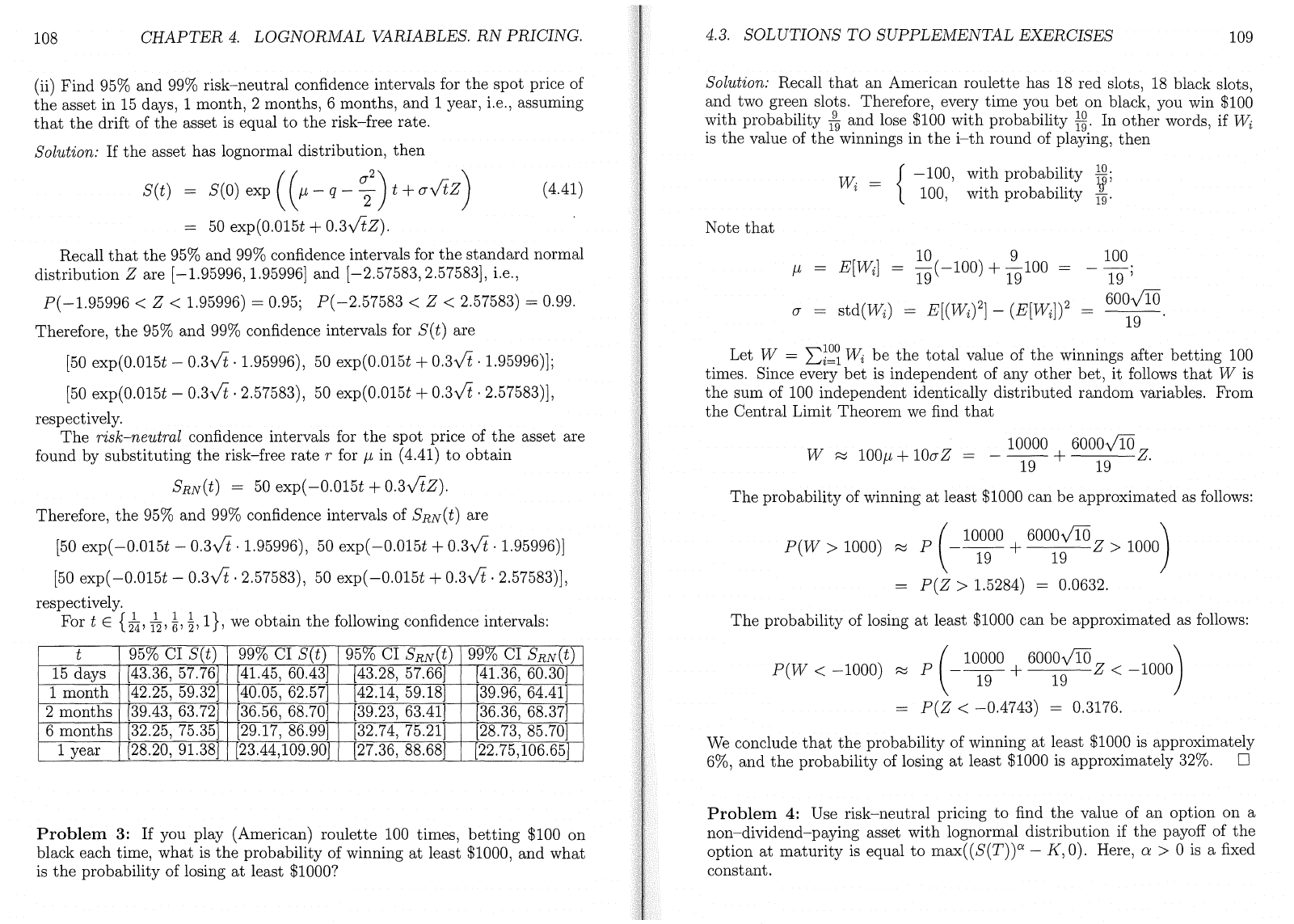
For

t

ε{

主

ι

i

，~，

1},

we

obtain

the

following confidence intervals:

t

95%

CI

S(t)

99%

CI

S(t)

95% CI SRN(t)

99%

CI SRN(t)

15

days 43.36, 57.76 4

1.

45

,

60

.4

3 43.28, 57.66

4

1.

36

, 60.30

1 month 42.25

, 59.32 40.05, 62.57 42.14, 59.18

39.96

,

64

.4

1

2 months

39

.4

3, 63.72 36.56, 68.70 39.23,

63

.4

1

36.36

, 68.37

6 months

32.25

, 75.35

29.17

, 86.99 32.74, 75.21

28.73

, 85.70

1 year

28.20

, 9

1.

38

23

.4

4,109.90 [27.36, 88.68

22.75

,106.65

Problem

3:

If

you play (American) roulette

100

times,

betting

$100

0

丑

black each time, what is

the

probability of winning

at

least $1000, and what

is

the

probability of losing

at

least $10007

Solution: Recall

that

an American roulette has

18

red slots,

18

black slots,

and two green

slo~s.

Therefore, every time you

bet

on black, you win $100

with

probability

击

and

lose $100

with

probability

击

In

other words, if

V

只

is

the

value of

the

winnings in

the

i-th

round of playing,

then

TXT

r

-100

, with

probability

器;

川-

1.

100, with

probability

盖

Note

that

10

, . _ _ ,

9.

__ 100

μ

=

E[

Wi]

=

一

(-100)

+

1~n

100

=一一

19\

--~/

'

19--~

19

'

600\/10

σ=

削

(Wi)

=

E[(W

i

)2]

一

(E[W

i

]?

=

二口.

Let W =

L:

i~~

Wi

be

the

total

value of

the

winr

山

gs

after betting

100

times. Since every

bet

is independent of any other

bet

, it follows

that

W

is

the

sum of 100 independent identically distributed random variables. From

the

Central Limit Theorem

we

自nd

that

100006000\/10

W

自

100μ+

10σ

Z

=

一一一+

---

~-

~Y

一

=-Z

19 19

The

probability of winning

at

least $1000 can be approximated as follows:

[

100006000

飞

/101

P(W>

1000)

臼

PI

一一一一十

v …

Z>

1000 I

\

19 19

. - J

=

P(Z

>

1.

5284) = 0.0632.

The

probability of losing

at

least $1000 can be approximated as follows:

[

100006000

飞

/101

P(vV <

-1000)

目

PI

一一一一十

v

一

Z

<

-1000

I

\

19 19

J

=

P(Z

<

-0

.4

743) = 0.3176.

We conclude

that

the

probability of winning

at

least $1000

is

approximately

6%

, and

the

probability of losing

at

least $1000

is

approximately

32%.

口

Problem

4: Use risk-neutral pricing

to

且

nd

the

value of an option on a

non-dividend-paying asset with lognormal distribution if

the

payoff of

the

option

at

maturity

is

equal

to

max((S(T))

α-

K ,

O).

Here

，

α>

0

is

a

在

xed

constant.

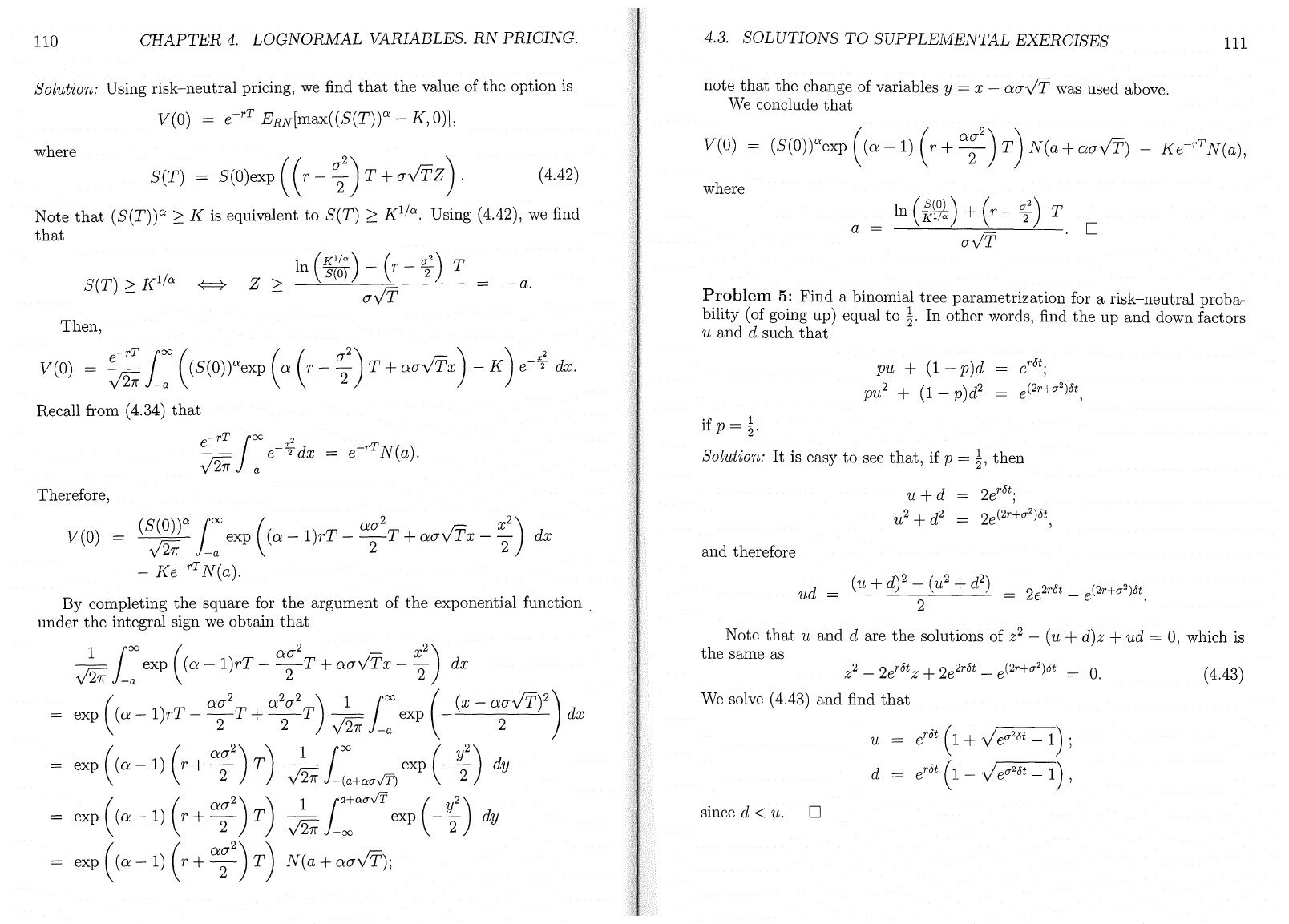
110

CHAPTER

4.

LOGNORMAL VARIABLES.

RN

PRICING.

Solution: Using

risk-neutral

pricing,

we

find

that

the

value of

the

option is

V(O)

=

e-

rT

ERN[max((S(T))

α

-

K ,

O)

],

where

盯)

=

S(O

叫

(γ-DT

十

σ

VTZ)

叫

Note

that

(S(T))

α

三

K

is equivalent

to

S(T)

三

K

1

/

α.

Using

(4

.4

2)

, we fin

S(T)

三

K

1

/

α

d

-a.

牛::::::}

Z>

The

丑

7

叩

V(O)

=

亏琴;江汇/汇川川~(川川川(牛←

μ

仙阳(侈问仰附附

S

贝仰俐(仰阶附附

0

创创)川)

Recall from

(但

4.34

刊

4

钊)

t

由

ha

创

t

5

且

:e

句

=

e-rTN

归)

Therefore,

叩)

=嘿

~1~

exp

((α-

1)ι

子

~dz-Zhz

-

Ke-rTN(

α)

V

卖

i

七言

1~ex

优叫叫

p(

均((←(归川

α

优叫

p(

←归忻川川一斗斗

1)

机)沙俨

叫叫(川川

α

←川一」斗

1)

(γ

十

子)川

T

才

)

VI

左

i

七言

ιι1:+Q

刊叩叮叫

αω

叫

σd

而叮而)

叫叫(-一

5

引)

dν

叫

(α

一刊+子

)

T)

在

1:

OUVT

叫引

dy

叫川

(r

十字

)T)

N(a+Q

σ

VT);

4.3.

SOLUTIONS

TO

SUPPLEMENTAL EXERCISES

111

note

that

the

change of variables y =

x

一

ασ

JT

was used above.

We

conclude

that

N

T

k

d

N

\111l/

T

\lliI/

//I11\

//ll1\

x

e

nu

s

--

nu

v

where

α

In

(吕

)+(γ-4)T

σ

JT

口

~~oblem

5:

Find

a binomia) tree parametrization for a risk-neutral proba-

bility (of going up) equal

to

~.

In

other

wo

旧

rd

出

s

，

直缸缸

r

时

1

t

包

L

a

缸

I

丑

ld

d such

that

pu +

(1

- p)d

pu

2

十

(1

- p)d

2

)

ifp=i

Sol

州附It

is

easy

to

see

that

,

if

p

=

~，

then

U

十

d

=2eTdt;

u

2

+

d

2

=

2e(2r

十

σ2)dt7

and

therefore

ud

(

叶

d)27(u2+d2)

-

2e

2rM

一俨

σ

2)M

Note

that

u

and

d

are

the

solutions

of

Z2

一(也十

d)z

十

ud

=

0

,

which

is

the

same

as

Z2

_

2e

rbt

z

十

2e

2rM

_

e(2r

十

σ

2)M

_

0.

We solve

(4

.4

3)

and

find

that

u

=

旷♂沪叫

M

叮

t

气(←

1

十

J

山

e

0-

2

刷耐几

6

衍

M_

一

O

d

工矿

M

(1

一

ν

e

o-

2M

-

1)

,

(

4

.4

3)

since

d

<

u.

口

wo

r

e

4EU

p

a

hu

c

Taylor's

formula

and

Taylor

series.

ATM

approximation

of

Black-Scholes

formulas.

5.1

Solutions

to

Chapter

5

Exercises

Problem

1: Show

that

the

cubic Taylor

approxi

口

lation

of

vfτ

x

around 0

IS

"十

Z

自

1

+王一旦+主

3

2

8'

16

Solution: Recall

that

the

cubic Taylor approximation of

the

function

f(x)

around

the

pointα

工

o

is

1(_.\

(x

一

α

)2.

£1/(_.\

,

(x

一

α)3

f(x)

臼

f(

α)

+

(x

一

α

)

1'

(

α)

+

\~

...

-/

f"(

α)

十

p

f(

的

(α)

2

'"

--

/

fi

f(O)

十

x

1'

(O)

十三

f

响)十三

f(

川

0).

(5.1)

2"

,/

6

For

f(x)

=

Vf石，

we

find

that

1'

(x)

=

卢

z

; fff ( zh - UZ )3/ 2 ;

尸内

)(μ

(x)

=

~

ar

丑

ld

therefore

f(O)

=

1;

1'

(0)

寸

;ff(0)=-j;

内

)=;(52)

From

(5.1)

and

(5.2)

we

conclude

由前

dτZ

但十三

-zf+21

口

2

8'

16

Problem

2: Use

the

Taylor series expansion of

the

function

eX

to

find

the

value of

eO.

25

with six decimal digits accuracy.

113

Stefanica D. Solutions Manual: A Primer For The Mathematics Of Financial Engineering

Подождите немного. Документ загружается.