Shani G. Radiation Dosimetry: Instrumentation and Methods
Подождите немного. Документ загружается.

430 Radiation Dosimetry: Instrumentation and Methods
Calculations were performed for parallel monoener-
getic beams of neutrons. The neutron cross-section data
library EFF1LIB used in these calculations is based on
the ENDF/B-5. The phantoms used for the calculations
were the ICRU slab phantom (30 cm 30 cm 15 cm),
consisting of 4-element ICRU tissue, and the male anthro-
pomorphic phantom ADAM.
For the calculation of the personal dose equivalent in the
ICRU slab phantom, a neutron quality factor was used which
takes into account the revised
Q(L) relationship of ICRP 60
and the new stopping power data for protons and alpha
particles in water by ICRU 49. The kerma values applied are
taken from ICRU 26. Above 10-MeV neutron energy, the
recommended kerma values of White et al. are used. Figure
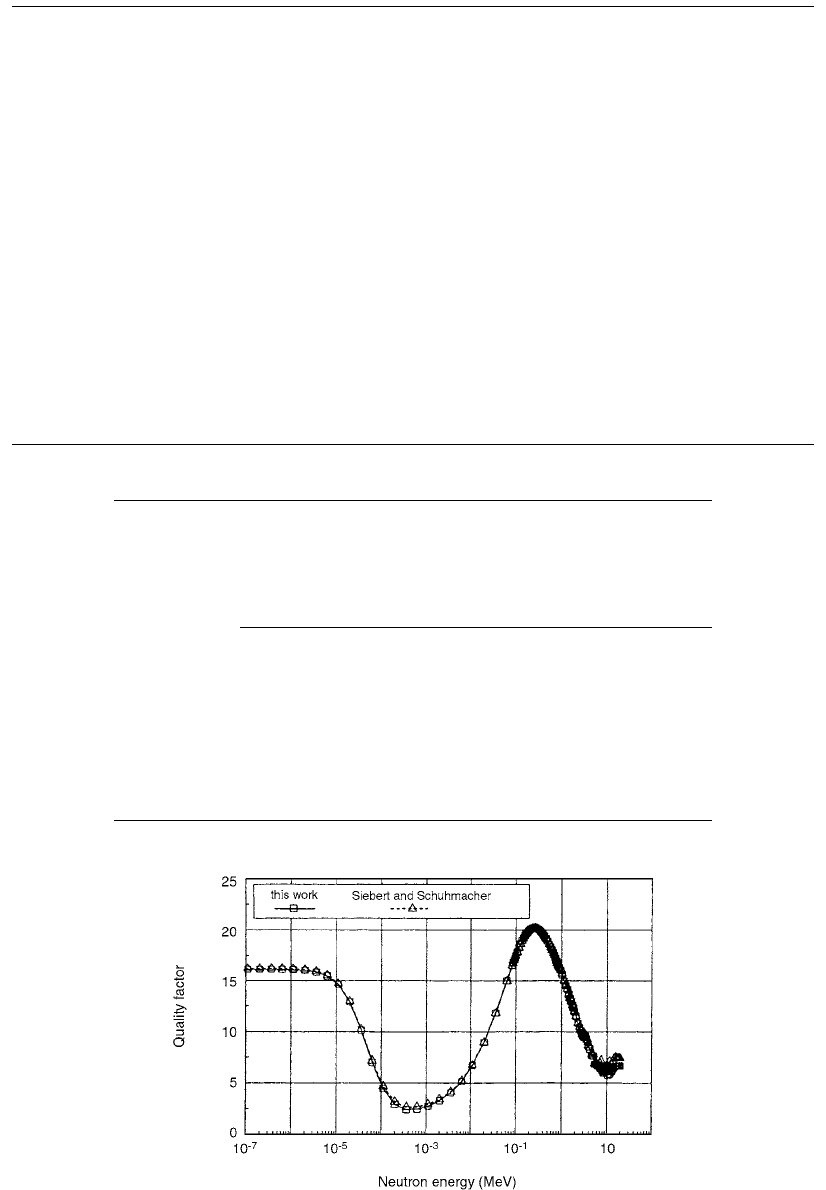
10.8 shows the quality factor as function of neutron energy.
Calculations of personal dose equivalent, ,
in the ICRU slab phantom were performed for energies
0.025 eV, 2 keV, 565 keV, and the high energies 14.8 and
19 MeV in order to see the influence of the above-men-
tioned difference in the kerma values and quality factor.
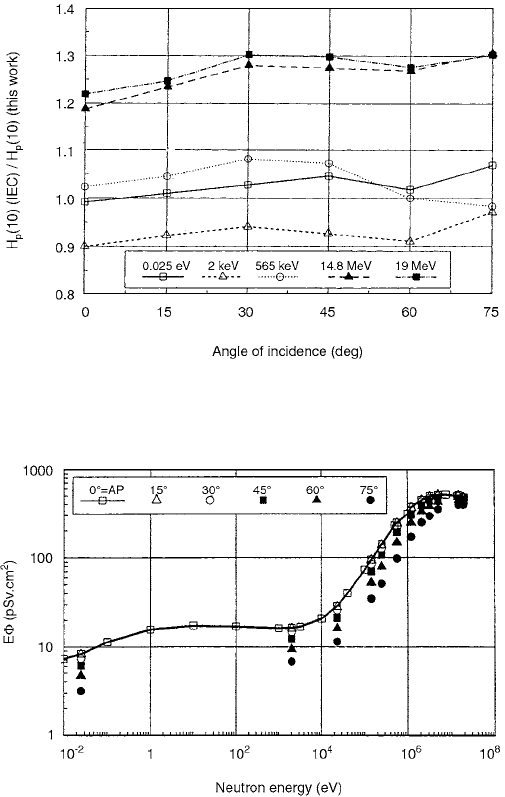
[7] The values are given in Table 10.3. Figure 10.9 shows
the ratio of personal dose equivalent, , given by
IEC to obtained in this work for the five selected
neutron energies as a function of the angle of incidence.
The differences at low neutron energies may be attributed
to the use of the S(
,
) cross-section tables for light water,
whereas for the calculation of the IEC data, the S(
,
)
cross sections of polyethelene were applied. The differ-
ence at high energies due to the different kerma values
used exceeds 20%.
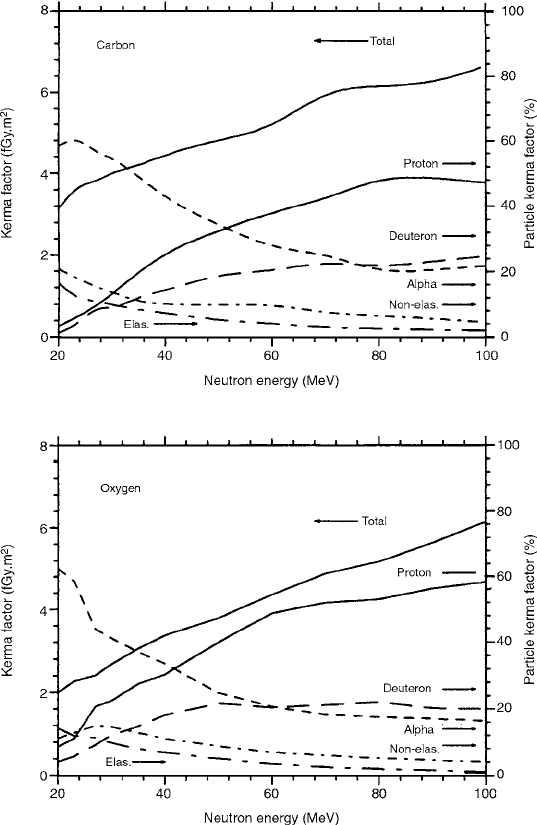
FIGURE 10.7 Calculated carbon and oxygen values for total kerma and for that due to different ejectiles plotted against neutron energy.
Total kerma is given on the left ordinate while percentage kerma values by particle type are given on the right ordinate. (From Reference [5].
With permission.)
H
p
10
,()
H
p
10
,()
H
p
10()
Ch-10.fm Page 430 Friday, November 10, 2000 12:04 PM
Neutron Dosimetry 431
TABLE 10.2
Experimental Results for A-150 Plastic (A) and Carbon (C)
E
p
MeV
E
n
MeV M
K
tot
/Q
pGy.nC
1
k
c
E
2
MeV
K
0
/Q
pGy.nC
1
0
/Q
cm
–2
.nC
–1
k
f
pGy.cm
2
31.9 26.3(29) A 1539(58) 0.562(11) 20 865(37) 11.44(64) 75.6(54) 0.469(36)
C 563(35) 0.721(11) 20 406(26) 11.44(64) 35.5(30)
43.4 37.8(25) A 1573(59) 0.493(11) 32 775(34) 19.81(55) 79.0(56) 0.539(45)
C 691(48) 0.605(11) 32 418(30) 9.81(55) 42.6(39)
50.0 44.5(26) A 1799(75) 0.470(10) 38 845(40) 10.52(70) 80.4(65) 0.506(45)
C 768(59) 0.560(10) 38 430(32) 10.52(70) 40.9(41)
63.8 58.4(29) A 1899(195) 0.427(12) (50) 811(86) 10.50(8) 77.0(10) 0.61(11)
C 976(134) 0.504(l1) (50) 492(68) 10.50(8) 46.8(74)
71.2 66.0(30) A 2030(128) 0.405(10) 58 822(55) 10.26(79) 80.1(82) 0.62(11)
C 1058(169) 0.480(10) 58 508(82) 10.26(79) 49.5(88)
The numbers in brackets denote the uncertainty (one standard deviation) of the last digits of the respective values.
From Reference [6]. With permission.
TABLE 10.3
Personal Dose Equivalent,
H
p
(10,
) in the ICRU Slab Phantom
for Five Neutron Energies at Different Angles of Incidence
E
n
(MeV)
H
p
(10,
)/(pSv.cm
2
)
0° 15° 30° 45° 60° 75°
2.5 10
8
11.50 10.50 8.87 6.32 3.97 1.62
2.0 10
3
9.69 8.91 7.73 5.86 3.80 1.72
0.565 347 334 321 292 245 117
14.8 472 456 454 449 454 396
19.0 492 478 477 473 486 436
From Reference [7]. With permission.
FIGURE 10.8 Quality factor as function of neutron energy in an ICRU tissue mass element. Differences in the high-energy region
come from different kerma values used. (From Reference [7]. With permission.)
k
f
c
/k
f
A
Ch-10.fm Page 431 Friday, November 10, 2000 12:04 PM
432 Radiation Dosimetry: Instrumentation and Methods
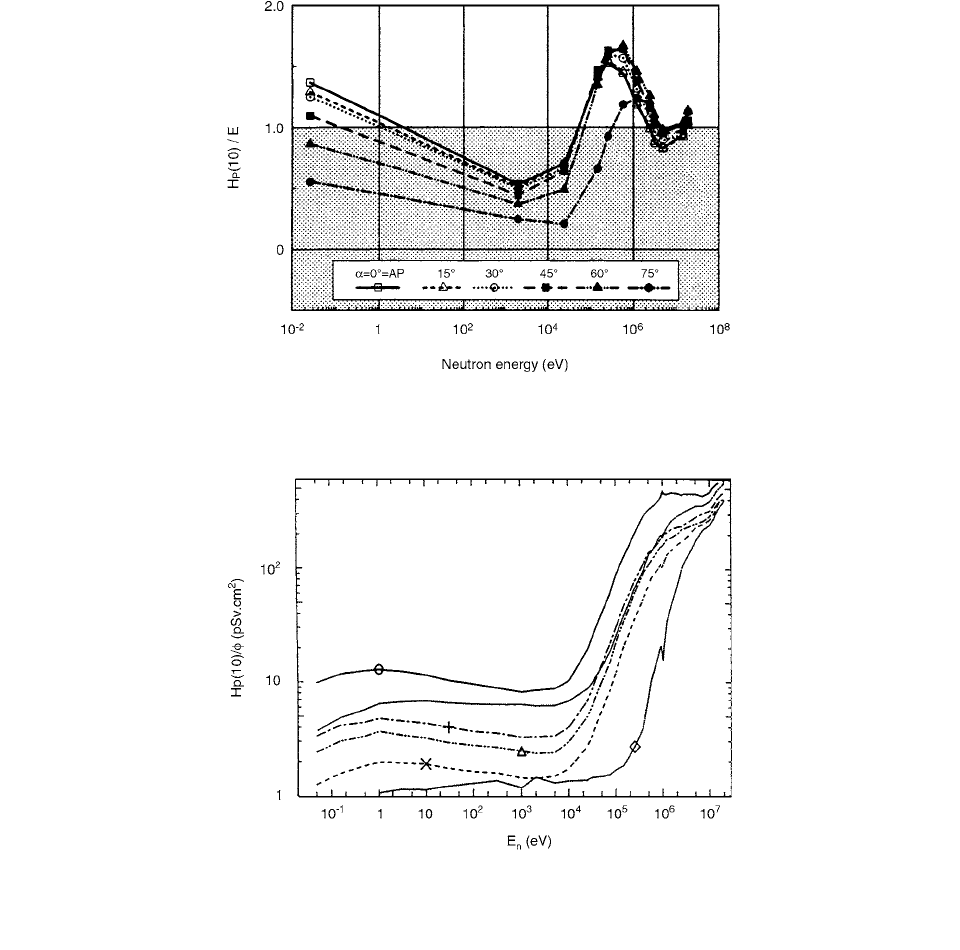
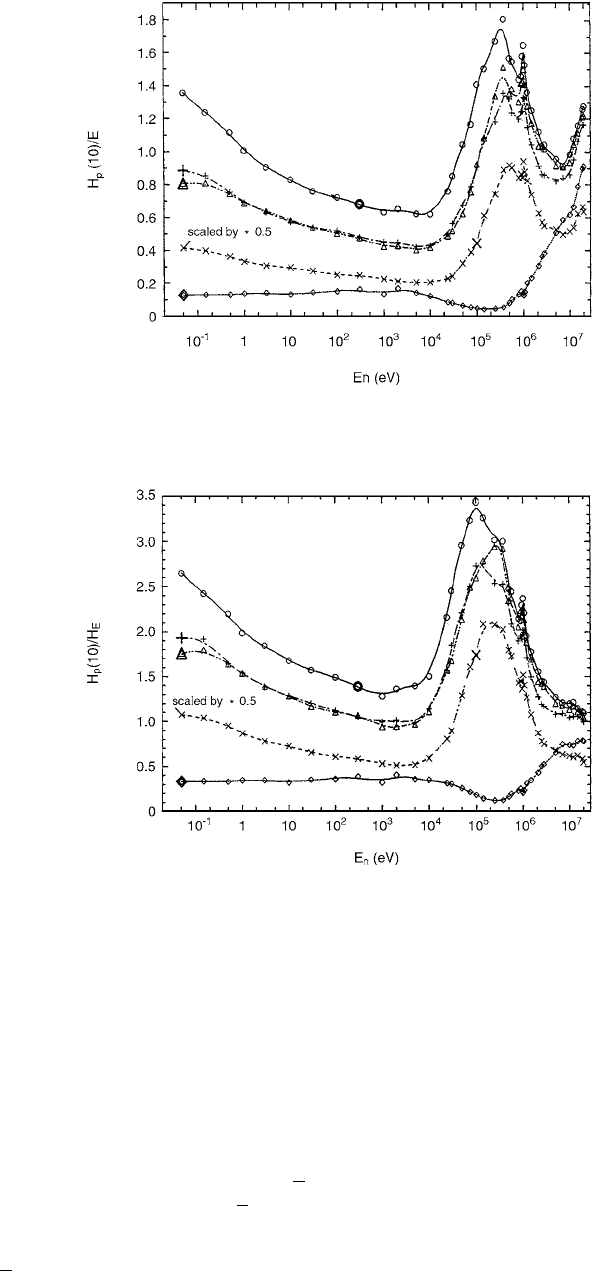
Figure 10.10 shows the result for the effective dose,
E, as a function of neutron energy for different angles of
incidence to the left side of the phantom. Figure 10.11
gives the ratio of personal dose equivalent to effective
dose, . For the personal dose equivalent, the
IEC values are used except at 14.8 and 19 MeV.
The personal dose equivalent, , was calculated
by Hollnagel [8] in the anthropomorphic MIRD phantom.
is compared with the primarily limited quantities,
the effective dose E, and the effective dose equivalent, ,
computed in the same Monte Carlo run. The operational
quantity, , in the ICRU slab was found to approx-
imate for multidirectional incidences.
The personal dose equivalent, , as defined in
ICRU Report 51, is the dose equivalent (DE) in ICRU soft
tissue at an appropriate depth d below a specified point
on the body. For strongly penetrating radiation, a depth of
10 mm is frequently employed, ICRU states, in which case
it is denoted as . The shape of the curves
in Figure 10.12 is roughly similar to that of other conversion
functions for neutrons; the PA curve is rather low, as
expected. The curve for at AP incidence is also dis-
played, showing that is a conservative estimate for
at AP geometry. Figure 10.13 shows that the ratio
ranges between 0.4 and 1.8 except for the PA
incidence, which is an exceptional situation for . In gen-
eral, underestimates the effective dose E below 50
keV neutron energy. In Figure 10.14 the ratio
exhibits a spread between 1 and 3.4, again except at PA.
Consequently, could replace the effective DE
as a limiting quantity. However, there exists no advantage
in calculating instead of or E, because the
degree of complexity of the computations is the same for
each quantity.
FIGURE 10.9 Ratio of personal dose equivalent, , in the ICRU slab phantom given by IEC to obtained for five
neutron energies as function of the angle of incidence. (From Reference [7]. With permission.)
FIGURE 10.10 Effective dose E in the ADAM phantom as function of neutron energy for six angles of incidence to the left side
of the phantom. (From Reference [7]. With permission.)
H
p
10() H
p
10()
H
p
10,
()E
H
p
10()
H
p
10()
H
E
H
sl
10()
H
p
10()
H
p
d()
H
p
10() H
p
10()
H
E
H
p
10()
H
E
H
p
10()E
H
p
H
p
10()
H
p
10()H
E
H
p
10() H
E
H
p
10() H
E
Ch-10.fm Page 432 Friday, November 10, 2000 12:04 PM
Neutron Dosimetry 433
The definition of the term “Computational Dosimetry”
was presented by Siebert and Thomas [9], interpreted as
the sub-discipline of computational physics, which is
devoted to radiation metrology. Computational simula-
tions directed at basic understanding and modeling are
important tools provided by computational dosimetry,
while another very important application is the support
that it can give to the design, optimization, and analysis
of experiments. The primary task of computational dosim-
etry is to reduce the variance in the determination of
absorbed dose. It is essential for the analysis and organi-
zation of the complex body of data needed in dosimetry,
and it provides models, evaluations, and concepts for this
purpose. The ability to use numerical means to simulate
practical situations is one of the most powerful tools of
computational dosimetry: experimental or clinical setups
may be represented; particle spectra and dose distributions
may be determined; and response functions (or matrices)
for instruments and conversion coefficients may be calcu-
lated. Computational dosimetry greatly facilitates the opti-
mization of experiments and supplements the analysis of
the data. In appropriate cases computational dosimetry can
even replace experiments.
The absorbed dose in tissue, , can be estimated from
the absorbed dose in A-150 plastic, , with ,
where
t,p
denotes the A-150 plastic-to-tissue absorbed
dose conversion factor. [10] In monoenergetic neutron
fields, and under charged particle equilibrium conditions,
FIGURE 10.11 Ratio of personal dose equivalent, , in the ICRU slab phantom to effective dose E in the ADAM phantom
for six angles of incidence. The shaded area exhibits the region of non-conservativity. (From Reference [7]. With permission.)
FIGURE 10.12 The personal dose equivalent (DE), H
p
(10), in the trunk of the male MIRD phantom for five irradiation geometries:
(
O) AP, () PA, (X) lat, () Rot, () Iso. The dotted curve represents the effective DE, H
E
, at AP in the same phantom. (From
Reference [8]. With permission.)
H
p
10()
D
t
D
p
D
t
tp,
D
p
Ch-10.fm Page 433 Friday, November 10, 2000 12:04 PM
434 Radiation Dosimetry: Instrumentation and Methods
the conversion factor can be calculated from the kerma
factors of the individual elements:
(10.12)
where and denote the elemental mass fraction tissue
and A-150 plastic, respectively calculated values of are
shown in Figure 10.15.
Ambient dose equivalent (
d) is defined as H
at the reference point inside the ICRU sphere, with given by:
(10.13)
where denotes the dose distribution in
L and Q(L)
is the quality factor. The TEPC (Tissue Equivalent Pro-
portional Counter) can be used to estimate . While
the different compositions of the TEPC wall (A-150 plas-
tic) and standard tissue influence D and may be conver-
ted with , their influence on requires further
consideration.
MCNP Monte Carlo calculations with an extended
cross-section data set have been performed by Mares et al.
[11] for an anthropomorphic MIRD (ADAM) phantom in
the neutron energy range between 20 and l00 MeV. The
irradiation conditions varied from 0
(frontal incidence)
up to 75 to the left side of the MIRD phantom. For the
calculation of organ dose equivalent (the index q
FIGURE 10.13 Ratio of the personal DE, H
p
(10), to the effective dose E, both in phantom ADAM for five neutron incidences.
Symbols as in Figure 10.12 apply. (From Reference [8]. With permission.)
FIGURE 10.14 Ratio of the personal DE, H
p
(10), to the effective DE, H
E
, both in phantom ADAM for five neutron incidences.
Symbols as in Figure 10.12 apply. (From Refernce [8]. With permission.)
t, p
t
i
K
i
i
p
i
k
i
i
t
i
p
i
tp,
H
*
QD
Q
Q
1
D
----
D
L
QL()Ld
0
D
L
D
L
t, p
D
L
H
Tq,
Ch-10.fm Page 434 Friday, November 10, 2000 12:04 PM
Neutron Dosimetry 435
indicates that the quality factor is applied) a quality
factor for neutrons was applied which takes into
account the revised
Q(L) relationship of ICRP 60 and
the new stopping power data for protons and alpha par-
ticles in water of ICRU 49. (Mares et al. [11])
(10.14)
where
is the quality factor as a function of neutron
energy
E
is the fluence-to-kerma conversion factors
as a function of neutron energy E, depending
on the elemental composition of the tissue in
organ T.
is the fluence-to-kerma conversion factor
as a function of photon energy
FIGURE 10.15 Absorbed dose conversion factor for A-150 plastic to ICRU standard tissue. The solid line is based on
evaluated kerma factor data. The dotted lines correspond to a 68% confidence level. The data points are derived from experimental
kerma factors of C and O that have been published for . The symbols refer to experimental works cited elsewhere.
(From Reference [10]. With permission.)
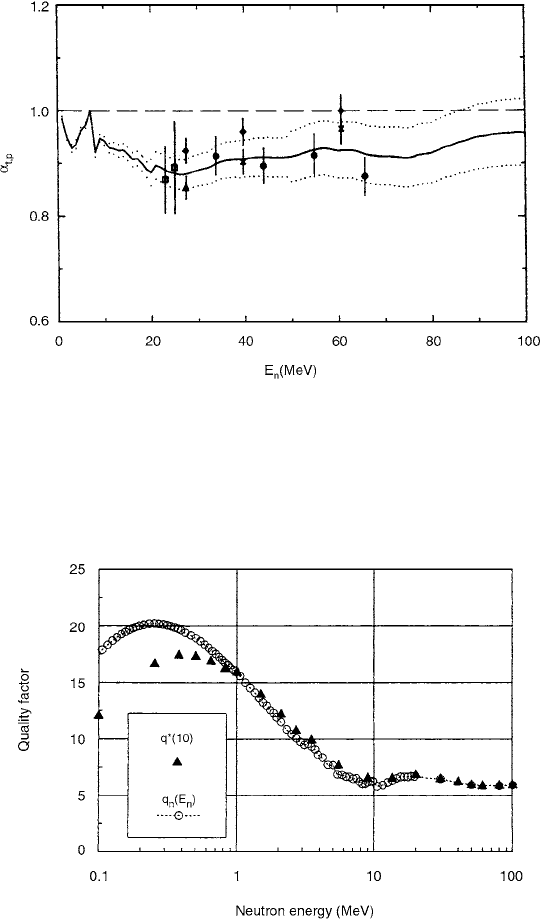
FIGURE 10.16 Neutron quality factor and q
*
(10) in the ICRU sphere as function of neutron energy. Above 20 MeV, data for q
*
(10)
of Sannikov et al. [60] are used as an approximation for the quality factor . (From Reference [11]. With permission.)
tp,
E
n
20 MeV
q
n
q
n
H
T ,q
E() q
n
E()k
n
E()
Tn,
E E,()Ed
k
ph
E()
Tph,
E E,()Ed
q
n
E()
k
n
E()
k
ph
E()
E
Ch-10.fm Page 435 Friday, November 10, 2000 12:04 PM

436 Radiation Dosimetry: Instrumentation and Methods
and are the neutron and
photon fluences in the organ.
For the calculation of the equivalent organ dose
(according to ICRP60), the smooth radiation weighting
factor function was applied:
(10.15)
Effective dose
E and effective dose equivalent were
calculated by use of the new organ weighting factors
of ICRP 60 where
and
Figure 10.16 shows the quality factor and the ratio
q
*
(10) H
*
(10)/D
*
(10) at 10 mm in the ICRU sphere as
a function of neutron energy.
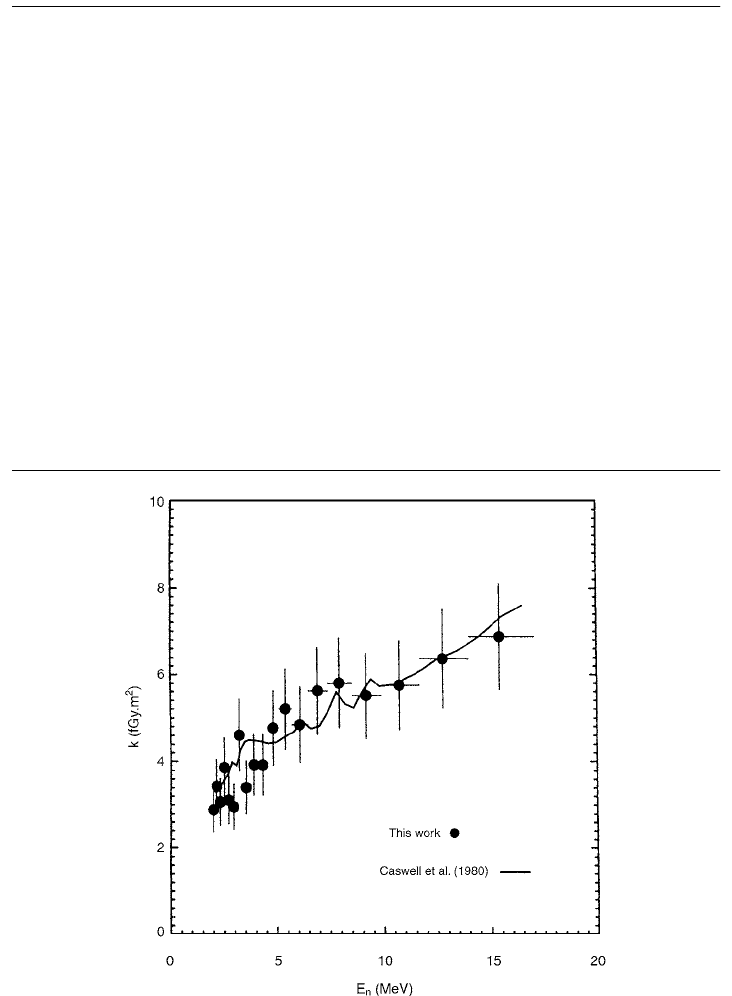
The measurement of neutron fluence-to-kerma con-
version coefficients, (commonly called kerma factors)
(k(E)), was performed by Newhauser et al. [12]; these
factors are shown in Figure 10.17.
A comprehensive set of kerma factor values for H, C,
O, N, and Si, as well as for tissue and frequently used
high-energy particle detectors, was given by Gorbatkov
et al. [13] Assuming charged in equilibrium at neutron
energies below 100–150 MeV, the absorbed dose rate
(D, Gy s
1
) can, with satisfactory accuracy, be found from
the following relation:
(10.16)
where
F(E) is the neutron spectrum (neutron cm
2
MeV
1
s
1
) and is the neutron kerma factor (Gy cm
2
). The
neutron kerma factor is defined as the average kinetic
energy released in matter (per unit mass and per unit
neutron fluence) and is the sum of all energy transferred
to light charged particles and residual nuclei in an elastic
or nonelastic nA-reaction. For composite materials, the
neutron kerma factor can be expressed as
(10.17)
where is Avogadro’s number, is the atomic
density of the ith isotope, is the charged particle
production cross section is the
average kinetic energy of all charged particles produced
by monoenergetic neutrons when interacting with a
nucleus of the ith isotope, is the neutron kerma
factor for the ith isotope, and is the ith isotope fraction
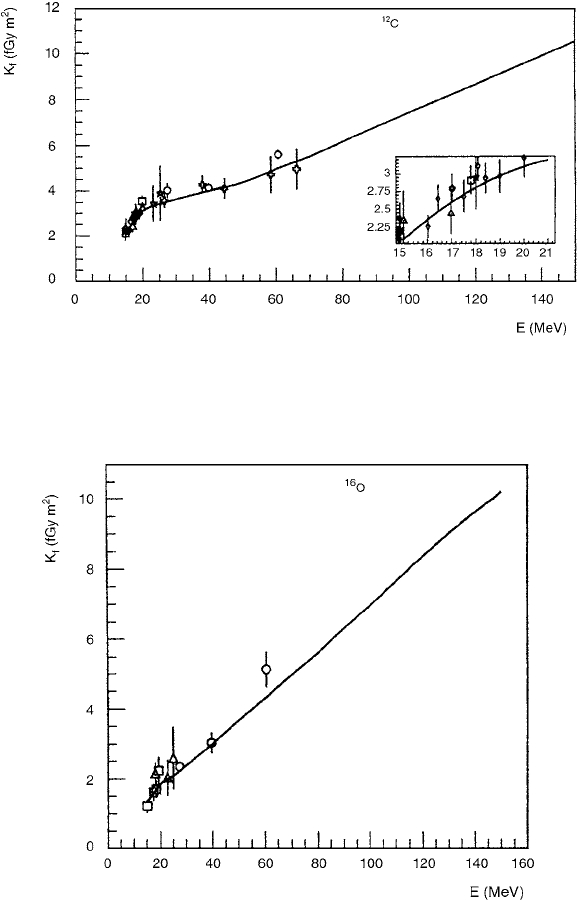
in the mixture. Comparison of calculated and measured
values of neutron kerma for , , and are shown
in Figures 10.18–10.20, respectively. Neutron kerma val-
ues for some elements are given in Table 10.4 and for
some materials in Table 10.5. Calculated values for kerma
factor are shown in Figure 10.21.
III. GAS-FILLED NEUTRON DOSIMETERS
Tissue-equivalent proportional counters became the popular
neutron dosimeters. The newer dosimeters were developed
from the well-known microdosimeters. Still, there are limi-
tations to those dosimeters’ applications. The accuracy
achievable in radiation-protection dosimetry of mixed neu-
tron-photon radiation fields still needs improvement. The area
monitors are heavy in weight because of the amount of mod-
erator needed for fast neutron dosimetry. Also, their dose
equivalent response has a strong energy dependence. Mea-
surement of dose due neutrons of energy above 15 MeV is
practically impossible with a gas-filled dosimeter. Some later
developments and investigations of the gas-filled detectors
are discussed in this section.
The original motivation to develop a tissue-equivalent
proportional counter (TEPC)-based device came from the
fact that a quantity can be derived from a TEPC that is
similar to H. H can be written as
(10.18)
with the quality factor
Q defined as a function of L and the
differential distribution, , of the absorbed dose with
Tn,
E,E()
Tph,
E,E()
H
T
W
R
H
T
E() w
R
E()
k
n
E()
Tn,
E E,()Ék
ph
E()
Tph,
E,E()Ed
d
H
E
w
T
Ew
T
H
T
T
H
E
w
T
H
Tq,
T
q
n
DFE()K
f
E()Ed
K
f
E()
K
f
E() N
A
i
i
E
i
i
K
fi
E()
i
N
A
i
i
i
i
non
el
i
()E
i
,
TABLE 10.4
Neutron Kerma Factors for H, C, O, N, Si (fGy m
2
)
E (MeV) H C O N Si
15 48.1 2.06 1.31 1.5 1.21
20 47.0 3.10 1.85 2.04 1.53
25 45.85 3.41 2.08 2.46 1.74
30 44.51 3.64 2.37 2.78 2.02
40 42.92 4.0 3.0 3.62 2.51
50 40.75 4.39 3.67 4.44 3.08
60 39.04 4.97 4.4 5.24 3.62
70 37.72 5.49 4.96 5.9 4.16
80 36.74 6.03 5.6 6.58 4.73
90 36.05 6.69 6.3 7.2 5.33
100 35.58 7.54 6.94 8.0 5.86
120 35.23 9.03 8.35 9.34 6.93
150 35.82 11.05 10.2 11.34 8.48
From Reference [13]. With permission.
K
fi
E()
i
C
12
O
16
N
14
HQD
L
Ld
D
L
Ch-10.fm Page 436 Friday, November 10, 2000 12:04 PM
Neutron Dosimetry 437
respect to L. Lineal energy, y, defined as the ratio of the
energy imparted to a volume (to the sensitive volume of the
detector) and the average chord length in that volume, is
closely related to L. [14] Various procedures can be used
to determine the dose equivalent reading of a TEPC, .
One method is:
(10.19)
with a weighting function q
which approximates the qual-
ity factor Q, e.g., using q Q and setting y L. A
corresponding reading for the mean quality factor can be
obtained from
(10.20)
TABLE 10.5
Neutron Kerma Factors for Composite Materials (fGy m
2
)
E
(MeV) Tissue CH NaI CsI BaF
2
CeF
3
BGO LS PbW0
4
15 6.12 5.6 0.08 0.02 0.46 0.62 0.20 0.36 0.18
20 6.55 6.45 0.21 0.08 0.52 0.69 0.36 0.50 0.27
25 6.66 6.67 0.35 0.16 0.59 0.78 0.44 0.58 0.33
30 6.78 6.75 0.47 0.25 0.68 0.89 0.56 0.69 0.41
40 7.16 6.99 0.77 0.47 0.91 1.15 0.83 0.94 0.62
50 7.52 7.19 1.08 0.72 1.21 1.49 1.13 1.23 0.85
60 7.98 7.59 1.40 0.99 1.50 1.82 1.44 1.54 1.11
70 8.35 7.97 1.72 1.24 1.82 2.18 1.72 1.82 1.35
80 8.82 8.39 2.02 1.49 2.11 2.51 2.01 2.12 1.59
90 9.37 8.95 2.29 1.72 2.38 2.80 2.26 2.40 1.82
100 9.93 9.70 2.57 1.94 2.66 3.12 2.54 2.70 2.06
120 11.2 11.0 3.15 2.42 3.28 3.79 3.12 3.32 2.58
150 12.9 13.0 3.97 3.08 4.08 4.68 3.95 4.16 3.28
From Reference [13]. With permission.
FIGURE 10.17 Neutron fluence-to-kerma coefficients k in A-150 plastic as a function of neutron energy from a proportional
counter measurement that was iteratively unfolded. Results are normalized to the value of
k at 12 MeV from Caswell et al. [61]
(From Reference [12]. With permission.)
E
n
M
H
M
H
qD
y
yd
M
Q
M
H
D
y
yd
Ch-10.fm Page 437 Friday, November 10, 2000 12:04 PM
438 Radiation Dosimetry: Instrumentation and Methods
An instrument for the direct measurement of dose equiv-
alent for any type of radiation seems to be feasible if the
relation between L and y, or more precisely, between
and , are known.
The neutron interaction with a person’s body greatly
influences the radiation field at a point of interest. In fact,
for neutron energies below about 10 keV, H is dominated
by the contribution of neutrons thermalized in the body
while the contribution of the primary neutrons is negligi-
ble. The “operational quantities,” such as the ambient dose
equivalent, penetrating
H
*
(10) are therefore defined at a
reference point in a 30-cm-diameter spherical phantom.
Each instrument must therefore be adjusted to achieve an
energy-independent dose equivalent response .
The energy dependence of three typical detector sys-
tems—a thick-walled TEPC (KFA), a thin-walled TEPC
(BIO), and a moderator-type dose equivalent rate meter
(Leake)—is shown in Figure 10.22. The systems were
calibrated in the radiation field of a -moderated Cf
source in terms of the neutron ambient dose equivalent.
While varies for Leake by a factor of 20, the factor
for the other two systems is 4 and 5, respectively. The
reasons for the energy dependence of the TEPC systems
are well understood: for the thick-walled TEPC, the low
FIGURE 10.18 Comparison between experimental (symbols) and calculated (curve) neutron kerma factors for carbon. (From
Reference [13]. With permission.)
FIGURE 10.19 Comparison between experimental (symbols) and calculated (curve) neutron kerma factors for oxygen. (From
Reference [13]. With permission.)
D
L
D
y
R
H
D
2
O
R
H
Ch-10.fm Page 438 Friday, November 10, 2000 12:04 PM
Neutron Dosimetry 439
at about 100 keV is mainly explained by the neutron
fluence attenuation in the detector wall, and for the thin-
walled TEPC, the low below 50 keV is mainly caused
by the insufficient thermalization of neutrons and the short
range of the recoil protons produced.
The main properties of systems which have been
developed for and applied in radiation protection dosim-
etry are listed in Table 10.6. The prices listed are approx-
imate values given by the manufacturers and compose
the complete systems. Depending on the intended field
TABLE 10.6
Technical Properties of TEPC-based Radiation Protection Dosimeters
CIRCE CIRCG EG&G HANDI KFA PNL REM-402
m (kg) 5 10 0.6 6 7
a
2 2
V (1) 6 30 0.8 18 10
a
1.5 6.4
V
s
(cm
3
) 98 27 109 110 270 1 97
T (h) – – 40 24 – 240 17
Price
b
25000 16000
c
2000 10000
d
40000 – 5000
Application
e
S A P A A S A
m total mass of the system (detector and electronics)
V total volume of the system (detector and electronics)
V
s
sensitive volume of detector
T minimum time of operation with internal power supply (if available)
a
Excluding a personal computer, required for data analysis.
b
Approximate price in US$.
c
Excluding the detector.
d
A small number of prototypes were produced on a net-cost basis.
e
Main area of application, A: area monitoring, P: personnel monitoring, S: space dosimetry.
From Reference [14]. With permission.
FIGURE 10.20 Comparison between experimental (symbols) and calculated (curve) neutron kerma factors for nitrogen. (From
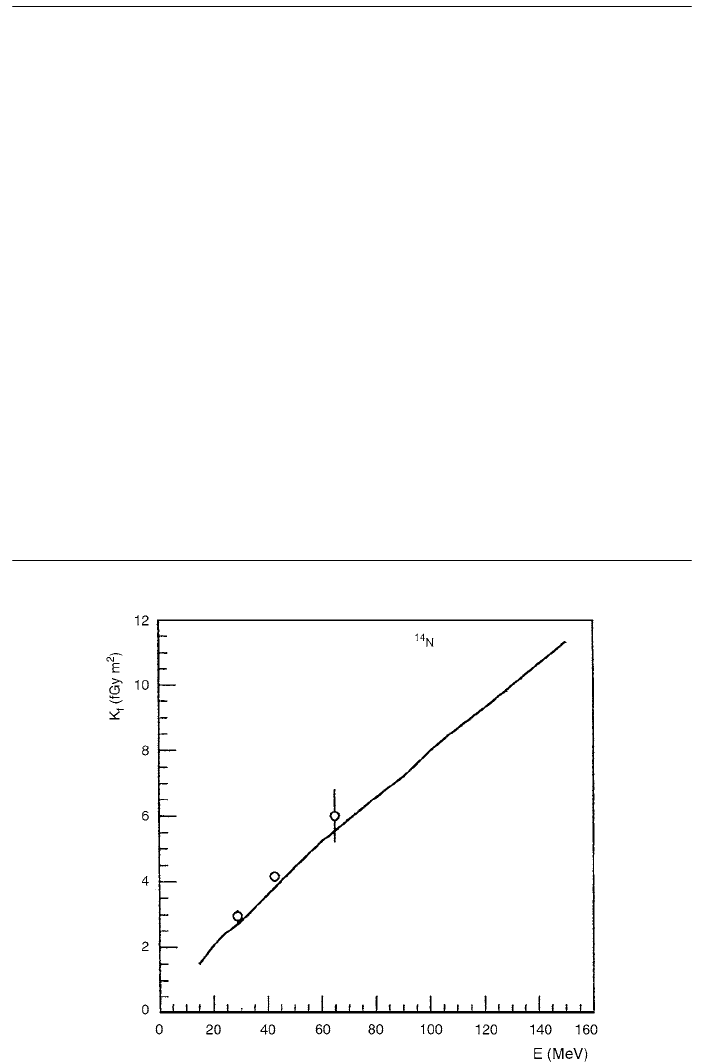
Reference [13]. With permission.)
R
H
R
H
Ch-10.fm Page 439 Friday, November 10, 2000 12:04 PM
