Shani G. Radiation Dosimetry: Instrumentation and Methods

Подождите немного. Документ загружается.

340 Radiation Dosimetry: Instrumentation and Methods

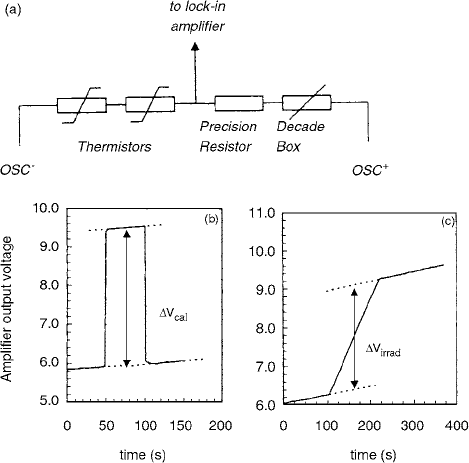
using the measuring bridge that is shown schematically in

Figure 6.13a. Two thermistors are serially connected in

one arm of the a.c. bridge, and in the opposite arm a ﬁxed

high-precision resistor and a variable resistor are con-

nected to compensate the bridge. The out-of-balance volt-

age is ampliﬁed by a lock-in ampliﬁer. Figures 6.13b and

6.13c show a typical calorimeter run in the 10-MV photon

beam with ﬁrst a “calibration run” and thereafter an “irra-

diation run.” The procedure used to determine the voltage

change at mid-run is shown. The calibration of the bridge

output voltage is performed in a “calibration run” by

adding a well-known resistor to the bridge circuit (oppo-

site arm as thermistors) and determining the correspond-

ing out-of-balance voltage. This calibration is then used

to derive the average fractional thermistor resistance

change R/R due to irradiation from the voltage change

at mid-run, V, during an “irradiation run.” On both cal-

ibration and irradiation runs, corrections for changes in

self-heating of the thermistors and for nonlinearity of the

bridge response are applied. T is calculated as T 

R/RS, where S is the average thermistor sensitivity result-

ing from calibration of the thermistors against standard

thermometry. [11]

III. GRAPHITE CALORIMETER

Potentially, water calorimeters are most accurate, but they

are delicate systems and require considerable care to con-

trol the purity of the water and the effects of radiochemical

reactions occurring in it. The standard based on the graphite

calorimeter is improving continuously. Graphite calorim-

eters are stable and are very accurate. However, suitable

conversion procedures are necessary for establishing the

absorbed dose to water. A particular problem in graphite

calorimetry is gap effect and its corrections.

The primary standard of absorbed dose to water estab-

lished at ENEA for the

Co gamma-ray quality is based

on a graphite calorimeter and an ionometric transfer sys-

tem. [12] The graphite calorimeter is of the Domen type.

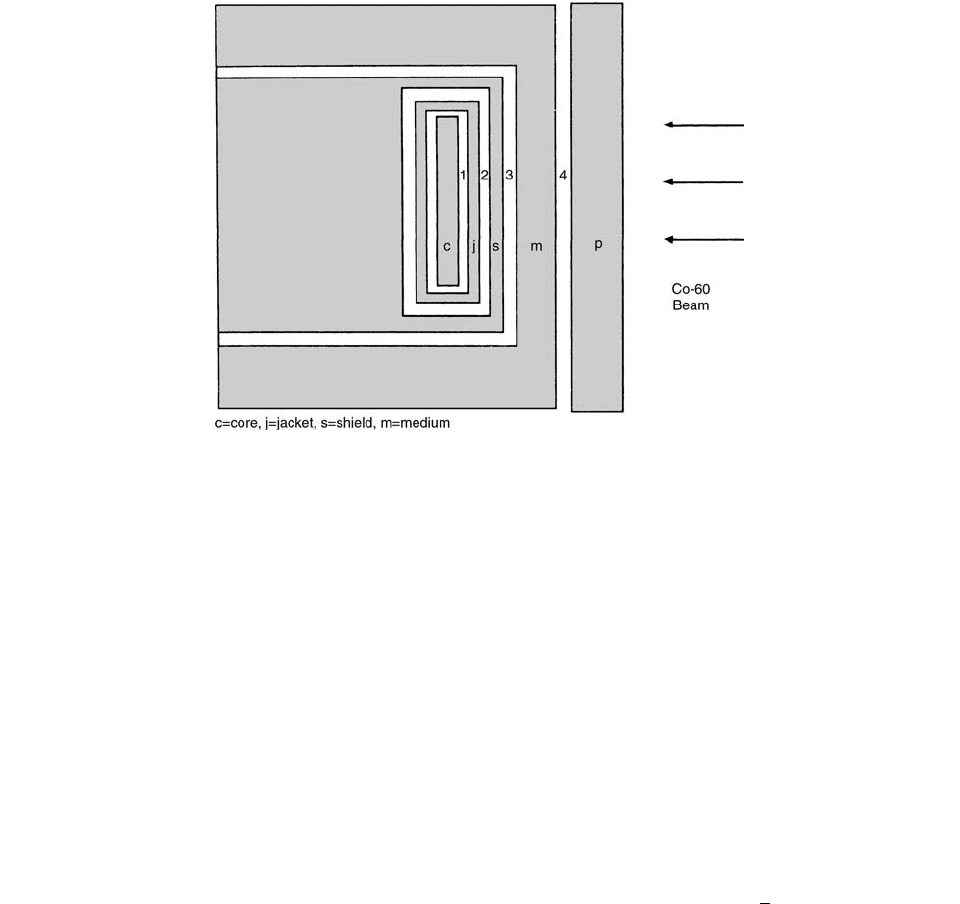
The gap conﬁguration of the ENEA calorimeter is shown

in Figure 6.14. The four calorimeter bodies are enclosed

in a 1-cm-thick PMMA housing with 152-mm external

diameter. The external housing is radially surrounded by a

close-ﬁtting 300-mm-diameter graphite annular body. It is

possible to increase the thickness of this annular body by

adding posterior 300-mm-diameter full backscattering

graphite plates and additional graphite front plates to

increase the measurement depth in graphite. The minimum

measurement depth (distance of the central plane of the

core from the calorimeter front surface) is 0.88 g cm

2

. The

calorimeter is thermoregulated at a temperature of about

27°C, with a stability better than 5  10

4

°C during a typical

measurement run. The jacket has approximately the same

heat capacity as the core, which is a disc of 20-mm diameter

and 2.75-mm thickness. In two holes radially drilled in the

core edge, two measuring thermistors are embedded, thus

doubling the sensitivity during measurements. Additional

thermistors are in the other calorimeter bodies (one ther-

mistor for each body) for temperature monitoring. Elec-

trical heaters are embedded in all of the four bodies of

the calorimeter. The heaters are manually operated to

inject heat amounts into each body for the calorimeter

electrical calibration and for controlling the heating or

cooling drifts in the different bodies. [12]

To transfer the dose from graphite to water, a thick-

walled (TW) ionization chamber is used. This is a cylin-

drical homogeneous chamber with graphite wall sufﬁ-

ciently thick (0.5 g cm

2

) to allow electron equilibrium at

the

Co gamma radiation.

In each gap of the calorimeter there are three different

regions contributing in a different way to the variation of

the radiation ﬂuence in the core. These regions are those

in front of (anterior gap), behind (posterior gap), and

around (annular gap) the core. The effects of these three

gap regions were evaluated, separately but a single correc-

tion was determined for the same region of all three gaps.

For conversion of the absorbed dose from graphite to

water, the TW chamber was irradiated in graphite phantom

at the same depth,

z(P

), where the absorbed dose to graph-

ite,

, was known by absolute measurement with the

calorimeter. A chamber calibration factor, N

, in terms of

absorbed dose to graphite, was then obtained as

(6.14)

FIGURE 6.13 (a) Schematic drawing of the a.c. measuring

bridge and typical time evolution of the bridge output voltage

during (

b) a “calibration run” and (c) an “irradiation run.” (From

Reference [11]. With permission.)

()QP

()

CH-06.fm Page 340 Friday, November 10, 2000 12:02 PM

Calorimetry 341

where Q(P

) is the ionization charge due to the absorbed

dose D

at P

. The chamber was then irradiated in the

water phantom at a depth z(P

), where the photon ﬂuence

differential in energy was expected to be the same as that

existing at the reference depth z(P

) in the graphite phan-

tom. The “corresponding points”

and P

were deter-

mined according to the scaling rule derived from the

photon ﬂuence scaling theorem

(6.15)

where

) is the absorbed dose to graphite at the center

of a small mass of graphite placed in water with its center

in P

; Q(P

) is the corrected charge reading due to the

absorbed dose D

at P

The existence of transient electron equilibrium condi-

tions (TCPE) in that mass of graphite allows us to express

the absorbed dose given by Equation (6.15) also as

(6.16)

where 

) is the photon energy ﬂuence at the center

of the mass of graphite placed in water at P

, (





)

the mass energy absorption coefﬁcient in graphite aver-

aged over the photon energy spectrum, and



is the ratio

of the absorbed dose to the collision part of kerma at the

center of the small mass of graphite.

Similarly, the absorbed dose to water at P in undis-

turbed water can be written as [12]

(6.17)

where the symbols have an analogous meaning to those

in Equation (6.16), with water in place of graphite. In

particular, 

) is the photon energy ﬂuence in undis-

turbed water at P

By the ratio of Equations (6.16) and (6.17), one then

obtains

Combining Equations (6.16) and (6.17) will give

(6.19)

where the notation represents the ratio (x)

(x)

. [12]

An electron beam graphite calorimeter has been devel-

oped by Burns and Morris [13] covering the dose range

from kilogray levels down to doses of 1 Gy, at dose rates

down to 5 Gy/min. The thermistor-bridge system is cali-

brated by reference to three triple-point temperatures, using

a platinum resistance thermometer as a transfer device. The

uncertainty in the measurement of the absorbed dose to the

graphite core is estimated lobe 1% at the 95% conﬁdence

level.

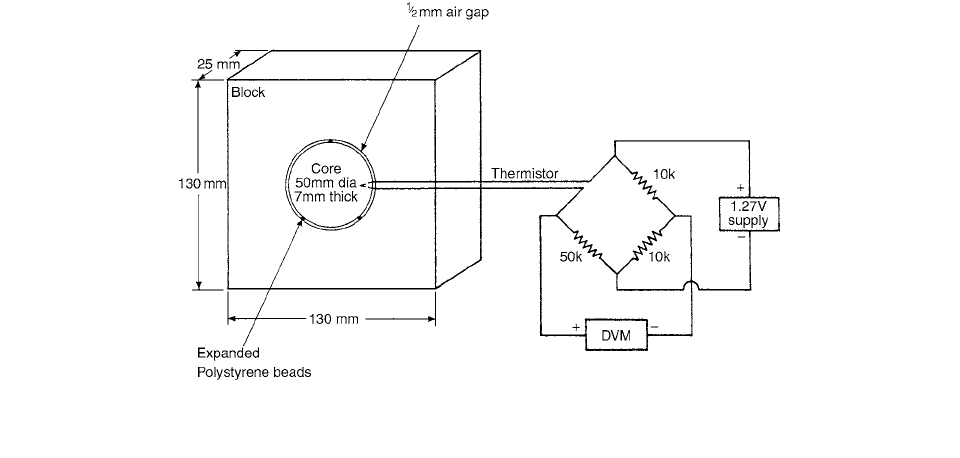
The calorimeter system is shown schematically in

Figure 6.15.

The calorimeter core is a disc of graphite 50 mm in

diameter and 7 mm in thickness. A hole, 1.5 mm in diam-

eter and 7 mm deep, is drilled radially into the mid-plane

FIGURE 6.14 The schematic conﬁguration of the gaps in the ENEA graphite calorimeter. The gaps around the calorimeter core, c,

are those denoted 1–3. Gap 4 is between the calorimeter and the frontal external plate,

p, used to vary the measurement depth. The

gap width is 0.5 mm for all of the anterior gaps and for the annular gaps 1 and 2, 1 mm for the annular gap 3, and 0.65 mm and

1.2 mm for the posterior gaps 1 and 2, respectively. (From Reference [12]. With permission.)

() QP

()N



() 

()





()





() 

()





()





() D

()







()









()

[]







()







(6.18)

() QP

()N





()







CH-06.fm Page 341 Friday, November 10, 2000 12:02 PM

342 Radiation Dosimetry: Instrumentation and Methods

of the core to accommodate a glass bead thermistor with a

nominal resistance of 50 k at 20°C. Thermal contact with

the graphite is improved by using a small amount of zinc

oxide–based heat sink compound around the thermistor

bead. This disc is inset into the surface of a rectangular

block of graphite approximately 130  130  25 mm

deep and is thermally isolated from the block by a nominal

0.5-mm air gap. Small expanded polystyrene beads sepa-

rate the disc from the block. The dimensions of the block

were chosen to be sufﬁciently large to ensure the complete

scattering of 15-MeV electrons into the core, but at the

same time they are small enough to give relatively uniform

heating of the rectangular block, in area and in depth, in

a scattered electron beam from the NPL linear accelerator

(linac). The core and surrounding block are completely

enclosed in 25 mm of expanded polystyrene, which has

been shown to have no measurable effect on the response

of the calorimeter. If this assembly is allowed to reach

equilibrium in an environment which is temperature con-

trolled to

200 mK, any temperature drift of the core is

linear over a period of tens of minutes and is always less

than 0.5 mK/min. Dose rates down to about 3 Gy/min,

and doses below 1 Gy, have been measured without the

need for further temperature control. In particular, no vac-

uum system or controlling heaters are necessary. [13]

A description was given by DuSautoy [14] of the UK

primary standard graphite calorimeter system. The calo-

rimeter measures absorbed dose to graphite for photon

radiations from

Co to 19-MV x-rays and is the basis of

the NPL therapy-level absorbed dose to water calibration

service. Absorbed dose to graphite from the photon calo-

rimeter has been compared with three other standards: an

ionization chamber and cavity theory, for

Co gamma

radiation; the NPL electron calorimeter, for 12–14-MeV

electron beams; and the BIPM

Co absorbed dose stan-

dard. The calorimeter has been called the photon-beam

calorimeter to distinguish it from the electron-beam calo-

rimeter. This calorimeter has been the primary standard

for the NPL calibrations in absorbed dose to water and

the chemical dosimetry services since 1988. The mean

absorbed dose to carbon (graphite) in the core of the

calorimeter D

is determined by measuring the energy

deposited by ionizing radiation, E

, in the core of mass

m, according to the deﬁnition of absorbed dose given by

(6.20)

For a dose of 1 Gy, the energy absorbed, E

, raises the

temperature of the core by about a millikelvin. This tem-

perature rise is measured with a resolution of a few tens

of microkelvin by a thermistor embedded in the core.

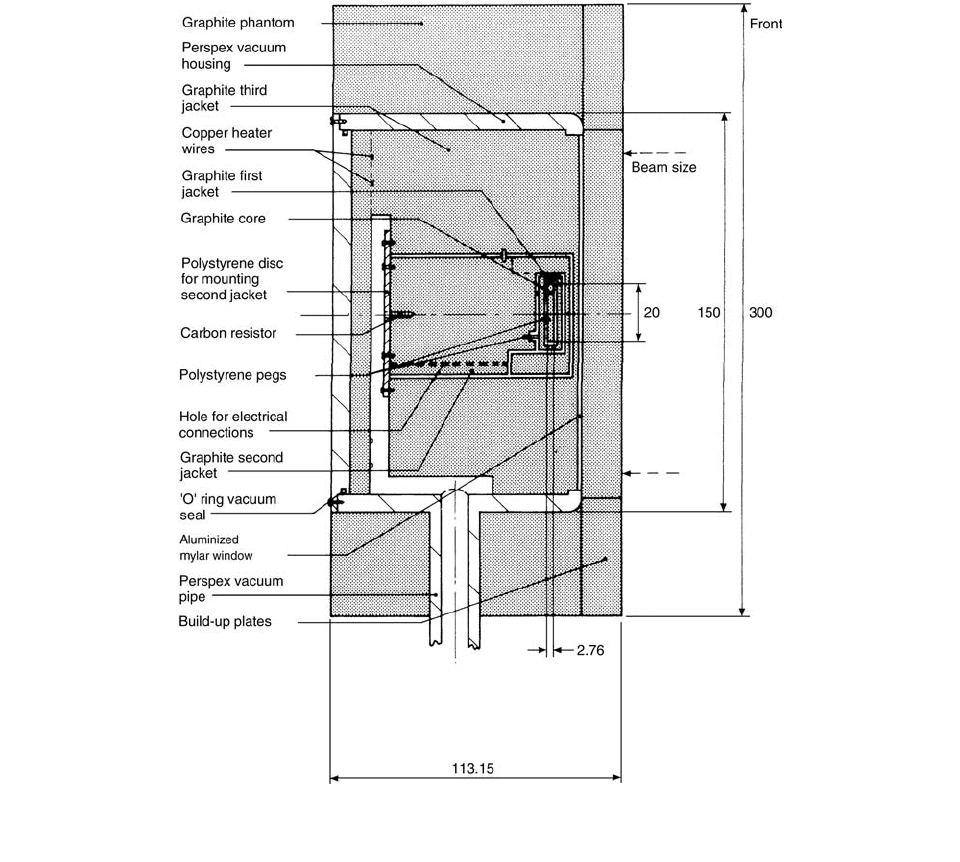
Figure 6.16 shows the vertical cross section through

the axis of the assembled calorimeter and gives the dimen-

sions in more detail. The core is placed near the front so

that electron beam measurements can be made. The min-

imum measurement depth is about 1 g cm

2

. Two ther-

mistors are included in the core; one is used as a sensor

and the other as a heater for the electrical calibration.

The core resistance measurement and heating circuits

are shown in Figure 6.17. Initially the second jacket heat-

ing is regulated to produce a constant second jacket tem-

perature, and the core and ﬁrst jackets are allowed to reach

thermal equilibrium. The core, ﬁrst jacket, and second

jacket bridges are then balanced by adjusting the balancing

resistor (e.g., R

in the core circuit). Before a measure-

ment, the heating power in the second jacket is ﬁxed at a

FIGURE 6.15 Schematic diagram of calorimeter system. The calorimeter block is completely enclosed in 25 mm of expanded

polystyrene. (From Reference [13]. With permission.)

------



CH-06.fm Page 342 Friday, November 10, 2000 12:02 PM

Calorimetry 343

level which gives steady increase in temperature at a rate

of approximately 12



K min

1

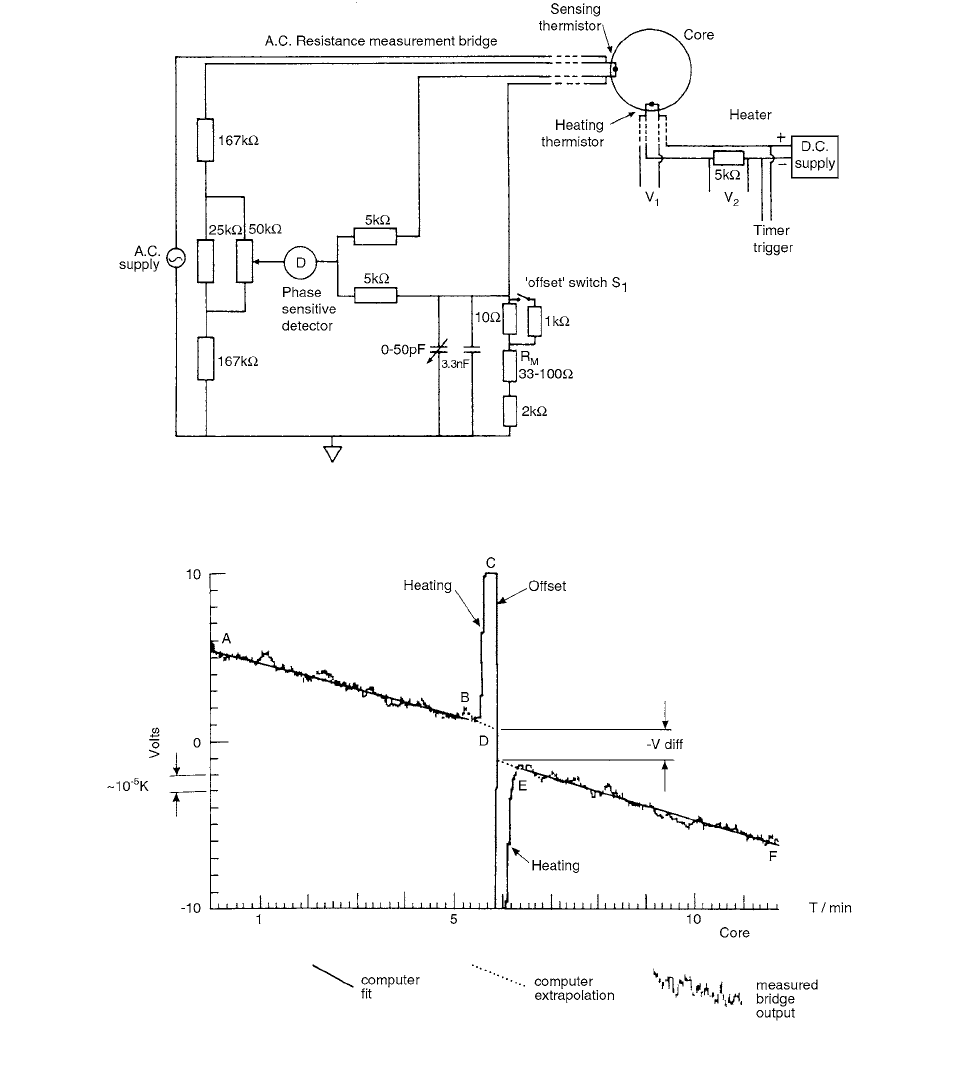
Figure 6.18 shows a typical measurement sequence.

The bridge ampliﬁer output voltages of the core and two

jackets are recorded for about 300 seconds (A to B). The

calorimeter is then irradiated (B to E), reducing the resis-

tance of the sensing thermistors embedded in the core and

two jackets and increasing their bridge output voltages.

Irradiations last for about 80 seconds. Midway through

the irradiation time (at C), the switch, S1, is closed, reduc-

ing the resistance of that arm of the core bridge by a small

ﬁxed amount (i.e., 0.1 ). Simultaneously, the balancing

resistances of the ﬁrst and second jacket bridges are

reduced to offset their bridge output voltages below their

balance points. The irradiation continues to heat the cal-

orimeter, increasing the bridge output voltages. The irra-

diation is stopped (at E) when the core bridge output

voltage returns to the pre-heating balance voltage (at B).

The output voltages of all the bridges then level off and

are recorded for a further 300 s (E to F) after the heating.

The dose the core would have received if it had been

entirely graphite, D

, can be calculated from [14] as

(6.21)

where m

is the mass of graphite, m

is the mass of the non-

graphite impurity identiﬁed by the subscript i, n is the num-

ber of non-graphite impurities, D

is the average absorbed

dose in graphite, and D

is the average absorbed dose in the

impurity labeled i. The ratio of the doses D

is sufﬁ-

ciently near unity and the impurities are so small for this

calorimeter that the effective mass of the core m was taken

(for all beams and energies) as i.e.,

1.5043 g  0.05%.

FIGURE 6.16 Schematic diagram of the NPL calorimeter. Side-view cross section. Not to scale. Dimensions in mm. (From Reference

[14]. With permission.)



------

i1















i1

,

CH-06.fm Page 343 Friday, November 10, 2000 12:02 PM

344 Radiation Dosimetry: Instrumentation and Methods

IV. OTHER MATERIAL CALORIMETERS

The thermal defects of A-150 plastic and graphite refer-

enced to aluminum were determined by Schulz et al. [15]

for 800-keV protons scattered by a 2-



m nickel foil (mean

transmitted energy  550 keV). Composite cores of A1/

A-150, graphite/A-150, and Al/graphite which could be

irradiated from one side or the other were employed. The

temperature increase of a core caused by 30–100 s of

irradiation (3–6 nA of proton beam current) was detected

by two thermistors mounted in opposite legs of a Wheat-

stone bridge. The thermal defect of A-150 plastic was

determined to be 0.0421  0.0036 (SE) referenced to

aluminum and 0.0402  0.0034 referenced to graphite.

The thermal defect of graphite referenced to aluminum is

0.0043  0.0034. No change in the thermal defect of

A-150 plastic was detected for accumulated doses up to

8  Gy.

FIGURE 6.17 Schematic diagram of electrical circuits. (From Reference [14]. With permission.)

FIGURE 6.18 Plot of bridge output voltage against time. (From Reference [14]. With permission.)

CH-06.fm Page 344 Friday, November 10, 2000 12:02 PM

Calorimetry 345

A key consideration in the application of tissue-

equivalent materials, solids, or liquids to absorbed-dose

calorimeters is the extent to which energy absorbed from

ionizing radiations is converted to heat. Speciﬁcally, the

thermal defect of a material is deﬁned as the expected

temperature change minus the observed temperature

change, the difference then divided by the expected tem-

perature change; positive thermal defects indicate endo-

thermicity, while negative thermal defects indicate exo-

thermicity. [15]

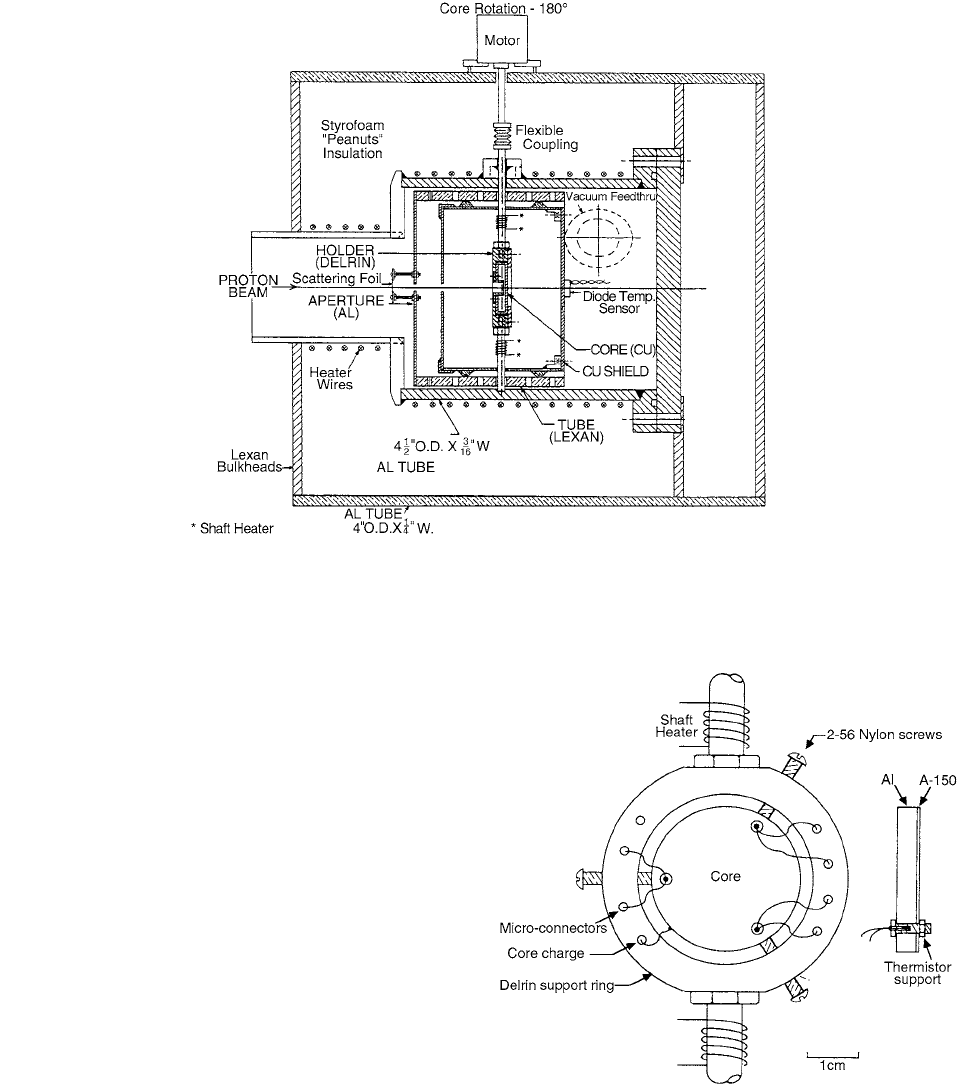
A ﬂat, cylindrical absorber (the core), one side of

which is the reference material, is mounted so that it can

be rotated through 180° (about a diameter passing through

its mid-thickness) and irradiated from one side or the

other. As the temperature rise caused by irradiation

depends on the heat capacity of the composite core, a

temperature rise caused by irradiation of the test material

which differs from that for the reference materials is indic-

ative of a thermal defect. A cross-sectional drawing of the

thermal-defect calorimeter is shown in Figure 6.19, and a

more detailed drawing of the core support and A-150/

aluminum core is shown in Figure 6.20. Each core has

three holes in its periphery to accept three thermistors,

two of which were connected to opposite legs of a Wheat-

stone bridge and the third used as an ohmic heater for

system testing. Because the thermal diffusivity of A-150

plastic (2.7  10

3

1

) is much lower than aluminum

(4.1 cm

1

) or graphite (4.6 cm

1

), the A-150 absorber

was made about 0.4 mm thick.

The core assembly was surrounded by an insulated cop-

per shield which is connected in series to an electrometer

and a high-voltage power supply. With this arrangement,

FIGURE 6.19 The thermal-defect calorimeter. Vacuum on the order of 10

3

Pa is provided by the accelerator beam-line diffusion

pump. The proton valve/beam-centering device is mounted directly to the left side of the calorimeter as shown above. (From

Reference [15]. With permission.)

FIGURE 6.20 The A-150 plastic/aluminum core and the core

support assembly. (From Reference [15]. With permission.)

CH-06.fm Page 345 Friday, November 10, 2000 12:02 PM

346 Radiation Dosimetry: Instrumentation and Methods

the bias applied to the shield can be varied between 500 V

and the shield charge recorded. The entire calorimeter is

maintained at 300 K by electrical heating wires wrapped

around its body. The calorimeter is suspended at the center

of a 25-cm OD aluminum tube.

The thermal defect was calculated from

(6.22)

where

d is the chart recorder deﬂection and Q is the sum

of core and shield charges.

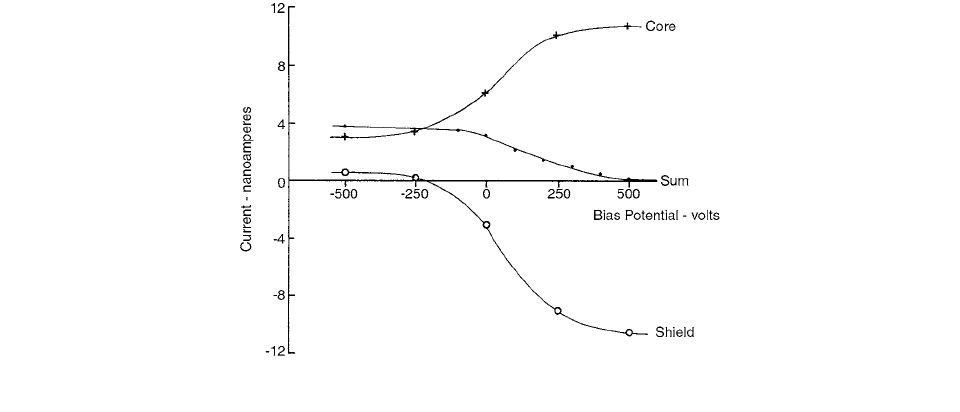
The effect of shield bias potential on the charge

collected from the core and shield was tested with the

aluminum/graphite core, and the results for aluminum

using potentials in the range

500 V are shown in

Figure 6.21. Data similar to those shown in Figure 6.21

were also measured for graphite and, although different

values for the sums of core and shield charges were

obtained for zero and positive shield bias, essentially

the same values as for aluminum were obtained when

the shield was negative. All of the thermal-defect data

reported below were obtained with negative 500 V

applied to the shield.

An experimental setup was described by Brede et al.

[16] which allows the calorimetric determination of the

heat defect of solids and ﬂuids relative to that of gilded

copper, which was used as the reference material. The

calorimeter operated in a quasi-adiabatic mode and was

suitable for protons, deuterons,



particles, and other

heavy ions with energies above 5 MeV. Corrections have

been made for secondary electron emission and also for

heat losses due to the temperature gradient on the surface

of the calorimeter core.

The heat defect,

h, describes that fraction of the absorbed

energy which does not result in a temperature change of the

absorber. It is deﬁned by the equation

(6.23)

where

represents the energy absorbed and W

is the

energy that appears as heat.

In mixed neutron-photon ﬁelds, the absorbed dose to

water is generated by secondary electrons and charged

particles such as protons, deuterons,



particles, and recoil

oxygen nuclei. The various particles produce markedly

different ionization densities along their tracks, and this

can be described by their linear energy transfer, LET.

Knowledge of the LET dependence of the heat defect in

water is therefore a prerequisite for the water calorimeter,

in order to reduce the main component of the uncertainty

in water calorimetry and to apply a correction factor in

mixed neutron-photon ﬁelds.

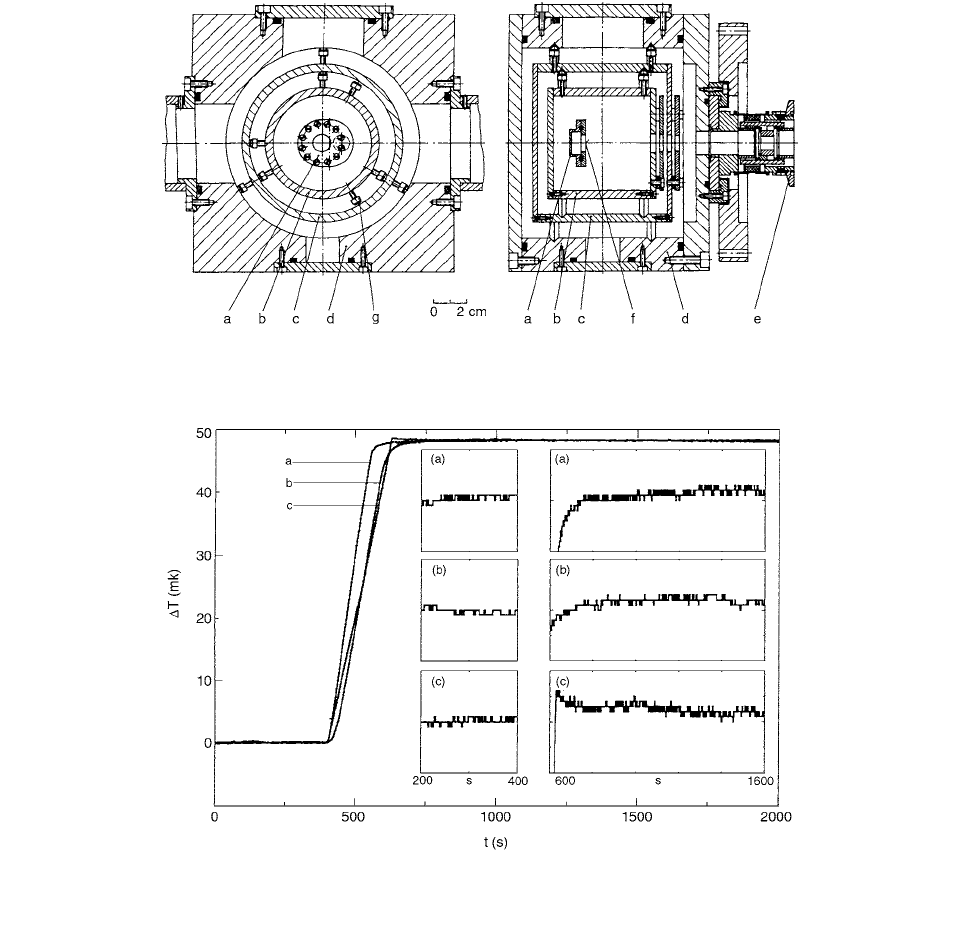
The material under investigation—in this case A-150

plastic or water—was contained in a cylindrical canister

of gilded copper with an inner diameter of 15 mm and a

length of 5 mm and which has an eccentric beam stop (see

Figure 6.22). Two different A-150 plastic absorbers from

different production lots were investigated. All samples

had the same geometrical dimensions—a cylindrically

shaped probe, 3.5 mm in thickness and 15 mm in diameter.

They were pressed into the canister. A 7-



m-thick indium-

sealed molybdenum entrance window allowed charged

particles to enter the core.

The calorimeter (Figure 6.22) comprised a core, inner

and outer jacket, and vacuum chamber which were ther-

mally and electrically insulated from one another and

operated in an adiabatic mode. The temperature increase,

T

, (i indicates either A-150 or water) measured on the

FIGURE 6.21 The effect of shield bias on the current collected by the core and shield. (From Reference [15]. With permission.)

d Q

ref

d Q

test

()

dQ

ref

------------------------------------------

()





CH-06.fm Page 346 Friday, November 10, 2000 12:02 PM

Calorimetry 347

core surface is given by

(6.24)

where

C is the total heat capacity of the calorimeter

core, h

is the heat defect in either A-150 plastic or

water, Q

is the total beam charge deposited in the core,

and U

is the voltage which is used to accelerate the

particles; i.e., the product Q

is the total energy

imparted in the absorber.

The temperature measurements of the core and

jackets were made with cylindrically shaped ther-

mistors (NTC) 0.35 mm in diameter and 0.5 mm in

length (type 6331 from Philips) and were connected to

thin copper wires 0.06 mm in diameter and 30 cm in

length.

The calorimetric measurement started with an analysis

of the initial drift for about 400 s. After an irradiation time

of 200 s, the system equilibrated during a time of approx-

imately 500 s. During the next 500 s, the ﬁnal temperature

drift was recorded (see Figure 6.23). The temperature

increase was determined from the linear region of the

initial and ﬁnal drifts and was normalized to the beam

charge collected from the core. The measurements were

carried out alternately with gilded copper and water or A-

150 plastic as the beam stop. The quantity (

T

T

) was

determined from sequential measurements in order to

reduce systematic errors. [16]

FIGURE 6.22 Core (a); inner jacket (b); outer jacket (c); vacuum chamber (d); ﬂange connection to the beam line (e); entrance

window (f); and screws (g) to align the core with nylon cords (not shown). (From Reference [16]. With permission.)

FIGURE 6.23 Time (t) plotted against temperature (T) response of the calorimeter. Beam stop: A-150 plastic (a), water (b), and

gilded copper (c). The inserts on the left-hand side show the initial temperature drifts; the inserts on the right-hand side show the

response after the end of irradiation. The temperature scale of all inserts is 2 mK. (From Reference [16]. With permission.)

T

C 1 h

()

1



CH-06.fm Page 347 Friday, November 10, 2000 12:02 PM

348 Radiation Dosimetry: Instrumentation and Methods

REFERENCES

1. Domen, S. R., J. Res., NBS 87, 211, 1982.

Ross, C. K. and Klassen, N. V., Phys. Med. Biol., 41,

1, 1996.

Fleming, D. M. and Glass, W. A., Rad. Res., 37, 316,

1969.

Selbach, H. J. et al., Metrologia, 24, 341, 1993.

Ross et al., Metrologia, 29, 59, 1992.

Schulz, R. J. et al., Med. Phys., 14, 790, 1987.

Domen, S. R., J. Res. Natl. Inst. Stand. Tech., 99, 121,

1994.

Schulz, R. J. et al., Med. Phys., 18, 1229, 1991.

Seuntjens, J. et al., Phys. Med. Biol., 38, 805, 1993.

10.

Palmans, H. et al., Med. Phys., 23, 643, 1996.

11.

Palmans, H. et al., Phys. Med. Biol., 44, 647, 1999.

12.

Guerra, A. S. et al., Phys. Med. Biol., 41, 657, 1996.

13.

Burns, D. T. and Morris, W. T., in Proc. High Dose

Dosimetry for Radiation Protection,

IAEA, 1991, 123.

14.

DuSautoy, A. R., Phys. Med. Biol., 41, 137, 1996.

15.

Schulz, R. J. et al., Phys. Med. Biol., 35, 1563, 1990.

16.

Brede, H. J. et al., Rad. Prot. Dos., 70, 505, 1997.

17.

ICRU49, stopping powers and ranges for protons and

alpha particles, 1973.

CH-06.fm Page 348 Friday, November 10, 2000 12:02 PM

349

Chemical Dosimetry

CONTENTS

References .......................................................................................................................................................................359

The major advancement of the last decade in chemical

dosimetry is the development of the gel dosimeter. This

is actually an application of a physical phenomenon for

better utilization of the well-known Fricke dosimeter. The

present chapter mentions in brief some of the contributions

made to the use of the already known chemical dosimeters.

Chapter 9 is devoted to gel dosimetry.

When dose is measured in water irradiated by an elec-

tron beam, the absorbed dose measured with the plane-

parallel chamber in the polystyrene phantom can be cor-

rected to obtain the dose to water, according to the equation

(7.1)

where the depth in water

water

is related to the depth in the

medium

med

(7.2)

where is the ratio of the mean unrestricted mass

collision stopping power in water to that in the medium;

is the ﬂuence factor, i.e., the ratio of electron ﬂuence

in water to that in the solid phantom;

is the depth of the

50% ionization; and

eff

is the effective density of the

medium and is discussed in the AAPM TG 25 protocol,

where recommended values of

eff

and are given.

An independent method of absolute dosimetry is the

use of the ferrous sulphate dosimeter. The Fricke solution

can be prepared by combining 1 mmol/l ferrous ammo-

nium sulphate with 1 mmol/l sodium chloride and 0.4 mol/l

sulfuric acid in double distilled water. [1] When the solu-

tion is irradiated, the ferrous ions Fe



are oxidized by

radiation to ferric ions Fe



. The oxidation of the ferrous

ions is directly proportional to the absorbed dose. The

ferric-ion concentration can be measured by the change

in the absorbance using a spectrometer.

The absorbed dose to the dosimeter solution,

fricke

can be calculated according to the equation

(7.3)

where



is the increase in the absorbance due to irradia-

tion at a temperature

during the spectrophotometric mea-

surement;



is the density of the Fricke solution (





1.024



/kg/ );

is the length of the light path in the

photometer cell (

is generally 0.01 m);



is the molar

absorption coefﬁcient for the ferric ions at temperature

;

and is the radiation chemical yield of ferric ions at the

irradiation temperature . For all the electron beam ener-

gies, an



value of 352



k G was

adopted from ICRU Report No. 35 [9] at an irradiation

temperature of 25°C and a spectrophotometer measure-

ment temperature

of 25°C. A correction for the temper-

ature

differing from 25°C was made according to the

equation

(7.4)

where the temperature coefﬁcient

, is approximately

0.0007° and is 0.0015° . Using the mass stop-

ping power ratio

W,Fricke



to convert the dose to

the ferrous sulphate solution,

Fricke

, to the dose to water,

water

, Equation 7.3 becomes

(7.5)

The ratios of the values of the absorbed dose to water

determined with the Exradin chamber, calibrated using all

three different calibration methods and the Fricke dosim-

etry, as a function of the mean energy at depth,

, are

shown in Figure 7.1.

As mentioned above, gel dosimetry is discussed in

Chapter 9. Because of the strong afﬁliation of that technique

to chemical dosimetry, an example is given here. The

nuclear magnetic resonance (NMR) longitudinal relaxation

water

()D

med

()S



[]

med

water



med

water



water

med



eff

 d

med

water

R

mef

()



()[]

med

water



med

water



med

water

fricke

A



l

t

------------------------



t

2

1

t



t



m25C

25C

1 k

25 t()[]

25 t()[]

1

1.004

water

A



l

--------------------



278.54A

1 0.007 25 t()[]1 0.0015 25 t()[]

----------------------------------------------------------------------------------------------------------

CH-07.fm Page 349 Friday, November 10, 2000 12:03 PM