Salby M.L. Fundamentals of Atmospheric Physics

Подождите немного. Документ загружается.

480

Atmospheric Waves

14.8. Construct a wavepacket of surface water waves r/'(x, t) from a Gaussian

spectrum of wavenumbers centered at k0 and of spectral width

dk:

(k-ko)2

~ql, =/c~/2"n'-'=---d -'~e

2d~2

14.9.

(a) Use this expression to produce a counterpart of (14.22). (b) Sketch

the wavepacket for given k0 and

dk.

for the group velocity in the limit

dk ~ O.

On approach to JFK Airport in New York, winds are gusting nearly

along the shoreline. Yet, whitecaps are observed to approach the shore

virtually head on. Construct a simple model based on shallow water

waves propagating in the

x-y

plane, with depth decreasing linearly to

zero at the shoreline, which coincides with y = 0. For a wavepacket

characterized initially by k = (k0, 10), (a) determine

k(y),

(b) sketch

phase lines corresponding to successive positions of the wavepacket for

l 0 > 0, and (c) plot rays that are initially oriented 60 ~ and 30 ~ from the

shoreline.

14.10.

14.11.

14.12.

14.13.

14.14.

14.15.

14.16.

14.17.

14.18.

Obtain the dispersion relation (14.34) for acoustic-gravity waves.

(Hinu

What condition must be satisfied for a homogeneous system of linear

equations to have nontrivial solution?)

Show that the dispersion relation for acoustic-gravity waves reduces to

that for sound waves in the limit of high frequency and short wave-

length.

Show that, at a given elevation, external gravity waves perform no net

work on the overlying column during a complete cycle and therefore

transmit no energy vertically. You may restrict the analysis to simple

gravity waves in the shortwave limit.

Lenticular clouds (Chapter 9) form preferentially during winter. Ex-

plain their seasonality in relation to conditions favoring gravity waves.

Recover the limiting dispersion relation for gravity waves (14.35.2) by

invoking incompressibility.

Derive the group velocity (14.44) for simple gravity waves.

Demonstrate that phase lines of a stationary gravity wave forced at

the surface in mean westerlies (e.g., undulations of isentropic surfaces)

must slope westward with height.

Show that the flux of zonal momentum transmitted vertically by simple

gravity waves,

poU'W',

is positive (negative) if their group velocity is

upward and they propagate eastward (westward).

Show that wave activity for simple gravity waves propagates vertically

one vertical wavelength for each horizontal wavelength that it propa-

gates horizontally.

Problems

481

14.19.

14.20.

14.21.

14.22.

14.23.

14.24.

14.25.

For gravity waves with horizontal and vertical wavenumbers k and m,

respectively, describe the limiting behavior of phase and group propa-

gation for (a)

m/k --+ O,

(b)

m/k --+ -oc.

The Manti-La Sal range in southern Utah comprises an isolated ridge

that has a characteristic width of 10 km and is oriented N-S. If winds

blow from the SW, the range's N-S extent is much longer than 10 km,

and static stability is characterized by

N 2 ----

2.0

x 10 -4 S -z,

(a) at what

polar angle are lenticular clouds likely to be found? (b) What will be

their orientation relative to the range?

Verify that (14.41) satisfies the Taylor-Goldstein equation within the

framework of the WKB approximation.

A gravity wave of zonal wavenumber k = 0.5 km -1 propagates vertically

through mean westerlies of uniform positive shear

= T 0 + Az,

with ~0 - 10 m s -1, A = 0.1 m s -1 km -1, and constant static stability

N 2 -- 2.0 x 10 -4 s -2.

In the framework of the Boussinesq and WKB

approximations, sketch the profiles of intrinsic frequency and vertical

wavenumber if the gravity wave (a) is stationary, (b) propagates west-

ward at 10 m s -1, and (c) propagates eastward at 10 m s -1.

Describe the physical circumstances under which reflection may be ig-

nored and the WKB approximation is a valid description of wave prop-

agation.

A stationary gravity wave of the form

w'(x, z) - W(z)e il'x

is excited by flow over elevated terrain. The horizontal and vertical

scales are short enough for wave propagation to be controlled by local

conditions. If the upstream flow ~ is independent of height but the

stratification varies as

(a) characterize the propagation according to levels where wave activ-

ity is vertically propagating and external, (b) sketch the vertical wave

structure, and (c) determine the range of ~ for which wave activity is

trapped at the surface.

(a) Show that, in the limit of no rotation and weak dissipation, vorticity

is a conserved property for incompressible gravity waves and is there-

fore rearranged by the motion. (b) In light of this feature, describe the

limiting behavior (t --+ ec) of 0 and ~ anticipated near a gravity wave

critical level, where the motion assumes the form of a cat's-eye pattern

(Fig. 14.22).

482

14 Atmospheric Waves

14.26. Show that, under the Boussinesq approximation, the linearized conti-

nuity equation has the counterpart

Dt~' dln~

-- + w~ - 0,

Dt Oz

where ~3'-

p'/-~.

14.27. A gravity wave of wavenumber k - (k, m) propagates into a region

where potential temperature varies with height as

0 (Z- Z0) 2

0 0 H 2 ,

with H - const. (a) Describe propagation in the

x-z

plane, sketching

the corresponding ray. (b) Sketch the vertical structure of the wave.

14.28. Flow over oscillating terrain excites a gravity wave of horizontal

wavenumber k - 0.5 km -1 that propagates horizontally and verti-

cally away from its source region at (x, z) = (0, 0). If the wave can

be treated within the Boussinesq approximation, the background flow

varies vertically as

14.29.

14.30.

- K0 + Az,

where ~0- 10 m s -1 and A = 1.0 m s -1 km -a, and

N 2 --

2.0

x 10 -4 s -2

= const, (a) plot the ray for a steady wave, (b) plot rays for an un-

steady spectrum of wave activity (e.g., generated by low-level flow that

fluctuates with time) of the form

exp(--o-2/2]~2),

with E - (2 hr) -1, for

frequencies ~r - 0, +E, and +2E, and (c) contrast the behavior for

E ~ ~ against that for steady forcing: E --+ 0.

Within the framework of the Boussinesq approximation, consider

inertio-gravity waves,

which are buoyancy waves with horizontal scales

long enough to be influenced by rotation. (a) Write down a set of

equations governing such motion on an f-plane. (b) Show that these

waves satisfy the dispersion relationship

m 2 N 2 _ w 2

k 2 092 _ f2'

which is a generalization of (14.36) and illustrates that inertio-gravity

waves propagate vertically only for Iw[ > Ill. Atmospheric tides, which

are generated by diurnal variations of heating, are inertio-gravity waves.

Estimate the range of latitude for vertical propagation of (c) the diurnal

tide, o- = 1.0 cpd, and (d) the semidiurnal tide, o- = 2.0 cpd.

Barometric registrations following the eruption of Krakatoa revealed

an oscillatory disturbance in surface pressure having an amplitude of

1 mb. If the period of the oscillation was 1 hr, estimate the amplitude

of (a) the pressure perturbation at 80 km and (b) the wind perturbation

at 80 km.

Problems

483

14.31. Show that the Lamb mode is the only nontrivial solution to the homo-

geneous boundary value problem (14.60).

14.32. Consider hydrostatic perturbations to a motionless isothermal atmo-

sphere that are introduced by unsteady vertical motion at the surface.

If the surface forcing is indiscriminate, with the power spectrum of

white noise,

I Wff

L 2 - const,

(a) determine the wave response in terms of the power spectrum of

pressure at some level and (b) for a particular wavenumber, sketch the

power spectra of forcing and response as functions of frequency.

14.33. Show that the power spectrum of white noise is recovered from (a) the

impulse

1 t2

w(t)

= ~e 2r2

in the limit r ~ 0 and (b) a superposition of such impulses at random

times

tj.

timescale for unsteadiness.

14.34.

Interpret the Lamb waves satisfying (14.61) in light of shallow water

waves.

14.35.

14.36.

14.37.

14.38.

14.39.

14.40.

Derive the vertical structure equation (14.59).

Show that the Lamb mode also satisfies the equations governing non-

hydrostatic motion.

Derive the thermodynamic equation (14.33.4).

Discuss how propagation in Figs. 14.14 and 14.15 would be modified

by realistic variations of N 2.

Demonstrate that Rossby wave propagation is restricted to intrinsic

frequencies smaller than 2f~.

See Problem 12.51.

14.41.

Consider bartropic nondivergent motion on a beta plane positioned at

latitude ~b 0, which is periodic in x, over length X, and unbounded in y.

Steady zonal flow ~ over an isolated mountain excites Rossby waves that

radiate away from the topographic feature. The effect of the mountain

may be treated as a vorticity source

( 2+y2 t

e(x,y)- o0

exp-~

2zrL 2 2L 2 9

(a) Provide a rationale for treating surface forcing in this manner.

(b) Write down an equation governing the motion. (c) Obtain an ap-

proximate solution for the wave field, subject to the condition of radia-

tion away from the source region, by (1) expressing the streamfunction

484

Atmospheric Waves

14.42.

14.43.

14.44.

14.45.

14.46.

14.47.

14.48.

14.49.

in terms of the Fourier transform and inverse:

K dlalkrslei(ksx+ly )

O(x, y) = 2,rrX s=-

%=fx/2

dy ~(x, y)e -i(k'x+ly),

,I -X/2 oo

217"

ks =s-~- s = 0, +1, +2,...,

which apply to semiperiodic geometry, and (2) treating propagation

independently in the positive and negative y half-planes. (d) Plot the

wave field for 4~0 = 45~ u = 10 m s -1, X = 2~ra cos 4~0, and

L/X =

1/4Ir.

In terms of the vorticity budget (12.36), discuss how volume heating

excites planetary waves.

Derive the group velocity for quasi-geostrophic Rossby waves (14.70).

Show that stationary planetary wave activity generated by westerly flow

over elevated terrain will be found downstream of its topographic forc-

ing.

Derive the Helmholtz equation governing propagation of quasi-

geostrophic planetary waves through sheared zonal flow (14.76).

Consider quasi-geostrophic motion in spherical geometry. (a) Provide

an equation governing the propagation of planetary waves in lati-

tude and height. For a stationary wave that is invariant with height,

O0'/3z

= 0, and propagates horizontally through uniform westerlies

of 20 m s -1, estimate the polar turning latitude for (b) zonal wave-

number 1 and (c) zonal wavenumber 4.

In light of Problem 14.46, describe the wave propagation that could

be established with a source at midlatitudes if potential vorticity is

homogenized at low latitudes by horizontal mixing (See. 14.7).

Steady flow over oscillating terrain at 45 ~ latitude excites a Rossby wave

of zonal wavenumber k that propagates zonally and vertically. If the

undisturbed motion,

K(z) - u0 + Az,

with u0 = 10

m s -1

and A = 0.05

m s -1 km -1,

varies slowly enough

for wave propagation to be treated locally, (a) plot the refractive index

squared as a function of height, (b) sketch the vertical wave structure

for k = 1-3, (c) plot rays emanating from the source at (x, y) = (0, 0)

for wavenumbers k = 1-3, and (d) describe vertical propagation of a

broad spectrum of stationary wave activity generated near the surface.

Under the conditions of Problem 14.48, but for A = -0.05 m s -1

km -1, k - 2, and in the presence of linear dissipation (i.e., Rayleigh

14.50.

14.51.

14.52.

Problems

485

friction and Newtonian cooling) with a timescale of 10 days, (a) plot

the refractive index squared as a function of height, (b) plot the vertical

structure of the wave, (c) plot the ray emanating from the wave source.

(d) What condition(s) must be satisfied for the behavior to remain

linear?

Show that propagation of quasi-geostrophic planetary waves in the pres-

ence of radiative dissipation is governed by (14.80).

Consider a gravity wave with potential temperature amplitude O(z)

propagating in a region of mean potential temperature

O(z)

and static

stability

N2(z).

(a) Determine the vertical wavenumber m at which the

wave will break. (b) Express the breaking wavenumber in terms of the

wave's horizontal wavenumber and phase speed to derive an expression

governing the breaking height. (c) If the wave's forcing is maintained

below, describe the propagation anticipated in the limit t --~ oo.

Under barotropic nondivergent conditions, evaluate the time for a

Rossby wavepacket to reach its critical line at y = 0 from y > 0,

where the mean flow varies as

g-c(1 +Ay).

14.53. Within the framework of the WKB approximation, consider a baro-

tropic nondivergent Rossby wave of wavenumber k and phase speed

c, which propagates on a beta plane toward its critical line, where the

mean flow behaves as

K=c(1 +Ay)

with A - 0.01 km -1, and in the presence of small linear dissipation

e- O.01kc. (a) Plot the streamfunction over one wavelength. (b) Plot

successive positions of the material contour that coincides initially with

y - 0. (c) Reversal of the potential vorticity gradient,

oQ/oy < O,

provides a condition for dynamical instability (Chapter 16). From ad-

vection of the material contour in (b), determine if, when, and where

this condition is met.

14.54. Associate the breaking of gravity waves with the breaking of Rossby

waves in terms of analogous properties and behavior.

Chapter 15

The General Circulation

Thermal equilibrium requires net radiative forcing to vanish for the earth-

atmosphere system as a whole. Although it applies globally, this requirement

need not hold locally. Net radiation (Fig. 1.29c) implies that low latitudes

experience radiative heating, whereas middle and high latitudes experience

radiative cooling. To preserve thermal equilibrium, that radiative heating and

cooling must be compensated by a mechanical transfer of heat from tropical

to extratropical regions. The simplest mechanism to transfer heat meridionally

is a steady zonally symmetric Hadley cell. This thermally direct overturning

(Sec. 1.5), can be driven by heating in the tropics and cooling in the extratrop-

ics, which make air rise and sink across isentropic surfaces. Although steady

zonally symmetric motion can balance radiative heating and cooling, the ob-

served circulation is more complex (Fig. 1.9).

How meridional heat transfer is actually accomplished in the atmosphere is

influenced profoundly by the earth's rotation. Net radiation is almost uniform

in longitude, so it tends to establish a steady zonally symmetric thermal struc-

ture with temperature and isobaric height decreasing poleward. Since contours

of isobaric height are then parallel to latitude circles, geostrophic equilibrium

requires steady motion to be nearly zonal--virtually orthogonal to the simple

meridional overturning hypothesized above. That zonally symmetric circula-

tion is also virtually orthogonal to the temperature gradient (a reflection of

the geostrophic paradox; Sec. 12.1), so it cannot transfer heat poleward ef-

ficiently. The meridional temperature gradient must then steepen until heat

transferred meridionally by the zonally symmetric Hadley cell balances radia-

tive heating in the tropics and cooling in the extratropics.

This produces a meridional gradient of zonal-mean temperature that is

much steeper than observed (e.g., such as that resulting under radiative-

convective equilibrium in Fig. 10.1). Thermal wind balance (12.11) then implies

a strong zonal jet aloft. The speed of this jet can be inferred from conserva-

tion of specific angular momentum (u + lIa cos ~b)a cos ~b (11.29) for individ-

ual air parcels drifting poleward in the hypothetical Hadley cell. An air parcel

that is initially motionless at the equator then assumes a zonal velocity at

486

15.1 Forms of Atmospheric Energy 487

latitude 4~ of

sin 2 4)

u=D.a~

COS ~ '

which predicts wind in excess of 300 m s -1 at 45 ~

Discrepancies from observed zonal-mean behavior follow from the absence

of zonally asymmetric eddies, which completely alter the extratropical circu-

lation. Those asymmetric motions transfer heat poleward far more efficiently

than can a zonally symmetric Hadley cell in the presence of rotation. In-

trinsically unsteady, extratropical disturbances are fueled by reservoirs of at-

mospheric energy and exchanges among them, which maintain the general

circulation.

15.1 Forms of Atmospheric Energy

From the time it is absorbed until it is eventually rejected to space, energy

undergoes a complex series of transformations between three basic reservoirs:

1. Thermal energy, which is represented in the temperature and moisture

content of air.

2. Gravitational potential energy, which is represented in the horizontal

distribution of atmospheric mass.

3. Kinetic energy, which is represented in air motion.

Transformations from thermal and potential energy to kinetic energy are re-

sponsible for setting the atmosphere into motion and maintaining the circula-

tion against frictional dissipation.

15.1.1 Moist Static Energy

The thermal energy of moist air has two contributions: Sensible heat content

reflects the molecular energy of dry air, which is the dominant component of

moist air. Latent heat content reflects the energy that can be imparted to the

dry air when the vapor component condenses. Both are represented in the

specific enthalpy (Sec. 5.3)

h = cpT + lvr + h o.

(15.1)

Here,

cpT

measures

the sensible heat content of a moist parcel, whereas

lvr

measures its latent heat content.

The first law (2.15) implies that the specific enthalpy of an individual air

parcel changes according to

dh dp

a-d- ~ - qnet, (15.2.1)

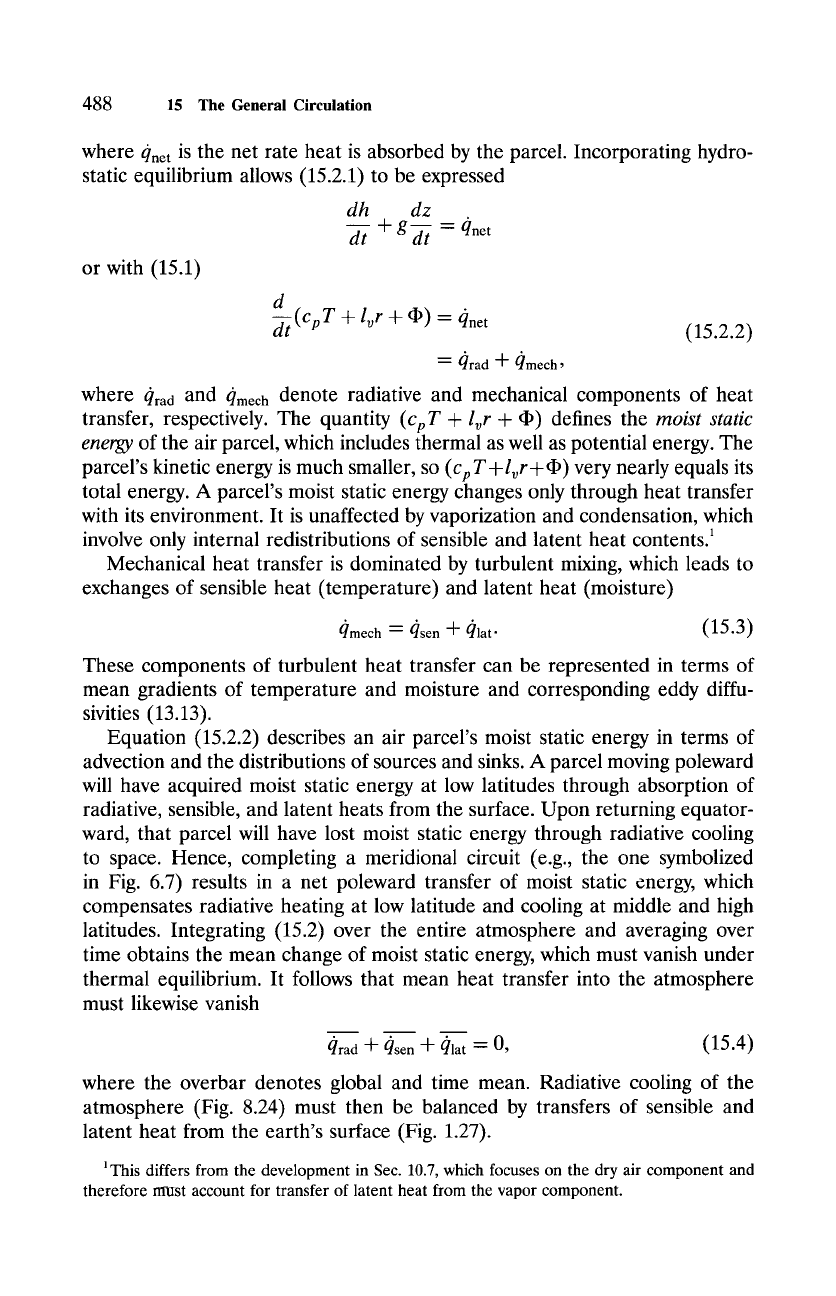
488

The General Circulation

where

qnet

is the net rate heat is absorbed by the parcel. Incorporating hydro-

static equilibrium allows (15.2.1) to be expressed

or with (15.1)

dh dz

dt -t- g ~- - r

dt(CpT + l,r + alp)

qnet

(15.2.2)

= r + qmech,

where

qrad

and

l)mec h

denote radiative and mechanical components of heat

transfer, respectively. The quantity

(cpT + lvr + ~)

defines the

moist static

energy

of the air parcel, which includes thermal as well as potential energy. The

parcel's kinetic energy is much smaller, so

(cpT+l~r+dp)very

nearly equals its

total energy. A parcel's moist static energy changes only through heat transfer

with its environment. It is unaffected by vaporization and condensation, which

involve only internal redistributions of sensible and latent heat contents. 1

Mechanical heat transfer is dominated by turbulent mixing, which leads to

exchanges of sensible heat (temperature) and latent heat (moisture)

l)mech -- 0sen -Jr- qlat" (15.3)

These components of turbulent heat transfer can be represented in terms of

mean gradients of temperature and moisture and corresponding eddy diffu-

sivities (13.13).

Equation (15.2.2) describes an air parcel's moist static energy in terms of

advection and the distributions of sources and sinks. A parcel moving poleward

will have acquired moist static energy at low latitudes through absorption of

radiative, sensible, and latent heats from the surface. Upon returning equator-

ward, that parcel will have lost moist static energy through radiative cooling

to space. Hence, completing a meridional circuit (e.g., the one symbolized

in Fig. 6.7) results in a net poleward transfer of moist static energy, which

compensates radiative heating at low latitude and cooling at middle and high

latitudes. Integrating (15.2) over the entire atmosphere and averaging over

time obtains the mean change of moist static energy, which must vanish under

thermal equilibrium. It follows that mean heat transfer into the atmosphere

must likewise vanish

qrad -I- qsen -}- t)lat -- O,

(15.4)

where the overbar denotes global and time mean. Radiative cooling of the

atmosphere (Fig. 8.24) must then be balanced by transfers of sensible and

latent heat from the earth's surface (Fig. 1.27).

1This differs from the development in Sec. 10.7, which focuses on the dry air component and

therefore must account for transfer of latent heat from the vapor component.

15.1 Forms of Atmospheric Energy

489

Inside the boundary layer, turbulent diffusion of heat and absorption of

longwave (LW) radiation from the surface increase an air parcel's sensible

heat content. Absorption of water vapor from the surface increases its latent

heat content. Both increase the moist static energy of the parcel. Outside the

boundary layer and convection, turbulent diffusion is small enough to be ig-

nored. Radiative cooling then takes over as the primary diabatic process and

gradually depletes the parcel's store of moist static energy, which is subse-

quently replenished when it returns to regions of positive heat transfer.

15.1.2 Total Potential Energy

Thermal energy is reflected in the internal energy of dry air. Heat transfer

from the surface increases an atmospheric column's temperature and hence

its internal energy. The hypsometric relationship (6.12) then implies vertical

expansion of the column. Since it elevates the column's center of mass, a pro-

cess that increases the column's internal energy must also increase its potential

energy.

Consider a dry atmosphere in hydrostatic equilibrium and in which air

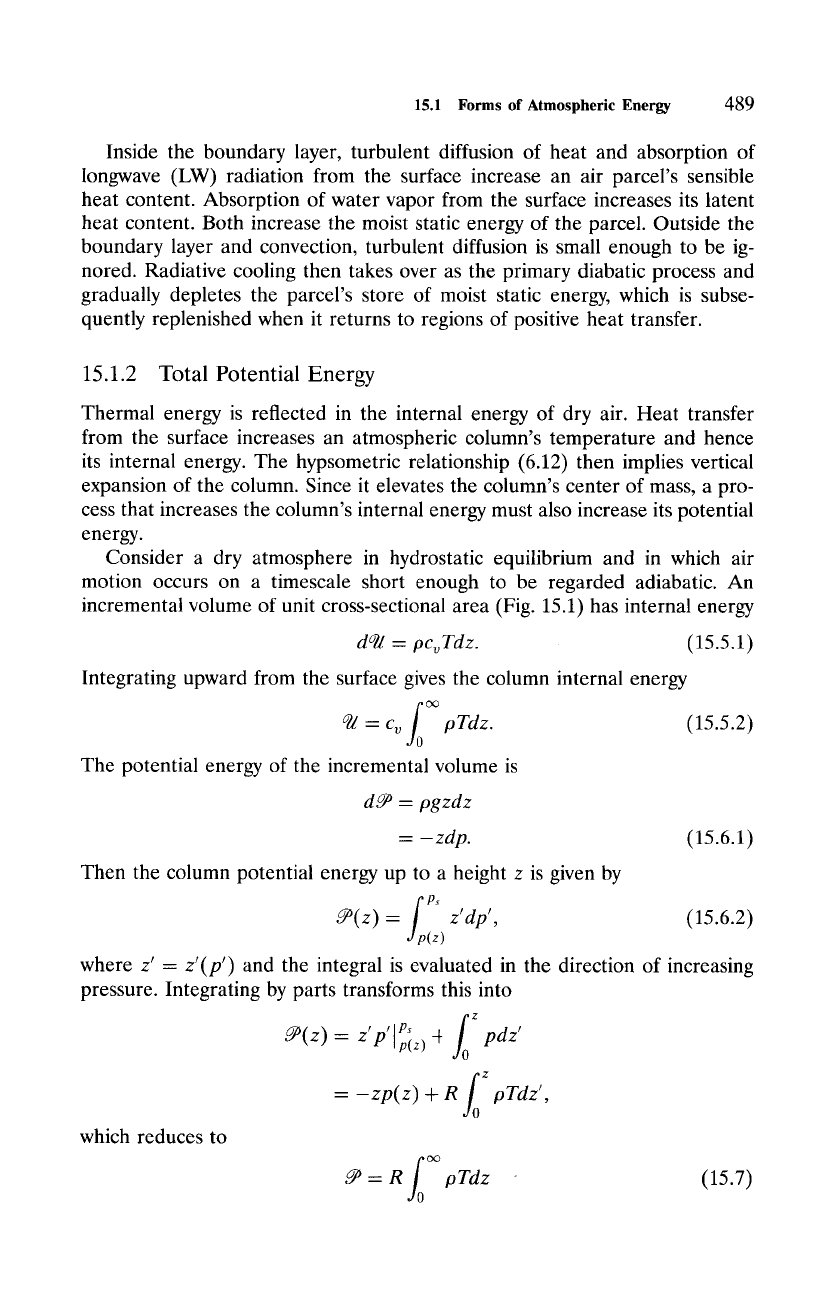
motion occurs on a timescale short enough to be regarded adiabatic. An

incremental volume of unit cross-sectional area (Fig. 15.1) has internal energy

d~ -- pcvTdz.

(15.5.1)

Integrating upward from the surface gives the column internal energy

~l - c v p Tdz.

(15.5.2)

The potential energy of the incremental volume is

d@- pgzdz

= -zdp. (15.6.1)

Then the column potential energy up to a height z is given by

~(z) - z'dp',

(15.6.2)

(z)

where

z' - z'(p')

and the integral is evaluated in the direction of increasing

pressure. Integrating by parts transforms this into

z'p'l p" fo

p(z) 4 pdz'

= -zp(z) + R prdz',

which reduces to

f0 X)

5 ~ - R pTdz

(15.7)