Raabe J. Hydro power - the design, use, and function of hydromechanical, hydraulic, and electrical еquipment
Подождите немного. Документ загружается.


~nass conscr~ation. Accordi~~g
to
Renau,
Jvhr~storr
and
Klblc
[5.41],
the pllenomcnn.
,,.hi&
happen in
:I
s\virl-free, plane and straight diffuser, can be c1;lssified
as
follo\~~s
eu,,jcd by Fig.
5.5.
l
b,
c:
-
~1,;~
figure shows
n
plot
of
lines wit11 constant diverging arigle
2
0
in
n
coordinate system
the
length
to
inlet area ratio LIA,
as
abscissa and the area ratio
A,/A,
as
the
ordinate. The types
of
flow regi~ne arc nearly indepcndcnt
of
the
Rejnoltls
number and
,,,
be described as follows.
close
to the line
2
@
=
0
there is a "steady" and "smooth flow regime". At larger divergence angles.
apcciaIly in long diffusers thc flow becomes "unsteady without stall". Furtlicr increase in
2
O
brillgs
in
I!~L'
reginle of unsymmetrical stall (Fig. 5.5.1 b, c). The main flow here follo\trs one wall and
it
ma:,
,ither wall. For very large values of
20
there is a jet flow regime.
Bt.t\tfcen both of the last regimes
a
hysteresis may be observed. Thus the regime of
a
diffuser (draft
,,,he) may depend also on the sequence
of
events.
AS
a
remed~l against stall due
to
the streamwise pressure gradient the cone
angle
of a
conical draft tube should not exceed about
7".
This holds true for
a
long diffuser with a
flow which is not swirling around the diffuser axis. Since the draft tube length stl-ongly
illflirences the excavation
cost
of
a
water turbine, remedies are required
to
increase the
vlilue
of
this
threshold.
One
such a remedy is the "bell-shaped" draft tube (Fig.
5.5.1
d).
Here in
a
conical diffuser.
the
cone angle of
7;
is exceeded in the inlet zone. This
is
possible, since the velocity profile
there
is
not
so
susceptible
to
stall
as it would
be
towards the outlet. Thereby
dirf
user
length and hence excavation cost may
be
econon~ized. Further studies on digusers see
15.42
to
5.501.
h
stall in the draft tube, even at higher diffuser angle can be avoided, when the flow rotates slightly
around the diffuser axis. Here the centrifugal force on the particle in the
flow
ou~side the boundary
layer causes
it
to replace a stalling fluid eleinent on the wall. liowever a too Ytrong rotation
of
t!ic
flow as
it
occurs under overload
in
rcaction turbines with fixed runner vanes, leads to a stalled region
along the centre line (Cap. 8.2) (5.511. Hcrc the tcndency of the rotating fluid \vith nearly constant
specific energy to form a free whirl with a high strain rate on the centre
line favours the formation
of
a
forced vortex there.
Moreover the low pressure in the vortex core leads to cavitation and may result in a back flow from
the draft tube outlet towards the hub (Cap.
8.2),
[5.2; 5.521.
Under part load the vortex core accepts
a
cork screw form (Cap. 8.2), 15.18; 5.53;
5.541
and precesses
with
a
slip of about
70%
against the speed of the runner. Stroboscopic observations by high speed
camera
demonstrate
clearly that the origin of it may be within the runner channel on the hub. Hence
the slip of this vortex relative to the runner against the sense of its rotational speed is an example
of
"rotating stall"
(5.1
8; 5.551.'
The laticr originates from a decrease of meridiocil velocity by local blockage of the flow due to the
vortex: core. The absolute flow direction being retained from the gate yields
via
the velocity triangle
an
impact of the relative inflow onto the suction face of the vane. This causes stall on the pressure
face, which sucks the vortcx: downstream by negative water hammer into the as yet unblocked
cllannel against the sense of rotation.
5.5.5.
Secondary
flow
in curved
and
rotating clucts
5-5.5.1.
Due to turbulcncc, consequcnces on energy conversion
Consider
a
planc flow through
a
straight channel, which rotates around
an
axis perpen-
dicular to
thc flow plane with angular velocity
o.
In
an
ideal steady flow the relative
velocity
\v
at
;I
station
n
distallcc
n
from the pressure hcc would incrc:l.sc from thc \la\ue
\I.,
on thc prcssurc Trice by thc rcl;\tivc whirl with
\c.
=
2(011
+
In
;I
rz:d flow
a
bo~rndary
layer
this increase of ,.olati\~c vclocily tow:~rcls the suction I'lcc of tllc channel
would be enhanced across thc bound:~ry Iilyer on thc pressklrc face and then
~~t~ti~~~~~
as before
up
to the outer edge of thc bottndary layer on the suction face of the
chnnne.
Consider now
an
element within the boundary layer of the pressure face,
011
a
stream-
line
1
with a time-averaged velocity w,. Thc elcmerit of volume
t11tclA (dtt
normal to the
channel wall,
dA
face normal to
rlrl)
dcvclops a Coriolis force in the
+
a
direction,
pointing from the pressure face towards the suction face
=
-
26),v,
gdnd~,
(Fig.
5.5.2
a).
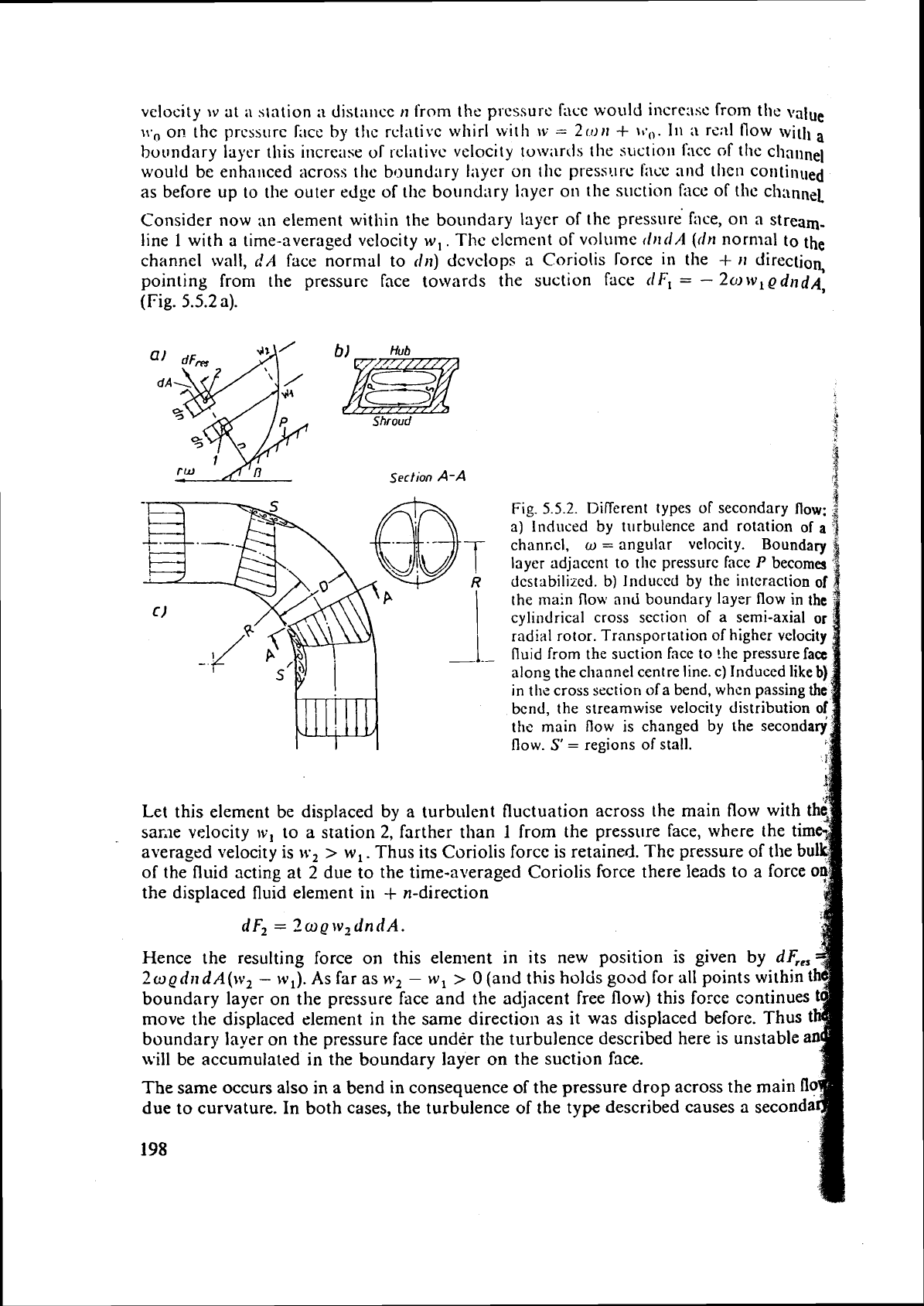
Shroud
r
IL)
-
section
A-A
Fig.
5.5.2.
Different types of secondary
flow;
a)
Induced by turbulence and rotation of
a
chan~el,
w
=
angular velocity. Boundary
T
layer sdjaccnt to the pressure face
P
becomes
R
dcstabilizcd. b) lntluced by the
interaction
of
the ma:n flow and boundary layer flow in
the
cylindrical cross section of
a
semi-axial
or
radial rotor. Transportation of higher velocity
I
fluid
from
the suction face to !he pressure face
-
--
alon~ the channel centre line. c) Induced
like
b)
in tl~c cross section
of
a
bend, when passing
the
bend, the streamwise velocity distribution
of
the main flow is changed by the secondary
flow.
S'
=
regions of stall.
Let this element be displaced by a turbulent fluctuation across the main flow with th
sar;le velocity
rv,
to
a
station
2,
farther than 1 from the pressure face, where the tim
averaged velocity is
w,
>
w,. Thus its Coriolis force is retained. The pressure of the bul
of the fluid acting at
2
due to the time-averaged Coriolis force there leads to
a
force
0
the displaced fluid element in
+
n-direction
dF,
=
2oe\vzdndA.
Hence the resulting force on this elenlent in its new position is given by
dF,,
2
w
Q~II
dA(wz
-
wl).
As
far as
w,
-
w,
>
0
(and this holds good for all points within
t
boundary Iayer on the pressure face and the adjacent free flow) this force continues
t
move the displaced element in the same direction as it
was
displaced before. Thus t
boundary layer on the pressure face under the turbulence described here is unstable a
will be accumulated in the boundary
layer on the suction face.
The
same occurs also
in
a bend
in
consequence of the pressure drop across the maill
fl
due to curvature. In both cases, the turbulence of the type described causes
a
seconda
198

n,,~
by
accumulating
botlndary
Izyer material from the pressure fncc of the channel
in
,jl,
boundary layer on the suction face. For basic considcrations on
the
gencrntion of
secondary flow, set. also the report of
J.
D.
Johnston
5).
In the channel of a rotor the cffccl due to Coriolis force i~sually prcdorninates. Hencc thc boundary
I;lyel. fiuid from the pressure face is accu~nulatcd along the boundary layer of the suction face iow;lrds
the
outlet of this channel. This phenomenon was observed by colour injection. Iiigh speed calncrn.
rotoscopc alld
transparent
shroud by
Fischer
and
Thornn
in
1930
15.561
in
a
pump impeller.
11
was rcconfirmcd
by
means of hot wire anemometry in a str;tight rotating channcl wit11
rectangular
cross section by
hloort
at
MIT
in
1962
[5.57].
The measurcmcnts of hfooi~
how
also thc rctclltion
of
the logarithmic velocity distribution at the wall in the decisive loss-generating zones at pressure
suction face.
~lthotrgh the energy relation for ideal flow postulates the velocity maximum shall be at the suction
race of the channel. the secondary flow induced by turbulence and othcr effects from the pressurc face
towards the suction face shifts the velocity maximum in the main flow
at
the outlet of
a
rotor channel
towards the pressure face. Sirnultancously the accumulation of bot~ndar? layer matcrial on thc
suction face towards the outlet of the channel tends to stall there at the rotor exit, rotating with blade
speed.
Will1 increasing streamwise channel length, this erect counteracts the energy conversion of a runner
(impeller) channel. One remedy consists
in
the choice of a short charinel together with an adcquate
number of rotor vanes. For the usual runner channel of turbines with forivard curved vanes, the
cffccts of curvature and Coriolis force are additive. For backward curved vilnes, as are employed in
purnps, pump-turbinzs and psrtially also in turbines, both effects act against each other. Howe\er,
a balance is not possible,
as
it
would annul the rotor's work.
As
was demonstrated by
Sirscheletzky,
also an ideal flow may have srailed zones in consequcnce of
the principle of least action.
In the rotor channel, the width of such a stalled zone could also ba pre-
dicted by
mans of this principle
[5.59].
A
criterion for the lninilnum vane number, t\hicli prcvcnt
stall, could be derived from this by the author
[5.59].
5.5.5.2.
Interaction
of
the
boundary layer and the
main
flow
Whereas the afcrementioned secondary flow may be spread over the main and boundary
layer
flow,
the followi~lg flow superimposed on the first, is usually due to the boundary
layer. This follows from the interaction of
a
pressure gradient across the main flow and
the boundary layers of
the
walls, covering the main flow in the direction, in which the
retained pressure acts.
Owing to the relatively slow flow in the boundary layer, the pressure gradient from the
main flow due to curvature and Coriolis force
is
not balanced by these velocity-linked
forces
withiil the layer. Therefore the fluid in the boundary layer is displaced from the
pressure to the suction face of
the duct.
[Jsually this shift is balanced by a niotion of the main flow in the mean stream facc of the
duct. Thus the secondary flow due to this effect usually consists of two loops turning
against each other.
This may occur in a rotor channel (Fig. 5.5.2b) or in a Send
{Fig.
5.5.2~).
The return flow in the mean stream face transports fluid of the main flow from the sucticn
to
the
pressure face, thus distorting the original velocity distribution across the channel
as
the fluid flows downstream by shifting the velocity maximum from the pressure to the
Suction face. The consequence is essentially the same
as
that of turbulence-conditioned
cross motion, described before.
-
$1
1.h~
erfccts
of
ro~ation on boundary
layer
in
a
turbomachine rotot.
Rep.
MD-24,
Mech.
Engg.
Dept.
Stanford
University
1970.
Additiorlal attention must
be
paid to the flow in
n
bend. Besides this
"secondary
floiv9v,
a
stalled region
is
observecl
in
a
i;cnd
011
tllc inner wall. near the outlet (Fig. 5.5.2~). This
may considerably corltract thc main flow. This incrci~ses tlie vclocity and consequently
also the loss.
A
small bilt unimportant "stalled region" can be also observed past the inlet
on the outer wall of the bend.
This effect can be explained as
fo!lows: Usually the specific energy of flow entering the
bend is constant across the section (with the exception of the region next to the wall):
When the main flow streamlines are forced into the curvature, a free vortex is produced
(Cap.
5.2).
Thus the pressure along the inner wall decreases with the increasing velocity
there.
Ncar the outlet the flow again is straightened and hence the velocity distribution becomes
more uniform over the cross section. The pressure on the inner wall then increases, in the
flow direction causing a stall there past the bend's exit. In
reality the aforementioned
secondary flow changes the velocity distribution across the tnain flow from that of a "free
vortex" at inlet to that of a forced one towards the outlet.
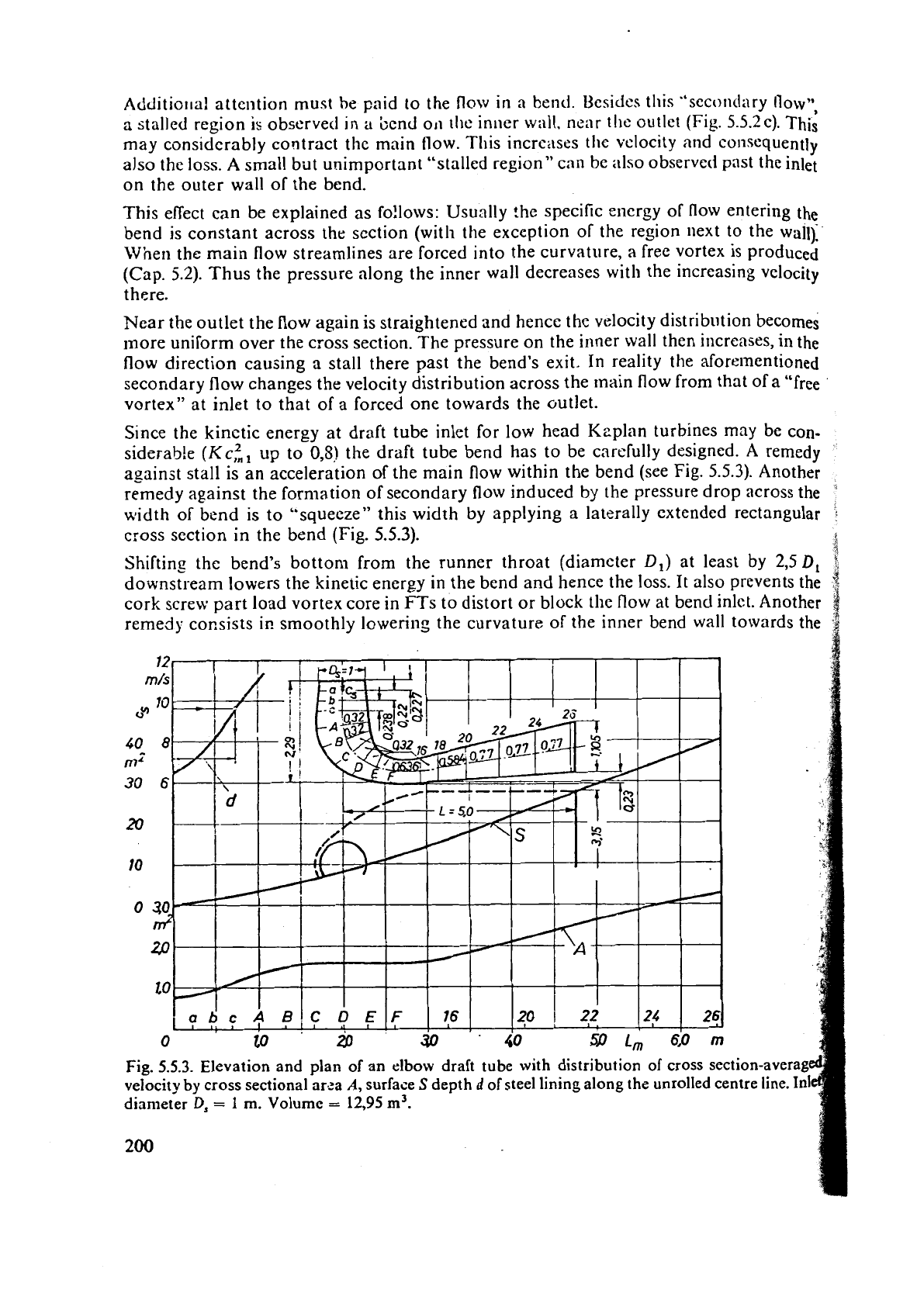
Since the
kinctic energy at draft tube inlet for low head Keplan turbines may be con-
siderab!e
(Kc:,
up to
0,s)
the draft tube bend has to be carefully designed. A remedy
against stall is an acceleration of the main flow within the bend (see
Fig.
5.5.3). Another
remedy against the formation of secondary flow induced by the pressure drop across the
'
width of bend is to "squeeze" this width by applying a laterally cxtended rectangular
cross section in the bend (Fig.
5.5.3).
i
Shiftins the bend's bottom from the runner throat (diameter
D,)
at least by
2,5
D,
downstream lowers the kinetic energy in the bend and hence the loss.
It
also prevents the
$
cork screw part load vortex core in FTs to distort or block the flow at bend inlet. Another
remedy
co~sists
in
smoothly lowering the curvature of the inner bend wall towards the
3
outjc~
(Fig.
5.5.3).
Somctimcs also laterally extcnded guide plates n~ay
help.
w11c~l
tile
me,,l
flow
is
not swirli~lg.
c.g.
in runner blade ailjusted
K.1-s.
The
kinetic energy
of
scco~liinry Row
is
more
or
lcss lost. l'his
is
the reason. why although
,,lc
loss in
a
bend
dcpcnds n~nit~ly on
ils
curv:itu~-e.
it
dcpcuds also to
:I
lesser extend
on
the
&vialion 2n2lt of the
bcnd
[5.60].
Tlic gencrntctl secondary floiv depcrids
also
on
this
,,gle
and
it prod~1ce.c
tile
lion's share
of
the
bend
loss
(Figs.
3.4.12;
3.4.14:
3.4.45).
5.5.5.3.
Sccorldary
flow
in
axial
turbolnachincs
I,,
n~;ichines the leakage
flow.
especially rhrough the tip clear;lncc, which
is
greatly
increased
in
a
PUIIIP
by shear flow close to the throat ring, may be the origin of
a
special type of secondar)
flow.
we
[5.13].
Another type of secondary flow, first described in detail by
IY
Giihr~zl
[9.60],
(see
c;,~.
9.6),
is limited to thc boundary layer of rotor vane and is oriented either to\~ards the hub or
the
tip and this, qonletlmcs
i!i
one
and
thc sanic boundary Inycr.
nis depcnds on whether thc whirl-induccd radial pressure gradient
,oct/r,
acting towards tlls hub
frorll
thc main
flow
on the l~oundary layer
is
larger or snlaller
rhan
t!~e centrifugal force due to the
fluid prticle in
the
bound;lry Inycr. Hence the secondary flo~ towards the hub occurs, when
the
whirl
component
C,
of thc main flow close to the boundary layer is oriented against the blade speed,
,.g.
at suction facc of runncr adjusted KTs.
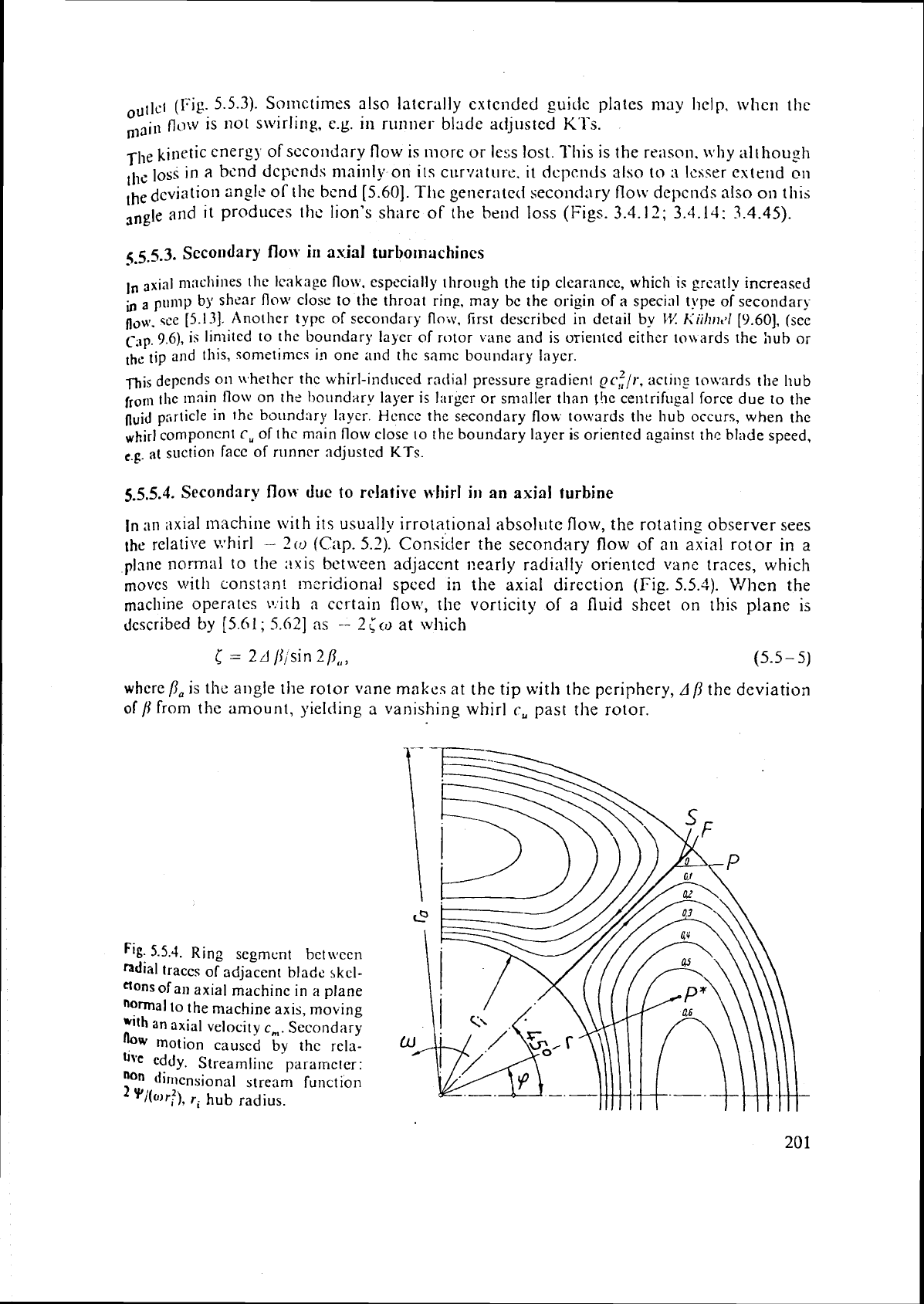
5.5.5.4.
Secondary
flow
duc
to
relative
whirl
in
an
axial
turbine
In
an
axial tnachi~le with
it5
usually irrotational absolute flow, the rotatins observer sees
the
relative whirl
-
2to
(Cap.
5.2).
Consicier the secondary flow
of
an
axial
rotor in
a
plnlle normal
to
the
axis betbyeen adjacent ]?early radially oriented
vane
traces, which
movcs with constan! lncridional speed
in
the
axial
direction
(Fig.
5.5.3).
When
the
machine operates
v:irh
a
certain floiv, the vorticity
of
a
fluid shcet on this plane is
Jcscribed
by
[5.6
1
;
5.621
as
-
2
i
to
at which
whcre
fi,
is thc angle the rotor vane
makes
at
the tip with the periphery,
A/?
the deviation
of
/j
from the amount, yielding
a
vanishing whirl
(-,
past the rotor.
Fig.
5.5.4.
Ring segment b~t\+~cen
"rdial traces of adjacent blade
~k~l-
QOns
of
an axial machine in
a
plane
normal
to
the machine axis, moving
with
an
axial velocity
c,.
Secondary
%W
motion caused by the rela-
eddy.
Strcarnlinc parameter:
wn
dinlcnsional stream function
P/(wrf),
ri
hub radius.
Ifitroducing
a
sirearn ftinction after
Cap.
5.2,
the planc and rotational flow in this circular
ring scctor can be rcduced to a Poisso~i equation
d
Y
=
--
2toC.
Ri~crbc
lias shown
a
co
solution of the type
'P
=
BQ*
+
B,
I~IQ
+
B2
+
Re
sin[r~n~~r(z/r~)/!n~,,],
at
which
n=
l
z
=
rei9,
Q
=
r/ri,
e,
=
ro/ri, ru is the tip radius,
ri
the hub radius, Re the rcnl part,
By
Bi,
A,*
are constants, [5.62].
The velocities
v,
and
v,
due to this secondary motion are linked to
Y
(sce Cap.
5.2)
by
v,
=
JtYjZr,
v,
=
-
d4Y/(rdq).
These velocities are connected with the compotlents
of
absolute velocity
c,
and
c,
by:
c,
=
v,
+
[or,
c,
=
or.
The kinetic energy of the secondary
flow due to the etTect described is assumed as loss
h:,.
With
z
as vane number,
K
ti
=
r, 0/(2
g
H)'I2,
where
An example with
(
=
0,l;
Ktr
=
1,6;
Q,
=
2
gives for
z
=
3,4,6, 12 blades the loss
It:,,
-
0,0159; 0,01604; 0,01635; 0,01702. Since
h:,
is nearly independent of the blade
number, it
can be approximated by
5.5.6.
The
prediction
of component loss
in
fluid
machines
5.5.6.1.
The
rotor
vane
IGSS
by rnrans of aerodynamics
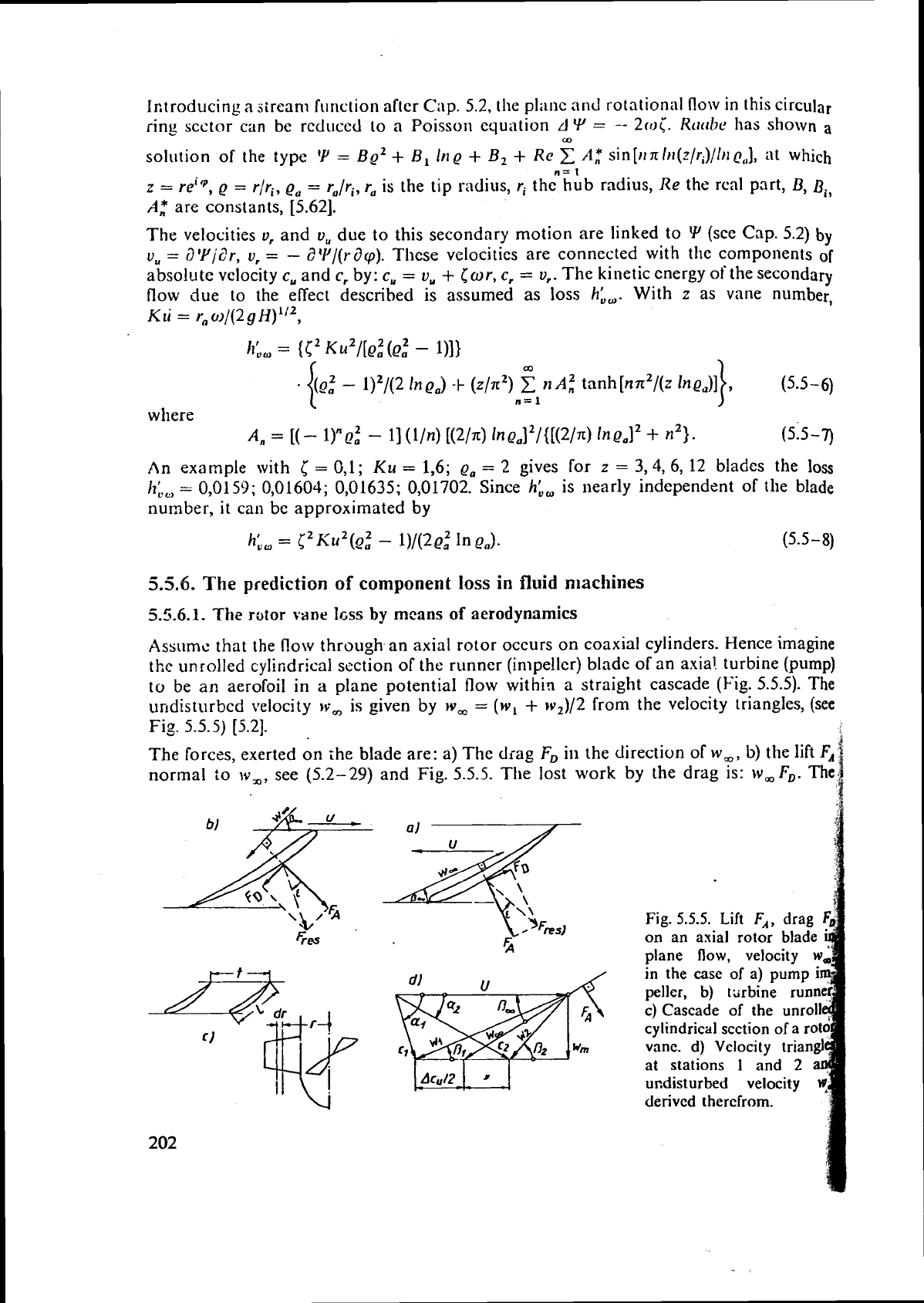
Assumc
that the flow through an axial rotor occurs on coaxial cylinders. Hence imagine
the
unrolled cylindrical section
of
the runner (inlpellcr) bladc of an axial. turbine (pump)
to be an aerofoil in
a
plane potential flow within a straight cascade (Fig. 5.5.5). The
undisturbed velocity
IV,
is given by
w,
=
(w,
+
1v2)/2 from the velocity triangles, (see
Fig.
5.5.5)
[5.2].
The forces, exerted on
ihe
blade
are:
a) The drag
FD
in the direction of
w?,
b)
the lift
FA
normal to
w,,
see (5.2-29) and
Fig.
5.5.5. The lost work by the drag is:
w,
FD.
The
Fig.
5.5.5.
Lift
FA,
drag
on an axial rotor blade
plane flow, velocity
in
the case of a) pump
peller, b)
t~rbine run
c) Cascade of the
un
cylindrical scction of
a
vane. d) Velocity trian
at stations
1
and
2
undisturbed velocity
derived therefrom.

effccti~e work done by the resulting peripheral conlponents of lift and drag with blade
sllct'd
u
is:
tr(i;;,
sin
bz
9
&
cos
B,,)
where
pa,
is the angle
w,,
makes with the periphery;
the positi!~~ sign holds true for an impeller, the negative for a runner.
The dirncnsionless loss is the ratio of the work done by drag to the energy transformed
this corresponds to the sum of the effective work done
+
the work done by drag.
Hence
hiR
=
wco
b;/[w,
FD
+
tl(FA sin
/I,
T
FD
cos pa)].
(5.5
-
9)
usually the drag is very small compared with the lift. Therefore the terms containing
F,
in the denominator may be omitted. Further the proportions of the csunl velocity tri-
angle~ of an axial
machine (Fig. 5.5.5) are such that:
\v,
=
11
[4.9]. Introducins the glide
angle
E,
tan
c
=
FD/FA
zz
E
[4.9], from (5.5-9) approximately
hi,,
z
+in
p,
z
c
II/C,
=
E/V,
(5.5-
10)
at which
47
=
c,/u
is the "local throughflow coefficient".
The last relation, which gives the runner (impeller) loss due to the main flo\v, shows that
the meridional velocity component must not be too small in relation to the blade speed.
Owing to this loss and especially due to cavitation in
a
rotor with adjustablc blades and
hence a
nonswirling flow
011
its suction side
1,
the ratio c,,/lr,, the "throughflow
coefficient", is linked
to
the angle /I1, the rotor vane makes with the periphery
at
tip and
suctio~~ side, and must be in the range
cp
=
c,,,/u,
=
tan
/?,
,
=
tan (15'.
.
.
25').
I,,
being the blade tip speed. To save costs,
u,
should be as high as possible. Usually
tr,
is related to thc spouting velocity (2yH)'12 for the head
1-I.
Thus the blade speed
coefficient is defined by
Kt4
=
U,/(~~N)'~~,
ranging
Ki1
=
1,3
.
.
.
1,s
. . .
2,4 for
H
=
70
..
.
15..
.
2 m in the case of axial iurbines.
5.5.6.2.
The
draft
tube
(diffuser)
loss
In an axial machine
rp
and Krr, last mentioned, yield the meridional velocity
c,,
and hznce
by
ci,/(2
LJ
H)
the kinetic encrgy referred to the head, e.g. at runner outlct. For
a
Kaplan
turbine under sated flow
Kc:
=
cil(2gH)
=
0,3
..
.
0,5
...
0,8
for
H
=
70..
.
15..
.
2 m.
Thus without a draft tube as a decelerating device past the rotor the efficiency of
a
Kaplan
turbine would not reach even 50% in the low head range. Therefore the draft tube has
to
transform this high kinetic energy into pressure with the lowest possiblc loss
h:,.
In
turbines with
a
whirl component
c,,
at runner exit
Hcre all the velocities and. hence also the loss coefficients C,,,,
iD,
are flow-averaged
quantities of the draft tube inlet section.
cD,
depends on the draft tube geometry.
iD,,
=
0,09
for the best straight draft tubes, as are used in tubular turbines (Fig. 3.4.25).
For the best elbow draft tubes as usaally installed in vertical turbines
i,,,
=
0,12
(Fig. 5.5.3).
Thc recovery of the whirl velocity
r,,
is comparatively smaller with an upper limit of
iu,
=
1.
This is one of the main reasons for the application of adjustable runner blades
in
machines which must often operate under off-design conditions. Owing to
a
stream-
wise increase of draft tube section (Fig. 5.5.3) and the nearly retained moment of
nlomentu~n along any streamline, there is also a reduction of
c,,
towards the draft tube
exit. Therefore
C,,
=
0,2..
.
0,4.
In
an impeller pump the velocity triangle for a radial flow rotor (Fig. 10.4.14) has cU2
=
c,
approxi~n;~tcly. Hcrc
ci
must bc bcst cvnvertcd into prcssurc cncrgy. Sincc thc loss
factor
[,
in an impcller diffuser is given, the diffuser loss
=
<,c5!(2!111)
c:111
be kept
low
only, w1ie11
c,
and hence
c,,
arc also low. For an impcllcr wit11 vanishing swirl at its eye
(at least at the bep), Euler's equation yiclds
cu2
=
gHvu-1/u2.
For
a
given pcriplicr;~l cficiency
PI,
and head
H,
c,,,
the whirl at impeller exit, can be reduced ollly
by increasing the bladc tip speed
u,
=
o)D/2,
thus increasing either the
impeller
tip diameter
D
its angular velocity
o.
Since the last nleiisure would dccrcase, for reasons of optimum suction
requirements, the diameter of the inipellcr eye by
D,
-
(~lco)"~,
and hence increase the subnlcrgence
and ground excavation, the only remedy for
a
reduction of diffuser loss in a pump is the increase
of
impellcr tip diameter
D,
comparcd with that of a turbine runner
DT.
This has to be done wit11 due consideration of disk friction loss, which yields
D,
1,4
DT
for
ma-
chines with the same operating data
H,
Q,
w.
5.5.6.3.
Disk'
friction
loss
4
1.
Simple treatment by disk model without le~kage influence:
il
Consider
a
circular disk of external diameter
D,,
that of the wheel, and a thickness
db,
which equals the axial thickness of hub and shroud at diameter
D,
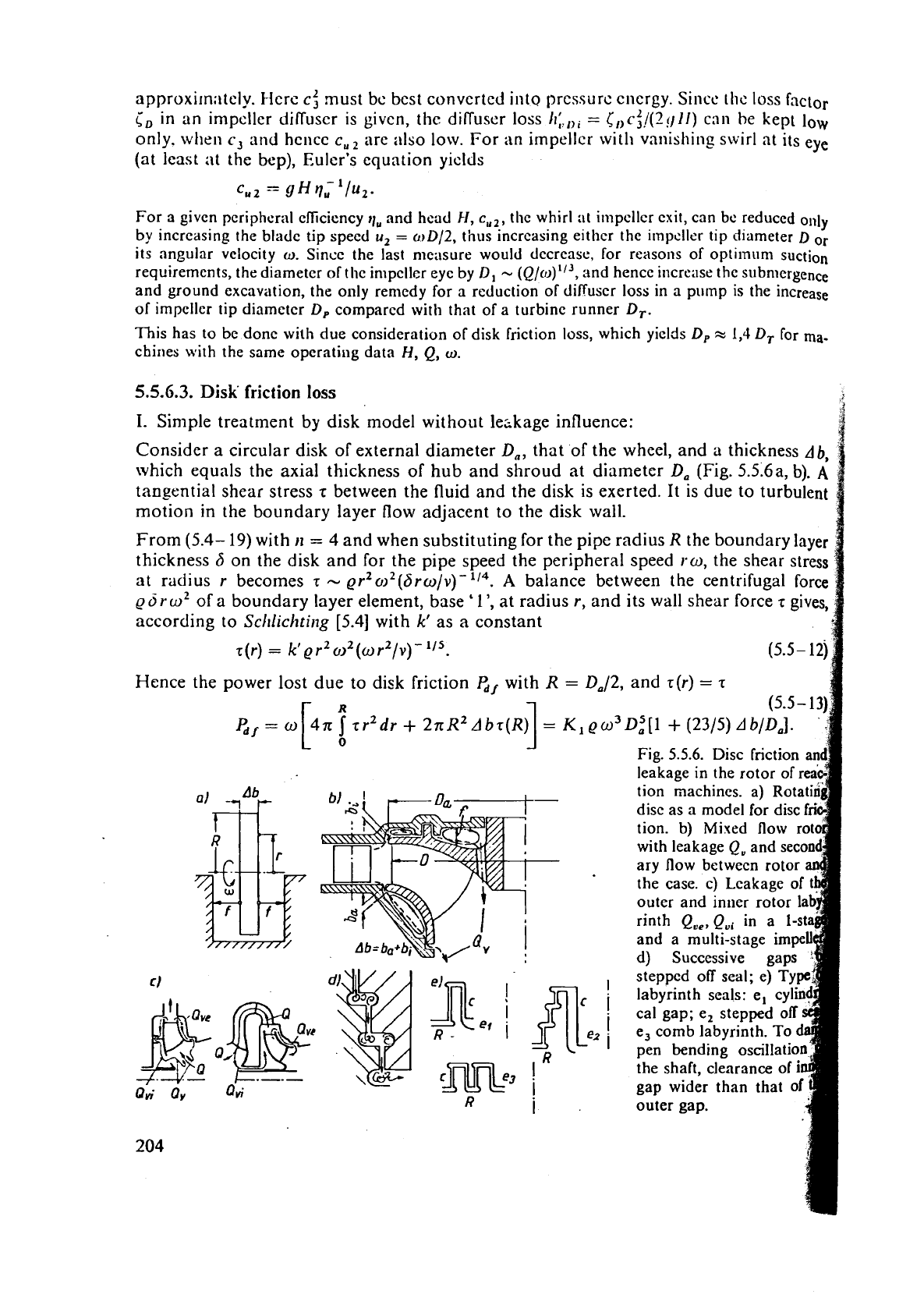
(Fig. 5.5.6a,
b).
A
tangential shear stress
r
between the fluid and the disk is exerted. It is due to turbulent
motion in the boundary layer flow adjacent to the disk wall.
From (5.4-
19)
with
n
=
4 and when substituting for the pipe radius
R
the boundary layer
thickness
6
on the disk and for the pipe speed the peripheral speed
ro,
the shear stress
at radius
r
becomes
r
--
er2
02(6ro/v)-
'I4.
A
balance between the centrifugal force
Q
6
ro2
of a boundary layer element, base
'
1
',
at radius
r,
and its wall shear force
r
gives,
according to
Scl~lichtiilg
[5.4] with
k'
as a constant
~(r)
=
k'er2m2(cor2/v)-
'I5.
(5.5-
12)
Hence the power lost due to disk friction
with
R
=
Da/2,
rr2dr
+
2n~~dbr(~)
=
Kleo3
1
,
and
r(r)
=
7
tion. b) Mixed flow
the case. c) Leakage
of
and a multi-stage impell
the shaft, clearance
of
outer
gap.

~ccording to tcsts of
Fijtfi~ig~r
[5.63],
and from (5.5-- 12),
K1
depends also
on
!lie axial
j
hctt~~ee~i
thc
casing and disk ils
JJ.
Detailed trcntment accounting for leakage:
A
more detailed in\lestigation shows. that
the torque due to disk friction
,%I,,,
depends
on
the radial \~ilrintion of mean angolor
velocit~, the fluid between the caslng and shroud has (Fig. 3.5.6 b). This locril mean
angi~lar velocity
o(r)
is also influcnccd by thc leakage
flow
Q,
passing betwccn the
and the casing. This
follo\t~s from thc moment of rnornentunl and direction of
Q,,.
~~surning the torque on the "disk face", which rotates
with
angular velocity
to
as
nfa
=
7
I<(rJr4[w
-
co(r)j2 dr,
knowlzdge of
~(r)
is required for evaluation. From [lie
r,
,noment balance on an elenlentary liquid ring bet\vecn the casing and disk wall, due to
the shear stress on the casing 2nd disk wall, duc
LO
slipp;l,gc of adjacent eleme11::iry fluid
rings and due to the
chancge of the moment of momentun-r by the leakage
Qv,
crossing
the elementary ring,
a
differential equaticn of second order for
o(r)
was found
by
Rtrabe,
j6.81.
Neglecting the highest derivative gives a linear differential equation with the following
solution for
c9(r.)
under thc ass~imption of
a
constant spacing
f
bctneen disk and casing
and with
11*
;IS
a
constant assulned eddy viscosity betwccn adjacent slipping fliiid rings
according to
Dotnnl
[5.64] and
k
=
1,2.38
g
(r:
cc),'~)
'15
IIere thc leakage
Q,
1s assumed in thc usual direction totilards the axis. The integretion
constant
ro,
can be determrl~ed from a pven anguiar veloclty of leakage at the entrancc
of
the gap be!n.een the casin_c and the rotor
o~(i;,)
=
(o,
given by the whirl component
oi
the flow efiteling the runner.
Starting from
(5.5-
12),
substituting there
(3
by
uj
-
~(r.),
on the model of
(5.5-
13),
but
without
[I
+
I,/l(f,'D,
-
0,1)] and
substituting the factor
0.256
on the rough base
(ri/r,)23/5
<,
1
by 0,s
.
0,256
-
223;5!2n
=
0,493 t11c function
k(r)
under the above integral
of
Mdf
(the disk friction torque of one shroud's face) becorncs
Elimination of
disk
friction by aerating the inter
spacc
bct\\fcen casing and runner has been dcvclo-
pcd
by
R.
Sprotrle
on Canadian turbines
[5.66j.
7'he dish friction on a low specific speed Franc~s
runner hzs
bel-n
in~cstigatcd
by
J.
O.stc~rn.oicit.1-
and
Gcis
[5.67].
In
tllr above. the loss due
to
disk
f:iction and leakijgc
ale
considered to be linked to each other.
On
the
other hand. in
a
pump. the
lcakagc may also
bc
associated with thc hydraulic loss, since it has to be delivered
by
the peripheral
blildc work. l'hc
analogue
holds for
a
turbine. Both considerations are
assessments
and in usage
(see
Cap.
9.3.).
Coiisider 11;e runner of
a
Fril~~cis turbine
(Fig.
5.5.6b-c).
The labyrinths there are located
0"
hub and shroud
at
nearly the same radial distance from the axis. Thus the axial thrust

OII
the 011tei
faces
of
11ul1
znd s1irot:d is b;~lanccd. For a minimum lenhagc. the seals are
ncnrly on the rotor throat diameter. With
n
figure
K*
that accounts for the dcgree
of
reaction
and
the
presssre drop betwccn rotor tip di;in~cter ;tnd !;ibyrinlli due to the rota-
tion
of
fluid betwccn the casing and rotor, tlie prcssilrc drop within the seal is give11
by
Ap
r=
R*(p,
-
p,). This pressure drop must overcome the following loss-conditioned
pressure
drops within the labyrinth:
a)
.Apf,, due to dissipation in the narrow gaps with small radial clearance
s
and a total
meridional length
i;
b) 4pin, due to dissipation at the gap inlet;
c) Ap,,,, due to dissipation at
the
gap
outlet. Hence
Since by continuity, the leakage
Q,,
that passes the seal is linked to its mean rneridional
speed c,,, the above loss terms are usually expressed in terms of
c,,.
Strictly speaking, the
ne3n resdting relative flow within the gap, considered now as the relcvant one, makes
a
mean ansle
/I,,,
with the circumference, approximated by tan
8,
=
cs,/(wr/2) and hence
ionnd only by trial and error. Hewever in the following,
0,
is assumed as preliminarily
gi-.en. Thus the resulting flow has the mean speed c,,jsir~
P,
and the mean real flow path
has
the icngth Llsin
P,,,
.
Neglecting the inlet length of gap flow, Apf, can be expressed by the pipe loss formula
(5.4--
13), in which
(i
is replaced by the hydraulic diameter 2s of a s~nall gap.
With
as a gap flow loss coefficient, depending after Petel-n1a11n
[5.19],
Wagt~er [5.68],
Kosy~la
[5.69], Ratrbe [5.70], Sturnpa [5.71], Keller [5.72], Eichler ant1 Wiet1eman;i [5.73] on
the
Reylzolds
numbers Re,
=
cs,s/v due to gap flow, and Re,
=
src~)/v
due to rotation
A
y,
,
=
:
@
(L/s)
cfp/(4 sin
PiW):
(5.5-
19)
For an i~dividual gap Ap, and Ap,,,, can be reduced to
c:,,
by
A
pout
=
rout
9
cf,/2
(5.5-21)
For
a
favourable gap inlet design (Fig. 5.5.6d) the lcss coenicient there can be assumed
as
;,
=
0,5. This design has successive gaps radially displaced. Thus the leakage jet from
an upstream
gzp is not in line with
a
downstream gap. Moreover a groove facilitates
the
formation of a ring vortex throttling the inlet, see Fig.
5.5.6d.
The
loss coefficient at gap outlet
C,,,
=
1,
when successive gaps are radially displaced
(Fig.
5.5.6d,
e,) and when an axially extended expansion chamber proirides for complete
dissipation of kinetic energy
czp/2 of the leakage jet. Recently Chaix informed on (o,,+lh.
For
a
rotor seal (labyrinth) with
z
equal gaps in series the last four relations yield the
n~eridional gap velocity
With
a
contraction coefficient
cP
the leakage through this seal
Qv
=
@n
DSpscSp.
206
