Naber G.L. The Geometry of Minkowski Spacetime. An Introduction to the Mathematics of the Special Theory of Relativity
Подождите немного. Документ загружается.


194 3 The Theory of Spinors
and
σ
a
A
˙
X
σ
b
B
˙
Y
F
aα
F
b
α
= −F
A
˙
XC
˙
Z
F
B
˙
Y
C
˙
Z
. (3.6.25)
For the proof of (3.6.24) we compute
F
C
˙
ZD
˙
W
F
C
˙
ZD
˙
W
= F
C
˙
ZD
˙
W
CC
1
¯
˙
Z
˙
Z
1
DD
1
¯
˙
W
˙
W
1
F
C
1
˙
Z
1
D
1
˙
W
1
=
σ
a
C
˙
Z
σ
b
D
˙
W
F
ab
CC
1
¯
˙
Z
˙
Z
1
DD
1
¯
˙
W
˙
W
1
σ
α
C
1
˙
Z
1
σ
β
D
1
˙
W
1
(η
αμ
η
βν
F
μν
)
=
CC
1
¯
˙
Z
˙
Z
1
η
μα
σ
α
C
1
˙
Z
1
DD
1
¯
˙
W
˙
W
1
η
νβ
σ
β
D
1
˙
W
1
σ
a
C
˙
Z
σ
b
D
˙
W
F
ab
F
μν
= σ
μ
C
˙
Z
σ
ν
D
˙
W
σ
a
C
˙
Z
σ
b
D
˙
W
F
ab
F
μν
=
σ
μ
C
˙
Z
σ
a
C
˙
Z
σ
ν
D
˙
W
σ
b
D
˙
W
F
ab
F
μν
=
−δ
a
μ
−δ
b
ν
F
ab
F
μν
= F
ab
F
ab
.
Exercise 3.6.8 Prove (3.6.25).
Substituting (3.6.24)a
nd(3.6.25)into(3.6.23)gives
T
A
˙
XB
˙
Y
=
1
4π
,
−F
A
˙
XC
˙
Z
F
B
˙
Y
C
˙
Z
+
1
4
AB
¯
˙
X
˙
Y
F
C
˙
ZD
˙
W
F
C
˙
ZD
˙
W
-
. (3.6.26)
Now we claim that if F
A
˙
XB
˙
Y
=
AB
¯
φ
˙
X
˙
Y
+ φ
AB
¯
˙
X
˙
Y
,then
F
C
˙
ZD
˙
W
F
C
˙
ZD
˙
W
=2
φ
CD
φ
CD
+
¯
φ
˙
Z
˙
W
¯
φ
˙
Z
˙
W
(3.6.27)
and
F
A
˙
XC
˙
Z
F
B
˙
Y
C
˙
Z
= −2φ
AB
¯
φ
˙
X
˙
Y
+
AB
¯
φ
˙
X
˙
Z
¯
φ
˙
Y
˙
Z
+¯
˙
X
˙
Y
φ
AC
φ
B
C
. (3.6.28)
We prove (3.6.27) as follows:
F
C
˙
ZD
˙
W
F
C
˙
ZD
˙
W
=
CD
¯
φ
˙
Z
˙
W
+ φ
CD
¯
˙
Z
˙
W
CD
¯
φ
˙
Z
˙
W
+ φ
CD
¯
˙
Z
˙
W
=
CD
CD
¯
φ
˙
Z
˙
W
¯
φ
˙
Z
˙
W
+
CD
φ
CD
¯
φ
˙
Z
˙
W
¯
˙
Z
˙
W
+
φ
CD
CD
¯
˙
Z
˙
W
¯
φ
˙
Z
˙
W
+
φ
CD
φ
CD
¯
˙
Z
˙
W
¯
˙
Z
˙
W
.
But observe that, by symmetry of φ,
CD
φ
CD
=
10
φ
10
+
01
φ
01
= −φ
10
+
φ
01
= 0 and, similarly, ¯
˙
Z
˙
W
¯
φ
˙
Z
˙
W
=0.Moreover,by(3.3.7),
CD
CD
=
¯
˙
Z
˙
W
¯
˙
Z
˙
W
=2so
F
C
˙
ZD
˙
W
F
C
˙
ZD
˙
W
=2
¯
φ
˙
Z
˙
W
¯
φ
˙
Z
˙
W
+0+0+2φ
CD
φ
CD
which gives (3.6.27).
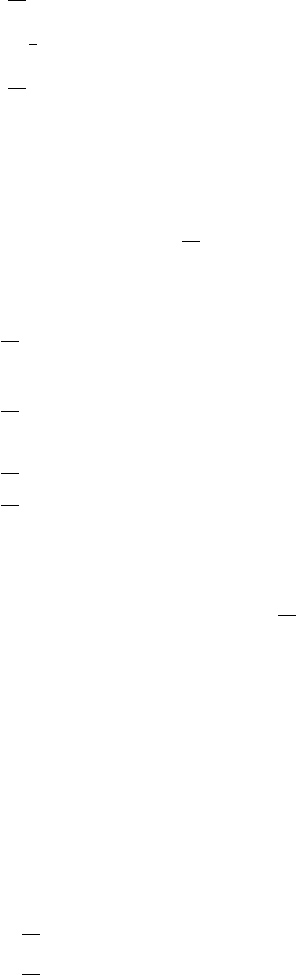
3.6 The Electromagnetic Field (Revisited) 195
Exercise 3.6.9 Prove (3.6.28).
With (3.6.27)and(3.6.28), (3.6.26) becomes
T
A
˙
XB
˙
Y
=
1
4π
,
2φ
AB
¯
φ
˙
X
˙
Y
−
AB
¯
φ
˙
X
˙
Z
¯
φ
˙
Y
˙
Z
− ¯
˙
X
˙
Y
φ
AC
φ
B
C
+
1
2
AB
¯
˙
X
˙
Y
φ
CD
φ
CD
+
¯
φ
˙
Z
˙
W
¯
φ
˙
Z
˙
W
-
.
T
A
˙
XB
˙
Y
=
1
4π
,
2φ
AB
¯
φ
˙
X
˙
Y
−
AB
¯
φ
˙
X
˙
Z
¯
φ
˙
Y
˙
Z
− ¯
˙
X
˙
Y
φ
AC
φ
B
C
+(det[φ
AB
])
AB
¯
˙
X
˙
Y
+(det[
¯
φ
˙
X
˙
Y
])
AB
¯
˙
X
˙
Y
, (3.6.29)
where we have appealed to part (3) of Exercise 3.3.6 and its conjugated ver-
sion. For the remaining simplifications we use part (4) of this same exercise.
If either A = B or
˙
X =
˙
Y all the terms on the right-hand side of (3.6.29)
except
t
he first
are zero so T
A
˙
XB
˙
Y
=
1
2π
φ
AB
¯
φ
˙
X
˙
Y
and (3.6.22)isproved.The
remaining cases are A
˙
XB
˙
Y =1
˙
10
˙
0, 1
˙
00
˙
1, 0
˙
11
˙
0and0
˙
01
˙
1 and all are treated
in the same way, e.g.,
T
1
˙
10
˙
0
=
1
4π
,
2φ
10
¯
φ
˙
1
˙
0
−
10
¯
φ
˙
1
˙
Z
¯
φ
˙
0
˙
Z
− ¯
˙
1
˙
0
φ
1C
φ
0
C
+(det[φ
AB
])
10
¯
˙
1
˙
0
+(det[
¯
φ
˙
X
˙
Y
])
10
¯
˙
1
˙
0
=
1
4π
[2φ
10
¯
φ
˙
1
˙
0
− (−1)(−det[
¯
φ
˙
X
˙
Y
]) − (−1)(−det[φ
AB
])
+(det[φ
AB
])(−1)(−1) + (det[
¯
φ
˙
X
˙
Y
])(−1)(−1)]
=
1
4π
[2φ
10
¯
φ
˙
1
˙
0
]
=
1
2π
φ
10
¯
φ
˙
1
˙
0
.
Exercise 3.6.10 Check the remaining cases to complete the proof of
(3.6.22).
W
e
use t
he spinor equivalent T
A
˙
XB
˙
Y
=
1
2π
φ
AB
¯
φ
˙
X
˙
Y
of the energy-
momentum T of F to give another proof of the dominant energy condition
(Exercise 2.5.6) that does not depend on the canonical forms of F .Begin
with two future-directed null vectors u = u
a
e
a
and v = v
b
e
b
in M.ByThe-
orem 3.4.3, the spinor equivalents of u and v can be written U
A
˙
X
= μ
A
¯μ
˙
X
and V
A
˙
X
= ν
A
¯ν
˙
X
,whereμ and ν are two spin vectors. Thus, we may write
u
a
= −σ
a
A
˙
X
μ
A
¯μ
˙
X
and v
b
= −σ
b
B
˙
Y
ν
B
¯ν
˙
Y
so that
Tu ·v = T
ab
u
a
v
b
= T
ab
−σ
a
A
˙
X
μ
A
¯μ
˙
X
−σ
B
B
˙
Y
ν
B
¯ν
˙
Y
=
σ
a
A
˙
X
σ
b
B
˙
Y
T
ab
μ
A
¯μ
˙
X
ν
B
¯ν
˙
Y
= T
A
˙
XB
˙
Y
μ
A
¯μ
˙
X
ν
B
¯ν
˙
Y
=
1
2π
φ
AB
¯
φ
˙
X
˙
Y
μ
A
¯μ
˙
X
ν
B
¯ν
˙
Y
=
1
2π
(φ
AB
μ
A
μ
B
)(
¯
φ
˙
X
˙
Y
¯μ
˙
X
¯ν
˙
Y
),

196 3 The Theory of Spinors
so
Tu ·v =
1
2π
|φ
AB
μ
A
ν
B
|
2
. (3.6.30)
In particular, Tu · v ≥ 0.
Exercise 3.6.11 Show that Tu ·v ≥ 0 whenever u and v are timelike or null
and both are future-directed. Hint: Any future-directed timelike vector can
be written as a sum of two future-directed null vectors.
We recall from Section 2.5 that, for any future-directed unit timelike vector
U, TU · U =
1
8π
[|
E
|
2
+ |
B
|
2
] is the energy density in any admissible frame
with e
4
= U.Consequently,ifF is nonzero, Tu · u = 0 for any timelike
vector u. We now investigate the circumstances under which Tv · v =0for
some future-directed null vector v. Suppose then that v is null and future-
directed and Tv · v =0.Writev
a
= −σ
a
A
˙
X
ν
A
¯ν
˙
X
for some spin vector ν
(Theorem 3.4.3). Then, by (3.6.30), φ
AB
ν
A
ν
B
= 0. Using the decomposition
(3.6.4)ofφ this
is equivalent to
(α
A
β
B
+ α
B
β
A
)(ν
A
ν
B
)=0,
(α
A
ν
A
)(β
B
ν
B
)+(α
B
ν
B
)(β
A
ν
A
)=0,
2 <ν,α><ν,β>=0
which is the case if and only if either <ν,α>=0or<ν,β>=0.But
<ν,α>=0ifandonlyifν is a multiple of α (Lemma 3.2.1(g)) and similarly
for <ν,β>=0.Butifν is a multiple of either α or β,thenv is a multiple of
one of the two null vectors determined by α or β, i.e., v is along a principal
null direction of φ
AB
. Thus, a future-directed null vector v for which Tv ·v =0
must lie along a principal null direction of φ
AB
. Moreover, by reversing the
steps above, one finds that the converse is also true so we have proved that a
nonzero null vector v satisfies Tv ·v =0ifandonlyifv lies along a principal
null direction of φ
AB
.
Exercise 3.6.12 Let F : M→Mbe a nonzero, skew-symmetric linear
transformation,
˜
F the associated bivector and
∗
˜
F the dual of
˜
F (Section 2.7).
By (2.7.16)(withM = Λ), the
Levi-Civita symbol
abcd
defines a (con-
stant) covariant world tensor of rank 4. We define its spinor equivalent by
A
˙
XB
˙
YC
˙
ZD
˙
W
= σ
a
A
˙
X
σ
b
B
˙
Y
σ
c
C
˙
Z
σ
d
D
˙
W
abcd
. Show that
A
˙
XB
˙
YC
˙
ZD
˙
W
= i(
AC
BD
¯
˙
X
˙
W
¯
˙
Y
˙
Z
−
AD
BC
¯
˙
X
˙
Z
¯
˙
Y
˙
W
)
and then raise indices to obtain
A
˙
XB
˙
Y
C
˙
ZD
˙
W
= i
δ
C
A
δ
D
B
δ
˙
W
˙
X
δ
˙
Z
˙
Y
− δ
D
A
δ
C
B
δ
˙
Z
˙
X
δ
˙
W
˙
Y
.
Now show that the spinor equivalent of
∗
˜
F is given by
∗
F
A
˙
XB
˙
Y
= i(
AB
¯
φ
˙
X
˙
Y
− φ
AB
¯
˙
X
˙
Y
).
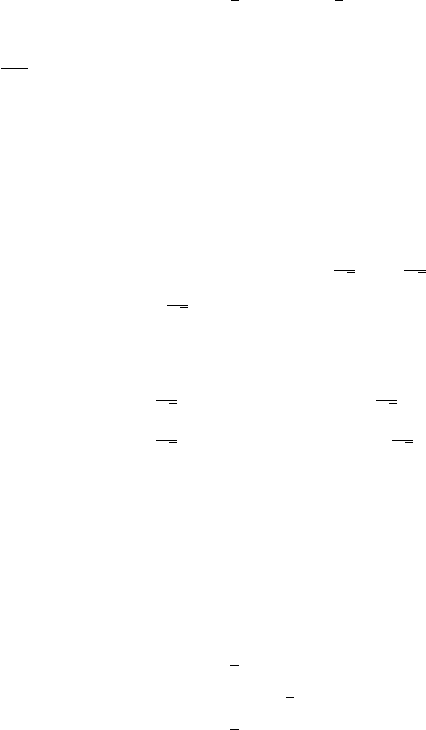
3.6 The Electromagnetic Field (Revisited) 197
Exercise 3.6.13 Define the spinor equivalent of an arbitrary world tensor
(Section 3.1) of contravariant rank r and covariant rank s, being sure to verify
the appropriate transformation law, and show that any such spinor equivalent
is Hermitian. Find an “inversion formula” analogous to (3.4.19)and(3.5.3)
t
h
at ret
rieves the world tensor from its spinor equivalent. For what type of
spinor can this process be reversed to yield a world tensor equivalent?
We conclude our discussion of electromagnetic theory by deriving the el-
egant spinor form of the source-free Maxwell equations. For this we fix an
admissible basis {e
a
} and a spin frame {s
A
} and let F denote an electro-
magnetic field on (a region in) M.Asusual,wedenotebyF
ab
= η
aα
F
α
b
the components of the corresponding bivector
˜
F , all of which are functions of
(x
1
,x
2
,x
3
,x
4
). Then the spinor equivalent of
˜
F is F
A
˙
XB
˙
Y
= σ
a
A
˙
X
σ
b
B
˙
Y
F
ab
and the electromagnetic spinor φ
AB
is given by
φ
AB
=
1
2
F
˙
U
A
˙
U
B
=
1
2
[F
A
˙
0B
˙
1
− F
A
˙
1B
˙
0
].
Next we introduce “spinor equivalents” for the differential operators ∂
a
=
∂
∂x
a
,a=1,2,3,4.Specifically,wedefine,foreachA =1, 0and
˙
X =
˙
1,
˙
0, an
operator ∇
A
˙
X
by
∇
A
˙
X
= σ
a
A
˙
X
∂
a
= σ
a
A
˙
X
(η
aα
∂
α
).
Thus, for example,
∇
1
˙
1
= σ
a
1
˙
1
∂
a
= σ
1
1
˙
1
∂
1
+ σ
2
1
˙
1
∂
2
+ σ
3
1
˙
1
∂
3
+ σ
4
1
˙
1
∂
4
= σ
3
1
˙
1
∂
3
+ σ
4
1
˙
1
∂
4
=
1
√
2
∂
3
+
1
√
2
∂
4
=
1
√
2
(∂
3
− ∂
4
).
Exercise 3.6.14 Prove the remaining identities in (3.6.31):
∇
1
˙
1
=
1
√
2
(∂
3
− ∂
4
), ∇
1
˙
0
=
1
√
2
(∂
1
+ i∂
2
),
∇
0
˙
1
=
1
√
2
(∂
1
− i∂
2
), ∇
0
˙
0
= −
1
√
2
(∂
3
+ ∂
4
).
(3.6.31)
With this notation we claim that all of the information contained in the
source-free Maxwell equations (2.7.15)and(2.7.21) can be written con-
cisely
as
∇
A
˙
X
φ
AB
=0,A=1, 0,
˙
X =
˙
1,
˙
0. (3.6.32)
Equations (3.6.32)arethespinor form of the s
ource-free Maxwell equations.
To verify the claim we write
φ
11
=
1
2
[(F
13
+ F
14
)+i(F
32
+ F
42
)],
φ
10
= φ
01
=
1
2
[F
43
+ iF
12
],
φ
00
=
1
2
[(F
41
+ F
13
)+i(F
42
+ F
23
)]
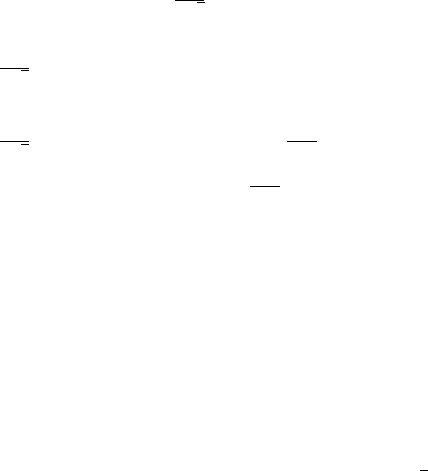
198 3 The Theory of Spinors
(see (3.6.14)–(3.6.16)). Now compute, for example,
∇
A
˙
1
φ
A0
= ∇
1
˙
1
φ
10
+ ∇
0
˙
1
φ
00
=
1
2
√
2
{(∂
3
− ∂
4
)(F
43
+ iF
12
)
+(∂
1
− i∂
2
)((F
41
+ F
13
)+i(F
42
+ F
23
))}
=
1
2
√
2
{[−(F
14,1
+ F
24,2
+ F
34,3
)+(F
13,1
+ F
23,2
− F
43,4
)]
+ i[(F
12,3
+ F
31,2
+ F
23,1
) − (F
12,4
+ F
41,2
+ F
24,1
)]}
=
1
2
√
2
6,
−div
E
−
,
(curl
B
) · e
3
−
∂E
3
∂x
4
--
+ i
,
div
B
−
,
(curl
E
) ·e
3
+
∂B
3
∂x
4
--7
.
Exercise 3.6.15 Calculate, in the same way, ∇
A
˙
1
φ
A1
, ∇
A
˙
0
φ
A1
,and
∇
A
˙
0
φ
A0
,andshowthat(3.6.32) is equivalent to Maxwell’s equations.
Generalizations of (3.6.32) are used in relativistic quantum mechanics as
field equa
tions f
or various types of massless particles. Specifically, if n is a
positive integer and φ
A
1
A
2
···A
n
is a symmetric spinor of valence
00
n 0
,then
∇
A
˙
X
φ
AA
2
···A
n
=0,A
2
,...,A
n
=1, 0,
˙
X =
˙
1,
˙
0,
is taken to be the massless free-field equation for arbitrary spin
1
2
n particles
(see 5.7 of [PR]). In particular, if n =1,
th
enφ
A
is a spin vector and one
obtains the Weyl neutrino equation
∇
A
˙
X
φ
A
=0,
˙
X =
˙
1,
˙
0,
which suggested the possibility of parity nonconservation in weak interactions
years before the phenomenon itself was observed (see [LY]).
Chapter 4
Prologue and Epilogue: The de Sitter
Universe
4.1 Introduction
In this final chapter we would like to take one small step beyond the special
theory of relativity in order to briefly address two issues that have been con-
scientiously swept under the rug to this point. These are related, although
perhaps not obviously so. The first is the issue of gravitation which we quite
explicitly eliminated from consideration very early on. We have proposed
Minkowski spacetime as a model of the event world only when the effects of
gravity are “negligible”, that is, for a universe that is effectively “empty”, but
it is doubtful that anything in our development has made it clear why such a
restriction was necessary. Here we will attempt to provide an explanation as
well as a gentle prologue to how one adapts to the presence of gravitational
fields. Then, as an epilogue to our story, we will confront certain recent astro-
nomical observations suggesting that, even in an empty universe, the event
world may possess properties not reflected in the structure of Minkowski
spacetime, at least on the cosmological scale. Remarkably, there is a viable
alternative, nearly 100 years old, that has precisely these properties and we
will devote a little time to becoming acquainted with it.
4.2 Gravitation
An electromagnetic field is a 2-form on Minkowski spacetime M that satisfies
Maxwell’s equations. A charged particle responds to the presence of such a
field by experiencing changes in 4-momentum specified by the Lorentz 4-Force
Law. This is how particle mechanics works. A physical agency that affects the
shape of a particle’s worldline is isolated and described mathematically and
then equations of motion are postulated that quantify this effect. It would
seem then that the next logical step in such a program would be to carry
, : An Introduction
, Applied Mathematical Sciences 92,
G.L. Naber The Geometry of Minkowski Spacetime to the Mathematics
of the Special Theory of Relativity
DOI 10.1007/978-1-4419-7838-7_4, © Springer Science+Business Media, LLC 2012
199
200 4 Prologue and Epilogue: The de Sitter Universe
out an analogous procedure for the gravitational field. In the early days of
relativity theory many attempts were made (by Einstein and others) to do
just this, but they all came to naught. However one chose to model a gravi-
tational field on M and however the corresponding equations of motion were
chosen, the numbers simply did not come out right; theoretical predictions
did not agree with the experimental facts (an account of some of these early
attempts is available in Chapter 2 of [MTW]). In hindsight, the reason for
these
fa
ilure
s appears quite simple (once it is pointed out to you by Einstein,
that is). An electromagnetic field is something “external” to the structure of
spacetime, an additional field defined on and (apparently) not influencing the
mathematical structure of M. Einstein realized that a gravitational field has
a very special property which makes it unnatural to regard it as something
external to the nature of the event world. Since Galileo it has been known
that all objects with the same initial position and velocity respond to a given
gravitational field in the same way (i.e., have identical worldlines) regardless
of their material constitution (mass, charge, etc.). This is essentially what
was verified at the Leaning Tower of Pisa and contrasts markedly with the
behavior of electromagnetic fields. These worldlines (of particles with given
initial conditions of motion) seem almost to be natural “grooves” in space-
time that anything will slide along once placed there. But these “grooves”
depend on the particular gravitational field being modeled and, in any case,
M simply is not “grooved” (its structure does not distinguish any collection
of curved worldlines). One suspects then that M itself is somehow lacking,
that the appropriate mathematical structure for the event world may be more
complex when gravitational effects are nonnegligible.
To see how the structure of M might be generalized to accommodate the
presence of gravitational fields let us begin again as we did in the Introduction
with an abstract set M whose elements we call “events”. One thing at least is
clear. In regions that are distant from the source of any gravitational field no
accommodation is necessary and M must locally “look like” M.Butagreat
deal more is true. In his now famous Elevator Experiment Einstein observed
that any event has about it a sufficiently small region of M which “looks
like” M. To see this we reason as follows. Imagine an elevator containing an
observer and various other objects that is under the influence of some uniform
external gravitational field. The cable snaps. The contents of the elevator are
now in free fall. Since all of the objects inside respond to the gravitational
field in the same way they will remain at relative rest throughout the fall.
Indeed, if our observer lifts an apple from the floor and releases it in mid-air
it will appear to him to remain stationary. You have witnessed these things
for yourself. While it is unlikely that you have had the misfortune of seeing a
falling elevator you have seen astronauts at play inside their space capsules
while in orbit (i.e., free fall) about the earth. The objects inside the elevator
(capsule) seem then to constitute an archetypical inertial frame. By estab-
lishing spacetime coordinates in the usual way our observer thereby becomes
an admissible observer, at least within the spatial and temporal constraints
4.2 Gravitation 201
imposed by his circumstances. Now, picture an arbitrary event. There are any
number of vantage points from which the event can be observed. One is from
a freely falling elevator in the immediate spatial and temporal vicinity of the
event and from this vantage point the event receives admissible coordinates.
There is then a local admissible frame near any event in M.
The operative word is local. The “spatial and temporal constraints” to
which we alluded arise from the non-uniformity of any real gravitational field.
For example, in an elevator that falls freely in the earth’s gravitational field,
all of the objects inside are pulled toward the earth’s center so that they do,
in fact, experience some slight relative acceleration (toward each other). Such
motion, of course, goes unnoticed if the elevator falls neither too far nor too
long. Indeed, by restricting our observer to a sufficiently small region in space
and time these effects become negligible and the observer is indeed inertial.
But then, what is “negligible” is in the eye of the beholder. The availability of
more sensitive measuring devices will require further restrictions on the size
of the spacetime region that “looks like” M and so one might say that M is
locally like M in the same sense that the sphere x
2
+ y
2
+ z
2
= 1 is locally
like the plane R
2
.Inthe19
th
century this would have been expressed by
saying that each point of the sphere has about it an “infinitesimal neighbor-
hood” that is identical to the plane. Today we prefer to describe the situation
in terms of local coordinate systems and tangent planes, but the idea is the
same.
What appears to be emerging then as the appropriate mathematical struc-
ture for M is something analogous to a smooth surface, albeit a 4-dimensional
one. As it happens there is in mathematics a notion (that of a “smooth man-
ifold”) that generalizes the definition of a smooth surface to higher dimen-
sions. With each point in such a manifold is associated a flat “tangent space”
analogous to the tangent plane to a surface. These are equipped with inner
products, varying smoothly from point to point, with which one can compute
magnitudes of tangent vectors that can then be integrated to obtain lengths of
curves. Such a smoothly varying family of inner products is called a “metric”
(“Riemannian” if the inner products are positive definite and “Lorentzian”
if they have index one) and with such a thing one can do geometry. In par-
ticular, one can introduce a notion of “curvature” which, just as for surfaces,
describes quantitatively the extent to which the manifold locally deviates
from its tangent spaces, that is, from flatness. In the particular manifolds of
interest in relativity (called “Lorentzian manifolds” or “spacetimes”) these
deviations are taken to represent the effects of a non-negligible gravitational
field. An object in free fall in such a field is represented by a curve that is
“locally straight” since it would indeed appear straight in a nearby freely
falling elevator (local inertial frame). These are called “geodesics” and cor-
respond to the “grooves” to which we referred earlier (the analogous curves
on the sphere are its great circles). Not every Lorentzian metric represents
a physically realistic gravitational field any more than every 2-form on M
202 4 Prologue and Epilogue: The de Sitter Universe
represents an electromagnetic field and Einstein postulated field equations
(analogous to Maxwell’s equations) that should be satisfied by any metric
worthy of physical consideration.
The study of these spacetime manifolds and their physical interpretation
and implications is called the General Theory of Relativity. The subject is
vast and beautiful, but our objective in this chapter is modest in the extreme.
We will introduce just enough mathematics to describe a few of the most
elementary examples and study them a bit. We will find, remarkably enough,
that the field equations of general relativity admit solutions that correspond
to an “empty” universe, but differ from Minkowski spacetime and it is one of
these that we will briefly consider as a possible alternative to the model we
have been investigating.
4.3 Mathematical Machinery
In truth, the mathematical machinery required to study general relativity
properly is substantial (a good place to begin is [O’N]), but our goal here
is
no
t s
o lofty. The examples of interest to us are rather simple and we will
introduce just enough of this machinery to understand these and gain some
sense of what is required to proceed further. We begin with a synopsis of some
standard results from real analysis taking [Sp1] as our guide and reference.
For n ≥ 1wedenotebyR
n
the n-dimensional real vector space of ordered
n-tuples of real numbers.
R
n
= {p =(p
1
,...,p
n
):p
1
,...,p
n
∈ R}
The standard basis for R
n
will be written e
1
=(1, 0,...,0, 0),...,e
n
=
(0, 0,...,0, 1) and, for i =1,...,n, the standard coordinate functions
u
i
: R
n
→ R
are defined by
u
i
(p)=u
i
(p
1
,...,p
n
)=p
i
.
The usual Euclidean inner product on R
n
is defined by
p, q = p
1
q
1
+ ···+ p
n
q
n
and its corresponding norm and distance function d are given by
p
2
= p, p
and
d(p, q)= q −p .
4.3 Mathematical Machinery 203
For any p ∈ R
n
and any ε>0theopen ball of radius ε about p is
U
ε
(p)={q ∈ R
n
: d(p, q) <ε}.
A subset U of R
n
is said to be open in R
n
if, for each p ∈ U,thereisanε>0
such that U
ε
(p) ⊆ U. A subset K of R
n
is closed in R
n
if its complement
R
n
− K is open in R
n
. More generally, if X is an arbitrary subset of R
n
,
then U
⊆ X is said to be open in X ifthereisanopensetU in R
n
with
U
= U ∩ X. A subset K
of X is closed in X if X − K
is open in X and
this is the case if and only if there is a closed set K in R
n
with K
= K ∩X.
If X ⊆ R
n
and Y ⊆ R
m
, then any mapping F : X → Y has coordinate
functions F
i
,i=1,...,m, defined by
F (x)=(F
1
(x),...,F
m
(x))
for every x ∈ X. F is continuous on X if, for every open set V
in Y, F
−1
(V
)
is open in X and this is the case if and only if each F
i
: X → R is a
continuous real-valued function on X.IfU ⊆ R
n
is open, then a continuous
map F : U → R
m
of U into R
m
is said to be smooth (or C
∞
) on U if
its coordinate functions F
i
: U → R,i =1,...,m, have continuous partial
derivatives of all orders and types at every point of U. More generally, if X
is an arbitrary subset of R
n
and F : X → R
m
,thenF is said to be smooth
(or C
∞
) on X if, for each x ∈ X, there is an open set U in R
n
containing
x and a smooth map
ˆ
F : U → R
m
such that
ˆ
F | U ∩ X = F | U ∩ X.A
bijection F : X → Y is called a homeomorphism if F and F
−1
: Y → X are
both continuous; if F and F
−1
are both smooth, then F is a diffeomorphism.
We will leave it to the reader to check that identity maps are smooth and
restrictions and compositions of smooth maps are smooth.
We are, in fact, not particularly interested in arbitrary subsets of Euclidean
spaces, but only in rather special ones. As motivation for the definition to
come we will first work out an example. The n-dimensional sphere S
n
is the
subset of R
n+1
consisting of all points p with p
2
=1.
S
n
= {p =(p
1
,...,p
n
,p
n+1
) ∈ R
n+1
: p
2
=1}
The north pole of S
n
is the point N =(0,...,0, 1) and the south pole is
S =(0,...,0, −1). We define two open subsets U
S
= S
n
−{N} and U
N
=
S
n
−{S} of S
n
and two maps
ϕ
S
: U
S
−→ R
n
and
ϕ
N
: U
N
−→ R
n
.
Geometrically, these maps are quite simple. For each p ∈ U
S
,ϕ
S
(p)isthe
intersection with the coordinate hyperplane u
n+1
= 0 of the straight line in
R
n+1
from N through p (see Figure 4.3.1).
