Mrinal K Pal. Power system stability
Подождите немного. Документ загружается.

VOLTAGE STABILITY
10-3
or
−+
++=
RRRR
RS
V
QR
V
PX
j
V
QX
V
PR
VV
(10.4)
Approximate relationships
Since in a transmission line the resistance is small in relation to the reactance ( R << X ), it can
be neglected without affecting the accuracy significantly. In the analysis presented here,
inclusion of resistance will only serve to obscure the more important issues. The effect of line
resistance will therefore be ignored in all the subsequent analyses.
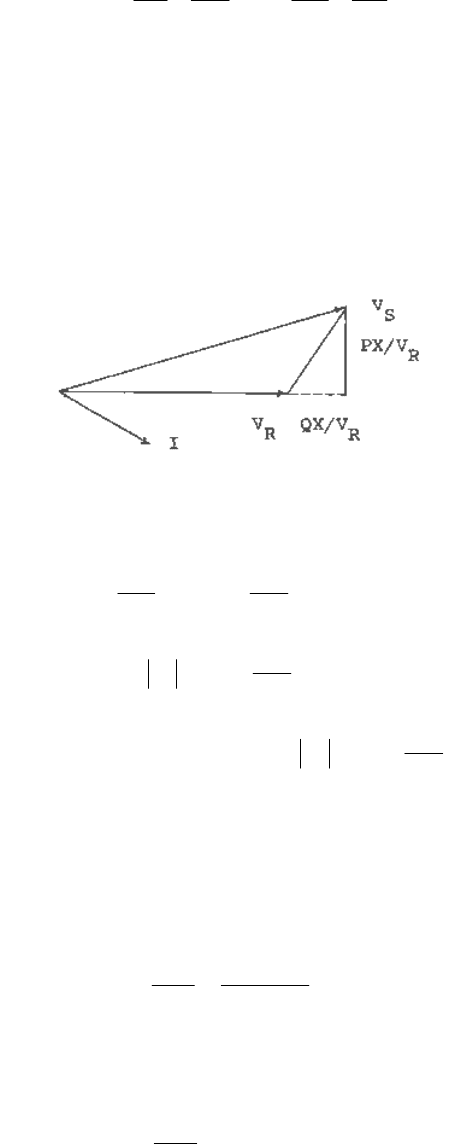
The approximate relationship of equation (10.4), neglecting resistance, is shown in the phasor
diagram of Figure 10.2.
Fig. 10.2 Phasor diagram pertaining to system of Fig.10.1.
Since, at normal levels of power flow
R
R
R
V
QX
V
V
PX
+<<
R
RS
V
QX
VV
+≈ (10.5)
(Note: If P and Q are specified at the sending end,
S
SR
V
QX
VV
−≈
)
Equation (10.5) shows that the voltage drop at the receiving end is predominantly dependent on
the reactive power delivered or the power factor of the load. At nominal levels of power transfer
at unity power factor over short to medium length transmission lines, the voltage drop at the
receiving end is minimal.
From (10.5)
RS
R
VV
X
dQ
dV
2−
≈
(10.6)
Under normal operating conditions
0.1
≈
≈
RS
VV
X
dQ
dV
R
−≈
∴
(10.7)
= –(reciprocal of the short-circuit current)
VOLTAGE STABILITY
10-4
As the reactive power flow increases and/or the active power reaches excessively high level, V
R
drops well below nominal value and the magnitude of
dQ
dV
R
increases significantly.
At low levels of active power flow, as
∞−→→→
dQ
dV
V
V
X
V
Q
R
S
R
S
and,
2
,
4
2
At
∞−==
dQ
dV
P
R
,0 when
X
V
Q
S
4
2
= (10.8)
This is the maximum amount of reactive power that can be delivered at the receiving end. At this
reactive level
2
S
R
V
V =
(10.9)
V
R
- V
S
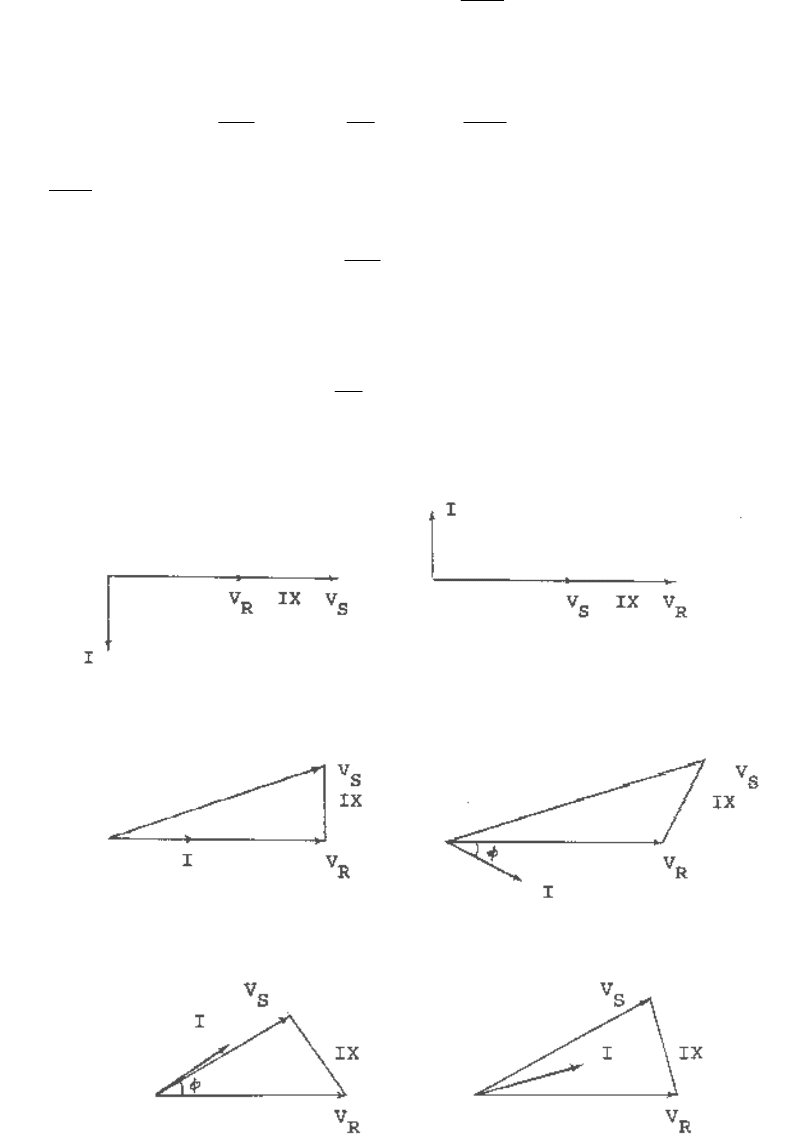
relationships under various receiving-end power factor conditions are illustrated by the
phasor diagrams in Figure 10.3.
(a) p.f. = 1.0, lagging (b) p.f. = 0, leading
(c) p.f. = 1.0 (d) p.f. = cos
φ
, lagging
(e) p.f.= cos
φ
, leading (f) p.f. adjusted for V
R
= V
S
Fig. 10.3 Phasor diagrams showing the V
R
- V
S
relationships at various power factors.
VOLTAGE STABILITY
10-5
From the above phasor diagrams it is clear that at a given power transfer, in order to maintain the
receiving-end voltage magnitude close to that at the sending end, a certain amount of reactive
support at the receiving end is necessary.
Power limit without specific voltage support at the receiving end
Consider the system shown in Figure 10.1, where the complex power P + jQ is being delivered at
the receiving end. From equation (10.4), neglecting line resistance,
22
2
+
+=
RR
RS
V
PX
V
QX
VV
from which
2
)(4)2()2(
2222222
2
XQXPQXVQXV
V
SS
R
+−−±−
=
(10.10)
Equation (10.10) shows that when the expression under the radical sign is positive, two solutions
for the receiving-end voltage are possible, both physically realizable. The solution having the
larger value (corresponding to the positive sign before the radical) represents the normal
operating condition. The solution with the lower value results in a correspondingly higher current
in order to meet the power demand. (Note that at P = 0, Q = 0, the two solutions correspond to
open- and short-circuit conditions, respectively.) The second solution is physically realizable
only for certain load types or load-voltage control combinations. In this operating state, an
increase in the power demand would be accompanied by an increase in current with a
disproportionately large drop in voltage and a runaway situation would occur if the load
attempted to restore to the demanded value. A total voltage collapse would possibly be averted
since below a certain voltage level most loads will cease to act as constant power load and resort
to constant current or constant impedance behavior. Operation in this region is not desirable. As
shown later, stable operation for constant power load can be realized in this operating state by
employing special controls. However, this may be impractical in most situations.
Writing Q = P tan
φ
, where
φ
is the power factor angle, (10.10) can also be expressed as
2
)tan1(4)tan2()tan2(
222222
2
φφφ
+−−±−
=
XPPXVPXV
V
SS
R
(10.11)
As the expression under the radical sign approaches zero, the two solutions approach each other.
No solution exists when the expression becomes negative. The maximum power that can be
delivered at the receiving end at a given power factor is, therefore, obtained by equating the
expression under the radical sign to zero. The maximum or critical power thus obtained is given
by
φ
φ
cos
sin1
2
2
max
−
=
X
V
P
S
(10.12)
The receiving-end voltage at the maximum power delivered is given by
φ
φ
2
cos
sin1
2
−
=
S
R
V
V
(10.13)
VOLTAGE STABILITY
10-6
Note that at unity power factor,
φ
= 0. Therefore
X
V
P
S
2
2
max
= (10.14)
and
S
S
R
V
V
V 7.0
2
≈= (18.15)
Using the expression for power,
δ
sin
X
VV
P
RS
= , and equations (10.14) and (10.15),
δ
at
maximum or critical power is given by
δ
= 45°. In the absence of specific voltage support at the
receiving end, the maximum power that can be delivered at unity power factor is, therefore,
given by equation (10.14), and this occurs at
δ
= 45°.
At power factors other than unity, from equations (10.12) and (10.13), and using the usual
expression for power transfer,
δ
φ
φ
δ
φ
φ
sin
cos
sin1
2
sin
cos
sin1
2
2
22
−
==
−
X
V
X
VV
X
V
SSRS
from which
2
sin1
sin
φ
δ
−
=
(10.16)
Therefore, at lagging power factors, the maximum power occurs at a power angle less than 45
°.
This point has important bearings in actual power system operation.
Problem: Show that when the transmission line has appreciable resistance, the maximum power
transfer at unity pf in the absence of specific voltage support at the receiving end occurs at a
power angle
δ
=
α
/2, where
α
is given by )/(tan
1
RX
−
=
α
, where R is the line resistance.
Plots of receiving-end voltage against power delivered at various power factors for a typical
system are shown in Figure 10.4. For satisfactory performance with good voltage profile the
operation should normally be restricted on the upper portion of the curves, well away from the
loading limit. It can be seen that when the system is operating close to the loading limit at a
given power factor, a slight change in the power factor can initiate a run away situation if the
load tended to restore to the demanded level. It can also be seen that although at unity and
lagging power factors the receiving-end voltage continuously drops as the power delivered is
increased and the voltage at maximum power is quite low compared with the sending-end
voltage, the situation is different at leading power factors. At leading power factors, the
receiving-end voltage can actually rise with increase in the power delivered, and the voltage at
maximum power can be quite high. In other words, at leading power factors, or if sufficient
reactive support is provided at the receiving end, the voltage at the maximum power point can be
comparable to or even higher than the sending-end voltage, although the maximum power would
be quite high. Therefore, the magnitude of the receiving-end voltage alone is not a good indicator
of closeness to the critical or maximum power point. In the same way, the change in the
receiving-end voltage corresponding to a change in the power delivered, dV
R
/dP, cannot be used
as a reliable guide to predict closeness to the maximum power point.
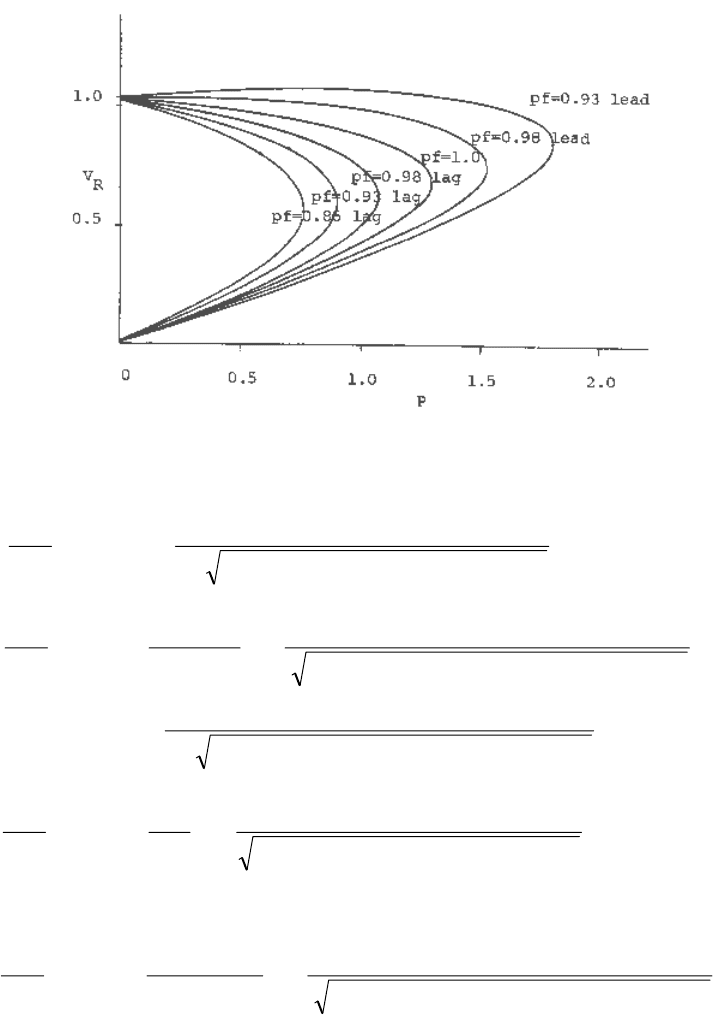
VOLTAGE STABILITY
10-7
The change in the receiving-end voltage with change in the reactive power at the receiving end,
on the other hand, is more or less independent of the power factor of the load, as seen from the
plots shown in Figure 10.4. This conclusion also follows from the analysis presented earlier,
where the observation was made that the receiving-end voltage is much more sensitive to
reactive power than to real power.
Fig. 10.4 Typical receiving-end voltage characteristics.
From (10.10) and (10.11), one can obtain the following derivatives:
)(4)2(
222222
2
constant
XQXPQXVV
PX
dP
dV
SR
Q
R
+−−
−
±=
(10.17)
)tan1(4)tan2(
)tan1(4)tan2(
1
2
tan
22222
2
22222
2
constant
φφ
φφ
φ
φ
+−−
−
±
+−−
±
−
=
XPPXVV
PX
XPPXV
V
V
X
dP
dV
SR
S
S
R
R
(10.18)
+−−
±
−
=
)(4)2(
1
2
222222
2
constant
XQXPQXV
V
V
X
dQ
dV
S
S
R
P
R
(10.19)
and
+−−
±
−
=
)tan1(4)tan2(
1
2
sec
22222
2
2
constant
φφ
φ
φ
XPPXV
V
V
PX
d
dV
S
S
R
P
R
(10.20)
All these derivatives approach infinity as the maximum power point is approached, since at that
point the expressions under the radical signs are zero. Also, at the first solution point,
corresponding to the positive sign before the radical in equation (10.10) or (10.11), the
derivatives are negative except for the one given by equation (10.18), which may be positive or
VOLTAGE STABILITY
10-8
negative depending on the power factor (leading or lagging) and the power level. At the second
solution point the derivatives are positive.
From the above expressions, it is also clear that for normal transmission line parameters, dV
R
/dQ
(or dV
R
/d
φ
) has greater magnitude than dV
R
/dP at any point in the upper portion of the curves in
Figure 10.4, and that it steadily increases as the maximum power point is approached. Although
at the maximum power point both dV
R
/dP and dV
R
/dQ become infinite, prior to reaching the
critical point dV
R
/dP may behave anomalously; at least, its variation with change in power level
near the critical point may not be indicative of the proximity to the critical point.
dV
R
/dQ, on the other hand, has the desirable property of gradually increasing in magnitude as the
critical point is approached, independent of the power factor of the load. As shown earlier, also
from (10.19), dV
R
/dQ ≈ −X (reciprocal of the short-circuit level at the receiving-end bus) in the
normal operating range. Any significant departure from this value would be indicative of
undesirable situation.
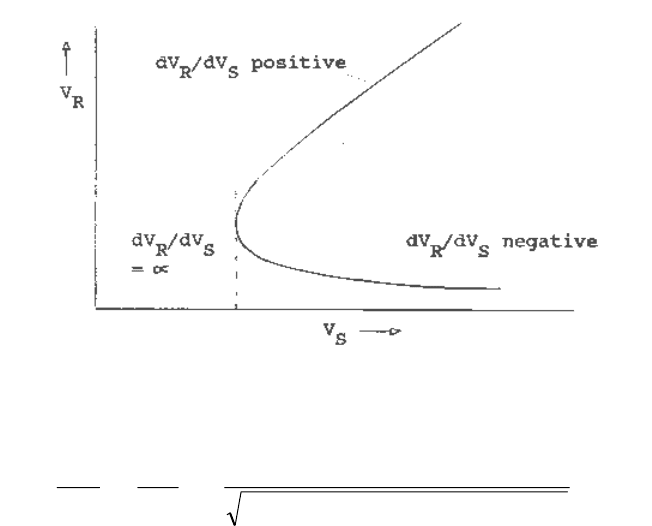
From equations (10.10) or (10.11) curves showing V
R
against V
S
could be drawn for given P and
Q (or pf) as shown in Figure 10.5. For each value of V
S
, two values of V
R
are possible
corresponding to the two signs before the radical. At the maximum power point these two values
coincide and dV
R
/dV
S
is infinite. For values of V
S
lower than this no solution exists. dV
R
/dV
S
is
positive at values of V
R
corresponding to the positive sign before the radical and represents
operation in the upper portion of the curve. Similarly, a negative value of dV
R
/dV
S
signifies
operation in the lower portion as indicated in Figure 10.5.
Fig. 10.5 Plots of V
R
against V
S
for given P and Q.
From (10.10), one can derive dV
R
/dV
S
as
+−−
−
±=
)(4)2(
2
1
2
222222
2
XQXPQXV
QXV
V
V
dV
dV
S
S
R
S
S
R
(10.21)
Equation (10.21) shows that dV
R
/dV
S
is positive in the normal operating region. Its value is
normally close to unity for operation well within the maximum power point. It rapidly increases
as the critical point is approached, becoming infinity at the critical point. A negative value would
indicate operation in the lower portion of the curve.
VOLTAGE STABILITY
10-9
Although the criterion dV
R
/dV
S
is equivalent to the criterion dV
R
/dQ and as convenient to apply
in the simple system studied so far, its application in more complex network can be cumbersome.
The use of other criteria, for example, dQ
S
/dQ
R
, is also possible. However, these are not deemed
suitable for general application.
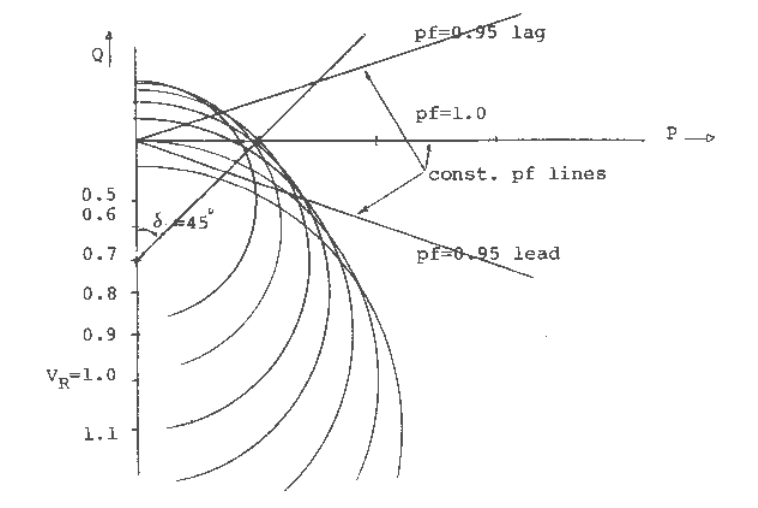
Power transfer at constant sending and receiving-end voltage
At constant sending- and receiving-end voltages, the power transfer increases with
δ
(equation
10.1)) until
δ
reaches 90°. For a given sending-end voltage, the receiving-end voltage can be held
constant by appropriately adjusting the reactive support at the receiving end as the power transfer
changes. The required reactive support to maintain the receiving-end voltage at a particular level
can be easily determined from the circle diagrams shown in Figure 10.6 (see Chapter 1 for
details of circle diagram). In Figure 10.6, a series of circles have been drawn corresponding to a
range of values for the receiving-end voltage, for a given sending-end voltage. For a given power
transfer, the required reactive support to maintain the receiving-end voltage at a specified level
can be read off the circle corresponding to that voltage. From Figure 10.6, it is also possible to
determine the receiving-end voltage for a given power and power factor by drawing the constant
power factor lines and noting the coordinate of the intersections of these lines with the circles. In
this way one can graphically construct the curves shown in Figure 10.4.
Fig. 10.6 Receiving-end circle diagram, V
S
= 1.0.
However, it will become clear from the analysis presented later in the chapter that, although with
reactive support at the receiving-end power transfer will increase with increasing
δ
, until
δ
reaches 90
°, in the absence of continuous voltage control, operation at
δ
> 45° (at unity power
factor) is not desirable.
VOLTAGE STABILITY
10-10
Effect of load characteristics
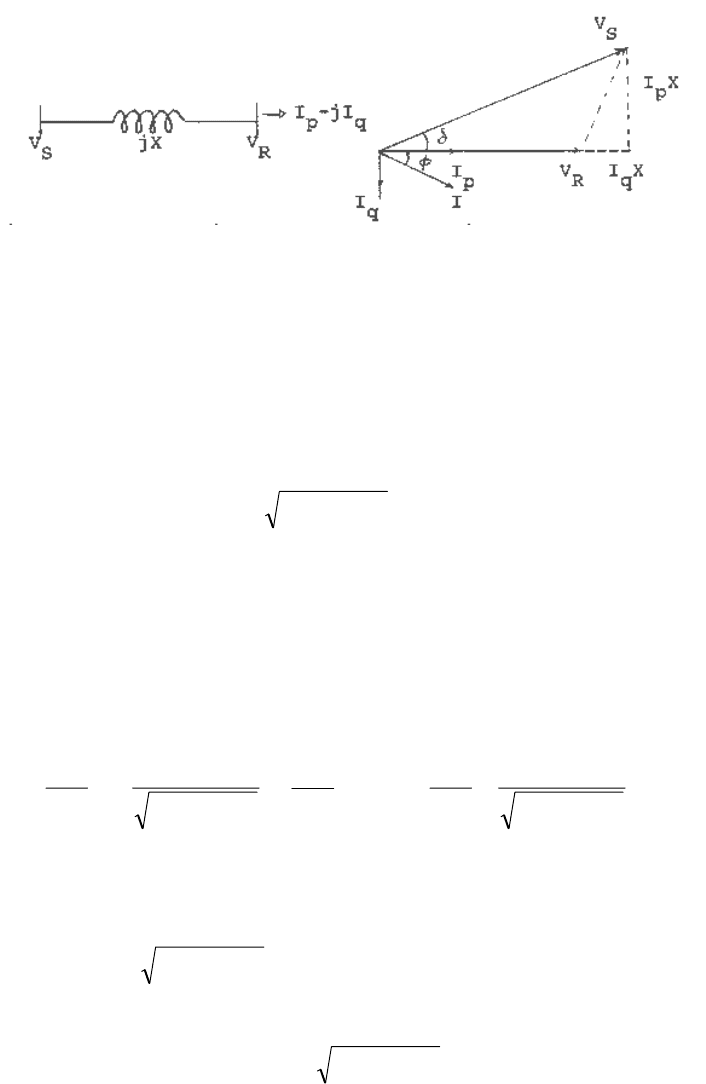
Constant current load
Consider a system supplying a constant current load I
p
− jI
q
as shown in Figure 10.7.
Fig. 10.7 Schematic of a system supplying a constant current load and phasor diagram.
From Figure 10.7 we can write, using V
R
as reference,
jXjIIVV
qpRS
)(
−
+
=
∠
δ
from which
2222
)( XIXIVV
pqRS
++=
The physically realizable solution for V
R
is obtained as
XIXIVV
qpSR
−−=
222
(10.22)
A necessary condition for the solution to exist is
XVIXIV
SppS
/or ≤≥ -- the short-circuit current at the receiving end.
However, for a non-zero solution of V
R
at
XVI
Sp
/
=
, the load must have a leading power factor
(I
q
negative).
From (10.22) we can obtain the following partial derivatives:
222
XIV
XI
I
V
pS
p
p
R
−
−=
∂
∂
, X
I
V
q
R
−=
∂
∂
,
222
XIV
V
V
V
pS
S
S
R
−
=
∂
∂
The first two derivatives are always negative and the third is always positive, indicating that the
current delivered can increase continuously, up to the limiting value (the short-circuit current),
although beyond a certain point the demanded MW/MVA will not be served.
Note that for I
q
= 0,
222
XIVV
pSR
−=
Therefore, the real power delivered
222
XIVIVIP
pSpRp
−==
Taking the partial derivative with respect to I
p
and equating to zero, we obtain the condition for
maximum power that can be delivered as
VOLTAGE STABILITY
10-11
X
V
I
S
p
2
=
and
2
222
S
pSR
V
XIVV
=−=
from which
X
V
P
S
2
2
max
=
δ
at maximum power is therefore equal to 45°.
The conditions for maximum power are thus the same as in constant power load.
Constant impedance load
Consider an impedance load
LL
jXR + , or in terms of admittance,
LL
jBG
−
, where
2222
and,
LL
L
L
LL
L
L
XR
X
B
XR
R
G
+
=
+
=
We can write
jXjBGVVV
LLRRS
)(
−
+
=∠
δ
from which
222
)1( XGXB
V
V
LL
S
R
++
=
(10.23)
The above expression shows that for constant impedance load the solution for V
R
is unique.
Note that at unity power factor, B
L
= 0, and
22
1 XG
V
V
L
S
R
+
=
22
2
2
1 XG
GV
GVP
L
LS
LR
+
==
∴
Taking the partial derivative with respect to G
L
and equating to zero, we obtain the condition for
maximum power as
G
L
X = 1
X
V
P
S
2
2
max
=
∴
, 45and,
2
max
==
P
S
R
V
V
δ
°
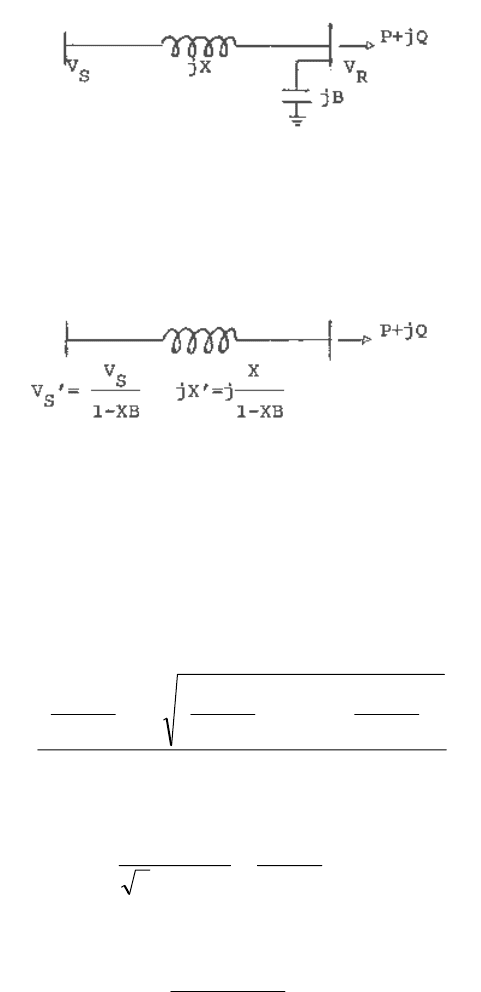
Effect of reactive support at the receiving end by shunt capacitors
Consider the system shown in Figure 10.8 where reactive support is provided at the receiving
end by shunt capacitors of admittance B (=
ω
C).
VOLTAGE STABILITY
10-12
Fig. 10.8 Schematic of a system with reactive support at the receiving end.
The system of Figure 10.8 can be converted to the Thevenin equivalent shown in Figure 10.9,
where the equivalent sending-end voltage and the equivalent line reactance are as shown the
figure.
Fig. 10.9 Thevenin equivalent of system of Fig. 10.8.
The system of Figure 10.9 is identical to the system studied earlier. Therefore, the expressions
derived earlier apply provided V
S
and X are replaced by their equivalent values.
For example, at unity power factor (Q = 0), the receiving-end voltage is given by, from equation
(10.10)
2
1
4
11
2
2
42
2
−
−
−
±
−
=
XB
X
P
XB
V
XB
V
V
SS
R
(10.24)
At maximum power
XB
V
XB
V
V
SS
R
−
≈
−
=
1
7.0
)1(2
(10.25)
and
)1(2
2
crit
XBX
V
P
S
−
=
(10.26)
As before, at maximum power
δ
= 45°.
Equation (10.26) shows the increase in maximum power that can be attained by the application
of shunt capacitors. There is also a proportionate increase in the receiving-end voltage at which
the maximum power occurs, as shown by equation (10.25). However, the angle at which the
maximum power occurs is still 45
°.
Example: Consider the system shown in Figure 10.10, where 1000 MW is being delivered at the
receiving end at 1.0 pu voltage. The sending-end voltage is also unity.
