Mrinal K Pal. Power system stability
Подождите немного. Документ загружается.

EFFECT OF EXCITATION ON STABILITY
6-7
)cos(
φ
θ
+
= Ii
q
(6.41)
θ
sin
td
Ve
=
(6.42)
θ
cos
tq
Ve
=
(6.43)
ddqq
ixee
′
+
=
′
(6.44)
The linearized model of the synchronous machine-infinite bus system described by equations
(6.17), (6.24), (6.29) and (6.30) is suitable for estimating machine damping under various
operating conditions. By adding to this model the equations representing the various controls
acting on the machine, we can evaluate the effects of the controls on machine damping.
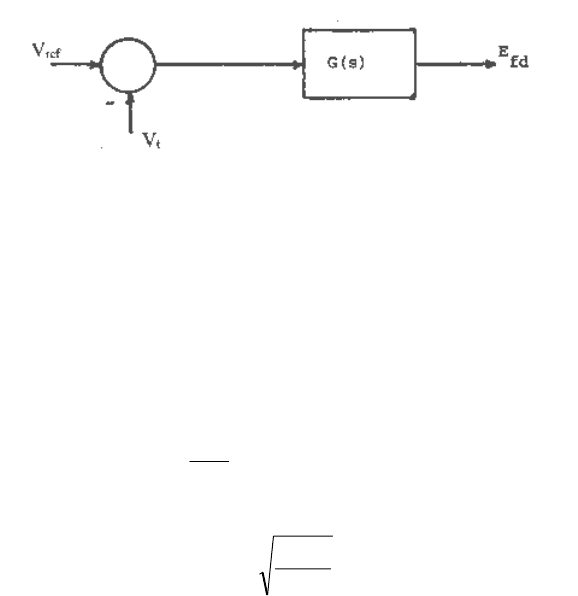
A schematic representation of the excitation control system is shown in Figure 6.5, where G(s)
represents the combined transfer function of the regulator and the exciter.
Fig. 6.5 Schematic of the excitation control.
From Figure 6.5 the linearized equation of the excitation control system can be written as
fdt
EVsG
∆
=
∆
− )( (6.45)
Synchronizing power coefficient with constant flux linkage
For constant flux linkage 0=
′
∆
q
e . Therefore, from (6.24),
δ
∆
=
∆
1
KP
e
. The synchronizing
power coefficient for constant flux linkage is therefore
1
K
P
e
=
∆
∆
δ
(6.46)
From (6.17), the natural frequency of oscillation for constant flux linkage is given by
H
K
n
2
1o
ω
ω
= (6.47)
Steady-state synchronizing power coefficent
(i) No voltage regulator action or constant field voltage
For constant field voltage,
0=∆
fd
E
. From equation (6.29), in the steady-state,
δ
∆
−
=
′
∆
43
KKe
q
Therefore, from equation (6.24),
(
)
δ
∆
−
=
∆
4321
KKKKP
e
from which the synchronizing power coefficient is

EFFECT OF EXCITATION ON STABILITY
6-8
4321
KKKK
P
e
−=
∆
∆
δ
(6.48)
(ii)
Effect of saturation
In order to keep the analysis simple, certain valid simplifying assumptions will be made. These
are:
1.
Saturation on the d axis can be expressed as a function of
[
]
)(
qq
efSe
′
=
′
only.
2.
x
q
(saturated value) is not affected appreciably by small changes in the operating point.
Therefore, the equations for the synchronous machine will be identical to equations (6.6) -
(6.12), except for equation (6.6) which will be replaced by
qdddfd
q
do
eSixxE
dt
ed
T
′
+−
′
−−=
′
′
)1()( (6.49)
where
)(
q
efS
′
=
(see Chapter 5)
Note that, in these equations, all the reactances, except x
q
, have unsaturated values.
Proceeding as before, after linearization of equations (6.7) - (6.12) and some algebraic
manipulations, we obtain
qe
eKKP
′
∆
+
∆
=
∆
21
δ
(6.24)
Linearizing equation (6.49), we have
qdddfd
q
do
ekixxE
dt
ed
T
′
∆+−∆
′
−−∆=
′
∆
′
)1()( (6.50)
where
q
q
e
e
S
Sk
′
′
∂
∂
+=
In the steady-state, assuming constant field voltage,
0
=
∆
fd
E
,
0)1()(
=
′
∆
+
+
∆
′
−
qddd
ekixx
Substituting the value of
∆i
d
from (6.22) and simplifying,
δ
∆
+
−=
′
∆
3
43
1 kK
KK
e
q
(6.51)
From (6.24) and (6.51), the steady-state synchronizing power coefficient can be derived as
3
432
1
1 kK
KKK
K
P
e
+
−=
∆
∆
δ
(6.52)
Comparing (6.52) with (6.48), it can be seen that the effect of saturation is to increase the
synchronizing power coefficient and, hence, extend the stability limit.
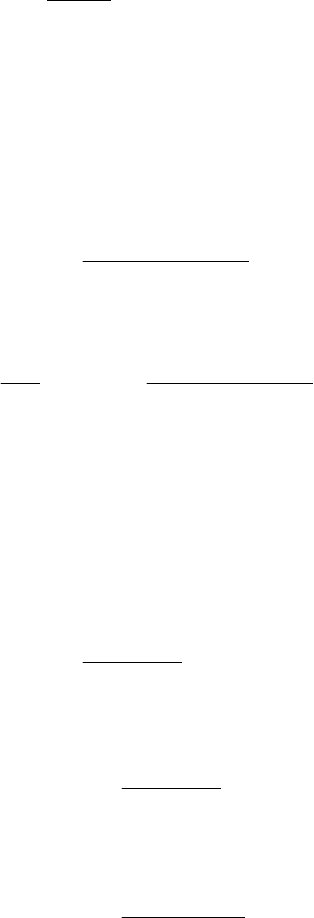
EFFECT OF EXCITATION ON STABILITY
6-9
(iii) With voltage regulator action
From (6.29), in the steady-state
δ
∆
−
∆
=
′
∆
433
KKEKe
fdq
(6.53)
Assuming that the excitation control is represented by a simple gain and time lag
s
K
sG
e
e
τ
+
=
1
)(
then, from equation (6.30) and (6.45),
(
)
qefd
eKKKE
′
∆
+
∆
−
=
∆
65
δ
(6.54)
since in the steady-state
0
=∆
ref
V
Substituting (6.54) into (6.53) and simplifying,
δ
∆
+
+
−=
′
∆
e
e
q
KKK
KKKKK
e
53
5343
1
(6.55)
Substituting (6.55) into (6.24), we have
e
ee
KKK
KKKKK
KK
P
53
5343
21
1+
+
−=
∆
∆
δ
(6.56)
Comparing (6.56) with (6.46), it is seen that for a value of K
e
equal to –K
4
/K
5
the synchronizing
power coefficient will be equal to that for constant flux linkage.
Evaluation of damping torque
(i) No regulator action
With no voltage regulator action
0
=
∆
fd
E
, and therefore from equation (6.29)
δ
∆
′
+
−=
′
∆
sTK
KK
e
do
q
3
43
1
(6.57)
Substituting (6.57) into (6.24), we have
δ
∆
′
+
−=∆
sTK
KKK
KP
do
e
3
432
1
1
(6.58)
For a frequency of oscillation
ω
, s can be replaced by j
ω
; then (6.58) reduces to
δ
ω
∆
′
+
−=∆
do
e
TKj
KKK
KP
3
432
1
1
(6.59)
The imaginary part of the above equation represents the damping torque component (the
component in quadrature with angle or in phase with speed). Note that at normal speed, the per
unit power and per unit torque are equal. Therefore, the damping torque is given by
Damping torque
δ
∆
=
jT
d
(6.60)
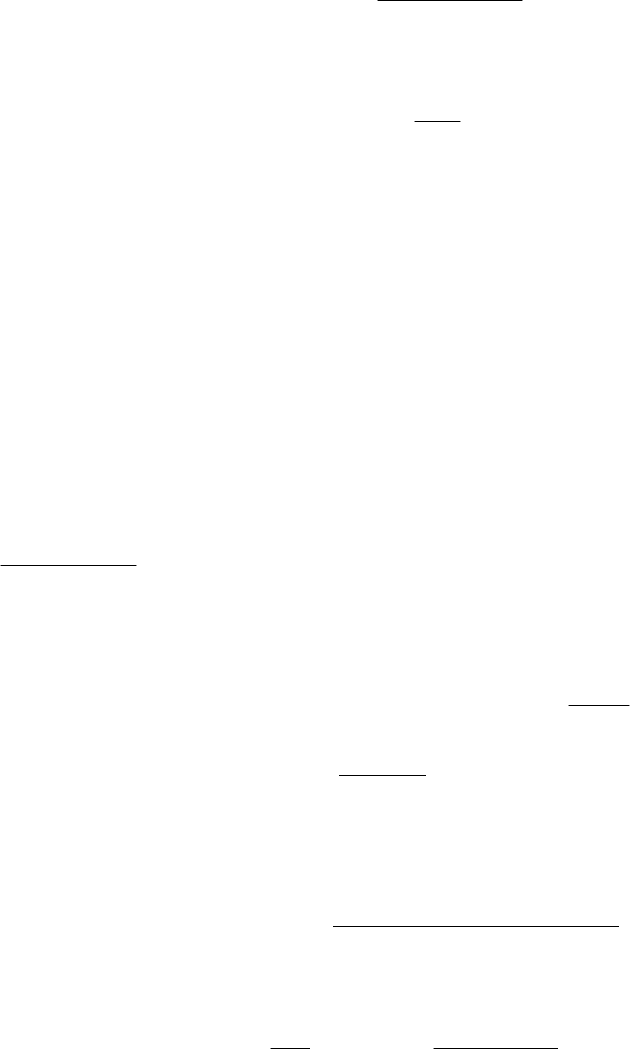
EFFECT OF EXCITATION ON STABILITY
6-10
where
22
3
2
4
2
32
1
do
do
d
TK
TKKK
T
′
+
′
=
ω
ω
(6.61)
The damping torque is also expressed as
Damping torque
δ
δ
∆=
∆
= sD
dt
d
D
where D is the equivalent damping constant.
At oscillation frequency
ω
, replacing s by j
ω
, the above can be written as
Damping torque
δ
ω
∆
=
jD (6.62)
Comparing (6.62) with (6.60), we can write
ω
/
d
TD
=
(6.63)
The per unit damping coefficient is, therefore
oo
)/(
ω
ω
ω
dd
TDK
=
= (6.64)
It may be seen from equation (6.59) that the synchronizing power coefficient is reduced
by
22
3
2
432
1
do
TK
KKK
′
+
ω
due to the demagnetizing effect of armature reaction. From (6.59), (6.60) and
(6.61) it may be concluded that, since K
2
, K
3
and K
4
are normally positive, a certain amount of
positive damping is introduced by the demagnetizing effect of armature reaction.
In situations where
22
3
2
do
TK
′
ω
>> 1, (6.61) reduces to
do
d
T
KK
T
′
=
ω
42
, and therefore
do
d
T
KK
K
′
=
2
42o
ω
ω
(6.65)
Substituting the expressions for K
2
, K
3
and K
4
in (6.61), the expression for the damping
coefficient can also be written as
2222
22
o
)()(
sin)(
dodede
doddb
d
Txxxx
TxxV
K
′′
+++
′′
−
=
ω
δω
(6.66)
From (6.59), the synchronizing power coefficient is given by
22
3
2
432
1
1
do
e
TK
KKK
KK
P
′
+
−==
∆
∆
ω
δ
(6.67)
Therefore, from (6.17), the natural frequency of oscillation in the absence of voltage regulator
action is given by

EFFECT OF EXCITATION ON STABILITY
6-11
H
K
2
o
ω
ω
=
(6.68)
Example
As an example, consider the system shown in Figure 4 with the following parameter values,
representative of a typical nuclear generator.
3.0,0.4,0.6,35.0,42.1,48.1
=
=
=
′
=
′
==
edodqd
xHTxxx
All values, except
do
T
′
, are in per unit on machine rated MVA base. Assume an operating point
where P = 1.0, V
t
= 1.06 and V
b
= 1.05.
Initial operating point values are, from equations (6.35) through (6.46),
o
69.61,013.1,7357.0,7631.0,5375.0,7923.0 ==
′
====
δ
qqdqd
eeeii
K
1
through K
6
are computed, from the expressions given in equations (6.25) through (6.32), as
K
1
= 1.3566, K
2
= 1.4223, K
3
= 0.3652, K
4
= 1.6071, K
5
= -0.0495, K
6
= 0.3203
The natural frequency calculated from equation (6.68) is approximately 7.9 rad/sec. The per unit
damping coefficient computed from equations (6.61) and (6.64) is K
d
= 2.32. The damping
coefficient computed from the approximate expression, (6.65), is K
d
= 2.33.
It may be seen, from (6.61) and (6.64), that at very low frequency of oscillations (
ω
≈ 0), the
damping coefficient becomes
dod
TKKKK
′
≈
4
2
32o
ω
(6.69)
(ii)
Including voltage regulator action
Substituting (6.30) into (6.45), and the result in (6.29), with
∆V
ref
= 0, we obtain
δδ
∆
′
+
−
′
∆+∆
′
+
−
=
′
∆
sTK
KK
sGeKK
sTK
K
e
do
q
do
q
3
43
65
3
3
1
)(][
1
which yields
δ
∆
′
+
+
+
′
+
−=
′
∆
)(
1
1
)(1
1
3
63
4
5
3
43
sG
sTK
KK
sG
K
K
sTK
KK
e
do
do
q
(6.70)
A comparison of the above expression with equation (6.57) shows that, under operating
conditions where K
5
is negative, the action of excitation control with transfer functions
commonly encountered in power systems will result in a reduction in the machine damping
produced by demagnetizing effect of armature reaction.
Following the steps outlined earlier, the damping torque coefficient is obtained from (6.24) and
(6.70) as
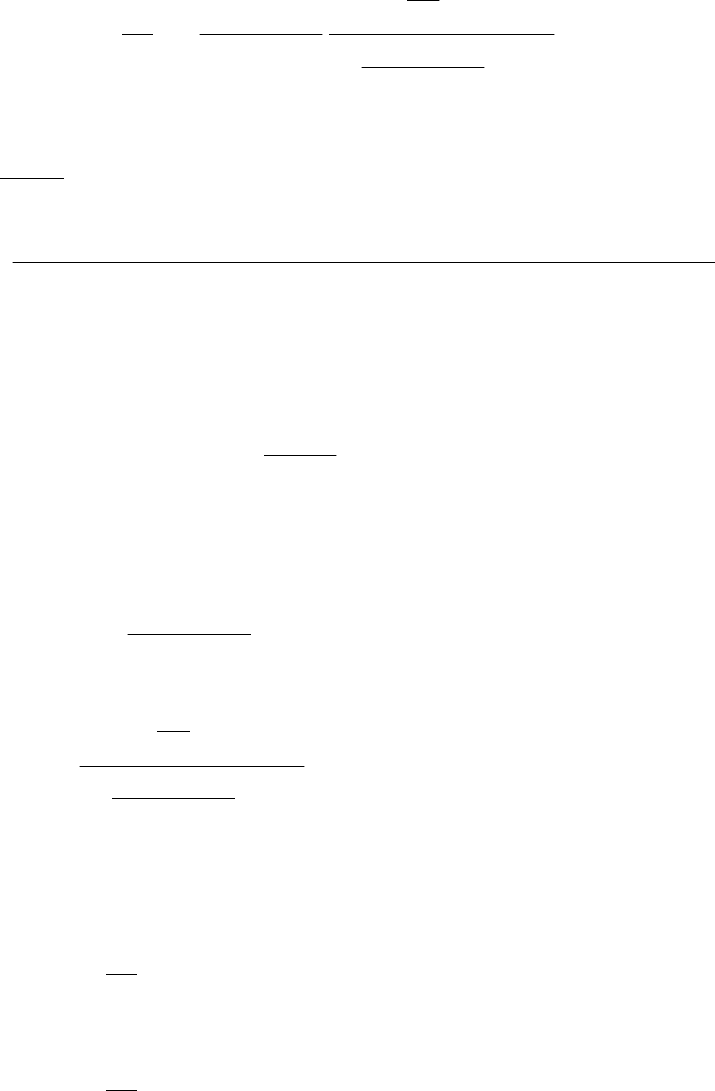
EFFECT OF EXCITATION ON STABILITY
6-12
′
+
+
+
′
+
−
=
)(
1
1
)(1
1
Im
3
63
4
5
3
432o
ω
ω
ω
ωω
ω
jG
TKj
KK
jG
K
K
TKj
KKK
K
do
do
d
(6.71)
For an exciter control with a simple gain and time lag, the transfer function
G(s) is represented
by
s
K
sG
e
e
τ
+
=
1
)(
, and the damping torque constant is obtained as
()()
(
)
()
()
2
3
2
2
2
63
2
634234252
//1
/1/
KTTKKK
TKKKKKKTKKKKK
T
edoedoe
edoeeedoe
d
τωτω
τωτωτω
+
′
+
′
−+
′
−+−+
′
+
=
(6.72)
Example
In the previous example, consider a fast acting high gain excitation control system having an
equivalent transfer function given by
s
sG
1.01
50
)(
+
=
which yields
o
13.3833.39)( −∠=
ω
jG
Performing the numerical computations, we have
o
67.86034.0
1
3
43
−∠−=
′
+
−
do
TKj
KK
ω
and
o
83.100856.0
)(
1
1
)(1
3
63
4
5
∠=
′
+
+
+
ω
ω
ω
jG
TKj
KK
jG
K
K
do
This example clearly demonstrates the mechanism of the reduction of machine damping by
conventional excitation control. It can be seen that, in this particular case, the resultant damping
is negative. Completing the computation, we have
]83.100856.067.86034.0[Im
2
o
oo
∠×−∠×−= KK
d
ω
ω
= – 0.48
Recall that for fixed excitation the damping coefficient would be
]67.86034.0[Im
2
o
o
−∠×−= KK
d
ω
ω
= 2.23
Next, consider a slow acting low gain excitation control system having an equivalent transfer
function given by
EFFECT OF EXCITATION ON STABILITY
6-13
s
sG
+
=
1
10
)( or,
o
74.82264.1)( −∠=
ω
jG
Performing the necessary computations, we have
]3.2.167.86034.0[Im
2
o
oo
∠×−∠×−= KK
d
ω
ω
= 2.31
Thus, the reduction in damping due to this particular slow acting excitation control is negligible.
The transfer functions of actual excitation control systems are much more complex than the
simple transfer function used in the above example. However, at a particular frequency of
oscillation
ω
, all transfer functions are easily reducible to the form G(j
ω
) = |G|∠
θ
and, therefore,
can be readily incorporated in the numerical computation.
As an example, consider the IEEE type 1 excitation system shown in lock diagram form in
Figure 6.6
a
Fig. 6.6
a Block diagram of the IEEE type 1 excitation system.
In linearized form this reduces to the block diagram shown in Figure 6.6
b
Fig. 6.6b Linearized block diagram of the system shown in Fig. 6.6a.
EFFECT OF EXCITATION ON STABILITY
6-14
Applying the block diagram reduction technique (see Appendix B), the above can be reduced to
the form
Fig. 6.7 Reduced form of the block diagram shown in Fig. 6.6
b.
where
)1()()1(
)1(
)(
sTsTKsTsKK
sTK
sG
FEEAAF
FA
++
′
++
+
=
EfdEE
SBEKK )1(
+
+=
′
Example
Consider the following set of parameter values.
K
A
= 400, T
A
= 0.01, K
E
= 1.0, T
E
= 1.079, K
F
= 0.079, T
F
= 1.0
S
E
at 75%E
fd max
= 0.568, S
E
at E
fd max
= 1.322
E
fd max
= 4.122
Using these parameter values and setting
s = j
ω
= j7.85, G(j
ω
) is computed as
o
2.215.11)( −∠=
ω
jG
Using this value of
G(j
ω
), the damping coefficient in the previous example is computed as
]17.15.697.067.86034.0[Im
2
o
oo
∠×−∠×−= KK
d
ω
ω
= 1.54
Note the reduction in damping due to the action of the excitation control.
Next, consider the following values
K
A
= 69.5, T
A
= 0.01, K
E
= 1.0, T
E
= 3.2, K
F
= 0.143, T
F
= 1.0
S
E
at 75%E
fd max
= 0.087, S
E
at E
fd max
= 0.875
E
fd max
= 5.991
For this set of parameter values,
G(j
ω
) is computed as
o
5.715.2)( −∠=
ω
jG
The damping coefficient is computed from
]7.4.995.067.86034.0[Im
2
o
oo
∠×−∠×−= KK
d
ω
ω
= 2.3
Note that the reduction in damping is negligible.
EFFECT OF EXCITATION ON STABILITY
6-15
Effects of Damper Windings
(a)
One damper winding on the q axis, no damper winding on the d axis
The equations for the synchronous machine with one damper winding on the q axis (model 2)
are:
qdddfd
q
do
eixxE
dt
ed
T
′
−
′
−−=
′
′
)( (6.73)
dqqq
d
qo
eixx
dt
ed
T
′
−
′
−=
′
′
)( (6.74)
qddqqqdde
iixxieieP )(
′
−
′
+
′
+
′
=
(6.75)
qqdd
ixee
′
+
′
=
(6.76)
ddqq
ixee
′
−
′
=
(6.77)
From (6.76), (6.77) and (6.11), (6.12), we obtain
de
b
de
q
d
xx
V
xx
e
i
′
+
−
′
+
′
=
δ
cos
(6.78)
qe
b
qe
d
q
xx
V
xx
e
i
′
+
+
′
+
′
−
=
δ
sin
(6.79)
Linearization of equations (6.73) through (6.79) yields
qdddfd
q
do
eixxE
dt
ed
T
′
∆−∆
′
−−∆=
′
∆
′
)( (6.80)
dqqq
d
qo
eixx
dt
ed
T
′
∆−∆
′
−=
′
∆
′
)(
(6.81)
)()(
qdqddqqqqqdddde
iiiixxieieieieP ∆
+
∆
′
−
′
+
∆
′
+
′
∆
+
∆
′
+
′
∆=∆ (6.82)
qqdd
ixee
∆
′
+
′
∆=∆
(6.83)
ddqq
ixee
∆
′
−
′
∆=∆ (6.84)
δ
δ
∆
′
+
+
′
∆
′
+
=∆
de
b
q
de
d
xx
V
e
xx
i
sin
1
(6.85)
δ
δ
∆
′
+
+
′
∆
′
+
−=∆
qe
b
d
qe
q
xx
V
e
xx
i
cos
1
(6.86)
Substituting (6.85) and (6.86) into (6.82) and rearranging, we obtain
dqe
eKeKKP
′
∆
+
′
∆
+
∆
=∆
921
δ
(6.87)
where
EFFECT OF EXCITATION ON STABILITY
6-16
[][]
qe
b
ddqq
de
b
qdqd
xx
V
ixxe
xx
V
ixxeK
′
+
′
−
′
+
′
+
′
+
′
−
′
+
′
=
δδ
cos
)(
sin
)(
1
(6.88)
de
b
q
de
qe
de
d
xx
V
i
xx
xx
xx
e
K
′
+
=
′
+
′
+
+
′
+
′
=
δ
sin
2
(6.89)
qe
b
q
qe
de
qe
q
xx
V
i
xx
xx
xx
e
K
′
+
−=
′
+
′
+
+
′
+
′
−=
δ
cos
9
(6.90)
Substituting (6.85) into (6.80) and (6.86) into (6.81), and rearranging, we obtain
δ
∆
′
+
−∆
′
+
=
′
∆
sTK
KK
E
sTK
K
e
do
fd
do
q
3
43
3
3
11
(6.91)
where
de
de
xx
xx
K
+
′
+
=
3
(6.92)
δ
sin
4 b
de
dd
V
xx
xx
K
′
+
′
−
=
(6.93)
and
δ
∆
′
+
=
′
∆
sTK
KK
e
qo
d
7
87
1
(6.94)
where
qe
qe
xx
xx
K
+
′
+
=
7
(6.95)
δ
cos
8 b
qe
qq
V
xx
xx
K
′
+
′
−
= (6.96)
Substituting (6.85) and (6.86) into (6.84) and (6.83), respectively, and the results in (6.21), and
rearranging, we obtain
dqt
eKeKKV
′
∆
+
′
∆
+
∆=∆
1065
δ
(6.97)
where
δδ
sincos
5 b
t
q
de
d
b
t
d
qe
q
V
V
e
xx
x
V
V
e
xx
x
K
′
+
′
−
′
+
′
=
(6.98)
t
q
de
e
V
e
xx
x
K
′
+
=
6
(9.99)
t
d
qe
e
V
e
xx
x
K
′
+
=
10
(6.100)
