Kopnin N.B. Theory of Nonequilibrium Superconductivity
Подождите немного. Документ загружается.


PREFACE
After more than four decades of existence, the microscopic theory of
superconnductivity due to Bardeen, Cooper, and Schrieffer (Bardeen et al. 1957)
(BCS) has established itself as one of the most beautiful theories in condensed
matter physics. Based on quite simple principles, it gives surprisingly good
description of many properties of superconductors. Before the discovery of high
temperature superconductors, the BCS theory with a phonon mediated electron
coupling could be regarded as an almost perfect piece of art. The high
temperature superconductivity appeared to be a challenge for the microscopic
theory. Apparently, its complicated nature goes, strictly speaking, beyond the
BCS model, and many attempts have been undertaken to build a microscopic
picture of this mysterious phenomenon. However, no reliable and commonly
accepted theory has emerged. Instead, the last decade of intensive studies in the
high temperature superconductivity revealed yet another important advantage of
the classical BCS theory: Sometimes it works reasonably well even when it is not
expected do so! It still remains the best available theory to describe the new
superconductors. It also appears that the BCS model originally designed to
describe the s-wave pairing in conventional (low temperature) superconductors,
can easily be generalized to deal with unconventional superfluids and
superconductors. The first successful application was to the p-wave superfluidity
in
3
He Fermi liquid. Here it provides the theory of a very exciting state with
much more complicated and rich structure of the superfluid order parameter
(Leggett 1975, Wölfle and Vollhardt 1990, Volovik 1992). Heavy-Fermionic and
high-temperature d-wave superconductors are examples where the BCS model
can also be used with a great chance for a success (Mineev and Samokhin 1999).
This is why the interest in the BCS theory remains alive despite its quite
respectable age.
The BCS model grew into a highly powerful theory of superconductivity also
because of its formulation in terms of the Green functions by Gor’kov (1958).
The Green function technique constitutes a complete tool for solving almost any
problem within the BCS theory. A very important and useful improvement in the
Green function theory of superconductivity has been provided by the so-called
quasiclassical method initiated by Eilenberger (1968). The method operates with
the Green functions integrated over the energy near the Fermi surface of the
normal state. This method is based on the fact that the energies involved in the
superconducting phenomena are normally much smaller than the Fermi energy
which is the scale of variations of the Green function in the normal state. In the
quasiclassical scheme, the calculations are reduced to a more or less automatic
action provided the problem is adequate for the model. It is the quasiclassical
version of the Green function formalism which is now most frequently used for
calculations of various properties of superconductors.
end p.v
Top
Privacy Policy and Legal Notice © Oxford University Press, 2003-2010. All rights reserved.
Kopnin, Nikolai, Senior Scientist, Low Temperature Laboratory, Helsinki University of
Technology, and L.D. Landau Institute for Theoretical Physics, Moscow
Theory of Nonequilibrium Superconductivity
Print ISBN 9780198507888, 2001
pp. [v]
第1页 共1页 2
010-8-8 10:29

Despite the quasiclassical methods being widely used for practical purposes, their
description is hard to find in the textbook literature. The review by Serene and
Rainer (1983) gives an introduction to the quasiclassical approach. The book by
Svidzinskii (1982) deals with the quasiclassical theory of static properties of
weak links. Applications of quasiclassical methods to various problems in
nonstationary superconductivity can only be found in original papers and
specialized reviews such as, for example, a review by Larkin and Ovchinnikov
(1986). The aim of the present book is to provide a basic knowledge of the
microscopic theory of nonstationary superconductivity including the most
advanced quasiclassical methods. We assume that the reader is familiar with the
main ideas of the BCS theory of superconductivity. There are many books on the
principles of the BCS theory, and we do not intend to give a comprehensive list of
them here. The personal preference of the author is with the book by de Gennes
(1966) which contains all the fundamentals which we would need to proceed with
the more recent microscopic theory.
We try to describe the quasiclassical method in such a way that a newcomer to
the field could learn it in a short time. First, we discuss stationary,
time-independent properties. We shall learn about the Green function, how to
find it for a particular superconducting state, and how to calculate the
superconducting properties once the Green function is known. The nonstationary
theory of superconductivity requires more efforts, which necessarily use
principles developed for stationary problems. In the theory of nonstationary
phenomena, the basic concept is the distribution function of excitations. The
specifics of superconductors is that the interaction of a superconductor with an
external electromagnetic field normally causes a considerable distortion of the
quasiparticle distribution on the energy scale relevant for the superconducting
parameters. As a result, the behavior of nonequilibrium excitations becomes one
of the most important factors which govern the response of the superconductor
to applied fields. This is why the problem of formulating the correct description of
the quasiparticle distribution is of the major concern throughout the book.
We consider several applications of both stationary and nonstationary
quasiclassical theory. For example, we derive and analyze the time-dependent
Ginzburg–Landau theory which is most frequently used to describe the dynamics
of superconductors. We demonstrate how powerful this theory is within certain
limits and, at the same time, we emphasize that it is far from being a complete
story about the kinetics of superconductors.
The great deal of attention is paid to the dynamics of vortices in the mixed state
of type II superconductors. There are two good reasons for the choice. First, it is
well established now that the dynamics of vortices controls almost all the
magnetic properties of type II superconductors, especially those of
high-temperature superconductors. It also determines hydrodynamics of
superfluids. Moreover, dynamics of superfluid vortices is now believed to have a
close relation to other fields of physics such as high energy physics and
cosmology (Achucarro and Vachaspati 2000, Shellard and Vilenkin 1994).
Second, the vortex dynamics has been the major interest of the author’s
research during many years, and it
end p.vi
Top
Privacy Policy and Legal Notice © Oxford University Press, 2003-2010. All rights reserved.
Kopnin, Nikolai, Senior Scientist, Low Temperature Laboratory, Helsinki University of
Technology, and L.D. Landau Institute for Theoretical Physics, Moscow
Theory of Nonequilibrium Superconductivity
Print ISBN 9780198507888, 2001
pp. [vi]
第1页 共1页 2
010-8-8 10:34

is hard to resist the temptation to say something about this fascinating subject.
The reader is assumed to be familiar with the basic properties of vortices at least
within the framework of the usual Ginzburg–Landau theory. Here we concentrate
on the motion of vortices under the action of a current passing through a
superconductor in the so-called flux flow regime. We shall see that, in presence
of vortices, the superconductor is no longer “superconducting” in a practical
sense: it offers a resistivity to the current! This is why the vortex dynamics plays
an important role in the physics of superconductors.
Of course, there are very many interesting and important phenomena left
beyond the scope of this book. For example, we do not consider Josephson
junctions and weak links; some aspects of this problem can be found in review by
Aslamazov and Volkov (1986), and in books by Likharev (1986) and Tinkham
(1996). We do not discuss propagation of sound through superconductors (see,
for example, Bulyzhenkov and Ivlev 1976 a, 1976 b). Neither we consider
thermoelectric phenomena, etc. Each of these topics deserves a book of its own.
In this context, we mention the book by Geilikman and Kresin (1974) which
treats acoustic, thermoelectric, and some other effects with the standard
Boltzmann kinetic equation. Nevertheless, the quasiclassical scheme is not
included there. We hope, therefore, that our presentation provides a basis for
description of these and many other properties of superconductors in a coherent
way using the simple and more advanced quasiclassical method.
The choice of the contents and of the presentation throughout the book is to a
larger extent affected by the research in the theory of superconductivity which
has been conducted at the Landau Institute for Theoretical Physics in Moscow. I
had the privilege to work together with many brilliant scientists during the time
when the Landau Institute was blooming with great scientific discoveries in a
unique unforgettable creative atmosphere. I have benefited a lot from the
Landau Institute seminars and discussions with A. Abrikosov, S. Brazovskii, I.
Dzialoshinskii, G. Eliashberg, M. Feigel’man, L. Gor’kov, S. Iordanskii, B. Ivlev, I.
Kats, I. Khalatnikov, D. Khmelnitskii, A. Larkin, V. Mineev, Yu. Ovchinnikov, V.
Pokrovskii, G. Volovik and many, many more. It is hard to over-estimate their
influence and the effect of great ideas that are generously shared by them with
everybody who is interested in physics. My special thanks are to D. Khmelnitskii
who read the manuscript and made valuable remarks helping to improve the
presentation.
The book is partially based on the lecture courses given at the Université
Paris-Sud, Orsay, France, and at the Low Temperature Laboratory, Helsinki
University of Technology, Finland. It is intended for graduate and post-graduate
students, and for researchers who work in the condensed matter theory.
Moscow and Espoo N.B.K.
1997 – 2000
end p.vii
Top
Privacy Policy and Legal Notice © Oxford University Press, 2003-2010. All rights reserved.
Kopnin, Nikolai, Senior Scientist, Low Temperature Laboratory, Helsinki University of
Technology, and L.D. Landau Institute for Theoretical Physics, Moscow
Theory of Nonequilibrium Superconductivity
Print ISBN 9780198507888, 2001
pp. [vii]
第1页 共1页 2010
-8-8 10:36

�
end p.ix
Kopnin, Nikolai, Senior Scientist, Low Temperature Laboratory, Helsinki University of
Technology, and L.D. Landau Institute for Theoretical Physics, Moscow
Theory of Nonequilibrium Superconductivity
Print ISBN 9780198507888, 2001
pp. [ix]
CONTENTS
I GREEN FUNCTIONS IN THE BCS THEORY
1 Introduction 3
2 Green Functions 27
3 The Bcs model 42
1.1 Superconducting variables 3
1.1.1 Ginzburg–Landau theory 4
1.1.2 Example: Vortices in type II superconductors 8
1.1.3 Bogoliubov–de Gennes equations 15
1.1.4 Quasiclassical approximation 16
1.2 Nonstationary phenomena 18
1.2.1 Time-dependent Ginzburg–Landau theory 18
1.2.2 Microscopic argumentation 21
1.2.3 Boltzmann kinetic equation 23
1.3 Outline of the contents 25
2.1 Second quantization 27
2.1.1 Schrödinger and Heisenberg operators 29
2.2 Imaginary-time Green function 30
2.2.1 Definitions 30
2.2.2 Example: Free particles 34
2.2.3 The Wick theorem 36
2.3 The real-time Green functions 38
2.3.1 Definitions 38
2.3.2 Analytical properties 39
3.1 BCS theory and Gor’kov equations 42
3.1.1 Magnetic field 47
3.1.2 Frequency and momentum representation 48
3.1.3 Order parameter of a d-wave superconductor 52
3.2 Derivation of the Bogoliubov–de Gennes equations 53
3.3 Thermodynamic potential 55
3.4 Example: Homogeneous state 57
3.4.1 Green functions 57
3.4.2 Gap equation for an s-wave superconductor 58
3.5 Perturbation theory 60
3.5.1 Diagram technique 60
3.5.2 Electric current 61

end p.x
Top
Privacy Policy and Legal Notice © Oxford University Press, 2003-2010. All rights reserved.
Kopnin, Nikolai, Senior Scientist, Low Temperature Laboratory, Helsinki University of
Technology, and L.D. Landau Institute for Theoretical Physics, Moscow
Theory of Nonequilibrium Superconductivity
Print ISBN 9780198507888, 2001
pp. [x]
4 Superconducting Alloys 64
II QUASICLASSICAL METHOD
5 General Principles of the Quasiclassical Approximation 77
6 Quasiclassical Methods in Stationary Problems 101
7 Quasiclassical Method for Layered Superconductors 125
4.1 Averaging over impurity positions 64
4.1.1 Magnetic impurities 70
4.2 Homogeneous state of an s-wave superconductor 71
5.1 Quasiclassical Green functions 77
5.2 Density, current, and order parameter 81
5.3 Homogeneous state 84
5.4 Real-frequency representation 86
5.4.1 Example: Homogeneous state 86
5.5 Eilenberger equations 88
5.5.1 Self-energy 90
5.5.2 Normalization 92
5.6 Dirty limit. Usadel equations 94
5.7 Boundary conditions 96
5.7.1 Diffusive surface 96
6.1 s-wave superconductors with impurities 101
6.1.1 Small currents in a uniform state 101
6.1.2 Ginzburg–Landau theory 103
6.1.3 The upper critical field in a dirty alloy 107
6.2 Gapless s-wave superconductivity 109
6.2.1 Critical temperature 110
6.2.2 Gap in the energy spectrum 111
6.3 Aspects of d-wave superconductivity 113
6.3.1 Impurities and, d-wave superconductivity 113
6.3.2 Impurity-induced gapless excitations 114
6.3.3 The Ginzburg–Landau equations 115
6.4 Bound states in vortex cores 117
6.4.1 Superconductors with s-wave pairing 118
6.4.2 d-wave superconductors 122
7.1 Quasiclassical Green functions 125
7.2 Eilenberger equations for layered systems 128
7.3 Lawrence–Doniach model 129
7.3.1 Order parameter 130
7.3.2 Free energy and the supercurrent 132
7.3.3 Microscopic derivation of the supercurrent 134
7.4 Applications of the Lawrence–Doniach model 135
7
.4
.1 Upper critical field 136
7.4.2 Intrinsic pinning 137
第1页 共1页 2010
-8-8 10:40

end p.xi
Top
Privacy Policy and Legal Notice © Oxford University Press, 2003-2010. All rights reserved.
Kopnin, Nikolai, Senior Scientist, Low Temperature Laboratory, Helsinki University of
Technology, and L.D. Landau Institute for Theoretical Physics, Moscow
Theory of Nonequilibrium Superconductivity
Print ISBN 9780198507888, 2001
pp. [xi]
III NONEQUILIBRIUM SUPERCONDUCTIVITY
8 Nonstationary Theory 143
9 Quasiclassical Method for Nonstationary Phenomena 170
10 Kinetic Equations 186
11 The Time-dependent Ginzburg–Landau theory 213
8.1 The method of analytical continuation 143
8.1.1 Clean superconductors 145
8.1.2 Impurities 149
8.1.3 Order parameter, current, and particle density 152
8.2 The phonon model 152
8.2.1 Self-energy 152
8.2.2 Order parameter 157
8.3 Particle–particle collisions 159
8.4 Transport-like equations and the conservation laws 161
8.5 The Keldysh diagram technique 163
8.5.1 Definitions of the Keldysh functions 163
8.5.2 Dyson equation 167
8.5.3 Keldysh functions in the BCS theory 168
9.1 Eliashberg equations 170
9.1.1 Self-energies 172
9.1.2 Order parameter, current, and particle density 174
9.1.3 Normalization of the quasiclassical functions 174
9.2 Generalized distribution function 175
9.3 s-wave superconductors with a short mean free path 177
9.4 Stimulated superconductivity 181
10.1 Gauge-invariant Green functions 186
10.1.1 Equations of motion for the invariant functions 188
10.2 Quasiclassical kinetic equations 192
10.2.1 Superconductors in electromagnetic fields 194
10.2.2 Discussion 197
10.3 Observables in the gauge-invariant representation 199
10.3.1 The electron density and charge neutrality 201
10.4 Collision integrals 203
10.4.1 Impurities 204
10.4.2 Electron–phonon collision integral 205
10.4.3 Electron–electron collision integral 207
10.5 Kinetic equations for dirty s-wave superconductors 209
10.5.1 Small gradients without magnetic impurities 210
10.5.2 Heat conduction 211
11.1 Gapless superconductors with magnetic impurities 213
11.2 Generalized TDGL equations 215
11.3 TDGL theory for d-wave superconductors 221
11.4 d.c. electric field in superconductors. Charge imbalance 226
第1页 共1页 2
010-8-8 10:41

end p.xii
Top
Privacy Policy and Legal Notice © Oxford University Press, 2003-2010. All rights reserved.
Kopnin, Nikolai, Senior Scientist, Low Temperature Laboratory, Helsinki University of
Technology, and L.D. Landau Institute for Theoretical Physics, Moscow
Theory of Nonequilibrium Superconductivity
Print ISBN 9780198507888, 2001
pp. [xii]
IV VORTEX DYNAMICS
12 Time-Dependent Ginzburg–Landau analysis 231
13 Vortex Dynamics in Dirty Superconductors 259
14 Vortex Dynamics in Clean Superconductors 271
12.1 Introduction 231
12.2 Energy balance 233
12.3 Moving vortex 234
12.4 Force balance 236
12.5 Flux flow 238
12.5.1 Single vortex: Low fields 238
12.5.2 Dense lattice: High fields 240
12.5.3 Direction of the vortex motion 242
12.6 Anisotropic superconductors 243
12.6.1 Low fields 245
12.6.2 High fields 246
12.7 Flux flow in layered superconductors 246
12.7.1 Motion of pancake vortices 247
12.7.2 Intrinsic pinning 247
12.8 Flux flow within a generalized TDGL theory 248
12.8.1 Dirty superconductors 248
12.8.2 d-wave superconductors 251
12.8.3 Discussion: Flux flow conductivity 252
12.9 Flux flow Hall effect 253
12.9.1 Modified TDGL equations 254
12.9.2 Hall effect: Low fields 255
12.9.3 High fields 256
12.9.4 Discussion: Hall effect 256
13.1 Microscopic derivation of the force on moving vortices 259
13.1.1 Variation of the thermodynamic potential 259
13.1.2 Force on vortices 260
13.2 Diffusion controlled flux flow 263
13.2.1 Discussion 269
14.1 Introduction 271
14.1.1 Boltzmann kinetic equation approach 272
14.1.2 Forces in s-wave superconductors 274
14.2 Spectral representation for the Green functions 277
14.3 Useful identities 279
14.4 Distribution function 282
14.4.1 Localized excitations 282
14.4.2 Delocalized excitations 287
14.5 Flux flow conductivity 290
14.6 Discussion 292
14.6.1 Conductivity: Low temperatures 292
第1页 共1页 2
010-8-8 10:41

end p.xiii
Top
Privacy Policy and Legal Notice © Oxford University Press, 2003-2010. All rights reserved.
Kopnin, Nikolai, Senior Scientist, Low Temperature Laboratory, Helsinki University of
Technology, and L.D. Landau Institute for Theoretical Physics, Moscow
Theory of Nonequilibrium Superconductivity
Print ISBN 9780198507888, 2001
pp. [xiii]
15 Boltzmann Kinetic Equation 303
14.6.2 Conductivity: Arbitrary temperatures 293
14.6.3 Forces 298
15.1 Canonical equations 303
15.2 Uniform order parameter 303
15.2.1 Boltzmann equation in presence of vortices 306
15.3 Quasiparticles in the vortex core 307
15.3.1 Transformation into the Boltzmann equation 307
15.4 Vortex mass 311
15.4.1 Equation of vortex dynamics 312
15.4.2 Vortex momentum 314
15.5 Vortex dynamics in d-wave superconductors 315
15.5.1 Distribution function 315
15.5.2 Conductivity 318
References 320
Index 326
第1页 共1页 2
010-8-8 10:42

Part I Green functions in the BCS theory
end p.1
end p.2
1 INTRODUCTION
Nikolai B. Kopnin
Abstract: This introductory chapter gives a brief outline of the general ideas of
the theory of superconductivity and the basic quantities that characterize the
superconducting state are introduced, such as the order parameter,
superconducting energy gap, the excitation spectrum, the coherence length, and
the magnetic field penetration length. The Ginzburg–Landau model is discussed
which provides the simplest description of stationary superconductors and allows
for the calculation of the critical magnetic fields. Its application to the vortex
state of type II superconductors is described. The upper critical magnetic field is
calculated. The microscopic Bogoliubov–de Gennes equations are introduced
together with the concept of quasiclassical approximation. The typical problems of
nonstationary theory are formulated; the simplest methods of their solution,
such as the kinetic equation approach and the time-dependent Ginzburg–Landau
model, are discussed.
Keywords: Ginzburg–Landau model, Bogoliubov–deGennes equations,
kinetic equation, energy gap, coherence length, critical field, vortex
We give a brief outline of general ideas of the theory of superconductivity
and introduce the basic quantities that characterize the superconducting
state. We discuss the Ginzburg–Landau theory which provides the simplest
description of stationary superconductors and consider its application to the
vortex state. The microscopic Bogoliubov–de Gennes theory is introduced
together with the concept of quasiclassical approximation. We also
formulate the typical problems of non-stationary theory and consider its
simplest methods such as the kinetic equation approach and the
time-dependent Ginzburg–Landau model.
1.1 Superconducting variables
The most important characteristic of a superconductor is the superconducting
order parameter
. The order parameter is proportional to the wave function of
“superconducting electrons” which form a “Bose condensate”. It is normalized in
such a way that its modulus is equal to the energy gap in the electronic spectrum
which usually appears after a transition into the superconducting state. The order
Kopnin, Nikolai, Senior Scientist, Low Temperature Laboratory, Helsinki University of
Technology, and L.D. Landau Institute for Theoretical Physics, Moscow
Theory of Nonequilibrium Superconductivity
Print ISBN 9780198507888, 2001
pp. [1]-[5]
PRINTED FROM OXFORD SCHOLARSHIP ONLINE (www.oxfordscholarship.com)
© Copyright Oxford University Press, 2003-2010. All Rights Reserved
PRINTED FROM OXFORD SCHOLARSHIP ONLINE (www.oxfordscholarship.com)
© Copyright Oxford University Press, 2003-2010. All Rights Reserved
Oxford Scholarship Online: Theory of Nonequilibrium Supe... http://www.oxfordscholarship.com/oso/private/content/phy...
第1页 共4页 2010-8-8 10:44
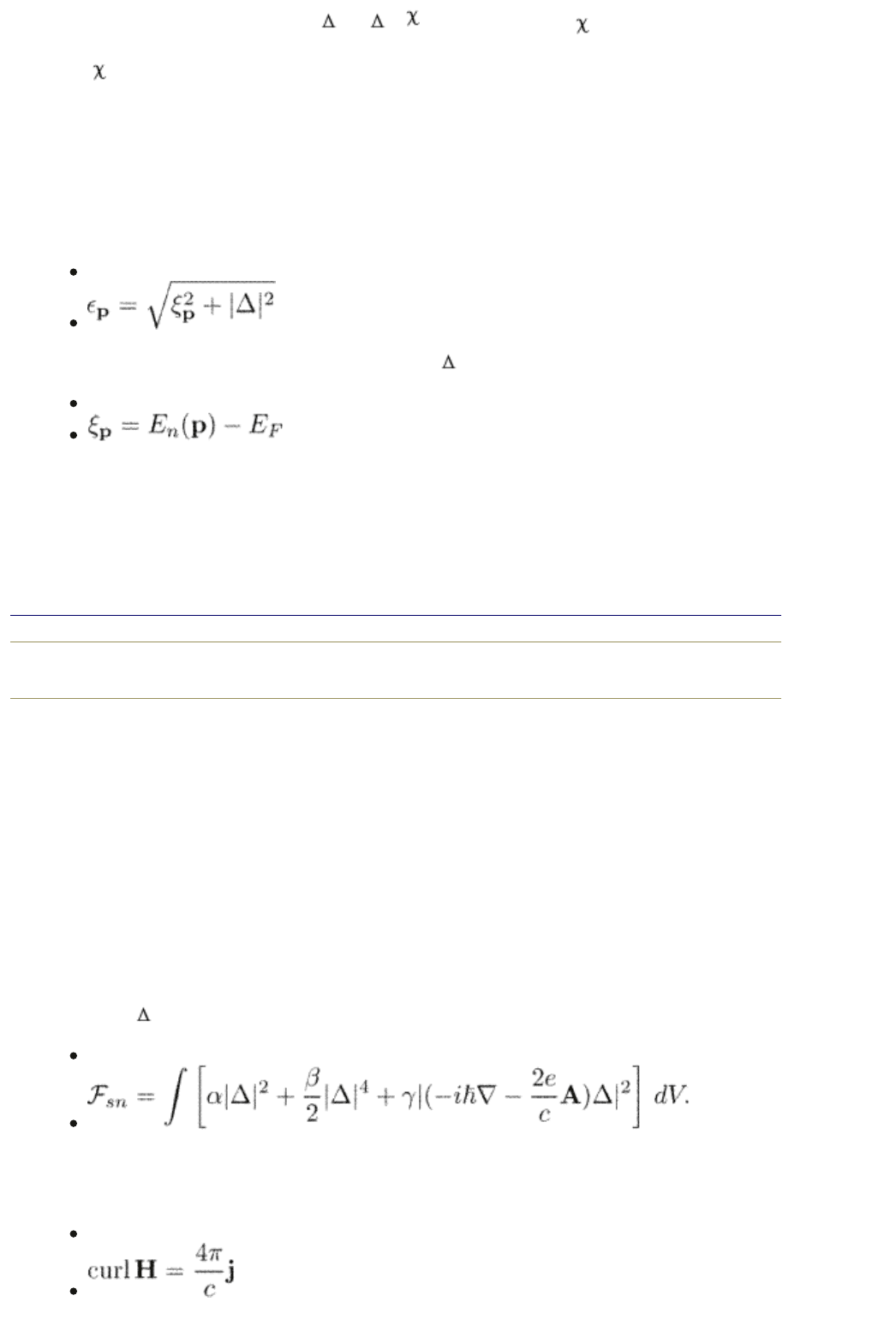
parameter is a complex function = | |e
i
where the phase is the same for
all condensate particles if there is no current. In presence of a supercurrent, the
phase
acquires a spatial dependence slowly varying from one point in the
superconductor to another. The existence of a coherent phase of the wave
function for all superconducting particles is the very essence of
superconductivity.
Another important characteristic is the energy spectrum of single-particle
excitations in a superconducting system. For a homogeneous clean
superconductor in absence of currents and magnetic field the energy spectrum
has a gap
(1.1)
such that all excitations have energies above | |. In eqn (1.1),
(1.2)
while E
n
(p) is the electronic spectrum in the normal state, and, E
F
is the Fermi
energy. The energy is counted from E
F
because, as in any Fermi liquid, relevant
excitations are concentrated near the Fermi level. The normal spectrum E
n
(p) of
the metal can have a complicated dependence on the momentum p reflecting the
band structure of the metal. In many cases, if we are not interested in the
end p.3
particular band-structure effects, it is sufficient to consider a simple parabolic
spectrum E
n
= p
2
/2m.
The order parameter determines both thermodynamic and transport properties of
a superconductor. To learn more of the order parameter as well as of other
important superconducting quantities we start with the Ginzburg–Landau theory
for a time-independent superconducting state.
1.1.1 Ginzburg–Landau theory
The Ginzburg–Landau (Ginzburg and Landau 1950) theory is a generalization of
the Landau theory of second-order phase transitions (Landau and Lifshitz 1959
b). Consider the free energy of a superconductor. Assume that we can expand it
in terms of
and its gradients:
(1.3)
The free energy expression is supplemented with the Maxwell equation for the
magnetic field
(1.4)
PRINTED FROM OXFORD SCHOLARSHIP ONLINE (www.oxfordscholarship.com)
© Copyright Oxford University Press, 2003-2010. All Rights Reserved
Oxford Scholarship Online: Theory of Nonequilibrium Supe... http://www.oxfordscholarship.com/oso/private/content/phy...
第2页 共4页 2010-8-8 10:44
