Kopnin N.B. Theory of Nonequilibrium Superconductivity

Подождите немного. Документ загружается.

where

(1.5)

The average of H gives the magnetic induction B.

The gradient term in eqn (1.3) is the momentum operator in presence of the

magnetic field

(1.6)

It refers to a Cooper pair having the charge 2e (e is the electronic charge).

Equation (1.6) implies a gauge invariance: the free energy of the system and the

magnetic field do not change if one makes a simultaneous transformation

(1.7)

where f(r) is an arbitrary function of coordinates.

At the transition temperature T = T

, the coefficient changes its sign and

becomes negative for T < T

, while and remain constant. Microscopic theory

(Gor’kov 1959 a, 1959 c) gives

(1.8)

where (0) is the density of states at the Fermi level, and (3) 1.202. We use

the units with the Boltzmann constant k

= 1. We will derive eqn (1.8) later in

Section 6.1.2. Equation (1.8) demonstrates that the free energy expansion in

end p.4

eqn (1.3) goes in powers of /T

. which suggests that should be much smaller

than T

The coefficient

depends on purity of the sample. The purity is characterized by

the parameter

, where is the electronic mean free time due to the

scattering by impurities. Superconductors are called clean when this parameter is

large, and they are dirty in the opposite case. We discuss the microscopic

justification of the Ginzburg–Landau theory later. Here we just write down the

expression for

in two limiting cases. For dirty superconductors, it is

(1.9)

where D is the diffusion coefficient. In the clean case

(1.10)

PRINTED FROM OXFORD SCHOLARSHIP ONLINE (www.oxfordscholarship.com)

Oxford Scholarship Online: Theory of Nonequilibrium Supe... http://www.oxfordscholarship.com/oso/private/content/phy...

第3页共4页 2010-8-8 10:44

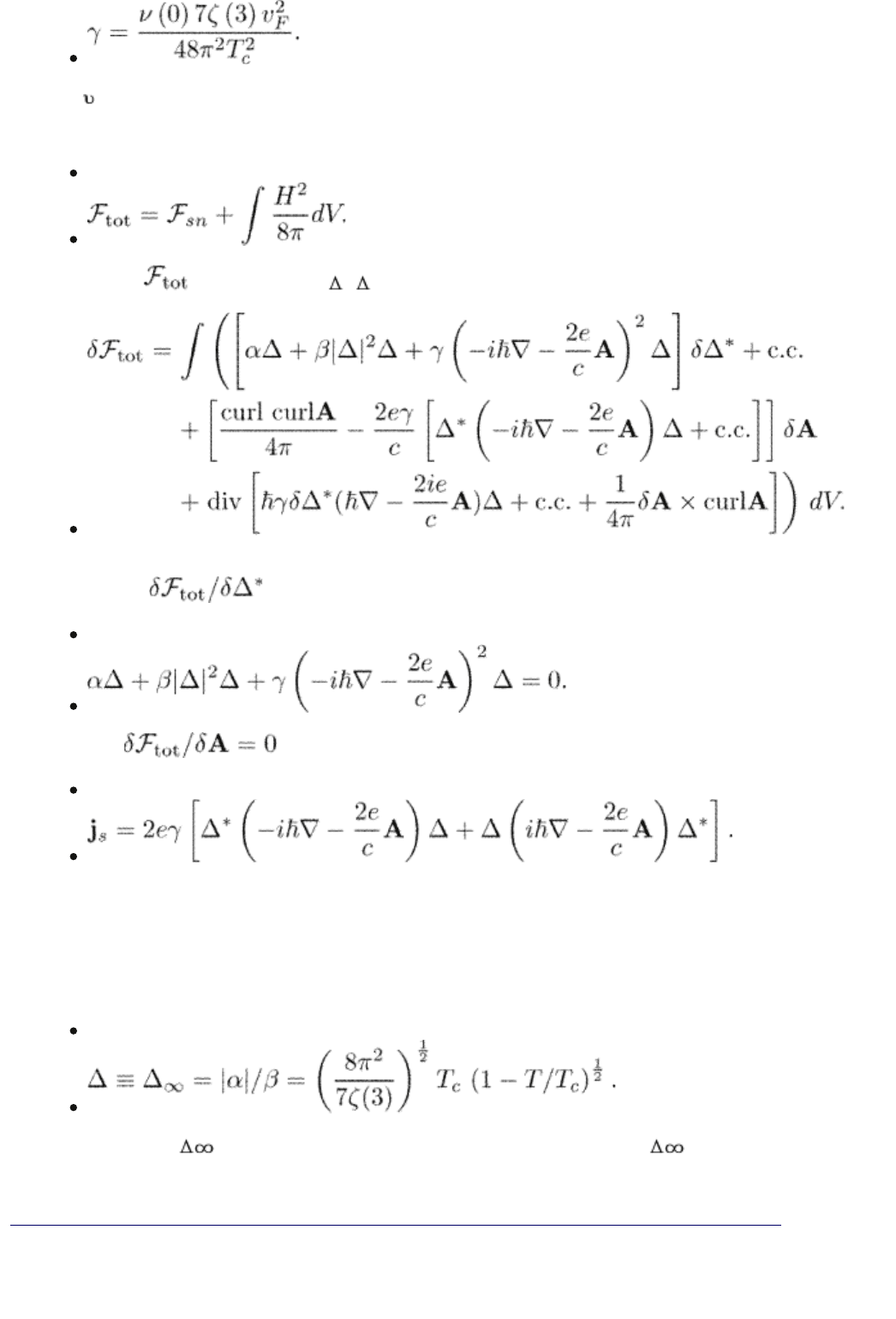
Here

is the velocity of electrons at the Fermi surface.

The total free energy is

(1.11)

Variation of with respect to , * and A gives

The requirement of extremum of the free energy leads to the GL equations.

Vanishing of

results in

(1.12)

Condition gives the expression for suprecurrent

(1.13)

1.1.1.1 Discussion of the GL equations

Consider first the equation (1.12) for the order parameter. In a homogeneous

case without a current and a magnetic field the order parameter is

(1.14)

We denote it by for the reason which will be clear later. The ratio /T

should be small. This implies that the GL theory works for temperatures close

end p.5

Top

Oxford Scholarship Online: Theory of Nonequilibrium Supe... http://www.oxfordscholarship.com/oso/private/content/phy...

第4页共4页 2010-8-8 10:44

to T

, i.e., when 1 T/T

1. Simultaneously we see that ~ T

when

temperatures are well below T

Equation (1.12) defines the length

(1.15)

which is a characteristic scale of variations of the order parameter. It is called the

coherence length. The notation

for the coherence length is to be distinguished

from

that denotes the energy variable in eqn (1.2). We keep both these

notations because they are commonly accepted in the literature.

In the clean case

we have from eqn (1.10)

(1.16)

where

(1.17)

is the “zero-temperature” coherence length. In the dirty case eqn (1.9) gives

(1.18)

where is the electron mean free path. The impurity parameter can be

expressed through the ratio of

and l:

(1.19)

so that a dirty limit corresponds to l

while a clean limit is for l

Consider now expression (1.13) for the current. Using the definition of the

momentum operator eqn (1.6) we introduce the superconducting velocity

(1.20)

for a Cooper pair with the mass 2m. We use here the bare mass m of an electron.

However, the bare electronic mass is not a good quantity for a metal where the

normal-state electronic spectrum may have a complicated form. In this case also

the “superconducting velocity” is not a real velocity of Cooper pairs. Bearing this

in mind we write the supercurrent as

(1.21)

Kopnin, Nikolai, Senior Scientist, Low Temperature Laboratory, Helsinki University of

Technology, and L.D. Landau Institute for Theoretical Physics, Moscow

Theory of Nonequilibrium Superconductivity

Print ISBN 9780198507888, 2001

pp. [6]-[10]

Oxford Scholarship Online: Theory of Nonequilibrium Supe... http://www.oxfordscholarship.com/oso/private/content/phy...

第1页共6页 2010-8-8 10:46

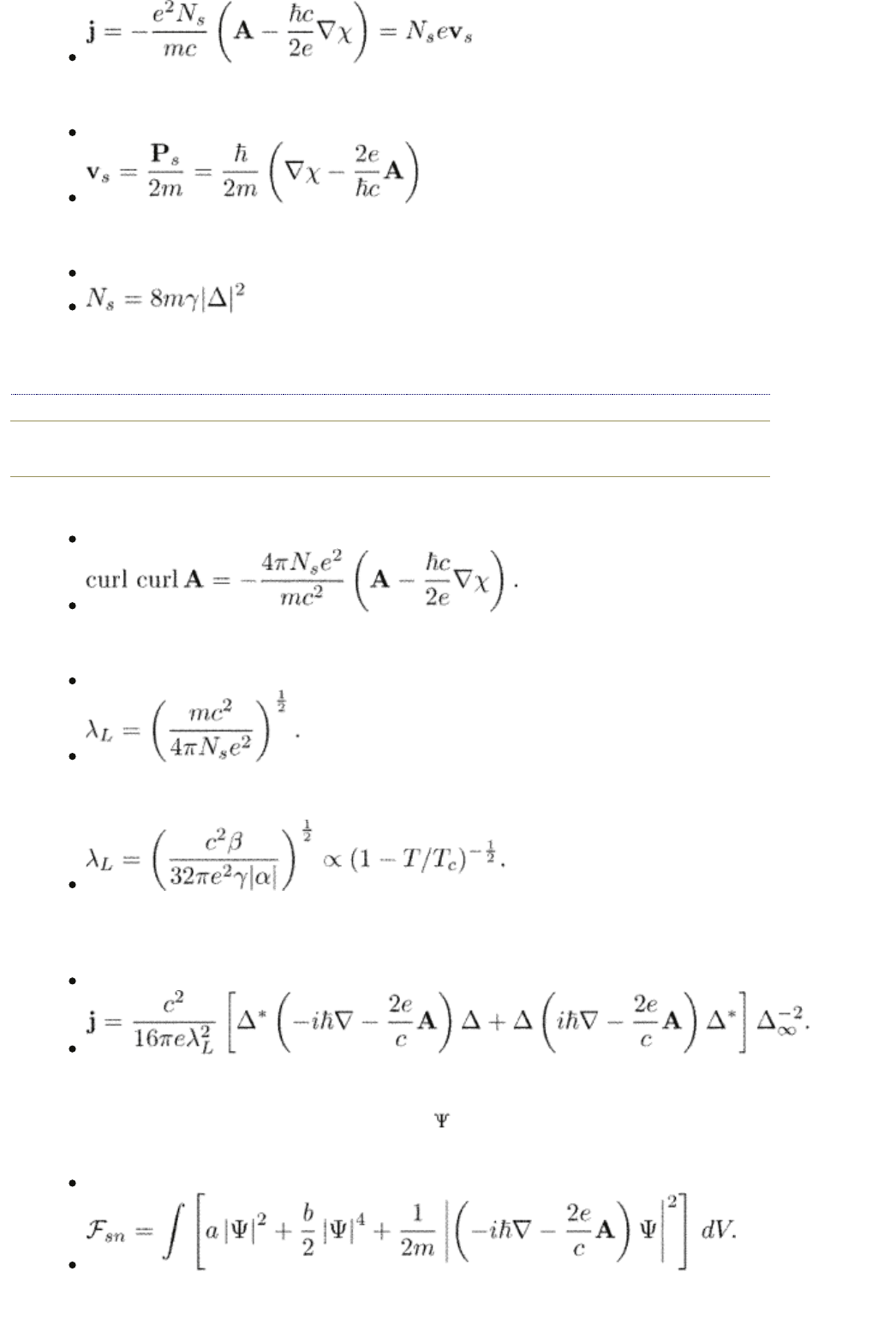
where

(1.22)

and

(1.23)

is the density of “superconducting electrons”.

end p.6

The Maxwell equation (1.4) combined with eqn (1.21) gives

(1.24)

Equation (1.24) defines the characteristic length

(1.25)

In a homogeneous case

This is called the London penetration length. If determines the characteristic

scale of variations of the magnetic field. The supercurrent can be written as

(1.26)

Sometimes, it can be convenient to use the normalization of the order parameter

such that it has the form of the wave function

of superconducting electrons.

The free energy becomes

(1.27)

PRINTED FROM OXFORD SCHOLARSHIP ONLINE (www.oxfordscholarship.com)

Oxford Scholarship Online: Theory of Nonequilibrium Supe... http://www.oxfordscholarship.com/oso/private/content/phy...

第2页共6页 2010-8-8 10:46

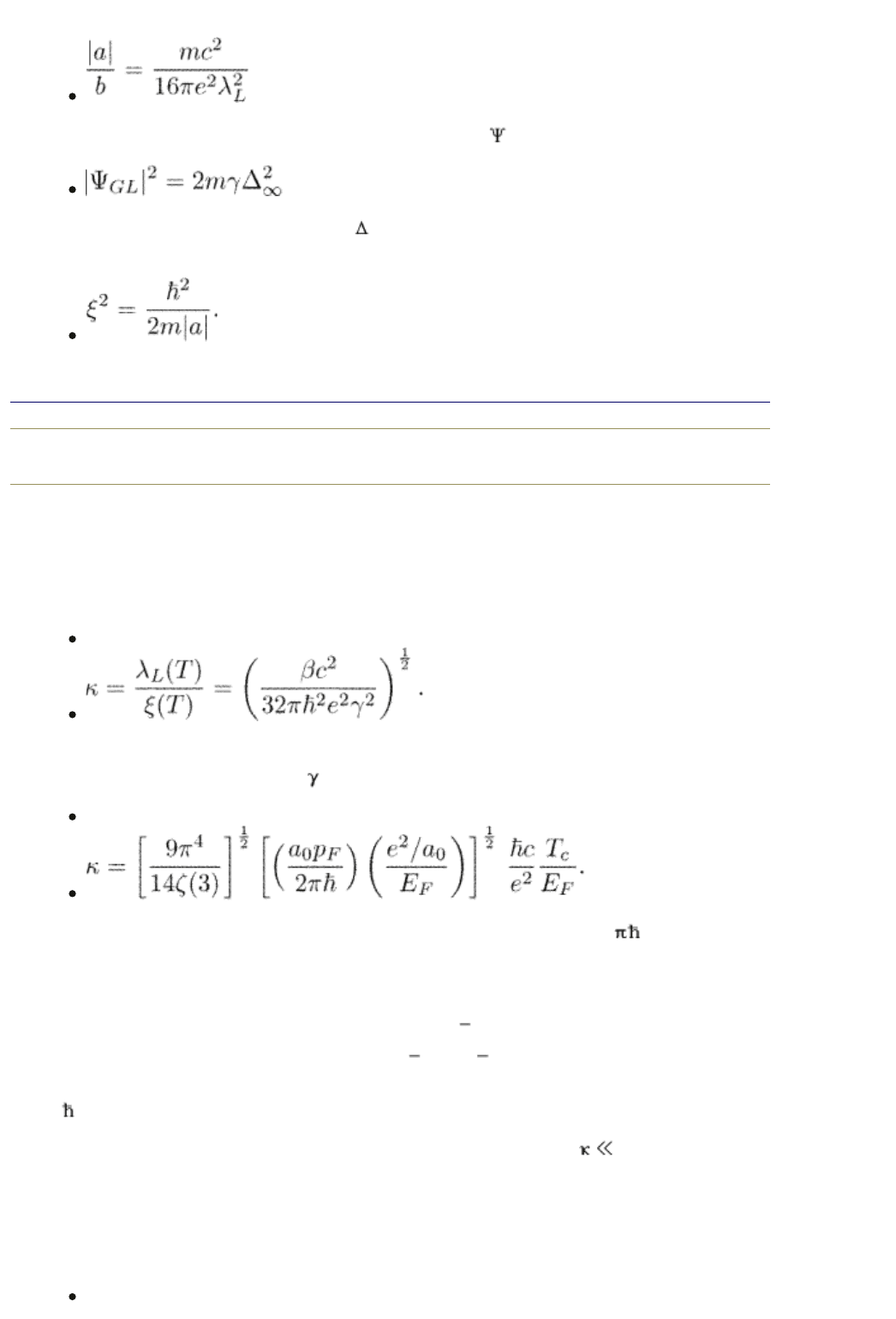
The constants a and b satisfy

and determine the new order parameter magnitude |

= |a| /b which is

in terms of the previous definition of . The coherence length is now expressed

through the electronic mass

end p.7

1.1.1.2 The Ginzburg–Landau parameter. Type I and type II

superconductors

The ratio of the two characteristic lengths is called the Ginzburg–Laacfati

parameter

(1.28)

It is independent of T near T

and is determined by the material characteristics.

For clean superconductors with

from eqn (1.10) it is

(1.29)

Here a

is the interatomic distance. Usually, it is of the order of 1/2 p

. The

ratio of the Coulomb interaction energy of conducting electrons e

to the

Fermi energy is of the order of unity for good metals, but it may become larger

for systems with strong correlations between electrons. The last factor in eqn

(1.29) is usually small: T

is of the order of 10

for conventional

superconductors, but it is of the order of 10

÷ 10

for high temperature

superconductors with T

~ 100K and E

~ 1000K. The fine structure constant,

/ c = 1/137.

We see that the Ginzburg–Landau parameter is normally small

1 for

conventional clean superconductors, though, in some cases it may be of the order

of 1. On the contrary, for high temperature superconductors, which have a

tendency to be strongly correlated systems with a not very low ratio of T

, the

parameter k is usually very large. The Ginzburg–Landau parameter increases for

dirty superconductors. According to eqn (1.9) it becomes

(1.30)

PRINTED FROM OXFORD SCHOLARSHIP ONLINE (www.oxfordscholarship.com)

Oxford Scholarship Online: Theory of Nonequilibrium Supe... http://www.oxfordscholarship.com/oso/private/content/phy...

第3页共6页 2010-8-8 10:46

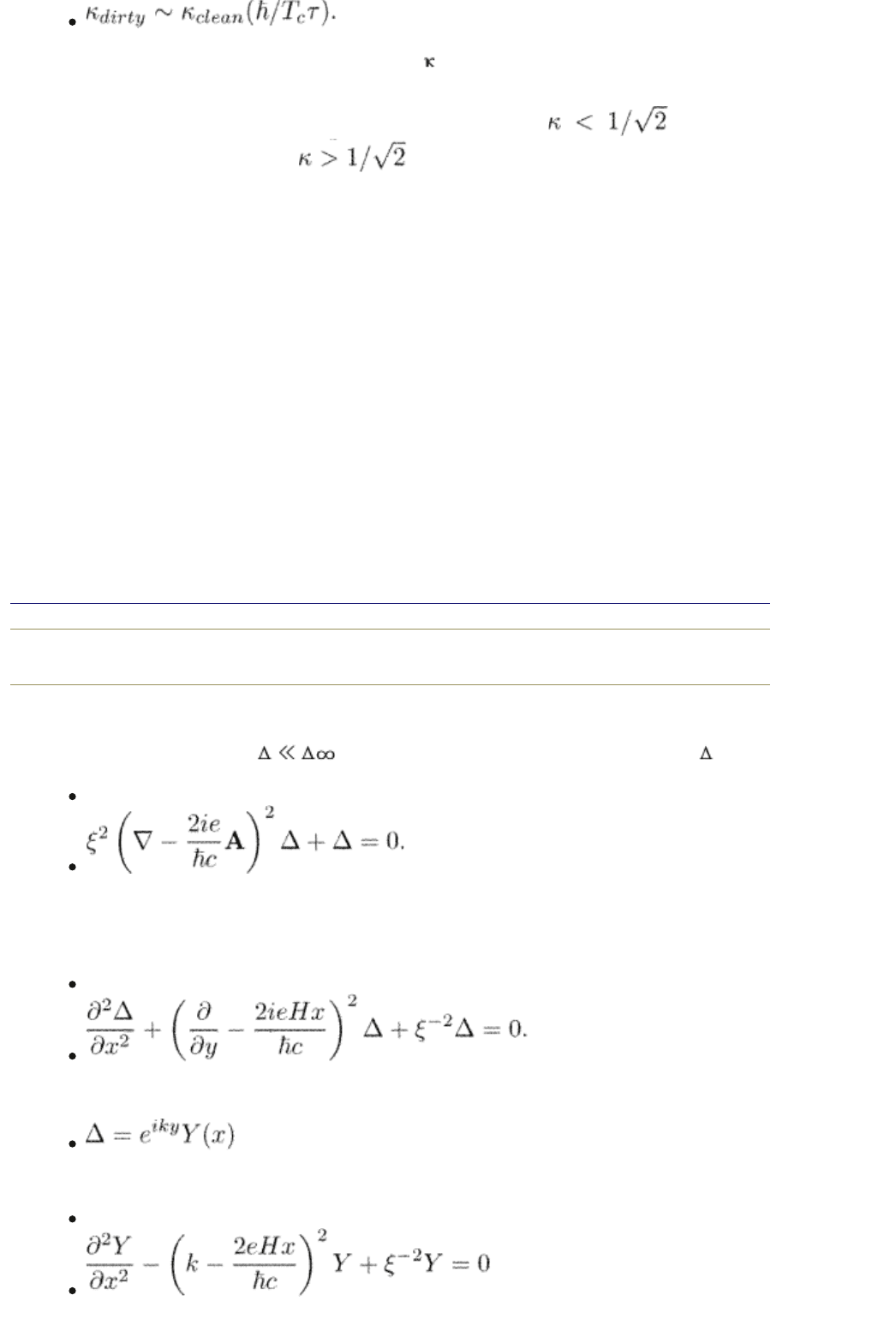
Therefore, dirty alloys normally have a large .

The value of the Ginzburg–Landau parameter divides all superconductors into

two classes: type I and type II superconductors. Those with

belong

to the type I, while those with

are the type II superconductors.

1.1.2 Example: Vortices in type II superconductors

Vortices are very important topological objects in superconductors and

superfluids. As already mentioned in the Preface, vortices determine the most

fundamental properties of superconductors and their responses to external d.c.

and a.c. electromagnetic fields. They play a crucial role also in hydrodynamics of

superfluids. We will study the dynamics of vortices in detail later in this book.

Here we summarize the main features of vortices which can be deduced from the

Ginzburg–Landau model. A more detailed Ginzburg–Landau theory of the vortex

state in superconductors can be found, for example, in the book by Saint-James

et al. (1989).

1.1.2.1 Transition from normal into the superconducting state

The transition from normal into the superconducting state in a magnetic field is

of the second

end p.8

order in type II superconductors. Let us find the magnetic field when a nonzero

order parameter first appears (Abrikosov 1957). Close to the transition point the

order parameter is small,

. We linearize the GL equations in a small :

(1.31)

Let the magnetic field be along the z-axis. The vector potential can be taken in

the Landau gauge A = (0, H x, 0). The order parameter depends on x and y. Now

we have

(1.32)

This is the Schrödinger equation for a charge in a magnetic field. We put

and obtain the oscillator equation

(1.33)

PRINTED FROM OXFORD SCHOLARSHIP ONLINE (www.oxfordscholarship.com)

Oxford Scholarship Online: Theory of Nonequilibrium Supe... http://www.oxfordscholarship.com/oso/private/content/phy...

第4页共6页 2010-8-8 10:46

where the oscillator frequency

and energy E are

respectively. The energy spectrum E =

(n + 1/2) gives

The highest magnetic field H = H

is for n = 0:

(1.34)

where

is the magnetic flux quantum (see below). H

is the upper critical magnetic field

below which the transition into superconducting state occurs. It is proportional to

T/T

near the critical temperature. Comparing it with the thermodynamic

critical field (de Gennes 1966) H

we observe that . For type

II superconductors with

, the upper critical field H

> H

end p.9

The solution for the lowest energy level is a Gaussian function

(1.35)

The function, in eqn (1.35) is centered at x = ck/2eH. Actually, the full solution

is a linear combination of these functions with different k. One can construct a

periodic solution in the form

(1.36)

This is periodic in y with the period y

= 2 /q. It will be periodic in x, as well, if

the coefficients C

satisfy periodicity condition C

n+p

= C

. Then,

The simplest case is realized when all the coefficients C

are equal. The period in

PRINTED FROM OXFORD SCHOLARSHIP ONLINE (www.oxfordscholarship.com)

Oxford Scholarship Online: Theory of Nonequilibrium Supe... http://www.oxfordscholarship.com/oso/private/content/phy...

第5页共6页 2010-8-8 10:46

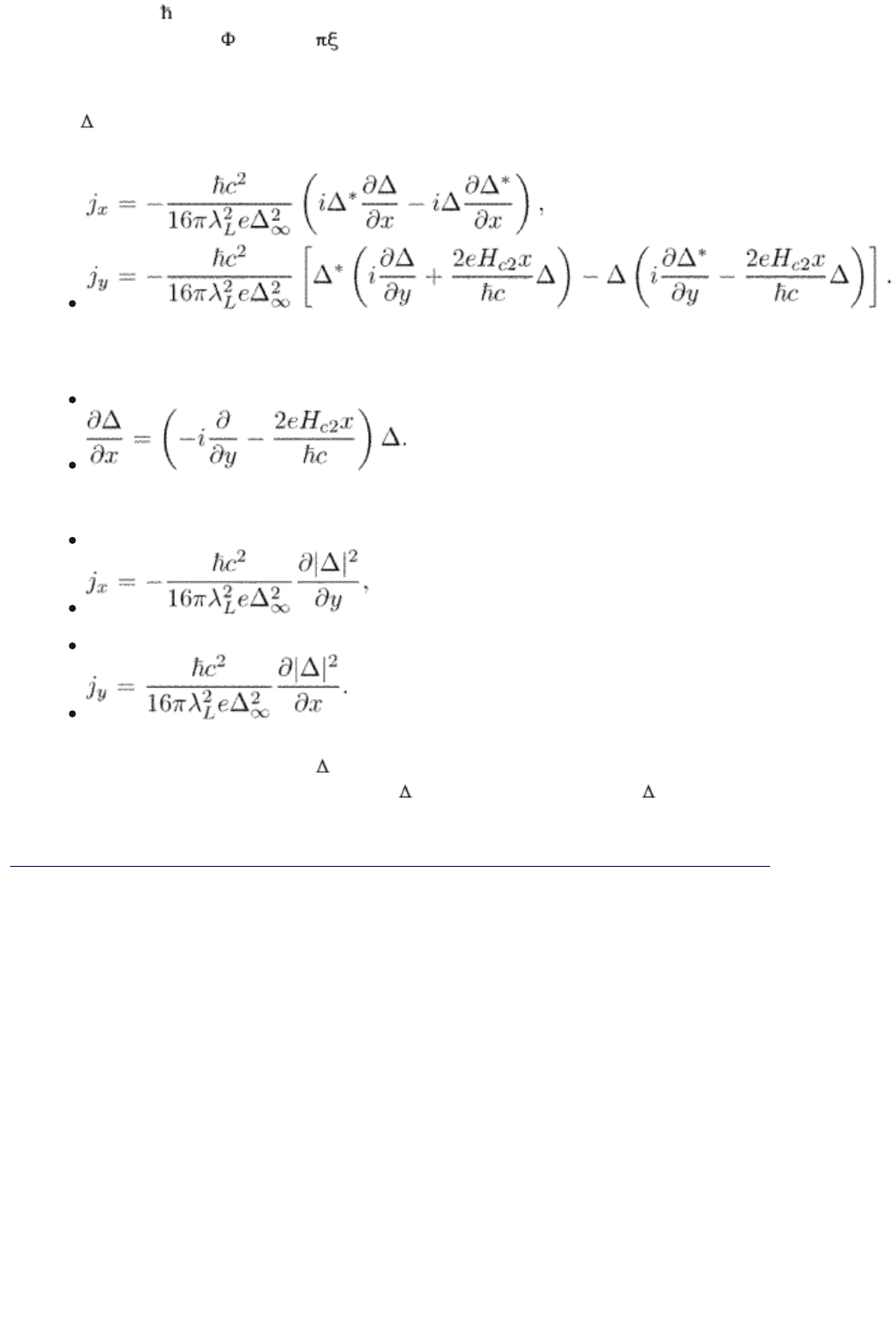
x is then x

= cq/2eH

. The solution eqn (1.36) forms a rectangular lattice with

the area S

= x

= 2

which corresponds to exactly one flux

quantum per unit cell. If q is chosen such that x

= y

, we obtain a square

lattice.

The |

|–pattern has zeroes at the points x = x

/2 + x

n, y = y

/2 + y

surrounded by supercurrent flow lines. Indeed, the supercurrent is

To transform this further we use the identity which holds for the function of the

type of eqn (1.36):

(1.37)

With help of eqn (1.37) we get

(1.38)

(1.39)

These expressions suggest that | (x, y)|

is a stream function, i.e., that the

current, flows along the lines of constant | |. If we place the node of | | in the

center of the Bravais unit cell, the current along the boundary of a unit cell

end p.10

Top

Oxford Scholarship Online: Theory of Nonequilibrium Supe... http://www.oxfordscholarship.com/oso/private/content/phy...

第6页共6页 2010-8-8 10:46

is zero: due to periodicity, the lines of constant | | are perpendicular to the

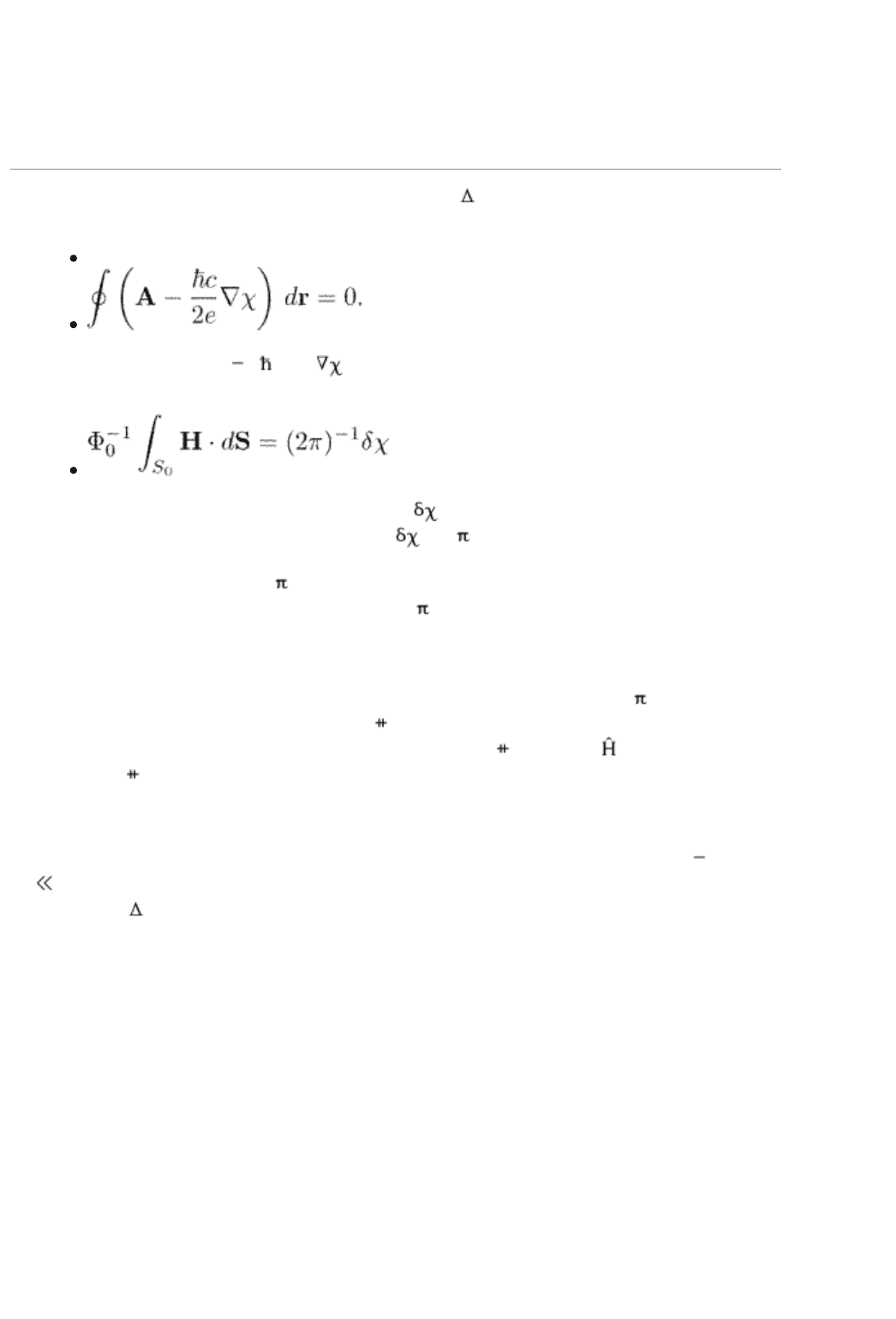
boundary. We now calculate the contour integral along the unit cell boundary

(1.40)

It vanishes because A ( c/2e) = 0 at the boundary, as we have just proven.

We obtain

where the integral is over the unit cell and is the variation of the order

parameter phase along this contour. It is

= 2 since the flux through the unit

cell is equal to one flux quantum. Therefore, the phase of the order parameter

acquires the increment of 2

after encircling the point where the order

parameter is zero. The phase variation by 2

is also necessary for single-

valuedness of the order parameter. Here we come to a vortex: A quantized

vortex is a linear (in three dimensions) topologieal object which is characterized

by a quantized circulation of the order parameter phase around this line. In

principle, vortices with a phase circulation of an integer multiple of 2

are also

possible. The axis of vortex circulation

is parallel to H if the charge of carriers

is positive and antiparallel to it in the opposite case:

= sign (e) . For

electrons,

is anitiparallel to H. We see that transition into a superconducting

state in a magnetic field below H

gives rise to formation of vortices. Vortices in

superconductors were theoretically predicted by Abrikosov (1957).

Let us consider an applied magnetic field H slightly below H

such that H

. The solution of the GL equation is the function eqn (1.36) plus a small

correction

. This correction is caused by (i) nonlinear term in the GL equation,

(ii) local variations in A due to the supercurrent eqns (1.38), (1.39), and (iii)

deviation of H from H

Kopnin, Nikolai, Senior Scientist, Low Temperature Laboratory, Helsinki University of

Technology, and L.D. Landau Institute for Theoretical Physics, Moscow

Theory of Nonequilibrium Superconductivity

Print ISBN 9780198507888, 2001

pp. [11]-[15]

Oxford Scholarship Online: Theory of Nonequilibrium Supe... http://www.oxfordscholarship.com/oso/private/content/phy...

第1页共7页 2010-8-8 10:48

Using the Maxwell equation

we obtain variations in the local field

(1.41)

Therefore, the vector potential becomes A = A

+ A

where

where A is due to the magnetic field H

Since the non disturbed function of eqn (1.36) satisfies the linearized GL

equation with A = A

, we obtain for the correction

end p.11

Now we apply the orthogonality condition: we multiply this equation by from

eqn (1.36) and integrate it over dx dy. After integration by parts using eqn

(1.37), we obtain

We introduce the average over the area occupied by the vortex array

and obtain using eqa (1.41)

It is convenient to introduce the ratio

(1.42)

PRINTED FROM OXFORD SCHOLARSHIP ONLINE (www.oxfordscholarship.com)

Oxford Scholarship Online: Theory of Nonequilibrium Supe... http://www.oxfordscholarship.com/oso/private/content/phy...

第2页共7页 2010-8-8 10:48