Jean-Laurent Mallet. Geomodeling
Подождите немного. Документ загружается.

398
CHAPTER
8.
ELEMENTS
OF
STRUCTURAL
GEOLOGY
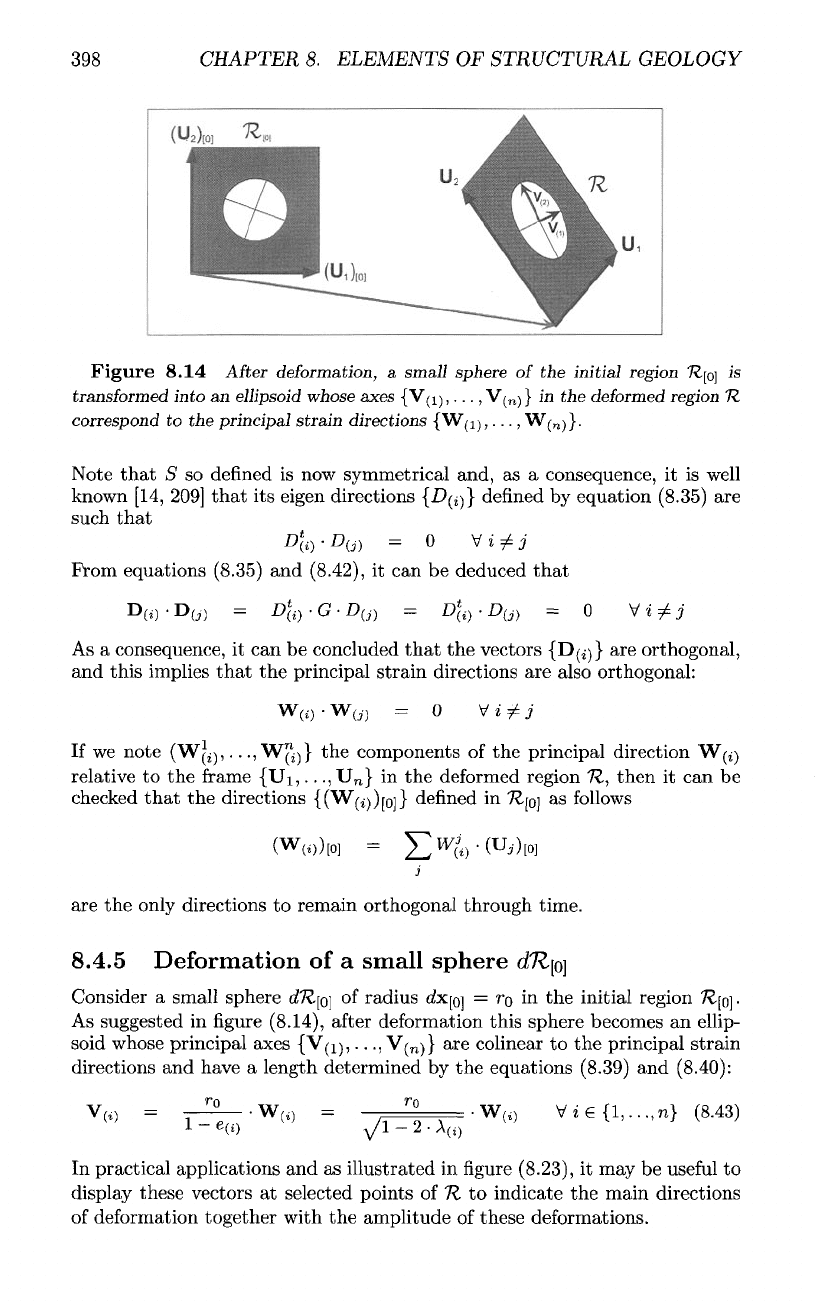
Figure
8.14 After deformation,
a
small sphere
of the
initial region
72.
[o]
is
transformed
into
an
ellipsoid
whose axes
{V(i),...,
V(
n
)}
in the
deformed
region
72,
correspond
to the
principal strain directions
(W^),...,
W(
n
)}.
Note
that
S so
defined
is now
symmetrical and,
as a
consequence,
it is
well
known
[14, 209]
that
its
eigen directions
(-D(i)}
defined
by
equation (8.35)
are
such
that
Prom
equations
(8.35)
and
(8.42),
it can be
deduced
that
As
a
consequence,
it can be
concluded
that
the
vectors
|D(j)}
are
orthogonal,
and
this implies
that
the
principal strain directions
are
also orthogonal:
If
we
note
(W^,...,
W"^}
the
components
of the
principal direction
W(^)
relative
to the
frame
{Ui,...,
U
n
}
in the
deformed region
72.,
then
it can be
checked
that
the
directions
{(W(j))[
0
]}
defined
in
72.[o]
as
follows
are
the
only directions
to
remain orthogonal through time.
8.4.5
Deformation
of a
small
sphere
d7l[^
Consider a small sphere d72.[o] of radius dxjo] = TO m the initial region 72.[o] •
As
suggested
in figure
(8.14),
after
deformation this sphere becomes
an
ellip-
soid
whose principal axes
{V(i),...,
V(
n
)}
are
colinear
to the
principal strain
directions
and
have
a
length determined
by the
equations (8.39)
and
(8.40):
In
practical applications
and as
illustrated
in figure
(8.23),
it may be
useful
to
display
these vectors
at
selected points
of
72.
to
indicate
the
main directions
of
deformation together with
the
amplitude
of
these deformations.
8.4.
DEFORMATION ANALYSIS
Moreover,
from
equation
(8.32),
it can be
noted
that
Taking into account
the
fact
that
{(1
—
2A(^)
: i =
1,..
.,n}
are the
eigen
values
of (/
—
25'),
the
above equation
can be
rewritten
as
follows:
Prom
equations
(8.24)
and
(8.39),
it can be
deduce
that
Comparing
the
above equation with equation (8.43) allows
us to
conclude
that
8.4.6
Practical
remarks
In
the
general case,
S as
defined
by
equation (8.32)
is a
non-symmetrical
matrix. Prom
a
numerical analysis point
of
view,
it is
well
known
that
it
is
easier
to
compute eigen values
and
eigen vectors
for a
symmetrical matrix
than
for a
non-symmetrical matrix. Taking into account
the
fact
that
principal
strain directions
and
elongations
are
intrinsic properties independent
of the
parameterization
of
7£
or
7£[o],
from
a
practical point
of
view
and
without loss
of
generality,
we can
always proceed
as
follows:
• As
explained
in
section (8.5.1),
in the
case where
n = 2 it is
convenient
to
choose
a
parameterization
of
7£[o]
such
that
G[o]
=
/•
In
such
a
case,
the
matrix
S
becomes symmetrical:
• As
explained
in
section
(8.6.7),
in the
case where
n = 3 it is
convenient
to
choose
a
parameterization
of
"R,
such
that
G = I. In
such
a
case,
the
matrix
S
also
becomes symmetrical:
It
should
also
be
noted
that,
in
practical
applications,
the
elongations
{&(i)}
are
generally
all
close
to
zero. This implies
that
the
vectors
(V^)}
defined
by
equation (8.43)
and
characterizing
the
deformation
of a
small sphere
are
all
approximately
the
same length.
As a
consequence,
the
image
of a
small
sphere
of
radius
TO
is
close
to a
sphere with
the
same radius
so
that,
from
a
graphical point
of
view,
the
vectors
{V^}
may be
inadequate
for
visualizing
the
deformations.
For
this reason,
for
graphical purposes,
as
shown
in figure
399
400
CHAPTER
8.
ELEMENTS
OF
STRUCTURAL
GEOLOGY
(8.23),
we
suggest replacing
the
vectors
{V(j)}
by
vectors
{V^\}
defined
as
follows:
In
this
definition
of
V*^,
the
exponent
m
is a
given "magnifying factor"
that
must
be
chosen greater than
1 to
increase
the
contrasts between
the
deformations
corresponding
to the
principal strain directions.
8.4.7 Eulerian
and
Lagrangean strain
tensors
The
components
of S can be
viewed
as the
components
15
of the
tensor
£
relative
to the
local
frame
{Ui,...,U
n
}
attached
to the
current deformed
region
7£.
Therefore, this tensor
is
called
the
"Eulerian Strain
Tensor"
and
corresponds
to a
measure
of the
deformations
from
the
point
of
view
of the
deformed
region
71.
It
should
be
noted
that
it is
also possible
to
adopt
the
point
of
view
of the
undeformed
region
7£[o],
which leads
to the
following
definitions
of
A(W[
0
])
and
5[oj:
The
components
of
S[
0
]
so
defined
can be
viewed
as the
components
of the
strain tensor relative
to the
frame
{(Ui)[
0
],...,
(U
n
)[
0
])}
attached
to
7£[
0
].
This tensor
is
called
the
"Lagrangean
Strain
Tensor"
and
corresponds
to a
measure
of the
deformations
from
the
point
of
view
of the
undeformed region
%)]•
8.4.8
Invariants
of the
strain
tensor
According
to the
definition
(8.19),
at any
point
of the
studied domain
71,
the
strain tensor
is
fully
defined
by a set of
(n
•
(n+1)/2
independent components
f
• •
(^
U
1
U
3
.
The
components
{S
u
i
u
j}
of the
strain tensor
so
defined
depend strongly
on the
coordinate system
(w
1
,...,
u
n
}
and
thus cannot
be
used directly
to
characterize
the
deformations
of
the
studied region
7£.
For
example,
let us
consider
a
linear
15
Note
that
these components
are
once covariant
and
once contravariant.

8.4.
DEFORMATION ANALYSIS
transformation
of
these coordinates into
a new
system
(w'
1
,...,
u'
n
)
such
that
where
A is an
invertible
n x n
square matrix. According
to the
rules
of
transformation
of
(covariant) tensors [190, 205],
the
components
of the
strain
tensor
are
transformed into
new
components such
that
Now
consider
the
polynomials
P(x)
and
P'(x)
defined
as
follows:
Prom
equations
(8.47)
and
(8.48),
we can
write
In
other words,
P(x)
is a
characteristic
of the
strain tensor independent
of
any
linear transformation
of the
coordinate system
(w
1
,...,
u
n
}.
Note
that
P(x]
can
always
be
written
as
follows:
It can be
shown that, whatever
the
value
of n:
The
invariance
of
P(x)
implies
that
the
coefficients
(Vi,...,
V
n
}
are
indepen-
dent
of the
coordinate system:
for
this reason,
these
coefficients
are
called
the
"invariants"
of the
strain tensor
S.
Note
that,
in
practical applications,
the
studied region
71
is
either
a
surface
(n
= 2) or a
volume
(n = 3). In
these particular cases,
• if n = 2,
then
the
invariants
Vi
and
V-2
are
fully
determined
by
equations
(8.51);
• if n = 3,
then
the
invariants
Vi
and
Vs
are
fully
determined
by
equations
(8.51)
while
the
invariant
V^
is
such
that
401
402
CHAPTER
8.
ELEMENTS
OF
STRUCTURAL GEOLOGY
The
rest
of
this
chapter shows
how to
approximate
the field of
strain ten-
sors everywhere
in the
region
7i.
In a
certain sense,
the
invariants
of
these
strain tensors
can be
considered
as
"structural
attributes"
characterizing
the
deformation
of the
region
71.
From
a
practical
point
of
view,
it may be
interesting
to
correlate these structural attributes statistically
to
geological
properties such
as, for
example, permeability.
8.4.9
Displacements
in
continuous
media
As
suggested
in figure
(8.13),
let v be the
"displacement vector"
of
X[Q]
defined
as
follows:
Deriving
this vector with respect
to
u
l
allows
us to
write
the
following
impli-
cations:
Consequently, according
to
equation
(8.22),
the
components
of the
strain ten-
sor
£ and the
derivatives
of the
vector
v are
linked
by the
following
differential
equation:
8.4.10
An
important
case
Let
us now
consider
the
particular case where
the
topological dimension
n of
the
region
71
is
equal
to the
dimension
of the
embedding space.
In
this case,
for
simplicity's sake
and
without loss
of
generality,
it is
always possible
to
choose
the
parameterization u(x) identical
to the
vector
x
itself:
This implies
that
where
the
vectors
b^
are
defined
as follows:
8.4.
DEFORMATION ANALYSIS
Let
{v
k
}
be the
(contravariant) components
of v in the
frame
{bj}:
On
differentiating
with respect
to
u
l
=
x
l
,
we
obtain
This
implies
that
equation (8.54) takes
the
following
form:
In
view
of the
fact
that
the
vectors
{bj}
constitute
an
orthonormal
frame,
it
can be
concluded that,
in
this
frame,
the
components
of the
strain tensor
can
be
determined thanks
to the
following
fundamental equation:
8.4.11 Minimum-Deformation principle
Prom
a
historical point
of
view,
the law of
"least action"
is
certainly
the
first
general
principle
that
has
been proposed
to
describe
the
behavior
of
our
physical world.
In
1744,
the
French scientist Pierre-Louis Moreau
de
Maupertuis
proposed this principle
for the
very
first
time
and
published
it in
1746
in a
famous
article entitled, "The laws
of
motion
and of
rest deduced
from
a
metaphysical principle"
[38].
This "metaphysical principle" relies
on
the
assumption
that
nature always operates with greatest economy:
If
some changes occurs
in
nature, then
the
amount
of
action nec-
essary
to
perform
this change
is
always
as
small
as
possible.
Several
examples
follows:
• In a
homogeneous medium, light always travels according
the
shortest
path.
•
Soap
films
always have minimum area.
• The
geometry
of the DNA
molecule, which
corresponds
to a
double
helix,
is
an
example
of a
surface with minimal
area.
•
Small crystals grow
to
minimize their
free
surface energy.
•
Mechanical systems always
tend
to
minimize their potential energy.
Dozen
of
other examples
can be
observed,
and
interested readers
are
referred
to a
fascinating book entitled "The Parsimonious Universe"
and
published
in
1996
by S.
Hildebrandt
and A.
Tromba
[106].
403

404
CHAPTER
8.
ELEMENTS
OF
STRUCTURAL GEOLOGY
Figure
8.15
Vertical
cross
section
showing
the
evolution
of
an
overturned
fold
throughout
geological
time
t.
Note
that
the
region
A has no
deformation
between
the
initial
state
(t = 0) and the
current
state
(t = 1) in
spite
of the
fact
that
its
intermediate
state
(t =
0.5)
is
clearly
severely
deformed.
The
rest
of
this chapter
is
devoted
to the
unfolding
of
geological hori-
zons
and
layers,
and we
propose applying
the
Maupertuis'
principle
to
such
a
problem.
In a
nutshell,
the
data
and the
goal
of the
unfolding problem
can
be
sketched
as
follows:
• The
data
consist
of
—
a
complete
knowledge
of the
current
geometry
of the
geological
objects
in
their
folded
state,
and
—
limited
information
concerning
the
geometry
of
these
geological
objects
in
their
unfolded
state.
• The
problem
consists
of
determining
the
complete geometry
of
geolog-
ical objects
in
their
unfolded
state.
Prom
a
mathematical point
of
view,
this
problem
has an
infinite
number
of
solutions,
only
one of
which
corresponds
to the
actual
solution
chosen
by
mother nature. According
to the
Maupertuis'
principle, this actual solution
should minimize
the
deformations.
In
practice,
this
is
equivalent
to
saying
that
the
magnitude
of the
components
of the
strain
tensor
must
be
minimum
everywhere:
This suggests calling equations (8.58),
the
"Minimum-Deformation
princi-
ple".

8.5.
UNFOLDING
A
HORIZON
405
It
should
be
noted, however,
that
the
Minimum-Deformation principle
is
not a
panacea.
If the
current
state
7£
can be
deduced
by a
displacement
from
the
initial
state
7£[
0
],
then
the
Minimum-Deformation principle
will
generate
a
solution where
the
strain
tensor vanishes everywhere even
if
severe deforma-
tions
occurred throughout geological time between these
two
states.
In
other
words:
The
Minimum-Deformation principle
is
unable
to
characterize
the
real
trajectory
of
geological terrains throughout geological time:
it
only characterizes
the
deformations between
the
current
(final)
state
and the
initial
state
of the
studied geological objects.
This
is not a
simple theoretical remark.
For
example,
as
suggested
in figure
(8.15),
consider
the
evolution
of the
region
A of an
overturned
fold
throughout
geological time:
• the
initial
state
at
geological
time
(t = 0) was
horizontal
and flat;
• the
intermediary
state
at
geological
time
(t =
0.5)
was
severely
folded;
and
• the
current
state
at
geological
time
(t = 1) is
still
horizontal
and flat.
In
such
a
case, according
to the
Minimum-Deformation principle,
the
interme-
diary
state
is
ignored,
and it
will
be
concluded
that
the
strain tensor vanishes
in
the
region
A.
This
may be a
serious concern
if one
wishes
to
correlate
the
strain
tensor
to a
physical property, like
the
permeability
of the
terrains,
depending
on all the
deformations
that
occurred throughout geological time.
8.5
Unfolding
a
horizon
In
sedimentary geology,
due to the
gravitational origin
of the
deposition
of
sedimentary layers,
it is
generally assumed
that
horizons were approximately
flat
surfaces
at
geological deposition time
t = 0
before
the
folding
and
faulting
processes occurred
as a
result
of
successive tectonic events. Accordingly,
the
current
shape
of
horizons
can be
viewed
as a
record
of
such
tectonic
events,
from
which
the field of the
resulting deformations
can be
computed.
For
this
purpose,
a
hypothesis must
be
advanced concerning
the
folding
mechanism
between
the
initial unfolded
state
H^
of a
horizon
at
geological time
t — 0
and
its
current folded/faulted version
H
=
H^
at
today's current normalized
geological time
t =
I.
Generally, structural geologists distinguish between
two
main
types
of
such mechanisms (e.g.,
see
[236,
95, 20,
187,
192]):
• The first
type
of
unfolding
mechanism,
called
"simple shear" [89, 230]
is
illustrated
in figure
(8.19).
Simple
shear
involves
assuming
that
H[
0
]
can be
obtained
by
projecting
H
=
H[i]
onto
a
given
"unfolding"
horizontal
plane
IA
according
to a
given
field of
projection
directions.
Note
that,
as
pointed
out by
Moretti
et
al.
[164, 191],
simple
shear
is
valid
only
for
unconsolidated
material
affected
by
syndepositional
deformations.
• The
second
type
of
unfolding
mechanism,
called
"flexural
slip"
[211]
is
illus-
trated
in figure
(8.16).
Flexural
Slip
consists
in
assuming
that
H[Q]
must
be,
as far as
possible,
isometric
to H and
located
in a
given
"unfolding"
horizontal
plane
U.

406
CHAPTER
8.
ELEMENTS
OF
STRUCTURAL GEOLOGY
In
both cases,
the
deformations
of the
horizon
can be
quantified
by
analyzing,
from
a
differential
geometry point
of
view,
how the
metric properties (areas,
angles,
distances)
are
transformed between
the
folded
and the
unfolded
states.
The
simplest
way to
analyze
the
deformations
is to
study
how the
metric
tensor associated with
a
given parameterization
u
attached
to the
horizon
H
evolves
between
the
geological times
t = 0 and t = 1:
this
is
precisely
the
goal
of
this section.
For
simplicity's sake,
the
horizon
H
=
H^
observed
at
current geologi-
cal
time
t =
I
and its
unfolded
version
H^
at
depositional time
t = 0 are
assumed
to be
approximated
by
triangulated surfaces
T(H]
and
T(H^),
re-
spectively.
These triangulated
surfaces
are
associated with
the
discrete models
A^
3
(fi,
N,
x,
C
x
)
and
.M
3
(0,
A/",
X[
0
],
Cx
[0]
),
which share
the
same
set of
nodes
0 and the
same topology
but
have distinct
functions
x(a)
=
x^^a)
and
X[Q]
(a)
defining
the
location
of
each node
a €
(7
in the 3D
space
at
current
time
t =
I
and at
initial time
t = 0,
respectively.
Thanks
to the
functions
x(a)
and
X[
0
](a),
the field of
"restoration vectors"
r(a)
illustrated
in figures
(8.16)
and
(8.21)
can
then
be
introduced
and
defined
on
0 as
follows:
These vectors
can be
linearly interpolated
on
each triangle
and it is
relevant
to
note
that
they
are the
opposite
of the
displacement vectors
v(a)
introduced
on
page 402:
As
will
be
seen
in the
next paragraph,
the
unfolding
techniques proposed
in
this book rely
on the
determination
of a
parameterization u(x)
of the
horizon
H
=
_H"[i]
to be
unfolded/unfaulted. Such
a
parameterization
is
also assumed
to be
represented
by a
discrete model
,M
2
(fJ,
AT,
u,Cu)
that
shares
the
same
set of
nodes
and the
same topology
as the two
discrete models introduced
above.
8.5.1
Unfolding
techniques
Paleo-geographic
coordinates
paradigm
As
shown
in figure
(8.16),
let H
=
H^
be a folded/faulted
horizon
at
current
geological
time
t =
1.
Taking into account
the
scale
of the
domain under study,
it
is
assumed
that
H was
horizontal
at
deposition time
t = 0 and
consisted
of
a
region
H[
0
-\
of a
given horizontal plane
U
referred
to as the
"unfolding
plane." This
unfolding
plane
U is
assumed
to be at
altitude
z =
z
u
and can
be
interpreted
in
different
ways, depending
on the
geological
context
and the
value
of
z
u
.
For
example,
• if
z
u
is
greater
than
zero,
then
IA
may
correspond
to a
paleo-flood
plain;
• if
z
u
is
equal
to
zero,
then
U may
correspond
to the
delta
of a
paleo-river;
• if
z
u
is
negative,
then
IA
corresponds
to the
bottom
of the
paleo-sea.
8.5.
UNFOLDING
A
HORIZON
407
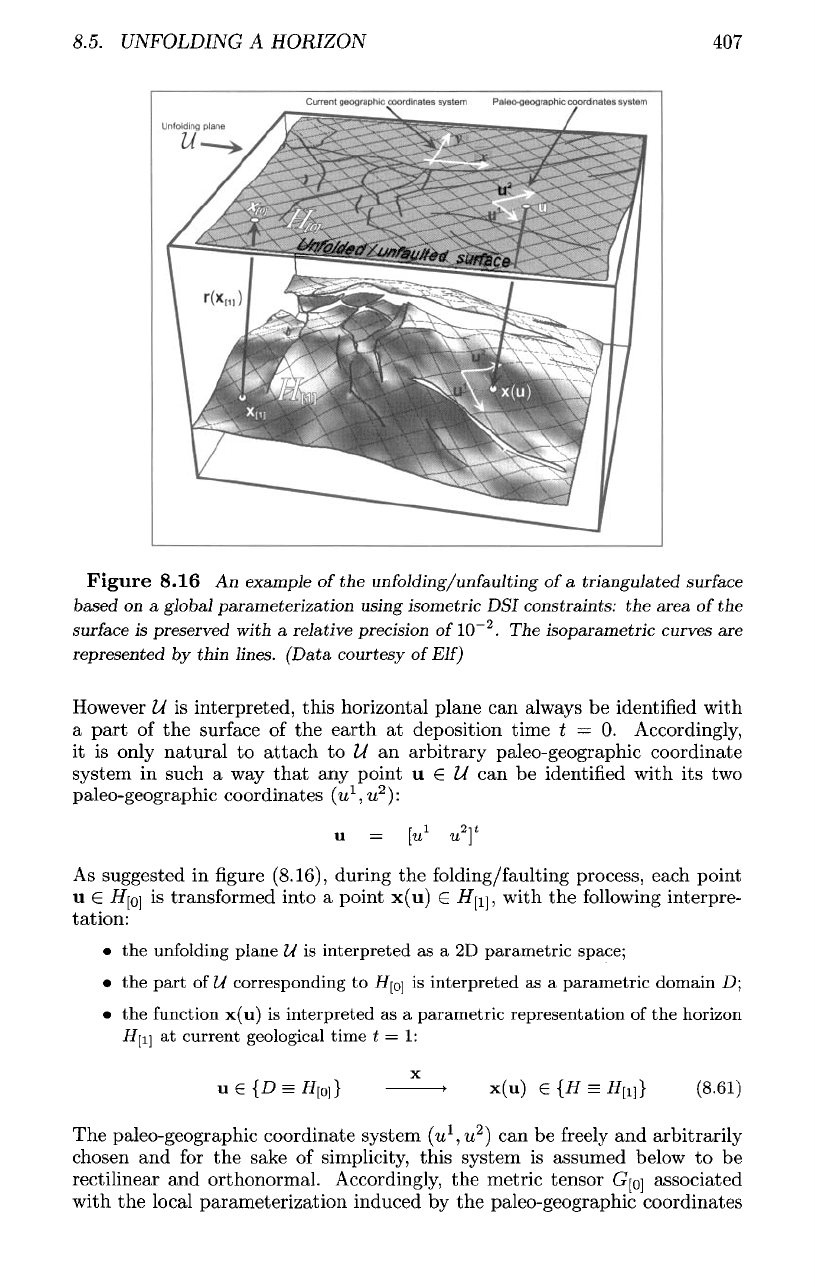
Figure
8.16
An
example
of the
unfolding/unfaulting
of
a
triangulated
surface
based
on a
global
parameterization
using
isometric
DSI
constraints:
the
area
of
the
surface
is
preserved
with
a
relative
precision
of
10~
2
.
The
isoparametric
curves
are
represented
by
thin
lines.
(Data
courtesy
of
Elf)
However
U
is
interpreted, this horizontal plane
can
always
be
identified with
a
part
of the
surface
of the
earth
at
deposition time
t — 0.
Accordingly,
it is
only
natural
to
attach
to
1i
an
arbitrary
paleo-geographic
coordinate
system
in
such
a way
that
any
point
u
€
U.
can be
identified with
its two
paleo-geographic
coordinates
(u
l
,u
2
):
As
suggested
in figure
(8.16), during
the
folding/faulting process, each point
u
e
f/"[o]
is
transformed into
a
point x(u)
e
H^,
with
the
following
interpre-
tation:
• the
unfolding
plane
U is
interpreted
as a 2D
parametric
space;
• the
part
of U
corresponding
to
H^
is
interpreted
as a
parametric
domain
D;
• the
function
x(u)
is
interpreted
as a
parametric
representation
of the
horizon
H[i]
at
current
geological
time
t = 1:
The
paleo-geographic coordinate system
(u
l
,u
2
)
can be
freely
and
arbitrarily
chosen
and for the
sake
of
simplicity, this system
is
assumed below
to be
rectilinear
and
orthonormal. Accordingly,
the
metric tensor
(7[
0
]
associated
with
the
local parameterization induced
by the
paleo-geographic coordinates
