Jean-Laurent Mallet. Geomodeling
Подождите немного. Документ загружается.

This page intentionally left blank
Chapter
5
Elements
of
Differential
Geometry
The
subsurface
is a
world
full
of
complex
surfaces
(horizons,
faults,...)
and
curves (channel axis, contact
horizon-fault,...),
and a
good understanding
of
the
geometry
of
these geological objects requires
a
minimal background knowl-
edge
of
differential
geometry. Thus,
the
basic notions related
to the
curvature
of
curves
and
surfaces
are
presented
briefly
in
this chapter.
For a
complete
overview
of
differential
geometry,
refer
to Do
Carmo
[61]
or
[162, 208]
for ex-
ample. Moreover, within
the
framework
of
this
chapter,
it
will
also
be
shown
that
differential
geometry
has
some direct practical applications
in the
realm
of
subsurface
modeling.
5.1
Parametric curves
Introduction
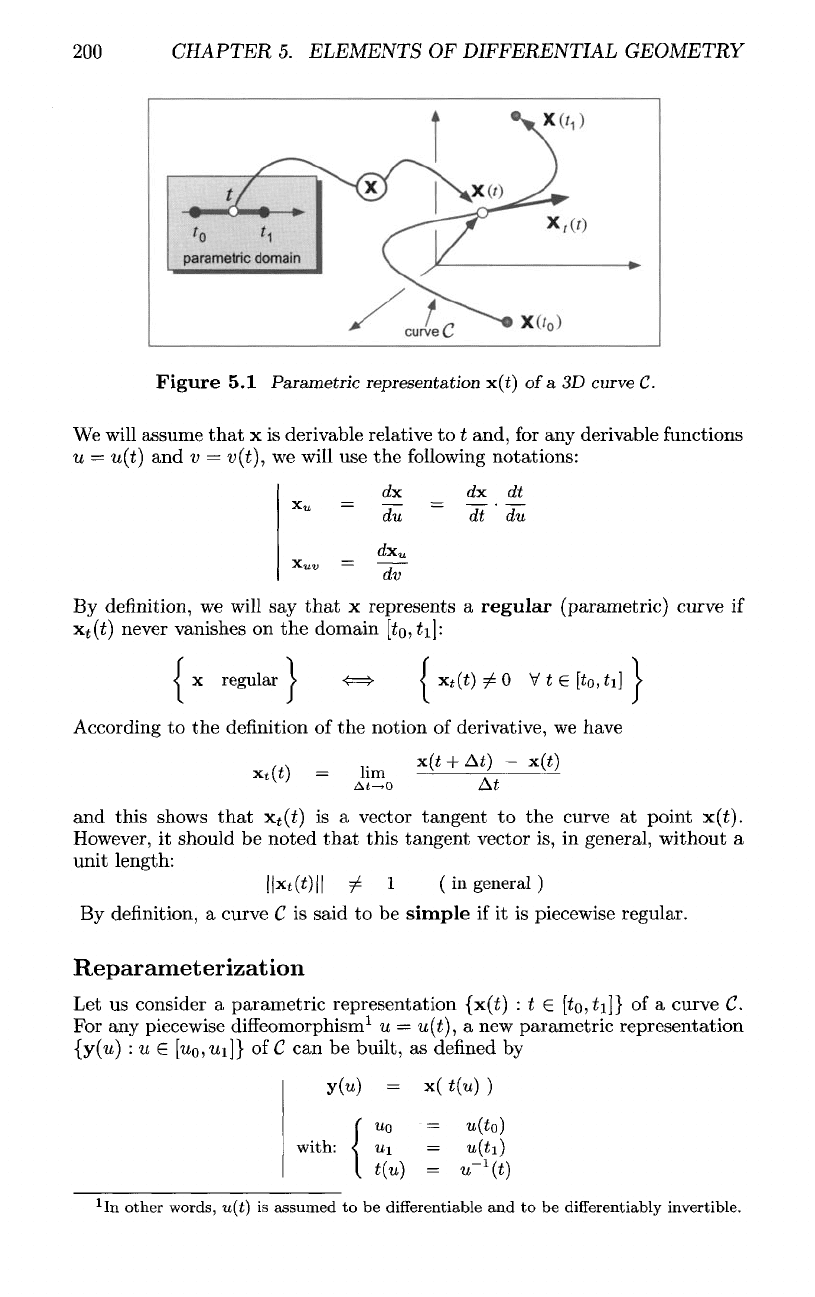
As
suggested
in figure
(5.1),
a
parametric
representation
of a
curve
C or,
more simply,
a
parametric curve,
is the
name given
to any
mapping
x
from
a
(domain)
segment
[io^i]
onto
C:
In
Computer-Aided Design (CAD) applications,
C
belongs
to the 3D
space
and
x(£)
has
three
components,
that
we
propose
to
note
as
follows:
199
200
CHAPTER
5.
ELEMENTS
OF
DIFFERENTIAL GEOMETR
Figure
5.1
Parametric
representation
x(t)
of a 3D
curve
C.
We
will
assume
that
x is
derivable relative
to t
and,
for any
derivable functions
u =
u(t)
and v —
v(t),
we
will
use the
following
notations:
By
definition,
we
will
say
that
x
represents
a
regular
(parametric)
curve
if
x
t
(t)
never vanishes
on the
domain
[to
5
^i]
:
According
to the
definition
of the
notion
of
derivative,
we
have
and
this shows
that
x
f
(t)
is a
vector tangent
to the
curve
at
point
x(i).
However,
it
should
be
noted
that
this
tangent
vector
is, in
general,
without
a
unit length:
(
in
general
)
By
definition,
a
curve
C is
said
to be
simple
if it is
piecewise regular.
Reparameterization
Let
us
consider
a
parametric representation
{x.(t)
:
t
e
[£o,£i]}
°f
a
curve
C.
For
any
piecewise
diffeomorphism
1
u
=
w(i),
a new
parametric representation
(y(i*)
:
u E
[1*0,1*1]}
of C can be
built,
as
defined
by
1
In
other words,
u(t]
is
assumed
to be
differentiable
and to be
differentiably
invertible.
5.1.
PARAMETRIC
If
x is
regular, then
y is
regular provided
that
du/dt
never vanishes
on the
parametric domain
[io»^i]-
Among
all the
possible parameterizations
of a
given curve
C, it is
important
to
mention
the
so-called "arc
length
parameterization
associated
with
the
unique
function
s
~
s(t) corresponding
to the
length
of the arc
(x(to),x(£)):
This implies
that
ds
is the
solution
of the
following
differential
equation:
As
will
be
seen
in the
following
paragraphs,
arc
length parameterization plays
a
central
role
in the
theory
of
differential
geometry
of
curves.
Unit
tangent t(s)
and
curvature k(s)
Let
us
consider
the
(unique)
arc
length parameterization x(s)
of a
curve
C
and let
t(s)
and
k(s)
be the
associated vectors,
as
defined
by
By
definition,
t(s)
is
called "unit
tangenf
vector
at
x(s),
while
k(s)
is
called
"curvature"
vector
at
x(s). Note
that
t(s)
is
really
a
unit vector,
while
k(s)
has,
in
general,
a
non-constant length,
but is
always orthogonal
to
t(s):
These
two
properties
are
easily checked
as
follows:
If
we
remember
that
the
curvature
of a
circle
is
equal
to the
inverse
of its
radius, then
the
name
of the
vector
k is
justified
by the
following
properties
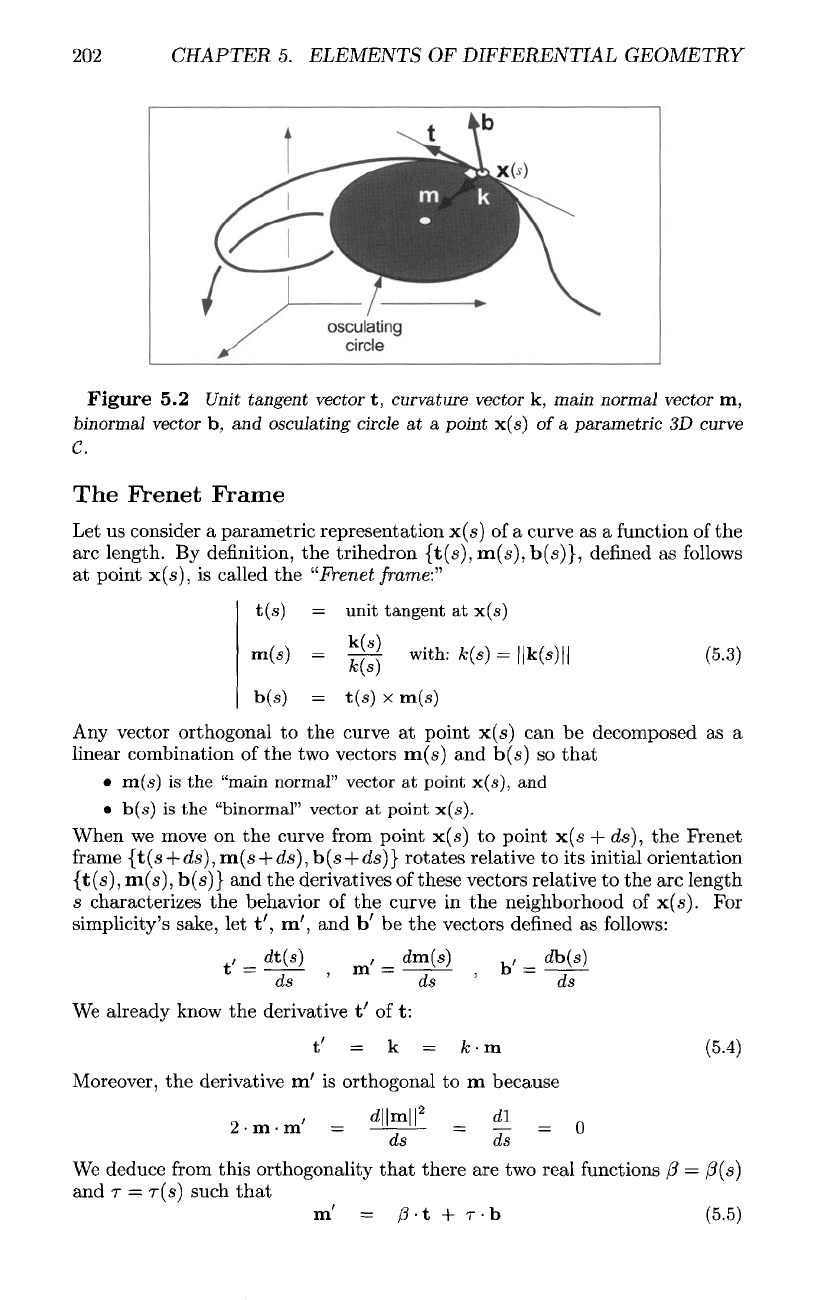
(see
figure
(5.2)):
• If the
curve
C is a
circle
of
radius
r,
then
k
points
toward
the
center
and we
haver
=
l/||k||.
• For a
general
curve
C, the
best
approximating
circle
at a
given
point
x of the
curve
is
called
the
"osculating
circle"
and has a
radius
r =
l/||k||,
which
is
called
the
"radius
of
curvature"
at
point
x.
201
202
CHAPTER
5.
ELEMENTS
OF
DIFFERENTIAL GEOMETRY
Figure
5.2
Unit
tangent
vector
t,
curvature
vector
k,
main
normal vector
m,
binormal
vector
b, and
osculating circle
at a
point
x(s)
of a
parametric
3D
curve
C.
The
Frenet
Frame
Let
us
consider
a
parametric representation x(s)
of a
curve
as a
function
of the
arc
length.
By
definition,
the
trihedron
{t(s),m(s),b(s)},
defined
as
follows
at
point x(s),
is
called
the
"Frenet
frame:"
Any
vector orthogonal
to the
curve
at
point
x(s)
can be
decomposed
as a
linear combination
of the two
vectors m(s)
and
b(s)
so
that
•
m(s)
is the
"main
normal"
vector
at
point
x(s),
and
•
b(s)
is the
"binormal"
vector
at
point
x(s).
When
we
move
on the
curve
from
point
x(s)
to
point
x(s +
ds),
the
Frenet
frame
(t(s
+
ds),
m(s
+
ds),
b(s +
ds)}
rotates relative
to its
initial orientation
|t(s),
m(s),
b(s)}
and the
derivatives
of
these vectors relative
to the arc
length
s
characterizes
the
behavior
of the
curve
in the
neighborhood
of
x(s).
For
simplicity's
sake,
let
t',
m',
and
b'
be the
vectors
defined
as
follows:
We
already know
the
derivative
t'
of t:
Moreover,
the
derivative
m'
is
orthogonal
to m
because
We
deduce
from
this orthogonality
that
there
are two
real functions
(3
=
(3(s]
and T =
T(S)
such
that
5.2.
PARAMETRIC SURFACES
203
Taking into account equations (5.3),
(5.4),
and
(5.5),
we can
write
If
we
remember
that
m =
—
(b x t),
then
we
obtain
Taking into account equations (5.3), (5.6),
and
(5.4),
we
obtain
If
we
remember
that
b
=
(t x m) and t = (m x b),
then
we
obtain
Equations
(5.4),
(5.7),
and
(5.6)
can be
grouped within
the
following
differ-
ential equations, which summarize
the
local evolution
of the
Frenet
frame:
Since
b is a
unit vector,
||b'||
measures
the
rate
of
change
of the
orientation
of
the
osculating plane
at
x(s).
In
other words,
||b'||
measures
how
rapidly
the
osculating plane turns around
the
tangent
t at
x(s)
and
this
is why T is
called
the
"torsion"
of the
curve
at
x(s).
According
to
equations
(5.6)
and
(5.8),
this torsion
is
such
that
Looking
at the
schema
in
figure
(5.2),
in the
neighborhood
of
x(s),
we can
physically
deduce
the
curve
from
the
straight line corresponding
to the
tangent
t by
applying
the
following
two
operations:
• a
bending
(curvature)
proportional
to
k,
and
• a
twisting
(torsion)
proportional
to T.
5.2
Parametric surfaces
Introduction
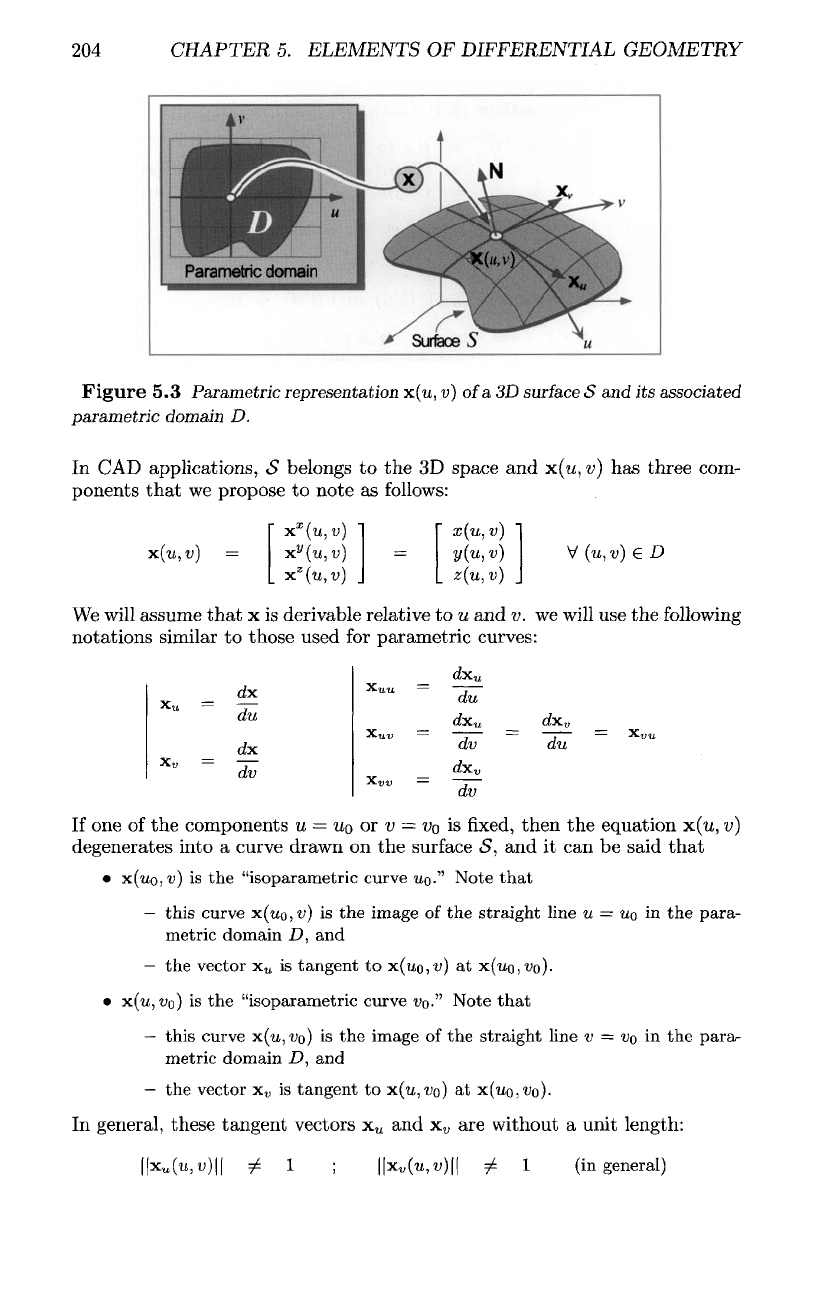
As
suggested
in
figure
(5.3),
a
parametric
representation
of a
surface
S
or,
more
simply,
a
parametric
surface,
is the
name given
to any
continuously
twice-differentiable
mapping
2
x
from
a 2D
(parametric) domain
D
onto
<S:
2
In
several
sections
of
this book (see chapter
6),
we
sometimes
use a
slightly
different
notation
for
parametric representation
of
surfaces
where
x(tt,
v]
is
replaced
by
x(u)
with
u
=
(it
1
,
u
2
)
=
(u, v).
204
CHAPTER
5.
ELEMENTS
OF
DIFFERENTIAL GEOMETRY
Figure
5.3
Parametric representation
x(u,
u)
of
a 3D
surface
S
and its
associated
parametric domain
D.
In CAD
applications,
S
belongs
to the 3D
space
and
x(w,
v) has
three com-
ponents
that
we
propose
to
note
as follows:
We
will assume
that
x is
derivable relative
to
u
and v. we
will
use the
following
notations similar
to
those used
for
parametric curves:
If
one of the
components
u —
UQ
or v =
VQ
is fixed,
then
the
equation
x(u,
v)
degenerates into
a
curve drawn
on the
surface
<5,
and it can be
said
that
•
x(uo,i>)
is the
"isoparametric curve
UQ."
Note
that
—
this
curve
x(uo,iO
is the
image
of the
straight
line
u =
UQ
in the
para-
metric domain
D,
and
—
the
vector
x
u
is
tangent
to
X.(UQ,V}
at
X.(UQ,VQ).
•
-X.(U,VQ)
is the
"isoparametric curve
VQ."
Note
that
—
this curve
x(u,
i>o)
is the
image
of the
straight line
v —
VQ
in the
para-
metric
domain
D,
and
—
the
vector
x
v
is
tangent
to
x(w,i>o)
at
x(uo,i>o).
In
general, these tangent vectors
x
u
and
x
v
are
without
a
unit length:
(in
general)

5.2.
PARAMETRIC SURFACES
205
By
definition,
if
x
u
(w,
v)
and
x
v
(w,
i>)
never vanish
and are not
colinear
on the
parametric domain
Z),
x
represents
a
regular
(parametric) surface;
in
other
words
If
the
surface
S is
regular, then
the two
vectors
x
u
(w,
v) and
x
w
(w,
v)
define
the
tangent plane
Tp(<S)
at p =
x(w,
v).
The
unit normal vector
N(w,
v)
to
<5
at
this point
is
such
that
In
practice,
the first-order and
second-order derivatives
of
x(w,
v)
relative
to
u and v are
gathered
into
two
fundamental
tensors:
• The first
fundamental
tensor
G =
G(u,
v)
also
called
the
metric
tensor:
Note
that
the
inverse
G~
1
exists
if, and
only
if, the
surface
is
regular
because
3
• The
second
fundamental
tensor
L =
L(u,
v)
also
called
the
curvature
tensor:
We
can
observe
that
We
deduce
from
these relations
that
L =
L(u,
v) can be,
equivalently,
defined
as a
function
of the
variations
of the
unit normal vector
N in the u and v
directions:
3
This
is a
direct
consequence
of the
formula
(ax
b)
-(c
x d) =
(a-c)
-(b-d)
—
(b-c)
•
(a-d)
206
CHAPTER
5.
ELEMENTS
OF
DIFFERENTIAL GEOMETRY
The first
fundamental
form
Let
us
assume
that
we are at the
point
(u, v) in the
parametric domain
D,
and we
move
a
step
du in the u
direction
and a
step
dv in the v
direction.
The
image
of
(w,
v)
moves
from
x(w,
v) on the
surface
<S
to
location
x.(u+du,
v+dv)
giving:
The arc
length
ds of
this displacement
on the
surface
is
thus:
The
square
arc
length
ds
2
of a
displacement (du,
dv) can
then
be
turned into
the
following
quadratic
form,
called
the
"first
fundamental
/orra,"
associated
with
the
metric tensor
G:
More
generally,
any
vector
W
belonging
to the
tangent
plane
T
X
(<S)
at x =
x(u,
v) has two
(contravariant) coordinates
W
u
and
W
v
relative
to the
(covariant
4
frame
(x^x^):
Multiplying
this equation (dot product)
by
x
w
and
x
v
,
respectively,
we
obtain
two
easily
verifiable
linear equations:
It is
also easy
to
check
that,
for any
pair
of
vectors
{Wi,
W2J
belonging
to
the
tangent plane
Tx(«S),
the dot
product
(Wi
•
W^)
can be
computed, thanks
to the
following
relationship:
Moreover,
according
to
equation (5.13),
it can be
observed
that
the
element
of
area
dA on the
surface
S
as
defined
by
is
such
that
4
The
notions
of
"covariant"
and
"contravariant" components
of a
tensor
are far
beyond
the
scope
of
this book
and
from
now on
will
appear
in
parentheses
as a
reminder
for
those
who
are
familiar with
tensor
calculus.
In a
nutshell,
• a
lower
index, such
as for
example
W
u
,
corresponds
to a
covariant component
of a
tensor
W,
• an
upper
index,
such
as for
example
W
u
,
corresponds
to a
contravariant component
of
a
tensor
W,
and
• the sum C =
^P
A
u
•
B
u
of
products
of
contravariant components
by
covariant
components
is a
scalar without index:
the
contravariant
and
covariant indexes "an-
nihilate" each other.
5.2.
PARAMETRIC SURFACES
207
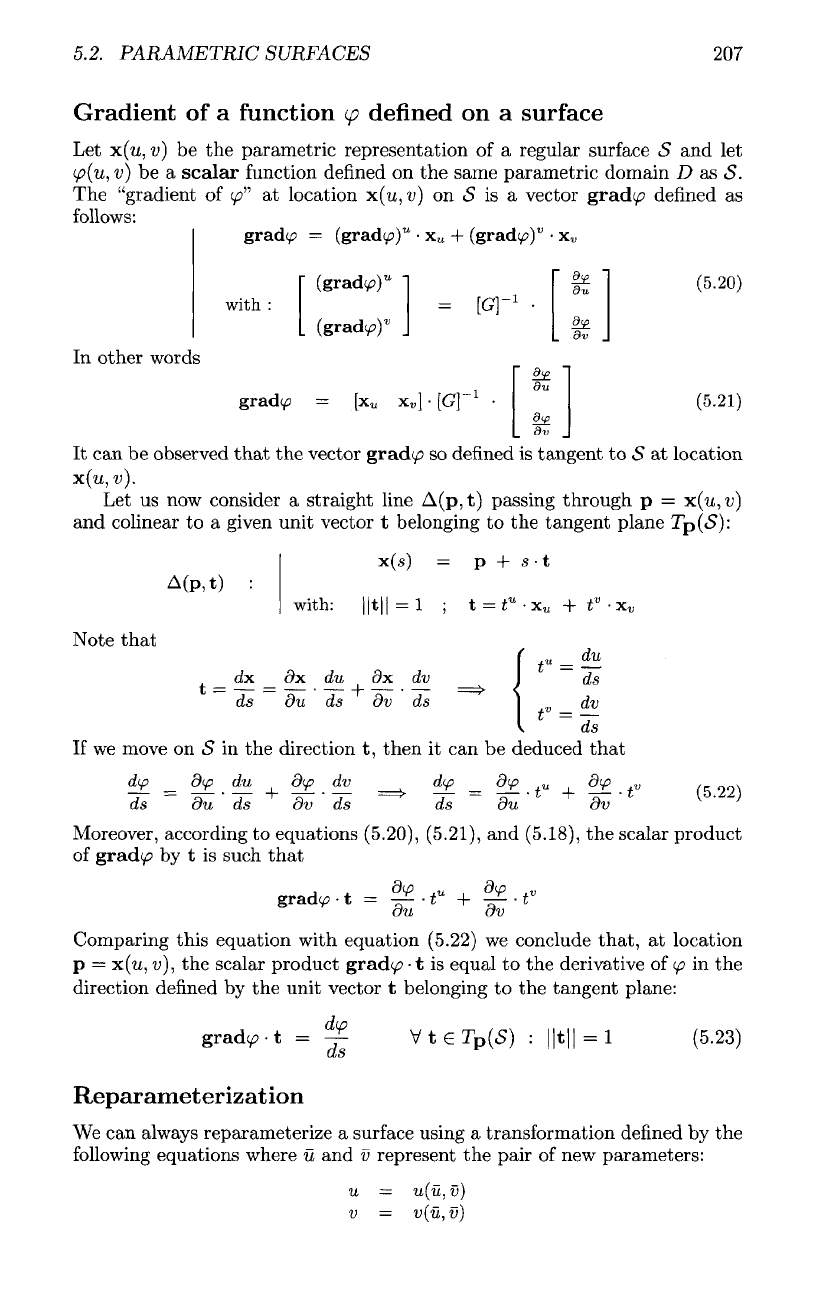
Gradient
of a
function
(p
defined
on a
surface
Let
x(w,
v]
be the
parametric representation
of a
regular
surface
S and let
(p(u,
v) be a
scalar
function
defined
on the
same parametric domain
D as
S.
The
"gradient
of
</?"
at
location
x(w,
v) on S is a
vector
grade/?
defined
as
follows:
In
other
words
It can be
observed
that
the
vector
grade/?
so
defined
is
tangent
to
S
at
location
x(w,
v}.
Let
us now
consider
a
straight
line
A(p,t)
passing
through
p =
x(w,
v)
and
colinear
to a
given unit vector
t
belonging
to the
tangent plane
Tp
(S):
Note
that
If
we
move
on S in the
direction
t,
then
it can be
deduced
that
Moreover,
according
to
equations
(5.20),
(5.21),
and
(5.18),
the
scalar product
of
grade/?
by t is
such
that
Comparing
this
equation with equation
(5.22)
we
conclude
that,
at
location
p =
X(M,
v),
the
scalar product
grady
• t is
equal
to the
derivative
of
(p
in the
direction
defined
by the
unit vector
t
belonging
to the
tangent plane:
Reparameterization
We
can
always reparameterize
a
surface
using
a
transformation
denned
by the
following
equations where
u
and v
represent
the
pair
of new
parameters:
