Jean-Laurent Mallet. Geomodeling
Подождите немного. Документ загружается.

208
CHAPTER
5.
ELEMENTS
OF
DIFFERENTIAL GEOMETRY
By
definition,
the
2x2
matrix
J
=
J(w,
v),
called
the
Jacobian
matrix
of
such
a
reparameterization,
is
defined
as
follows:
Using
the
derivation chain rule,
it is
easy
to see
that
It can
also
be
observed
that
we
have:
If
G =
G(w,
v) and L —
L(u,
v)
are, respectively,
the first and
second funda-
mental tensors associated with
the
parameterization
(«,
v) and if G =
G(u,
v)
and L =
L(u,
v) are the first and
second fundamental
tensors
associated
with
the
reparameterization
(w,
v),
then using equations
(5.12),
(5.14),
and
(5.25),
we
can
verify
that
In
practice,
a
reparameterization
is
admissible only
if it is
invertible,
that
is,
if,
for any
pair
(w,t>),
the
associated
Jacobian matrix
J(u,v)
has a
non-null
determinant, called
the
"Jacobian:"
Canonic reparameterization
Let
x(u,
v}
be a
regular parameterization
of a
surface
S.
Among
all the
possible
parameterizations,
the
simplest
one is
defined
as
follows
in the
neigh-
borhood
of a
point
p =
X(WQ,
VQ):
1.
Choose
two
vectors
U =
U(uo,t"o)
and V =
V(wo,fo)
in the
tangent
plane
Tp(5)
such
that
i
is
right-handed
2.
Define
the
reparameterization
u =
u(u,
v) and v —
v(u,
v) in
such
a way
that,
for
any
vector
W
belonging
to the
tangent
plane
Tp(S)
at p =
X.(UQ,VQ),
the
coordinates
(u,v)
represent
the
components
of W
relative
to (U, V):
The
reparameterization
so
defined
has the
following nice
properties:
5.2.
PARAMETRIC SURFACES
209
Multiplying
equation
(5.29)
by U and V,
respectively,
we
obtain
the
following
linear system:
It is
easy
to
check
5
that
In
view
of the
fact
that
S
is
regular,
x
u
x
x
v
is
non-null and, since
U x V is
also
non-null,
this
ensures
that
F"
1
exists
and can be
written
as
According
to
equation (5.31),
we
obtain
By
definition,
the
reparameterization
u(u,v)
and
v(u,v)
so
defined
will
be
called
the
"canonic
reparameterization"
associated
with
(U, V).
We
conclude
that
the
Jacobian matrix
J —
J(u,v]
of
such
a
canonic
reparameterization
can be
computed
as
follows:
Cookie
cutter
reparameterization
The
canonic reparameterization presented
in the
previous section gives
a
tool
for
reparameterizing
a
regular surface
S at a
given point
p
belonging
to S.
This section addresses
the
problem
of
extending this reparameterization
to a
neighborhood
of p:
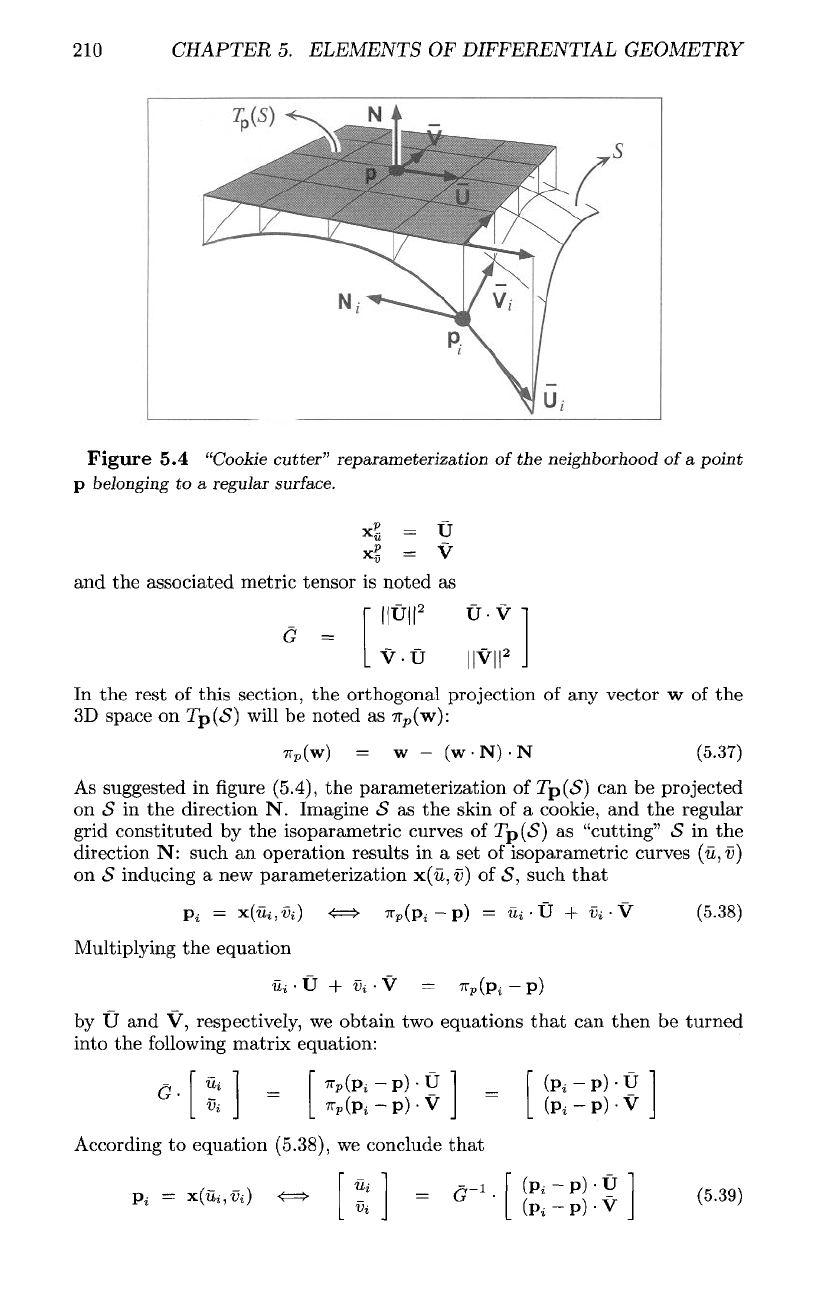
As
suggested
in
figure
(5.4),
let N be the
unit normal vector
at a
given
point
p of a
regular
surface
<S,
and let (U, V) be a
pair
of
vectors
6
such
that
Let
x
p
(w,
v)
be the
parametric equation
of the
tangent plane
Tp(<S),
as
defined
by
This
implies
that
5
For
this
purpose,
use the
formula
(a x b) • (c x d) = (a • c) • (b • d) — (b • c) • (a • d)
6
It
is not
mandatory
for U and V to be
unit
vectors
nor for
them
to be
orthogonal.
210
CHAPTER
5.
ELEMENTS
OF
DIFFERENTIAL GEOMETRY
Figur e
5.4
"Cookie
cutter"
reparameterization
of
the
neighborhood
of
a
point
p
belonging
to a
regular
surface.
and the
associated metric tensor
is
noted
as
In the
rest
of
this section,
the
orthogonal projection
of any
vector
w of the
3D
space
on
Tp(<S)
will
be
noted
as
TT
P
(W):
As
suggested
in
figure
(5.4),
the
parameterization
of
7p(<S)
can be
projected
on
<S
in the
direction
N.
Imagine
S as the
skin
of a
cookie,
and the
regular
grid
constituted
by the
isoparametric curves
of
Tp(<S)
as
"cutting"
S in the
direction
N:
such
an
operation results
in a set of
isoparametric curves
(u,
v)
on
S
inducing
a new
parameterization
x(-u,
v)
of
5,
such
that
Multiplying
the
equation
by
U and V,
respectively,
we
obtain
two
equations
that
can
then
be
turned
into
the
following
matrix equation:
According
to
equation (5.38),
we
conclude
that
5.3.
CURVATURE
OF
CURVES DRAWN
ON A
SURFACE
211
We
will
now
show
how to
determine
the
tangent
vectors
x^
and
x^
at
point
p^
located
on
<S
in the
neighborhood
of p. As
suggested
in figure
(5.4),
these
vectors
are
such
that
Let
Nj
be the
unit normal vector
at
point
p^.
According
to
equation
(5.37)
and the
above equations,
we can
write
From
equation
(5.41)
, we
deduce
that
A
similar result
can be
obtained
for
V^
and,
provided
that
N{
is
given
and
is
not
orthogonal
to N, it can be
concluded
that
This implies
that
the
metric tensor
Gi
associated with
x
s
and
x^
at
point
p^
is
such
that
5.3
Curvature
of
curves
drawn
on a
surface
The
second fundamental
form
Let
F
be a
parametric curve
defined
in the
parametric space
(u,
v)
of a
para-
metric
surface
<S:
Assuming
that
F
is
fully
contained
in the
parametric domain
D of
<S,
then
the
parameterization
x(w,
v) of
<S
can be
used
to
define
a
curve
C =
x(F)
with
the
following
parameterization:
This
curve
is
drawn
on
<S
and its
unit tangent
t =
t(£)
is
such
that
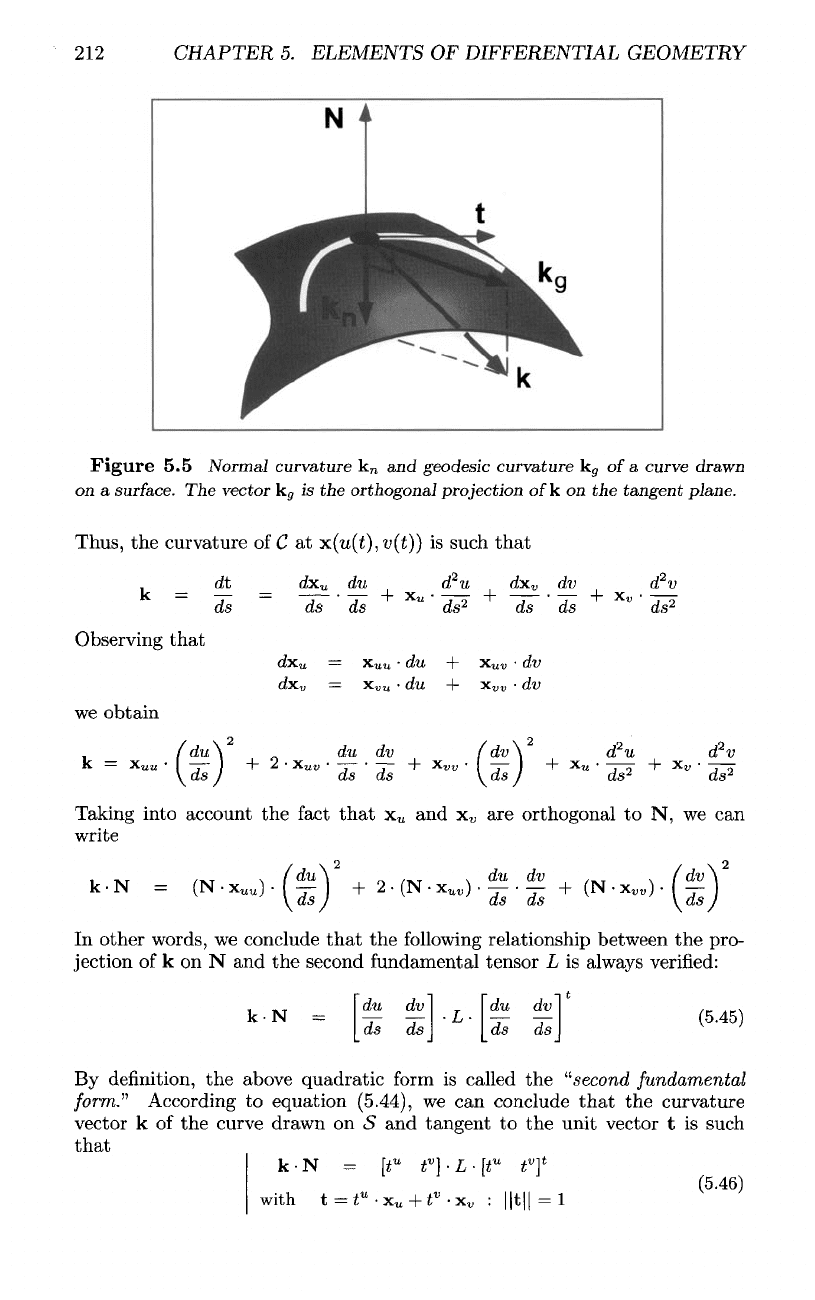
212
CHAPTER
5.
ELEMENTS
OF
DIFFERENTIAL GEOMETR
Figure
5.5
Normal
curvature
k
n
and
geodesic
curvature
k
g
of
a
curve
drawn
on
a
surface.
The
vector
k
g
is the
orthogonal
projection
of
k on tie
tangent
plane.
Thus,
the
curvature
of C at
x(w(i),
v(t})
is
such
that
Observing
that
we
obtain
Taking into account
the
fact
that
x
u
and
~x.
v
are
orthogonal
to N, we can
write
In
other words,
we
conclude
that
the
following
relationship between
the
pro-
jection
of k on N and the
second fundamental
tensor
L is
always verified:
By
definition,
the
above quadratic
form
is
called
the
"second
fundamental
form."
According
to
equation
(5.44),
we can
conclude
that
the
curvature
vector
k of the
curve drawn
on
<S
and
tangent
to the
unit vector
t is
such
that

5.3.
CURVATURE
OF
CURVES
DRAWN
ON A
SURFACE
213
Normal
and
geodesic curvatures
Let
us
consider
a
curve drawn
on a
surface
S
and
having
a
given unit tangent
t =
t(w,
v)
at
point
x(w,
i>).
By
definition,
the
real
value
fc
n
(x, t)
corre-
sponding
to the
scalar product
of the
unit normal vector
N =
N(w,
v]
by
the
curvature vector
k of
this curve
is
called
the
normal curvature
of S at
x =
x(w,
v}
in the
direction
t.
Contrary
to the
(scalar) curvature
of
curves, which
is
always positive
(or
equal
to
zero),
it is
important
to
notice (see
figure
(5.5))
that
fc
n
(x, t) has a
sign
and
that
• fc
n
(x,t) > 0 if the
center
of the
osculating circle
is
above
the
tangent
plane
T
x
(5)
relative
to N.
• fc
n
(x,t) < 0 if the
center
of the
osculating circle
is
below
the
tangent
plane
T
x
(5)
relative
to N.
In
practice,
the
(scalar) normal curvature
fc
n
(x, t) is
associated with
a
normal
curvature
vector
k
n
(x,
t), as
defined
by
As
suggested
in figure
(5.5),
the
curvature vector
k of any
curve drawn
on a
surface
<S
can
then
be
decomposed into
a
normal component
k
n
(x,
t) and a
tangent component
k
s
(x,
t):
The
component
k
9
(x,
t) so
defined
belongs
to the
tangent plane
T
X
(«S)
and is
called
the
geodesic
curvature
of the
curve.
Principal curvatures
It is
always possible
to find a
pair
(U, V) of
orthonormal
vectors tangent
to
<S
at
x(w,
v) and
such
that
Let
x(w,
v) be the
canonic reparameterization
of
<S
associated with
the
pair
(U, V) so
defined
(see page 208).
In
this case,
the
associated metric tensor
G
—
G(u,v]
becomes
a
unit
matrix.
According
to
(5.46),
for any
unit vector
t in the
tangent plane
TX(£),
we
have:
According
to
equation
(5.27),
the
matrix
L
=
L(u,v)
associated
with such
a
canonic reparameterization
is
symmetrical, which implies
the
following:
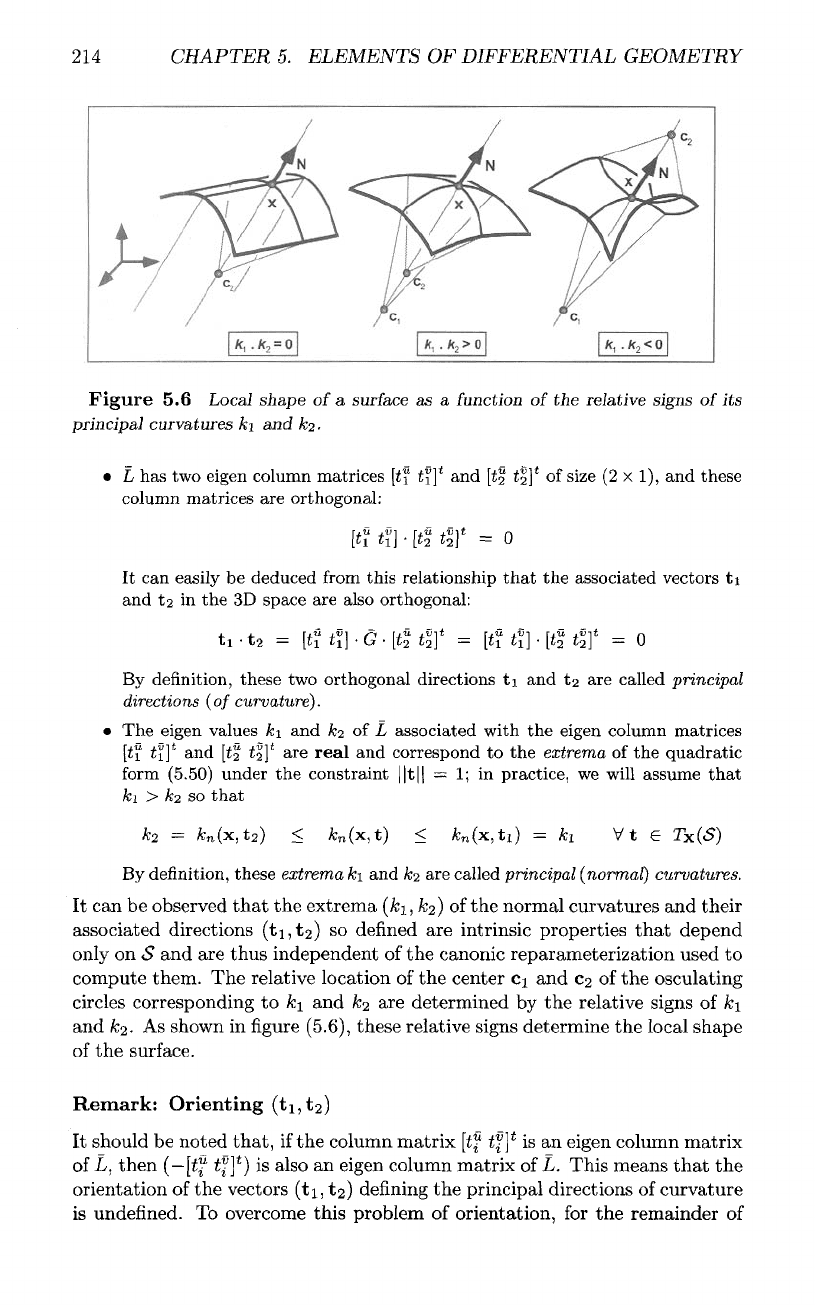
214
CHAPTER
5.
ELEMENTS
OF
DIFFERENTIAL GEOMETRY
Figure
5.6
Local
shape
of a
surface
as a
function
of the
relative
signs
of its
principal
curvatures
k\
and
k^.
• L has two
eigen
column
matrices
[t™
£"]*
and
\t%
££]*
°f
si
ze
(2 x
1),
and
these
column
matrices
are
orthogonal:
It
can
easily
be
deduced
from
this relationship that
the
associated vectors
ti
and
t2
in the 3D
space
are
also
orthogonal:
By
definition,
these
two
orthogonal
directions
ti
and t2 are
called
principal
directions
(of
curvature).
• The
eigen
values
k\
and fo of L
associated
with
the
eigen
column
matrices
[ti
ti]*
and
[£2
ty,]*
are
real
and
correspond
to the
extrema
of the
quadratic
form
(5.50)
under
the
constraint
||t||
=
1; in
practice,
we
will
assume
that
fei >
k-z
so
that
By
definition,
these
extrema
k\
and
kz
are
called
principal
(normal)
curvatures.
It can be
observed
that
the
extrema
(&i,
^2)
of the
normal
curvatures
and
their
associated directions
(ti,t2)
so
denned
are
intrinsic properties
that
depend
only
on S and are
thus
independent
of the
canonic reparameterization used
to
compute them.
The
relative location
of the
center
GI
and
c-2
of the
osculating
circles
corresponding
to
ki
and
A/2
are
determined
by the
relative signs
of
ki
and
k-2-
As
shown
in figure
(5.6), these relative signs determine
the
local shape
of
the
surface.
Remark:
Orienting
(ti,t2)
It
should
be
noted
that,
if the
column matrix
[tf
ty]*
is an
eigen column matrix
of
L,
then
(—[tf
£%]*)
is
also
an
eigen column
matrix
of L.
This
means
that
the
orientation
of the
vectors
(ti,
t2)
defining
the
principal directions
of
curvature
is
undefined.
To
overcome
this
problem
of
orientation,
for the
remainder
of
5.3.
CURVATURE
OF
CURVES
DRAWN
ON A
SURFACE
215
this chapter
we
will
assume
that
the
orientation
of
(ti,
t
2
)
has
been chosen
in
such
a way
that
(ti,t
2
,N)
is
right-handed
Gaussian
and
mean
curvatures
By
definition,
the
following
two
real numbers
K =
K(u,
v) and H
=
H(u,
v)
are
called
the
Gaussian
and the
mean curvature, respectively:
Let
us
observe
that,
for any
orthonormal reparameterization
u(u,
v) and
v(u,
v)
associated with
a
Jacobian matrix
J,
we
have:
We_can
thus conclude
that
the
real numbers
K and
H,
which
are
invariants
of
L,
can be
computed directly thanks
to the
following
formula:
7
According
to
their
definitions,
K and H
represent
the
product
and the sum
of
the
solutions
of a
second degree polynomial:
This polynomial
has two
zeros
k' and
k"
from
which
we can
deduce
the
prin-
cipal
curvatures
k\
and
k<2\
The
Gaussian
and
mean curvatures
K =
K(u,v]
and H —
H(u,v),
defined
above,
are
intrinsic properties
of the
surface
S
that
depend neither
on the
ori-
entation
of the (x,
y,
z)
coordinate system
nor on the
parameterization
x(w,
v}
of
the
surface.
They
are
used
for
characterizing
two
very important classes
of
surfaces:
• If H = 0
everywhere,
then
the
surface
is
said
to be
minimal.
For
example,
it
can
be
demonstrated
that
any
soap
film
bounded
by a
regular
closed
curve
is
a
minimal
open
surfaces [61,
165].
7
It
can be
shown [19]
that
the
following
properties
are
always true
for
square matrices:
det(^
•
B)
=
det(A)
•
det(S)
;
det(A'
1
)
=
l/det(.4)
;
trace(^4
•
B]
=
trace(B
•
A]
216
CHAPTER
5.
ELEMENTS
OF
DIFFERENTIAL GEOMETRY
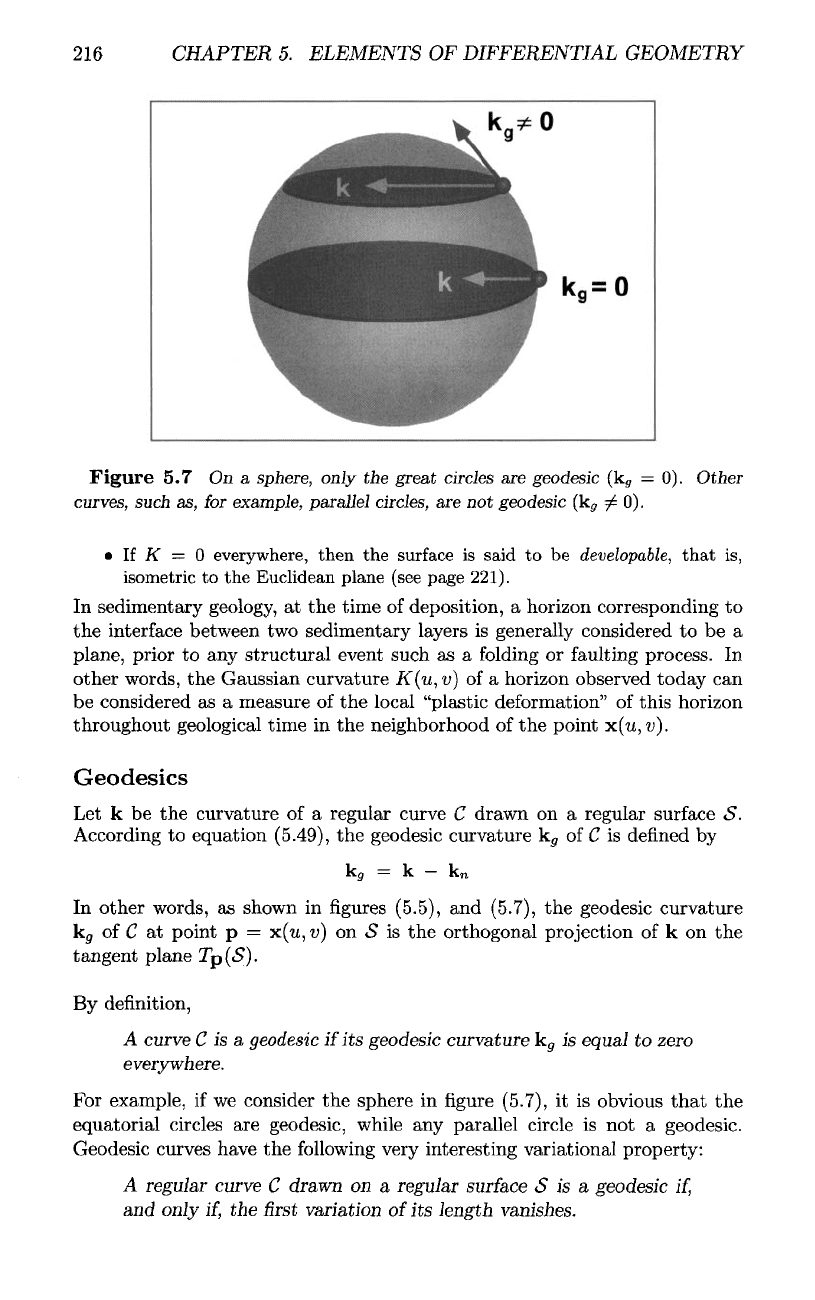
Figure
5.7 On a
sphere,
only
the
great circles
are
geodesic
(k
g
=
0).
Other
curves, such
as, for
example,
parallel circles,
are not
geodesic
(k
g
/
0).
• If K — 0
everywhere,
then
the
surface
is
said
to be
developable,
that
is,
isometric
to the
Euclidean
plane
(see
page
221).
In
sedimentary geology,
at the
time
of
deposition,
a
horizon corresponding
to
the
interface between
two
sedimentary layers
is
generally considered
to be a
plane, prior
to any
structural
event such
as a
folding
or
faulting
process.
In
other words,
the
Gaussian curvature
K(u,
v]
of a
horizon observed today
can
be
considered
as a
measure
of the
local "plastic deformation"
of
this horizon
throughout geological time
in the
neighborhood
of the
point
x(w,
v).
Geodesies
Let k be the
curvature
of a
regular curve
C
drawn
on a
regular surface
S.
According
to
equation (5.49),
the
geodesic curvature
k
9
of C is
defined
by
In
other words,
as
shown
in
figures
(5.5),
and
(5.7),
the
geodesic curvature
kg
of C at
point
p =
x(u,
v]
on S is the
orthogonal projection
of k on the
tangent
plane
Tp(«S).
By
definition,
A
curve
C is a
geodesic
if
its
geodesic curvature
k
g
is
equal
to
zero
everywhere.
For
example,
if we
consider
the
sphere
in
figure
(5.7),
it is
obvious
that
the
equatorial circles
are
geodesic, while
any
parallel circle
is not a
geodesic.
Geodesic curves have
the
following
very interesting variational property:
A
regular curve
C
drawn,
on a
regular
surface
S is a
geodesic
if,
and
only
if,
the first
variation
of
its
length vanishes.

5.3.
CURVATURE
OF
CURVES
DRAWN
ON A
SURFACE
217
To
prove
this
property,
let us
consider
the
length
t
of
such
a
curve
C
assumed
to be
parameterized
by its arc
length
s and
joining
the two fixed
points
p
0
=
x(s
0
)
and
p
:
=
x(si):
Any
infinitesimal change
<5x
in x
induces
a
variation
<5x
s
in
x
s
which
in
turn
induces
a
variation
6i
such
that
Due to the
fact
that
the
curve must stay
on the
surface
*S,
<5x
must
be
located
on
the
tangent plane
to S at
point
x. As a
consequence,
in
this tangent plane,
<5x
can
always
be
decomposed into
a
component
^x
1
-
orthogonal
to t and a
component
<5x"
parallel
to t:
The
points
x and x +
6x\\
are
both located
on the
curve, which implies
that
the
variation
<5x"
does
not
change
the
length
of the
curve. This shows
that
the
component
<5xll
generates
no
variation
of
^,
so
that
we can
write
It can be
observed
that
Taking into account
the
fact
that
<5x
stays
in the
tangent plane
Tx(<S)
implies
that
(k •
(5X
1
-
=
kg •
<5x).
The
proof
can be
completed
as
follows:
As
a
practical consequence,
we
deduce
from
this result
that
the
curves, drawn
on
a
surface
S and
corresponding
to the
longest
and the
shortest
paths
between
two
points
of
this surface
for
which
8i
vanishes,
are
geodesies
for
which
k
5
= 0.
Variations
of the
unit
normal
vector
First
of
all,
we
have:
In
other words,
N
u
and
N
w
are
orthogonal
to N and
belong
to the
tangent
plane spanned
by
x
u
and
x
v
.
This implies
that
N
u
and
N
w
can be
represented
