Hua C., Wong R. Differential Equations and Asymptotic Theory in Mathematical Physics
Подождите немного. Документ загружается.


302
where
Set
So
we introduce a relative price system, then the Cauchy problem
(3.2)
can
be reduced
as
follows:
where
Z2(t)
=
[A2(t)?i
-
2p?o~A(t)
+
g2].
So,
we reduce the dimension of the problem fortunately (including both
equation and boundary) From
(3.5)
it yields
P(2,t)
=
KN(-&)
-
ZN(-d?),
where
Thus, the value of the European put option is
-s
V(S,
r,
t)
=
P(r,
t)V(-
P(r,
t)
7
t).
We can use this method in pricing interest rate derivatives especially for
different kinds of European style options.
(1).
pricing convertible bond with default which can exercise only at
maturity’.
(2).
pricing cross currency derivatives
lo.
3.2.
American style option-valuation
of
mortgage
Mortgage is a derivative of risk asset
St
and stochastic interest ratert.
St
is the price of real estate asset. It has two features: prepayment and
default.We can divide the domain into three regions:
C1
is continuation
region;
C2
is prepayment region and
C3
is default region.
M(t)
denotes
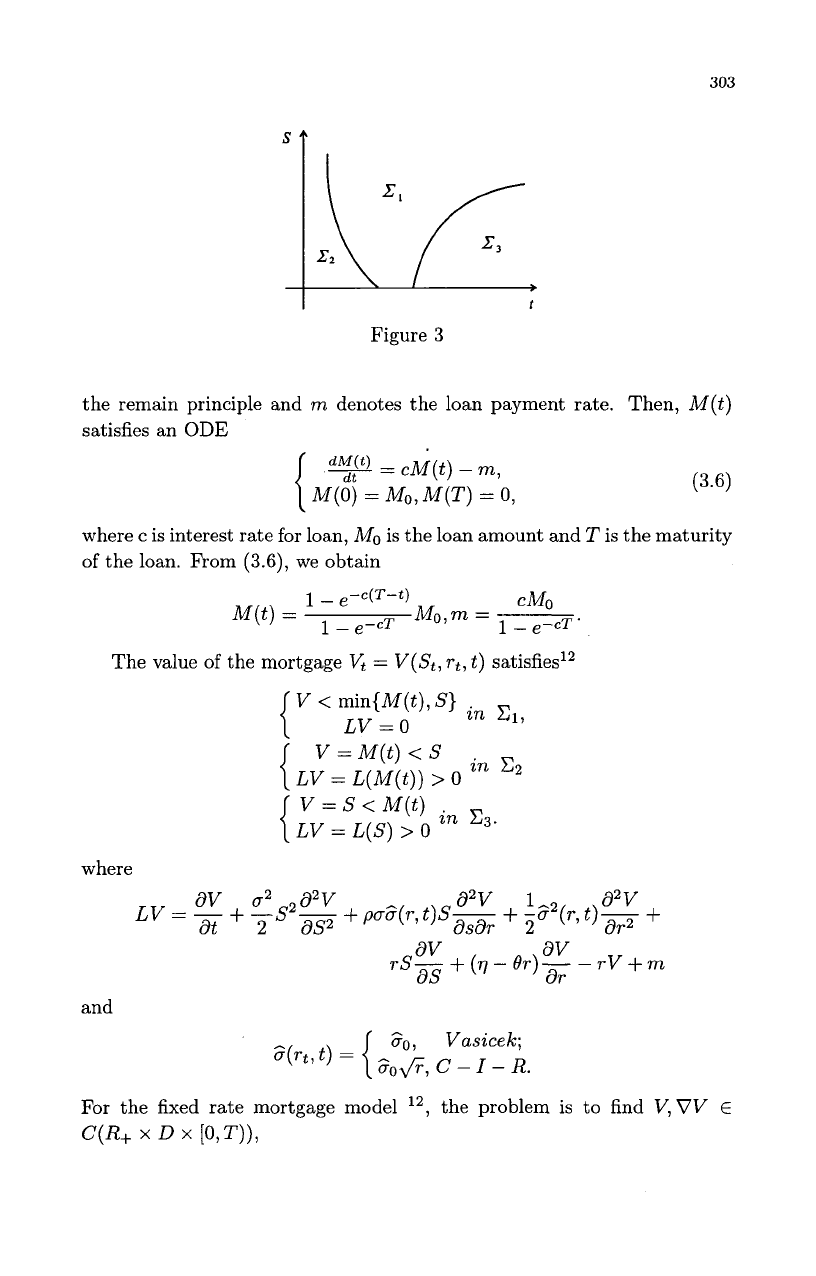
303
Figure
3
the remain principle and
m
denotes the loan payment rate. Then,
M(t)
satisfies an
ODE
(3.6)
=
cM(t)
-
m,
M(0)
=
Mo, M(T)
=
0,
{
where c is interest rate for loan,
Mo
is the loan amount and
T
is the maturity
of the loan. From
(3.6),
we obtain
The value of the mortgage
V,
=
V(St,rt,t)
satisfied2
{
V
<
min{M(t),S}
.
LV=O
an
El,
V
=
M(t)
<
S
{
LV
=
L(M(t))
>
0
V=S<M(t)
.
in
CZ
an
Cs.
LV
=
L(S)
>
0
where
dV
2
d2V
d2V
l,,
a2v
LV
=
-
+
-s'-
+
pai?(r,
t)S-
+
-a
(r,
t)-
+
rS-
+
(q
-
Or)-
-rV+m
at
2
as2
dsdr
2
dr2
dV
dV
dS
dr
and
For the fixed rate mortgage model
12,
the problem is to find
V,VV
E
C(R+
x
D
x
lO,T)),

304
s.t.
ma{ -LV, V
-
min{M(t),
S}}
=
O
v
Jt=T
=
0
(3.7)
And for the fixed rate mortgage model without default risk, the problem
is to find V, VV
E
C(D
x
[0,
T)),
s.t.
max{-LoV, V
-
M(t)}
=
0,
V(r,T)
=
0,
where
dV
l,,
d2V dV
LoV
=
-
+
-0
(r,t)-
+
(7
-
Or)-
-
rV
+
m.
at
2
ar2
dr
In
l2
we give a characteristic-difference scheme to solve the variational
inequality
(3.7)
and prove the convergence of the numerical result for
Bermudan-Style mortgage problem. The mortgage problem
(3.8)
as
a free
boundary problem is concerned with the optimal prepay boundaryr(t). We
prove that
?:
r(t)
E
C",
r(t)is a monotonic increasing function
and
4.
Variable volatility
&
implied volatility
Volatility
CJ
is the only parameter in Black-Scholes formula that is not
directly observable. But, we can find some information from the option
market, because the option price in the market is the market's view of
volatility over the life of the option.
4.1.
Implied volatility
surface
The implied volatility is the volatility derived from Black-Scholes formula
using the real option price.
For
one underlying asset, there are a series of
option prices with different strike prices and maturities.
It
is clear that the implied volatility is not
a
constant even for same
maturity. This means that it strongly depends on
K
and
T.
It also exhibits
the phenomena of 'smile' and 'skew'.
So,
the constant volatility assumption
made in Black-Scholes model is not valid in real market. One of the reason-
able ways is to assume
cr
is
a
determinate function of
St
and
t.
Following
305
T,
...
V21
v2,
”’
‘2
n
...
...
... ...
Dupire’s idea,
a(S,
t)
can be determined through quoted option prices4.
If
at time
t
=
t0,the asset price is
S
=
SO,
a series of option prices with dif-
ferent strike price and maturities are known and can be given by a form of
continuous function
V(So,tO, K,T),
where
0
5
K
<
00
and
TI
5
T
5
T2,
then from Dupire’s formula, we have
a(K,T)
=
Unfortunately, the option pricing
is
typically limited to
a
finite set of
strike prices and maturities, a naive interpolation yields to instability
of
volatility.
So,
it
is
an ill-posed problem.
4.2.
Related Optimal
Control
Problem
Problem
I
(to determine implied volatility): Find
a(S)
such that
and
V(So,to,K,T)
=
C(K),
(0
5
K
<
..)
where
to
E
[O,T)
,
T
are constants. According to Dupire’s idea,
V
=
V(S,
t;
K,T)
of a function of
K,
T
is
a
solution of Cauchy problem
(4.1).
Thus, follow Dupire’s idea, the problem
I
can be rewritten in the following
form: find
a(K)
such that
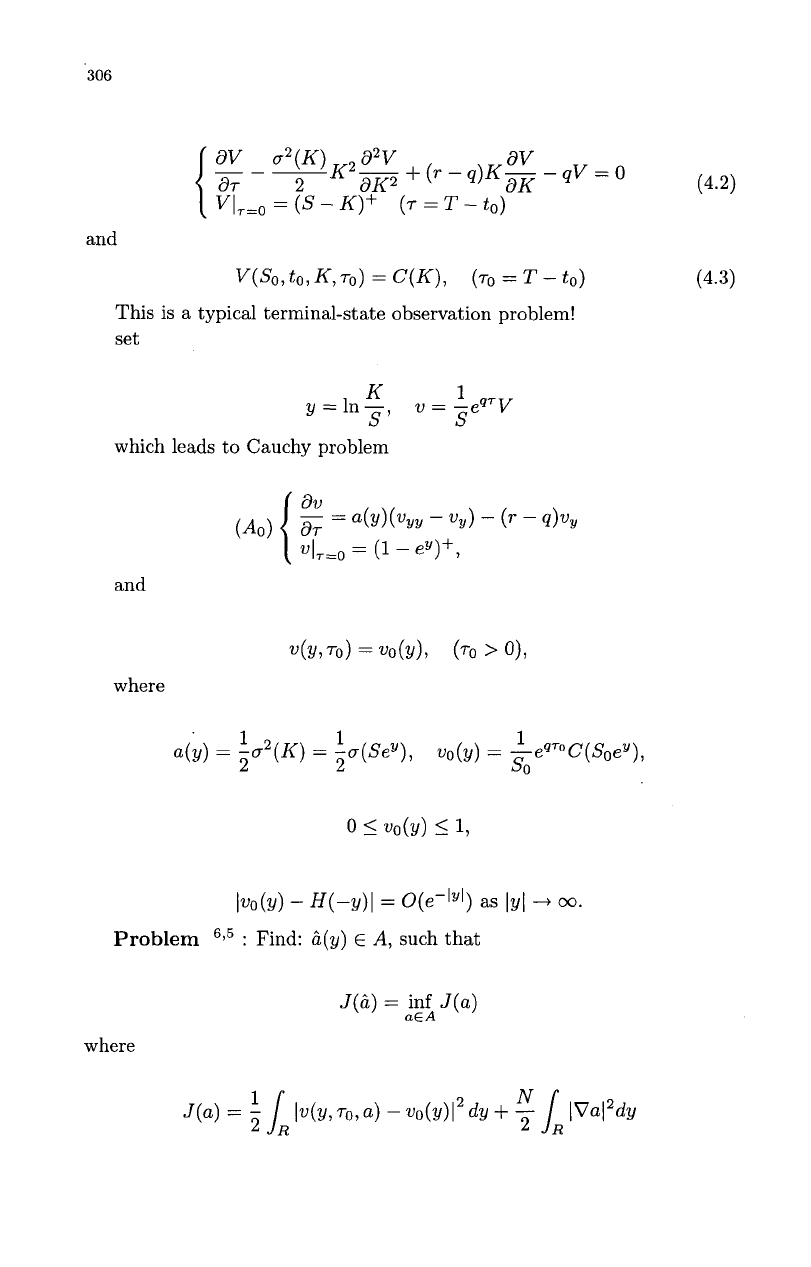
and
This is a typical terminal-state observation problem!
set
which leads to Cauchy problem
and
where
where
306
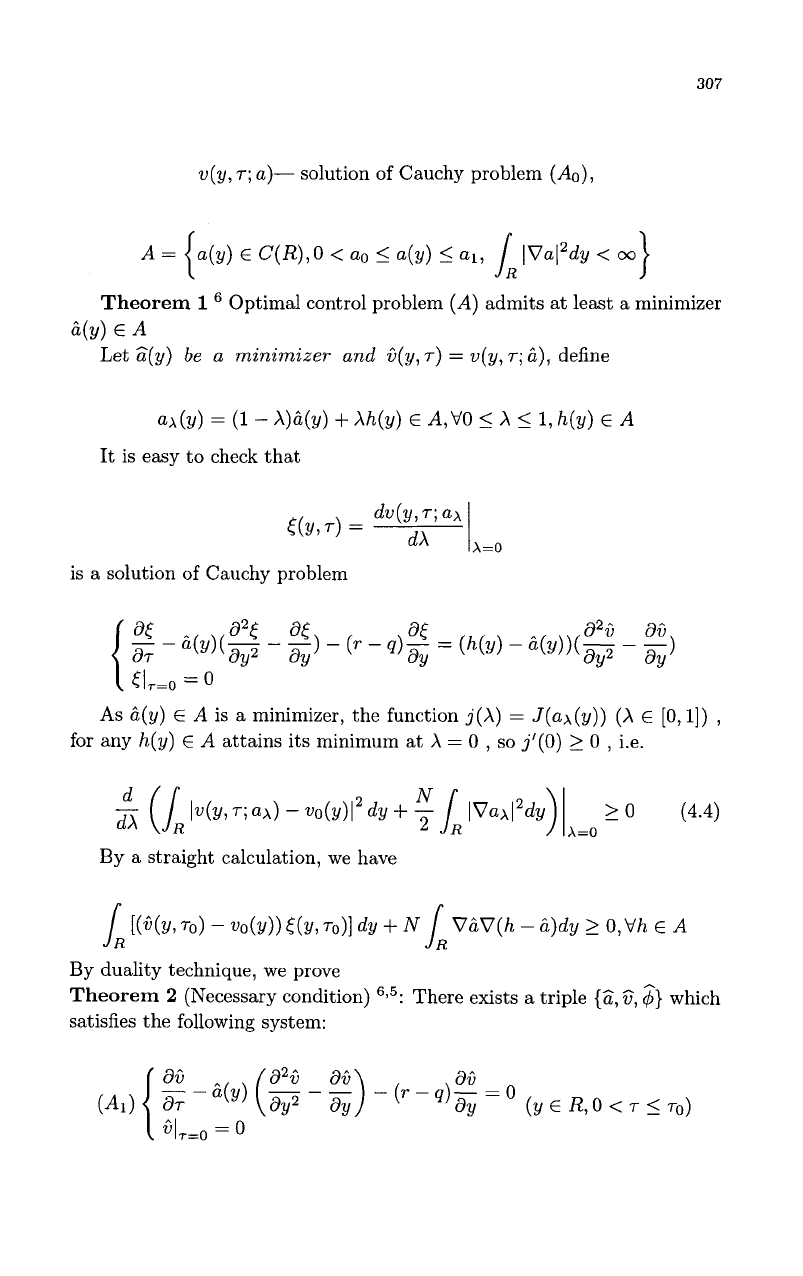
Theorem 1 6 Optimal control problem (A) admits at least a minimizer
It is easy to check that
is a solution of Cauchy problem
By a straight calculation, we have
By duality technique, we prove

308
70
(A3)NLViLV(h-ii)dy+l
~TL$
(q
-
”>
(h-&)dy
2
0,b’h
E
A
8Y
aY
Remark:
The optimal control problem is nonconvex. Therefore one can-
not expect uniqueness
of
the minimizer in the
global.
However in some
special situations the uniqueness can be expected to prove only in the local
time, i.e.
ltazlo(
=
IT
-
to1
<<
1
6.
4.3.
Numerical
results
Given
TO
=
T
-
to,Vo
=
eqToC(K)/So,
&(y) can be obtained from system
(Al)
-
(A3)
by iteration procedure.
If
a,(y)
is known, then
and we define
References
1. Amin.K.1.: Jump diffusion option valuation in discrete time, J. Finance Vol
2.
Black
F.:
The holes in Black-Scholes, <From Black-Scholes to black holes>,
Risk publishing,51-56.
3. Yang
C.,
Biao
B.
:
Free boundary and American options in a jump-diffusion
model
,
working paper,Tongji University (2003).
4. Dupire
B.:
Pricing with smile, Risk, Vol 7, 18-20.
5. Jiang
L.,
Chen Q., Wang
L.
and Zhang J.:
A
new well-posed algorithm to
recover implied local volatility, Quantitative Finance, (to appear).
6. Jiang L., Tao
Y:
Identifying the volatility
of
underlying assets from option
prices, Inverse Problems, Vol 17(2001) 137-155.
7.
Jiang
L.,
Biab
B.
and Yi F.:
A
parabolic variational inequality arising from
valuation of fixed rate mortgages, working paper, Tongji University, 2003.
8.
Li
S.
and Ren
X.:
The pricing theory of convertible bonds with the conversion
clause at maturity considering the risk
of
default, working paper, Tongji
University,2003.
48(1993) 1833-1863.

309
9. Qian X.,Jiang
L.
and Xu C.: Convergence of BTM for American options in
a
jumpdiffusion model, SIAM Numer. Anal. (to appear).
10. Xu G.:Analysis of pricing European call foreign currency option under the
Vasicek interest model, working paper,. Tongji University,2003.
11.
Xu
C., Jiang
L.
and Qian
X.:
Numerical analysis on BMT
for
a jump-diffusion
model,
J.
Computational and Applied Math., Vol 56(2003) 23-45.
12. Yuan G., Jiang
L.
and
Luo
J.:
Pricing the fixed-rate-mortgage contracts-
Limiting on payment dates to prepay or default, System Engineering-Theory
&
Practice, Vol 23
No.
9
(2003) 48-55.
13. Zhang X.: Formules quasi-explicites pour les American options dans un mod-
ele de diffusion avec sautks, Math. Comp. Simu.,Vol 38(1998) 51-61..

EXACT BOUNDARY CONTROLLABILITY OF UNSTEADY
FLOWS IN
A
NETWORK OF OPEN CANALS
TATSIEN LI (DAQIAN LI
)
Fudan University,
Shanghai
200433,
China
dqli@fudan. edu.cn
We
will establish the exact boundary controllability
of
unsteady
flows
in a tree-like
network
of
open canals with general topology (joint work with
B.
P.
Rm).
1.
Introduction
The mathematical model of unsteady flows in an open canal was established
by de Saint-Venant
[l]
in
1871.
As
an one-dimensional model, Saint-Venant
system is a first order quasilinear hyperbolic system and has been frequently
used by hydraulic engineers in their practice.
In recent years, the control problem for Saint-Venant system was studied
by many authors (see
[2]-[7]).
In particular, from
a
unified point of view,
G.
Leugering and
E.
G.
Schmidt
[2]
obtained the corresponding model of
Saint-Venant system for a network of open canals, in which the interface
conditions at any given joint point of open canals are given.
In this talk we will establish the exact boundary controllability of un-
steady flows in a tree-like network of open canals with general topology.
2.
General consideration
First of all, we will give
a
general consideration on the exact boundary
controllability for hyperbolic equations(systems),
For
a
given hyperbolic equation (system), for any given initial data
'p
and final data
$,
if we can find a time
T
>
0
and suitable boundary controls
on
the boundary
aR
of the domain
R,
such that the corresponding mixed
initial-boundary value problem with the initial data
'p
admits
a
unique
classical solution
u
=
u(t,
x)
on the whole domain
[0,
TI
x
fi,
which verifies
310

311
exactly the final condition
namely, if, by means of boundary controls, the system can drive any given
initial state
'p
to any given final state
$
at
t
=
T,
then, we say that this
system possesses the exact boundary controllability.
More precisely, if the exact boundary controllability can be realized only
for
initial and final states small enough in
a
certain sense, we say that the
system possesses the local exact boundary controllability; Otherwise, we
say the system possesses the global exact boundary controllability.
Since the hyperbolic wave has a finite speed of propagation, the exact
boundary controllability of
a
hyperbolic equation (system) requires that
the controllability time
T
must be suitably large
so
that two maximum
determinate domains associated with the initial state and the final state
respectively are separated. Then, in order to have
a
classical solution to the
corresponding mixed initial-boundary value problem on the domain
[0,
T]
x
172,
we should first prove the existence and uniqueness
of
the semi-global
classical solution, namely, the classical solution on the time interval
0
5
t
5
T,
where
T
>
0
is a preassigned and possibly quite large number. The exact
boundary controllability will be based on the existence and uniqueness
of
semi-global classical solution to the mixed initial-boundary value problem
for quasilinear hyperbolic equations (systems).
There are a number of publications concerning the exact controllability
for linear hyperbolic equations (systems) (see
J.
L.
Lions
[8],
D.
L.
Rus-
sell
[9]
etc.). For the semilinear case, using the HUM method suggested
by
J.
L. Lions and Schauder's fixed point theorem,
E.
Zuazua
[lo]
proved
the global (resp. local) exact boundary controllability for semilinear wave
equations in the asymptotically linear case (resp. the super-linear case
with suitable growth conditions). Furthermore, using
a
global inversion
theorem,
I.
Lasiecka and R. Triggiani
[ll]
established an abstract result
on the exact controllability for semilinear equations.
As
applications, they
gave the global exact boundary controllability for wave and plate equations
in the asymptotically linear case. However, only a few results are known
for quasilinear hyperbolic systems. In an earlier work,
M.
CirinL
[12]-[13]
considered the zero exact boundary controllability for quasilinear hyper-
bolic systems with linear boundary controls, but the author needed some
very strong conditions on the coefficients of the system(global1y bounded
and globally Lipschitz continuous) and his results are essentially valid only
for the system of diagonal form.
