Hua C., Wong R. Differential Equations and Asymptotic Theory in Mathematical Physics
Подождите немного. Документ загружается.


272
The corresponding expression for the growth rate is
y
+
So,t
=
Two limiting cases are considered. To compare with the cylindrical case
one sets
Rseed
=
1
and expands
(24)
in
a
power series of
(I:
yielding
OO(2,
t)
=
‘Osh
{
1
-
p(z
-
SO)
+
&(z2
-
U)
tanh
&z
-
cash
&So
&(S,”
-
U)
tanh
J‘iSo]}
+
O(a2)
with
3
U
=
5
+
26
(1
-
0,h
cosh
hS0
which should be compared to equation
(22).
Since
Rseed
<<
1,
a simple form
of
(24)
can be obtained by expanding the solution in
Rseed,
as
3.1.3.
Comments
We note that the model for a one-dimensional temperature variation in the
axial direction is not new. For example, it has been used in
l7
as the model
allows for simple analytical solutions. However, the model has not been
formally justified in the crystal growth literature.
From the explicit solutions for cylindrical crystals one can observe that
the rate of interface growth (therefore the rate of crystal growth) is asymp-
totically (for large
SO)
fi
-
y,
when
y
is constant. For small
SO,
on the
other hand, the speed is approximately
2So
-
y
for insulated chuck or
6
+
(2
-
h2)So
-
y
for a cold chuck. This suggests, for both cases, that
initially a small
y
is necessary to establish the growth.
In addition, the
value of
y
will also affect the total time required for growing crystals of
certain sizes.

273
3.2.
Radial variations: resolution
of
the first order model
Having solved the zeroth order model, to give
00
and
SO,
we can resolve
the radial variations in temperature which occur at first order in
01
and
also consider the shape of the crystal/melt interface
as
it evolves, through
S1.
From resolution of the zeroth order model we have that
which integrates with respect to
r
to give
Ol(r,z,t)
=
01(O,z,t)
+
or
ol(r,z,t)
=
Oy(z,t)
+r2@:(z,t),
(25)
(26)
where
Oy(z,
t)
=
01(0,
z,
t)
and using equation
(18)
1
@:(.z,
t)
=
-(R’(Z)@~,~(Z,
t)
-
0o(z,
t)).
Note that the function
O:(z,
t)
is known from the data and the zeroth order
solution. By adopting the same procedure as for the zeroth order model
we can find
Oy(z,t),
i.e. integrating
(13)
with respect to
r
and using the
boundary condition at
r
=
R
to eliminate
02,r.
We derive:
2Rk)
0Y(z,O)
=
0,
0
<
z
<
So(t),
(28)
0:,=
=
so:,
z
=
0,
(29)
@(z,
t)
=
-s;(t)@O,z(z,
t),
z
=
So(t)
(30)
where
Sy(t)
=
Sl(0,
t)
and its value is determined
by
the Stefan condi-
tion
(17)
at
r
=
0
as
s:,t
=
(qz
+
S;%Z)z=so(t)
7
S,o(O)
=
0.
(31)
The evolution of the interface shape is governed by
(17)
which reduces to

274
in which
r
appears
as
a parameter.
We note that
(27)-(32)
has the same structure
as
the zeroth order prob-
lem
(16), (18)-(20),
but is inhomogeneous, i.e. the zeroth order solution
provides the forcing (or heating).
A
further key difference is in the cou-
pling with the crystal/melt interface position
5’1.
Equation
(30)
provides
the lower boundary condition for
01
and
5’1
advances through
(32),
which
is consequently a first order quasi-linear partial differential equation.
In general, the coupled system
(27)-(32)
must be solved numerically.
For the pseudo-steady case, the formula can be simplified
as
follows.
From the definition of
0:
and using the pseudo-steady condition
00,~~
=
-40i,
expressions
(27)
&
(32)
reduce to
2
R
O:,zz
+
-(R’OY,z
-
0:)
1
1
4 4R
=
-(-RR”’
+
5R’R’’
+
2R’)00,z
-
-(5RR”
-
4Rt2
+
2R)Oo (33)
and
5
(R’
+
(RR”
-
2Rt2
-
R)Oo,Z
+
-
)]
(34)
2R2
004
z=S,(t)
3.2.1.
Constant radius crystals
Here
R(z)
=
1
and
00,~~
=
200.
From the definition of
0:
we have
01
-
1’ 1
1
-
-(R
Oo,z
-
00)
=
--00
2R 2
giving
Oi,z
=
-00,z/2
and
0i,2z
=
-00.
Consequently
1
2
OY,zz
-
20:
=
--0o.
Solving for
0:
in the case that
6
=
0
yields
(33)
becomes
00
1--
-
‘Osh
(SO
tanh
hS0
-
z
tanh
4.z
-
85’:
tanh
&So)
4Jz
cash
JzSo
from which
S1
can be obtained with equation
(32).

275
3.2.2.
Conical crystals
Here
R(z)
=
Rseed
+
az
and we have
1 1
0,
-
-(R'OO,~
-
00)
=
-((Q~o,~
-
00)
'
-
2R 2R
with the identity
0:
=
-@0,~~/4
from
(21).
Using these we derive
qz
=
-
(g
+
A)
00,z
+
-00,
3a
0;,+
=
($
+
g)
00,z
-
(g
+
-&)
00
2R2
and finally simplify
(33)
to:
In general numerical methods will have to be used to find a solution to
either case above, even for the pseudo-steady solution. In the following we
turn our discussion to thermal stress inside the crystal.
4.
Thermal Stress
The thermal stress experienced by the crystal during its growth leads to
the generation of structural defects in the crystal
16.
If
we want to elim-
inate these undesirable defects then one must control the thermal stress.
Although InSb is anisotropic with respect to its elasticity, we focus on the
isotropic case in this paper. Some
of
the anisotropic effects are discussed
in
2.
4.1.
Plain strain assumption
Assuming that the displacement vector is
of
the form
ii
=
(~(r),
0,O) (plain
strain) and converting to non-dimensional units reduces the equilibrium
expression for
Z
to
Note that the stress has been non-dimensionalized by
aoATE/(l-
v).
We
have assumed here that
2i
0
since we want to focus on the sole effect
of any radial temperature variations. The solution satisfying the boundary
conditions is
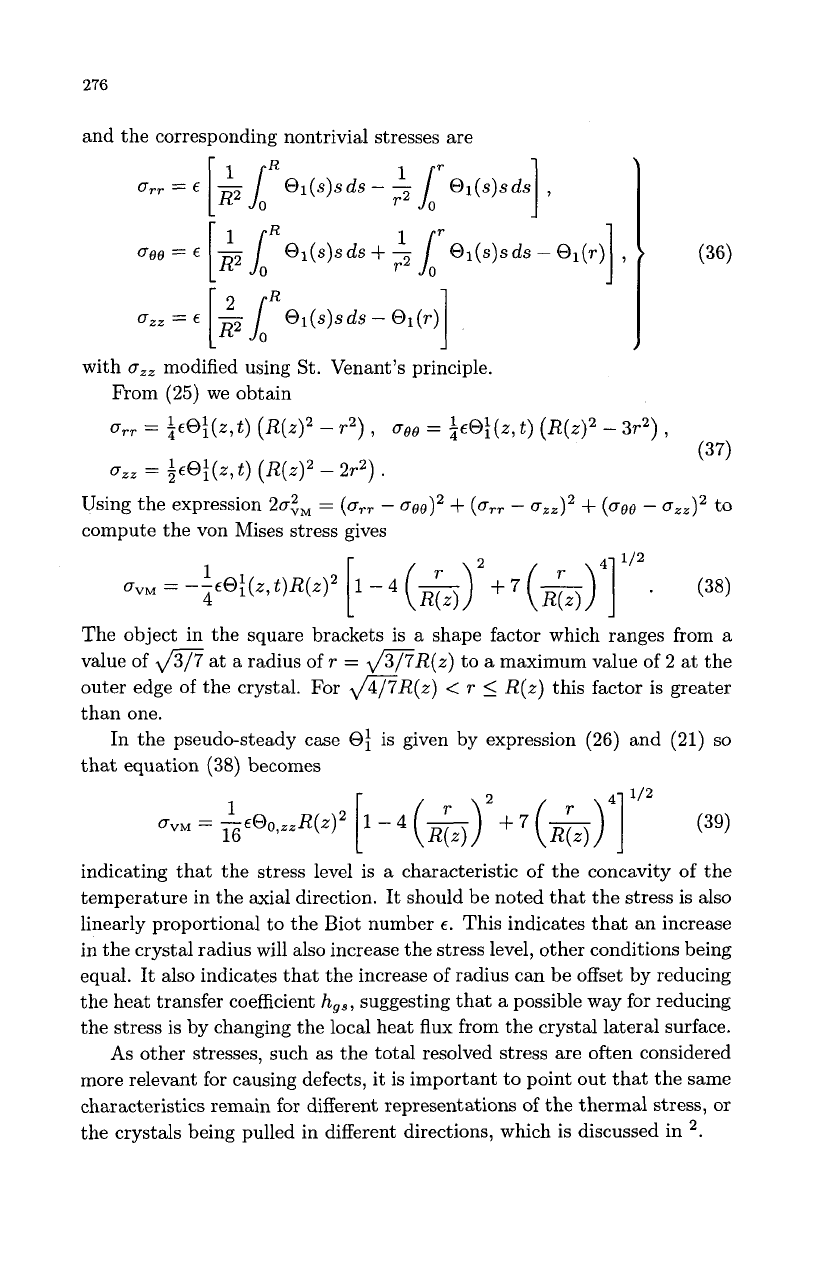
276
and the corresponding nontrivial stresses are
with
aZz
modified using St. Venant's principle.
From
(25)
we obtain
urr
=
a~Q:(z,t)
(R(z)'
-
r2)
,
gee
=
i&;(~,t)
(R(z)'
-
3r2)
,
(37)
022
=
;€0:(%,
t)
(R(z)2
-
2r2)
.
Using the expression
2a:,
=
(arr
-
compute the von Mises stress gives
+
(arr
-
azz)2
+
(aee
-
azz)2
to
The object in the square brackets is a shape factor which ranges from a
value of at a radius of
r
=
mR(z)
to
a
maximum value of
2
at the
outer edge of the crystal. For
&?R(z)
<
r
5
R(z)
this factor is greater
than one.
In the pseudo-steady case
0;
is given by expression (26) and
(21)
so
that equation
(38)
becomes
indicating that the stress level is a characteristic of the concavity of the
temperature in the axial direction. It should be noted that the stress is also
linearly proportional to the Biot number
E.
This indicates that an increase
in the crystal radius will also increase the stress level, other conditions being
equal. It also indicates that the increase of radius can be offset by reducing
the heat transfer coefficient
hgs,
suggesting that a possible way for reducing
the stress is by changing the local heat flux from the crystal lateral surface.
As
other stresses, such
as
the total resolved stress are often considered
more relevant for causing defects, it is important to point out that the same
characteristics remain for different representations of the thermal stress, or
the crystals being pulled in different directions, which is discussed in
'.

277
oylinder:
o1
pseudo.sleadyqlinder.
e2
pseudo-steady
cow:
E,
pseudo-steady
cone:
e2
pseudo4eady
1
0.9
0.8
0.1
06
*I
0.5
0.4
0.3
0.2
0.1
0
01
2
1.8
1.6
1.4
1.2
N1
0.8
0.6
0.4
0.2
0
01
I
Figure
2.
von
Mises
stress
in
MPa
for
the
cylindrical and conical crystal cases.
4.2.
Results
Due to page limitation, we only present some typical numerical results on
thermal stress obtained using the formula derived earlier. More results are
available in
’.
Assuming pulling in the
(111)
direction, the values of
E
and
v
are
E{lll)
=
6.18
x
lo4
MPa and
v{lll)
=
0.364. Thus,
E/(1
-
v)l{lll)
=
9.72
x
lo4
MPa. As a result, the dimensional constant for the
stress calculations is
cuoATE/(l
-
v)
21
107
MPa.
Figure
2
shows the stress contours of the von Mises stress in units of
MPa for the cylinder and the cone at the end of the growth. For a fixed
value of
E
the stress in the conical case is about one-half that
of
the cylin-
drical case.
Also, increasing
E
increases the stress level dramatically. By
growing a conical crystal the stress can be reduced significantly. For
a
given
temperature the amount of stress at which crystal deformation begins to
occur is known
as
the critical resolved shear stress,
ocrss.
In the case of
InSb,
ocrss
varies from 0.245 MPa
l2
to 4.90 MPa
as
the temperature
varies from
T,
=
798.4
K
to 491
K
respectively indicating that the conical
crystal remains below this critical stress level.
5.
Conclusion
In
this study, we present
a
semi-analytical approach for the temperature and
thermal stress inside a type 111-V (InSb) crystal. An important feature of

278
the approach is that it allows us to derive explicit relationships between the
thermal stress and relevant physical and geometrical parameters. This is
achieved by using asymptotic expansion
of
the solution in the Biot number,
characterizing the lateral heat flux. The asymptotic solution is obtained
by solving essentially one-dimensional problems. The results show that
the stress induced by radial temperature variation is related to the size of
the crystal (radius) and heat flux through the side surface. On the other
hand, the effect
of
the crystal radius on the stress induced by the axial
temperature variation is much weaker. The heat flux through the side
surface is an important factor for reducing the overall thermal stress inside
the crystal. The other advantages of our semi-analytical approach is that
it can be extended to cases with more complicated models for the melt and
gas flows. For example, the effect
of
the gas flow on the lateral heat flux
between the crystal surface and the gas can be modelled by
a
non-constant
heat exchange coefficient
hgs.
The motion of the melt may be modelled by
a
similar approach, which will be the subject of
a
subsequent paper.
Acknowledgement.
The authors wish to thank Firebird Semiconductors
Inc., MITACS, NSERC (Canada) and
BC
AS1 for their financial support.
References
1.
Alexander, H.
&
Hassen,
P.
(1968).
Dislocation and Plastic Flow in the Di-
amond Structure.
Solid State Physics,
22,
pp.
27-158.
2.
Bohun, C.S., Frigaard, I., Huang, H.
&
Liang,
S.
(2003).
A semi-analytical
model for the Cz growth
of
type 111-V compounds, submitted.
3.
Brown, R.A.
(1988).
Theory
of
transport processes in single crystal growth
from the melt.
AIChE Journal,
34,
pp.
881-911.
4.
Chan, Y.T., Gilbeling, H.J.
&
Grubin, H.L.
(1988).
Numerical simulation of
Czochralski growth.
Journal
of
Applied Physics,
64(3),
pp.
1425-1439.
5.
Chaudhuri, A.R., Patel, J.R.
&
Rubin, L.G.
(1962).
Velocities and densities
of
dislocations in germanium and other semiconductor crystals.
Journal
of
Applied Physics,
33,
pp.
2736-2746.
6.
Derby, J.J.
&
Brown, R.A.
(1988).
On the quasi-steady-state assumption in
modeling Czochralski crystal growth.
Journal
of
Crystal Growth,
87(2-3),
pp.
251-260.
7.
Dupret, F.
&
van den Bogaert, N.
(1994).
Modelling Bridgeman and
Czochralski Growth. In
Handbook
of
Crystal Growth,
2B,
Chapter
15,
Hurle,
D.T.J. ed., North-Holland: Amsterdam.
8.
Gulluoglu, A.N.
&
Tsai, C.T.
(1999).
Effect
of
growth parameters on dislo-
cation generation in InP single crystal grown by the vertical gradient freeze
process.
Acta Materialia,
47(8),
pp.
2313-2322.
9.
Hurle, D.T.J.
(1994).
Handbook of Crystal Growth,
1
&
2.
North-Holland:
Amsterdam.

279
10.
Hurle, D.T.J.
(1993).
Crystal Pulling from the Melt. Springer-Verlag: Berlin.
11.
Jordan, AS., Caruso, R.
&
von Neida, A.R.
(1980).
A Thermoelastic Anal-
ysis of Dislocation Generation in Pulled GaAs Crystals.
The Bell System
Technical Journal,
59(4),
pp.
593-637.
12.
Mil'vidskii, M.G.
&
Bochkarev, E.P.
(1978).
Creation of defects during the
growth of semiconductor single crystals and films.
Journal
of
Crystal Growth,
44(1),
pp.
61-74.
13.
Muller, G.
(2002).
Experimental analysis and modeling
of
melt growth prc-
cesses.
Journal
of
Crystal Growth,
237-239,
Part
3,
pp.
1628-1637.
14.
Miiller,
G.
(1988).
Convection and Inhomogeneities in Crystal Growth from
Melt. In
Crystals,
12,
F'reyhardt, F.C. ed., Springer-Verlag: Berlin.
15.
Prasad, V., Zhang, H.
&
Anselmo, A.
(1997).
Transport Phenomena in
Czochralski Crystal Growth Process.
Advances
in
Heat Transfer,
30,
pp.
313-
435.
16.
Sinno, T., Dornberg,
E.,
von Ammon,
W.,
Brown, R.A.
&
Dupret,
F.
(2002).
Defect Engineering of Czochralski SingleCrystal Silicon.
Material Science
and Engineering Reports,
28,
pp.
149-198.
17.
Tatachenko, Y.A.
(1993).
Shaped Crystal Growth. Kluwer Academic Pub-
lishers: Boston.
18.
Tanahasji, K., Kikuchi, M., Higashino,
T.,
Inoue, N.
&
Mizokawa, Y.
(2000).
Concentration of point defects changed by thermal stress in growing CZ
silicon crystal: effect
of
the growth rate.
Journal
of
Crystal Growth,
210(1),
pp.
45-48.
19.
Vokl, J.
(1994).
Stress Computation in Czochralski. In
Handbook
of
Crystal
Growth,
2B,
Chapter
14,
Hurle, D.T.J. ed., North-Holland: Amsterdam.

ASYMPTOTIC BEHAVIOUR OF THE TRACE FOR
SCHRODINGER OPERATOR ON IRREGULAR DOMAINS*
HUACHENANDCHUNYU
School
of
Mathematics and Statistics,
Wuhan University,
Wuhan,
430072,
P.R.
China
1.
Main Result
In
1953,
L.M. Gelfand and
B.M.
Levitan studied the trace
of
the Sturm-
Liouville problem
(1)
-Y"
+
dZ)Y
=
XY,
z
E
(077r),
i
Y(0)
=
Y(.rr)
=
0,
where
q(z)
is bounded and differentiable on
[O,.rr].
They obtained the
fol-
lowing trace identity
This result reveals a direct relation between the eigenvalues and the oper-
ator quantities. After that, the research focus is on the trace identity of
Sturm-Liouville equation with general boundary conditions and the equa-
tion with singularities.
The trace identity in k-dimensional case has been studied by Cao Cewen
in
1979.
He considered the eigenvalue problem
-Au
+
q(z)u
=
Xu,
in
R,
on
dR,
(2)
I
u
=
0,
where
R
c
Rk
is
a bounded, connected domain with piecewise smooth
boundary
dR,
A
is the k-dimensional Dirichlet Laplacian on
R,
q(z)
is
*The research
is
supported
by
the
NNSFC
280

281
bounded and differentiable in
R.
Let
Xj
be the j-th eigenvalue,
pj
be the
j-th eigenvalue of the Dirichlet Laplacian, i.e. the case of
q(z)
=
0,
he
proved the following average displacement formula
which
lfllk
represents the k-dimensional Lebesgue measure of
a.
and in a
special case, when
R
is k-dimensional open cube, he proved
where
IdflIk-1
denotes the
(k
-
1)-dimensional Lebesgue measure of
80,
wk-1
=
(2J;;>"'I'(?
+1).
The second result
is
the extension of Gelfand-
Levitan's trace identity under the special situation.
In this paper, we consider the eigenvalue problem of Schrodinger oper-
ator defined on more general domain
(P)
Lu
=
-Au
+
q(z)u
=
Xu,
u
=
0,
on
aR,
in
R,
00
where
R
=
U
Rm
is an open subset
of
Wk
with boundary
dR,
and the
connected regions
R,
with piecewise smooth boundaries are bounded and
Rj
fii
=
8
if
j
#
i.
we assume throughout this paper that
101
lk
2
)fl2)k
2
m=
1
+m
where
I
.
Ik
denotes the k-dimensional Lebesgue measure-of
R.
As
is
well-known that the scalar
X
is said to be an eigenvalue
of
(P)
if there exists
a
nonzero
u
E
Hi(R)
which satisfies
(P)
in the distributional sense. The
problem
(P)
has discrete eigenvalues which can be written as a sequence
(Xj)zl
with
Aj
-+
+co
as
j
-+
+a.
When
q(z)
=
0,
the eigenvalue problem of Dirichlet Laplacian associated
with
(P)
is
as
follows:
LOU
=
-Au
=
Au,
u
=
0,
on
aR,
in
R,
(D)
