Hua C., Wong R. Differential Equations and Asymptotic Theory in Mathematical Physics
Подождите немного. Документ загружается.


252
LECTURE
V
DIFFERENCE EQUATIONS AND ORTHOGONAL
POLYNOMIALS
Difference equations might be a handy and practical means to compute diffe-
rential equations, but they are considerably more complicated to analyze.
Arieh Iserles
1.
Introduction
Orthogonal polynomials play an important role in many branches of
mathematical physics; for instance, quantum mechanics, scattering theory,
and statistical mechanics. A major topic in orthogonal polynomials is the
study of their asymptotic behavior
as
the degree grows to infinity. Since the
classical orthogonal polynomials (Hermite, Laguerre, and Jacobi) all satisfy
a second-order linear differential equation, their asymptotic behavior can
be obtained from the WKB approximation
or
the turning point theory
(Lecture 111).
For
discrete orthogonal polynomials (e.g., Charlier, Meixner,
and Krawtchouk), one can use their generating function to obtain a Cauchy
integral representation and then apply the steepest descent method
or
its
extensions (Lecture
11).
However, there are orthogonal polynomials that
neither satisfy any differential equation nor have integral representations.
A
powerful method, known
as
the steepest descent method for the Riemann-
Hilbert problem, has recently been developed that can be applied to such
polynomials. Two papers that deserve special mention are Deift
&
Zhou
[lo],
and Bleher
&
Its
[5].
In fact, there is a whole group of people now
working in this area, which includes, in addition to the authors of the two
above mentioned papers, Kuijlaars, Kriecherbauer, McLaughlin, Vanlessen,
Venakides and Van Assche; see, e.g.,
[8,
9,
12,
13,
141.
However, in our view, a more desirable approach to derive asymptotic
expansions for orthogonal polynomials is to develop an asymptotic the-
ory
for
linear second order difference equations, in the same way as Langer,
Cherry, Olver and others have done for linear second-order differential equa-
tions (Lecture
111).
Our view is based on the fact that any sequence of
253
orthogonal polynomials satisfies the three-term recurrence relation
P,+l(S)
=
(an2
+
b,)p,(z)
-
cnp,-l(z),
n=1,2,...
,
(1.1)
where
a,,
b,
and
c,
are constants; see
[16,
p.421.
If
2
is a fixed number, then
this recurrence relation is equivalent to the second-order linear difference
equation
y(n
+
2)
+
npa(n)y(n
+
1)
+
nqb(n)y(n)
=
0,
(1.2)
where
p
and
q
are integers.
asymptotic expansions of the form
When the coefficients
a(n)
and
b(n)
have
with
QO
#
0
and
PO
#
0,
asymptotic solutions to equation
(1.2)
have
been given by Birkhoff [3], Birkhoff and Trjitzinsky [4]. But, their papers
have been considered far too complicated and even impenetrable.
A
more
accessible approach to these results has been given in more recent years
by Wong and Li [23, 241. However, the results in all these papers can be
applied to (1.1) only when
5
is a fixed number. When
z
is a parameter
and allowed to vary, not much work has been done in this area until just
recently. In a series
of
papers [19, 20,
211,
Wang and Wong have derived
asymptotic expansions for the solutions to (l.l), which hold uniformly for
z
in infinite intervals. They first define
a
sequence
{K,}
recursively by
Kn+1/K,-1
=
c,,
with
KO
and
K1
depending on the particular sequence
of polynomials. Then they put
A,
=
anKn/K,+l,B,
--
b,K,/K,+1
and
P,(z)
=
p,(z)/K,,
so
that (1.1) becomes
Pn+1(z)
-
(A,z
+
B,)P,(z)
+
P,-l(z)
=
0.
(1.4)
The coefficients
A,
and
B,
are assumed to have asymptotic expansions of
the form
where
0
is a real number and
a0
#
0.
In this lecture, we summarize the results in [23, 241 and [20, 211. More
precisely, in Sec.
2
we present the results for equation (1.2) when
p
=
q
=
0,
and discuss the general case in Sec. 3. Equation (1.4) is studied in Sec. 4
when
0
#
0,
and in Sec.
5
when
0
=
0.
Interested readers are referred to
the original papers for proofs of the results.
254
2.
Normal and Subnormal
Series
When
p
=
q
=
0,
equation
(1.2)
becomes
y(n
+
2)
+
a(n)y(n
+
1)
+
b(n)y(n)
=
0.
(2.1)
Asymptotic solutions to this equation are classified by the roots
of
the
characteristic equation
p2
+
aop
+
bo
=
0.
(2.2)
Two possible values
of
p
are
p1,p2
=
--a0
f
-a;
-
bo
.
2
c
Y2
If
p1
#
p2,
i.e.,
a;
#
4b0,
then Birkhoff
[2]
showed that
(2.1)
has two linearly
independent solutions
yj(n),j
=
1,2,
such that
where
c0,j
=
1
and
s
=
1,2,....
This construction fails when and only when
p1
=
p2,
i.e.,
when
a:
=
4bo.
The series in
(2.4)
are known as
normal
series
or
normal
solutions.
If
p1
=
p2
but their common value
p
=
-5uo
is not a root of the
auxiliary equation
alp
+
bl
=
0,
(2.7)
i.e.,
2bl
#
aoal,
then Adams
[l]
showed that
(2.1)
has two linearly inde-
pendent solutions
yj(n),j
=
1,2,
such that
255
where
1
bl
4 2bo
a=-+-,
(2.10)
and
co
=
1.
Series
of
the form
(2.8)
are called subnormal series or subnor-
mal solutions. Higher coefficients can be determined by formal substitution.
When the double root of the characteristic equation
(2.2)
satisfies the
auxiliary equation
(2.7),
i.e., when
2bl
=
aoal,
we have three (exceptional)
cases to consider, depending on the values of the zeros al,az(Re
a2
2
Re
al)
of the indicia1 polynomial
q(a)
=
a(a
-
l)p2
+
(~1a
+
a2)p
+
b2.
(2.11)
Case (i)
:
a2
-
a1
#
0,
1,2,...
.
In this case,
(2.1)
has independent
solutions yj(n),j
=
1,2,
of the form
M
n
-+
00,
(2.12)
with
CO,~
=
1.
j
=
1.
A
second independent solution is given by
Case (ii)
:
012
-
a1
=
1,2,
s..
.
Here,
(2.12)
applies only in the case of
(2.13)
where the prime on
C
denotes that the term for
s
=
a2
-
a1
is absent. The
coefficients c and
d,
can be determined by formal substitution, beginning
with
do
=
1.
Case (iii)
:
a2
=
al.
As
in case (ii),
(2.12)
again gives only one solution
y1
(n).
The second solution is given by
(2.14)
3.
Equation
(1.2)
with
p
and
q
#
0.
Many orthogonal polynomials satisfy difference equations of the form
(1.2),
but not
of
the form
(2.1).
For example, the recurrence relation for
the Charlier polynomials is
c:2,(.)
+
(n
+a
-
.)Cp(.)
+
anc:”l(.)
=
0,
a
#
0,
(3.1)

256
and the recurrence relation for the Bessel polynomials
y,(~)
is
Yn+l(Z)
=
(2n
+
l)ZYn(Z)
+
Yn-l(Z);
(3.2)
see
[6,
Chap.VI].
(1.2)
can be reduced to
(2.1)
by using the transformation
If
the exponents
p
and
q
in
(1.2)
are related in the manner
q
=
2p,
then
x(n)
=
[(n
-
2)!]Py(n).
~(n
+
2)
+
np+P~(n)~(n
+
1)
+
n"+""b*(n)Z(n)
=
0,
(3.3)
(3.4)
Indeed, substitution of
(3.3)
in
(1.2)
gives the equivalent equation
where
b*(n)=b(n)(1-;)'=xila.
b:
s=O
(3.5)
(Note that the constant term in the expansion
(3.5)
is again not zero.) If
q
=
2p
then, by taking
p
=
-p, (3.4)
becomes an equation
of
the same
form as
(2.1).
Thus, our discussion of equation
(1.2)
consists of only two
cases, namely, (i)
q
<
2p
and (ii)
q
>
2p.
We set
k
=
2p
-
q.
Each of these
two cases has two or three subcases, depending on the values
of
k.
In case (i), i.e., when
k
>
0,
it has been shown in
[23]
that equation
(1.2)
has an asymptotic solution of the form
00
y1(n)
-
[(n
-
2)!]Pp"n"
c
c",
ns
s=o
where
p
=
--ao,a
=
UI/UO
if
k
>
1,
and
A
second independent solution is given by
Mr
y2(n)
=
[(n
-
2)!]9-Pp"n"
x
5,
ns
s=o
where
p
=
-bo/ao,
and
bl
a1
-
--
-p+q
bo
a0
if
k
=
1,
if
k>l.
(3.9)
(3.10)

257
Recursive formulas can be obtained for the coefficients
cs
and
ds
by formal
substitution
.
In case (ii), i.e., when
k
<
0,
we have three subcases to consider de-
pending on whether
k
=
-1,
or
k
5
-3
and is odd,
or
k
5
-2
and is even.
If
k
=
-1,
then two asymptotic solutions are
of
the form
00
j
=
1,2,
where
pj”
=
-bo,yj
=
-ao/pj
and
(3.12)
If
k
5
-3
and
k
is odd, then the exponential factor in
(3.11)
is absent and
equation
(3.11)
becomes
(3.13)
where
p
and
a
are as given in
(3.11)
and
(3.12).
If
k
5
-2
and
k
is even,
then coefficients of odd powers of
n-f
all vanish and
(3.11)
simplifies to
(3.14)
with
pj”
=
-bo(j
=
1,2),
aj
=
a
given in
(3.12)
if
k
=
-4,
-6,.
.
.
,
and
if
k
=
-2. (3.15)
4.
Airy-type
Expansion
Let
TO
be
a
constant, and put
I/
:=
n
+
TO.
Clearly, the expansions in
(1.5)
can be recast in the form
In
(1.4),
we now let
z
=
vet
and
P,
=
An.
Substituting
(4.1)
into
(1.4)
and
letting
n
+
00
(and hence
u
-+
m),
we obtain the characteristic equation
x2
-
(aht
+
p;)x
+
1
=
0.
(44
The roots of this equation are given by

258
and they coincide when
t
=
tf,
where
a;t*
+
0;
=
f2.
(4.4)
The values
th
play an important role in the asymptotic theory of the three-
term recurrence relation
(1.4),
and they correspond to the transition points
(i.e., turning points and poles) occurring in differential equations; cf.
[15,
p.3621. For this reason, we shall also call them
transition points.
Since
t+
and
t-
have different values, we may restrict ourselves to just the case
t
=
t+.
For
t
near
t+,
we try
a
formal series solution to
(1.4)
in the form
00
Pn(X)
=
CXs(W,
(4.5)
s=o
where
b
is a small quantity depending on
v
(e.g.,
a
power of
v-’)
and
5
depends on
x
and
v.
This particular form of expansion was suggested by
Costin and Costin
[7].
In terms of the exponent
B
in
(4.1)
and the transition
point
t+,
we have three cases to consider; namely, (i)
B
#
0
and
t+
#
0;
(ii)
B
#
0
and
t+
=
0;
and (iii)
B
=
0.
In this section, we shall consider only the first case, namely, case (i).
For simplicity, we assume
B
>
0.
The analysis for the case
6’
<
0
is very
similar; for an important example with
8
=
-1,
the interested reader is
referred to
[19].
In case (i), we choose
so
that
a;t+
+
pi
=
0.
(4.7)
Also, in
(4.5),
we choose
6
=
v-4
and
,$
=
b-2<(t),
where
<(t)
is an
increasing function with
<(t+)
=
0.
Substituting
(4.5)
into
(1.4),
we find
that
xo
satisfies the Airy equation
x”(0
=
O3tX(S),
(4.8)
where
O3
=
ab/B2t:C”(t+),
and that each
xs,
s
=
1,2,.
. .
,
is a solution
of an inhomogeneous Airy equation. (For details, see
[18,
Chap.31.) This
suggests that instead of
(4.5),
we might
as
well try the more accurate formal
series solution

259
which we have encountered in the differential equation theory
[15,
p.4091
and the integral approach
[22,
p.3701. It turns out that this form of solution
is not sufficiently general, unless
a;
=
p;
=
0.
(4.10)
It is interesting to note that this condition holds in most of the classical
cases. In fact, in
[ll]
Dingle and Morgan have assumed that
ag,+l
=
,&+I
=
0
for
s
=
0,1,2,...
.
For a more general form of the solution,
we refer the reader to
[20].
To show that (4.9) is indeed an asymptotic
expansion, we need first to determine the function
('(t)
in the argument of
the Airy function.
To
do this, we first substitute
(4.9)
in (1.4), then match
the coefficients of Ai and Ai', and finally let
u
--f
03.
This leads us to
2
-
[<(t)]
p
=
a;
tl'@
3 (4.11)
ds
a$
+
p:,
+
J(abt
+
-
4
-
log
9
A
if
t
2
t+,
and
2
-1
a:,t+p:,
2
$-<(t)]?
=cos
s-v
(4.12)
-
a:,
tlP
I"+
ds
J4
-
(abs
+
pp
if
t
<
t+.
To make the presentation simpler, we have assumed in
[20]
that
t-
<
0
<
t+.
This assumption is equivalent to the condition
lpol
<
2.
The second solution, independent of (4.9), is given by
The coefficients
A,(<)
and
Els(<)
are determined successively
from
some
recursive formulas, beginning with
Ao((')
=
1
and
('~Bo((')
=
0.
5.
Bessel-type
Expansion
We now consider case (iii), i.e.,
6
=
0
in (4.1). As in Sec. 4, we let
TO
be a constant and define
N
:=
n
+
TO.
The characteristic equation
(4.2)
is obtained in the same manner, except that
t
is replaced
by
z.
The
260
characteristic roots again coincide when
x
=
xf,
where
aoxi
+
Po
=
f2.
For
x
near
x+,
we try a formal series solution of the form
where
[
depends on
x
and
N.
In the present case, we choose
<
=
Nc1j2(x),
where
c(x)
is an increasing function with
c(x+)
=
0.
Substituting
(5.1)
into
(1.4),
one finds that
xo(f)
satisfies the Bessel equation
)
xo.
F=(W
E2
d2Xo
..b
+
a$+
+Pb
Thus, it follows that
xo(<)
can be expressed in terms of either the Bessel
functions
Jv(<)
and
Yv(f),
or the modified Bessel functions
Iv(E)
and
Ky(E).
That
is,
there are constants
C1
and
C2
such that
Xo(C)
=
C,E1/2Jv(E)
+
c2
E1’2yv(E)
if
ah
<O
and
xo(E)
=
clE1/2L(E)
+
c2
E1/2Kv(<)
if
ah
>
0,
where
and we take the square root with nonnegative real part. Moreover, each
of the subsequent coefficient functions
xs(<),
s
=
1,2,.
. .
,
in
(5.1)
satisfies
an inhomogeneous Bessel equation. This suggests that instead of
(5.1),
we
might as well try the formal series solution of the form
motivated from the difference equation theory
[15,
p.4411.
In
(5.3),
Zv(<)
can be any solution of the modified Bessel equation
yff
+
-yf
-
1
+
-
y
=
0.
X
(
1:)
(5.4)
The function
<(x)
in
(5.3)
can be determined by subtituting
(5.3)
in
(1.4),
matching the coefficients of
2,
and
Zv+l,
and letting
n
--t
m.
The result
is
(5.5)
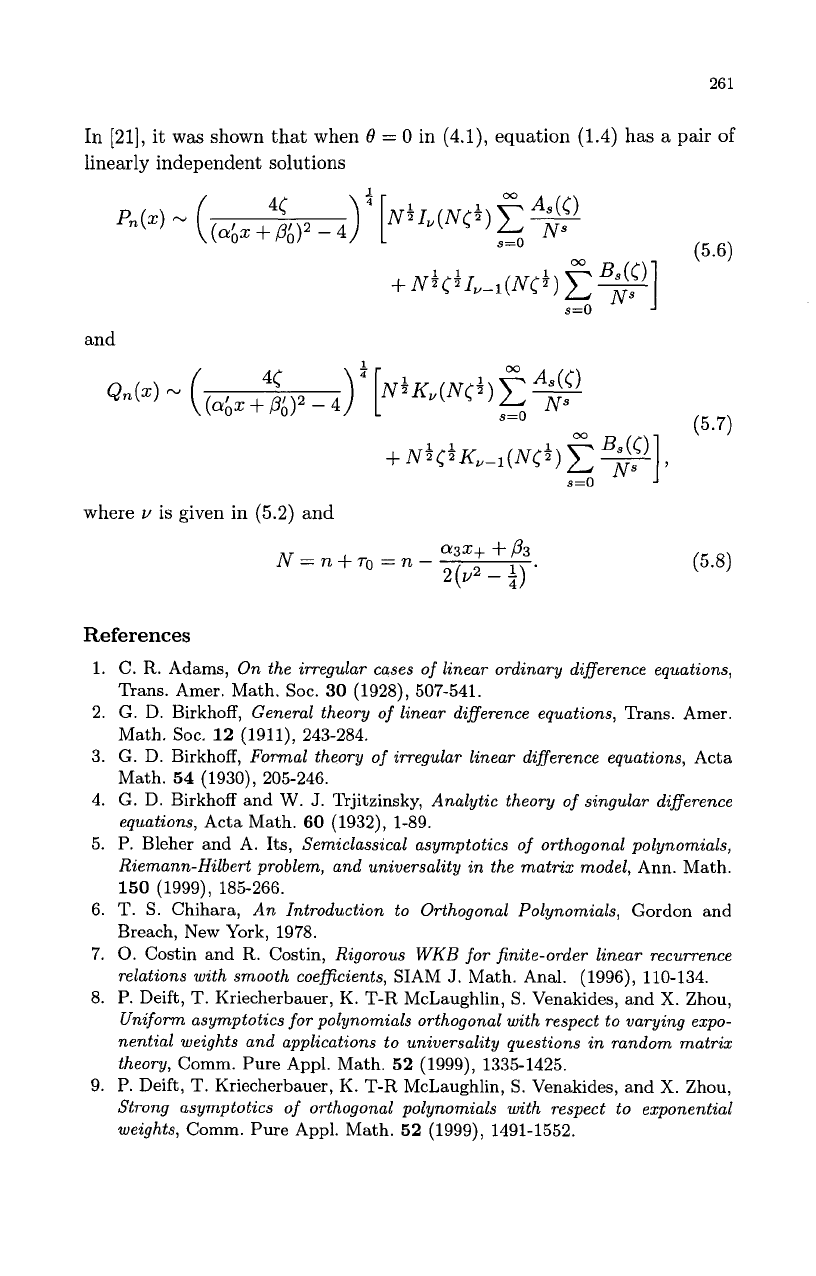
261
In
[21],
it
was
shown
that
when
0
=
0
in
(4.1),
equation
(1.4)
has
a
pair
of
linearly independent solutions
and
where
u
is given in
(5.2)
and
a32+
+
P3
N
=
n
+
70
=
n
-
2(v2
-
i)
.
References
1.
C. R. Adams,
On the irregular cases
of
linear ordinary difference equations,
Trans. Amer. Math. SOC.
30
(1928), 507-541.
2.
G.
D.
Birkhoff,
General theory
of
linear difference equations,
Trans. Amer.
Math. SOC.
12
(1911), 243-284.
3.
G.
D. Birkhoff,
Formal theory
of
irregular linear difference equations,
Acta
Math.
54
(1930), 205-246.
4.
G.
D.
Birkhoff and
W.
J.
Trjitzinsky,
Analytic theory
of
singular digerence
equations,
Acta Math.
60
(1932), 1-89.
5.
P.
Bleher and A. Its,
Semiclassical asymptotics
of
orthogonal polynomials,
Riemann-Hilbert problem, and universality
in
the matrix model,
Ann. Math.
150
(1999), 185-266.
6.
T.
S.
Chihara,
An
Introduction to Orthogonal Polynomials,
Gordon and
Breach, New
York, 1978.
7.
0.
Costin and R. Costin,
Rigorous
WKB
for
finite-order linear recurrence
relations with smooth coeficients,
SIAM
J.
Math. Anal.
(1996), 110-134.
8.
P.
Deift,
T.
Kriecherbauer, K. T-R McLaughlin,
S.
Venakides, and
X.
Zhou,
Uniform asymptotics
for
polynomials orthogonal with respect to varying expo-
nential weights and applications to universality questions
in
random matrix
theory,
Comm. Pure Appl. Math.
52
(1999), 1335-1425.
9.
P.
Deift,
T.
Kriecherbauer, K. T-R McLaughlin,
S.
Venakides, and
X.
Zhou,
Strong asymptotics
of
orthogonal polynomials with respect to exponential
weights,
Comm. Pure Appl. Math.
52
(1999), 1491-1552.
