Hua C., Wong R. Differential Equations and Asymptotic Theory in Mathematical Physics
Подождите немного. Документ загружается.


2
and define the Hankel determinants
It
is clear from
(1.1)
that the quadratic form
xIk=O&+kXj?i?k
is
sw
IC:zjzj12dp(z),
hence is positive definite. Thus
D,
>
0
for all
n,n=O,l,
....
Theorem
1.1.
The
polynomials
pn(x)
have the
form
Po
P1
...
Pn
PI
PZ
...
Pn+1
..
..
..
Pn-1
Pn
. . .
PZn-1
1
2
...
Zn
Proof.
Denote the right-hand side of
(1.3)
by
qn(x).
Thus
qn(z)
=
/%
zn
+
lower order terms,
whence the leading coefficients in
qn(z)
is positive,
For
k
<
n,
..
..
..
which is zero.
If
k
=
n
then the right-hand side of the above equation is
Dn/dG
=
d=.
Therefore
When
p
is absolutely continuous, we write
~'(z)
=
w(z)
and
w
is called
a
weight function.
3
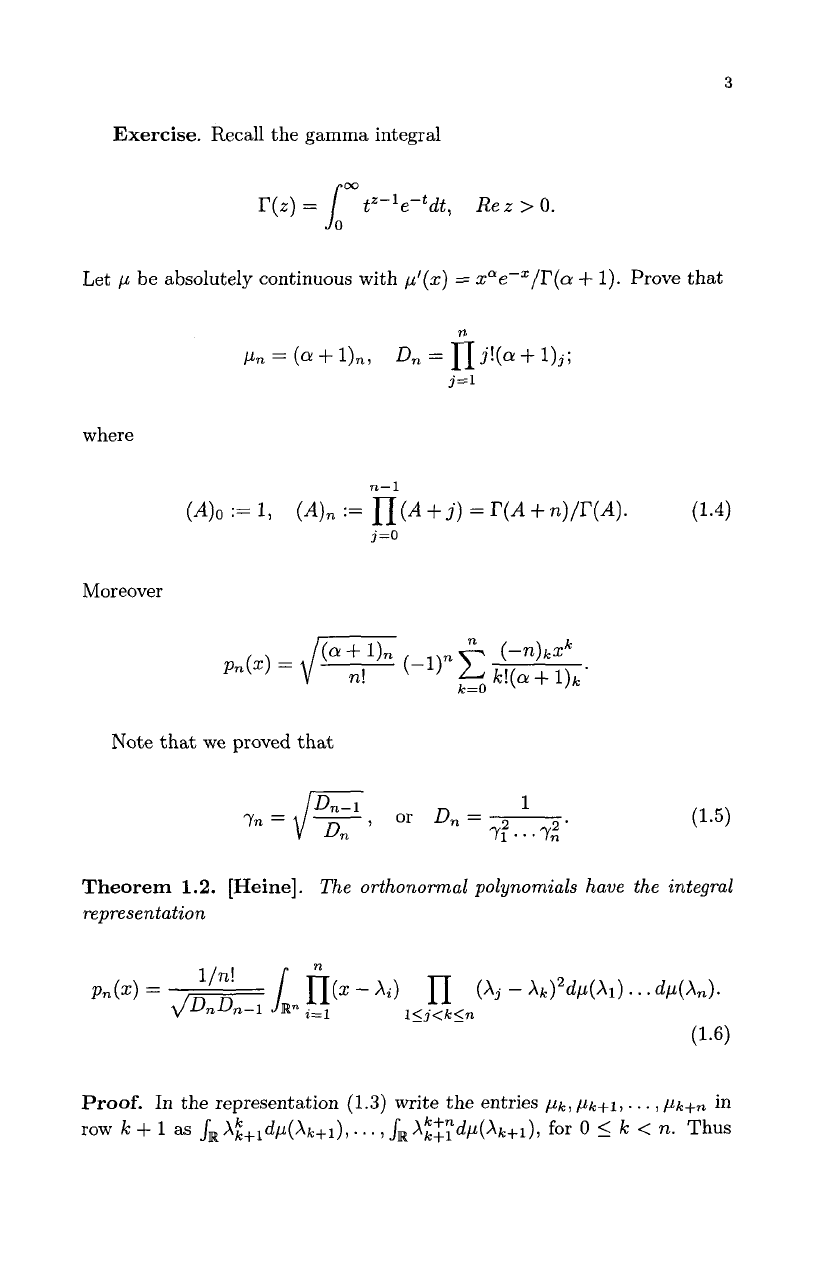
Exercise.
Recall the gamma integral
Let
p
be absolutely continuous with
p'(x)
=
x"e-"/r(a
+
1).
Prove that
where
Moreover
Note that we proved that
Theorem
1.2.
[Heine].
The orthonormal polynomials have the integral
representation
Proof.
In
the representation
(1.3)
write the entries
pk, pk+l,.
. .
,
pk+n
in
row
k
+
1
as
J,
Xk+,dp(Xk+l),
.
. .
J,
X~~~dp(Xk+l),
for
0
I
k
<
n.
Thus

4
dGp,(3:)
has the representation
1
1
3:
...
2"
Recall the evaluation of the Vandermonde determinant
Xi).
Let
a
be a permutation on
1,.
. .
,
n
and
S,
denote the symmetric group.
In the above integral we can replace
XI,.
. .
,A,
by
Xo(l),
.
.
.
,
Xo(,)
then
rearrange the rows in the determinant to have
XI,.
. .
,A,
in rows
1,2,.
. . ,
n.
This produces a multiplicative factor sign
(a),
sign (a) being the sign of
the permutation
a.
By summing over
a
E
S,
and dividing by the order
of
the symmetric group
(=
n!)
we see that
dzp,(3:)
is
Corollary
1.3.
The Hankel determinants have the representation
5
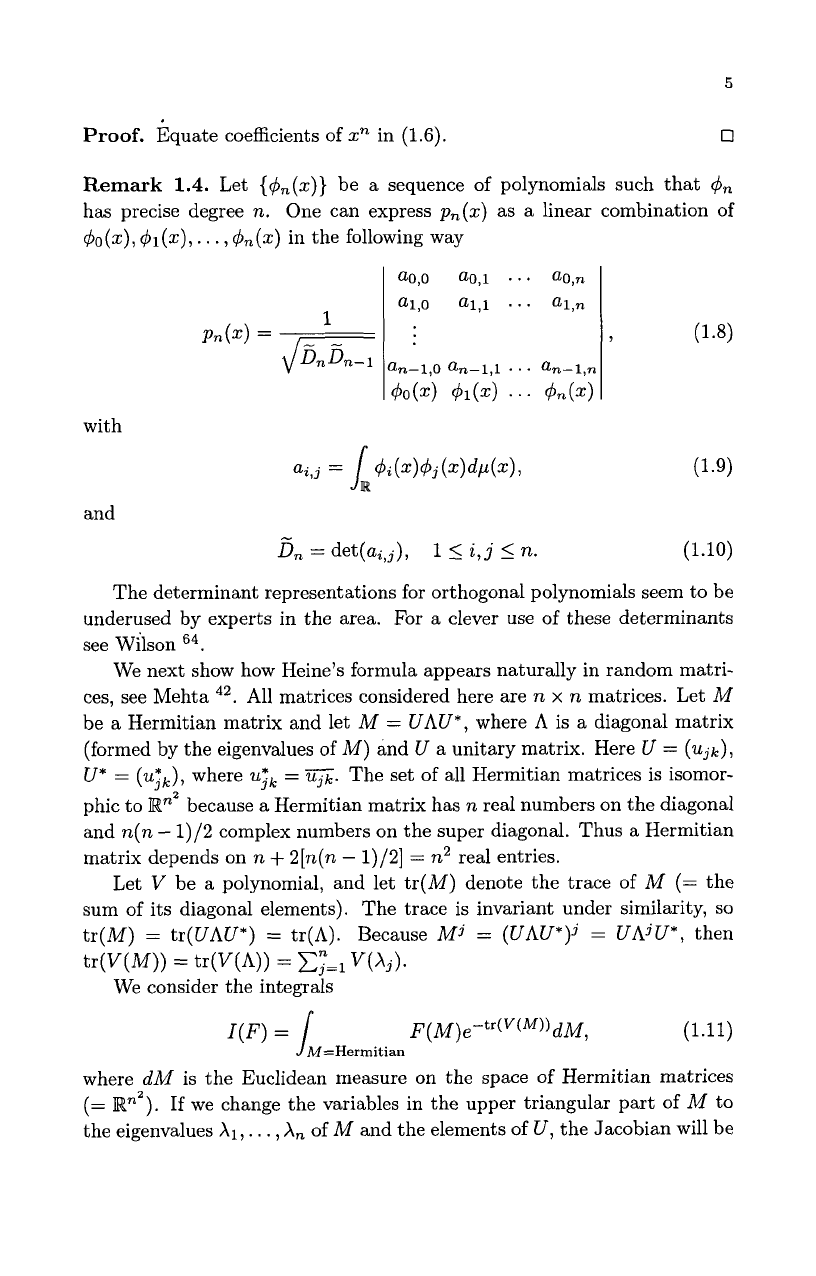
Proof.
Equate coefficients of
xn
in
(1.6).
0
Remark
1.4.
Let
{$,(x)}
be a sequence of polynomials such that
4,
has precise degree
n.
One can express
pn(z)
as a linear combination
of
40
(x)
,
41
(
X)
,
. . .
,
4n
(x)
in the following way
with
and
-
D,
=
det(ai,j),
1
5
i,j
5
n.
(1.10)
The determinant representations for orthogonal polynomials seem to be
underused by experts in the area. For a clever use of these determinants
see Wilson
64.
We next show how Heine’s formula appears naturally in random matri-
ces, see Mehta
42.
All matrices considered here are
n
x
n
matrices. Let
M
be a Hermitian matrix and let
M
=
UAU*,
where
A
is a diagonal matrix
(formed by the eigenvalues of
M)
and
U
a
unitary matrix. Here
U
=
(ujk),
U*
=
(u;~),
where
2~;~
=
ujk.
The set of all Hermitian matrices
is
isomor-
phic to
RnZ
because
a
Hermitian matrix has
n
real numbers on the diagonal
and
n(n
-
1)/2
complex numbers on the super diagonal. Thus a Hermitian
matrix depends on
n
+
2[n(n
-
1)/2]
=
n2
real entries.
Let V be a polynomial, and let tr(M) denote the trace of
M
(=
the
sum of its diagonal elements). The trace is invariant under similarity,
so
tr(M)
=
tr(UAU*)
=
tr(A). Because
Mj
=
(UAU*)j
=
UAjU*,
then
tr(V(M))
=
tr(V(h))
=
Cj”=,
V(Aj).
We consider the integrals
F
(
M)e-t‘(V(M))
dM,
(1.11)
J
MZHermitian
I(F)
=
where
dM
is the Euclidean measure on the space of Hermitian matrices
(=
Itnz).
If
we change the variables in the upper triangular part of
M
to
the eigenvalues
XI,.
. .
,
A,
of
M
and the elements of
U,
the Jacobian will be

6
nlli<jln(Ai
-
Aj)2,
see Deift
l7
for this calculation. We now assume that
F(M)
depends only on the eigenvalues
of
A4
and is a symmetric function
of the eigenvalues. Perform integration on the
U
parameters to obtain
n
I(F)
=
Constant
F(x~,
. . .
,A,>
JJ,
-
Xk)'
n
e-v(x3)d~s.
l<j<k<n
s=l
(1.12)
Ln
Some details are omitted in the last step regarding the ordering of the
Xj's
but the details are in Deift
17.
The representation (1.12) reminds us
of Heine's formula (1.6). Indeed (1.6) gives the evaluation of the integral
in (1.12) when
F
is an elementary symmetric function of
XI,.
. .
,
A,.
Let
ek(X1,.
. .
,An)
denote the kth elementary symmetric function of
XI,.
. .
,An.
It
is clear that
n
ek(A1,.
.
.A,)
n
(xi
-
A,)'
JJ,
e-v(xs)dAs
=
s,.
l_<i<j<n
s=l
=
(-l)kcoefficient of
zn-k
in
n!Jmpn(z),
where
{p,(z)}
are orthonormal with respect to exp(-V(x)) on
R.
One important problem in this area is to determine the distribution
of
the spacing of eigenvalues as
n
---$
co.
Some of the results on this topic are
in
42
and
17.
2.
Some Properties
of
Orthogonal Polynomials
One fundamental property of orthogonal polynomials is that they satisfy
three term recurrence relations.
To
see this let
It
is clear that the expansion
(2.1)
exists since
{pn(x)}
forms
a
basis for the
space of polynomials. Equating coefficients
of
zn+'
in (2.1) and employing
condition (i) of
1
we see that
yn
=
dn,n+lyn+l, hence
dn,n+l
=
^/n/yn+1
>
0.
Ifj<n-lthen

7
because
pj(x)x
has degree
j
+
1
which is less than
n,
It is also clear that
Thus
d,,,-i
=
d,-l,n
=
3;~-1/7,.
Moreover
d,,,
=
Jwxpi(x)dp(x),
SO
dn,,
E
R.
Thus we proved the following result.
Theorem
2.1.
Let {p,(x)} be orthonormal with respect to
p.
{p,(z)} satisfies the three term recurrence relation
Then
xPn(x)
=
an+l~n+l(x)
+
bn~n(x)
+
anpn-l(x),
an
=
yn-l/yn
=
@ZZ
IDn-1.
(2.2)
(2.3)
with b,
E
R
and
a,
>
0.
Moreover
Another important normalization is the monic form
Pn(X)
=
xn
+
lower order terms.
(2.4)
Thus
In
this normalization the recurrence relation
(2.2)
becomes
.P,(.)
=
P,+I(Z)
+
b,P,(z)
+
.2n(z).
(2.6)
A
consequence
of
(2.2)
is the following theorem, see Chapter
2
in Szeg6
58
Theorem
2.2.
The zeros
of
p,(x) are real and simple and belong to the
smallest interval containing the support
of
p.
Moreover the zeros
of
p,(x)
and p,+l(x) interlace.
One source of information on classical orthogonal polynomials has been
that they satisfy
a
Sturm-Liouville problem. Consider the eigenvalue prob-
lem
for
x
E
(a,
b)
and
y
E
L2(a,
b;
w).
It
is assumed that
w(z)
>
O,p(x)
>
0
on
(a,
b),w
and
p
are continuous on
(a,b)
with
w(a+)
=
w(b-)
=
0.
Further
we assume. that
J,
IQ,(x)lw(x) dx
<
00.
The eigenvalues of this symmetric
eigenvalue problem are all real.
If
A1
#
A2
then
b
(2.8)

8
The Jacobi polynomials
{Pp’P’(z)}
satisfy
=
n(n
+
a
+
p
+
l)PpP’(z),
(2.9)
where
we,p(z)
=
(1
-
zy(l
+
zy,
z
E
[-1,1].
(2.10)
The ultraspherical polynomials correspond to the case
a
=
p.
The Legendre
polynomials occur when
a
=
,B
=
0,
while the Chebyshev polynomials
of
the first and second kinds correspond to
a
=
p
=
~i,
respectively. The
let
a
+
00.
The Hermite polynomials arise when
a
=
/3
and
z
is replaced
by
z/a
and we let
a
-+
co.
As an example of a classical polynomial we derive some
of
the properties
of Laguerre polynomials. We require the use of the Chu-Vandermonde sum,
Andrews, Askey, Roy
Laguerre polynomials
{L,
(0)
(z)}
arise when
3:
is scaled
as
-1+2z/a
then we
The weight function is
w,(z)
=
zae-z/I’(a
+
l),
z
>
0.
Let the polynomials be
Lp)
(x)
,
(2.11)
(2.12)
(2.13)
where
{c,,k}
are coefficients to be determined. The factor
(-n)k
makes the
sum terminate at
k
=
n,
since
(-n),
=
0,
m
>
n.
(2.14)

9
We first evaluate
Jr
xmL?)(x)
e-"x"dx
and demand that it vanishes for
m
<
n.
Clearly
To
evaluate the above sum we take
C,,k
=
C,/(A)k
in order to apply
(2.11).
Thus we want, for
m
<
n,
n
(-n)k(a
+
m
+
1)k
-
(A
-
m
-
a
-
1)n
0
=.c
-
k=O k!(A)k
From
(2.14),
it follows that
A
=
a
+
1
will work. Thus
(2.15)
where
c,
was chosen
a.s
(a
f
l)n/n!.
This normalization has been adopted
for over
a
hundred years and
{L?)(x)}
are neither orthonormal nor monic.
Observe that the above calculations establish
=
(-iyr(a
+
n
+
1).
To find
Jr(L?)(x))2x"e-"dx,
we proceed
as
follows, where use the above
integral evaluation.
lw
x"e-"(L?)(x))2 dx

10
Therefore
(2.16)
It
is important to note that in the above derivation the only integral
evaluation used is the gamma function integral. When we expressed
L?)(x)
as
a linear combination of
xk
we used the same integral that evaluated the
total mass of the measure, namely
xLYecxdx,
with
(Y
replaced by
a+a+k.
In other words
xnfk
attaches nicely to
xae-x.
This attachment procedure
has been very useful in constructing closed form expressions for orthogonal
polynomials, see Andrews and Askey
'
and Berg and Ismail
lo.
To find the three term recurrence relation satisfied by
Lp)(x)
we first
observe that the coefficient of
xn
in
Lp)(x)
is
(-l)n/n!.
Hence
xLp)(x)
+
(n
+
l)L?:)(x)
is a polynomial of degree at most
n.
Let
-rcLp)(x)
=
(n
+
l)L?Jl
+
BnL?)(x)
+
C,L?2'(2).
By equating coefficients of
xn
and
xn-'
on both sides
of
the above equation
and applying
(2.15)
we establish the recursion relation
-xLp) (x)
=
(n
+
l)L?il
(x)
-
(n
+
a
+
1)Lp) (x)
+
(n
+
a)Lfl
(x)
.
(2.17)
In
3
3
we shall establish the differential equation
(2.18)
d d
dx dx
-.--pyx)
+
(a
+
1
-
x)-Lp)(x)
+
nLp)(x)
=
0.
The Christoffel-Darboux formula
3.
Differential Equations
In this section we derive differential equations and raising and lowering
operators for general orthogonal polynomials when
dp
=
w(x)dx,
and
W(X)
=
e-"("),
Theorem
3.1.
Assume
w'
is
continuous
on
(a,
b)
x
E
(a,
b).
(3.1)
and

11
exists for nonnegative integers
n.
Define
An(x), Bn(x)
by
(3.3)
and
assume that the boundary
terms
in
(3.2)-(3.3)
exist. Then
d
-~n(x) dx
=
An(x)pn-l(x)
-
Bn(x)pn(x).
(3.4)
Theorem
3.1
follows from the Christoffel-Darboux identity
(2.18)
and
Theorem
3.1
is due to Bauldry
’,
Bonan and Clark
11,
Mhaskar
43,
and
Define operators
L1,n
and
Ls,~
by
integration by parts. The details are in
15.
Chen and Ismail
15.
d d
dx
L1,n
=
-
+
Bn(x),
L2,n
=
-z
+
Bn(x)
+
~‘(x).
(3.5)
Thus we write
(3.4)
as
L1,npn (x)
=
An (x)pn-
1
(x),
(3.6)
hence
L1,n
is
a
lowering or annihilation operator for
{pn(x)}.
By eliminat-
ing
pn-l(x)
between
(3.4)
and
(2.2)
we establish
Thus
L2,,
is a raising or creation operator.
adjoints with respect to the inner product
In fact
LI,~
and
Ls,~
are
b-
(f,
9)
=
/
f(x)g(x)w(Wx,
see Chen and Ismail
15.
Combining
(3.6)
and
(3.7)
we arrive at
which is a second order differential equation satisfied by the polynomials.
One important application
of
the differential equation
(3.8)
is that one
can apply the Liouville-Green
(or
WKB)
approximation and derive the large
