Hua C., Wong R. Differential Equations and Asymptotic Theory in Mathematical Physics
Подождите немного. Документ загружается.

282
The spectrum of
(D)
is discrete and consists of
a
sequence
(pj)zl
and can
be written in non-decreasing order according to their finite multiplicity:
0
p1
<
p2
I
...
<
pj
<
-
...
with
pj
--f
+00
as
j
-+
+00.
Here we denote
vj
E
Hi(fl)
as
unit eigenfunctions associated with
pj.
Now, we can state our main results
as
follows:
Theorem
1.1.
The following formula
is true, where
(.,
.)
denotes the inner product
in
L2(R).
Theorem
1.2.
There exists
R
4
+00
such that
where
A(R)
=
#{k
L
1;pk
<
R}.
Remark
1.1.
The results can be extended to the case of that the domain
Q
c
Rk
is a fractal drum, i.e.,
R
is an opened subset with fractal boundary
aR.
In
this
case, if
6
E
(k-1,
k)
is the Minkowski dimension
of
the boundary
80,
then we know that
A(R)
=
(21r)-~ukIRlkR~/~
+
O(Rs/')
as
R
-+
00.
2.
Preparation
For
our purpose, we shall first discuss the problem on a single domain
Rj,
i.e.,
Lu
=
-Au
+
q(x)u
=
Xu,
in
Rj,
u
=
0,
on
dRj,
(Pj
)
and
Luo
E
-
Au
=
Xu,
u
=
0,
on
dOj,
in
Rj
,
(Q)
Without loss of generality, we assume that
q(z)
is nonnegative, other-
wise, instead of
q(x),
we shall use
q(x)
+
E,
where
E
>
0
and
1q(x)1
<
E.
Suppose that
Qj
is
the upper bound
of
q(x)
in
Rj
and
Q
the upper bound
of
q(x)
in
0.
So,
0
5
Qj
5
Q.
283
Lo
and
L
are unbounded self-adjoint operator in the real Hilbert space
Hd(0j).
L
=
Lo
+
q
is nonnegative since
Lo
is nonnegative. We write the
eigenvalues of
LO
and
L
in
Qj
as
0
<
py’
5
p!$
5
...
and
0
<
A?)
I
A!$
I
...
respectively.
v;)
and
21.2’
are unit eigenfunctions associated
with
p$)
and
A:)
respectively, and
{v;’}
and
(21.2’)
are both complete
orthonormal systems in
Hi(0j).
We put all
p;)
and
A;)
together, and
rearrange them, which are denoted by
{a;)},
in non-decreasing order. For
any positive number
R,
let
Thus we have
Lemma
2.1.
For
any
positive number
R,
we have
1)
0
I
pp)
5
A:)
5
p2)
+
Qj;
2)
Aj(R
-
Qj)
5
Bj(R)
I
Aj(R);
3)
Cj(R)
=
Aj(R)
+
Bj(R);
2Aj(R
-
Qj)
5
Cj(R)
I
2Aj(R).
Secondly, we have
for
{fn}
being orthogonal unit vectors,
N N
N N
m=l
n=l
m=l
n=l
Thus
Lemma
2.2.
For any positive number
R,
where
284
and
By
Lemma
2.1,
A$)
-
p;)
2
0,
Bj(R)
5
Aj(R),
hence
Lemma
2.3.
Let
S,
R
be any two real numbers satisfying
0
<
S
<
R,
then
Proof.
Notice that
{ug)}
and
{&I}
are both complete orthonormal
sys-
285
tems in
HA(Oj),
we deduce from Lemma
2.2
and the Parseval’s formula
m
As
the same way, we have

286
Finally,
0
5
Fj(R)
5
111
+
1111,
Lemma
2.3
follows.
0
The following two lemmas are the restrictions uniformly on the increas-
ing rate of
Aj(R).
Lemma
2.4.
For arbitrary
E
>
0,
there exists suficiently small
6
>
0
so
that we have forj
Aj(R)
-
Aj(R
-
SR)
lim
-
<
E.
R++CC
4
(R)
Proof.
Since
Aj(R)
=
(2n)-‘~kIRjlkR~/~
+
o(Rkl2),
as
R
-+
+m,
where
wk
denotes the volume of the unit ball in
Rk,
then
.
Aj(R)
-
Aj(R
-
6R)
=I-
(2~)-~~k(Rjlk(R
-
6R)k/2
+
0
((R
-
SR)”’)
4
(R) (~T)-~w~IR~I~R~/’
+
0(Rk/’)
N
1
-
(1
-
S)”’,
as
R
+
+m.
Thus, for any
E
>
0
take
0
<
b
<
1
-
(1
-
E)~/~,
Lemma
2.4
holds.
0
Lemma
2.5.
For the operator
Lo,
we have uniformly estimate on j:
3.
Proof of
the
Main Results
In section
2,
we consider the asymptotic behaviour of the trace for the
operators
L
and
LO
in a single domain
Rj.
Next, we shall consider the same
problem in the case of
R
=
u
Rj.
First, we confirm that the eigenvalues
of
(P)
have the following relation with the eigenvalues of
(Pj)jOo_,.
Lemma
3.1.
Suppose that
A;)
is an eigenvalue
of
(Pj),
then
A;)
is cer-
tainly the eigenvalue
of
(P);
on the other hand,
for
an eigenvalue
A,
of
(P),
there exists
j
such that
A,
is the eigenvalue
of
(Pj).
03
j=1
Also we can prove that the eigenvalues between problem
(D)
and
(Dj)gl
have the same relation. Thus to consider the eigenvalue prob-
lem
(P)
and
(D)
would be equivalent to consider the eigenvalues
(Pj)El
ww
and
(D3)g1
together. Now, we rearrange the all eigenvalues
u u
{p“}
?=1
m=l
of
(D)
which are rewritten by
{p,}
in non-decreasing order. Denote that
287
vn
are the unit eigenfunctions associated with
pn.
For any
pn,
there exist
j
and
m
such that
pn
=
p:).
Corresponding to
p,,
we rewrite
A%)
by
A,.
Then by Lemma
3.1,
u
{A,}
is
the
whole eigenvalues
U
U
{A:)}
.
Perhaps,
A,
are not arranged in non-decreasing order, but
An
+
+00
as
n
+
00.
Denote
A(R)
=
#{nlpn
5
R},
then
A(R)
=
C
Aj(R),
and the
sum is finite
for
and fixed
R.
00
0000
n=
1
j=1
m=l
00
j=1
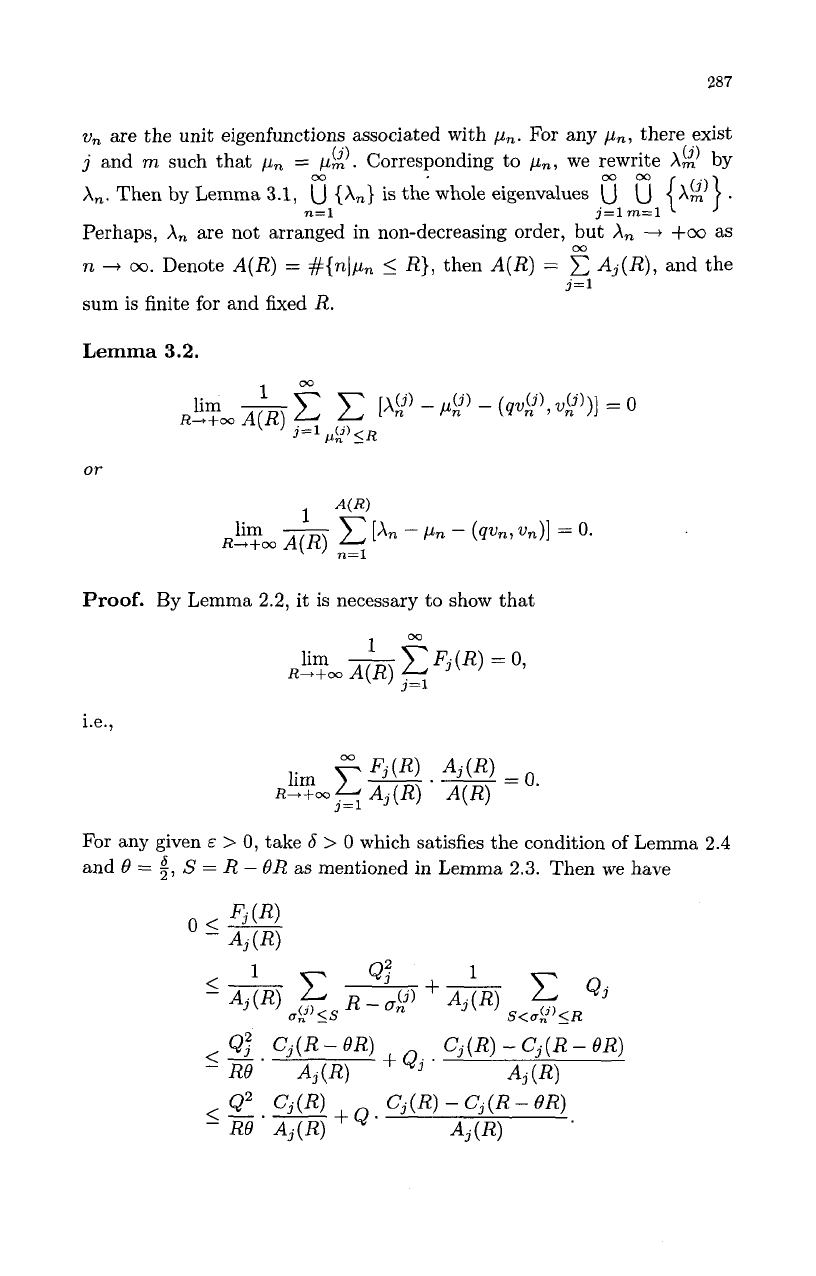
Lemma
3.2.
or
Proof.
By Lemma
2.2,
it is necessary to show that
i.e.,
For any given
E
>
0,
take
S
>
0
which satisfies the condition
of
Lemma
2.4
and
O
=
i,
S
=
R
-
OR
as
mentioned in Lemma
2.3.
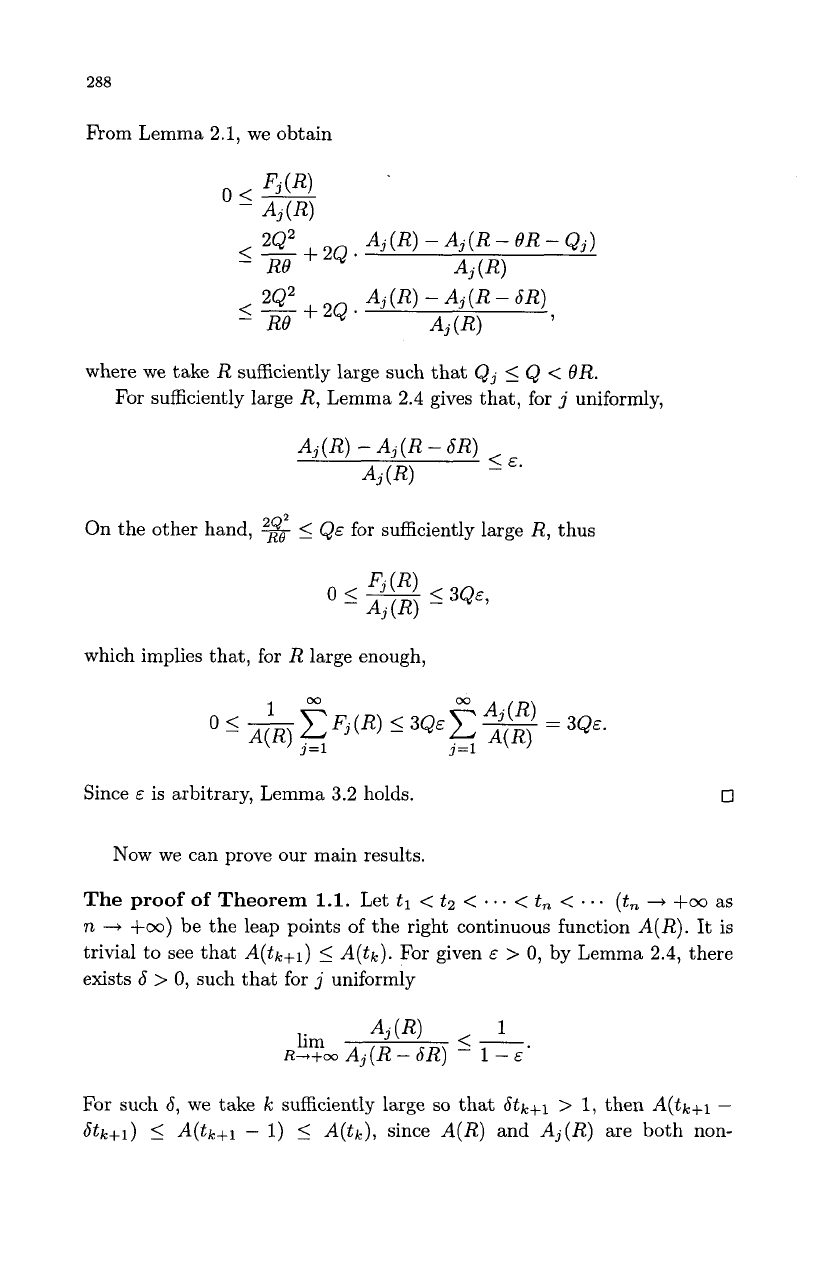
Then we have
Q2
Cj(R-OR) Cj(R)
-
Cj(R
-
OR)
RO
Aj(R)
Q2
cj(R)
+
Q.
Cj(R)
-
Cj(R
-
OR)
4
(R)
52.
+
Qj
.
I--.-
Re
Aj(R) A, (R)
288
&om Lemma
2.1,
we obtain
2Q2
Aj(R)
-
Aj(R
-
OR
-
Qj)
4
(R)
5-+2Q*
RB
where we take
R
sufficiently large such that
Qj
5
Q
<
9R.
For
sufficiently large
R,
Lemma
2.4
gives that, for
j
uniformly,
Aj(R)
-
Aj(R
-
bR)
5
E.
4
(R)
On the other hand,
$
5
QE
for sufficiently large
R,
thus
which implies that, for
R
large enough,
Since
E
is arbitrary, Lemma
3.2
holds.
0
Now we can prove our main results.
The
proof
of Theorem
1.1.
Let
tl
<
t2
<
.
<
t,
<
. . .
(t,
+
+CQ
as
TI
--+
+m)
be the leap points of the right continuous function
A(R).
It
is
trivial to see that
A(tk+l)
5
A(tk).
For
given
E
>
0,
by Lemma
2.4,
there
exists
6
>
0,
such that for
j
uniformly
1
lim
Aj (R)
<-
~-++oo
Aj(R
-
6R)
-
1
-
E'
For such
6,
we take
k
sufficiently large
so
that
6tk+l
>
1,
then
A(tk+l
-
&+I)
5
A(tk+l
-
1)
5
A(tk),
since
A(R)
and
Aj(R)
are both non-
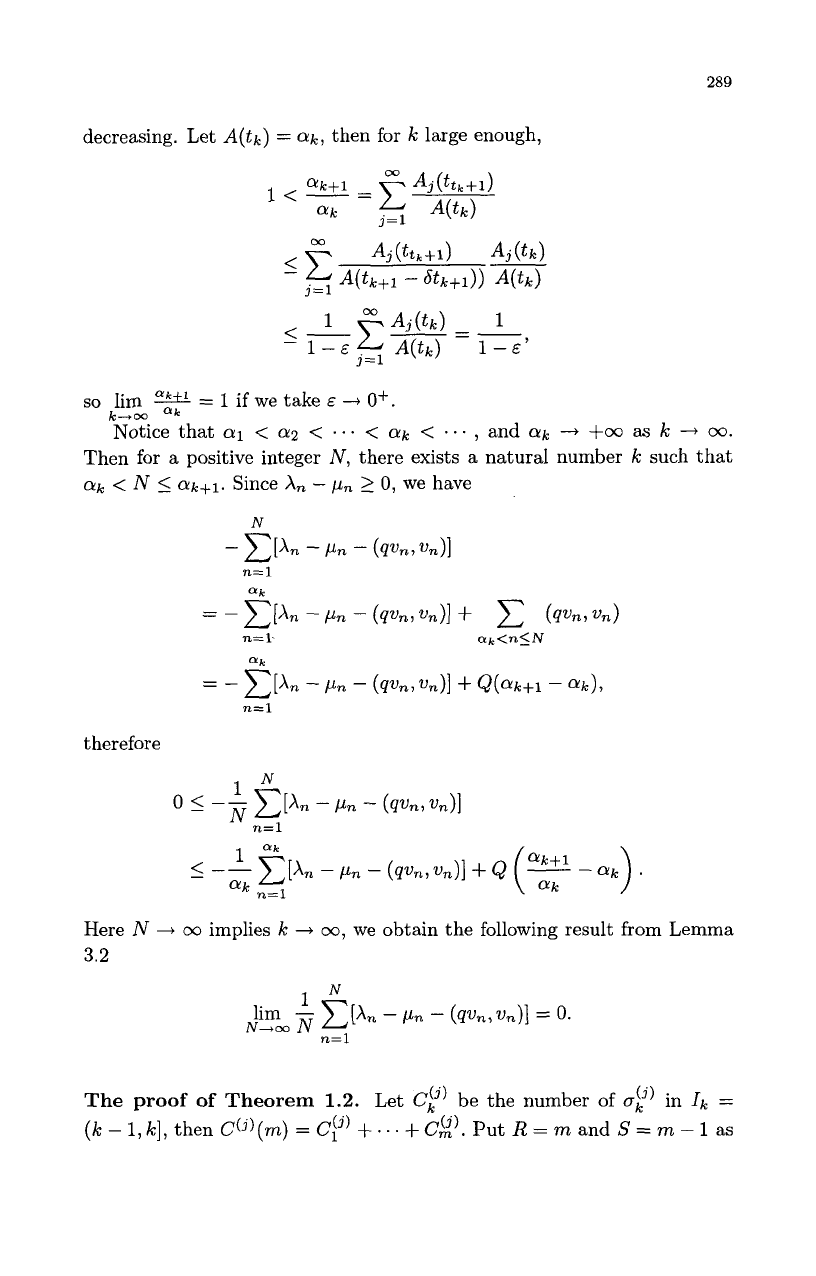
289
decreasing. Let
A(tk)
=
ak,
then for
k
large enough,
so
lirn
=
1
if we take
E
-+
O+.
k-oo
Notice that
a1
<
a2
<
...
<
(Yk
<
...
,
and
ak
-+
+00
as
k
-+
co.
Then for a positive integer
N,
there exists a natural number
k
such that
(Yk
<
N
5
(Yk+l.
Since
An
-
pn
2
0,
we have
therefore
Here
N
+
00
implies
k
+
00,
we obtain the following result from Lemma
3.2
The
proof
of
Theorem
1.2.
Let
Cf)
be the number of
cf)
in
Ik
=
(k
-
1,
k],
then
C(j)(m)
=
Cp)
+.
..
+
Cg).
Put
R
=
m
and
S
=
m
-
1
as
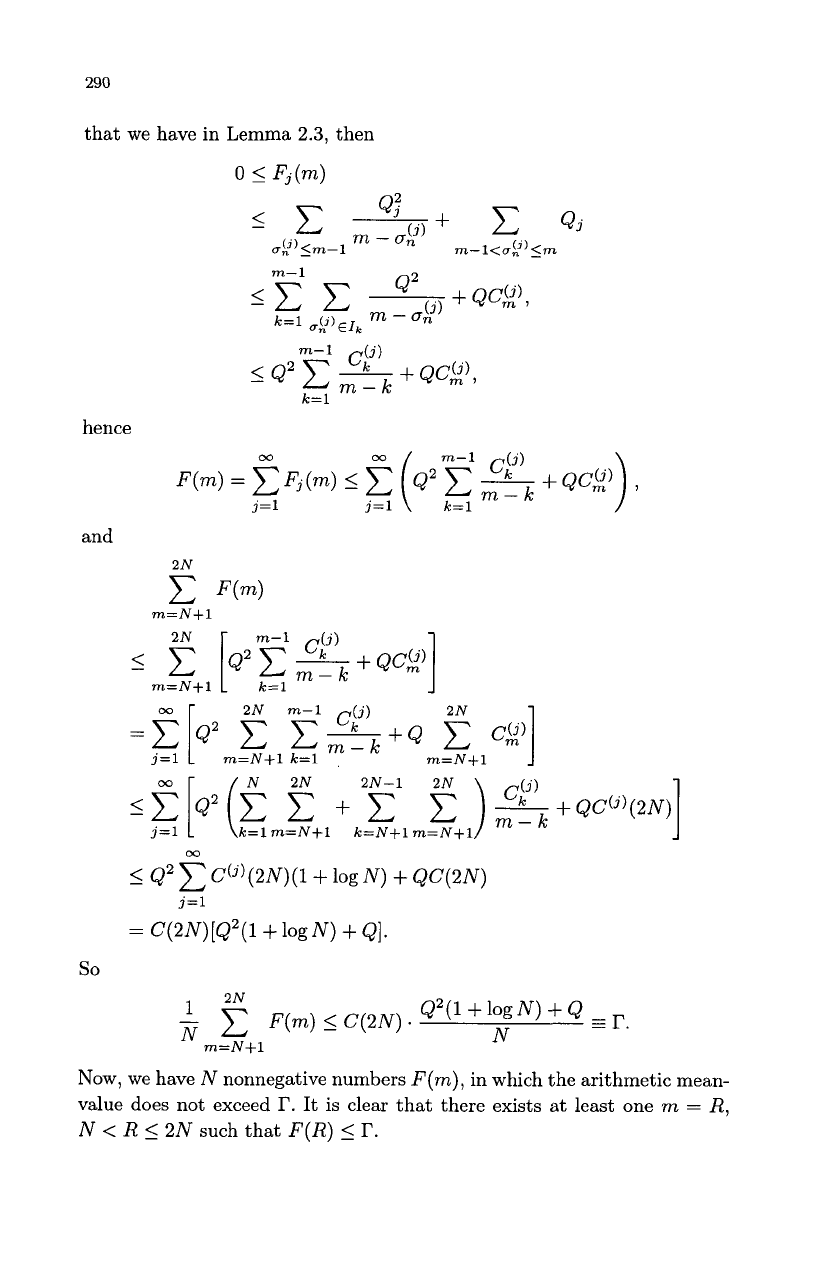
290
that we have
in
Lemma
2.3,
then
hence
and
2N
2N
m=N+l k=l
mr
2N m-1
A;)
2N
1
~~
=
c
[Q2
c
c
m-lc
52
[i?’(X
c
+
c c
)
*+QC(j)(2N)]
m-lc
+Q
c
c~)I
j=1 m=N+l k=l m=N+1
N 2N 2N-1 2N
j=1 k=l m=N+1 k=N+1 m=N+1
00
5
Q2
c
C(j)(2N)(l+
log
N)
+
QC(2N)
j=1
=
C(2N)[Q2(1
+
bgN)
+
Q].
so
E
r.
Q2(1
+
log
N)
-t
Q
N
2N
1
-
c
F(m)lC(2N).
N
m=N+l
Now, we have
N
nonnegative numbers
F(m),
in which the arithmetic mean-
value does not exceed
r.
It is clear that there exists at least one
m
=
R,
N
<
R
5
2N
such that
F(R)
5
r.
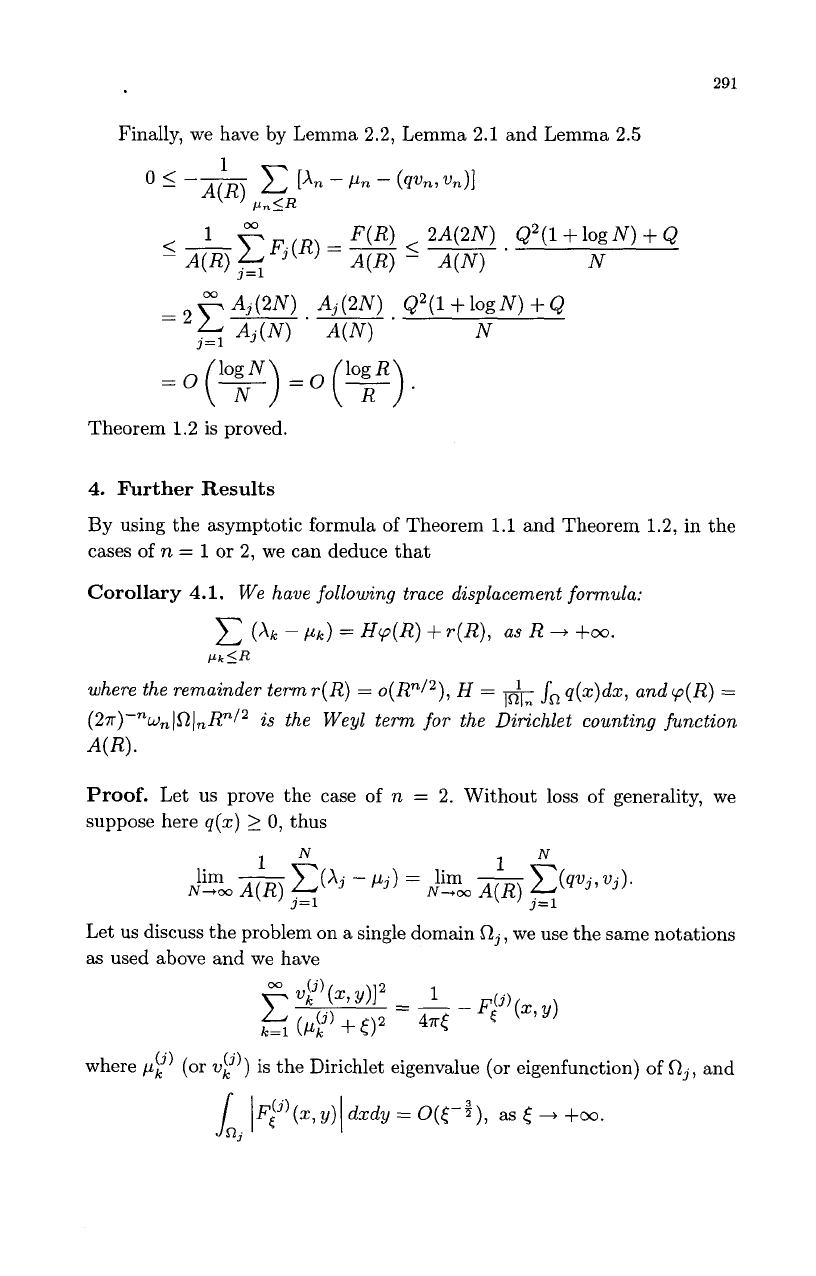
291
Finally, we have by Lemma
2.2,
Lemma
2.1
and Lemma
2.5
loo
F(R)
2A(2N)
Q2(1
+log
N)
+
Q
N
5
-CFj(R)=-
4R)
j=1
A(R)
'
A(N)
'
Theorem
1.2
is
proved.
4.
Further Results
By using the asymptotic formula
of
Theorem
1.1
and Theorem
1.2,
in the
cases
of
n
=
1
or
2,
we can deduce that
Corollary
4.1.
We have following trace displacement formula:
C
(xk
-
pk)
=
Hp(R)
+
r(R),
as
R
4
+a.
Pk<R
where the remainder term
r(R)
=
o(R"/~),
H
=
&
J,
q(x)dx,
and
p(R)
=
(~T)-"W,JS~J,R~/~
is
the Weyl term
for
the Dirichlet counting function
A(W
Proof.
Let us prove the case of
n
=
2.
Without loss
of
generality, we
suppose here
q(X)
2
0,
thus
Let us discuss the problem on a single domain
slj
,
we use the same notations
as
used above and we have
where
pf)
(or
vf))
is
the Dirichlet eigenvalue (or eigenfunction)
of
Rj,
and
dxdy
=
O([-c),
as
5
4
+a.
