Hua C., Wong R. Differential Equations and Asymptotic Theory in Mathematical Physics
Подождите немного. Документ загружается.


292
SO
we get,
as
<
+
+m,
where
Also
we know
and
1
4n
A(R)
=
-1S212R
+
o(R),
as
R
+
+co.
C
(qvf),
vf'),
then
aj(R)
is nonnegative and non-
Let
aj(R)
=
decreasing function, we have
$)_<R
aj(R)
I
QjAj(R)
=
O(R),
as
R
-+
+co.
We notice that
aj(R)
=
0
when
0
<
R
<
p?),
and
aj(pkfl)
(j
-
aj(pf))
=
(qv,+,,
(j)
v~+~).
(j)
Therefore, we have
Let
N
-+
co,
we have
293
From Tauberian Theorem, we have
OD
where
I
=
C
Ij
=
J,
q(z,
y)dzdy,
which implies
j=1
The Corollary
4.1
is proved.
0
Finally, if
R
c
R",
n
=
1
or
2,
is
a
fractal
drum,
and
the Minkowski
dimension of
dR
is
6
E
(n
-
1,
n),
then we have
Corollary
4.2.
For the remainder term
r(R),
we have
r(R)
=
o(R~),
as
R
4
+a.
References
Gelfand I.M., Levitan
B.M.,
On
a
simple identity for the characteristic values
of
a differential operator
of
the second order (Russian) D.A.N.
88(1953), 593-
596.
Cao Cewen, On the asymptotic estimation
of
the trace
of
a partial differential
operator,
Scientia Sinica,
Special Issue
11(
1979), 56-68.
Cao Cewen, On the asymptotic trace
of
the second order self-adjoint elliptic
operator, Proceedings
1980
Beijing symposium Diff. Geo. and
Equ.,
vol
3,
1 1707- 1 1 14.
1.
1.
1.

294
4.
Chen Hua, Sleeman
B.D.,
Fractal drums and n-dimensional modified Weyl-
Berry conjecture, Comm. Math. Phys., 168(1995), 581-607.
5. E.C. Titchmarsh, Eigenfunction expansions associated with second order dif-
ferential equations, Part
11,
Oxford, 1958.

LIMITATIONS AND MODIFICATIONS OF
BLACK-SCHOLES MODEL
*
LISHANG JIANG AND XUEMIN REN
Institute
of
Math., Tongji University, Shanghai, China
In this paper we investigate the limitations of Black-Scholes model and provide
some modifications and some applications in pricing of real options and option-like
contracts also.
Our
modifications are based on the following assumptions: jump-
diffusion process, stochastic interest rate, variable volatility and implied volatility.
1.
Introduction
In this paper, we start by a brief review of Black-Scholes model, discuss its
limitations in detail, and show some modifications.
1.1.
Motivation
of
Black-Scholes model
The call(put) option gives the holder the right to buy(sel1)
a
fixed amount
of an asset
S
at a specified time
T
in future for an agreed price
K.
The
key problem is to find a way to determine the fair price of the option in its
whole life, such that
(ST
-
K)+,
call;
(K
-
ST)+,
put.
V(ST,T)
=
There are two different style options: European option can be exercised
only on maturity while American option can be exercised at any time before
maturity.
1.2.
Foundations
of
Black-Scholes model
To derive Black-Scholes formula, we need following assumptions and argu-
ments
*supported by the national natural science foundation
of
china (no.
10171078)
295

296
0
Asset price
st
is governed by a geometric Brownian motion
where
E(dWt)
=
0,
Var(dWt)
=
dt;
0
Interest rate
T
never changes and is same for borrowing or lending;
0
Asset's volatility
o
is
known and never changes for all maturities;
0
There are no transaction costs and taxes for either asset or option;
0
Unlimited short selling is allowed;
0
The underlying asset can be traded continuously and in infinitesi-
mally small numbers of units;
Upon the assumptions above, for European put option, there is an
unique martingale measure
Q
such that
V,
=
e-T(T-t)EQ((K
-
StlFt)),
(1.2)
From the point of view of PDE, there exists a function
V
=
(S,
t)
which
satisfies
where
q
is the dividend rate
of
St.
From the equation
(1.3),
we can obtain
the famous Black-Scholes formula
:
As
for American put option, we can find
a
function
V
=
V(S,t)
which
satisfies
and
min{-LV,
v
-
(K
-
S)+}
=
0,
{
V(t=T
=
(K
-
S)+,
297
(1.5)
av
02
a2v
aV
at
2
as2
as
LV
=
-
+
-s2-
+
(r
-
4)s-
-
rv.
where
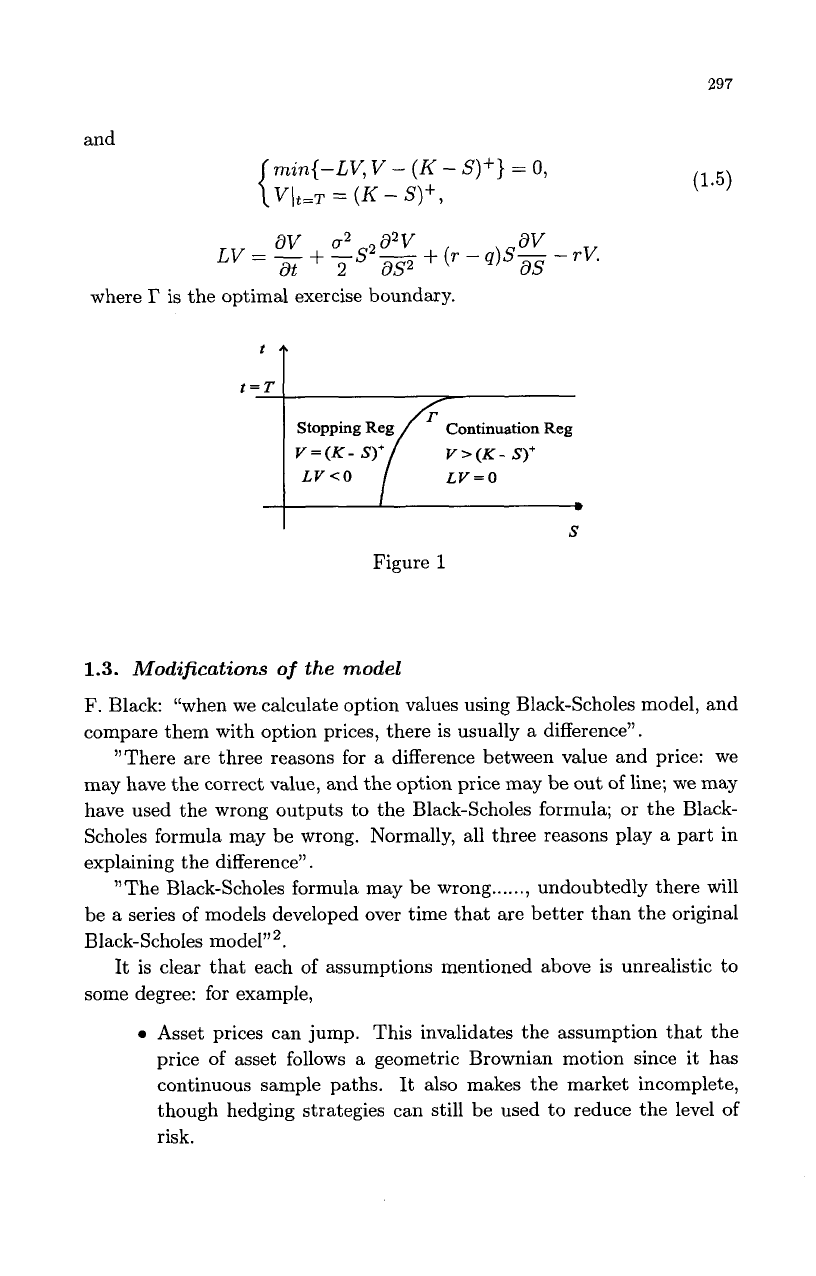
I’
is the optimal exercise boundary.
‘T
t=T
Continuation
Reg
V>(K-
S)’
LV=O
S
Figure
1
1.3.
Modifications
of
the
model
F.
Black: “when we calculate option values using Black-Scholes model, and
compare them with option prices, there is usually a difference”.
”There are three reasons for a difference between value and price: we
may have the correct value, and the option price may be out of line; we may
have used the wrong outputs to the Black-Scholes formula; or the Black-
Scholes formula may be wrong. Normally, all three reasons play a part in
explaining the difference”.
”The Black-Scholes formula may be wrong
......,
undoubtedly there will
be
a
series of models developed over time that are better than the original
Black-Scholes model”
’.
It
is clear that each of assumptions mentioned above is unrealistic to
some degree: for example,
0
Asset prices can jump. This invalidates the assumption that the
price of asset follows a geometric Brownian motion since it has
continuous sample paths. It also makes the market incomplete,
though hedging strategies can still be used to reduce the level of
risk.

298
0
The risk-free interest rate does vary and usually in an unpredictable
way. We can adapt some models to allow for
a
stochastic risk-
free rate, especially when we price the option with relatively long
maturity.
Among the inputs for Black-Scholes formula, only the volatility
CJ
can not be observed directly from the market.
If
we derive it from
Black-Scholes formula using quoted option prices, we will find the
assumption that the volatility is a constant
is
not true. The reason
may be that the distributions of asset returns tend to have fatter
tails than suggested by the log-normal model.
Despite
all
of the potential
flaws
in the model assumptions, analysis of
market derivative prices show that the Black-Scholes model does provide a
quite good approximation to the market and an insight into the usefulness
of dynamic hedging. The fact that the assumptions not hold in practice
does not mean that the model has no use. In our opinion, the best way is
to consider some more realistic assumptions.
In the following sections, we will modify Black-Scholes model under the
assumption of jump diffusion process and stochastic interest rate, and find
a robust method to derive the implied volatility.
2.
Jump-diffusion
process
If
the market gets some good or bad news, the prices of assets can jump.
So
the jump-diffusion process sounds more realistic.
2.1.
Movement
of
asset price
We assume that the asset price follow
a
jump-diffusion process
_-
dSt
-
pdt
+
adWt
+
Udq,
S-
where a Poisson process
dq
is defined by
Prob(a1)
=
1
-
Xdt,
Prob(az)
=
Xdt
,
that is, there are no jump in case
(wl)
,
and
a
jump in case
(az)
with intensity
A.
In case
(wz)
,
the price
will move from
S-
to
JS-(J
>
0),
and
U
=
(J
-
l)S-,
-1
<
U
<
+cc
,
is
independently equal distribution r.v.

299
2.2.
Black-Scholes model with jump-diffusion
It can be showed that under martingale measure, the European put option
price
V(S,
t) satisfies
av
ff2
,a2v
dV
LJDV
=
-
+
-S
-
+
(T
-
-
M)S-
-
rV+
at
2
as2
dS
where
k
=
E(U)
=
E[.]
is the expectation taken over the jump size. For American put
option, it turns into
(2.3)
min{-LJDV, V
-
(K
-
S)+}
=
0,
{
Vjt=T
=
(K
-
S)+.
I'
:
S
=
S(t)
is the optimal exercise boundary.
'T
r
:
s
=
s(t)
S
I
Figure
2
2.3.
Some results
As
we know, there exists a closed form solution for European option. Amin
gives the
C-R-R
Scheme for jumpdiffusion as a discrete model to value the
European option and proves the convergence in weak sense'. Zhang uses
a
FEM
to value American option price and prove the convergence in
H1
sense13. For European option, we prove that there are estimates
IVA(S,t) -V(S,t)I
5
CJat,

300
VA(S,
t)
is given by C-R-R schemes, and
IpA(S,t)
-
V(S,t)l
5
Cat,
pA(S,
t)
is given by modified C-R-R schemes'l. For American put option,
we claim that the optimal exercise boundary
S
=
S(t)
satisfies:
S(t)
E
Cm[O,
T),
S(t)
is a monotonic increasing function on the interval
[O,t)
and
S(T)
=
min{K,
S},
where
s^
is the root of transcendental equation
-rK
+
qg
+
X
llw[(l
+
y)s^
-
K]+dlV(y)
=
0,
andS,
5
S(t)
5
S(T),
where
S,
is the optimal exercise point for perpetual American put
opti011~9?*~.
For American put option, in local uniform sense, we have
lim
lim
At
--t
00
VA(S,
t)
=
V(S,t),At
-
00
SAt
=
S(t),
where
V(S,
t)
is viscosity solution
,
VA(S,
t)
and
SAt
are given by C-R-R
scheme.
3.
Stochastic interest rate
3.1.
The models
of
stochastic interest rate
We assume the interest rate is governed by following stochastic process:
where
j2(rt,
t)
=
77
-
Ort.
It incorporates the mean reverting feature
of
the interest rate.
301
Remark
3.1:
the range of
rt
is
Vasicek model
:
-m
<
rt
<
+m
C-I-R
model:if2q>$,O<rt
<+a
3.2
Black-Scholes model with stochastic interest rate
satisfies
Under
a
martingale measure, European put option price
V
=
V(S,
T,
t)
av
u=
2a2v
aV
Lsrv
=
+
TS
+(q-Or)%
-rv
=
O(0
<
s
<
+m,r
E
D,O
5
t
<
t),
+
pcTsz(r,
t)g
+
4~2(T,
t)g
+
rsm
(3.2)
{
V(S,r,T)
=(K-S)+(OIS<+m,rED),
where
D={
R,
Vasicek;
R+,
C-I-R.
Remark: From Fichera theory for second order partial differential equa-
tions with nonnegative characteristic form, we know that European put
option problem is well posed, if
2q
>.
6j2
in C-I-R model, that is, we should
not impose any boundary condition on
r
=
0.
We introduce
a
zero coupon bond with maturity
T
as a numeraire.
P(r,
t)
denotes the value of this zero coupon bond. Under this relative price
system, there exists a martingale measure
Q
such that
2
=
EQ[gIFt].
P(r,
t)
satisfies
+
;z2(r,t)$$
+
(q--Or)%
-rP=
0
,(r
E
D,O
5
t
<
T)
P(r,T)
=
1,
(r
E
0).
(3.3)
~(r,
t)
=
B(t)e-A(t)T, (3.4)
It has affine structure solution
