Hua C., Wong R. Differential Equations and Asymptotic Theory in Mathematical Physics
Подождите немного. Документ загружается.


222
Exponential asymptotics of other well known entire functions can be
investigated in a similar manner. However, the analysis could be consider-
ably more complicated.
For
instance, in
[14]
and
[15],
Wong and Zhao have
studied the Berry transition of the generalized Bessel function
where
-1
<
p
<
ca and
p
is a real
or
complex number.
They have also
discussed the exponential asymptotics of the Mittag-Leffler function
[16]
Re
Q!
>
0,
where
p
may again be real
or
complex. These two entire functions have
played an important role in the study of fractional differential equations
[lo];
see also
[Ill.
References
1.
E.
Artin,
The Gamma Function,
Holt, Reinehart and Winston, New
York,
1964.
2.
M.
V.
Berry,
Uniform asymptotic smoothing of Stokes’ discontinuities,
Proc.
Roy. SOC. Lond. A,
422
(1989), 7-21.
3.
M.
V.
Berry,
Asymptotics, superasymptotics, hyperasymptotics,
in
Asymp-
totics Beyond All Orders, H. Segur,
S.
Tanveer, and
H.
Levine (eds), Plenum,
Amsterdam,
1991, 1-14.
4.
M.
V.
Berry and
C.
J.
Howls,
Hyperasymptotics,
Proc. Roy. SOC. Lond. A,
430
(1990), 653-668.
5.
M.
V.
Berry and
C.
J.
Howls,
Hyperasymptotics
for
integrals with saddles,
Proc. Roy. SOC. Lond. A,
434
(1991), 657-675.
6.
W.
G.
C. Boyd,
Stieltjes transforms and the Stokes phenomenon,
Proc. Roy.
SOC. Lond.
A,
429
(1990), 227-246.
7.
R.
B. Dingle,
Asymptotic Expansions: Their Derivations and Interpretation,
Academic Press, New
York,
1973.
8.
F.
W.
J.
Olver,
Why
steepest descent?
SIAM Rev.,
12
(1970), 228-247.
9.
F.
W.
J.
Olver,
Uniform, exponentially improved, asymptotic expansions
for
the generalized exponential integrals,
SIAM
J.
Math. Anal.,
22
(1991), 1460-
1474.
10.
I. Podlubny,
Fractional Dzflerential Equations,
Academic Press, New
York,
1999.
11.
J.
Wimp,
Review
of
I.
Podlubny
’s
book “Fractional Differential Equations”,
SIAM Rev.
22
(ZOOO),
766-768.
12.
R. Wong,
Asymptotic Approximations
of
Integrals,
Academic Press, Boston,
1989.

223
13.
R.
Wong,
Eqonential asymptotics,
in Special Functions
2000:
Current Per-
spective and Future Directions,
J.
Bustoz et al. (eds),
NATO
Science Series
11,
vo1.30,
Kluwer,
2001, 505-518.
14.
R.
Wong and Y.-Q. Zhao,
Smoothing of Stokes’ discontinuity
for
the gener-
alized Bessel function,
Proc.
Roy.
SOC. Lond.
A,
455
(1999), 1381-1400.
15.
R.
Wong and Y.-Q. Zhao,
Smoothing of Stokes’ discontinuity for the gener-
alized Bessel function,
11,
Proc.
Roy.
SOC. Lond.
A,
455
(1999), 3065-3084.
16.
R.
Wong and Y.-Q. Zhao,
Exponential asymptotics of the Mittag-Lefler func-
tion,
Constr. Approx.,
18
(2002), 355-385.
17.
M.
Wyman,
The method of Laplace,
Trans.
Roy.
SOC. Canada,
2
(1964),
227-256.

224
LECTURE I11
WKB
METHOD AND TURNING POINT
The transition from the classical physics of the late nineteenth century
to
the quan-
tum mechanics of the early twentieth century
is
examplified by the problem
of
find-
ing uniformly asymptotic solutions of the LiouvilleGreen
(WKB)
equation.
A.F.
Nikiforov and
V.B.
Uvarov
1.
Introduction
Differential equations of the type
y"(x)
+
{X2a(x)
+
b(x)}y(z)
=
o
(1.1)
arise frequently in mathematical physics, where
X
is a positive parameter.
We first consider the simplest case, in which
a(.)
is a real, positive, and
twice continuously differentiable function in a given finite or infinite interval
(al, az).
We also assume that
b(x)
is
a continuous real- or complex-valued
function. Let
w
=
u'/"x)y(x).
It is readily verified that undei this transformation, equation
(1.1)
becomes
where
5
Uyx)
1
a'/(z)
b(x)
$(E)
=
-3
-
--
+
-
16
a
(x)
4a2(x)
a(.)
(1.4)
The change of variables from
(x,y)
to
(<,w)
is known as the
Liouville
transformation.
If we discard
+
in
(1.3),
then we obtain two linearly independent solu-
tions
eiixt.
In terms of the original variables, we get
y(z)
-Aa-ll4(z)
exp{iX
/
a1/2(x)dx}
(1.5)
+
Ba-1/4(x)
exp{-iX
a1/2(x)dz},
s

225
where
A
and
B
are arbitrary constants. Equation (1.5) is known as the
Liouville-Green approximation,
whereas physicists refer to (1.5) as the
WKB
(or
semiclassical) approximation
in recognition
of
the work of Wentzel
(1926), Kramers (1926) and Brillouin (1926). The contribution of these au-
thors was, however, not really the construction of approximation (1.5), but
the connection of exponential and oscillatory approximations across a
turn-
ing
point,
i.e.,
a
zero of
a(.).
In Sec. 2, we will give
a
rigorous proof
of
(1.5)
from
which one will
learn
a
basic argument frequently used to establish the validity of asymp-
totic solutions
to
differential equations. From this proof, we will also see
a
double asymptotic feature in the Liouville-Green approximation, that
is,
it sometimes holds either
as
X
-+
00
with
x
fixed, or as
x
-+
00
with
X
fixed. In Sec. 3, we introduce the Langer transformation, and present a
uniform asymptotic solution in the neighborhood of
a
turning point.
Sec.
4
deals with the case in which the coefficient functions
a(x)
and
b(x)
in
(1.1) have, respectively, a simple and
a
double pole in the interval
(al, a2).
The final section contains several examples to illustrate the usefulness
of
the approximations obtained in the previous sections. Most of the material
for this lecture is taken from the definitive book by Olver
[8].
2.
Successive Approximations
The most frequently used method to prove asymptotic results for differ-
ential equations is probably the method of successive approximations.
In
this section, we shall illustrate this method by establishing the validity
of
(1.5).
In (1.3) we substitute
w(t)
=
eiXE[l
+
h(~)],
(2.1)
and obtain
h”(C)
+
i2Xh’(C)
=
-$(C)[l
+
h(C)].
(2.2)
We view (2.2)
as
an inhomogeneous second-order differential equation in
h(().
By the principle of variation
of
parameters, one can convert
(2.2)
into the integral equation
where
a
is the value of
E
at
x
=
a, a
=
a1
or
a2,
and we assume
a
is finite.
One can easily verify that any solution of this integral equation is also a
solution of the differential equation (2.2).
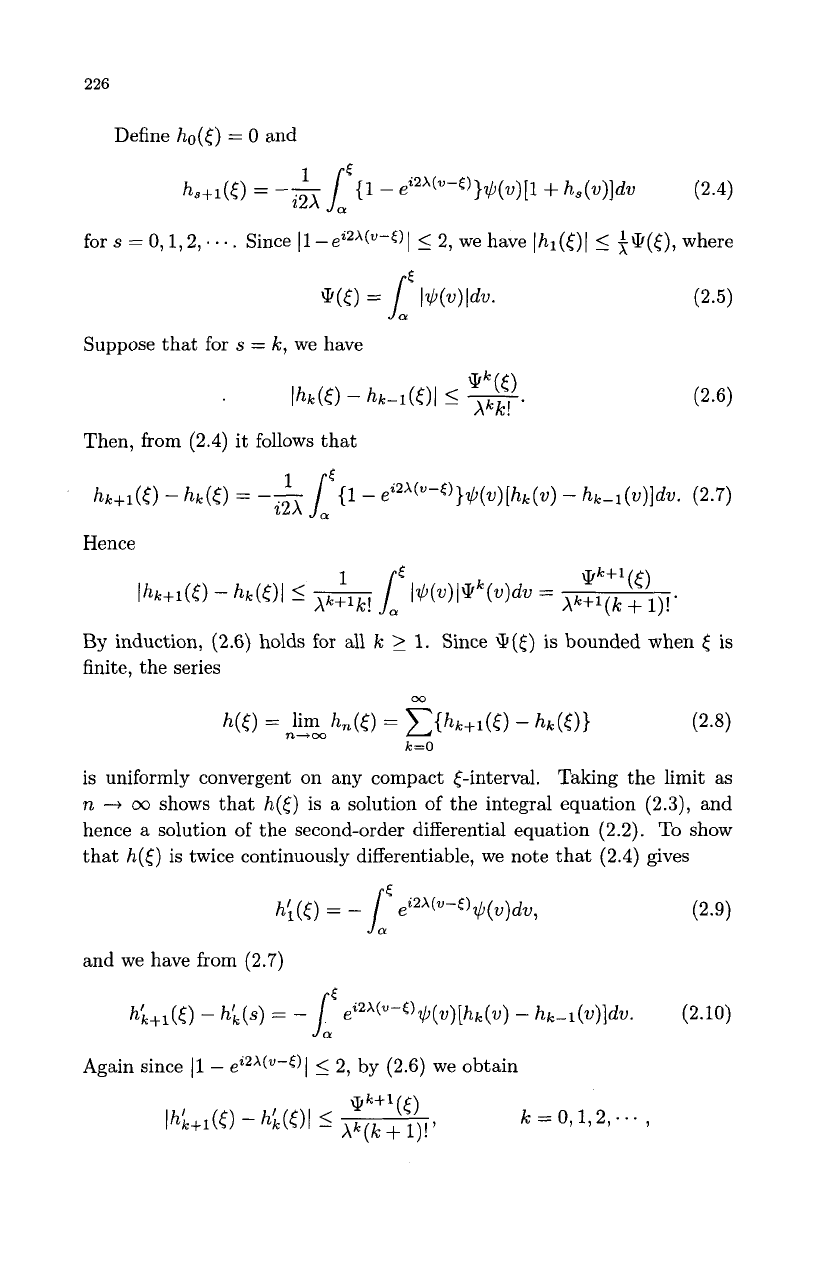
226
Define
ho(<)
=
0
and
fors=0,1,2,...
.
SinceIl-ei2'("-E)I <2,wehaveIhl(<)l
5
;@(<),where
Suppose that for
s
=
k,
we have
Then,
from
(2.4)
it follows that
Hence
By induction,
(2.6)
holds for all
k
2
1.
Since
9((E)
is
bounded when
(E
is
finite, the series
M
is uniformly convergent on any compact <-interval. Taking the limit as
n
-+
cm
shows that
h(<)
is a solution of the integral equation
(2.3),
and
hence a solution of the second-order differential equation
(2.2).
To
show
that
h(<)
is twice continuously differentiable, we note that
(2.4)
gives
E
hi(<)
=
-
S,
ei2'(+F)$(u)dv,
(2.9)
and we have from
(2.7)
E
hi+&)
-
h',(s)
=
-
/
ei2x(w-E)
$(V)[hk(V)
-
hk-l(V)ldV.
(2.10)
a
Again since
11
-
ei2'("-E)l
5
2,
by
(2.6)
we obtain

227
which clearly shows the uniform convergence of the series
Z{/L~+~(<)
-
hL(<)}
in any compact interval. Furthermore, from
(2.2)
we have
hi+,(<)
-
hi(<)
=
-i2"L+,(<)
-
hL(E)I
-$(<)Me)
-
hk-l(<)l.
Uniform convergence of
Z{hi+l(<)
-
hi(<)}
is now evident, and
h(<)
is
twice continuously differentiable.
In summary, we have shown that equation
(1.3)
is satisfied by the func-
tion
w(<)
in
(2.1)
with
h(<)
given by
(2.8).
Summation of
(2.6)
gives
lh(<)l
I
exP{
+)}
-
1.
(2.11)
To express the result in terms of the original variables, we introduce the
control function
and the notation
(2.12)
(2.13)
for the total variation of
F
over the interval
(a,
z).
It
is readily verified that
O(<)
=
V,,z(F).
Hence, on account of
(1.2),
equation
(2.1)
can be written
as
yl(z)
=
a-1/4(z)
exp{iA
a1/2(z)dx}[1
+
&1(A,z)]
(2.14)
J
with
The same argument will yield the second linearly independent solution
y2(z)
=
~i-l/~(z)
exp{-iA
a1/2(z)dz}[l
+
E~(A,Z)],
(2.16)
J
where
&~(A,z)
also satisfies
(2.15),
i.e.,
IE~(x,~)I
I
exp{
~v~JF)}
-
1. (2.17)
For fixed
z
and large
A,
the right-hand sides of
(2.15)
and
(2.17)
are both
O(A-l).
Hence, a general solution to
(1.1)
has the asymptotic behavior
given in
(1.5).

228
In (2.15) and (2.17), we can take
a
=
a1
and
a
=
a2,
respectively.
If
Val,a2(F)
<
co,
then the 0-terms obtained from (2.15) and (2.17) are
uniform with respect to
z.
The corresponding result for the equation
y"(z)
-
{X2a(z)
+
b(z)}y(z)
=
0
(2.18)
is that we have two linearly independent solutions of the form
yl(z)
=
~-l/~(z)
exp{X a1/2(z)dz}[l
+
~1(X,z)]
(2.19)
J
and
where
(j
=
1,2).
If
Val,a2(F)
<
co,
then (2.21) gives
(2.20)
(2.21)
y.(z)
3
=
~-l/~(z) exp{(-l)j-' Ja'qz)dz}[l
+
O(X-9,
(2.22)
j
=
1,2,
which holds uniformly for
z
E
(al,
a2).
tion corresponding to (2.3) is
The proof of (2.21) is very similar to that of (2.15). The integral equa-
where
11
-
ei2x(w-E)I
5
2
used in proving (2.6), we can now use the estimate
=
a1
corresponds to
z
=
al.
Since
t
-
w
2
0,
instead of the bound
o
<
-
1
-
e2x(v-E)
<
1.
(2.24)
As
a result, we have the extra factor
4
in the total variation
Va1,%(F)
in
(2.21). The result for
j
=
2
follows by replacing
z
in (2.18) by
-z.
It
is interesting to note that the error bounds in (2.21) can also be used
to give asymptotic properties of the approximations (2.19) and (2.20) in the
neighborhood of a singularity of the differential equation. Because of this
double asymptotic feature, the Liouville-Green approximation is indeed a
remarkably powerful tool for approximating solutions of linear second-order

229
differential equations.
To
illustrate
our
point, we let
X
=
1
in
(2.18),
and
assume that
V,,,,,(F)
<
co
and also
as
x-+ay. (2.25)
Under these conditions, we shall show that the error term
E~(x)
:=
~i(1,~)
in
(2.19)
satisfies
E~(x)
--f
a constant
as
x--ta;. (2.26)
This together with
(2.19)
shows that there is
a
solution
y3(2)
such that
y3(2)
N
a-1/4(rC)
exp{
1
a1/2
z)dx
,
x
-+
a;.
(2.27)
Coupling
(2.20)
and
(2.27),
we obtain two linearly independent asymptotic
solutions
as
x
-+
a;.
From
(2.21),
we know that
E~(z)
is bounded in
(al,a2).
What
(2.26)
says is that
E~(x)
does not oscillate
infinitely often
as
x
+
a2.
In view of
(2.25),
we have
a2
=
00
by
(1.2).
Since
V,l,a2(F)
<
00,
for any given
E
>
0,
there exists a positive number
6
E
(cq,
co)
such that
0
We now proceed
to
prove
(2.26).
Now assume that
E
2
6,
and subdivide the interval of integration in
(2.9)
at
S.
Note that in the present case,
X
=
1
and
"2"
is absent;
cf.
(2.23).
Thus, we have
lhi(<)ls
e2(U-E)I$(v>ldv
+
I$(w)ldv
5
e2(6-c)*(6)
+
E.
011
6'
Similarly, from
(2.10)
and
(2.6)
we obtain
for
k
2
1.
The extra factor of
2-k
comes from the fact that we use the
estimates in
(2.24)
for the case of eqution
(2.18),
instead of the bound
11
-ei2A(v-E)l
5
2
for the case of equation
(1.1).
From
(2.8),
it follows that
~h'(t)l
5
2e2('-E){,*(')/2
-
1)
+
e*(m)/'&. (2.28)
The first term on the right-hand side tends to zero as
t
4
00,
and since
E
is arbitrary,
(2.28)
implies that
h'(0
--f
0
as
E
-+
00.

230
With
X
=
1
in
(2.23),
it can be verified by differentiation that
(2.29)
1 1E
h(J)
=
--h’(J)
-
5
1
$(v)[l+
h(v)]dv.
(11
2
If
we let
c
c
lo(<)
=
l,
$(v)dv,
lk(6)
=
l,
“(v){hk(v)
-
hk-l(v))dv
(Ic
2
then in view of
(2.8)
we can rewrite
(2.29)
as
For
J
2
6,
we have from
(2.6)
(2.30)
(2.31)
since
a(()
is an increasing function. Coupling
(2.30)
and
(2.31)
gives
The right-hand side tends to zero
as
and
6
approach infinity indepen-
dently. Hence,
h(J)
must tend to a constant as
J
4
00;
this completes the
proof of
(2.26).
3.
Turning
Point
Problem
The problem of finding asymptotic solutions to the differential equation
(2.18)
becomes much more complicated, when the coefficient function
u(x)
has
a
zero, say at
x
=
zo,
in the interval
(all
u2).
Such a point is known
as
a
turning point
of the differential equation. In this case, there is an
ambiguity in taking the square root of the function
u(z),
and hence the
Liouville transformation
(1.2)
is not well-defined.
For
definiteness, we assume that
u(z)
has the same sign
as
z
-
zo;
i.e.,
u(z)(x
-
zo)
>
0
for all
z
#
zo.
(3.1)
Instead of
(1.2),
we now make the change of variables

231
and
It is easily verified that
The transformation
(2,
y)
H
(c,
w)
was first introduced by Langer
[3],
under
which equation
(1.1)
becomes
(3.5)
where
c
cb(z).
(3.6)
5
16 16a3(x)
a(.)
$(C)
=
-c-2
+
{4a(x)a”(x)
-
5[d(x)]2}--
+
-
If
+
in
(3.5)
is neglected, then we have the Airy equation
two linearly independent solutions of which are the Airy functions Ai(X2/3<)
and Bi(X2/3<). Using the method of successive approximation, one can
show,
as
in Sec.
2,
that equation
(3.5)
has twice continuously differentiable
solutions given by
yl(x)
=
6-1/4(z)(Ai(X2/3C)
+
cl(A,x)],
y2(x)
=
6-1/4(2)[Bi(X2/3c)
+
Q(X,
x)],
where
6(z)
=
a(z)/C;
see
(3.3)
and
(3.4)..
To give an estimate for the error
terms
E~(X,
x)
and
E~(X,
x),
we first introduce the error-control function
(3.7)
c
H(x)
:=
-
1
Ivl-1’2t,b(v)dv.
In terms of the original variable, it is equivalent to
The
modulus
function
M(x)
and the
weight function
E(x)
associated with
the Airy functions Ai(x) and Bi(x) are defined by
E(z)
=
1
for
-co
<
z
5
c,
E(z)
=
{Bi(z)/Ai(x)}1/2,
c5x<co,
(3.10)
