Hua C., Wong R. Differential Equations and Asymptotic Theory in Mathematical Physics
Подождите немного. Документ загружается.


332
max
lxllr'
When
e
=
0,
we understand that Exp(q/O;
C)
=
0,
which does not make
any contradiction in the results.
By the above choice of the Cauchy data
p(x)
we have for the Gevrey
order of the formal solution
f(t,
x)
-
EN-lfn(x)tn
n=O
5
CKN(N!)"ltlN,
Vt
E
S'\{O},
(8)
Remark
1.
Our Cauchy problem (CP) is only for a simple equation, but
the generalization is possible for an equation in two variables which has a
quasi homogeneity in derivatives
at
and
a,.
In fact, it is enough to follow
the arguments? consulting the arguments developed in this paper.
2.
a(0)-Gevrey summability (Review)
We, first, give a short review of the definition of the Gevrey summability
and the Gevrey sum.
Let
6'
E
R,
a
>
0
and
0
<
p
5
+m.
Then a sector
S(O,a,p)
in the
complex
t
plane is defined by
a
s(e,
a,
p)
=
{t
E
c
;
o
<
~tl
<
p,
larg(t)
-
el
<
2}
.
We refer
8,
a
and
p
as the direction, opening angle and the radius of the
sector, respectively.
Let
f(t,
x)
=
fn(x)tn
E
0,[[t]lU
with
a
>
0.
Then, by the defini-
tion, we say that
f(t,x)
is a-Gevrey summable in
0
direction (in t-plane)
if there exists an analytic function
f(t,
x)
on
S(t9,
a7r
+
E,
p)
x
{
1x1
5
r}
for
some positive constants
E,
p
and
r
such that for any closed sector
S'
with
a
vertex at the origin such that
(S'\{O})
c
S(0,
m++,
p)
there exist positive
constants
r',
C
and
K
such that the following asymptotic estimates hold
for all
N
2
1.
This kind of asymptotic expansion is called a-Gevrey asymp-
totic expansion, and it is written by
f(t,x)
Nu
f^(t,x)
in s(e,a7r+E).
(9)
In this expression, we omit to write the radius
p
of the sector
S(0,
m+~,
p),
since the radius is not an essential problem for the Gevrey summability. We
note that thanks of the condition that the opening angle of the sector is
larger than
a7r
for the definite domain of
f(t,
x),
one can show that such an
analytic function
f(t,
x)
is unique if such analytic functions exist, and it is

333
called the a-Gevrey sum of
f(tl
x)
in
0
direction. For the detail of Gevrey
summability, one can consult the book by W. Balser2.
We denote by
O,{t},,e
the set of formal power series
f(t,
x)
E
0,[[t]],
which is a-Gevrey summable in
0
direction.
We remark that if
C(t,x)
E
0,{t},(o),e
for the formal solution, then
its a(0)-Gevrey sum
u(t,
x)
is an actual analytic solution of the equation
a,"u(t,x)
=
a,"u(t,x)
satisfying the Cauchy data
~(x)
in the asymptotic
meaning as
t
-,
0
along the sector
S(0,
a(0)n
+
E,
p).
Now the characterization of u(O)-Gevrey summability of the formal
so-
lution
C(t,
x)
is obtained as follows;
THEOREM
1l0
O,{t},(o),e
if
and only
if
the following conditions are satisfied;
Let
cp(x)
E
0,
for the Cauchy data. Then,
ii(tl
x)
E
+92nm
(ii)
by
some positive constants
C
and
6.
Here,
O(52)
denotes the set
of
holo-
lcp(x)l
5
Cexp(6lxlq/(q-p))
for
x
E
uq-'
m=O
morphic functions on the domain
52.
The conditions (i) and (ii) can be expressed in a simplified form as
follows. Let us define
q-1
~(x,
5)
:=
C
cp(x
+
w:<)
(wq
=
e2.1ri/q).
m=O
Then the conditions (i) and (ii) are equivalent to that
uniformly in
x
in a neighborhood of the origin
x
=
0,
by some positive
constant
E.
This means that
@(x,
<)
is defined on
{
1x1
5
r}
x
S@O/q,
E,
cm)
for some
r
>
0
and satisfies the following growth condition
by some positive constants
C
and
6.
About the integral representation of the a(0)-Gevrey sum, we have

334
THEOREM
26*7
Under the conditions in
Theorem
1,
the a(0)-Gevrey
sum u(t, x)
of
C(t, x) in
8
direction is given
by
the following integral formula
W(PQ/Q)
u(t,x)
=
1
WG
C)Eo(t,
C)
dC,
(11)
where the integration is taken from the origin
to
the infinity along the half
lane
of
argument
p8/q,
i.e.,
e(pe/Q)iR>O,
-
and the kernel function Eo(t,
C)
is
given
by
Here,
we
use the following notations and definitions.
cpq
=
5,
and G;::
(
Z
1
z))
(Z
E
C\{O}) denotes the Meijer G-function
defined
by
by
an integral path
I
=
{T
=
k
+
iy;
y
E
R}
with
a
fixed
-l/q
<
k
<
0.
3.
The case of entire function Cauchy data
PROPOSITION
1.
Let
p(z)
E
Exp(q/C;
C)
(1
5
C
5
q
-
p
-
1)
for the
Cauchy data. Then G(t,
x)
E
O,{t},(t),e
(a(C)
=
(q
-
p
-
C)/p)
if
@(z,
C)
satisfies the condition
(10).
Moreover, the a(-!?)-Gevrey sum u(t,x) is given
by.the formula
(11).
Remark
2.
In the above proposition, the condition is only a sufficient
condition which is just the same with the a(0)-Gevrey summability and
the integral expression of the a(l)-Gevrey sum is also nothing but the
a(0)-
Gevrey
sum.
As
a
natural question, the condition will be expected to be
a
necessary condition for the o(C)-Gevrey summability. But we do not know
whether it is true or not. On the other hand, one can find
a
necessary and
sufficient condition for the a(l)-Gevrey summabiIity in different form by
following Balser's argument
'I2:
Let

335
be a formal solution of the Cauchy problem (CP). (The restriction for
a
such as
a(!)
is not necessary.) Let us define
In this case, the necessity of the condition is easily proved. For the proof
of the sufficiency we take the a-Gevrey sum
+j(z)
of
dj(z).
Then one can
prove that
is the a-Gevrey sum of
C(t,z).
Here we have to mention that it is not an
easy task to realize the conditions in
(16)
as those for the Cauchy data
cp(z)
itself as in Proposition
1.
The proof of Proposition
1
is done by making step by step formal Borel
transformations which sends the divergent series to a convergent series, and
after checking the analytic continuation property and exponential growth
condition along a sector of infinite radius for the obtained convergent series,
we make again step by step Laplace transformations to obtain the Gevrey
sum. The procedure is already done in the previous
paper^^^,^^^,
therefore
the proof does not include or need anything new, but at each step of making
the formal Borel transformation we get a partial differential equation of
non-Kowalevsh type which is Fuchsian in Baouendi-Goulaouic's sense for
which the kernel function or the fundamental solution for the Gevrey sum
is obtained by
a
special function which is given by Meijer's G-functionss.
Such a procedure will be given Section
5.
4.
Proof
of
Proposition
1
The proof of Proposition
1
follows immediately from those for Theorems
1
and
2,
and essentially we will repeat the proofs of them which are given in
the paper~''?~*~.
The fundamental criterion of the Gevrey summability is given by
LEMMA
1l0
Let
f(t,z)
E
O,[[t]],.
Then the following two statements
are equivalent:

336
(i)
?(ti
.)
E
Ox{t}u,o.
(ii)
Let
{aj}:=l
(aj
>
0,
k
2
1)
satisfy
a
=
01
+
. ..
+
ak,
and define
g(s,
x)
E
Os,x
by iterated formal Borel transformations
*-
g(s,
x)
:=
(BUk
0
. .
.o
B,,
f)(s,
x).
(18)
Then
g(s,
x)
E
Exp,(l/a;
S(6,
E,
m))
uniformly
in
x
in
a neighborhood
of
x
=
0,
by
a positive constant
E.
Moreover, under the condition
(ii),
the a-Gewrey
sum
f
(t,
x)
of
f(t,
x)
is given by the following iterated Laplace transformations
f
(t,
=
(LI/~,
,e
0
. .
.O
Li/uk
,o
9)
(t,
x),
(19)
where
Exactly speaking, the analytic continuation of
f
(t,
x)
in
t
variable into
a sector
S(0,
an
+
E,
p)
for some positive constant
E
is done by rotating the
argument of the path of integration
eieR>o
-
(R>o
-
:=
[O,+oo)),
which is
permitted by the condition that
g(s,
x)
E
Exp,(l/a;
S(6,
E,
00))
uniformly
in
x
in a neighborhood of the origin.
For
the proof of Proposition
1,
we define
w(s,
x)
=
({Bl/p}q-P-eii)(s,
x)
E
(21)
Then for the proof
of
a([)-Gevrey summability in
6
direction, it is enough
to prove the following exponential growth property:
4s,x)
E
EXP,
(
9-P-e
;
,S(0,E,rn))
(22)
uniformly in
x
in a neighborhood of
x
=
0.
For
that purpose we further
take
C
times iterated formal Borel transformations and put
477,
.)
=
({~l,p}em,
.)
It is obvious that
4%
.)
=
c.C;,,
W)(Sl.>.
(24)
This suggests that the desired property
for
~(s,
x)
will be reduced from that
for
~(7,x).

337
In order to establish an integral expression of
w(q,x)
by using the
Cauchy data
@(x,
c)
=
xkzo
~(x
+
CUT)
and
a
kernel function, we need
to use the generalized hypergeometric function (or series). We notice that
where
(q/q)n
=
nq,,
(j/q)n
((C),
:=
C(C
+
1)
.
*
.
(C
+
n
-
1)).
The series on
the right hand side is a generalized hypergeometric series which is written
bY
where
lq-p-l
:=
(l,...
,1)
((q-p-1)-ple
of
1's).
By using this generalized
hypergeometric series
or
function, we get the following integral expression
of
4%
x).
by taking small
r
>
0,
for
x
near the origin and
17
depending on the choice
of
r
such that
171
<
(pP/qq)'/P&P
.
Here we notice that
qFq-l
(.
. .
;
z)
is a holomorphic solution at the ori-
gin of
a
F'uchsian ordinary differential equation with three regular sin-
gular points at
z
=
0,1,
m.
Therefore,
qFq-l(.
. .
;
z)
is holomorphic on
C\[1,
+m),
and has a moderate growth when
z
tends to the singular point
z
=
1
or
z
=
00.
By this observation we see that the condition
(10)
for
a(.,<)
(or
see
the conditions (i) and (ii) in Theorem
1)
implies that
uniformly in
x
in a neighborhood of
x
=
0.
This means that when
e
=
0,
the condition
(10)
implies that
G(t,
x)
E
O,{t},(o),~.
In order to prove the a([)-Gevrey summability for general
e
and to get
the integral expression
(11)
of the Gevrey sum, we rewrite the integral
representation of
w(q,
x)
by deforming the path of integration by consid-
ering that the part of hypergeometric function in the integrand of
(25)
has
q
singular points in <-plane at roots of
cq
=
(qq/pP)qP
for any fixed
VE
S(8,
E,
m).
(We want to move
7
-+
00
along
S(8,
E,
m)).
Let
<(q)
be the

338
root of
cq
=
(qq/l?p)qP
of argument arg(q)p/q
(=
pO/q
when
77
E
eieR,o)
.
For the convenience
or
the simplification of notations we put
We restrict
77
E
eieR,o on the half line of argument
6'
for the simplicity of
notation, since it is complicated and tedious to specify the dependence on
77
of path at each integration. We get the following expression
of
w(q,
x).
By
recalling that
w(s,
x)
=
(~C;,~w)(s,
x),
we have
Here, we need the integral expression of
f(q,<)
and their difference
appeared in the integrand.
where Iarg(-X;')l
5
T,
I
=
{K
f
iy;y
E
R}
by
a fixed
K.
such that
-l/q
<
K
<
0
and
Cpq
=
I'(p/p)/I'(q/q).
(See the Appendix7 for delicate
points.) Fkom this expression, we have
Moreover
by
taking
C
times iterated Laplace transformations, we have
=
277-i
c,,
G;!~,~
ps
IP/P;I;p;q-p-e)
.
By
inserting this into the formula
(28),
we get the following integral ex-
pression of
w(s,x).

339
In order to estimate the exponential growth of
v(s,x)
as
s
+
co
along
S(0,
E,
co),
we recall the following asymptotic expansion for the Meijer
G-
function':
as z
+
co,
larg(z)l
5
er.
Combining with this estimate and that
uniformly in
x
in a neighborhood of the origin, one can easily prove that
v(s,
z)
E
Exp,(p/(q-p-e);
S(0,
E,
00))
uniformly in
z
in a neighborhood of
the origin for some
E
>
0
which assures the (q-p-e)/pGevrey summability
of the formal solution
C(t,
z)
in
0
direction.
The integral representation of the
(q
-
p
-
C)/p-Gevrey sum
is
obtained
by taking
(q
-
p
-
e)
times iterated
p
Laplace transformations to
v(s,
z),
that is,
u(t,
z)
=
{L;;-,P-ev}(t,
z)
This is nothing but the integral expression
(11)
which we want to prove.
Remark
3.
The above proof shows that under the condition
(10)
for the
Cauchy data
p(z)
E
0,
we have
(dflpC)(t,z)
E
Ox{t}(q-p-e)/p,O
and its
(q
-
p
-
t)/p-Gevrey sum is given
by
w(P0lq)
(L;;-,P-ew)(t,
x)
=
.I
C)Ee(t,
C>
d~,
where
340
5.
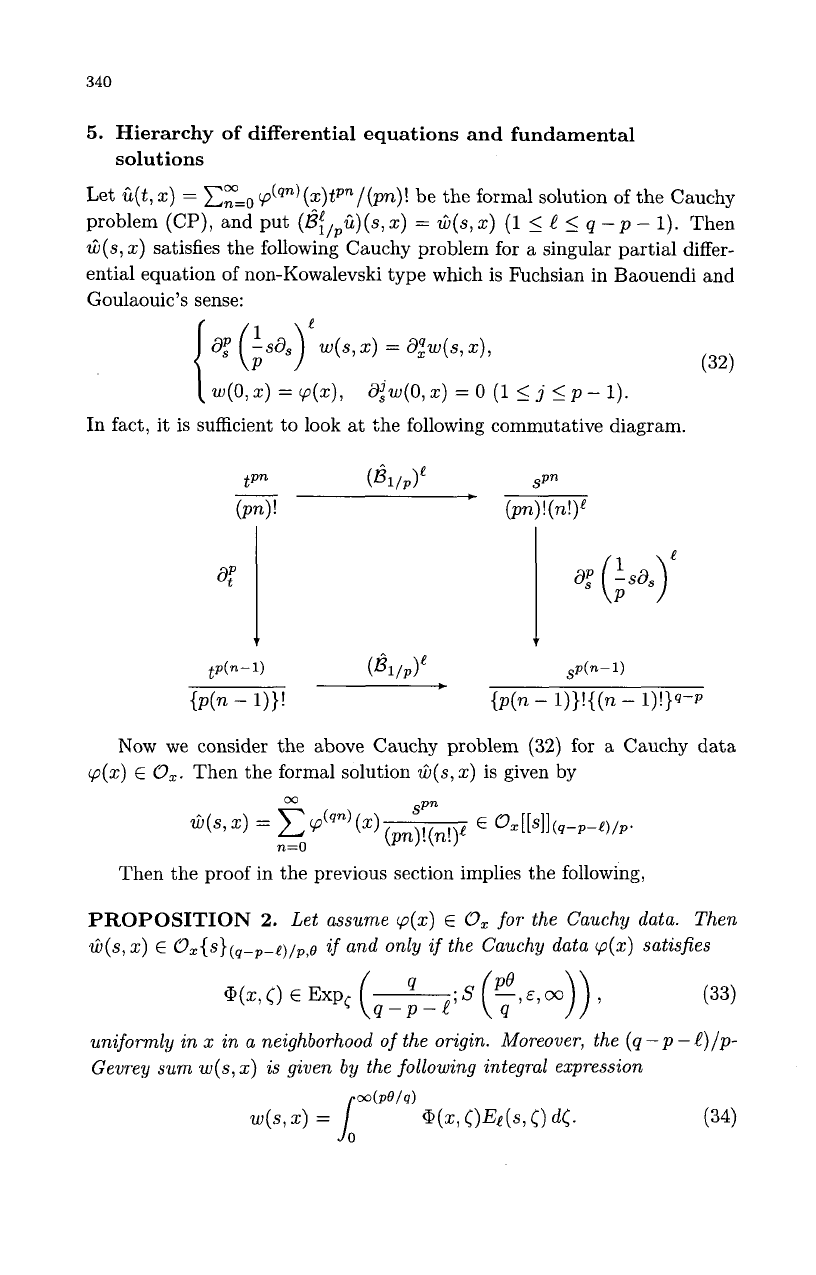
Hierarchy of differential equations and fundamental
Let
G(t,
x)
=
C,"==,
p(qn)(f)tPn/(pn)!
be the formal solution of the Cauchy
problem
(CP),
and put
(B:,PO)(~,x)
=
G(s,x)
(1
5
t
5
q
-p
-
1).
Then
G(s,
x)
satisfies the following Cauchy problem for a singular partial differ-
ential equation of non-Kowalevski type which is Fuchsian in Baouendi and
Goulaouic's sense:
solutions
(32)
W(0)
x)
=
cp(x),
ajw(0,
x)
=
0
(1
5
j
I
p
-
1).
In fact, it
is
sufficient to look at the following commutative diagram.
a;
t
sp(n-1)
{p(n
-
l)}!{(n
-
1)!}"-"
1.3~
we consider the above Cauchy prc lem
(32)
for a Cauchy data
p(x)
E
0,.
Then the formal solution
G(s,
x)
is given by
Then the proof in the previous section implies the following,
PROPOSITION
2.
Let assume
p(x)
E
0,
for
the Cauchy data. Then
G(s,
x)
E
0,{~}(~-~-e)/~,~
if
and only
if
the Cauchy data
p(z)
satisfies
uniformly
in
x
in
a neighborhood
of
the origin. Moreover, the
(q
-p
-
t)/p-
Gevrey sum
w(s,x)
is given by the following integral expression

341
We omit the proof, since the sufficiency follows directly from the argu-
ments in the previous section and the necessity follows from the argumentlo.
6.
Singular perturbation
The idea developed in the previous sections can be extended directly to the
study
of
Gevrey summability of divergent solutions to singular perturbed
ordinary differential equations. In this section, we shall present the idea.
Let
p
and
q
be two natural numbers, and consider the following equation
(I
-
t"a:)u(t,
x)
=
p(Z)
E
ox,
(35)
where
I
denotes the identity operator. Then a unique formal solution is
obtained by
m
qt,
x)
=
c
p(qn)(x)tpn
E
o,"t]]q/p'
(36)
n=O
From this expression
of
formal solution, we easily see that
C(t,
x)
E
Ot,x
if and only if
p(x)
E
Exp(1;
C).
Now as
a
fundamental result on the Gevrey summability and the integral
expression of the Gevrey sum, we can prove the following proposition which
corresponds to Theorems
1
and
2.
PROPOSITION
3.
only
if
Let
p(x)
E
Ox.
Then
ii(t,x)
E
OX{t},/,,~
if
and
uniformly
in
x
in
a
neighborhood of the origin for some constant
E
>
0.
Moreover,
in
this case, the qlp-Gevrey sum
u(t,
x) is given by the following
integral formula
w(pQ/q)
C)Fo(t,
we,
(38)
I
u(t,x)
=
where the kernel function Fo(t,(') is given
by
The proof of this proposition is reduced to those of Theorems
1
and
2
for
the Cauchy problem
(CP).
In fact, by taking formal Bore1 transformation of
