Hua C., Wong R. Differential Equations and Asymptotic Theory in Mathematical Physics
Подождите немного. Документ загружается.


342
order
1
(
81)
to
the
equation
(35),
we obtain
an
integro-differential equation
{I
-
d;Pa$}w(s,
x)
=
(p(x),
since
the
multiplier
by
tP
is
send
to
an
integral
operator
a;..
Therefore
by
operating
a,P
to
the
obtained
equation,
we get
the
following Cauchy problem.
Thus,
when
q
>
p,
the
problem
is
reduced
to
the
Cauchy problem
(CP)
and
the
previous
results
are
applicable.
On
the
other hand,
when
p
2
q,
by putting
T
=
tP
we
change
the problem
to
the
following
one,
{I
-
T~$}w(T,
x)
=
cp(z)
by which
the
problem
is
reduced
to
the
above
case.
We
can
present
the
corresponding results
to
Propositions
1
and
2,
but
we
omit
to
write
them
down
in
explicit
form,
since
they will
be
easily
recognized.
References
1.
W. Balser, Divergent solutions of the heat equations: on the article of Lutz,
Miyake and Schafke
,
Pacific
J.
Math., Vol.
188 (1999), 53
-
63.
2.
W. Balser, Formal power series and linear systems of meromorphic ordinary
differential equations, Springer-Verlag, New York,
2000.
3.
W. Balser, Summability of formal power series solutions of partial differential
equations with constant coefficients, to appear in Proceedings of the Inter-
national Conference
on
Differential and F'unctional Differential Equations,
Moscow
(2002).
4.
W. Balser,
Multisummability of formal power series solutions of partial
differential equations with constant coefficients, manuscript
(2003).
5.
W. Balser and M. Miyake, Summability of formal solutions of certain partial
differential equations
,
Acta Sci. Math. (Szeged), Vol.
65
(1999), 543
-
551.
6.
K. Ichinobe, The Borel sum of divergent Barnes hypekgeometric series and
its application to
a
partial differential equation, Publ. RIMS, Kyoto Univ.,
7.
K. Ichinobe, Integral representation for Borel sum of divergent solution to
a
certain non-Kowalevski type equation, to appear in Publ. RIMS, Kyoto
Univ., Vol.
39,
No.
4 (2003).
8.
Y.
L. Luke, The special functions and Their Approximations, Vol. I, Aca-
demic Press,
1969.
9.
D. A. Lutz, M. Miyake and
R.
Schafke,
On
the Borel summability of divergent
solutions
of
the heat equation
,
Nagoya Math.
J.,
Vol.
154 (1999), 1
-
29.
10.
M. Miyake, Borel summability of divergent solutions of the Cauchy problem
to non-Kowalevskian equations, Partial differential equations and their ap-
plications, (Wuhan,
1999), 225
-
239,
World Sci. Publishing River Edge,
NJ,
1999.
Vol.
37 (2001), 91
-
117.

ON THE SINGULARITIES OF SOLUTIONS OF
NONLINEAR PARTIAL DIFFERENTIAL EQUATIONS IN
THE COMPLEX DOMAIN, I1
HIDETOSHI TAHARA*
Department
of
Mathematics,
Sophia
University,
Kioicho, Chiyoda-ku, Tokyo
102-8554,
JAPAN
E-mail: h-tahara@hoffman. cc. Sophia.
ac.jp
The paper deals with the nonlinear first order partial differential equation
(E)
au/dt
=
F(t,
I,
u,
au/az)
in the complex domain
Ct
x
Cz,
and consider the fol-
lowing problem: does
(E)
has a solution which possesses singularities only on the
hypersurface
{t
=
O}?
In
a
generic case, the main result is stated
as
follow: we can
find such
a
negative rational number
u
that
(E)
has no solutions with singularities
only on
{t
=
0)
of order
u
=
o(ltl')
(as
t
0)
but that
(E)
has a solution with
singularities only on
{t
=
0)
of order
u
=
O(lt1")
(as
t
-+
0).
1.
Introduction
Let
C
be the complex plane
or
the set
of
all complex numbers, let
(t,x)
=
(t,q,.
. .
,x,)
E
C
x
P,
y
E
C,
z
=
(21,.
.
.
,
z,)
E
C,,
and let
F(t,z,y,z)
be a holomorphic function with respect to the variables
(t,
x,
y,
z)
E
Ct
x
In
this paper, as in
[7]
we will consider the first order nonlinear partial
CE
x
Cy
x
C:.
differential equation
au
-
at
=
F(t,x,u,g),
where
u
=
u(t,x)
is the unknown function and
du/ax
=
(du/ax1,. . .
,
du/dx,).
Throughout this paper, we will assume:
F(t, x,
y,
z)
is
a
holo-
morphic function on
R
x
C,
x
C:,
where
R
is an open neighborhood
of
the
origin
(0,O)
E
Ct
x
C:.
~~
*This work was partially supported by the Grant-in-Aid for Scientific Research
No.
14540185
of Japan Society for the Promotion of Science.
343

344
The following theorem is one of the most fundamental results in the
theory of partial differential equations in the complex domain:
Theorem
1.1
(Cauchy-Kowalewski).
For any holomorphic function
cp(s)
the equation
(1.1)
has a unique holomorphic solution
u(t,
x)
in
a neigh-
borhood
of
(0,O)
E
@t
x
@:
satisfying ~(0,s)
=
p(x)
near
x
=
0.
By this theorem the structure of local holomorphic solutions of
(E)
is
completely known; though, it says nothing about singular solutions (that is,
solutions with some singularities).
Does
(1.1)
has a solution with some sin-
gularities? What kinds
of
singularities appear
in
non-holomorphic solutions
As
a first attempt to answer these questions, in this paper we will con-
of
(1.1)?
sider the following problem:
Problem 1.2.
Let
s
be a real number. Does (1.1) have a solution which
possesses singularities only on the hypersurface
{t
=
0)
with the growth
order
u(t,
x)
=
O(ltl")
(as
t
-
O)?
If the equation (1.1) is linear, it is known by Zerner
[ll]
that such
singularities do not appear in the solutions
of
(1.1). In the nonlinear case,
Tsuno's result in
[9]
asserts that such singularities also do not appear in the
solutions of
(1.1)
if
s
is a non-negative real number; but, if
s
is
a
negative
real number we have the following example:
Example 1.3.
Let
(t,x)
E
C2.
The equation
au
with
m
E
I+?*(=
{1,2,.
.
.})
at
has a family of solutions
u(t,x)
=
(-l/m)l/m(z+c)/tl/m
with an arbitrary
constant
c
E
@.
Clearly, this has singularities only on
{t
=
0)
of order
u
=
O(lt(-l/m)
(as
t
-
0).
2. Non-existence
of
singularities
In this section we will give a brief survey on the non-existence
of
singularities
By the Taylor expansion in
(y,
z)
we can express
F(t,
x,
y,
2)
in the form
F(t,
x,
Y,
2)
=
c
aj,a(t,
.)
Yj
za
(%,)€A

345
where
(j,a)
E
N
x
N",
A
is a subset of
N
x
N",
aj,a(t,x)
are holomorphic
functions on
a,
and
za
=
zlal
. . .
znan.
Without loss of generality we may
suppose that
aj,a(t,
x)
f
0
for any
(j,
a)
E
A;
then we can write
qa(t,x)
=
tkj+
bj,a(t,x)
where
kj,a
is
a
non-negative integer and
bj,,(O,x)
$
0.
Using the above,
the equation
(1.1)
may now be written
as
Set
A2
=
{(j,
a)
E
A;
j
+
la1
2
2)
(where
la/
=
a1
+
.
.
.
+
an).
We
remark that the equation (1.1) is linear
if
and only if
A2
=
0;
it is nonlinear
otherwise. Since we already have Zerner's result in the linear case, we will
assume henceforth that
(1.1)
is nonlinear, that
is,
A2
#
0.
Then we define
the index
(T
by
-kj,a
-
1
ff
=
sup (2.2)
(j,a)EA2
j
+
-
'
which was introduced by Kobayashi
[5].
Note that
(T
is
a
non-positive
real number. Set
R+
=
{(t,x)
E
0;
Ret
>
0).
For
a
function
f(t,x)
and
a
neighborhood
w
of
x
=
0
E
CE
we define the norm
lif(t)llw
=
supzEw
If(t,
.)I.
We have the following result (originally by Kobayashi
[5],
and improved by Lope-Tahara
[S]):
Theorem
2.1.
Suppose
A2
#
0.
Let
(1)
If
a
holomorphic solution
u(t,x)
of
(1.1)
in
R+
satisfies
Ilu(t)IIw
=
o(ltl')
(as
t
-
0),
then
u(t,
x)
can be extended holomorphically up
to
some
neighborhood
of
the origin
(0,O)
E
Ct
x
Cg.
be the index defined by
(2.2).
(2)
Set
-kj,a
-
1
=
.}.
j
+
IaI
-
1
If
M
=
8
and
if
a
holomorphic solution
u(t,x)
of
(1.1)
in
R+
satisfies
Ilu(t)llw
=
O(ltl")
(as
t
-
0),
then
u(t,z)
can be extended holomorphically
up
to
some neighborhood
of
the origin
(0,O)
E
Ct
x
Cg.
Hence we can get the following result on the non-existence of the sin-
gularities on
{t
=
0).
Corollary
2.2.
Suppose
A2
#
0.
Let
u
be the index defined by
(2.2).

346
(1)
There appears no singularities on
{t
=
0)
with growth order o(lt1")
(2)
If
M
=
0,
there appears no singularities on
{t
=
0)
with growth
(as t
-
0)
in the solutions
of
(1.1).
order O(ltl") (as t
-
0)
an the solutions
of
(1.1).
3.
Criteria for the existence of singularities
Suppose A2
#
0,
M
#
0
and set
P(z,
Y,
2)
=
c
bj,a(O,
.)
Yj
za.
(3.1)
(j,a)EM
It is easy to see that
P(z,y,z)
$
0
and that
P(z,y,z)
is
a
holomorphic
function on
{z
E
C;
(0,z)
E
52)
x
CY
x
C:;
moreover in
(3.1)
we have
j
+
la/
2
2.
Since
M
#
0,
we have
o
=
(-kj,a
-
l)/(j
+
la1
-
1)
for any
(j,
a)
E M:
this implies that
o
is a negative rational number.
To
prove the existence of singularities of order
It["
on
{t
=
0),
we will
construct
a
solution of the form
+,
.)
=
t"
(44
+
w(t,
XI),
(3.2)
where
p(z)
is a holomorphic function in
a
neighborhood of
z
=
0
with
p(z)
$
0,
and w(t,z) is a function belonging in the class
6+
which is
defined by the following:
Definition
3.1.
A
function w(t,
z)
is said
to
be in the class
6+
if
it satisfies
the following conditions c1) and 122): cl) w(t,
z)
is
a
holomorphic function
inadomain{(t,z)
ER(C~\(O))XC~;O<
It1 <rl(argt),Izl <R)forsome
positive-valued, continuous function
~(s)
on
R,
and some
R
>
0;
c2) there
is an
a
>
0
such that for any
B
>
0
we have
SU~~~~<~
Iw(t,z)I
=
O(lt1")
(as
t
+
0
under
I
argtl
<
Q).
Here
R(Ct
\
(0))
denotes the universal covering
space of
Ct
\
(0).
Since
o
<
0,
p(z)
$
0
and w(t,z)
--f
0
(as
t
--f
0),
we easily see
that this function
(3.2)
has really singularities of order Itl" on {t
=
0).
Hence, if we can construct such
a
solution
as
in
(3.2),
we can conclude that
singularities of order ltl" on
{t
=
0)
really appear in the solutions of
(1.1).
Let
us
recall the result in
[7]
which guarantees the existence of singu-
larities only on {t
=
0)
of the growth order
lt1".
For
a
function
p(z)
we
define
a
vector field
X(p)
by
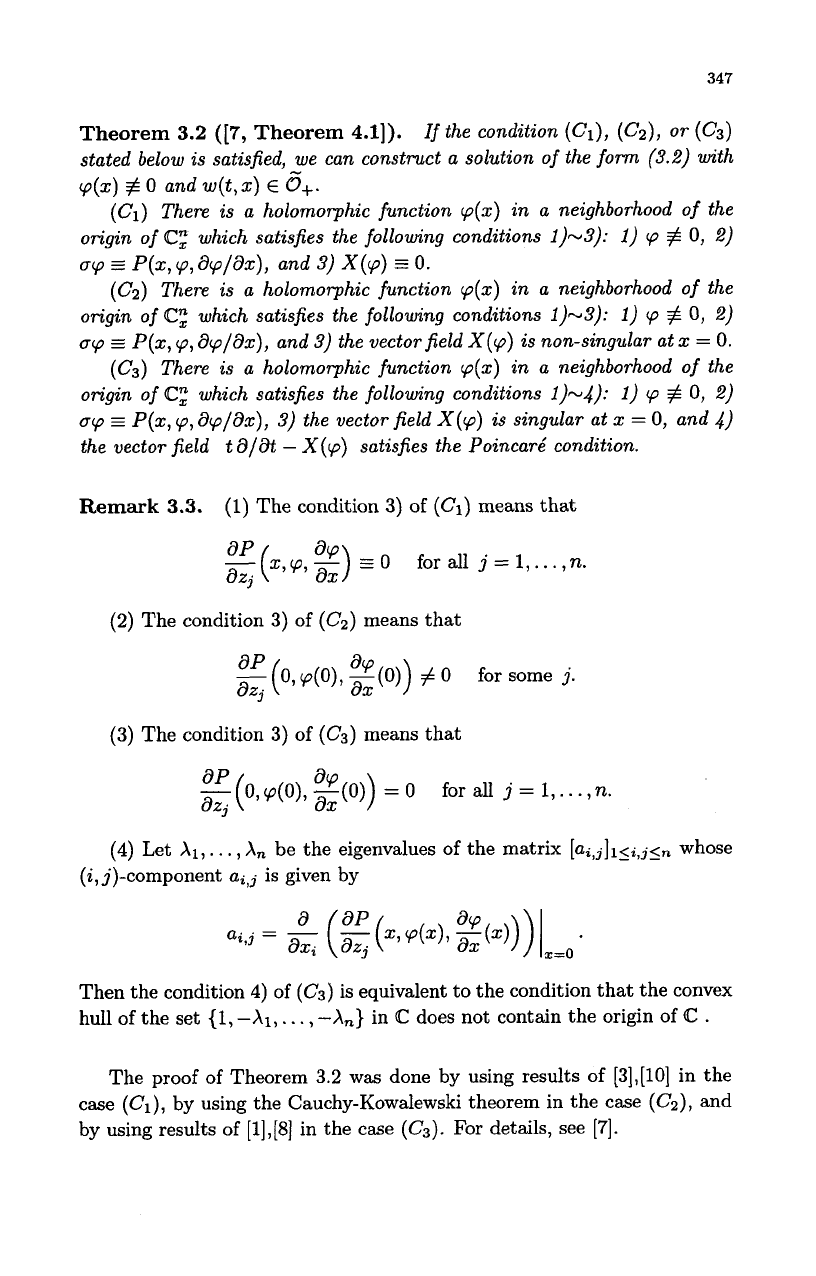
347
Theorem
3.2
([7,
Theorem
4.11).
If
the condition
(Ci),
(C2),
or
(C3)
stated below is satisfied, we can construct a solution
of
the
forn
(3.2)
with
cp(x)
$0
and
w(t,x)
E
6+.
((71)
There is a holomorphic function
~(x)
in
a neighborhood
of
the
origin
of
C:
which satisfies the following conditions
l)m3):
1)
cp
$
0,
2)
((72)
There is a holomorphic function
cp(x)
in
a neighborhood
of
the
origin
of
Cy
which satisfies the following conditions
l)-3):
1)
cp
f
0,
2)
ucp
3
P(x,
cp,
acplax),
and
3)
the vector field
X(cp)
is non-singular at
x
=
0.
(C3)
There is
a
holomorphic function
cp(x)
in
a neighborhood
of
the
origin
of
Cy
which satisfies the following conditions
l)-4):
1)
cp
$
0,
2)
up
=
P(x,
cp,
acplax),
3)
the vector field
X(cp)
is
singular at
x
=
0,
and
4)
the vector field
t
81%
-
X(cp)
satisfies the Poincare' condition.
ocp
3
P(x,
cp,
acpldx),
and
3)
X(cp)
=
0.
Remark
3.3.
(1)
The condition
3)
of
(C1)
means that
ap
azj
-<x,cp,g>
r~
forall
j=~,
...,
n.
(2)
The condition
3)
of
(C2)
means that
-
aP
(0,
cp(01,
%(o))
acp
+
o
for some
j.
at;.
(3)
The condition
3)
of
(C3)
means that
(4)
Let
Xi,.
.
.
,
A,
be the eigenvalues of the matrix
[~i,j]~si,js~
whose
(i,j)-component
ai,j
is given by
Then the condition
4)
of
((33)
is equivalent to the condition that the convex
hull of the set
(1,
-XI,.
. .
,
-A,}
in
C
does not contain the origin of
C
.
The proof of Theorem
3.2
was done by using results of
[3],[10]
in the
case
(C,),
by using the Cauchy-Kowalewski theorem in the case
(CZ),
and
by using results of
[1],[8]
in the case
(Cs).
For
details, see
[7].
348
4.
Sufficient conditions for the existence of singularities
Suppose
A,
#
0,
M
#
0,
and let
P(x,
y, z)
be the one in
(3.1).
We write
">
and
8~
ap
_-
ax
-
(a,,,...'
oxn
_-
In this section, using the criteria in section
3
we will present four sufficient
conditions for the existence of singularities of the growth order
Itl"
only on
{t
=
0).
The four conditions correspond to the following four cases:
dP
dP
dX
dz
Case
(0)
:
-(z,
y,O)
=
(0,.
. .
,0)
and
-(x,
y,O)
=
(0,.
. .
,0)
;
dP
dz
dP
dz
Case
(1)
:
-(z,
y,
z)
=
(0,.
.
.
,o)
;
Case
(2)
:
-(O,
y,
z)
f
(0,.
. .
,O)
;
dP
dP
dz dz
Case
(3)
:
-(O,
y,
z)
=
(0,.
. .
,0)
and
-(x,
y,
z)
f
(0,.
.
.
,0)
.
We call the case
(0)
a special case:
this comes from the fact that in the case
(0)
we can take
p(x)
as
a constant function and we have
dp/dx
=
0.
The
classification of the cases
(1),(2),(3)
will be not
so
strange; these correspond
to the criteria
(C~),(CZ),(C~)
in Theorem
3.2.
Now, let us first consider the case
(0).
In this case, by the condition
(aP/dx)(z,y,
0)
3
(0,.
. .
,
0)
we see that
P(x,
y,
0)
is independent of
x
and we can write
P(x,y,O)
=
q(y)
for some entire function
q(y)
satisfying
q(0)
=
0
and
q'(0)
=
0.
Theorem
4.1
(Case
(0)).
Suppose
A2
#
0,
M
#
0
and the conditions
in
Case
(0).
Let
q(y)
be as above and set
C*
=
{y
E
CC
\
(0);
q(y)
=
ay}.
Then,
if
C*
#
0
the equation
(1.1)
has a solution which possesses
singularities only on
{t
=
0)
with the growth order
Itl".
Proof.
Since
C*
#
8
is assumed, we can take
b
E
C*
which satisfies
q(b)
=
ab
and
b
#
0.
Then,
by
setting
p(x)
=
b
we can verify the cri-
terion
(C,)
in Theorem
3.2
in the following way:
1)
p(x)
f
0
comes from
the fact
b
#
0;
2)
P(x,p,dp/dx)
=
P(x,b,O)
=
q(b)
=
ab
=
up;
and
3)
(dP/dz)(x,
p,
dp/dx)
=
(dP/dz)(x,
b,O)
=
(0,. .
.
,0)
(since one of the
0
conditions in Case
(0)
is
(dP/dz)(x,
y,
0)
=
(0,.
.
. ,
0)).

349
Example
(0).
Let
(t,
x)
E
C2
and let us consider
where
b(z)
and
c(t,x)
are holomorphic functions. Then
a
=
-1,
P
=
y2
+
b(z)z2
and
so
the conditions in Case
(0)
are satisfied. Since
q(y)
=
y2
we have
C*
=
{y
E
C
\
(0)
;
q(y)
=
-y}
=
{-l}
#
8.
Thus we can apply
Theorem
4.1
to this case and obtain the following: this equation has a
solution with singularities
only
on
{t
=
0)
of
order
It/-'.
Next, let us consider the case
(I).
In this case, by the condition
(dP/dz)
(x,
y;z)
=
(0,
... .
,
0)
we see that
P(x,
y,
z)
is independent
of
z
and we can
write
P(xl
y,
z)
=
p(x, y).
Theorem
4.2
(Case
(1)).
Suppose
A2
#
0,
M
#
0
and the condition
in
Case
(1).
Let
p(z,
y)
be
as above
and
set
C*
=
{y
E
C
\
(0)
;
p(0, y)
=
ay}.
Then,
if
aP
C*
#
0
and -(O,y)
$
a
on
C*
aY
the equation
(1.1)
has
a
solution which possesses singularities only on
{t
=
0)
with the growth order
Proof.
By the assumption
(4.1)
we can choose
b
E
C
such that
b
#
0,
p(0,
b)
=
ab
and
(ap/dy)(O,
b)
#
a.
Applying the implicit function theorem
to
P(S,Y)
=
ay
at
(X,Y)
=
(016)
we have
a
holomorphic function
'p(x)
in
a
neighborhood
of
x
=
0
which
satisfies
p(x,
'p(x))
=
a'p(x)
and
p(0)
=
b.
Then we can see that this
~(x)
satisfies the conditions
1)-3)
of
((21)
in Theorem
3.2
in the following way:
1)
(~(5)
$0
is verified by
~(0)
=
b
#
0;
2)
P(x,cp,a'p/ax)
=
p(x,'p)
=
acp;
and
3)
(aP/&)(x,
'p,
a'p/dx)
E
(0,.
. .
,
0)
comes from the condition of Case
(1)-
Example
(1).
Let
(t,z)
E
C2
and let
us
consider
where
a(.)
and
c(t,x)
are holomorphic functions.
Then
a
=
-1,
P
=
u(x)y2
and
so
the condition in Case
(1)
is satisfied. Since
p(0, y)
=
u(0)y2

350
we have
C’
=
{y
E
C
\
(0);
a(0)y2
=
-y};
if
a(0)
#
0
we have
C*
=
{-l/a(O)}
#
8
and
(dp/dy)(O,
-l/a(O))
=
-2
#
a.
Thus, if
a(0)
#
0
we
can apply Theorem 4.2 to this case and obtain the following: this equation
has a solution with singularities only on
{t
=
0)
of
order
ItJ-l.
Thirdly, let us consider the case (2). This is the most generic case and
we have:
Theorem
4.3
(Case
(2)).
Suppose
A2
#
0,
M
#
0
and the condition
in
Case
(2).
Set
C
=
{(y,z)
E
U2
x
Cn;
P(O,y,z)
=
ay}.
Then,
if
-(4.2)
the equation
(1.1)
has
a
solution
which
possesses singularities only on {t
=
0)
with the growth order
JtJ“.
Proof.
By the assumption (4.2) we can choose
(b,c)
E
C
x
Cn
such that
P(0,
b,c)
=
ab
and
(dP/dz)(O,b,c)
#
(0,. . .
,O).
Since the degree of each
term of
P(0,
y,
z)
in (3.1) is greater than or equal to 2 with respect to
(y,
z),
we see that the condition
(dP/dz)(O,
b,
c)
#
(0,. . .
,0)
implies
(b,
c)
#
(0,O).
Without loss of generality we may assume
(dP/dzn)(O,
b,
c)
# 0.
Set
x’
=
(XI,.
. .
,x,-l),
z’
=
(21,.
. .
,
z,-1),
c
=
(cI,.
. .
,
c,)
and
c’
=
(cl,.
. .
,
c,-~).
Let us apply the implicit function theorem to
P(z,
9,
z)
=
ff
Y
at
(x,
Y,
z)
=
(074
c).
Since
(aP/dzn)(O,
b,
c)
#
0,
we have a holomorphic function
H(z,
y,
2‘)
in
a neighborhood of
(x,
y,
z’)
=
(0,
b,
c’)
which satisfies
P(rc,y,z’,H(rc,y,z’))
=
ay
and
H(O,b,c’)
=
c,.
(4.3)
We now consider the following Cauchy problem:
Since
H(x,
y,
z’)
is holomorphic in a neighborhood of
(x,
y,
z’)
=
(0,
b,
c’),
by
the Cauchy-Kowalewski theorem we can get a unique holomorphic solution
p(x)
in a neighborhood of
x
=
0.
By (4.4) we have
p(0)
=
b,
(acp/dx‘)(O)
=
c‘
and
so
(dp/dx,)(O)
=
H(O,p(O),
(dp/dx’)(O))
=
H(O,b,c’)
=
c,;
thus
we obtain
(dcp/drc)(O)
=
c.
Then we can see that this
p(x)
satisfies the condition
1)-3)
of
(C,)
in Theorem 3.2 in the following way:
1)
p(z)
$
0
comes from the fact
351
(cp(O),
(dp/ax)(O))
=
(b,c)
#
(0,O);
2)
P(z,cp,dp/dx)
=
up
is verified by
(4.3) and the first equation of (4.4); and 3)
(dP/dzn)(O,
cp(O),
(dp/ax)(O))
0
=
(aP/azn)(O,
b,
c)
#
0.
*
Example
(2).
i) Let
(t,
x)
E
C2
and let us consider
dU
m
-=u(g)
at
,
mEN*.
Then
(T
=
-l/m,
P
=
yzm, aP/dz
=
myzm-',
and
C
=
{(y,z)
E
@
x
@;
yzm
=
(-l/m)y}.
If
we take
(l,(-l/m)l/m)
E
C
we have
(dP/d~)(O,l,(-l/m)~/")
=
-(-m)l/m
#
0.
Thus, we can apply The-
orem 4.3 to this case and obtain the following: this equation has a solution
with singularities only on
{t
=
0)
of order
ltl-l/m.
Compare this with
Example
1.3.
ii) Let us consider
where
a(x), b(x)
and
c(t,z)
are holomorphic functions. Then
(T
=
-1,
P
=
a(x)y2 +b(x)z2
and
so
if
b(0)
#
0
the condition in Case
(2)
is satisfied.
We have
C
=
{(y,
z)
E
@
x
@
;
a(0)y2
+
b(0)z2
=
-y}.
If
we take
a
so
that
a
+
a(0)a2
#
0
and define
p
by
p2
=
-(a
+
a(0)a2)/b(0),
then we have
,f3
#
0,
(a,p)
E
C
and
(dP/dz)(O,a,P)
=
2b(O)P.
Thus, if
b(0)
#
0
we can
apply Theorem 4.3 to this case and obtain the following: this equation has
a solution with singularities only on
{t
=
0)
of
order
1tJ-l.
Lastly, let us consider the case
(3).
We will give a sufficient condition
only in the case
n
=
1;
in the general case
n
2
2
we have no good results.
Suppose
n
=
1
and
set
Theorem
4.4
(Case
(3)).
Suppose
n
=
1,
A,
#
0,
M
#
0
and the
conditions
in
Case
(3).
If
(4.5)
in
@,
the equation
(1.1)
has a solution which possesses singularities only
on
{t
=
0)
with the growth order
Itl'.
