Houze Robert A., Jr. Cloud Dynamics

Подождите немного. Документ загружается.

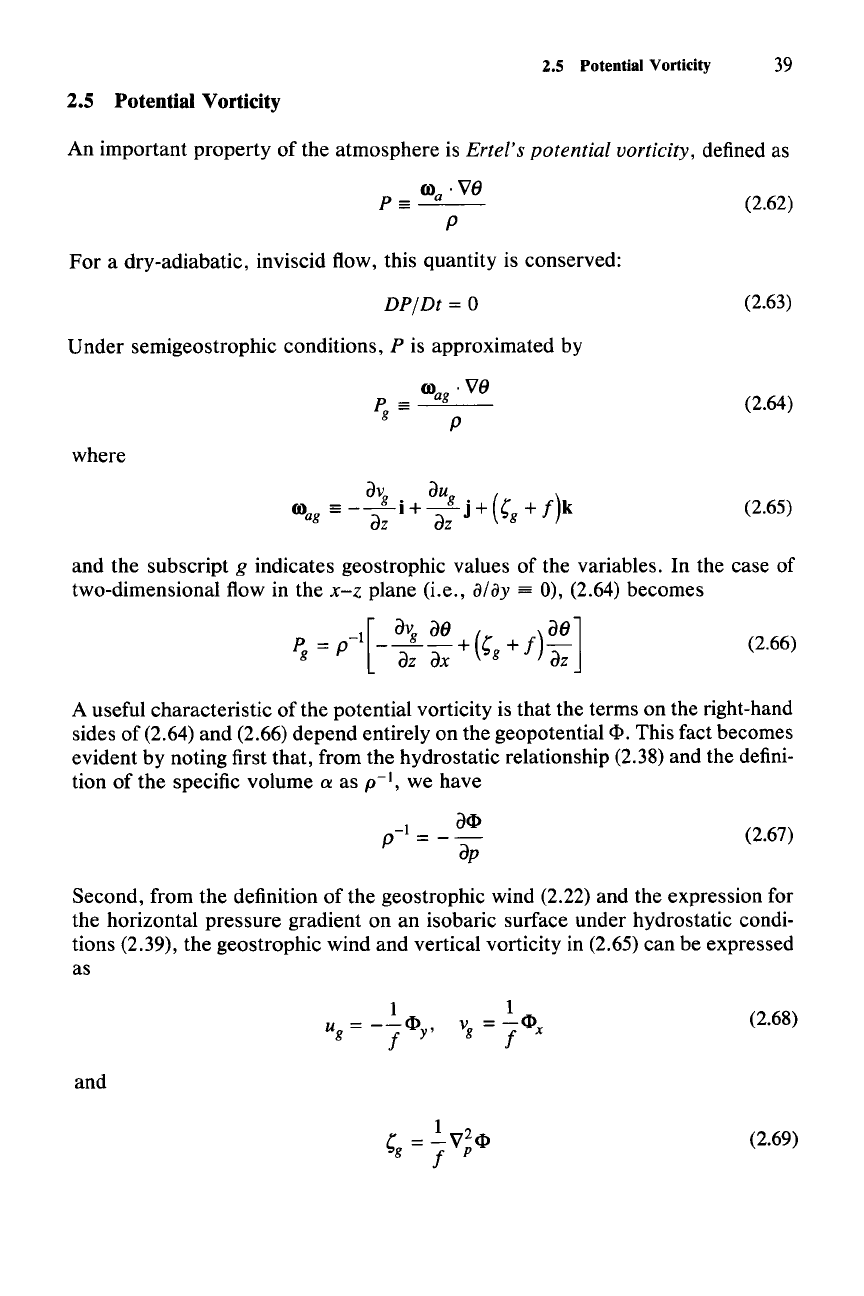
(2.62)

2.5 Potential Vorticity 39

2.5

Potential Vorticity

An important property of the atmosphere is Ertel's potential vorticity, defined as

m ·'\10

p==~a

For

a dry-adiabatic, inviscid flow, this quantity is conserved:

DP/Dt

= 0

Under semigeostrophic conditions, P is approximated by

mag'

'\10

-"-----

g p

where

g•

aUg.

( )

==--I+-J+J'+!k

az az

»s

(2.63)

(2.64)

(2.65)

and the subscript

g indicates geostrophic values of the variables. In the case of

two-dimensional flow in the

x-z plane (i.e., a/ay

0), (2.64) becomes

-1[

( )

ao]

p = p

---

+ ,

(2.66)

A useful characteristic of the potential vorticity is that the terms on the right-hand

sides of (2.64) and (2.66) depend entirely on the geopotential

<1>.

This fact becomes

evident by noting first that, from the hydrostatic relationship (2.38) and the defini-

tion of the specific volume

a as o:', we have

-1

a<l>

=--

(2.67)

Second, from the definition of the geostrophic wind

(2.22) and the expression for

the horizontal pressure gradient on an isobaric surface under hydrostatic condi-

tions (2.39), the geostrophic wind and vertical vorticity in (2.65) can be expressed

and

U =

--<I>

g !

(2.68)

(2.69)

(2.71)

2 Atmospheric Dynamics

Third, from the definition of (J in (2.8), the definition of a as

p-I,

the equation of

state (2.4), and the hydrostatic relationship (2.38), we have

pIC

(d4»)

e = - - (2.70)

RdpIC-l

Substitution of (2.67)-(2.70) into (2.64) and (2.66) demonstrates that the geo-

strophic potential vorticity depends on only one dependent variable

(<1»

in a

pressure-coordinate system. Conservation of

P, expressed by (2.63), then implies

a prediction of

<1>

and [according to (2.68)] the geostrophic wind. This behavior is

an extremely useful and important characteristic of a geostrophically balanced

flow.

Under saturated conditions,

(J is not conserved, and hence neither is P. A

quantity analogous to

P, which we call the equivalent potential vorticity, is de-

fined as

IDa'

Vee

----=-~

e p

Because of (2.18), P, behaves in an analogous fashion to P when the air is satu-

rated; thus,

P, is conserved under saturated conditions. However, P, is not ex-

actly conserved under unsaturated conditions. The

geostrophic equivalent poten-

tial vorticity

Peg

is obtained by replacing (J with

(Je

in (2.64).

2.6 Perturbation Form of the Equations

For a wide variety of analytical purposes, it is useful to consider air motions in

terms of deviations from an average over some arbitrary spatial volume of air

(e.g., a grid volume in a numerical model). Any variable

st1

is then expressed as

S'l

+ S'l'

(2.72)

where the overbar represents the average value and the prime the deviation.

When variables are decomposed in this way, the basic equations split into two

sets: the

mean-variable equations, which predict the behavior of the mean vari-

ables, and the

perturbation equations, which predict the departures from the

mean state. In the following subsections, we will write out the mean-variable and

perturbation equation forms of the anelastic equations. Since the Boussinesq

equations are a simplification of the anelastic form, the results for the anelastic

case will also indicate the mean-variable and perturbation equations in the Bous-

sinesq case. In addition to the basic equations for all the mean and perturbation

variables, the form of the kinetic energy equation for the eddy motions will be

indicated.P

For

further discussion of the averaging techniques used in this section, see Chapter 2 of Stull

(1988).

2.6 Perturbation Form of the Equations

2.6.1 Equation of State and Continuity Equation

Since the equation of state (2.4) and the continuity equation for anelastic flow

(2.54) contain no time derivatives, they take on rather simple forms when split into

mean-variable and perturbation forms. In the atmosphere, perturbations of den-

sity and virtual temperature are always small compared to their mean values.

Hence, if the variables

p ; p, and Tv are written as in (2.72), and (2.4) is then

averaged, we obtain the approximate equation of state for the mean variables,

(2.73)

(2.74)

If this relation is subtracted from (2.4), we obtain the equation of state for the

perturbation variables,

=--+-

If v is written as in (2.72), and (2.54) is averaged, we obtain the mean-variable

continuity equation,

(2.75)

To obtain this relation, it must be assumed that the derivative of a spatial average

is equal to the spatial average of the spatial derivative. The assumption is valid as

long as the deviations from the average occur on a considerably smaller scale than

the region over which the average is taken. This assumption is sometimes called

scale

separation.

If this relation is subtracted from (2.54), we obtain the continuity

equation for the perturbation wind,

(2.76)

2.6.2 Thermodynamic and Water-Continuity Equations

If the First

Law

of Thermodynamics (2.9) is combined with the continuity equa-

tion (2.54), we obtain

1 .

- +

V· P Ov =

'lJ(',

0 0

(2.77)

This form of the equation is referred to as the

flux

form,

since

pof}v

is the three-

dimensional potential temperature flux.

the variables 0,

'ie,

and v are written as

in (2.72), both sides of (2.77) are averaged, and we again make the scale separation

assumption, then the mean-variable thermodynamic equation is found to be

1 -

- =

'lJ(',

V· P v' 0'

Dt 0 0

(2.78)

42 2 Atmospheric Dynamics

where

D a a a a

-+u-+v-+w-

(2.79)

Since products

the dependent variables

(}

and

v appear in (2.77), positive or

negative covariances of the variables (i.e., v'

(}')

may contribute to changes

following a parcel of air. The covariance is proportional to the

eddy

flux

of poten-

tial temperature Pov'(}'. When the averaged thermodynamic equation (2.78) is

used, the convergence of the eddy flux becomes an additional diabatic effect.

If (2.78) is subtracted from (2.77) and (2.75) is invoked, we obtain the perturba-

tion thermodynamic equation,

(2.80)

If the perturbations remain small enough, the last two terms

can

be neglected. In

that case, the equation is said to'be linearized about a state of mean motion vand

mean potential temperature

The water-continuity equations (2.21) have the same form as the thermody-

namic equation (2.9), where qi replaces

(}

and S, replaces

'ie.

Hence, the mean-

variable and perturbation forms of the water-continuity equations (2.21) are, by

analogy to (2.78)

and

(2.80),

Dqi

-1'["7

-,-;

--=

i-Po

v'Povqi'

i = 1,

...

, n

(2.81)

and

(2.82)

i = 1,

...

, n

l5q~

--'

= Si -

V·

Poqi

v' +

V·

Poqiv' -

V·

Poqi

where, as in (2.21), i = 1,

...

, n represent the various categories of

water

sub-

stance being considered.

2.6.3 Equation of Motion

Now

consider the equation of motion for the Boussinesq flow (2.47). If v,

p*,

and

Band

F are written as in (2.72) and substituted in (2.47), then averaging (2.47)

and making the scale separation assumption yields the mean-variable form of the

equation of motion,

-----,;-

_ _ -

- =

--Vp

-fkxv+Bk+F

(2.83)

where

@i==

_p~1

[(V.

u'v')i +

v'v')j

w'v')k]

(2.84)

Equation (2.83) is the momentum analog

(2.78). The term

;ffi

is the three-

dimensional convergence

the eddy flux

momentum.

may be regarded as

2.6 Perturbation Form of the Equations 43

the frictional force associated with eddy motions of the air, while

F is the smaller-

scale molecular friction force. Subtracting (2.83) from (2.47) leads to an equation

for the velocity perturbation:

Dv'

- =

-(v',

V)v -

(v'·

V)v' -

-V(p

*) -

x v' +

B'k

-;g;

(2.85)

If the perturbations remain small enough and molecular friction is negligible, the

last two terms in (2.85) can be neglected, and the linearized form

ofthe

equation is

obtained.

We now have obtained the complete set of equations for anelastic flow decom-

posed into the mean-variable equations (2.73), (2.75), (2.78), (2.81), and (2.83) and

the perturbation equations (2.74), (2.76), (2.80), (2.82), and (2.85). In the case of

Boussinesq flow, the continuity equation (2.55) is used instead of (2.54). Exactly

the same equations as (2.73)-(2.85) are then obtained, except that the density term

disappears from (2.75)-(2.78), (2.80)-(2.82), and (2.84).

2.6.4 Eddy Kinetic Energy Equation

Taking v' . (2.85) and then averaging both sides of the equation leads to the eddy

kinetic energy equation

where

'{b

-u'v'

. Vii - v'v' . Vv - w'v' . Vw

w'B'

_p~lV"

V(p*)'

qj)

v' .

- v' . <j - v' . (v' . V)v'

= v' .

- v' . <j - v' .

V'X

(2.86)

(2.87)

(2.88)

(2.89)

(2.90)

(2.91)

'J{

is the eddy kinetic energy, and '{b is the rate at which

'J{

is created by conversion

from mean-flow kinetic energy, when down-gradient eddy momentum fluxes oc-

cur.

is the creation (destruction) of'J{ by thermally direct (indirect) flow pertur-

bations, which are those eddies for which buoyancy is positively (negatively)

correlated with vertical velocity [e.g., eddies characterized by warm (cold) air

rising and cold (warm) air sinking].

'W is the creation of

'J{

by pressure-velocity

correlation, which physically is the rate at which work is done by the force of the

gradient of perturbation pressure acting on parcels over distances given by the

perturbation velocity.

qj) (a negative quantity) is frictional dissipation of

'J{

eddies or molecular friction.

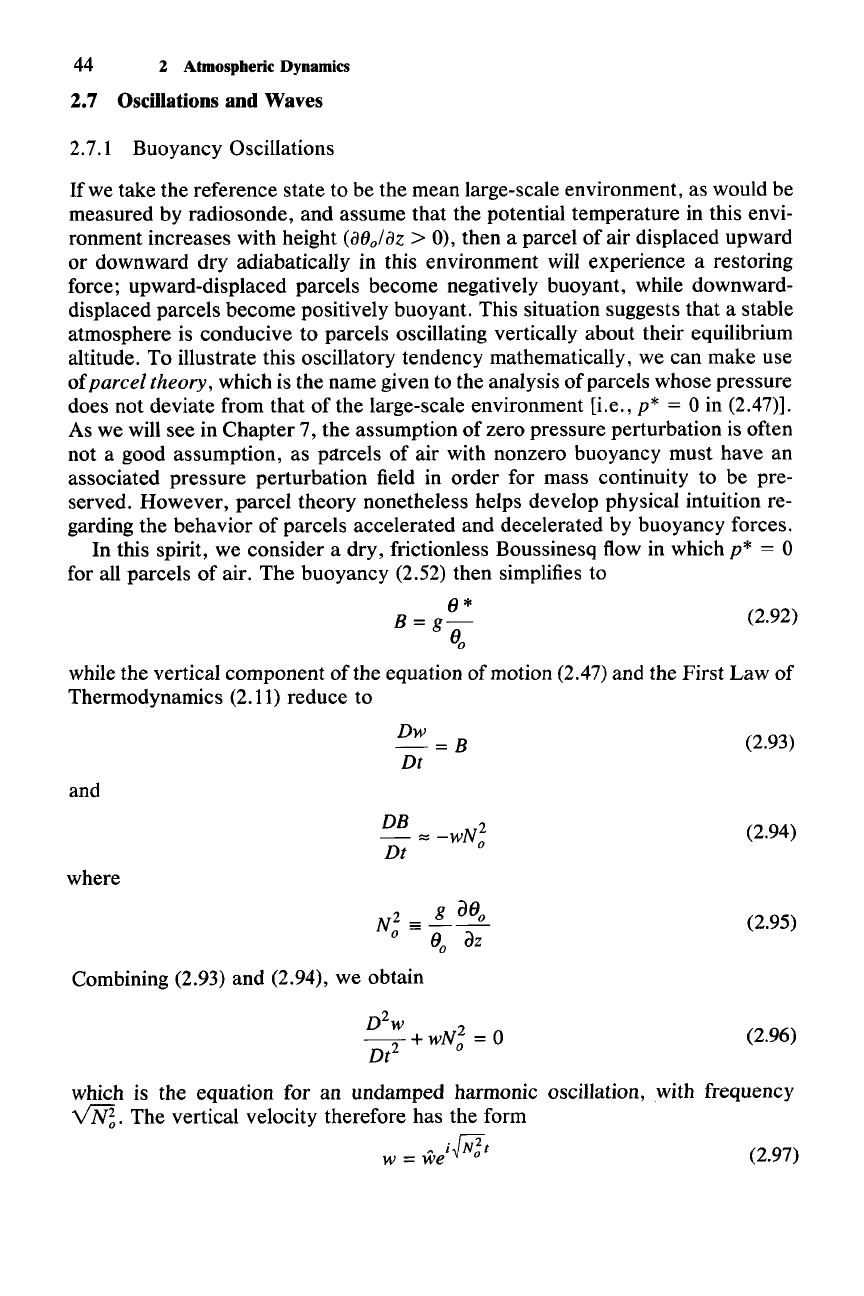
44 2 Atmospheric Dynamics

2.7 Oscillations and Waves

2.7.1 Buoyancy Oscillations

If we take the reference state to be the mean large-scale environment, as would be

measured by radiosonde, and assume that the potential temperature in this envi-

ronment increases with height

(iJOoliJz

> 0), then a parcel of air displaced upward

or downward dry adiabatically in this environment will experience a restoring

force; upward-displaced parcels become negatively buoyant, while downward-

displaced parcels become positively buoyant. This situation suggests that a stable

atmosphere is conducive to parcels oscillating vertically about their equilibrium

altitude. To illustrate this oscillatory tendency mathematically, we can make use

of parcel theory, which is the name given to the analysis of parcels whose pressure

does not deviate from that of the large-scale environment [i.e.,

p* = 0 in (2.47)].

As we will see in Chapter 7, the assumption of zero pressure perturbation is often

not a good assumption, as parcels of air with nonzero buoyancy must have an

associated pressure perturbation field in order for mass continuity to be pre-

served. However, parcel theory nonetheless helps develop physical intuition re-

garding the behavior of parcels accelerated and decelerated by buoyancy forces.

In this spirit, we consider a dry, frictionless Boussinesq flow in which

p* = 0

for all parcels of air. The buoyancy (2.52) then simplifies to

B =

(2.92)

°0

while the vertical component of the equation of motion (2.47) and the First Law of

Thermodynamics (2.11) reduce to

-=B

and

DB 2

-,,=,-wN

where

2 g

=---

Combining (2.93) and (2.94), we obtain

(2.93)

(2.94)

(2.95)

+ wN

= 0 (2.96)

which is the equation for an undamped harmonic oscillation, with frequency

YNio,

The vertical velocity therefore has the form

w =weiR, (2.97)

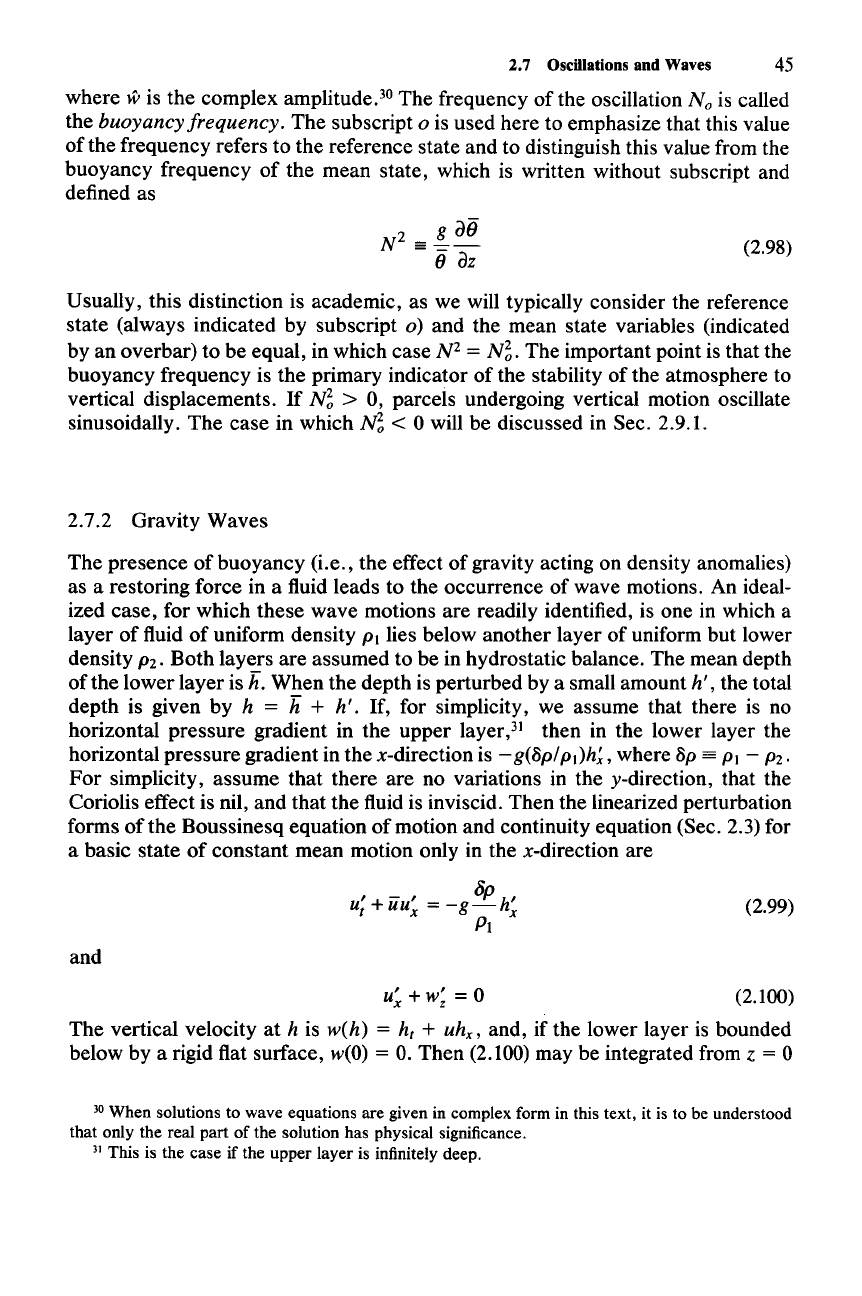
2.7 Oscillations and Waves 45

where

11>

is the complex amplitude.t"

The

frequency of the oscillation No is called

the

buoyancy frequency.

The

subscript 0 is used here to emphasize that this value

of the frequency refers to the reference state and to distinguish this value from the

buoyancy frequency

the mean state, which is written without subscript and

defined as

2 g

==-

()

(2.98)

Usually, this distinction is academic, as we will typically consider the reference

state (always indicated by subscript

0) and the mean state variables (indicated

by an overbar) to be equal, in which case

NZ =

N~.

The important point is that the

buoyancy frequency is the primary indicator

the stability of the atmosphere to

vertical displacements.

> 0, parcels undergoing vertical motion oscillate

sinusoidally.

The

case in which

< 0 will be discussed in Sec. 2.9.1.

2.7.2 Gravity Waves

The

presence

buoyancy (i.e., the effect

gravity acting on density anomalies)

as a restoring force in a fluid leads to the occurrence of wave motions. An ideal-

ized case, for which these wave motions are readily identified, is one in which a

layer

fluid

uniform density PI lies below another layer of uniform but lower

density

pz.

Both

layers

are

assumed to be in hydrostatic balance. The mean depth

ofthe

lower layer is h. When the depth is perturbed by a small amount

h',

the total

depth is given by

h = h +

h',

If, for simplicity, we assume that there is no

horizontal pressure gradient in the upper

layer,"

then in the lower layer the

horizontal pressure gradient in the x-direction is

-g(8p/PI)h;,

where 8p

PI - pz.

For

simplicity, assume that there are no variations in the y-direction, that the

Coriolis effect is nil,

and

that the fluid is inviscid. Then the linearized perturbation

forms

the Boussinesq equation

motion and continuity equation (Sec. 2.3) for

a basic state

constant mean motion only in the x-direction are

and

8p ,

uu~

-g-h

PI x

(2.99)

(2.100)

The

vertical velocity at h is

w(h)

= h, + uh

and, if the lower layer is bounded

below by a rigid flat surface,

w(O)

Then (2.100) may be integrated from z = 0

30 When solutions to wave equations are given in complex form in this text, it is to be understood

that only the real part of the solution has physical significance.

31 This is the case if the upper layer is infinitely deep.

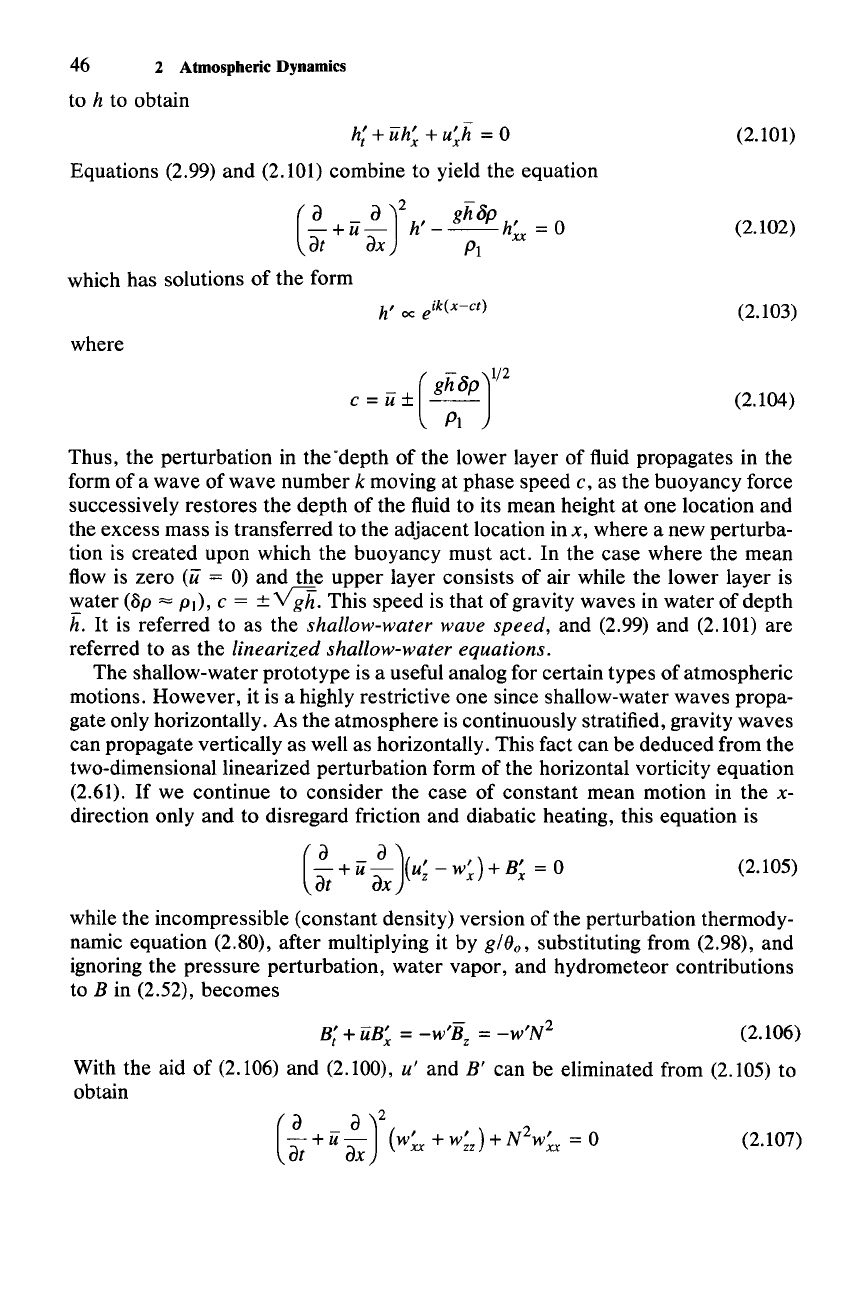
46 2 Atmospheric Dynamics

to h to obtain

+ iih'. + u' h = 0

t x x

Equations (2.99) and (2.101) combine to yield the equation

(

a _

a)2,

-+u-

---h

= 0

which has solutions of the form

h' oc

eik(x-ct)

where

(2.101)

(2.102)

(2.103)

(2.104)

(2.105)

(

c=li±

Thus, the perturbation in the "depth of the lower layer of fluid propagates in the

form of a wave of wave number

k moving at phase speed c, as the buoyancy force

successively restores the depth of the fluid to its mean height at one location and

the excess mass is transferred to the adjacent location in

x, where a new perturba-

tion is created upon which the buoyancy must act. In the case where the mean

flow is zero

(ii

= 0) and the upper layer consists of air while the lower layer is

water

(8p = PI), c = ±

Yg'h.

This speed is that of gravity waves in water of depth

is referred to as the shallow-water wave speed, and (2.99) and (2.101) are

referred to as the

linearized shallow-water equations.

The shallow-water prototype is a useful analog for certain types of atmospheric

motions. However,

is a highly restrictive one since shallow-water waves propa-

gate only horizontally. As the atmosphere is continuously stratified, gravity waves

can propagate vertically as well as horizontally. This fact can be deduced from the

two-dimensional linearized perturbation form of the horizontal vorticity equation

(2.61). If we continue to consider the case of constant mean motion in the

direction only and to disregard friction and diabatic heating, this equation is

(

a _ a

)('

') ,

-+u-

-w

= 0

z x x

while the incompressible (constant density) version of the perturbation thermody-

namic equation (2.80), after multiplying it by

g/Oo' substituting from (2.98), and

ignoring the pressure perturbation, water vapor, and hydrometeor contributions

B in (2.52), becomes

B' + liB' =

-w'B

-w'N

x z

(2.106)

With the aid of (2.106) and (2.100),

and

can be eliminated from (2.105) to

obtain

(

a a

-+li-

(w'

+w'

)+N

2w'

= 0

xx zz xx

(2.107)

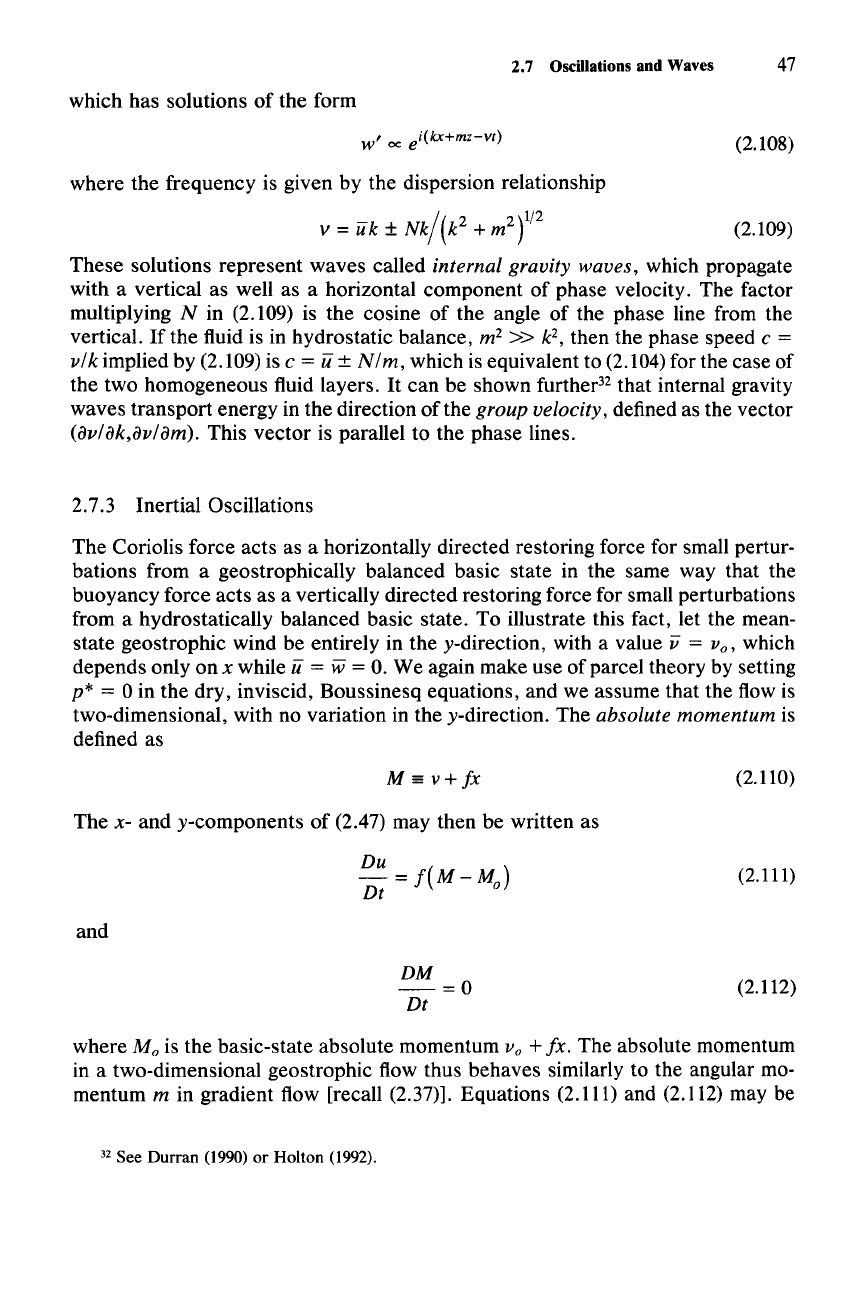
2.7 Oscillations and Waves 47

which has solutions of the form

ei(kx+mz-vt)

(2.108)

where the frequency is given by the dispersion relationship

v = uk±

Nk/

+ m

)1/2

(2.109)

These solutions represent waves called

internal

gravity

waves,

which propagate

with a vertical as well as a horizontal component of phase velocity. The factor

multiplying

N in (2.109) is the cosine of the angle of the phase line from the

vertical. If the fluid is in hydrostatic balance,

» k

, then the phase speed c =

k implied by (2.109) is c = Ii ±

Nlm,

which is equivalent to (2.104)for the case of

the two homogeneous fluid layers.

can be shown further'" that internal gravity

waves transport energy in the direction of the

group

velocity,

defined as the vector

(ovlok,ovlom).

This vector is parallel to the phase lines.

2.7.3 Inertial Oscillations

The Coriolis force acts as a horizontally directed restoring force for small pertur-

bations from a geostrophically balanced basic state in the same way that the

buoyancy force acts as a vertically directed restoring force for small perturbations

from a hydrostatically balanced basic state. To illustrate this fact, let the mean-

state geostrophic wind be entirely in the y-direction, with a value F

vs,

which

depends only on

x while

We again make use of parcel theory by setting

p* = 0 in the dry, inviscid, Boussinesq equations, and we assume that the flow is

two-dimensional, with no variation in the y-direction. The

absolute

momentum

defined as

The x- and y-components of (2.47) may then be written as

Dt =

f(M

-M

)

and

-=0

(2.110)

(2.111)

(2.112)

where

is the basic-state absolute momentum v; + [x, The absolute momentum

in a two-dimensional geostrophic flow thus behaves similarly to the angular mo-

mentum

m in gradient flow [recall (2.37)]. Equations (2.111) and (2.112) may be

32 See Durran (1990) or Holton (1992).

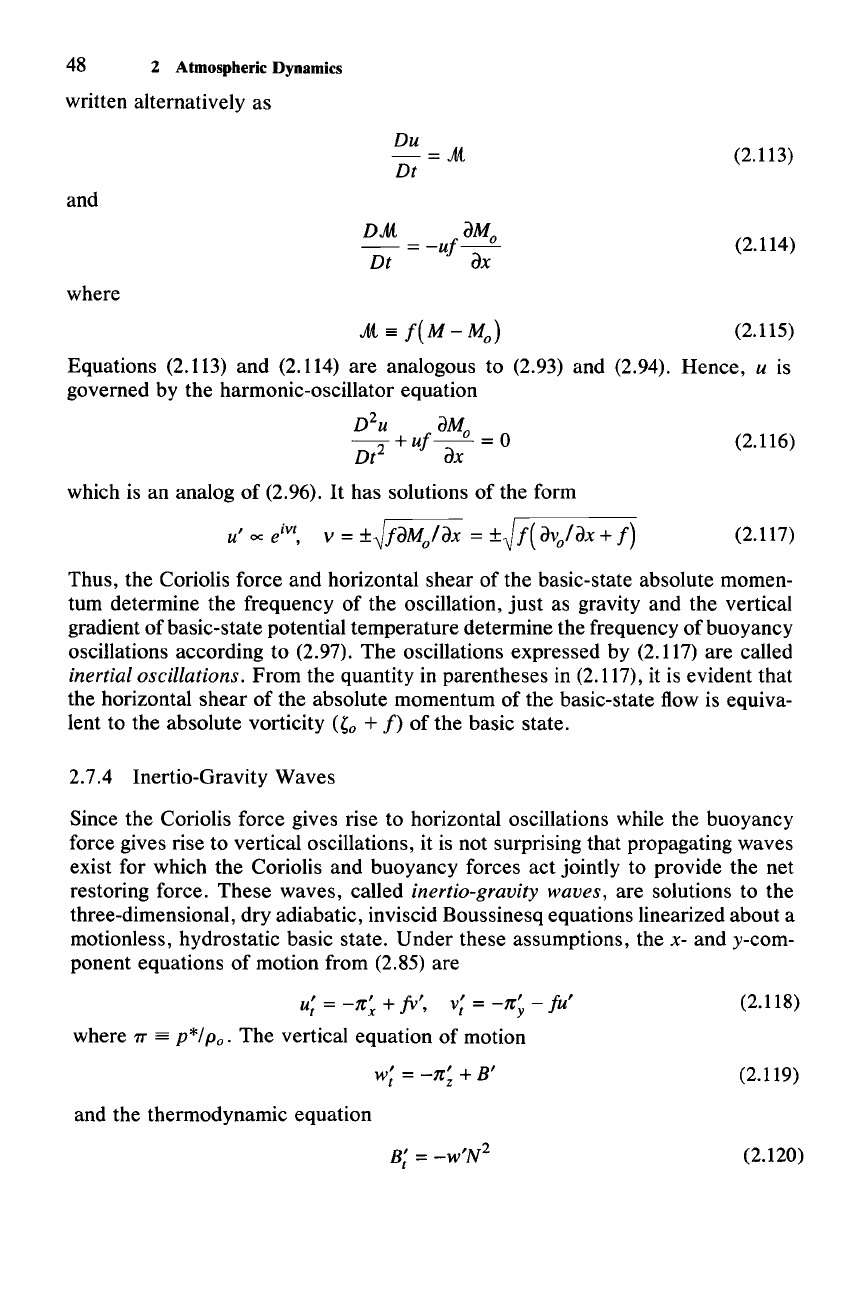
48 2 Atmospheric Dynamics

written alternatively as

and

where

.M,

D.M,

-=-uf-

(2.113)

(2.114)

.M,

M - M

) (2.115)

Equations (2.113) and (2.114) are analogous to (2.93) and (2.94). Hence, u is

governed by the harmonic-oscillator equation

u dM

+ uf

a;-

= 0 (2.116)

which is an analog of (2.96).

has solutions of the form

(2.117)

Thus, the Coriolis force and horizontal shear of the basic-state absolute momen-

tum determine the frequency of the oscillation,

just

as gravity and the vertical

gradient of basic-state potential temperature determine the frequency of buoyancy

oscillations according to (2.97). The oscillations expressed by (2.117) are called

inertial oscillations. From the quantity in parentheses in (2.117), it is evident that

the horizontal shear of the absolute momentum of the basic-state flow is equiva-

lent to the absolute vorticity

('0

of the basic state.

2.7.4 Inertio-Gravity Waves

Since the Coriolis force gives rise to horizontal oscillations while the buoyancy

force gives rise to vertical oscillations, it is not surprising that propagating waves

exist for which the Coriolis and buoyancy forces act jointly to provide the net

restoring force. These waves, called inertia-gravity waves, are solutions to the

three-dimensional, dry adiabatic, inviscid Boussinesq equations linearized about a

motionless, hydrostatic basic state. Under these assumptions, the

x- and y-com-

ponent equations of motion from (2.85) are

-n~

fv',

= -n; - fu'

where 7T

p*/ Po. The vertical equation of motion

-n;

and the thermodynamic equation

B' =

-w'N

(2.118)

(2.119)

(2.120)