Houze Robert A., Jr. Cloud Dynamics
Подождите немного. Документ загружается.

2.1 The Basic Equations 29
(2.15) is along a path in which the air is always maintained at saturation. Numeri-
cal evaluation of the integral shows that a good approximation to (2.15) is
For
unsaturated air we define the equivalent potential temperature as
0e
==
O(T,p)eLqv/CpT.(T,qv,p)
(2.16)
(2.17)
(2.18)
where TsCT,qv,p) is the temperature at which a parcel of air at temperature T,
water vapor mixing ratio
q",
and pressure p would become saturated by lowering
its pressure dry-adiabatically. As defined in (2.17),
(Je
is conserved in dry-adiabatic
motion, since all the variables in the expression on the right-hand side are con-
stant in that case. Moreover, (2.15) and (2.17) are identical at and below the
pressure where saturation is reached. Taking
D(2.16)/Dt, while making the ap-
proximation that
T-\ can be brought outside the integral, verifies that
(Je
is very
nearly conserved under saturated conditions. Thus, regardless of whether or not
the air is saturated, we have
DOe
... 0
Dt
The conservation of
(Je
expressed by (2.18) is often used in place of (2.1l) when
condensation and evaporation are the only diabatic effects. Note also that both
(2.16) and (2.17) are approximated by
0e
"'O(l+Lqv/
cpT)
where it is understood that
a,
= qvs, whenever the air is saturated.
(2.19)
2.1.4 Mass Continuity
In addition to the equation of motion, equation of state, and the First Law of
Thermodynamics, parcels of air obey two mass-continuity constraints. Overall
mass conservation for an air parcel is expressed by the
continuity equation
2.1.5 Water Continuity
Dp
-=-p'V·v
Dt
(2.20)
Conservation of the mass of water in an air parcel is governed by a set of water-
continuity equations
Dqi
--
=
S.,
i = 1,
...
, n
Dt I
(2.21)

(2.22)
30
2 Atmospheric Dynamics
is a mixing ratio
of
one
type
of
water
substance (mass of
water
per
unit
mass of air). In addition to the
vapor
mixing ratio
q.;
there
is a mixing ratio
of
total
liquid
water
and
total ice
content
of
the
air parcel.
The
liquid
and
ice
content
can
be further subdivided into categories according to drop size in the
case
of
liquid
and according to ice particle type
and
size in the
case
of
ice. Since there
are
so
many possible categories
of
water
substance,
we indicate simply
that
there
is
some total
number
of
categories n, including the
vapor
category, and that there
are various sources
and
sinks
of
each
type
of water.
The
sum of
the
sources and
sinks for a particular category
of
water
is indicated by Si.
The
water-continuity
equations (2.21)
are
discussed
further
in Sec. 3.4.
2.1.6
The
Full
Set
of
Equations
Equations (2.1), (2.4), (2.9), (2.20), and (2.21) form a set of equations in v, p, T,
p,
qi,
'ie,
Si,
and
F.
If
'ie,
Si,
and
F
can
be
expressed
in
terms
of
the
other
variables,
then
the
set is a closed
system
of differential equations that
can
be solved for
the
wind, thermodynamic, and
water
variables as functions
of
x, y, z. and t.
2.2 Balanced Flow
Atmospheric motions
often
proceed
through a series of near-equilibrium states.
Although small accelerations are changing
the
flow, the forces in the equation
of
motion are so close to balanced
that
the
instantaneous flow
can
largely be de-
scribed as if the forces
were
in fact balanced. Some basic properties
of
the
types
of
near
force balance
that
occur
in the
context
of
cloud dynamics are summarized in
the following subsections.
2.2.1 Quasi-Geostrophic Motion
Clouds usually involve air motions on the mesoscale
and
convective scale.
How-
ever, it is nonetheless
important
to be cognizant
of
the
dynamics
of
the larger-
scale environment, within which
the
clouds are embedded. Typically, the larger-
scale environment (synoptic scale
and
larger) is in a state
where,
above
the
friction layer,
the
Coriolis
and
horizontal pressure-gradient forces are in a state
of
quasi-geostrophic balance, especially in midlatitudes.
Under
this
type
of
balance,
the horizontal velocity
and
length scales in
both
the x
and
y directions are charac-
teristic
of
the
large-scale atmospheric motion.
If
the
pressure,
height, and vertical
velocity scales
are
also characteristic
of
the
large-scale flow,
then
the horizontal
wind is given, to a first approximation, by its
geostrophic value
1
v
g
=ugi +vgj
==
-(-pyi+
Pxj)
pI

2.2 Balanced Flow
31
which is the horizontal wind for which the Coriolis force in (2.1) would be exactly
balanced by the pressure-gradient force in both the x and y directions.s' The small
acceleration
of
the geostrophic wind components is determined by the Coriolis
force acting on the
ageostrophic part
of
the wind:
Dv
~
=
-fk
x v (2.23)
Dt a
where
(2.24)
(2.25)
and
D
g
a a a
-=:-+u
-+v-
Dt
at
g
ax
gay
andfis
assumed to be constant. In
general,fvaries
with latitude. In this book we
have no need to take this variation into account. When it is necessary to consider
larger scales of motion, for which the variation of
f with latitude must be taken
into account, an equation similar in form to (2.23) is still obtained. However, the
analysis required to obtain the more general version is beyond the scope of this
book.P
While (2.23) illustrates the physical nature of the forcing that changes the
geostrophic wind components, they cannot be solved, as they stand, for the
geostrophic flow, since u and v are not determined. This problem is overcome by
forming a potential vorticity equation, as discussed below in Sec. 2.5.
2.2.2 Semigeostrophic Motions
In the vicinity
of
a front, which is one of the major producers of midlatitude
clouds, the scales
of
motion are quite different in directions taken across and
along the front. As a matter of convention, we take x and u to be in the cross-front
direction and
y and v to be in the along-front direction. If we then let L
ef
and V
ef
be
the cross-front length and velocity scales, respectively, and L
af
and V
af
be the
along-front scales, and assume that
Lcf«
l-«,
V
ef
«
U«,
and
D/Dt
- Vef/Lef,
then the magnitudes of the acceleration in the cross front and along-front direc-
tions, respectively, may be compared to the Coriolis acceleration as follows:
(
DU)/
fv
_
(U~)(
[(.f )
Dt U
af
fL
ef
(~;)/fU
-
(~:J
(2.26)
(2.27)
24 Independent variables used as subscripts indicate partial derivatives. Thus. in (2.22). p,
==
ap/ax.
This shorthand notation will be used frequently throughout the text to indicate partial derivatives.
25 See Holton (1992) for a full discussion of the scale analysis leading to the quasi-geostrophic
equations, as well as various other topics presented in this section.
32 2 Atmospheric Dynamics
If the front is strong, accelerations in the along-front direction are large enough
that Uaf/fLef - 1 and
(~:)/fv«
1
(2.28)
which implies that the v component of the wind is geostrophic. At the same time,
DV!
-
fu-l
Dt
(2.29)
(2.30)
(2.31)
which implies that fu is not in balance with the pressure-gradient acceleration
(i.e.,
U is not geostrophic). In this case, which is referred to as semigeostrophic,
the equation of motion in the along-front direction becomes-"
DAv
g
--=-fu
Dt a
D
A
==
~+(u
+u
)~+
V
~+w~
Dt
at
g a
ax
g
ay
az
This form differs from the geostrophic case in that the ageostrophic circulation
(ua,w), which is directed transverse to the along-front flow, contributes to advec-
tion along with the geostrophic wind. The form of (2.30) and (2.31) can be simpli-
fied mathematically by a special coordinate transformation, which leads to a set of
equations that are of the same form as the geostrophic equations. This coordinate
transformation will be discussed in Chapter 11. As in the geostrophic case, a
potential vorticity equation must be formed to predict the flow (Sec. 11.2.2).
Equations (2.30) and (2.31) are a special case of the more general geostrophic
momentum approximation, according to which motions on a time scale greater
than
l/f
are closely approximated by the vector equation of motion
DV
g
--=-fkxv
Dt a
(2.32)
This relation is an extension of (2.30) since the use of the full total derivative
DIDt,
defined in (2.2), includes advection by the ageostrophic flow in all directions
(U
a,
Va ,w). Equation (2.32) is similar to the geostrophic equation of motion (2.23)
except that ageostrophic advection and vertical advection of geostrophic momen-
tum are
retained." This generalized form of the geostrophic momentum approxi-
mation may also be recast in terms of a coordinate transformation that leads to a
26 For a thorough review of the semigeostrophic approximation in relation to fronts, see Hoskins
(1982).
27 The geostrophic momentum approximation was introduced by Eliassen (1948) and further devel-
oped by Hoskins (1975, 1982). See Bluestein (1986) for a summary of the derivation of the approxi-
mation.
(2.34)
(2.33)
(2.37)
2.2 Balanced Flow 33
set of
semigeostrophic
equations,
which are analogous to the geostrophic equa-
tions.
2.2.3 Gradient-Wind Balance
When horizontal flow becomes highly circular, as in a hurricane, it is convenient
to consider the air motions in a cylindrical coordinate system, with the center of
the circulation as the origin. If the circulation is assumed to be axially symmetric
and devoid of friction, then the horizontal components of the equation of motion
(2.1) are
Du 1 dp v
2
-=---+fv+-
Dt p dr r
Dv uv
-=-fu--
Dt r
where u and v represent the radial and tangential horizontal velocity components
Dr/Dt
and
rD@/Dt,
respectively, where r is the radical coordinate and @ is the
azimuth angle of the coordinate system. The centrifugal term v
2/r
in (2.33) and the
term
-uv/r
in (2.34) are apparent forces that arise from the use of the cylindrical
coordinates.
Gradient-wind
balance
is said to occur when the three forces, pres-
sure-gradient, Coriolis, and centrifugal, are balanced in (2.33). Hurricanes and
other strong vortices on the mesoscale to the synoptic scale tend to be in such a
balance.
It
is convenient to write the equations of gradient-wind balance in terms of the
angular momentum
m about the axis of the cylindrical coordinate system, where
fr
2
m
==
rV
+ - (2.35)
2
In the case of gradient-wind balance
(DulDt
= 0), (2.33) and (2.34) become
1 dp m
2
f2
r
0=---+---
(2.36)
P dr r
3
4
Dm
-=0
Dt
The last relation expresses the conservation of angular momentum around the
center of the circulation. Note that it applies whether or not the circulation is
balanced, as long as it is cylindrically symmetric.
2.2.4 Hydrostatic Balance
The above discussions of geostrophic and gradient flow refer to approximate force
balance in the horizontal. The atmosphere is also often in a state of force balance
in the vertical. When the gravitational term in (2.1) nearly balances the vertical
34 2 Atmospheric Dynamics
component of the pressure gradient acceleration so that both terms are much
larger than the net vertical acceleration and the vertical component of the Coriolis
force, the atmosphere is said to be in
hydrostatic balance. This balance is ex-
pressed by
a<l>
-=-a
ap
(2.38)
where
<I>
is the geopotential, defined as gz + constant. Synoptic-scale and larger-
scale circulations are typically in hydrostatic balance, as are the circulations at
fronts, in hurricanes, and in a variety of other mesoscale phenomena.
Under
hydrostatically balanced conditions, the pressure gradient acceleration in (2.1)
may be written as
1
.
--
Vp =-'\1
<I>
P p
where
(
a
)\
( a
)\
( a
)1
'\1=i-
+j-
+k-
p -
ax
y,p,t
ay
x,p,t
ap
x,y,t
2.2.5 Thermal Wind
(2.39)
(2.40)
(2.41)
When a circulation is in both hydrostatic and geostrophic balance, the winds at
different altitudes are related by the
thermal wind equation,
aV
g
aa aa
j-=-i--j
ap
ay
ax
which is obtained by taking
-i'
a(2.38)/ay + r a(2.38)/ax, substituting from the
geostrophic wind equation (2.22) and making use of (2.39). When a cylindrically
symmetric circulation is in both hydrostatic and gradient-wind balance, the winds
(expressed by
m) at different altitudes are related by the somewhat more general
thermal wind equation:
2m
am
=
7ap
aa
ar
(2.42)
which is obtained by taking
a(2.38)/ar, substituting from the gradient-wind equa-
tion (2.36), and making use of (2.39). From the equation of state (2.4), it is evident
that at a constant pressure level,
a is directly proportional to the virtual tempera-
ture. Hence, the thermal wind relations (2.41) and (2.42) imply that the vertical
shear of the horizontal wind is directly proportional to the horizontal thermal
gradient whenever these balanced conditions apply.
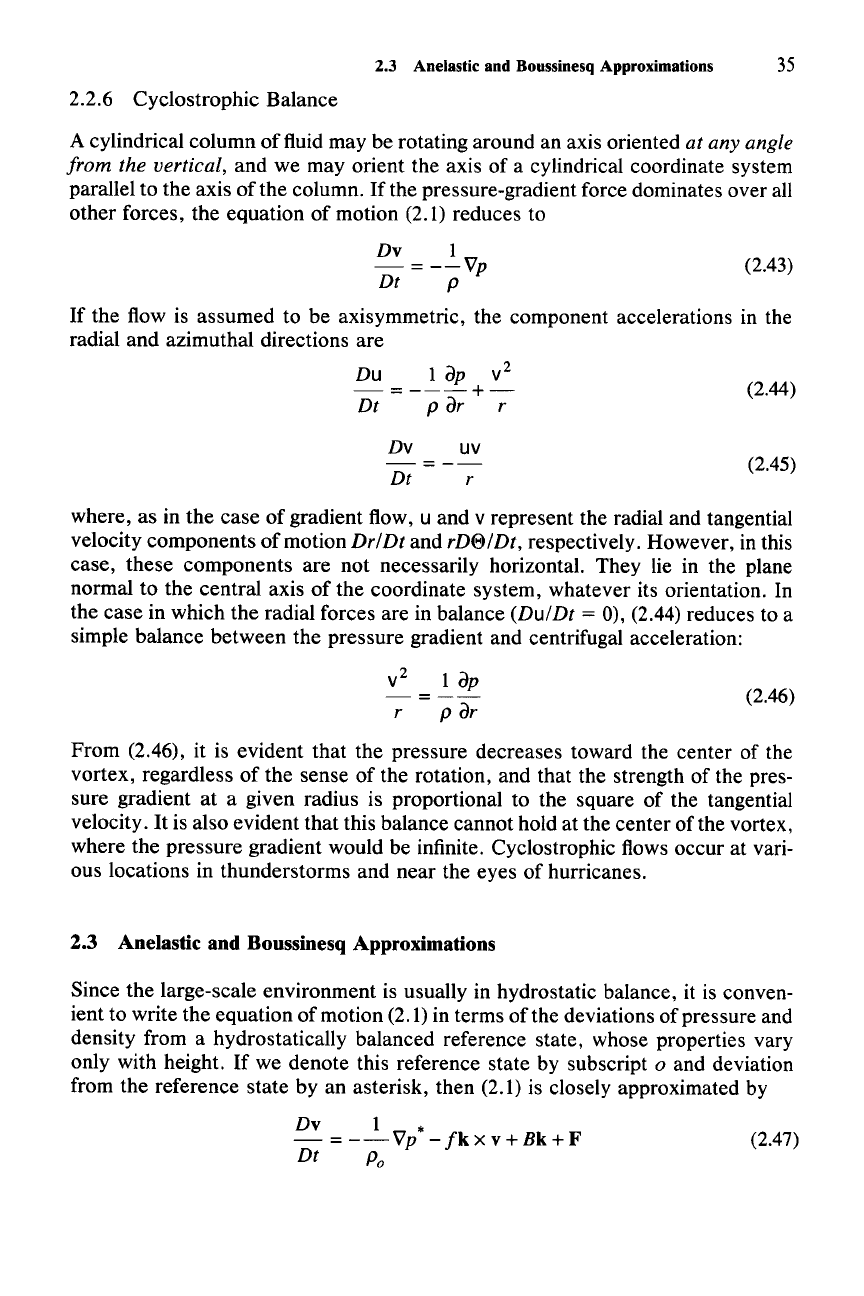
(2.43)
(2.44)
2.3 Anelastic and Boussinesq Approximations
35
2.2.6 Cyclostrophic Balance
A cylindrical column of fluid may be rotating around an axis oriented
at any angle
from
the vertical, and we may orient the axis of a cylindrical coordinate system
parallel to the axis of the column. If the pressure-gradient force dominates over all
other forces, the equation of motion (2.1) reduces to
Dv 1
- =
--Vp
Dt P
If the flow is assumed to be axisymmetric, the component accelerations in the
radial and azimuthal directions are
Du 1
op
v
2
-=---+-
Dt P or r
Dv
Dt
=
uv
r
(2.45)
(2.46)
where, as in the case of gradient flow, u and v represent the radial and tangential
velocity components of motion
Dr/
Dt
and
rDe/
Dt,
respectively. However, in this
case, these components are not necessarily horizontal. They lie in the plane
normal to the central axis of the coordinate system, whatever its orientation. In
the case in which the radial forces are in balance
(Du/Dt
= 0), (2.44) reduces to a
simple balance between the pressure gradient and centrifugal acceleration:
v
2
1
op
=
r p or
From (2.46), it is evident that the pressure decreases toward the center of the
vortex, regardless of the sense of the rotation, and that the strength of the pres-
sure gradient at a given radius is proportional to the square of the tangential
velocity.
It
is also evident that this balance cannot hold at the center of the vortex,
where the pressure gradient would be infinite. Cyclostrophic flows occur at vari-
ous locations in thunderstorms and near the eyes of hurricanes.
2.3 Anelastic
and Boussinesq Approximations
Since the large-scale environment is usually in hydrostatic balance, it is conven-
ient to write the equation of motion (2.1) in terms of the deviations of pressure and
density from a hydrostatically balanced reference state, whose properties vary
only with height.
If
we denote this reference state by subscript 0 and deviation
from the reference state by an asterisk, then (2.1) is closely approximated by
Dv 1 *
- = - - Vp -
fk
x v +
Bk
+ F
Dt
Po
(2.47)
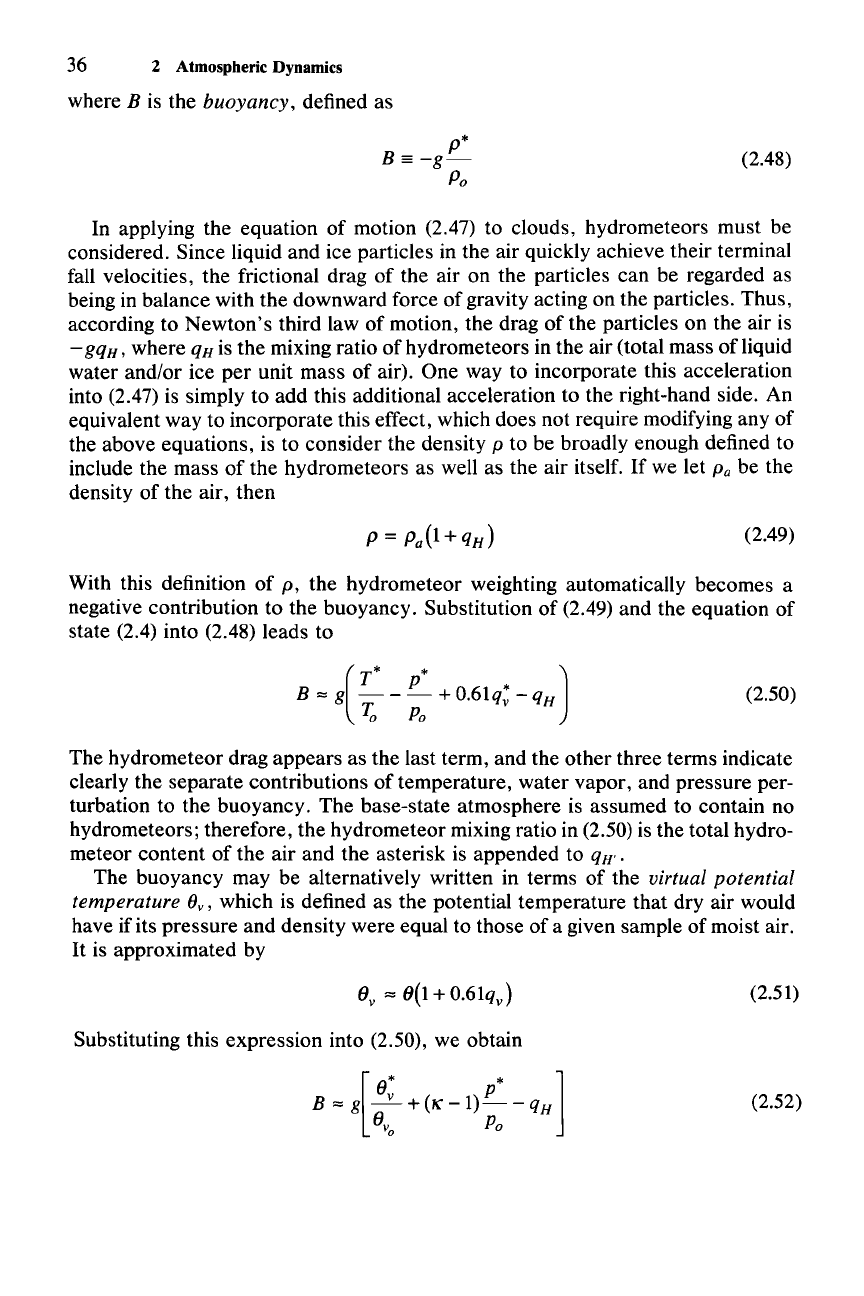
36 2 Atmospheric Dynamics
where B is the buoyancy, defined as
p*
B=-g-
Po
(2.48)
In applying the equation of motion (2.47) to clouds, hydrometeors must be
considered. Since liquid and ice particles in the air quickly achieve their terminal
fall velocities, the frictional drag of the air on the particles can be regarded as
being in balance with the downward force of gravity acting on the particles. Thus,
according to Newton's third law of motion, the drag of the particles on the air is
- gqH, where
qn
is the mixing ratio of hydro meteors in the air (total mass of liquid
water and/or ice per unit mass of air). One way to incorporate this acceleration
into (2.47) is simply to add this additional acceleration to the right-hand side. An
equivalent way to incorporate this effect, which does not require modifying any of
the above equations, is to consider the density
p to be broadly enough defined to
include the mass of the hydrometeors as well as the air itself. If we let
Pa
be the
density of the air, then
(2.49)
With this definition of
p, the hydrometeor weighting automatically becomes a
negative contribution to the buoyancy. Substitution of (2.49) and the equation of
state (2.4) into (2.48) leads to
[
T*
* )
B""g
--L+0.61q~-qH
t;
Po
(2.50)
The hydrometeor drag appears as the last term, and the other three terms indicate
clearly the separate contributions of temperature, water vapor, and pressure per-
turbation to the buoyancy. The base-state atmosphere is assumed to contain no
hydrometeors; therefore, the hydrometeor mixing ratio in (2.50) is the total hydro-
meteor content of the air and the asterisk is appended to
qH'.
The buoyancy may be alternatively written in terms of the virtual potential
temperature
()v, which is defined as the potential temperature that dry air would
have if its pressure and density were equal to those of a given sample of moist air.
It
is approximated by
(2.51)
Substituting this expression into (2.50), we obtain
(2.52)

2.3 Anelastic and Boussinesq Approximations 37
If
we make use of the Exner function defined by (2.14), we may rewrite the
equation of motion as
Dv
*
((]
- =
-c
(J vn -
fk
x v + g - - qH k + F
Dt
P Va (J
Va
(2.53)
In this form the pressure perturbation term disappears from the buoyancy force.
For
this and a variety of other reasons, a system of thermodynamic variables
based on
()v and
Il
instead of T and p is often used in cloud dynamics and other
branches of mesoscale meteorology.
For
most of this book we will use T and p.
The continuity equation (2.20) can be written to a high degree of approximation
as
(2.54)
This form retains the essential density variation with height. The absence of the
time derivative of density eliminates sound waves from the equations, but these
waves are usually of no interest in cloud dynamics. The use of (2.47) and (2.54) in
place of (2.1) and (2.20) is usually referred to as the
anelastic approximation.
Actually, in its purest form, the anelastic approximation requires that the refer-
ence state be isentropic as well as hydrostatic. In practice, the reference state is
often taken to be nonisentropic.
For
example, the assumed reference state might
be that of the environment observed by a radiosonde. When the nonisentropic
form is used, some caution is required because the full set of equations does not
conserve energy exactly. On the other hand, the atmospheric basic state is usually
substantially nonisentropic, and the errors involved in assuming a nonisentropic
basic state do not appear to be especially large."
If the vertical extent of the air motions is confined to a shallow layer,
Pa
can be
replaced by a constant in (2.47) and (2.54). The latter then takes the incompress-
ible form
v'V = 0
(2.55)
The use of (2.47) and (2.55) in place of (2.1) and (2.20) is usually referred to as the
Boussinesq approximation.
For
many applications in cloud dynamics, the Bous-
sinesq equations are either sufficient or easily generalized [through the use of
(2.54) in place of (2.55)] to include compressibility effects without changing the
physical interpretation that can be gleaned from the simpler form. We will fre-
quently use the Boussinesq approximation in this text.
28 For further discussion of these problems, see Ogura and Phillips (1962), who provide a rigorous
derivation of the anelastic equations; Wilhelmson and Ogura (1972), who introduced a commonly used
nonisentropic form and asserted that the nonisentropic form is accurate to within 10%in the context of
a numerical cloud model; and Durran (1989), who has reexamined all the previous forms of the
anelastic approximation and has suggested further improvements to it.

38 2 Atmospheric Dynamics
2.4 Vorticity
An intrinsic characteristic of a fluid is its tendency to rotate about a local axis. The
air motions associated with clouds are particularly prone to rotation, and an
appreciation of fluid rotation is most helpful to understanding the dynamics of
clouds. The local measure of the rotation is the
vorticity, which is defined as the
curl of the wind velocity:
ID
==
V X V =(w
y
-
vz)i
+ (u
z
- wx)j + ( V
x
- uy)k
==
17i
+;j
+
'k
(2.56)
Equations governing time changes of the components of this vector are found by
taking the curl of the Boussinesq equation of motion with frictional forces ne-
glected and making use of
(2.55). The resulting equations are
D17
- = By +
17ux
+ ;u
y
+
(,
+
f)u
z
Dt
D;
=
-B
x
+
;v
y
+
(,
+ iw, +
17
v
x
Dt
(2.57)
(2.58)
(2.59)
D(j
+
,)
=
(j
+
')w
z + (
;w
y
+
17wx)
Dt
where the terms
B,
and By comprise the baroclinic generation of horizontal vortic-
ity. The quantity
(,
+
f)
is the sum of the vertical component 1 of the
earth's
vorticity and the vertical component , of the vorticity of the wind. When the
vertical component of the
earth's
vorticity is added to the vorticity of the wind,
W,
we obtain
IDa
==
17i
+
;j
+
(,
+
f)k
(2.60)
which is called the absolute vorticity, since it incorporates the rotating motion of
the rotating coordinate system. The terms
gv
y,
'T/U
x
,
and (I +
')w
z
in (2.57)-(2.59)
are vortex-stretching terms; they express the concentration of vorticity that oc-
curs when vortex tubes are elongated. The last two terms in each equation [i.e.,
(, +
f)v
z
+
'T/V
x
,
gUy
+ (, +
f)uz,
and
(~Wy
+
'T/W
x
) ]
are tilting terms; they express
the conversion of vorticity parallel to one coordinate axis to vorticity around
another axis as the orientations of vortex tubes change.
Often in cloud dynamics, the scales of phenomena are sufficiently small that the
Coriolis effect is negligible, and
1 disappears from (2.57)-(2.59). We also fre-
quently encounter flows which are quasi-two-dimensional (e.g., fronts and squall
lines). When the flow is two-dimensional in the
x-z plane
and/is
negligible, the
horizontal vorticity-component equation (2.58) simplifies to
J: =
-B
-
uJ:
-
wJ:
or
D;
=
-B
(2.61)
~t
x
~x
~z
Dt x
Thus, in this case, vorticity is generated only baroclinically and is redistributed by
advection in the
x-z plane.
