Houze Robert A., Jr. Cloud Dynamics
Подождите немного. Документ загружается.

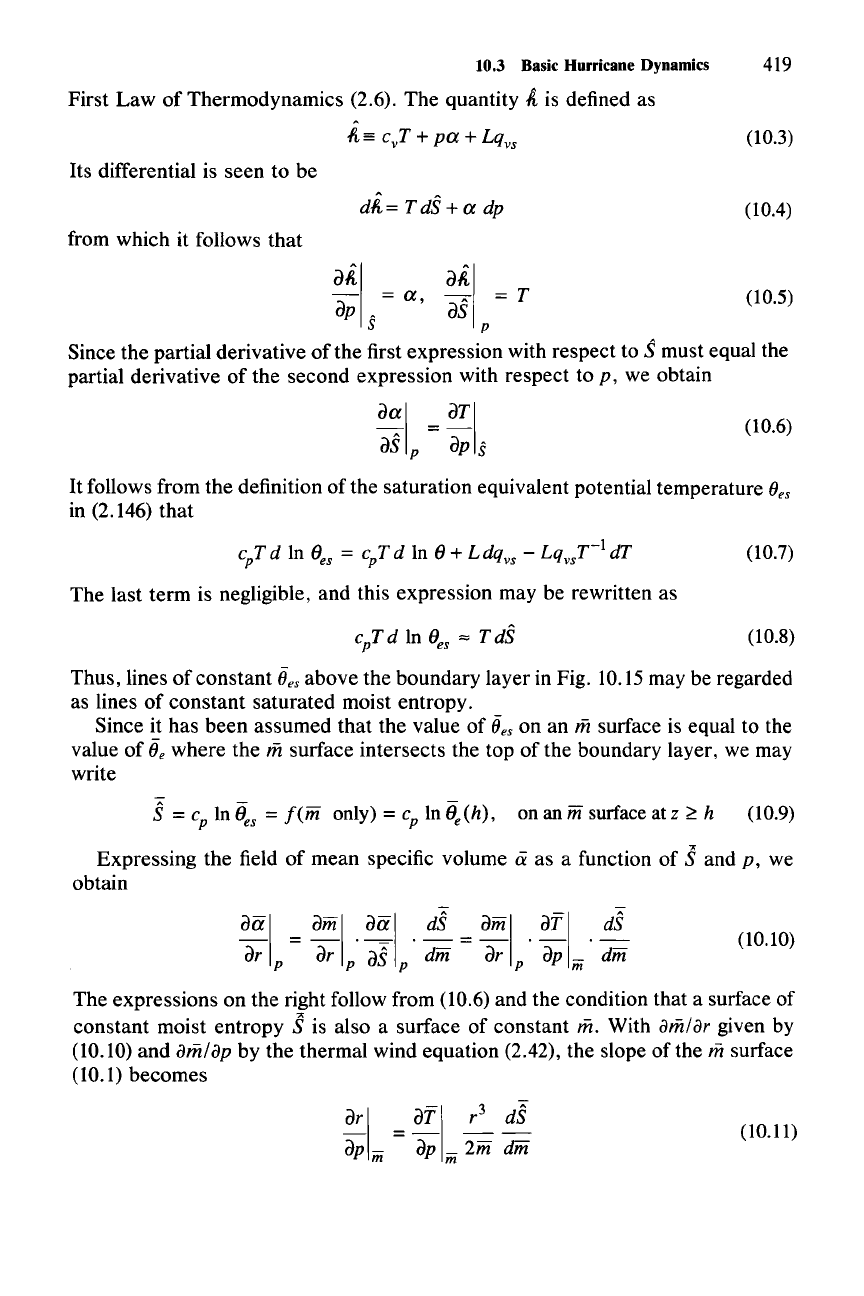
10.3 Basic
Hurricane
Dynamics 419
First Law of Thermodynamics (2.6). The quantity
It is defined as
(10.3)
Its differential is seen to be
(10.4)
(10.6)
(10.10)
from which it follows that
a~ a~
ap
,=
a,
as
= T (10.5)
s p
Since the partial derivative of the first expression with respect to S must equal the
partial derivative of the second expression with respect to
p, we obtain
aal
aTI
as
p =
ap
s
It follows from the definition of the saturation equivalent potential temperature
()es
in (2.146) that
cpT
din
0es
= cpT
din
0+
Ldqvs - LqvsT-1 dT (10.7)
The last term is negligible, and this expression may be rewritten as
cpT d In
0es
""
T dS (10.8)
Thus, lines of constant B
es
above the boundary layer in Fig. 10.15 may be regarded
as lines of constant saturated moist entropy.
Since it has been assumed that the value of
B
es
on an msurface is equal to the
value of
Be
where the msurface intersects the top of the boundary layer, we may
write
S = c
p
In
~s
=
fern
only) = c
p
In
~(h),
on an msurfaceat z
~
h (10.9)
Expressing the field of mean specific volume a as a function of
oS
and
p,
we
obtain
alii
ami
alii
dS
ami
aTI
dS
a;
p =
a;
p'
as
p' dm =
a;
p'
ap
m din
The expressions on the right follow from (10.6) and the condition that a surface of
constant moist entropy
oS
is also a surface of constant
m.
With
amlar
given by
(10.10) and
ami
ap
by the thermal wind equation (2.42), the slope of the m surface
(10.1) becomes
arl
aTI
r
3
dS
-
----
ap
m
ap
m 2m din
(10.11)
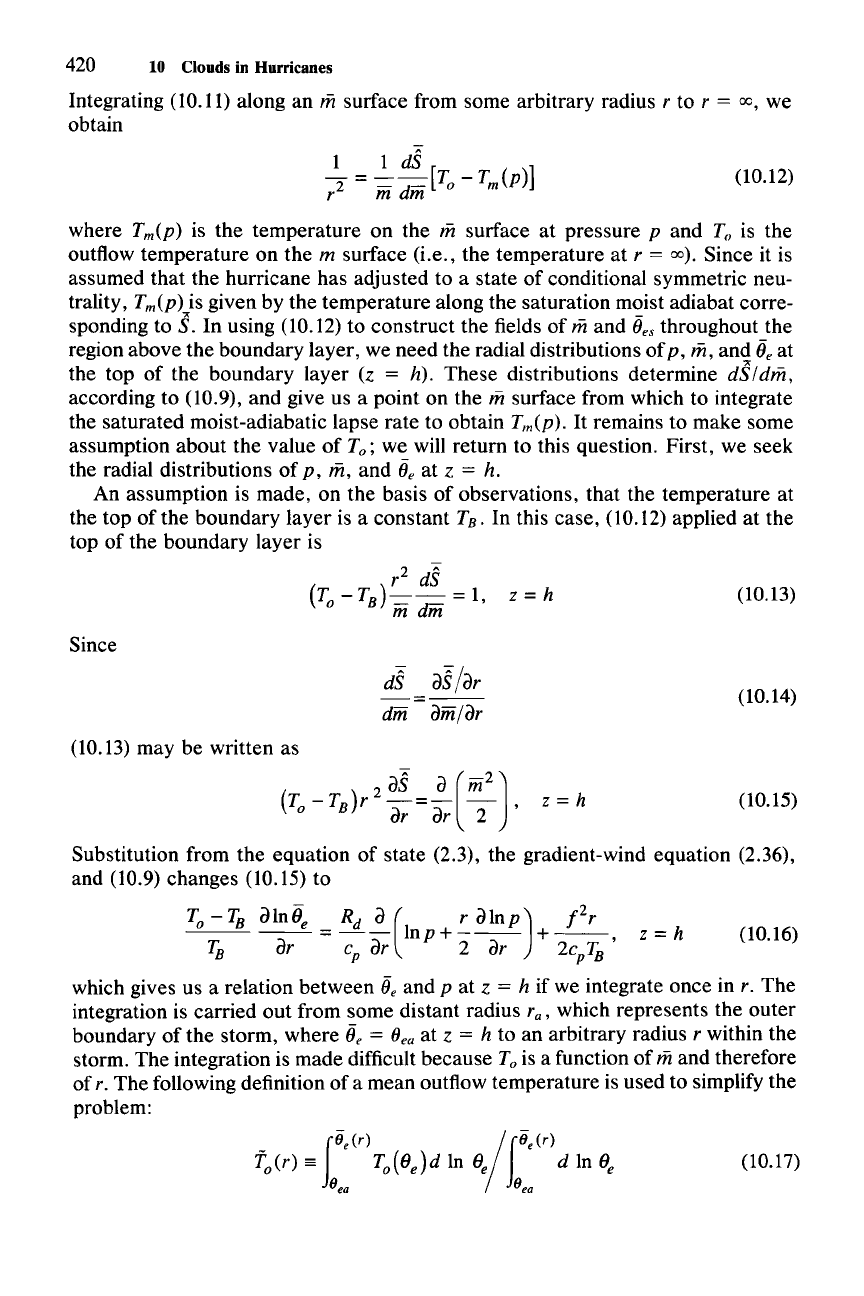
420 10 Clouds in Hurricanes
Integrating (10.11) along an msurface from some arbitrary radius r to r =
00,
we
obtain
lIdS
- =
--[T
-T
(p)]
r
2
m diii 0 m
(10.12)
where Tm(p) is the temperature on the m surface at pressure p and
To
is the
outflow temperature on the
m surface (i.e., the temperature at r =
00).
Since it is
assumed that the hurricane has adjusted to a state of conditional symmetric neu-
trality,
Tm(p) is given by the temperature along the saturation moist adiabat corre-
sponding to
S.
In using (10.12) to construct the fields of mand ii.
s
throughout the
region above the boundary layer, we need the radial distributions of
p,
m,
and ii. at
the top of the boundary layer (z
= h). These distributions determine
dSldm,
according to (10.9), and give us a point on the m surface from which to integrate
the saturated moist-adiabatic lapse rate to obtain
Tm(p).
It
remains to make some
assumption about the value of
To; we will return to this question. First, we seek
the radial distributions of
p,
m,
and ii. at z = h.
An assumption is made, on the basis of observations, that the temperature at
the top of the boundary layer is a constant
Te-
In this case, (10.12) applied at the
top of the boundary layer is
r
2
dS
(To
- T
B
)
m dm = 1, Z = h (10.13)
Since
dS
as/ar
-=--
dm am/ar
(10.14)
(10.13)
may be written as
(To
-
Tn)r
,
~~
=:r (
~').
z = h (10.15)
Substitution from the equation of state (2.3), the gradient-wind equation (2.36),
and (10.9) changes (10.15) to
To
-T
B
alne
e
= R
d
~(lnp+~
alnp)+~
z = h (10.16)
T
B
ar c
p
ar 2 ar
2cp~
,
which gives us a relation between ii. and p at z = h if we integrate once in r. The
integration is carried out from some distant radius
r-,
which represents the outer
boundary of the storm, where
ii. = B
ea
at z = h to an arbitrary radius r within the
storm. The integration is made difficult because
To
is a function of mand therefore
of
r. The following definition of a mean outflow temperature is used to simplify the
problem:
(10.17)
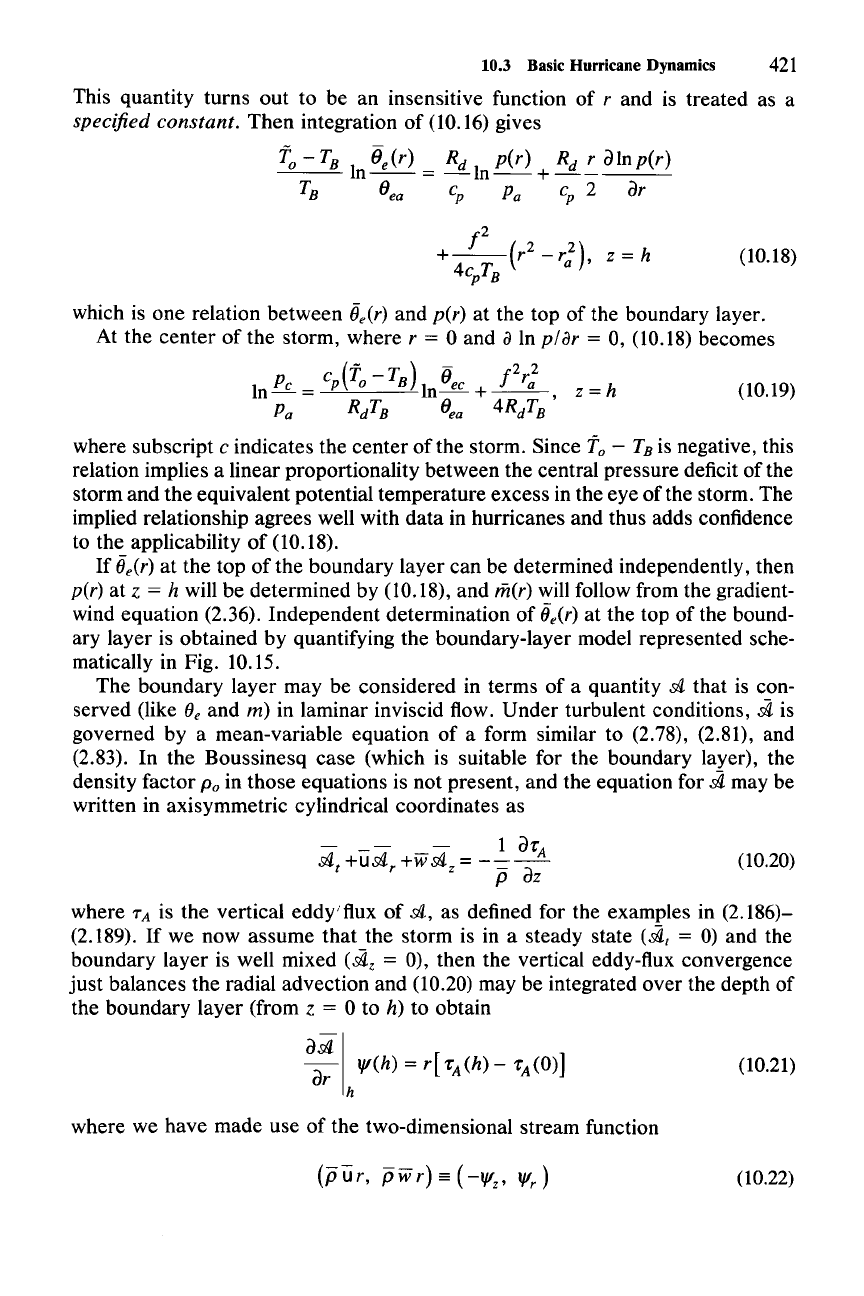
(10.18)
(10.21)
10.3 Basic Hurricane Dynamics 421
This quantity turns out to be an insensitive function of r and is treated as a
specified constant. Then integration of (10.16) gives
i;
- T
B
Oe(r) R
d
p(r)
R
d
r
c)Inp(r)
---"-----'~
In
--
=
-In
--
+ - -
-,------
T
B
(}ea
c
p
o; c
p
2
ar
+L(r
2
-r,n,
z = h
4c
pT
B
which is one relation between iJe(r) and
p(r)
at the top of the boundary layer.
At the center of the storm, where
r = 0 and a In
plar
= 0, (10.18) becomes
c (f T) (j
/2
2
In Pc = p 0 - B
In~
+~,
z = h (10.19)
r;
RdT
B
(}ea
4R
dTB
where subscript c indicates the center of the storm. Since
To
- T
B
is negative, this
relation implies a linear proportionality between the central pressure deficit of the
storm and the equivalent potential temperature excess in the eye of the storm. The
implied relationship agrees well with data in hurricanes and thus adds confidence
to the applicability of
(10.18).
If iJe(r) at the top of the boundary layer can be determined independently, then
p(r)
at z = h will be determined by (10.18), and
m(r)
will follow from the gradient-
wind equation
(2.36). Independent determination of iJe(r) at the top of the bound-
ary layer is obtained by quantifying the boundary-layer model represented sche-
matically in Fig.
10.15.
The boundary layer may be considered in terms of a quantity stl that is con-
served (like
Be
and m) in laminar inviscid flow. Under turbulent conditions,
.sa
is
governed by a mean-variable equation of a form similar to (2.78), (2.81), and
(2.83). In the Boussinesq case (which is suitable for the boundary layer), the
density factor
Po
in those equations is not present, and the equation for
.sa
may be
written in axisymmetric cylindrical coordinates as
- I
a~A
stl +ustl
+wd
=
---
(10.20)
t r z
15
az
where
TA
is the vertical eddy/flux of
d,
as defined for the examples in (2.186)-
(2.189).
If we now assume that the storm is in a steady state
(.sal
= 0) and the
boundary layer is well mixed
(.sa
z
= 0), then the vertical eddy-flux convergence
just balances the radial advection and
(10.20) may be integrated over the depth of
the boundary layer (from
z = 0 to h) to obtain
ad
a;:-
l/I(h) = r[
~A
(h)
-
~A
(0)]
h
where we have made use of the two-dimensional stream function
(10.22)
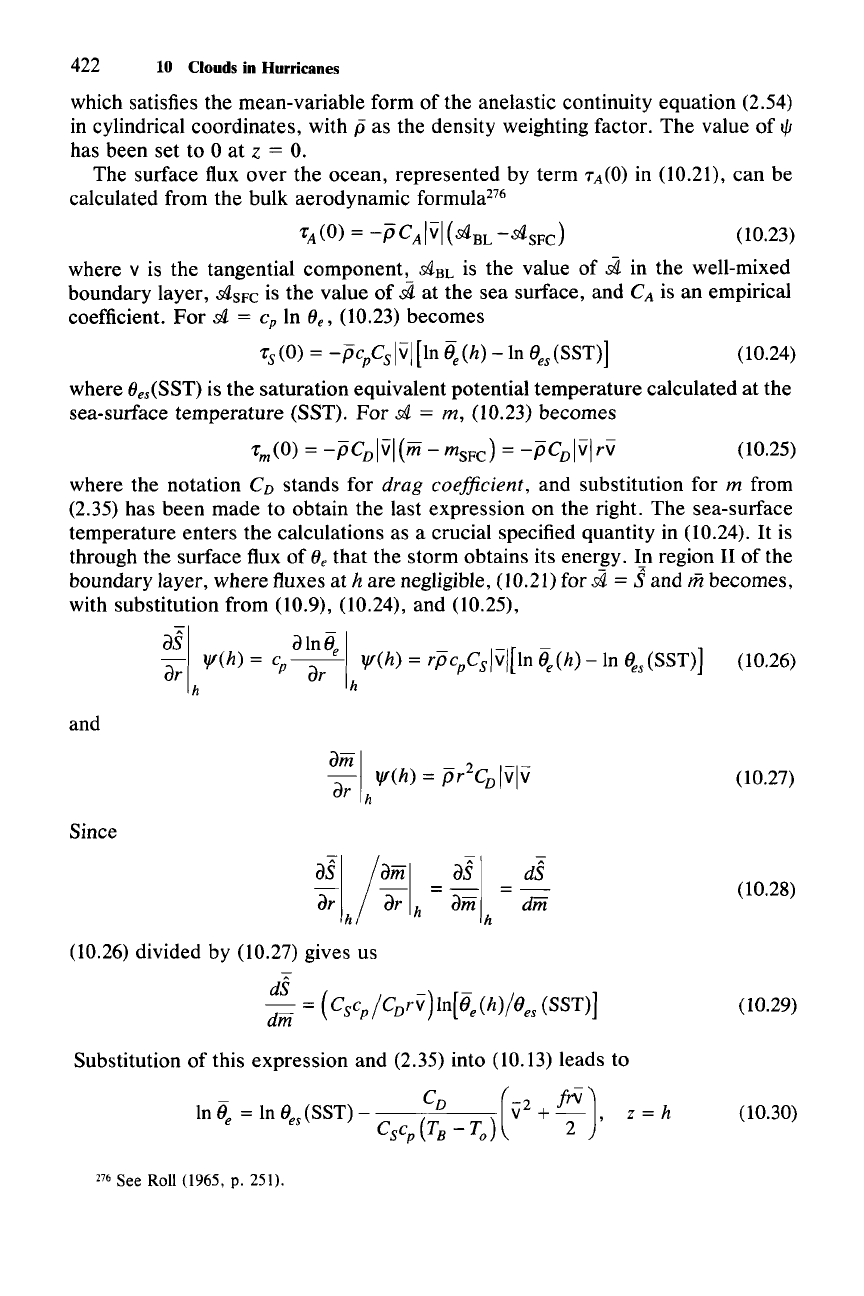
422 10 Clouds in Hurricanes
which satisfies the mean-variable form of the anelastic continuity equation (2.54)
in cylindrical coordinates, with
p as the density weighting factor. The value of
l/J
has been set to 0 at z =
O.
The surface flux
over
the ocean, represented by term
TA(O)
in (10.21), can be
calculated from the bulk aerodynamic forrnula-"
1"A(O)
=
-pCAlvl(sd
BL
-sd
SFC
)
(10.23)
where v is the tangential component, sd
BL
is the value of di in the well-mixed
boundary layer,
sd
SFC
is the value of di at the sea surface, and C
A
is an empirical
coefficient.
For
sd = c
p
In Be, (10.23) becomes
1"s(O)
=
-pcpcslvl[ln
e;,(h)
-In
8
es
(SST )] (10.24)
where Bes(SST) is the saturation equivalent potential temperature calculated at the
sea-surface temperature (SST).
For
sd = m, (10.23) becomes
(10.25)
where the notation
CD stands for drag coefficient, and substitution for m from
(2.35) has been made to obtain the last expression on the right. The sea-surface
temperature enters the calculations as a crucial specified quantity in (10.24).
It
is
through the surface flux of
Be
that the storm obtains its energy. In region II of the
boundary layer, where fluxes at
h are negligible, (10.21) for di =
oS
and
iii
becomes,
with substitution from (10.9), (10.24), and (10.25),
as
alne;,
I
I-If
- ]
ar
h
lfI(h)
=
Cp-----a;-
h
lfI(h)
= rpcpC
s
V
In8
e(h)-ln
~s(SST)
(10.26)
and
ami
2
I-I~
a;:
h
lfI(h)
=
pr
CD
V V
Since
as
jaml
=
as
ar
h
ar
h
am
h
(10.26) divided by (10.27) gives us
:~
= (CScp/CDrv)
In[Oe
(h)/8
es
(SST)]
Substitution of this expression and (2.35) into (10.13) leads to
-
CD
(-2
f
rV
)
In 8
e
= In 8
es
(SST) - ( ) v +
-,
z = h
Csc
p
T
B
-
To
2
276 See Roll (1965, p. 25\).
(10.27)
(10.28)
(10.29)
(10.30)

10.3 Basic Hurricane Dynamics 423
When this expression is substituted into (10.18) for In
Oe
and Ii is expressed in
terms of radial pressure gradient by means of the gradient-wind equation
(2.36),
(10.18) becomes a differential equation for per) at z = h in region II. Thus, (10.18)
and (10.30) form a set of simultaneous equations for
Be
and p at the top of the
boundary layer in region II.
Equation
(10.30) was derived assuming that the fluxes at the top of the bound-
ary layer
[TA
(h)]
are negligible in region II. That such an assumption is inappro-
priate for region III can be seen from the ratio
Oe!Bes(SST)
implied by (10.30). This
ratio is directly proportional to the surface relative humidity.
277
It
can be seen
from
(10.30) that In 0" and hence the surface relative humidity, is a minimum at
the radius of maximum wind, implying that the relative humidity would increase
with increasing radius (and decreasing
Ii) in region III. Observations indicate that
the surface relative humidity is approximately constant in hurricanes, with a value
of about
80%. This result evidently is not given by (10.30) because the eddy flux at
the top of the boundary layer was set equal to zero. In region III, strong down-
drafts are associated with convection in rainbands. These drafts evidently contrib-
ute strongly to the fluxes at the top of the boundary layer and thus keep the
relative humidity lower than predicted by
(10.30).
Therefore, outside the radius of maximum wind (i.e., in region III) the bound-
ary layer is parameterized simply by setting the surface relative humidity empiri-
cally to a constant value of
80%. The radius of maximum wind is then that radius
at which the relative humidity in region II, implied by
(10.30), reaches 80%, and
the value of
Oe
in the boundary layer outside this radius is the value corresponding
to
80% relative humidity. Use of this value of
Oe
in (10.18) then gives an equation
for
per) at z = h in region III, which matches that in region II at the radius of
maximum wind. Since
(10.30) determines per) in region II,
Oe(r),
and mer) at z = h
can now be calculated throughout regions II and III. [The value of mer) is deter-
mined from
per) and the gradient-wind equation (2.36).] Since these quantities are
known at z
= h, (10.12) can be solved for the msurfaces above the boundary layer,
and according to
(10.9) the m surfaces can also be labeled as Ssurfaces corre-
sponding to the value of
Oe
at z = h at the radius where they intersect the boundary
layer. Thus, the streamlines within the eyewall cloud can be constructed if the
sea-surface temperature SST, the outflow temperature
To, the ambient surface
relative humidity
RH
a
(80% in the above discussion), and several less critical
quantities
(!,
Po,
rs,
Cs/C
D
,
and T
B
)
are specified.
The results of an example of this type of calculation are shown in Figs.
10.16-
10.19 for a case in which the sea-surface temperature SST = 300 K, T
B
= 295 K,
To = 206 K, RHo = 80%,f(cP) =
!(28°),
Po = lOIS mb,
r;
= 400 km, and C, = CD.
The central pressure of the model storm is 941 mb, and the maximum tangential
wind component is
58 m
S-I.
To obtain these results, the region II boundary-layer
model was assumed to extend into the eye (region
1). The solutions in region I are
thus not reasonable since in region I the gradient wind balance
(2.36) does not hold
there. This procedure was adopted only for convenience. Since our main purpose
in this chapter is to gain some insight into the nature of the air motions in the
277 See Emanuel (l986a) for details.
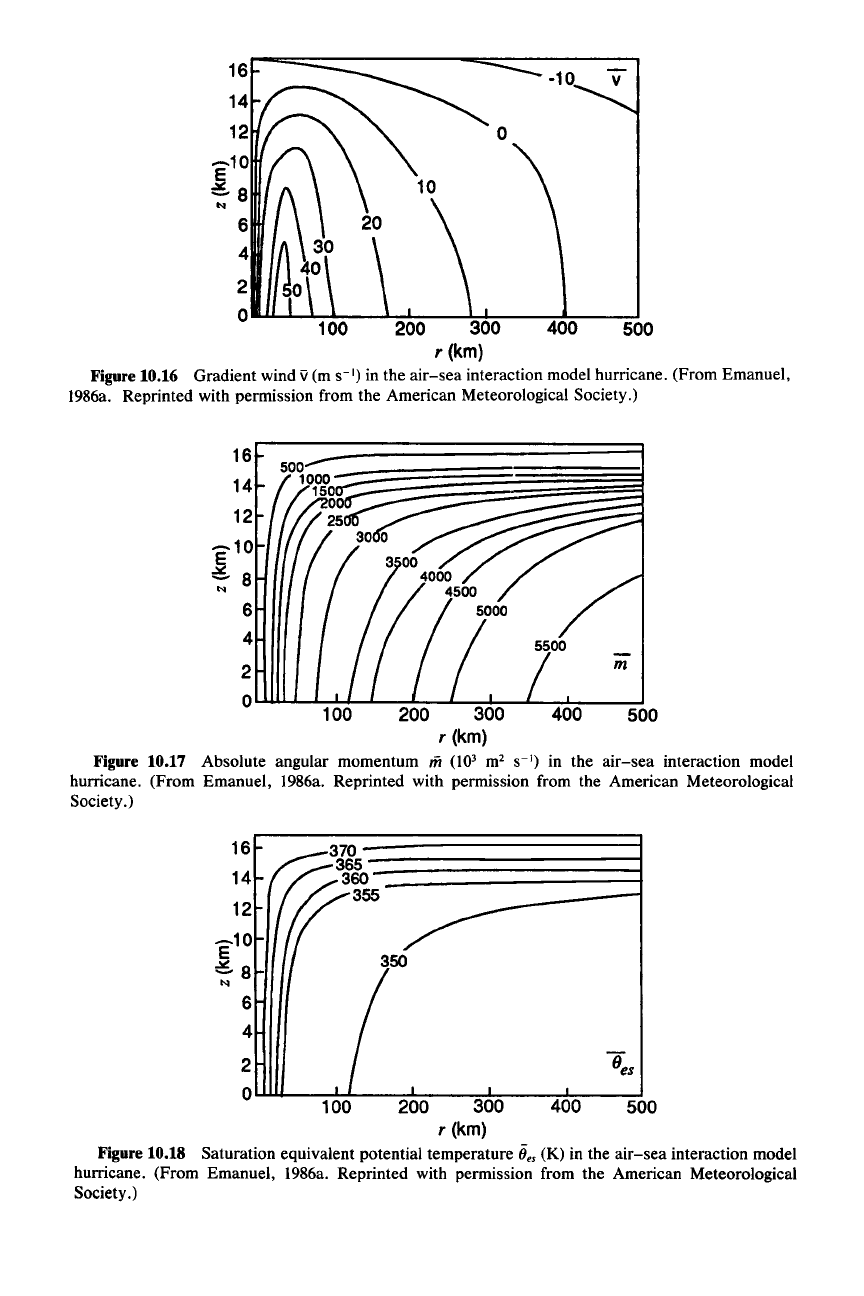
o
500
400
6
4
2
O
................
"""""'":~-"""""'":~----'.::+=--~~-~
300
r
(km)
Figure 10.16 Gradient wind Ii (m
S-I)
in the
air-sea
interaction model hurricane. (From Emanuel,
1986a. Reprinted with permission from the American Meteorological Society.)
500
m
400
1Sr----=====================t
14
12
-10
'&a
..
s
4
2
0~..L..l-...L.:-~.....L..--=~.....L.."""":"::!-=-.....L..~:!-=-_~
300
r
(km)
Figure 10.17 Absolute angular momentum m (10
3
m
2
S-I)
in the
air-sea
interaction model
hurricane. (From Emanuel, 1986a. Reprinted with permission from the American Meteorological
Society.)
500
400
200
350
2
o
u.u'-----:~
_
___::~
_ ___::+=
__
~~~~
1Sr---:;;~================1
14
12
-10
E
~a
..
S
4
300
r
(km)
Figure 10.18 Saturation equivalent potential temperature ii" (K) in the
air-sea
interaction model
hurricane. (From Emanuel, 1986a. Reprinted with permission from the American Meteorological
Society.)

10.4 Clouds and Precipitation in the Eyewall
425
hurricane's clouds, which occur only in regions II and III, the inadequate treat-
ment of the eye dynamics is not a serious deficiency. The primary vortex circula-
tion is shown by Fig. 10.16. The distribution of maximum winds in the eyewall
resembles that seen in Fig. 10.7, although the winds are stronger in the calculated
case than in the composite section, where averaging from many cases has smeared
the basic pattern. The
Iii and B
es
surfaces in Fig. 10.17 and Fig. 10.18 may be
regarded as the streamlines
of
the flow above the boundary layer. The upward and
outward flow demanded by the conservation of
Iii is clearly evident from these
cross sections. That the upward flow is highly concentrated in the eyewall region
is indicated by Fig. 10.19, where the vertical velocity at the top of the boundary
layer implied by the stream function in (10.26) and (10.27) is seen to have a strong
maximum in an annular zone corresponding to the eyewall.
The theoretical picture
just
developed has been further examined by numerical
model simulations, which use a nonhydrostatic cloud model of the type discussed
in Sec. 7.5.3. The model is adapted to the hurricane by formulating it in cylindrical
coordinates and making it two-dimensional by assuming uniformity in the azi-
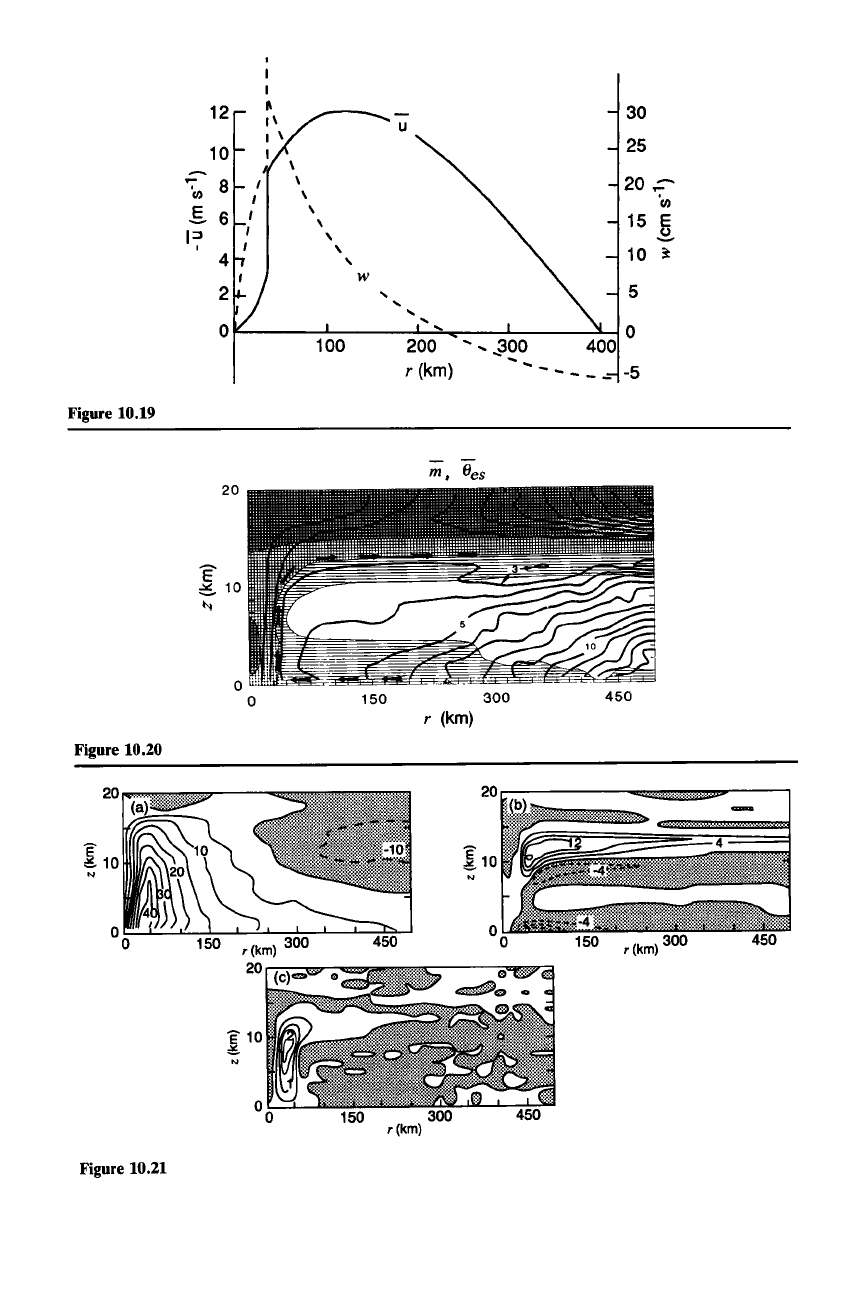
muthal direction. Results from such a model are shown in Fig. 10.20. In this
particular calculation, the undisturbed environment was assumed to be neutral to
conditional instability but unstable in the conditionally symmetric sense. This
assumption represents real hurricane environments fairly well, except that the
latter are not actually neutral but characterized by a small degree of conditional
instability. The numerical experiment was meant to minimize the role of vertical
convection in the development
ofthe
storm. The idea was to see if storm develop-
ment could take place without a conditionally unstable environment. The result
was a rather realistic hurricane vortex. As we will see below, this model storm
was similar to model storms simulated with conditionally unstable environments
and resembles observations of intense hurricanes reasonably well. In Fig. 10.20it
can be seen that, after the model storm became well developed, streamlines in the
eyewall region were parallel to the
Iii and S surfaces. Thus, it is evident that the
eyewall region of the model storm developed as a conditionally symmetrically
neutral circulation connected to a boundary layer in contact with the warm sea
surface.
10.4 Clouds and Precipitation in the Eyewall
10.4.1 Slantwise Circulation in the Eyewall Cloud
As always in this book, our objective here is to gain insight into the air motions
associated with clouds and precipitation. Since, in the hurricane, the clouds are
mainly associated with the eyewall and the rainbands, we devote the last two
sections
ofthis
chapter to these components of the storm. In this section we focus
on the eyewall. Then in Sec. 10.5 we will examine the rainbands.
We begin our discussion of the eyewall clouds by returning to the observations
of the inner-core region of the hurricane (Figs. 10.13 and 10.14) to compare them
E 10
~
"
E
~
10
~
150
300
450
r (km)
Figure 10.20
20
110
E
"
~
10;;
0
00
0
150
r(km) 300
450
I
I
12
~
30
1\
I \
U
10
I
25
-
\
~
8
,
\
20 .::-
'I/)
,
\
'I/)
.s
\
6
,
\
15
E
1::::1
,
,
.2-
I
,
,
10
;t
,
w
2
...
5
...
...
0
...
...
0
100
200
,
,
,3~0
400
r (km)
'--
---
-5
Figure 10.19
m,
fJ
es
20
Figure 10.21

10.4 Clouds and Precipitation in the Eyewall 427
to the theoretical picture developed in Sec. 10.3 (Figs. 10.15-10.20). We have
already noted that at the top of the boundary layer the strong vertical velocity in
the theoretical circulation is concentrated at the location of the eyewall cloud (Fig.
10.19). Airborne Doppler radar observations, such as those illustrated in Fig.
10.13, also show the strong narrow updraft maximum in the eyewall. In addition,
the radar observations indicate that the upward flux occurs in a slantwise pattern.
Thus, the vertical mass transport in the eyewall cloud appears to be dominated by
the momentum-conserving flow described by theory. Further consistency is seen
in the upper-level radial outflow, which in both the theoretical and observed cross
sections occurs in a concentrated layer aloft. The radial outflow is an important
feature
ofthe
cloud and precipitation pattern since it spreads ice particles outward
and thus accounts for the stratiform precipitation region lying typically just out-
side and adjacent to the eyewall convection. The strong tangential circulation
predicted by theory also agrees well with the observed primary circulation, which
in the context of the clouds and precipitation is important in distributing ice
particles around the storm (Fig. 10.14).
10.4.2 Strength of the Radial-Vertical Circulation in the
Eyewall Cloud
The comparisons made in Sec. 10.4.1 show
qualitatively that the air motions
observed in the eyewall region are generally similar to those computed from the
theory of the symmetrically neutral circulation connected to the well-mixed
boundary layer over the warm sea surface. We now explore the nature of the
eyewall motions
quantitatively by inquiring about the strength of the radial-verti-
cal circulation responsible for determining the structure of the eyewall clouds and
precipitation. We proceed by examining the results of both model simulations and
data. We saw at the end of Sec. 10.3 that, when adapted to the hurricane, the
nonhydrostatic cloud model of the type discussed in Sec. 7.5.3 produces a mature
vortex with a conditionally symmetrically neutral eyewall circulation (Fig. 10.20).
A few more results of this simulation are shown in Fig. 10.21. In addition, Fig.
Figure 10.19 Radial distribution of vertical velocity w (ern
S-I)
at the top of the boundary layer and
mean radial velocity (j (m
S-I)
within the boundary layer in the
air-sea
interaction model hurricane.
(From Emanuel, 1986a. Reprinted with permission from the American Meteorological Society.)
Figure 10.20 Results of a two-dimensional nonhydrostatic numerical-model simulation of a
hurricane. Fields were averaged over a 20-h period in which the storm was approximately steady.
Shading indicates equivalent potential temperature values of 345-350 K (horizontal lines), 350-360 K
(medium shading), and >360 K (heavy shading). Absolute angular momentum is shown by contours
labeled in nondimensional units. Also indicated is the airflow through the updraft. (From Rotunno and
Emanuel, 1987. Reprinted with permission from the American Meteorological Society.)
Figure 10.21 Results of a two-dimensional nonhydrostatic numerical-model simulation of a
hurricane. Fields were averaged
over
a 20-h period in which the storm was approximately steady. (a)
Tangential, (b) radial, and (c) vertical wind components (m
S-I)
are shown. Negative values of fields
are indicated by shading. (From Rotunno and Emanuel, 1987. Reprinted with permission from the
American Meteorological Society.)
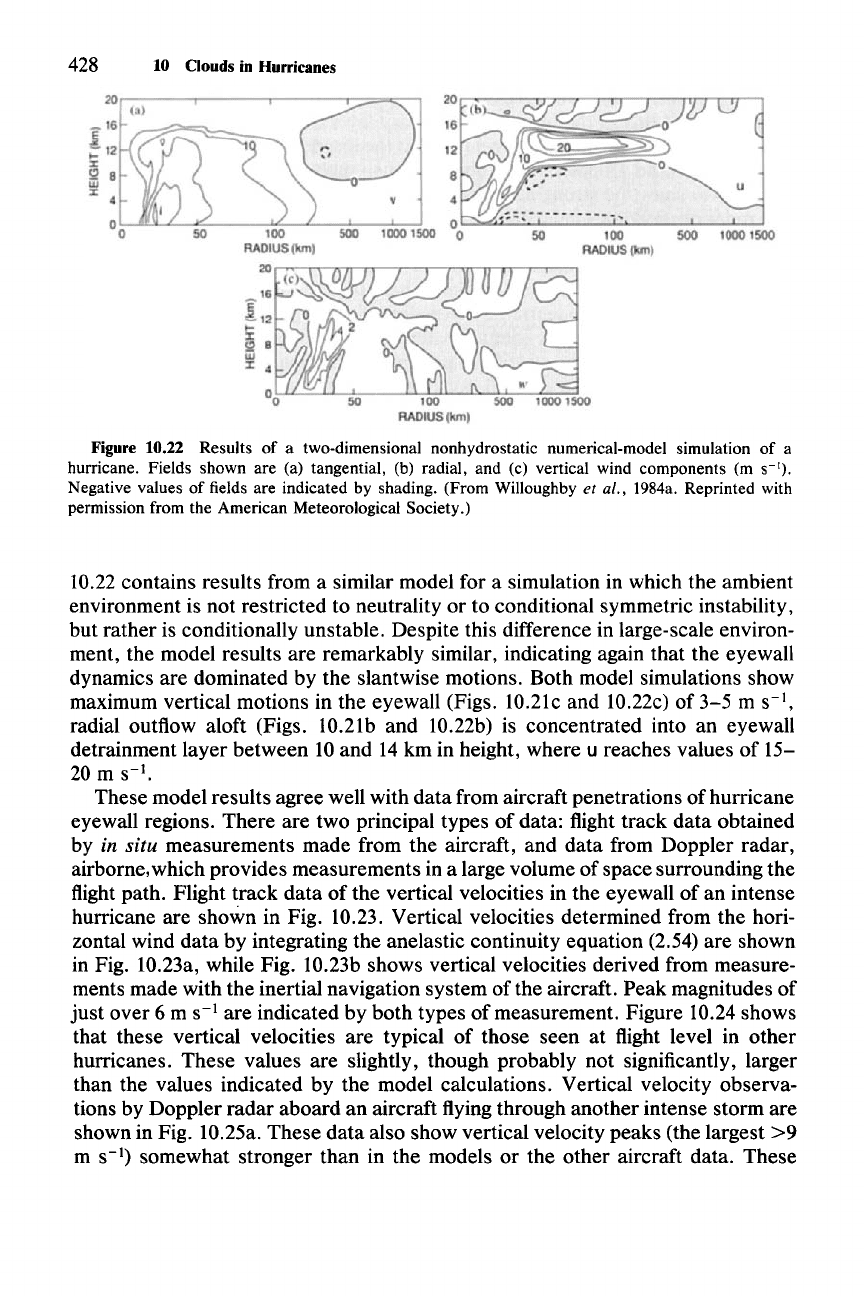
428 10 Clouds in Hurricanes
Figure 10.22 Results of a two-dimensional nonhydrostatic numerical-model simulation of a
hurricane. Fields shown are (a) tangential, (b) radial, and (c) vertical wind components (m
S-I).
Negative values of fields are indicated by shading. (From Willoughby et al., 1984a. Reprinted with
permission from the American Meteorological Society.)
10.22 contains results from a similar model for a simulation in which the ambient
environment is not restricted to neutrality or to conditional symmetric instability,
but rather is conditionally unstable. Despite this difference in large-scale environ-
ment, the model results are remarkably similar, indicating again that the eyewall
dynamics are dominated by the slantwise motions. Both model simulations show
maximum vertical motions in the eyewall (Figs.
1O.21c
and 10.22c)
00-5
m s
",
radial outflow aloft (Figs.
1O.21b
and 10.22b) is concentrated into an eyewall
detrainment layer between 10 and 14 km in height, where
u reaches values of
15-
20 m
S-I.
These model results agree well with data from aircraft penetrations of hurricane
eyewall regions. There are two principal types of data: flight track data obtained
by
in situ measurements made from the aircraft, and data from Doppler radar,
airborne, which provides measurements in a large volume of space surrounding the
flight path. Flight track data of the vertical velocities in the eyewall of an intense
hurricane are shown in Fig. 10.23. Vertical velocities determined from the hori-
zontal wind data by integrating the anelastic continuity equation (2.54) are shown
in Fig. 10.23a, while Fig. 10.23b shows vertical velocities derived from measure-
ments made with the inertial navigation system of the aircraft. Peak magnitudes of
just over 6 m
S-l
are indicated by both types of measurement. Figure 10.24 shows
that these vertical velocities are typical of those seen at flight level in other
hurricanes. These values are slightly, though probably not significantly, larger
than the values indicated by the model calculations. Vertical velocity observa-
tions by Doppler radar aboard an aircraft flying through another intense storm are
shown in Fig. 10.25a. These data also show vertical velocity peaks (the largest
>9
m
S-I)
somewhat stronger than in the models or the other aircraft data. These
