Hillert M. Phase Equilibria, Phase Diagrams and Phase Transformations: Their Thermodynamic Basis
Подождите немного. Документ загружается.


10
Projected and mixed phase diagrams
10.1 Schreinemakers’ projection of potential phase diagrams
Another method of reducing the number of axes is based on projection. By projecting all
the features onto one side of the phase diagram, one will retain all the features, but the fea-
tures of the highest dimensionality will no longer be visible because the dimensionality of
a geometrical element will decrease by one unit by projection and they may thus overlap
each other and also overlap features of the next-higher dimensionality. As an example,
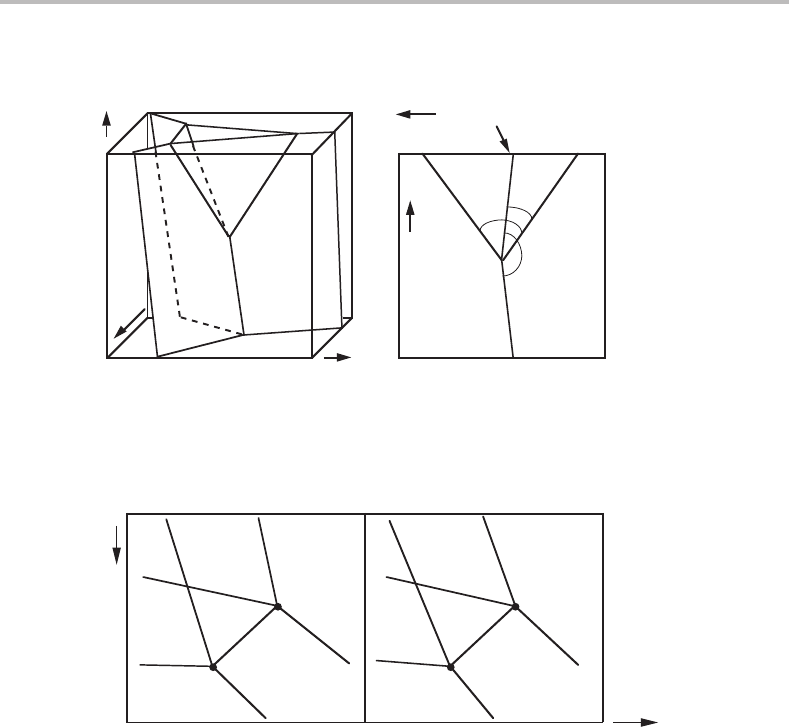
Fig. 10.1(b) shows a P, T diagram obtained by projection of Fig. 8.11 (shown again as
Fig.10.1(a))inthe µ
B
direction. Such a P, T diagram is called Schreinemakers’ projection
[16]. In a system with c components it is obtained by projecting in the directions of c − 1
µ
i
axes. It will show invariant equilibria with c + 2 phases as points, univariant equi-
libria with c + 1 phases as lines and in the angles between them there will be surfaces
representing divariant equilibria with c phases. Using a short-hand notation developed
by Schreinemakers, the coexistence lines for c − 1 phases are here identified also by
giving in parentheses the phases from the invariant equilibrium which do not take part.
Forexample, the (α) curve represents the α-absent equilibrium, i.e. β + γ + δ.Bycom-
parison with Fig. 10.1(a) it can be seen that the angle between (α) and (β)iscovered
by the γ + δ surface but also by the α + δ surface which extends to the (γ) line and by
the β + γ surface which extends to the (δ) line. The α one-phase field covers the whole
diagram and the other one-phase fields each cover part of it.
Suppose we have a binary system with five phases, denoted 1, 2, 3, 4 and 5. An invariant
equilibrium would have four phases. Suppose the system shows two such equilibria and
by giving the absent phase they may be denoted (1) and (5). The complete phase diagram
would have three dimensions (same as for a one-phase field). Projection would give just
one of the diagrams shown in Fig. 10.2 but by presenting two diagrams obtained by
projection in slightly different directions as a stereographic pair one can preserve the
three-dimensional information. It is thus evident that the apparent intersection between
the lines (1, 4) and (5, 3) is not an intersection in three dimensions. Therefore, it does
not represent an invariant equilibrium.
T, P diagrams obtained by projection are particularly useful for multinary systems and
are obtained by projecting in the direction of all the independent chemical potentials. We
shall return to such diagrams in Section 10.5 but first we shall consider simpler cases.
In a projected diagram one sometimes includes a series of parallel sections drawn
with thinner lines. Such lines may be called equipotentials (or isotherms or isobars when
206 Projected and mixed phase diagrams
T
P
µ
B
α
β
γ
δ
P
T
α+β+δ (γ)
β+γ+δ (α)
α+γ+δ (β)
α+β+γ (δ)
α+δ
γ+δ
β+γ
(a) (b)
Figure 10.1 (a) The binary potential phase diagram of Fig. 8.11 reproduced to illustrate the
projection in the µ
B
direction. (b) The diagram obtained by projection. The positions of some of
the two-phase surfaces are shown.
P
T
(5.3)
(1.4)
(5.4)
(5)
(1)
(1.5)
(1.3)
(1.2)
(5.2)
(5.3)
(1.4)
(1.3)
(5.4)
(1.5)
(1)
(5)
(1.2)
(5.2)
Figure 10.2 Stereographic pair of Schreinemakers’ projection of a binary system, showing the
three-dimensional shape. The phases not taking part in an equilibrium are given in parentheses.
It can be seen that lines (1.4) and (5.3) do not intersect.
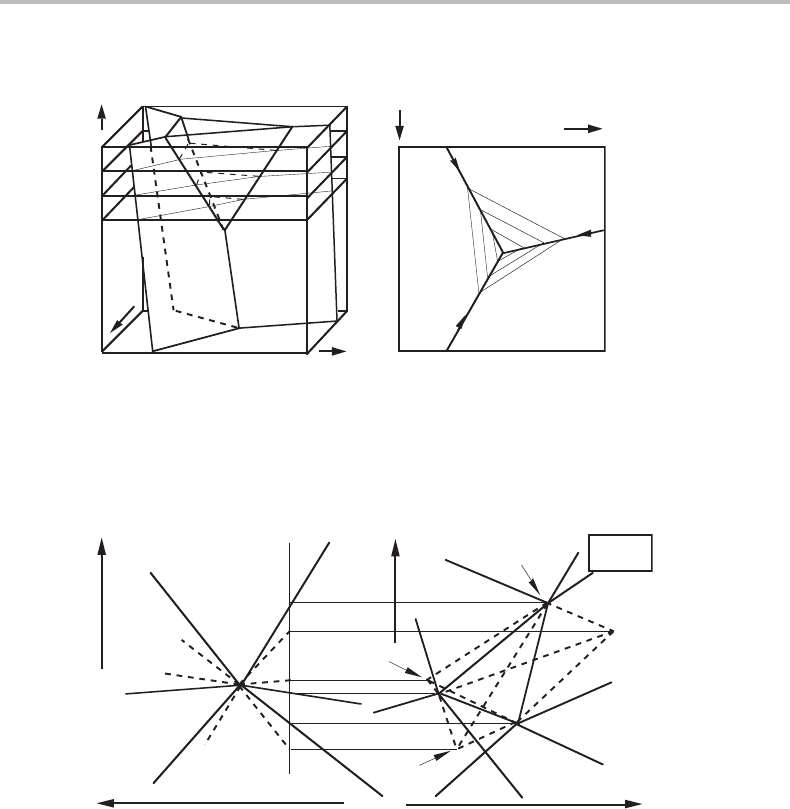
appropriate). Such a section was presented in Fig. 8.12.Figure 10.3(b) shows a diagram
with several parallel sections. In order to simplify this picture, only the equilibria with
the δ phase are shown here. Arrows within the figure show the direction of decreasing
temperature.
Sometimes one uses both projecting and equipotential sectioning in order to reduce
the number of axes. One may be interested in the changes of various phase equilibria
with T and the chemical potential of some volatile component, e.g. oxygen, and one
is willing to limit the information by making an equipotential section at P = 1 bar.
Figure 10.4 gives an illustration from a quaternary system. According to Gibbs’ phase
rule an invariant equilibrium is obtained with c + 2 = 6 phases for c = 4, and six uni-
variant equilibria should radiate from it. Let us denote the phases by 1 to 6. A section
at P = P
1
will cut through the lines (1), (2) and (3). They will thus appear as points in
the right-hand part where one of the projected axes, µ
O
2
,isnow shown. The surfaces
extending between the lines in the T, P diagram (see Fig. 10.1(b)) will appear as lines in
10.1 Schreinemakers’ projection of potential phase diagrams 207
T
P
µ
B
µ
B
α
β
γ
δ
P
1
α
β
γ
2
3
(a) (b)
Figure 10.3 (a) Equipotential sections inserted in the potential phase diagram of Fig. 8.11.
(b) The projection of the same potential phase diagram with inserted equipotential sections of
the two-phase surfaces involving the δ phase.
(1)
(1)
(2)
(2)
(3)
(3)
(4)
(4)
(5)
(5)
(6)
(6)
(1.3)
(2.3)
(2.4)
(2.5)
(2.6)
(3.6)
1
1
1
1
1
2
2
2
2
2
34
3
4
3
3
3
4
4
4
5
5
5
5
6
6
6
6
6
5
T
P
P
1
µ
O
2
P = P
1
T
(a) (b)
(1.5)
(1.4)
(1.6)
(1.2)
(3.4)
(3.5)
Figure 10.4 (a) Schreinemakers’ projection of a quaternary system. (b) Section at P = P
1
. The
new axis, µ
O
2
,isone of those projected in the T, P diagram.
the section. A major difference between the two diagrams is that in (a) all other potentials
were projected but in (b) one of them, P,was sectioned.
Exercise 10.1
Find the section of the 2 + 4 + 5 + 6 equilibrium in the T,µ
O
2
diagram of Fig. 10.4.
Then, find its surface in the T, P diagram.
Hint
How would that equilibrium be denoted using the absent phases?

208 Projected and mixed phase diagrams
Solution
It would be (1.3). In the T,µ
O
2
diagram it is represented by a line between the points (1)
and (3). In the T, P diagram its surface covers the angle between the (1) and (3) lines.
Exercise 10.2
A series of Fe–Cr alloys are heat treated together in a flowing gas of constant C and N
potentials. After heat treatment for a long time at 1273 K, it is sometimes found that
four phases are present but not all in the same specimen. The experiment is repeated
several times with different C and N potentials and in some of those experiments the
four phases are again found. It may be assumed that Cr is not transferred between the
alloys. Is it possible that the four phases are found in more than one experiment, i.e. at
different combinations of C and N potentials?
Hint
We may treat T and P as constant in addition to the potentials of C and N. With four
components we thus have the same situation as in a binary system at variable P and T.
We may use Fig. 10.1(b) and identify µ
B
with µ
Cr
, T with µ
C
and − P with µ
N
.
Solution
It is thus useful to look at a µ
N
,µ
C
diagram obtained by projection in the µ
Cr
direction.
Each experiment should be represented by a point in that diagram but individual spec-
imens would fall on different positions along the projected µ
Cr
axis, which may also
be regarded as a projection along the conjugate molar axis, z
Cr
according to Table 9.2.
Such a diagram is given in Fig. 10.1(b).Wecan see that all experiments falling between
lines (α) and (β)may cut through three two-phase surfaces, together involving all four
phases. With all such combinations of µ
C
and µ
N
we will cut through all four one-phase
fields in Fig. 10.1(a).Four phases can thus be found in several of the experiments with
different values of µ
C
and µ
N
.
10.2 The phase field rule and projected diagrams
In Section 8.5 we derived the phase field rule for equipotential sections. Expressed in
terms of the number of components, c,itwasgivenbyEq. (8.23) and in terms of the
number of axes in the diagram, r,byEq. (8.24)bythe use of r = c + 1 − n
s
. The rule
will now be extended to include projections as well. The dimensionality of phase fields
with a large number of phases will not change their dimensionality by projecting. For
example, the phase field for an invariant equilibrium will still be a point, Eq. (8.23)would
still hold,
d = c + 2 − p − n
s
. (10.1)

10.2 The phase field rule and projected diagrams 209
On the other hand, the dimensionality of the diagram would decrease by each projection,
yielding the following relation,
r = c + 1 − n
s
− n
pr
. (10.2)
Inserting this in Eq. (8.23), here reproduced as Eq. (10.1), we obtain instead of Eq. (8.24),
d = υ − n
s
= c + 2 − p − n
s
= 1 + r − p + n
pr
. (10.3)
The dimensionality of the diagram after a number of projections may have decreased to
the dimensionality of a phase field, i.e. to r = d, and that happens when n
pr
= p −1as
demonstrated by Eq. (10.3). Each further projection will decrease the dimensionalities
of the diagram and the phase field by one unit because a feature in the diagram can never
have a higher dimensionality than the diagram itself. We thus find that
d = r = c + 1 − n
s
− n
pr
for n
pr
≥ p −1. (10.4)
whereas Eq. (10.3) holds for n
pr
≤ p −1.
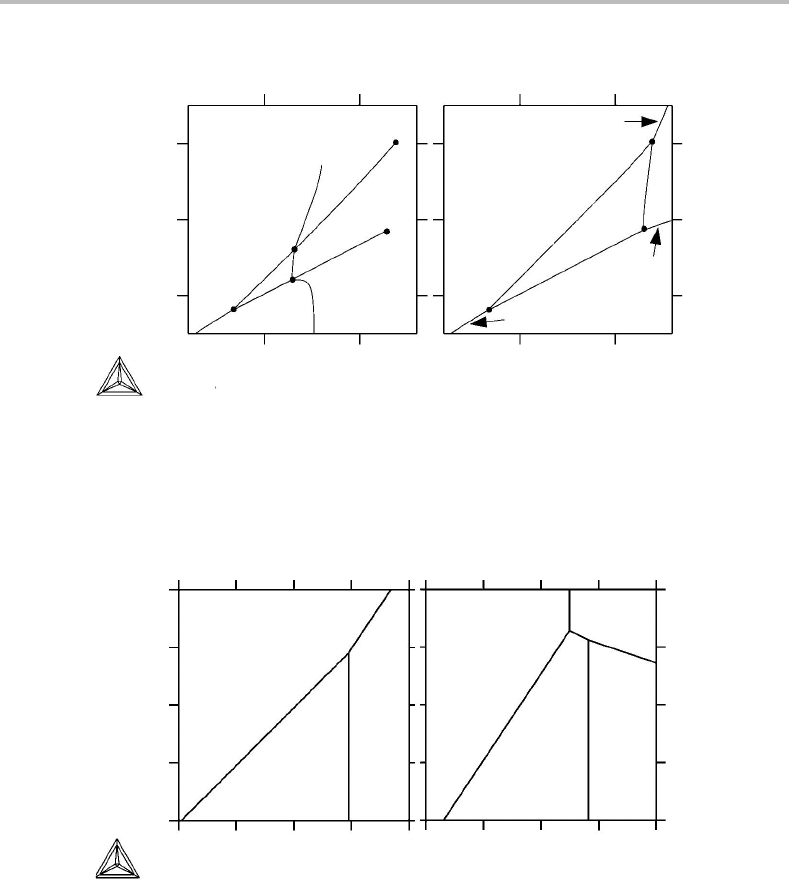
A practical example is given in Fig. 10.5, concerning the Fe–O–S system. Since there
are four lines radiating from each point we may conclude that the invariant equilibria
concern four phases. The phase field rule in Eq. (10.3)gives, for large p,
0 = c + 2 − p − n
s
= 3 + 2 − 4 − n
s
= r + 1 − p + n
pr
= 2 + 1 − 4 + n
pr
n
s
= 1; n
pr
= 1.
It is evident that one has sectioned at a constant value of some potential, probably P at
1 bar. Then one has projected once, in the direction of µ
s
or µ
Fe
.However, it must be
remembered that the complete phase diagram was first obtained from the fundamental
property diagram by projecting in the direction of some µ
i
, the one which was consid-
ered as the dependent variable. Figure 10.5 has thus been obtained from the fundamental
property diagram by projecting twice, and sectioning once, and it does not matter which
projection was made first, µ
s
or µ
Fe
.Itshould be emphasized that n
pr
represents the num-
ber of projections of the complete phase diagram. The first projection of the fundamental
property diagram is not included in n
pr
.
It is interesting to note that the two three-phase lines h (FeO +Fe
3
O
4
+ L) and
f(Fe+ FeO + L) stop inside the diagram. They stop at invariant three-phase points
in the binary Fe–O system which overlaps the diagram. In principle, the whole surface
of the diagram is covered by the binary Fe–O diagram which may be regarded as the
bottom plate of the three-dimensional phase diagram, where µ
s
=−∞, assuming that
the fundamental property diagram was first projected in the µ
Fe
direction to give a phase
diagram. On the bottom plate, there is no S and the number of components c is thus 2
instead of 3. Three-phase equilibria would thus appear as points and two-phase equi-
libria as lines. That bottom plate is shown in (b) but was not included in (a) because
210 Projected and mixed phase diagrams
(a)
−200
−300
−400
1000 1500 1000 1500
(b)
h
h
f
f
d
e
Temperature (K)
RT InP
O
2
(kJ)
Temperature (K)
Fe+Fe
3
O
4
Fe+L
Fe
3
O
4
+L
Fe
+
FeO
FeO +Fe
3
O
4
c
a
b
g
I
II
III
Figure 10.5 (a) Projected and sectioned (P = 1bar) phase diagram for the Fe–O–S system.
(a) Fe + Fe
3
O
4
+ FeS; (b) FeO + Fe
3
O
4
+ FeS; (c) Fe + FeO + FeS; (d) FeO +FeS + L;
(e) Fe + FeS + L; (f) Fe + FeO + L; (g) Fe
3
O
4
+ FeS + L; (h) FeO + Fe
3
O
4
+ L. The invariant
four-phase equilibria are (I) Fe + FeO + Fe
3
O
4
+ FeS; (II) Fe + FeO + FeS + L; (III)
FeO + Fe
3
O
4
+ FeS + L. (b) The Fe–O bottom plate.
5
0
−5
−10
−15
−25 −20 −15 −10 −5 −20 −15 −10 −5
logP
O
2
logP
SO
2
logP
O
2
Cu
2
S
Cu
2
O
Cu
MnS
MnO
MnSO
4
Mn
3
O
4
(a) (b)
Figure 10.6 Potential phase diagram for (a) Cu−O−S and (b) Mn−O−Sat1bar and 1000 K.
These phase diagrams are two-dimensional and are not projections.
it would have made the diagram difficult to interpret. Only the binary end-points for
FeO + Fe
3
O
4
+ L and Fe + FeO + Lwere marked.
In many cases one should consider the top plate as well as the bottom plate. A
log P
SO
2
, log P
O
2
diagram of the Cu–Mn–O–S system under P = 1 bar and T = 1000
Kwould be an example. The top and bottom would represent the Cu–O–S and Mn–O–S
systems, respectively, if the projected axis is taken as (µ
Cu
− µ
Mn
). These diagrams are
given in Fig. 10.6.
The solubilities of Cu in the Mn phases and of Mn in the Cu phases are all very
low. The equilibria between the Cu–O–S phases will not be affected by the presence
10.2 The phase field rule and projected diagrams 211
5
0
−5
−10
−15
−25 −20 −15 −10 −5
MnS + Cu
2
S
+ MnSO
4
MnO+Cu
Mn
3
O
4
+Cu
Mn
3
O
4
+Cu
2
O
Mn
3
O
4
+Cu
2
S
Cu
2
O
MnSO
4
+Cu
2
S
MnS
+Cu
Cu
2
S
+
MnO
logP
O
2
logP
SO
2
Figure 10.7 Projected phase diagram for Cu–Mn–O–S at 1 bar and 1000 K. For clarity, all the
lines from the Cu–O–S side are presented with dashed lines here.
of Mn, nor the Mn–O–S phases by Cu. Both diagrams can thus be plotted in the same
RT ln P
SO
2
, RT ln P
O
2
coordinate frame to form the Cu−Mn−O−S diagram (Fig. 10.7).
The lines in the ternary systems can be copied into the quaternary system and they
become surfaces in the projected direction and still appear as lines in the Cu–Mn–O–S
diagram. New two-phase surfaces form between the previous one-phase fields and they
are identified in the diagram.
An interesting question is the choice of projected axis in Fig. 10.7.Inorder to treat Cu
and Mn in a symmetric way, it is convenient to consider (µ
Cu
+ µ
Mn
)asthe projected axis
to give a phase diagram from the fundamental property diagram and then (µ
Cu
− µ
Mn
)
as the axis used for projection of the phase diagram to reduce the number of axes to two.
Exercise 10.3
Only three lines intersect at the invariant equilibrium I in Fig. 10.5. What line is not
shown and why not?
Hint
The fourth line should be the one without FeS.
Solution
The Fe + FeO + Fe
3
O
4
line is not shown because one has projected the diagram exactly
in its direction and the line thus appears as a point. The reason is that the projection has

212 Projected and mixed phase diagrams
been made in the µ
S
direction and S does not dissolve in any one of these three phases.
Thus, the equilibrium Fe + FeO + Fe
3
O
4
is not affected by S and its line goes exactly in
the µ
s
direction. It exists at a certain T, P
O
2
, only, and it is shown in the binary Fe−O
diagram in Fig. 10.5(b).
Exercise 10.4
At 1000 K one measures the emf of an electrolytic cell where one electrode is a mixture
of MnS, MnO, Cu
2
S and Cu and the other is a mixture of Cu
2
O and Cu. The electrolyte
is solid zirconia stabilized with calcia. Use Fig. 10.7 to estimate the resulting emf.
Hint
The electrical current can pass through this electrolyte mainly by the diffusion of
O
−2
ions. The emf will thus be an expression of the difference in oxygen potential
between the two electrodes and it can be estimated from the difference in RT ln P
O
2
for
two points in the diagram representing the electrodes.
Solution
The point for Cu
2
O + Cu can be taken anywhere on the corresponding line yield-
ing log P
O
2
=−10.2inFig.10.6(a) (also dashed line in Fig. 10.7). The other point
is obtained as the intersection between two lines in the lower left part of Fig. 10.7
and yields log P
O
2
=−21.2. We get µ
O
= 0.5µ
O
2
= 0.5RT (1n P
O
2
− 1n P
O
2
) =
0.5RT 1n 10 ·(−10.2 + 21.2) = 12.7 RT. Remembering that the O ion is divalent we
get E · 27 = µ
o
where 7 is Faraday’s constant (96 486 coulomb/mole) and thus E =
0.547 V.
10.3 Relation between molar diagrams and Schreinemakers’
projected diagrams
As demonstrated byFigs.8.7 and 8.11, the elementary units of potential diagrams are very
simple from the topological point of view. In this sense, the projections of such diagrams
are more interesting. This is evident if one considers the dashed extrapolations shown in
the projected diagram in Fig. 10.8(b). Between the lines there are two extrapolations in
one case, one extrapolation in two cases, and no extrapolation in one case. In fact, this
is the only way to draw four lines in different directions if the 180
◦
rule is to be obeyed.
It is evident that, in the projected direction, the four phases are related to each other in a
special way. This phenomenon will now be examined. In order to simplify the discussion
the method of identifying a univariant line by giving within parentheses the absent phase
is used in Fig. 10.8(b).
If potential axes are chosen for plotting the complete, three-dimensional phase diagram
of a binary system, the four phases of an invariant equilibrium will fall on one point. If
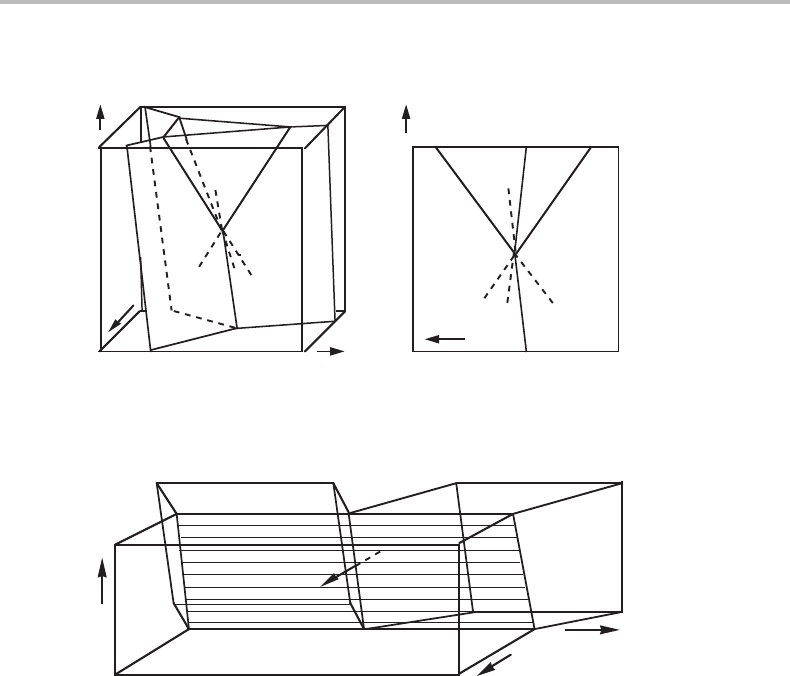
10.3 Relation between molar and Schreinemakers’ projected diagrams 213
T
P
µ
B
α
β
γ
δ
(γ)
(α)
(β)
(δ)
P
T
(a) (b)
Figure 10.8 (a) Binary phase diagram and (b) projection in the µ
B
direction, taken from
Fig. 10.1. The extrapolations of the three-phase lines are marked with dashed lines.
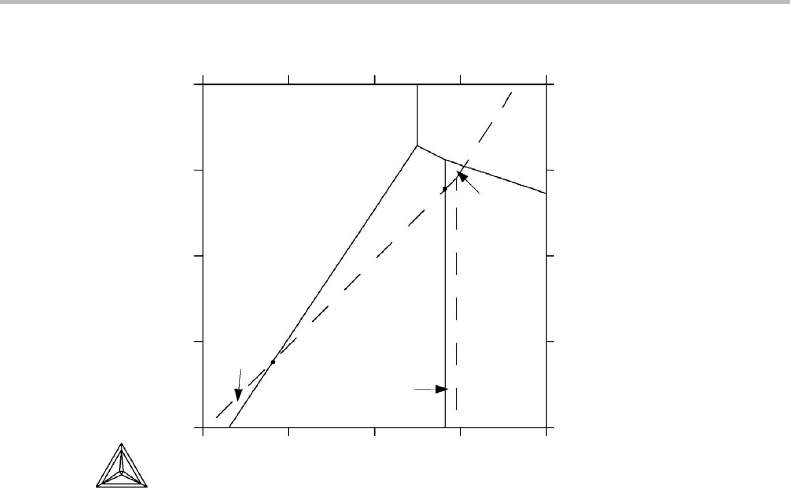
T
P
z
B
α
β
γ
Figure 10.9 Introduction of a molar axis into the potential diagram of Fig. 10.8. Only the lower
half of that diagram is used here. The surface marked with horizontal tie-lines represents the
α + β + γ equilibrium.
one molar quantity is introduced, say, instead of Y
j
, then the four phases will fall on a
line, just as the three phases in the three-phase equilibrium in Fig. 9.2(a) fall on a line
in Fig. 9.2(b).Inthat case, it is easy to see the order in which the phases are arranged
along the line. The hcp phase must be placed between bcc and fcc. Otherwise, there
would be some overlapping of the one-phase fields which is not allowed according to
Section 9.1. Using the same reasoning, it is easy to see the order in which the four phases
of Fig. 10.8 will be arranged when a molar quantity is introduced. One simply looks at
the direction of the two-phase surfaces. Each one will turn into a two-phase volume when
z
B
is introduced instead of the µ
B
axis. In Fig. 10.9 these volumes are demonstrated for
the three surfaces between α, β and γ.Itisevident that γ must fall between α and β
along the z
B
axis.
When the phase diagram of Fig. 10.8(a) is projected in the µ
B
direction and
Fig. 10.8(b) is formed, much information is lost. However, the information regarding the
order of arrangement along the projected direction, obtained when the molar quantity is
introduced, is not lost. This is because some conclusions can still be drawn regarding the
directions of the two-phase surfaces. They are situated between the three-phase lines.
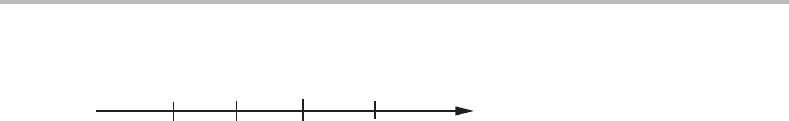
214 Projected and mixed phase diagrams
α
β
δ
z
B
γ
Figure 10.10 One-dimensional molar phase diagram at constant T and P, showing the relative
position of four phases along the z
B
axis, introduced instead of a µ
B
axis, through the invariant
equilibrium in Fig. 10.8(a). The composition is here expressed with the z
B
variable because it is
the conjugate variable to µ
B
according to Table 9.2. The relative positions of all the four phases
along a z
B
axis, going through the invariant phase equilibrium are demonstrated schematically in
Fig. 10.3.
This was demonstrated in Fig. 10.1(a).Itisthus possible to get an impression of the
relative positions of the six surfaces and thus of the relative positions of the phases along
the molar axis of the projection.
The simplest method to interpret an experimental diagram like Fig. 10.8(b) is to
draw the four extrapolations and then turn the diagram in the same way as Fig. 10.8(b)
with respect to the dashed extrapolations. The compositions of the phases will then be
arranged in the order given by Fig. 10.10 or in the completely reverse order. A more
logical method will be described in the following section.
Exercise 10.5
In Exercise 10.2 we considered a heat treatment of several Fe–Cr specimens under
carburizing and nitriding conditions at constant T and P. It had been found that four
phases could be present in some experiments but not in the same specimen. Now try to
find what is the maximum number of phases in any one specimen.
Hint
As already explained, we can use Fig. 10.1(b) because our quaternary system at constant
T and P behaves like a binary system at variable T and P.Inour case the two axes should
be µ
C
and µ
N
and the projection has been made in the direction of µ
Cr
,which is the
same as the direction of z
Cr
.
Solution
In Exercise 10.2 we saw that four phases can be present if µ
C
and µ
N
fall between
the lines (α) and (β)inFig. 10.1. The specimens fall on different positions along the
projected axis. Most of them may fall between the surfaces representing two phases and
they will thus have only one phase. What is the chance that some fall on the two-phase
surface? Since the specimens are defined by their contents of Cr we should regard the
projected molar axis rather than the potential axis. The two-phase surface in the potential
diagram has a thickness when the molar axis has been introduced. There is thus definite
chance that a specimen falls within the two-phase region.
