Hassani S. Mathematical Methods: For Students of Physics and Related Fields

Подождите немного. Документ загружается.

452 Tensor Anal ysi s

Symmetrization

Some important tensors in physics have the property that when two of its

symmetric and

antisymmetric

tensors

indices are interchanged the tensor does not change or it changes sign. In the

ﬁrst case, we say that the tensor is symmetric, in the second case, antisym-

metric. For example, if T is a tensor of type (2, 0) and U of type (0, 2), and if

= T

and U

= −U

then T is symmetric and U is antisymmetric.

Given any tensor, one can always construct from it a tensor which is

symmetric or antisymmetric in the interchange of any pair of its indices. In

particular, if T is any tensor of type (2, 0), then the tensors S and A with

components

+ T

)andA

− T

)

are called the symmetric and antisymmetric parts of T,and

+ T

− T

) ≡ S

+ A

. (17.27)

The symmetric part S

is sometimes denoted by T

(ij)

and the antisymmetric

part A

by T

[ij]

17.2.2 Numerical Tensors

There are certain “constant” tensors which play important roles in tensor

analysis. We have seen one such tensor already: the (1, 1)-type Kronecker

delta. In fact, all the so-called numerical tensors are built form this funda-

mental tensor. The generalized Kronecker delta δ

···i

···j

is deﬁned asgeneralized

Kronecker delta

···i

···j

=det

⎛

⎜

⎝

··· δ

⎞

⎟

⎠

. (17.28)

The determinant of an r × r matrix is a sum of terms each consisting

of the product of r matrix elements. In (17.28), each term is a product of

r Kronecker deltas. Since the Kronecker delta is a (1, 1)-type tensor, each

term, thus the determinant, and thus the generalized Kronecker delta, is an

(r, r)-type tensor.

It is clear from (17.28) that the upper indices label the rows and the lower

indices the columns of the matrix. Thus interchanging any two of the upper

indices is equivalent to interchanging two rows of the matrix. This changes

the sign of the determinant. Similarly for the interchange of two columns.

17.2 From Vectors to Tensors 453

Box 17.2.4. The generalized Kronecker delta is a completely antisym-

metric tensor in its upper and lower indices: interchanging any two of its

upper indices or any two of its lower indices changes its sign.

Example 17.2.4. In this example, we demonstrate a useful property of the gen-

eralized Kronecker delta. We illustrate the property for r =3andn =3,

but the

result can easily be generalized. Expand the determinant of δ

ijk

lmp

about the last row

starting from the right:

ijk

lmp

=det

⎛

⎝

⎞

⎠

= δ

det





− δ

det





+ δ

det





= δ

− δ

+ δ

Now contract over the indices k and p to obtain

ijk

lmk

= δ

−δ

+δ

=3δ

−δ

+δ

=2δ

+δ

= δ

−δ

where in the next to the last step we used the antisymmetry of the generalized Kro-

necker delta. Note that because of the antisymmetry of the generalized Kronecker

delta in both upper and lower indices, we can move both the upper and the lower

last indices to the beginning:

···i

···j

= δ

···i

r−1

···j

r−1

In particular,

kij

klm

= δ

ijk

lmk

= δ

− δ



The procedure of the example above can be generalized to arbitrary r and

n. Furthermore, one can contract over more than one pair of indices. The

result is the following useful identity:

···i

s+1

···i

···j

s+1

···i

(n − s)!

(n −r)!

···i

···j

. (17.29)

From the generalized Kronecker delta two other important numerical ten-

sors are built. These are called the Levi-Civita symbols. They are deﬁned

Levi-Civita

symbols

as follows:



···j

= δ

12···n

···j

and 

···i

= δ

···i

12···n

. (17.30)

Note that both Levi-Civita symbols are antisymmetric in all their indices and

will thus vanish if any two of their indices are equal. Moreover,



12···n

= δ

12···n

=1 and 

12···n

= δ

12···n

=1, (17.31)

so that we have



···i

= 

···i

⎧

⎪

⎨

⎪

⎩

+1 if i

···i

is an even permutation of 1,2,. . . n,

−1ifi

···i

is an odd permutation of 1,2,. . . n,

0otherwise.

(17.32)

Recall that n is the dimension of the space.

454 Tensor Anal ysi s

Now consider the quantity

···i

···j

= 

···i



···j

− δ

···i

···j

which is clearly antisymmetric in all its upper as well as lower indices. This

means that the only nonzero elements of A

···i

···j

are those obtained from

12···n

. But this is zero by (17.31) and the deﬁnition of A

···i

···j

.Wehavejust

shown the following important result



···i



···j

= δ

···i

···j

. (17.33)

17.3 Metric Tensor

Let {x

i

} denote a set of Cartesian coordinates, and {x

} some other coordi-

nates of which {x

i

} are functions. We then have

i

∂x

i

∂x

(sum over j implied as usual).

The element of length (squared)—which is customarily denoted by ds

—in

the Cartesian coordinate system is

=(dx

1

)

+(dx

2

)

+ ···+(dx

n

)



i=1

(dx

i

)

In terms of the other coordinates, this can be written as



i=1

(dx

i

)



i=1

i



i=1



∂x

i

∂x



∂x

i

∂x







i=1

∂x

i

∂x

i

∂x



The expression in parentheses on the last line, denoted by g

(x), is a sym-

metric tensor of type (0, 2), which as indicated, is a function of the {x

(x)=



i=1

∂x

i

∂x

i

∂x

. (17.34)

That g

(x) is symmetric should be obvious. To show that it is a tensor

of type (0, 2), let {¯x

} be some new set of coordinates of which {x

i

} are

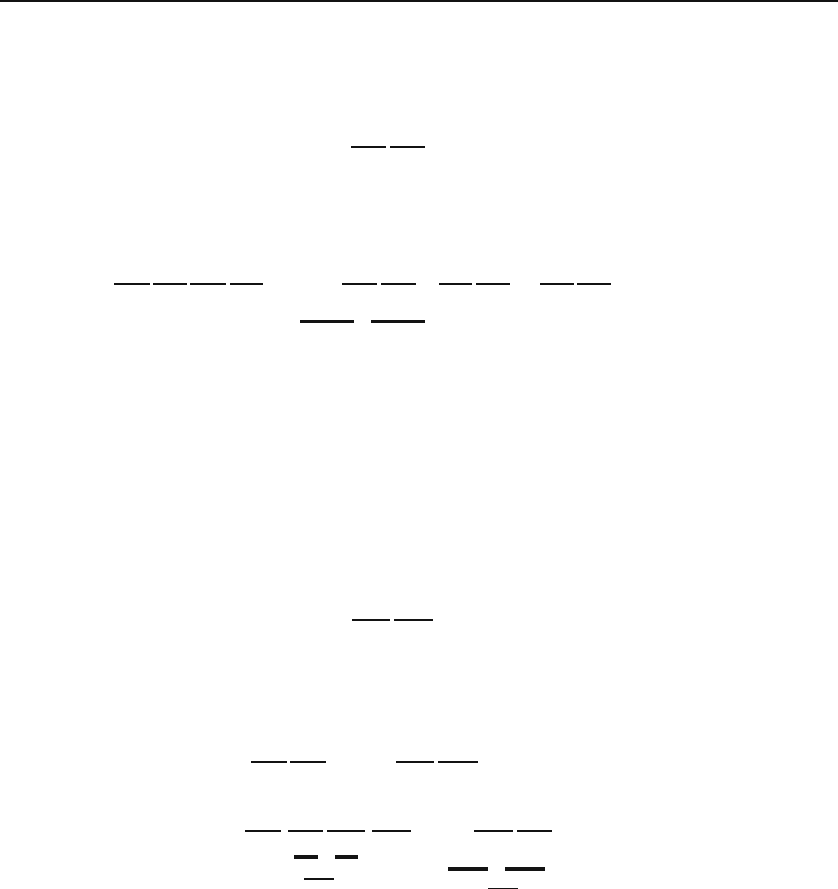
17.3 Metric Tensor 455

functions. We assume that all functional dependences are invertible. This

means that {¯x

} can be thought of as functions of {x

i

}, and through {x

i

as functions of {x

}.Intermsofthe¯x variables,

¯g

(¯x) ≡



i=1

∂x

i

∂¯x

∂x

i

∂¯x

Using the chain rule, this can be written as

¯g

(¯x)=



i=1

∂x

i

∂x

∂¯x

∂x

i

∂x

∂¯x





i=1

∂x

i

∂x

i

∂x





 

(x)

∂x

∂¯x

∂x

∂¯x

∂x

∂¯x

∂x

∂¯x

(x),

which shows that g

transforms as a (0, 2)-type tensor. In terms of this

tensor, ds

is written as



i=1

(dx

i

)

= g

(x)dx

. (17.35)

The matrix whose elements are g

is invertible. In fact, consider

(x) ≡



p=1

∂x

p

∂x

p

which the reader can show to be a tensor of type (2, 0). Then

(x)h

(x)=





i=1

∂x

i

∂x

i

∂x





p=1

∂x

p

∂x

p





i,p=1

∂x

i

∂x

i

∂x

p

  

∂x

i

∂x

p

=δ

∂x

p



i=1

∂x

i

∂x

i

∂x

  

∂x

= δ

where on the second line use was made of the chain rule and Box 17.1.5.

This equation shows that the matrix whose elements are h

(x)isinverse

to the matrix whose elements are g

(x). It is common to use the same

symbol for the inverse as for the original tensor. Thus, instead of h

(x), we

use g

(x).

The (0, 2)-tensor g

(x) was deﬁned in terms of the transformation rule

between a Cartesian and a second coordinate system. It turns out that one

can abstract the properties of g

(x) and deﬁne the metric tensor:

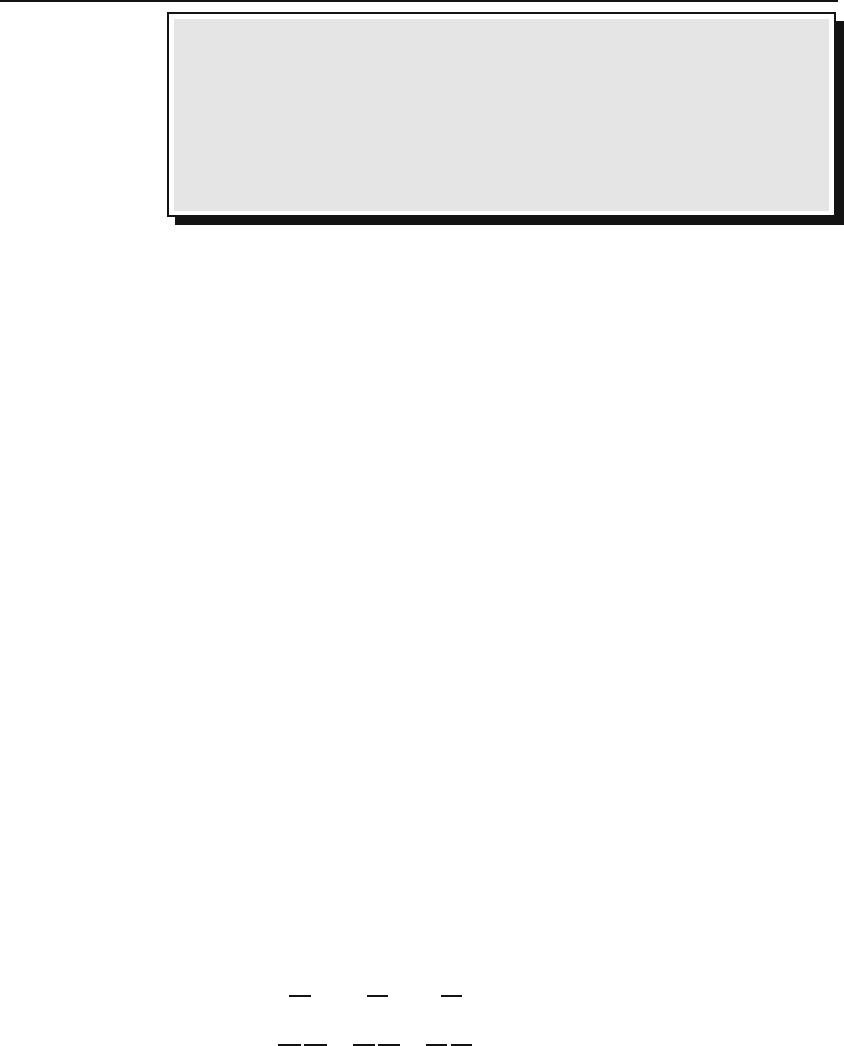
456 Tensor Anal ysi s

Box 17.3.1. A metric tensor g with components g

is a symmetric

type-(0, 2) tensor whose matrix has an inverse g

−1

with components g

Every metric tensor deﬁnes a geometry in which the (square of the)

element of length ds

is given by

= g

(x)dx

where {x

} are some appropriate coordinates in that geometry.

The word “geometry” in this Box is used rather loosely. A precise deﬁni-geometry,

manifold, and

metric tensor

tion of “geometry” is beyond the scope of this book. Nevertheless, we mention

that the notion of geometry starts with the concept of a manifold,whichisa

“space” that locally looks like a Euclidean space. For example, the surface of

a sphere is a two-dimensional manifold, because a very small area of a sphere

looks like a two-dimensional Euclidean space, i.e., a ﬂat plane. Mathemati-

cians study manifolds that have no metric tensors deﬁned on them. However,

in physics, almost all manifolds have a metric, and this metric deﬁnes the

geometry of that manifold.

In our discussion of the inner product in Section 6.1.2, we also encountered

the metric tensor, although we called it the metric matrix. There, we deﬁned

the notion of positive deﬁniteness. In the context of the discussion here, this

Riemannian

manifold

property becomes the cornerstone of a special kind of geometry: if ds

Box 17.3.1 is always strictly greater than zero for nonzero dx

and dx

,then

the manifold on which g

is deﬁned is a called a Riemannian manifold.

Relativity requires manifolds that are not Riemannian, i.e., for which ds

can

be zero or negative.

Geometry is an intrinsic property of a space, while g

(x) depends on the

coordinates used. This is evident in Equation (17.35) where ds

is given in

terms of Cartesian coordinates as well as the other general coordinates. De-

spite this coordinate dependence, the metric tensor does deﬁne the geometry

of a manifold. In fact, there are some quantities obtained from the metric

which characterize the intrinsic geometry of the manifold. We shall return to

this discussion later.

Example 17.3.1.

Let us ﬁnd the metric tensor in spherical coordinates. Use

spherical coordinate symbols as indices with r, θ,andϕ as ﬁrst, second, and third

coordinates, respectively. Recalling that x

1

= x, x

2

= y,andx

3

= z,with

x = r sin θ cos ϕ, y = r sin θ sin ϕ, z = r cos θ,

and using Equation (17.34), we get

(r, θ, ϕ)=



∂x

∂r





∂y

∂r





∂z

∂r



=(sinθ cos ϕ)

+(sinθ sin ϕ)

+(cosθ)

rθ

(r, θ, ϕ)=

∂x

∂r

∂x

∂θ

∂y

∂r

∂y

∂θ

∂z

∂r

∂z

∂θ

=(sinθ cos ϕ)(r cos θ cos ϕ)+(sinθ sin ϕ)(r cos θ sin ϕ)+(cosθ)(−r sin θ)

= r sin θ cos θ cos

ϕ + r sin θ cos θ sin

ϕ − r cos θ sin θ =0.

17.3 Metric Tensor 457

Similarly, the reader can show that g

rϕ

= 0, and in fact all the oﬀ-diagonal elements

vanish. On the other hand,

θθ

(r, θ, ϕ)=



∂x

∂θ





∂y

∂θ





∂z

∂θ



=(r cos θ cos ϕ)

+(r cos θ sin ϕ)

+(−r sin θ)

= r

ϕϕ

(r, θ, ϕ)=



∂x

∂ϕ





∂y

∂ϕ





∂z

∂ϕ



=(−r sin θ sin ϕ)

+(r sin θ cos ϕ)

= r

sin

θ.

Therefore,

=(dr)

+ r

(dθ)

+ r

sin

θ(dϕ)

= dr

+ r

dθ

+ r

sin

θdϕ

which agrees with Equation (2.25). Note how the parentheses have been removed

from around the diﬀerentials. This is a very common (albeit inaccurate)

practice.



17.3.1 Index Raising and Lowering

After Box 17.1.6, we mentioned that the length of a covariant or contravari-

ant vector cannot be deﬁned without a metric tensor. Now that we have a

metric tensor, we deﬁne them. In fact, we can do better! We can deﬁne the

dot product of any two vectors. If one vector is covariant and the other con-

travariant, their dot product is the usual one: the sum of the product of their

components as shown in (17.16). If both vectors A and B are contravariant,

deﬁne the dot product as

A · B = g

, (17.36)

and if both vectors are covariant, deﬁne the dot product as

A ·B = g

. (17.37)

The reader can routinely show that

A ·

B = A · B in both cases.

Equations (17.36) and (17.37) have an interesting interpretation. Take the

ﬁrst equation and recall from Equation (17.26) that the product g

is a

tensor of type (1, 2). Contracting the indices i and k turns that into a tensor

of type (0, 1), i.e., a covariant vector, say C with components C

. But now

note that

C · A = C

= g

Aj = A ·A.

It is therefore natural to denote g

—which is equal to g

because of the

symmetry of the metric tensor—by A

. Thus,themetrictensorg

provides

us with a way of changing contravariant vectors to covariant vectors, i.e.,

lowering their indices. Similar arguments show that the inverse of the metric

tensor g

can be used to raise indices; and these two processes are consistent,

in the sense that if we lower the index of a contravariant vector with g

and

then raise the index of the resulting covariant vector with g

,wegetthe

458 Tensor Anal ysi s

original contravariant vector. Here is a proof! Let C

= g

,whereA

the covariant vector obtained from A

. Then,

= g

= δ

= A

and the original contravariant vector is restored. The process of raising and

lowering of indices works for arbitrary tensors:

Box 17.3.2. Any contravariant index i of a general tensor can be made

into a covariant index j by multiplying the component that includes i by

. Any covariant index i of a general tensor can be made into a con-

travariant index j by multiplying the component that includes i by g

In Cartesian coordinates the (Euclidean) metric tensor is just the Kronecker

delta. Therefore

= g

= δ

= A

, in Cartesian coordinates with Euclidean metric,

(17.38)

and the distinction between covariant and contravariant vectors (and indices)

disappears.

In special relativity and in Cartesian coordinates, the metric tensor is η

αβ

whose matrix is given in Equation (8.8). This tensor has components

=1,η

= η

= −1,η

αβ

=0ifα = β in special relativity.

The inverse of η

αβ

is itself: η

αβ

= η

αβ

. In raising and lowering of an index,

the time component does not change, while the space components change sign

(see Box 17.2.3 for the meaning of Greek and Roman indices in relativity):

= η

αβ

⇒ A

= A

= −A

(17.39)

Example 17.3.2.

The Levi-Civita symbols are conveniently used to express the

components of the cross product of two vectors in Cartesian coordinate systems.components of

cross product Since there is no diﬀerence between covariant and contravariant indices in Cartesian

coordinate system, we use only covariant indices.

(A × B)

= 

ijk

,i=1, 2, 3, (17.40)

where a sum over j and k is understood. As a practice in index manipulation, the

reader is urged to verify the above relation. The order of the two vectors on both

sides of the equation is important!

Using Equation (17.40) and some properties of the Levi-Civita symbol, we can

derive the bac cab rule:

A × (B × C)=B(A ·C) − C(A · B).

17.3 Metric Tensor 459

Start with a general component of the LHS and work through index manipulations

until you reach the corresponding component of the RHS:

{A × (B × C)}

= 

ijk

(B × C)

= 

ijk



kmn

= 

kij



kmn

=(δ

− δ

) A

= δ

− δ

= A

− A

= B

) − C

)=B

(A · C) − C

(A · B).

On the second line we used (17.33) and the result obtained in Example 17.2.4. The

last expression above is the ith component of the RHS of the bac cab rule.



Example 17.3.3. Example 15.3.1 calculated the angular momentum diﬀerential

operator using Cartesian coordinates. To illustrate the power of indices and the

ease with which they allow some complex manipulations, we redo the calculation of

Example 15.3.1 using indices.

We have −L

f =(r ×∇) · (r × ∇)f . Letting ∂

stand for the partial derivative

with respect to x

, using Einstein summation convention, and recalling that no

raising or lowering of indices is necessary for Euclidean space, we write

−L

f =(r × ∇)

(r × ∇)

f =(

ijk

∂

)(

ilm

∂

) f = 

ijk



ilm

∂

f) ,

where we used (17.40). Continuing, refer to (17.33) and write the above equation as

−L

f =(δ

− δ

) x

∂

f)=x

∂

f) − x

∂

= x

∂

f + x

∂

f − x

∂

f − x

∂

f (17.41)

= x

∂

f + r

∇

f − 3x

∂

f − x

∂

f = r

∇

f − 2(r ·∇)f − x

∂

because ∂

= δ

, x

= r

, δ

=3,x

∂

= r · ∇,and∂

∂

= ∇

.Thelast

term in (17.41) above can be found from the following relation:

∂

f)=x

∂

f + x

∂

f = x

∂

f + x

∂

f =(r · ∇)

f − (r · ∇)f.

Substituting in (17.41) yields Equation (15.22). Compare this derivation with the

laborious calculation of Example 15.3.1!



17.3.2 Tensors and Electrodynamics

Relativity was a logical outcome of the electromagnetic theory. It should

therefore come as no surprise if the equations of electromagnetism found their

most natural form in the language of relativity and tensors associated with

it. In the discussion that follows, it is convenient and common practice to set

the speed of light equal to 1; then since c =1/

√



,wehave

c =1,



= μ

Consider the Lorentz force law

f = q(E + v ×B)orf

= q (E

+ 

ijk

) , (17.42)

460 Tensor Anal ysi s

where as in Example 17.3.2, we used covariant indices for all tensors in the

second equation. Since this is the fundamental force of electromagnetism, we

expect it to have a natural expression in relativity.

As a starting point, we note that the magnetic part is of the form v

where F

= 

ijk

is an antisymmetric tensor of rank two. The obvious

generalization that might lead to a connection with relativity is to consider

an expression of the form u

αβ

,whereu

is the velocity 4-vector and F

αβ

an antisymmetric tensor of rank two which reduces to F

when both α and

β are nonzero. Let us look at u

αβ

when α is i:

iβ

= u

+ u

where we used the convention of Example 17.2.2. Equation (8.21) now gives

= γ,andu

= γv

. Then the equation above giveselectromagnetic

ﬁeld tensor

iβ

= γF

+ γv

= γ

+ v

= γ (F

+ v



ijk

) ,

where in the last step, we disregarded the diﬀerence between covariant and

contravariant indices. Comparison with Equation (17.42) shows that it is

natural to set F

= E

. The second rank antisymmetric tensor F

αβ

is called

the electromagnetic ﬁeld tensor.

Maxwell’s equations (15.29) take a specially simple form when written in

terms of the electromagnetic ﬁeld tensor. The ﬁrst equation can be written

∂

∂F

∂x



= μ

ρ. (17.43)

The obvious generalization of the left-hand side to relativity is ∂F

αβ

/∂x

.But

there is something wrong with this! Both α’s are lower indices—recall that the

superscript of a coordinate in the denominator leads to a subscript—and you

cannot sum over them. In the Euclidean case, this causes no problem because

by (17.38), there is no diﬀerence between lower and upper indices and we can

simply raise one of the i’s. In relativity, however, there is a diﬀerence. So, we

have to introduce the (inverse) η tensor. The left-hand side now becomes

αν

∂F

αβ

∂x

Since β is a free index, we expect the right-hand side to have a free index as

well. So, we write the generalization of Maxwell’s ﬁrst equation as

αν

∂F

αβ

∂x

= μ

, (17.44)

with V

to be determined. For β =0,weget

αν

∂F

α0

∂x

= μ

, or η

iν

∂F

∂x

= μ

, or −

∂F

∂x

= μ

where we used the fact that F

αβ

is antisymmetric, so all its “diagonal” com-

ponents are zero. We also used the fact that η is diagonal with the space

elements being −1. Comparing with (17.43), we see that V

= −ρ.

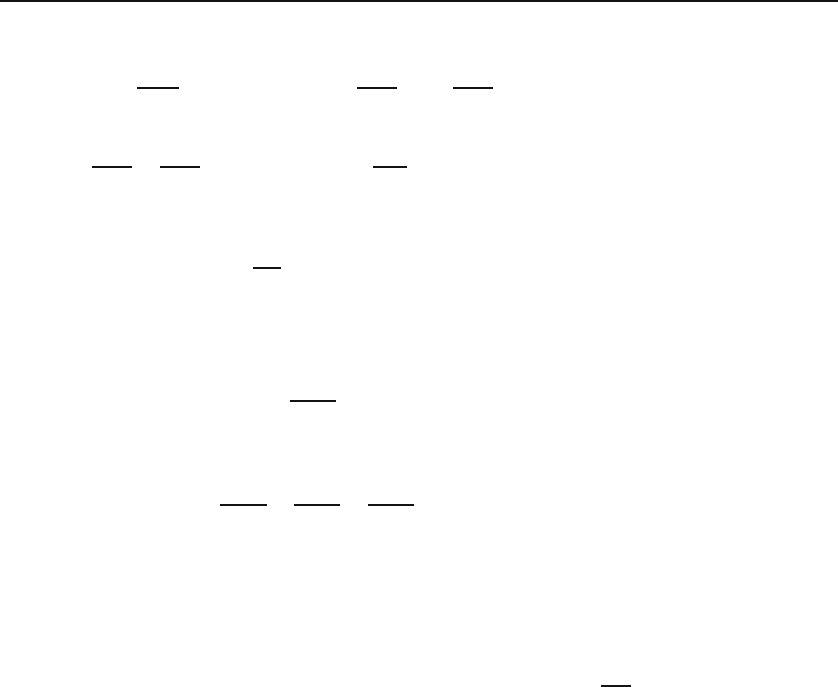
17.3 Metric Tensor 461

Now let β = i in (17.44). Then

αν

∂F

αi

∂x

= μ

, or η

0ν

∂F

∂x

+ η

jν

∂F

∂x

= μ

∂F

∂x

−

∂F

∂x

= μ

, or −

∂E

∂t

+ 

ijk

∂

= μ

This is the ith component of the vector equation

−

∂E

∂t

+ ∇×B = μ

Comparing this with the fourth Maxwell’s equation, we identify V as J.Thus,

Maxwell’s 1st and

4th equations and

four-current

the ﬁrst and fourth equations, the inhomogeneous Maxwell’s equations

are combined into

αν

∂F

αβ

∂x

= μ

, (17.45)

where J

=(−ρ, J)isthe4-current. We leave it to the reader to verify that Maxwell’s 2nd and

3rd equations

∂F

αβ

∂x

∂F

να

∂x

∂F

βν

∂x

= 0 (17.46)

combines the second and third equations, the homogeneous Maxwell’s

equations.

Equation (17.46) is satisﬁed if F

αβ

= ∂

− ∂

for any 4-vector A

as the reader can easily verify. For α = i and β =0,thisgives

= ∂

− ∂

, or E

= ∂

− ∂

, or E = ∇A

−

∂A

∂t

Comparing this with (15.31) identiﬁes A

with the negative of the scalar

potential Φ and A with the vector potential. We can thus write

αβ

= ∂

− ∂

=(−Φ, A). (17.47)

Now that we have solved the homogeneous Maxwell’s equations by in-

troducing the 4-potential, we can insert the result in (17.45) to write the

inhomogeneous Maxwell’s equations in terms of the 4-potential as well. We

then have

αν

∂

(∂

− ∂

)=μ

αν

∂

− ∂

(η

αν

∂

)=μ

. (17.48)

The expression in parentheses—when set equal to zero—gives the Lorentz

gauge condition [see Equation (15.32)]. The remaining part of the equation

gives the wave equation for A and Φ.