Hassani S. Mathematical Methods: For Students of Physics and Related Fields

Подождите немного. Документ загружается.

82 Integration: Formalism

3.2.1 Change of Dummy Variable

The symbol used as the variable of integration in the integral is completely

irrelevant. Thus, we have

Feel free to use

any symbol you

like for the

integration

variable!

g(t) dt =

g(x) dx =

g(s) ds

g(t



) dt



g() d.

Note how the limits of integration remain the same in all integrals. The fact

that these limits use the same symbol as the ﬁrst dummy variable should not

confuse the reader. What is important is that they are ﬁxed real numbers.

3.2.2 Linearity

For arbitrary constant real numbers a and b,wehave

[af(t)+bg(t)] dt = a

f(t) dt + b

g(t) dt.

3.2.3 Interchange of Limits

Interchanging the limits of integration introduces a minus sign:

f(t) dt = −

f(t) dt. (3.7)

This relation implies that



f(t) dt = 0. (Show this implication!)

3.2.4 Partition of Range of Integration

If q is a real number between the two limits, i.e., if p<q<r,then

f(t) dt =

f(t) dt +

f(t) dt. (3.8)

which is a special case of Equation (3.6). This property is used to evaluate

piecewise

continuous

functions

piecewise continuous functions, i.e., functions that have a ﬁnite number

of discontinuities in the interval of integration. For instance, suppose f (t)is

deﬁned to be

f(t)=

⎧

⎪

⎨

⎪

⎩

(t)ifp<t<q

(t)ifq

<t<q

(t)ifq

<t<r,

where f

(t), f

(t), and f

(t) are, in general, totally unrelated (continuous)

functions. Then one divides the interval of integration into three natural

parts and writes

f(t) dt =

(t) dt +

(t) dt.

3.2 Properties of Integral 83

Figure 3.2: The integral is deﬁned as long as there is only a ﬁnite number of disconti-

nuities (jumps) in the function.

This is illustrated in Figure 3.2.

3.2.5 Transformation of Integration Variable

When evaluating an integral it is sometimes convenient to use a new variable

of integration of which the old one is a function. Call the new integration

variable y and assume that t = h(y). Then we have

f(t) dt =

f(h(y)) h



(y) dy, (3.9)

where p and q are the solutions to the two equations

Transformation of

integration

variable

accompanies a

change in the

limits of

integration.

a = h(p),b= h(q).

Each of these two equations must have a unique solution, otherwise, the trans-

formation of the integration variable will not be a valid procedure. This con-

dition puts restrictions on the type of function h can be. Note that we have

essentially substituted h(y)fort in the original integral including the dif-

ferential h



(y) dy for dt. It is vital to remember to change the limits of

integration when transforming variables.

3.2.6 Small Region of Integration

When the region of integration is small, in the sense that the integrand does When is the

region of

integration small?

not change much over the range of integration, then the integral can be ap-

proximated by the product of integrand and the size of the range.

We thus

can write

f(t) dt ≈ (b −a)f(t

), (3.10)

where t

is a number between a and b, mostly taken to be the midpoint of

the interval (a, b).

This is simply a restatement of Equation (3.5) for the case of one variable.

84 Integration: Formalism

3.2.7 Integral and Absolute Value

A useful property of integrals that we shall be using sometimes is



f(t) dt



≤

|f(t)| dt. (3.11)

This should be clear once we realize that an integral is the limit of a sum and

the absolute value of a sum is always less than or equal to the sum of the

absolute values.

3.2.8 Symmetric Range of Integration

By a symmetric range of integration, we mean a range that has 0—the origin—

as its midpoint. For certain functions, partitioning such a range into two equal

pieces can simplify the evaluation of the integral considerably. So, let us write

−T

f(t) dt =

−T

f(t) dt +

f(t) dt.

For the ﬁrst integral, make a change of variable t = −y to obtain

h(y)=−y ⇒ h



(y) dy =(−1) dy = −dy.

The limits of integration in y are determined by

h(−T )=y

lower

,h(0) = y

upper

⇒ y

lower

=+T, y

upper

=0.

We therefore have

−T

f(t) dt =

f(−y)(−dy)=

f(−y) dy =

f(−t) dt,

where we have used the properties in Subsections 3.2.3 and 3.2.1. Combining

our results and using the second property, we get

−T

f(t) dt =

f(−t) dt +

f(t) dt

[f(t)+f(−t)] dt. (3.12)

A real-valued function f is called even if f (−x)=f(x), and odd if

even and odd

functions deﬁned

f(−x)=−f(x). Thus, from Equation (3.12), we obtain

−T

f(t) dt =

[f(t)+f(−t)] dt =2

f(t) dt (3.13)

when f is even, and

−T

f(t) dt =

[f(t)+f(−t)] dt = 0 (3.14)

when it is odd.

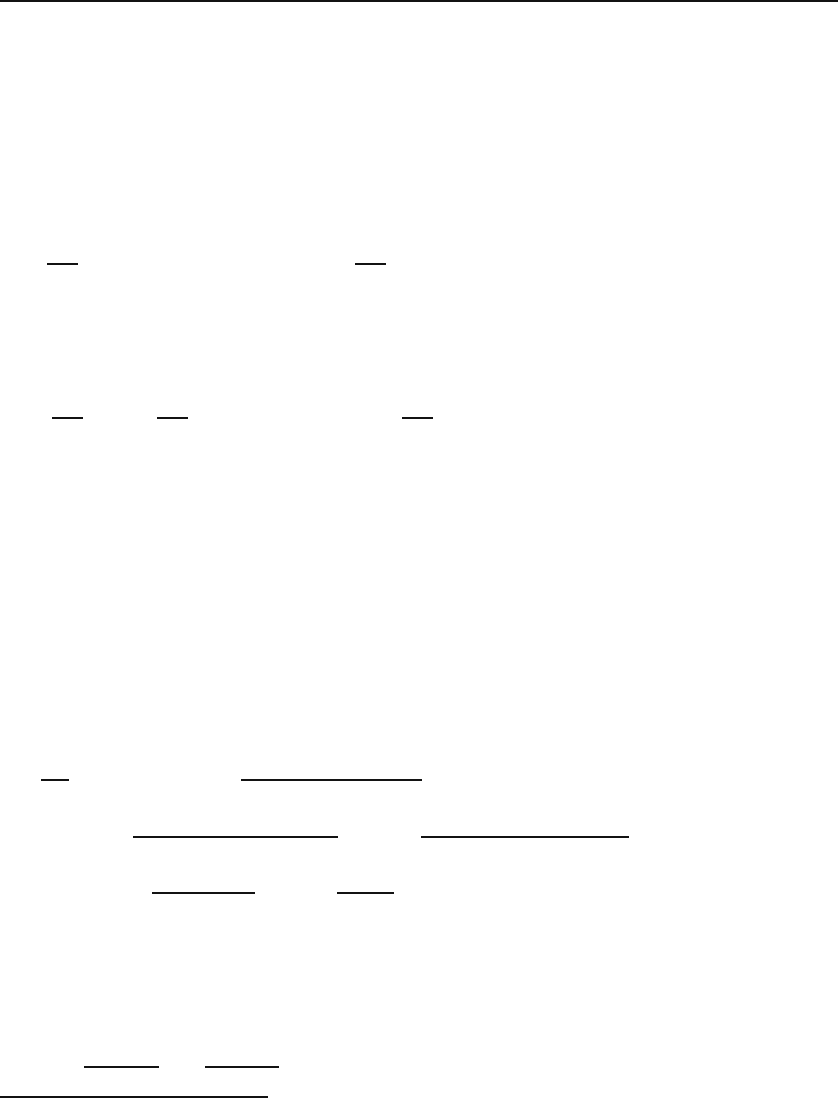
3.2 Properties of Integral 85

3.2.9 Diﬀerentiating an Integral

We have seen that an integral can have an integrand which depends on a set of

parameters, and that the result of integration will depend on these parameters.

Thus, we can think of the integral as a function of those parameters, and in

particular, we may want to know its derivative with respect to one of the

parameters. Using the deﬁnition of integral as the limit of a sum, and the

fact that the derivative of a sum is the sum of derivatives, it is easy to show

that

∂

∂x

f(x

,...,x

,t) dt =

∂

∂x

f(x

,...,x

,t) dt, (3.15)

where we have represented the list of parameters as (x

,...,x

). We can

write exactly the same relation for the integral of Equation (3.4). Assuming

that r =(x

,...,x

), we have

∂

∂x

h( r)=

∂

∂x

f(r, r



) dQ( r



∂

∂x

f(r, r



) dQ( r



). (3.16)

In both cases the region of integration is assumed to be independent of x

Restricting ourselves to single integrals,

we now consider the case where

the limits of integration depend on some parameters. First, consider an inte-

gral of the form

f(t) dt

and treat the result as a function of the limits. So, let us write

F (u, v) ≡

f(t) dt ⇒ F (v, u)=−F (u, v)

and evaluate the partial derivative of F with respect to its arguments:

∂F

∂u

≡ ∂

F (u, v) = lim

→0

F (u + , v) −F (u, v)



= lim

→0



u+

f(t) dt −



f(t) dt



= − lim

→0



f(t) dt +



u+

f(t) dt



= − lim

→0



u+

f(t) dt



= − lim

→0

f(u

)



= − lim

→0

f(u

)=−f(u).

The last equality follows from the fact that as  → 0, u

, lying between u and

u + , will be squeezed to u. Note that the derivative above is independent of

the second variable. To ﬁnd the other derivative, we use the result obtained

above and simply note that

∂F(u, v)

∂v

= −

∂F(v, u)

∂v

= −∂

F (v, u)=−

−f(v)

= f(v).

Since all multiple integrals are reducible to single integrals, this restriction is not severe.

86 Integration: Formalism

Putting these two results together, we can write

∂

∂v

f(t) dt = f(v),

∂

∂u

f(t) dt = −f(u). (3.17)

In words,

Box 3.2.1. The derivative of an integral with respect to its upper (lower)

limit equals the integrand (minus the integrand) evaluated at the upper

(lower) limit.

By evaluation, we mean replacing the variable of integration. If the integrand

has parameters, they are to be left alone.

By combining Equations (3.15) and (3.17) we can derive the most general

equation. So, assume that both u and v are functions of (x

,...,x

), and

write

G(x

,...,x

,u,v) ≡

v(x

,...,x

)

u(x

,...,x

)

f(x

,...,x

,t) dt.

Then, using the chain rule. we get

G ≡

∂G

∂u

∂x

∂G

∂v

∂x

+ ∂

where D

G stands for the “total” derivative with respect to x

. This meanstotal derivative

that the dependence of u and v on x

is taken into account. In contrast, ∂

is evaluated assuming that u and v are constants. We note that

∂G

∂u

∂

∂u

f(x

,...,x

,t) dt = −f(x

,...,x

,u),

∂G

∂v

∂

∂v

f(x

,...,x

,t) dt = f (x

,...,x

,v),

∂G

∂x

∂

∂x

f(x

,...,x

,t) dt =

∂

∂x

f(x

,...,x

,t) dt,

where the partial derivative in the last equation treats u and v as constants.

It follows that

Box 3.2.2. The most general formula for the derivative of an integral is

∂

∂x

v(r)

u(r)

f(r,t) dt =

∂v

∂x

f(r,v) −

∂u

∂x

f(r,u)+

v(r)

u(r)

∂

∂x

f(r,t) dt,

where r =(x

,...,x

As indicated in Equation (2.16), it is common to ignore the diﬀerence between

and ∂

; and the formula in Box 3.2.2 reﬂects this.

3.2 Properties of Integral 87

3.2.10 Fundamental Theorem of Calculus

A special case of Box 3.2.2 is extremely useful. Consider a function g of

a single variable x. We want to ﬁnd a function called the primitive,or

primitive

(antiderivative) of

a function

antiderivative, or indeﬁnite integral

whose derivative is g.Thiscanbe

easily done using integrals. In fact using Box 3.2.2, we have

G(x) ≡

g(s) ds ⇒

g(s) ds = g(x), (3.18)

where a is an arbitrary constant. We can add an arbitrary constant to the

RHS of the above equation and still get a primitive. Adding such a constant,

evaluating both sides at x = a, and noting that the integral vanishes, we ﬁnd

that the constant must be G(a). We, therefore, obtain

G(x) −G(a)=

g(s) ds. (3.19)

Now suppose that F (x)isany function whose derivative is g(x). Then,

from Equation (3.18), we see that

[G(x) − F (x)] =

−

= g(x) −g(x)=0.

Therefore, G(x) −F (x) must be a constant C. It now follows from (3.19) that

F (x) −F (a)=G(x) −C − [G(a) − C]=G(x) −G(a)=

g(s) ds,

and we have

fundamental

theorem of

calculus

Box 3.2.3. (Fundamental Theorem of Calculus).LetF (x) be any

primitive of g(x) deﬁned in the interval (a, b), i.e., any function whose

derivative is g(x) in that interval. Then,

F (b) − F (a)=

g(s) ds. (3.20)

The founders of calculus such as Barrow, Newton,andLeibniz thought of

an integral as a sum. At the beginning no connection between integration and

Connection

between integrals

and antiderivatives

was not apparent

at the time

integration was

introduced. It was

discovered later.

diﬀerentiation was established, and to obtain the result of an integral one

had to go through the painstaking process of adding the terms of a (inﬁnite)

sum. It was later, that the founders of calculus realized (but did not prove)

that the process of summation and taking limits was intimately connected

We would like to emphasize the concept of integral as the limit of a sum. Therefore,

we think it is better to reserve the word “integral” for such sums and will avoid using the

phrase “indeﬁnite integral.”

88 Integration: Formalism

to the process of (anti) diﬀerentiation. In this respect, Equation (3.20) is

indeed a fundamental result, because it eliminates the cumbersome labor of

summation.

Another useful result is

G(x) −G(a)=

g(s) ds =

(s) ds =

dG. (3.21)

In words, the integral of the diﬀerential of a physical quantity is equal to the

quantity evaluated at the upper limit minus the quantity evaluated at the lower

limit.

Example 3.2.1.

The properties mentioned above can be very useful in evaluating

some integrals. Consider the integral



∞

−∞

−t

dt whose value is known to be

√

π (see

Example 3.3.1). We want to use this information to obtain the integral



∞

−∞

−t

dt.

First, we note that

∞

−∞

−xt

dt =



This can be shown readily by changing the variable of integration to u =

√

xt and

using the result of Example 3.3.1. Next, we diﬀerentiate both sides with respect to

x and use Box 3.2.2 with u = −∞ and v = ∞.Wethenget

LHS =

∂

∂x

∞

−∞

−xt

dt =

∞

−∞

∂

∂x

−xt

dt =

∞

−∞

(−t

−xt

for the LHS, and

∂

∂x



= −

√

3/2

for the RHS. Sousing derivative of

integral to obtain

new integral

formulas from

known integral

formulas

∞

−∞

−xt

dt =

√

−3/2

(3.22)

or, setting x =1,



∞

−∞

−t

dt =

√

We can obtain more general results. Diﬀerentiating both sides of Equation

(3.22), we obtain

∞

−∞

−xt

dt =

√

−5/2

√

1 · 3

−5/2

Continuing the process n times, we obtain

∞

−∞

−xt

dt =

√

1 · 3 · 5 ···(2n −1)

−(2n+1)/2

. (3.23)

In particular, if x =1,wehave

∞

−∞

−t

dt =

√

1 · 3 · 5 ···(2n − 1)



Example 3.2.2. Integrals involving only trigonometric functions are easy to

evaluate:

sin tdt = −cos t



=cosa − cos b,

cos tdt =sint



=sinb − sin a.

3.2 Properties of Integral 89

However, integrals of the form I ≡



sin tdt,inwhichn is a positive integer,

are not as easy to evaluate although they occur frequently in applications. One can

of course evaluate these integrals using integration by parts. But that is a tedious

process. A more direct method of evaluation is to use the ideas developed above.

A pair of slightly more complicated trigonometric integrals which will be useful

for our purposes is

sin st dt = −

cos st



cos sa − cos sb

cos st dt =

sin st



sin sb − sin sa

. (3.24)

If we diﬀerentiate both sides with respect to s, we can obtain the integrals we are

after.

This is because each diﬀerentiation introduces one power of t in the integrand.

For example if we are interested in I with n = 1, then we can diﬀerentiate the second

equation in (3.24):

LHS =

cos st dt =

∂

∂s

(cos st) dt = −

t sin st dt.

On the other hand,

RHS =

∂

∂s



sin sb − sin sa



= −

sin sb − sin sa

b cos sb − a cos sa

Setting s = 1 in these equations yields

t sin tdt=sinb − sin a − b cos b + a cos a. (3.25)

We can also ﬁnd the primitive of functions of the form x

sin x. All we need to

do is change b to x as suggested by Equation (3.18). For example, the primitive

(indeﬁnite integral) of x sin x is obtained by substituting x for b in Equation (3.25):

x sin xdx=sinx − sin a − x cos x + a cos a =sinx −x cos x + C

because −sin a + a cos a is simply a constant.



Historical Notes

After a lull of almost two millennia, the subject of “exhaustion,” like any other form

of human intellectual activity, was picked up after the Renaissance. Johannes Kepler

is reportedly the ﬁrst one to begin work on ﬁnding areas, volumes, and centers of

gravity. He is said to have been attracted to such problems because he noted the

inaccuracy of methods used by wine dealers to ﬁnd the volumes of their kegs.

Some of the results he obtained were the relations between areas and perimeters.

For example, by considering the area of a circle to be covered by an inﬁnite number

of triangles, each with a vertex at the center, he shows that the area of a circle is

We can set s = 1 at the end if need be.

90 Integration: Formalism

its radius times its circumference. Similarly, he regarded the volume of a sphere as

the sum of a large number of small cones with vertices at the center. Since he knew

the volume of each cone to be

its height times the area of its base, he concluded

that the volume of a sphere should be

its radius times the surface area.

Galileo used the same technique as Kepler to treat the uniformly accelerated

motion and essentially arrived at the formula x =

. They both regarded an

area as the sum of inﬁnitely many lines, and a volume as the sum of inﬁnitely many

planes, without questioning the validity of manipulating inﬁnities. Galileo regarded

a line as an indivisible element of area, and a plane as an indivisible element of

volume.

Inﬂuenced by the idea of “indivisibles,” Bonaventura Cavalieri, a pupil of

Galileo and professor in a lyceum in Bologna, took up the study of calculus upon

Galileo’s recommendation. He developed the ideas of Galileo and others on indivis-

ibles into a geometrical method and in 1635 published a book on the subject called

Geometry Advanced by a thus far Unknown Method, Indivisible of Continua.

Cavalieri joined the religious order Jesuati in Milan in 1615 while he was still

a boy. In 1616 he transferred to the Jesuati monastery in Pisa. His interest in

mathematics was stimulated by Euclid’s works and after meeting Galileo, considered

himself a disciple of the astronomer. The meeting with Galileo was set up by Car-

dinal Federico Borromeo who saw clearly the genius in Cavalieri while he was at the

monastery in Milan.

Bonaventura

Cavalieri

1598–1647

Cavalieri was largely responsible for introducing logarithms as a computational

tool in Italy. The tables of logarithms which he published included logarithms of

trigonometric functions for use by astronomers. Cavalieri also wrote on conic sec-

tions, trigonometry, optics, and astronomy. He showed by his methods of indivisibles

that, in the modern notation,

dx =

n+1

n +1

for positive integral values of n up to 9.

The next important step in the development of integral calculus began when

the seventeenth-century mathematicians generalized the Greek method of exhaus-

tion. Whereas this method requires diﬀerent rectilinear approximation for diﬀerent

geometrical ﬁgures, the new generation of mathematicians approximated the area

under any curve by a large number of rectangles of equal width (much like it is

done today), summed up the areas, and neglected the “small corrections” in the

sum. Using essentially this kind of summation technique, Fermat showed the above

integral formula for all rational n except −1 before 1636.

Before Newton and Leibniz, the man who did most to replace the geometrical

techniques with analytical ones in calculus was John Wallis. Although he did

not begin to learn mathematics until he was about twenty, he became professor

of geometry at Oxford and the ablest British mathematician of the century, next

to Newton. One of Wallis’s notable results, obtained while he was trying to ﬁnd

the area of a circle analytically, was a new formula for π. He calculated the area

bounded by the axes and the curves y =(1− x

)

for n =0, 1, 2,....Thenby

interpolation and further complicated reasoning he related the area of a unit circle

John Wallis

1616–1703

y =(1− x

)

1/2

to the previous areas and showed that

2.2.4.4.6.6.8.8 ...

1.3.3.5.5.7.7.9 ...

3.3 Guidelines for Calculating Integrals 91

3.3 Guidelines for Calculating Integrals

The number of situations in which integrals are used is unlimited, and we shall

see many examples of such usage in this chapter and throughout the book. Be-

fore embarking on speciﬁc examples, let us summarize some guidelines which

will be helpful in applying integrals in physical problems:

• Make sure you understand what physical quantity you are trying to

Let the problem

determine

formulas!

calculate. Instead of searching randomly for formulas, think about the

problem and let it determine the formulas.

• Determine which coordinate system is most suited for the problem.

Then place the origin and orient the axes in such a way that the prob-

lem takes the simplest form. Usually spherical coordinates are suited

Choose

coordinates,

origin, and

orientation of axes

wisely!

for regions of integration which are symmetric about a single point. If

there is a natural “axis” associated with the problem, then cylindrical

coordinates are useful, and if the region of integration is in the shape of

a rectangular box, Cartesian coordinates may be most suitable. If there

is no obvious symmetry, then any one of the systems is just as good (or

just as bad).

• Write down the local formula ﬁrst, i.e., conﬁne the problem to a small

Write the local

formula, then put

it inside the

integral.

region and write the formula, for instance, in terms of dQ(r



), dm(r



etc., then put the formula inside the integral. Do this in a coordinate-

independent way ﬁrst. All physical laws are written with no reference

to a particular coordinate system, anyway.

• Now express the formula in terms of the coordinates you have chosen.

When dealing with vector quantities, pay particular attention to unit

vectors whose directions depend on the integration point. They cannot

in general be taken out of the integral sign (see Section 3.3.2 for details).

• Determine the limits of integration. In a typical situation, if you have

chosen a good coordinate system, placed the origin properly, and ori-

ented the axes nicely, then the limits of integration should be easy to

write.

• Never take anything out of the integral unless you are absolutely sure

Never take

anything out of

the integral

unless. . . .

that it is independent of the integration variables. This is easily said,

but most often also easily forgotten.

• Once you have evaluated the integrals and found the physical quantity

you are after, try to express your result in a coordinate-free language.

This is not, in general, easy, but in special circumstances you can im-

mediately guess the coordinate-free form of the result.

• As a general rule—valid in all physical calculations—check your ﬁnal

Always check the

dimension of your

ﬁnal result!

answer for correct dimensions. The dimension of the LHS must match

that of the RHS.