Hassani S. Mathematical Methods: For Students of Physics and Related Fields

Подождите немного. Документ загружается.

2.4 Problems 71

(b) Show that the vector

∂f

∂x

∂f

∂y

∂f

∂z

,whenwrittenentirelyinterms

of cylindrical coordinates and cylindrical unit vectors, becomes

∂f

∂ρ

∂f

∂ϕ

∂f

∂z

2.19. In each of the following, the partial derivative of a function is given.

Find the most general function with such a derivative.

(a) ∂

f(x, y, z)=xy

z. (b) ∂

f(x, y, z)=xy

h(z,x, y)=

sin z

. (d) ∂

g(z,x, y)=e

(e) ∂

g(z,x, y)=e

. (f) ∂

h(x, y, z)=

sin z

(g) ∂

f(x, y, z)=xy

z. (h) ∂

g(z,x, y)=e

(i) ∂

h(y, x,z)=

sin z

2.20. Finish the calculation of Example 2.2.8.

2.21. Find y∂f/∂z − z∂f/∂y and z∂f/∂x − x∂f/∂z in terms of spherical

coordinates. Warning! These will not be as nice-looking as the expression

calculated in Example 2.2.8.

2.22. Given that f



(1) = 2, ﬁnd

∂f

∂x

∂f

∂y

∂f

∂z

for f (xyz)attheCartesianpoint(−1, 2, −1/2).

2.23. Given that f



(3) = −1, ﬁnd the radial component of the vector

∂f

∂x

∂f

∂y

∂f

∂z

for f (



+ y

+ z

)attheCartesianpoint(2, 1, −2).

2.24. Show that the function F (k·r−ωt) satisﬁes the three-dimensional wave

equation:

∂

∂x

∂

∂y

∂

∂z

−

∂

∂t

if k ≡k

 is a constant vector, ω is a constant, and a certain relation

exists between k = |k| and ω. Find this relation.

2.25. In electromagnetic radiation theory one encounters an equation of the

form

t =



[x − f(t)]

+[y − g(t)]

+[z − h(t)]

72 Diﬀerentiation

and one is interested in the partial derivative of t with respect to x, y,andz.

Note the hybrid role that t plays here as both a dependent and an independent

variable. Show that

∂t

∂x

x − f(t)

[x − f(t)]f



(t)+[y − g(t)]g



(t)+[z − h(t)]h



(t) − F

3/2

where F (x, y, z, t) ≡ [x−f(t)]

+[y−g(t)]

+[z−h(t)]

. Find similar expressions

for partial derivatives of t with respect to y and z.

2.26. Consider the function f(|r − r



|)withr = x

+ y

+ z

and r



+ y



+ z



being the position vectors of P and P



(a) Find a general expression for the vector

V =

∂f

∂x

∂f

∂y

∂f

∂z

in terms of r and r



(b) If f



(3) = 3 and the coordinates of P and P



are (1, −1, 0), and (0, 1, 2),

respectively, ﬁnd the numerical value of V.

2.27. Find an expression in cylindrical and spherical coordinates analogous

to Equation (2.21).

2.28. A function f(x, y) of Cartesian coordinates can also be thought of as a

function of some other coordinates u and v deﬁned by

x = u sin v, y = u cos v.

(a) Applying the procedure of Example 2.3.2, ﬁnd the unit vectors

and

(b) Find u and v as functions of x and y.

and

in terms of

and

(d) Write the vector

A =

∂f

∂x

∂f

∂y

entirely in the (u, v)coordinatesystem.

2.29. Find the cylindrical unit vectors in terms of Cartesian unit vectors

using the procedure of Example 2.3.2.

2.30. Find the spherical unit vectors in terms of Cartesian unit vectors using

the procedure of Example 2.3.2.

2.31. In the ﬁrst part of Example 2.3.2, assume that f(u, v)=uf

(v)and

g(u, v)=ug

(v)wheref

and g

are functions of only one variable.

(a) Find a relation between f

and g

to make

and

perpendicular.

(b) Can you recover the polar coordinates as a special case of (a)?

2.4 Problems 73

2.32. The elliptic coordinates (u, θ)aregivenby

x = a cosh u cos θ,

y = a sinh u sin θ,

where a is a constant.

(a)Whatarethecurvesofconstantu?

(b)Whatarethecurvesofconstantθ?

and

in terms of the Cartesian unit vectors, and examine their

orthogonality.

2.33. The parabolic coordinates (u, v)aregivenby

x = a(u

− v

y =2auv,

where a is a constant.

(a)Whatarethecurvesofconstantu?

(b)Whatarethecurvesofconstantv?

and

in terms of the Cartesian unit vectors, and examine their

orthogonality.

2.34. The two-dimensional bipolar coordinates (u, v)aregivenby

x =

a sinh u

cosh u +cosv

y =

a sin v

cosh u +cosv

where a is a constant.

(a)Whatarethecurvesofconstantu?

(b)Whatarethecurvesofconstantv?

and

in terms of the Cartesian unit vectors, and examine their

orthogonality.

2.35. The elliptic cylindrical coordinates (u, θ, z)are given by

x = a cosh u cos θ,

y = a sinh u sin θ,

z = z,

where a is a constant.

(a) What are the surfaces of constant u?

(b) What are the surfaces of constant θ?

,and

in terms of the Cartesian unit vectors and examine

their orthogonality.

74 Diﬀerentiation

2.36. The prolate spheroidal coordinates (u, θ, ϕ)aregivenby

x = a sinh u sin θ cos ϕ,

y = a sinh u sin θ sin ϕ,

z = a cosh u cos θ,

where a is a constant.

(a) What are the surfaces of constant u?

(b) What are the surfaces of constant θ?

,and

in terms of the Cartesian unit vectors, and examine

their mutual orthogonality.

2.37. The toroidal coordinates (u, θ, ϕ)aregivenby

x =

a sinh u cos ϕ

cosh u − cos θ

y =

a sinh u sin ϕ

cosh θ − cos θ

z =

a sin u

cosh u − cos θ

where a is a constant.

(a) What are the surfaces of constant u?

(b) What are the surfaces of constant θ?

,and

in terms of the Cartesian unit vectors, and examine

their mutual orthogonality.

2.38. The paraboloidal coordinates (u, v, ϕ)aregivenby

x =2auv cos ϕ,

y =2auv sin ϕ,

z = a(u

− v

where a is a constant.

(a) What are the surfaces of constant u?

(b) What are the surfaces of constant v?

,and

in terms of the Cartesian unit vectors, and examine

their mutual orthogonality.

2.39. The three-dimensional bipolar coordinates (u, θ, ϕ)aregivenby

x =

a sin θ cos ϕ

cosh u − cos θ

y =

a sin θ sin ϕ

cosh u − cos θ

z =

a sinh u

cosh u − cos θ

2.4 Problems 75

where a is a constant.

(a) What are the surfaces of constant u?

(b) What are the surfaces of constant θ?

,and

in terms of the Cartesian unit vectors, and examine

their mutual orthogonality.

2.40. Acoordinatesystem(R, Θ,φ) in space is deﬁned by

x = R cos Θ cos φ + b cos φ,

y = R cos Θ sin φ + b sin φ,

z = R sin Θ,

where b is a constant, and 0 <R<b.

1. Express the unit vectors

,and

in terms of Cartesian unit

vectors with coeﬃcients being functions of (R, Θ,φ).

2. Are unit vectors mutually perpendicular?

Chapter 3

Integration: Formalism

It is not an exaggeration to say that the most important concept, whose mas-

tery ensures a much greater understanding of all undergraduate physics, is the

concept of integral. Generally speaking, physical laws are given in local form

Physical laws are

given for

mathematical

points but applied

to extended

objects.

while their application to the real world requires a departure from locality.

For instance, Coulomb’s law in electrostatics and the universal law of gravity

are both given in terms of point particles. These are mathematical points and

the laws assume that. In real physical situations, however, we never deal with

a mathematical point. Usually, we approximate the objects under considera-

tion as points, as in the case of the gravitational force between the Earth and

the Sun. Whether such an approximation is good depends on the properties

of the objects and the parameters of the law. In the example of gravity, on

the sizes of the Earth and the Sun as compared to the distance between them.

On the other hand, the precise motion of a satellite circling the earth requires

more than approximating the Earth as a point; all the bumps and grooves of

the Earth’s surface will aﬀect the satellite’s motion.

This chapter is devoted to a thorough discussion of integrals from a phys-

ical standpoint, i.e., the meaning and the use of the concept of integration

rather than the technique and the art of evaluating integrals.

3.1 “



”Means“



um”

One of the ﬁrst diﬃculties we have to overcome is the preconception instilled

in all of us from calculus that integral is “area under a curve.” This pre-

conception is so strong that in some introductory physics books the authors

translate physical concepts, in which integral plays a natural role, into the

unphysical and unnatural notion of area under a curve. It is true that calcula-

Integral is not just

area under a

curve!

tion of the area under a curve employs the concept of integration, but it does

so only because the calculation happens to be the limit of a sum, and such

limits ﬁnd their natural habitat in many physical situations.

78 Integration: Formalism

Take the gravitational force, for example. As a fundamental physical law,

it is given for point masses, but when we want to calculate the force between

the Earth and the Moon, we cannot apply the law directly because the Earth

and the Moon cannot be considered as points with the Moon being only 60

Earth radii away. This problem was recognized by Newton who found its solu-

tion in integration. Inherent in the concept of integration is the superposition

principle whereby, as mentioned in Chapter 1, diﬀerent parts of a system are

assumed to act independently. Thus a natural procedure is to divide the

big Earth and the big Moon into small pieces, write down the gravitational

force between these small pieces, invoke the superposition principle, and add

the contribution of these pieces to get the total force. Now, nothing is more

natural than this process, and no example is a more illustrative example of

integration than such a calculation.

In order to deﬁne and elucidate the concept of integration,

let us recon-calculation of

ﬁelds of

continuous

distribution of

sources as the

natural setting for

the concept of

integral

sider the gravitational ﬁeld of Box 1.3.5. Instead of a known collection of point

masses, let us calculate the gravitational ﬁeld at a point P of a continuous

distribution of mass such as that distributed in the volume of the Earth.

The point P is called the ﬁeld point.

We divide the large mass into N

pieces, denoting the mass of the ith piece, located around the point P

,by

Δm

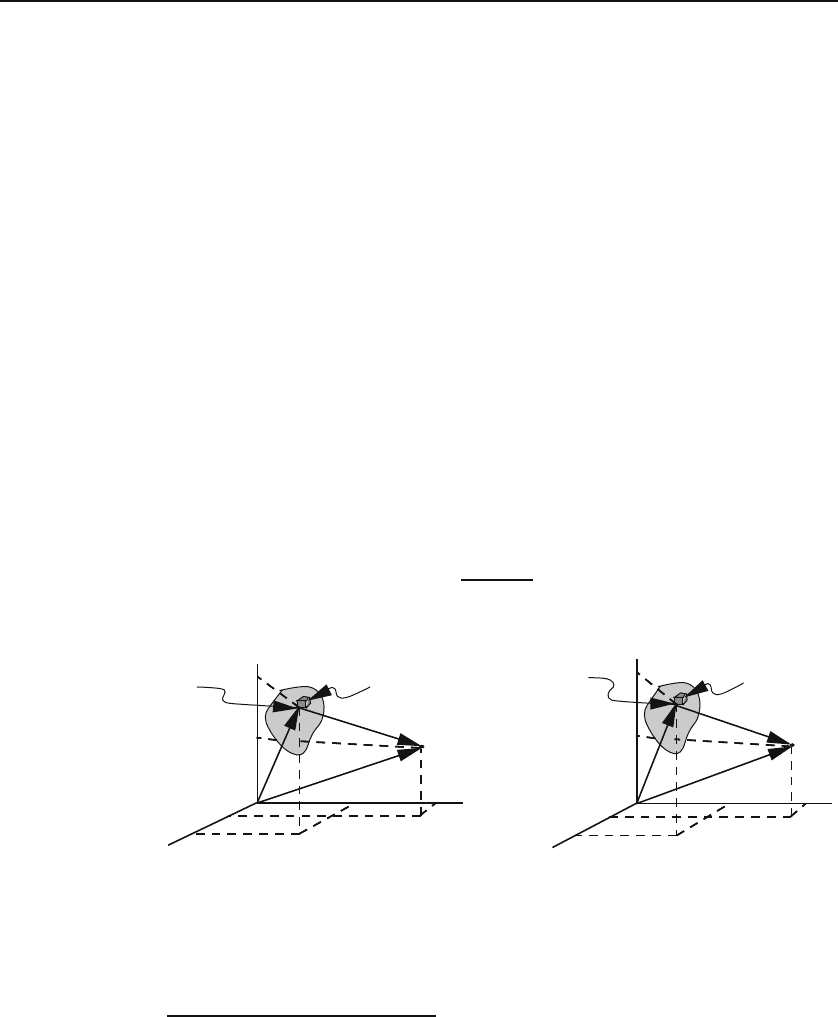
asshowninFigure3.1. Tobeabletoevenwritetheﬁeldequationfor

the ith piece of mass, we have to make sure that the size of Δm

is small

enough. We thus write

≈−

GΔm

|r − r

(r − r

−

(a)

(

)

−

(b)

Figure 3.1: The mass distribution giving rise to a gravitational ﬁeld. (a) The mass is

divided into discrete pieces labeled 1 through N with the ith piece singled out. (b) The

mass is divided into inﬁnitesimal pieces with the piece located at r



singled out.

The discussion that follows may seem speciﬁc to one example, but in reality, it is much

more general. Instead of the gravitational law one can substitute any other local law, and

instead of mass, the appropriate physical quantity must be used. The examples that follow

throughout this chapter will clarify any vague points.

The same terminology applies to electrostatic ﬁelds as well.

3.1 “



”Means“



um” 79

The smaller the size, the better this expression approximates the ﬁeld due to

Δm

. Invoking the superposition principle, we write

g(r) ≈



i=1

= −



i=1

GΔm

|r − r

(r −r

)=−



i=1

GΔm(r

)

|r − r

(r − r

), (3.1)

where in the last equality we have replaced Δm

with Δm(r

). Aside from a

change in notation, this replacement emphasizes the dependence of the small

piece of mass on its “location.” The quotation marks around the last word

need some elaboration. In any practical slicing of the gravitating object, such

as the Earth, each piece still has some nonzero size. This makes it impossible

to deﬁne the distance between Δm

and the point P . We can deﬁne this

distance to be that of the “center” of Δm

from P , but then the diﬃculty

shifts to deﬁning the center of the piece. Fortunately, it turns out that, as long

as we ultimately make the size of all Δm

’s indeﬁnitely small, any point—such

as P

shown in the ﬁgure—in Δm

can be chosen to deﬁne its distance from

P . We are thus led to taking the limit of Equation (3.1) as the size of all

pieces tends to zero, and, necessarily, the number of pieces tends to inﬁnity.

If such a limit exists, we call it the integral of the gravitational ﬁeld and

integral as the

limit of a sum

denote it as follows:

g(r)=− lim

Δm→0

N→∞



i=1

GΔm(r

)

|r − r

(r − r

) ≡−

Gdm(r



)

|r − r



(r − r



). (3.2)

An identical procedure leads to a similar formula for the electrostatic ﬁeld

and potential:

E =

dq(r



)

|r − r



(r − r



), Φ=

dq(r



)

|r − r



. (3.3)

Equations (3.2) and (3.3) will be used frequently in the sequel as we try

to illustrate their use in various physical situations. Note that Equations

(3.2) and (3.3) are independent of any coordinate systems as all physical laws

should be.

In the symbolic representation of integral on the RHS, Ω, called the region

region of

integration

of integration,

is the region—for example, the volume of the Earth—in

which the mass distribution resides, and dm(r



) is called an element of mass

located

at point P



whose position vector is r



. P



is called the source

point because it is the location of the source of the gravitational ﬁeld, i.e.,

the mass element at that point. We also call it the integration point.The

integration point,

integration

variables, and

integrand deﬁned

We shall use the symbol



(or simply



) to indicate general integration without

regard to the dimensionality (single, double, or triple) of the integral.

When the region of integration is one dimensional, such as an interval (a, b)onthereal

line, one uses



instead of



(a,b)

Whenever r



is used as an argument of a quantity, it will refer to the coordinates of a

point not the components of its position vector.

80 Integration: Formalism

coordinates of r



upon which the mass element depends—and in terms of

which it will eventually be expressed—are called the integration variables,

and whatever multiplies the products of the diﬀerentials of these variables is

called the integrand.

It is not hard to abstract the concept of integration from the speciﬁc

example of gravity. Instead of the speciﬁc form of the integral in Equations

(3.2) and (3.3), we use f(r, r



), and instead of the element of mass, we use the

element of some other quantity which we generically designate as dQ(r



). We

thus write

h(r) = lim

ΔQ→0

N→∞



i=1

f(r, r

)ΔQ(r

) ≡

f(r, r



) dQ(r



), (3.4)

where h(r), the result of integration, will be a function of r,theposition

vector of P whose coordinates are called the parameters of integration.

integration

parameters

Although we have used r and r



, the concept of integration does not require

the parameters and integration variables to be position vectors. They could be

any collection of parameters and variables. Nevertheless, we continue to use

the terminology of position vectors and call such collections the coordinates

of points.

An immediate—and important—consequence of the deﬁnition of integral

is that if the region of integration Ω is small, then, for practical calculations,

we do not need to subdivide it into many pieces. In fact, if Ω is small enough,

only one piece may be a good approximation to the integral. We thus write

ΔΩ

f(r, r



) dQ(r



) ≈ f(r, r

)ΔQ, (3.5)

where it is understood that ΔΩ is a small region around point M whose

“position vector” is r

Another immediate and important consequence of the deﬁnition of integral

is that if Ω is divided into two regions Ω

and Ω

,then

f(r, r



) dQ(r



f(r, r



) dQ(r



f(r, r



) dQ(r



) (3.6)

In order to be able to evaluate integrals, one has to express both dQ(r



)

and f(r, r



) in terms of a suitable set of coordinates. f(r, r



) poses no problem,

and in most cases it involves a mere substitution. The element of Q,onthe

other hand, is often related, via density, to the element of volume (or area,

or length) whose expression is more involved. Section 2.3 dealt with the

construction of elements of length, area, and volume in the three coordinate

systems.

Historical Notes

Integral calculus, in its geometric form, was known to the ancient Greeks. For

example, Euclid, by adding pieces to the area of a square inscribed in a circle,

3.2 Properties of Integral 81

constructing newer polygons of larger numbers of sides, and continuing the process

until the circle is “exhausted” by regular polygons, proved the theorem: Circles

are to one another as the squares on the diameters. In essence, Euclid thinks of a

circle as the limiting case of a regular polygon and proves the above theorem for

polygons. Then he uses the argument of “exhaustion” to get to the result. Although

mathematicians of antiquity made frequent use of the method of exhaustion, no one

did it with the mastery of Archimedes.

Archimedes is arguably believed to be the greatest mathematician of antiquity.

The son of an astronomer, he was born in Syracuse, a Greek settlement in Sicily.

As a young man he went to Alexandria to study mathematics, and although he

went back to Syracuse to spend the rest of his life there, he never lost contact with

Alexandria.

Archimedes possessed a lofty intellect, great breadth of interest—both theoret-

Archimedes

287–212 B.C.

ical and practical—and excellent mechanical skills. He is credited with ﬁnding the

areas and volumes of many geometric ﬁgures using the method of exhaustion, the

calculation of π, a new scheme of presenting large numbers in verbal language, ﬁnd-

ing the centers of gravity of many solids and plane ﬁgures, and founding the science

of hydrostatics.

His great achievements in mathematics—he is ranked with Newton and Gauss

as one of the three greatest mathematicians of all time—did not overshadow his

practical inventions. He invented the ﬁrst planetarium and a pump (Archimedean

screw). He showed how to use levers to move great weights, and used compound

pulleys to launch a galley of the king of Syracuse. Taking advantage of the focusing

power of a parabolic mirror, so the story goes, he concentrated the Sun’s rays on

the Roman ships besieging Syracuse and burned them!

Perhaps the most famous story about Archimedes is his discovery of the method

of testing the debasement of a crown of gold. The king of Syracuse had ordered

the crown. Upon delivery, he suspected that it was ﬁlled with baser metal and

sent it to Archimedes to test it for purity. Archimedes pondered about the problem

for some time, until one day, as he was taking a bath, he observed that his body

was partly buoyed up by the water and suddenly grasped the principle—now called

Archimedes’ principle—by which he could solve the problem. He was so ex-

cited about the discovery that he forgetfully ran out into the street naked shouting

“Eureka!” (“I have found it!”).

3.2 Properties of Integral

Now that we have developed the formalism of integration, we should look

at some applications in which integrals are evaluated. As we shall see, all

integral evaluations eventually reduce to integrals involving only one variable.

Thus, it is important to have a thorough understanding of the properties

of such integrals. Some of these properties are familiar, others may be less

familiar or completely new. We gather all these properties here for the sake

of completeness.