Greiner W., Schrammm S., Stein E. Quantum Chromodynamics

Подождите немного. Документ загружается.

3.3 The MIT Bag Model 127

It will be shown in Exercise 3.10 that eigenvectors to the eigenvalue −iare

just the antiparticle solutions to those with the eigenvalue +i. We can therefore

restrict ourselves to one sign. Normally

in(θ, ϕ) ·γ q(x) =−q(x)



R=R (θ,ϕ)

(3.103)

is chosen as the particle solution.

In principle we can choose any bag shape, i.e., an arbitrary function R =

R(θ, ϕ) and a corresponding normal vector n(θ, ϕ). The simplest shape is nat-

urally a sphere, i.e.,

R(θ, ϕ) = R = const , n(θ, ϕ) = e

⇒−i e

·γ q(|x|=R) =q(|x|=R). (3.104)

Obviously there now remains only one parameter that is not ﬁxed by the model

assumptions, the bag radius R. Since it is unsatisfactory to choose R arbitrarily

for every hadron, one is lead to still another form of the boundary condition. This

is obtained by demanding that the pressure of the quarks on the bag surface be

constant. The model assumption leading to this is that the vacuum around the bag

is a complex state exerting some kind of pressure on the bag. If this exceeds the

interior pressure, the bag shrinks; otherwise it inﬂates further. How we can visu-

alize this pressure as arising from the interacting ﬁelds in the vacuum is discussed

in Sect. 7.2. In the following we shall derive this pressure boundary condition.

EXERCISE

3.10 Antiquark Solutions in a Bag

Problem. Show that a quark wave function obeying the equation

in ·γ (x)q(x) =−q(x)



R=R (ϑ,φ)

(1)

corresponds to antiquark solutions that fulﬁll

in ·γ (x)

q(x) =

q(x). (2)

Solution. We have to recall that antiparticle wave functions are

T transforms

of the corresponding particle solutions:

q(x) =

T q(x). (3)

Indeed

T transforms a spinor Ψ(x) into a spinor Ψ(−x) up to a phase factor,

i.e. a particle moving forward in space and time is transformed into one mov-

ing backward in space and time. This corresponds to the Feynman–Stueckelberg

interpretation of antiparticles.

See also W. Greiner and J. Reinhardt: Field Quantization (Springer, Berlin, Heidel-

berg 1996) and W. Greiner: Relativistic Quantum Mechanics – Wave Equations,3rd

ed. (Springer, Berlin, Heidelberg 2000).

128 3. Scattering Reactions and the Internal Structure of Baryons

Exercise 3.10

In the standard representation

C = iγ

P = γ

T = iγ

K being complex comjugation, and therefore

T ...= γ

iγ



iγ

(...)

∗



∗

. (4)

Applying (4) to (1) we obtain

q(x) =



−in ·γ q(x)



= γ

iγ



iγ

(in ·γ

∗

)



q(x)



∗



∗

= γ

iγ



(−in ·γ )iγ



q(x)



∗



∗

= γ

iγ

(in ·γ

∗

)



iγ



q(x)



∗



∗

= in ·γ γ

iγ



iγ



q(x)



∗



∗

= in ·γ

q(x). (5)

Here we have repeatedly made use of the fact that γ

and γ

are real and that γ

purely imaginary.

The antiparticle solutions therefore fulﬁll the modiﬁed boundary condi-

tion (2), i.e. we have only to determine the solutions of (1) in order to obtain the

quark spectrum in the MIT bag.

To calculate the bag pressure we start from the energy momentum tensor for

Dirac particles

µν





qγ

∂

∂x

q −

←−

∂

∂x



. (3.105)

Its canonical form reads

µν



qiγ

∂

∂x

q −g

µν



qiγ

∂

∂x



The last term vanishes because ( p/ −m)q = 0. Writing the ﬁrst term as

(

q∂

q +

q∂

q) and integrating the second expression by parts leads to

(3.105).

W. Greiner and J. Reinhardt: Field Quantization (Springer, Berlin, Heidelberg 1996).

3.3 The MIT Bag Model 129

Using the boundary condition (3.103), which in its most general form is

q =−iq



surface

= i



surface

, (3.106)

it follows that

µν



surface

=−





∂

∂x

q +

←−

∂

∂x





surface

=−

∂

∂x





surface

. (3.107)

With (3.101) it also follows from (3.99) that



surface

= 0 . (3.108)

The gradient in (3.107) therefore points in the direction of the outward normal.

According to the usual deﬁnition the absolute value of n

µν

is just the pressure,

so we conclude that

µν



surface

=−n

. (3.109)

Since n

=(0, −n), the force exerted on the volume element by the pressure is

directed outward. The Dirac pressure (n

=−1)

µν

=−

∂

∂x





surface

(3.110)

is positive. For n

= (0, n) this becomes with n

∂/∂x

=(0, n)



∂/∂x

, −∇



−n ·∇

n ·∇





surface

. (3.111)

According to the model, this quark pressure must equal a constant exterior

pressure B,

B =

n ·∇





R=R (θ,ϕ)

. (3.112)

Equations (3.112) and (3.104), together with the convention that the quarks are

to be considered free inside the bag, deﬁne the MIT bag model. By introducing

the new parameter B, the radii of all hadron bags are ﬁxed.

130 3. Scattering Reactions and the Internal Structure of Baryons

In the following we shall investigate spherical bags.

This is for sure the

most obvious assumption for hadron ground states and has the additional ad-

vantage that the solutions can be found mostly analytically. We are thus looking

for solutions of the stationary free and (for the time being) massless Dirac equa-

tion. The ﬁnite-mass case is discussed in Exercise 3.11. The Dirac equation for

massless particles reads

p/Ψ = 0 (3.113)

with the boundary conditions

−e

·γ Ψ =Ψ



|x|=R



0 −iσ

iσ



Ψ =Ψ



|x|=R

(3.114)

and

−

∂

∂r





|x|=R

= B . (3.115)

Owing to the spherical symmetry of the problem, an expansion into spherical

spinors suggests itself, for example, as in the solution of the hydrogen problem

Ψ =



g(r)χ

(θ, ϕ)

−i f(r)χ

−κ

(θ, ϕ)



−iEt

. (3.116)

The operator α · p is then

α · p =−iα

∂

∂r

(β

K −1) (3.117)

with

Kχ

=−κχ

. (3.107a)

The operator

K = β(Σ ·L +1) has eigenvalues −κ and κ,withκ being deﬁned

κ =−j −

for  = j −

κ = j +

for  = j +

. (3.118)

With α



0 σ



we obtain from (3.113)

−i



0 σ



∂

∂r

−



Ψ = E Ψ. (3.119)

Deformed bags are discussed in D. Vasak, R. Shanker, B. Müller and W. Greiner:

J. Phys. G 9, 511 (1983) in connection with the study of ﬁssioning bags as a form

of overcritical quark–antiquark production.

See e.g. W. Greiner: Relativistic Quantum Mechanics – Wave Equations,3rded.

(Springer, Berlin, Heidelberg 2000).

3.3 The MIT Bag Model 131

Ansatz (3.116) then yields by virtue of

=−χ

−κ

(3.120)

the two coupled equations



−



f(r) = Eg(r) (3.121a)

and





g(r) =−Ef(r) (3.121b)



d(Er)

1 −κ



f = g (3.122a)

and



d(Er)

1 +κ



g =−f . (3.122b)

We replace Er =: z, take the derivative of (3.122a) with respect to z and

eliminate

g via (3.122b):



−

1 −κ



f =

g =−

1 +κ

g − f . (3.121)

From (3.122a) it follows that

1 +κ

g =



1 +κ

1 −κ



f (3.122)

and, by inserting this into (3.121), one arrives at



κ(1 −κ)



f =0 . (3.123)

This is exactly the differential equation satisﬁed by the Bessel function. As f

and g may not diverge more strongly than 1/r at the origin, we must choose the

functions:

f(r) =const × j



(Er), =

)

−κ for κ<0

κ −1forκ>0

. (3.124)

Analogously it follows that

g(r) = const × j



(Er), =

−κ −1for κ<0

κ for κ>0

. (3.125)

132 3. Scattering Reactions and the Internal Structure of Baryons

The factor from (3.125) can be absorbed into overall normalization. It remains

to determine the relative factor A between the f and g functions from (3.122):

f(r) = j



(Er), g(r) = Aj



(Er), (3.126)

⇒



1 −κ



(z) = Aj



(z), (3.127a)



1 +κ



(z) =−j



(z). (3.127b)

For κ<0, (3.127) gives for A = 1 only the usual recursion relations between

spherical Bessel functions of order  and

 =  −sgn(κ), while for κ>0, (3.127)

leads to A =−1.

The wave function of a massless quark in an MIT bag is thus

Ψ = N





(Er)χ

(θ, φ)

isgn(κ) j



(Er)χ

−κ

(θ, φ)



−iEt

. (3.128)

The normalization will be determined later. First we check whether a wave func-

tion of the structure (3.128) can satisfy the boundary conditions at all. We start

with the linear boundary condition (3.114). Using (3.128) and (3.120) it reduces



−sgn(κ) j



(ER)χ

(θ, φ)

−i j



(ER)χ

−κ

(θ, φ)







(ER)χ

(θ, φ)

isgn(κ) j



(ER)χ

−κ

(θ, φ)



(3.129)



(ER) =−sgn(κ) j



(ER). (3.130)

Thus the linear MIT boundary condition can indeed be satisﬁed and yields an

eigenvalue equation for ER.Forκ =−1 we obtain, for example, the solutions

ER|

κ=−1

≈ 2.043, 5.396, 8.578, ... . (3.131)

Some interesting properties of the quark spectrum can be read from the asymp-

totic expansions of the Bessel function.

(1) For a given κ, e.g., κ =−1, the levels become equidistant for large energies.

This follows immediately from

(z) 

cos



z −

l +1



for z →∞. (3.132)

For κ =−1, (3.122) therefore asymptotically becomes

cos



ER−



= cos(ER−π) or tan(ER) =−1 , (3.133)

which is obviously periodic in ER.

3.3 The MIT Bag Model 133

(2) The smaller |κ| becomes, the higher the lowest eigenvalue will be. For very

large values of l,

(z) 

√





,→∞. (3.134)

Condition (3.122) can only be satisﬁed if

1 =





(z)

−1

(z)



√

l −1

√

(l −1)

l−1



l ·z





l ·z



l−1

√

l −1

√

(l −1)

l−1



l ·z





z( −1)

−1

√

 −1



√



⇒ z >>|κ|−1 . (3.135)

In the last but one step, l ≈ 2and(l −1) =l for large l has been used. The small-

est eigenvalue is thus always larger than |κ|−1. These two properties can also

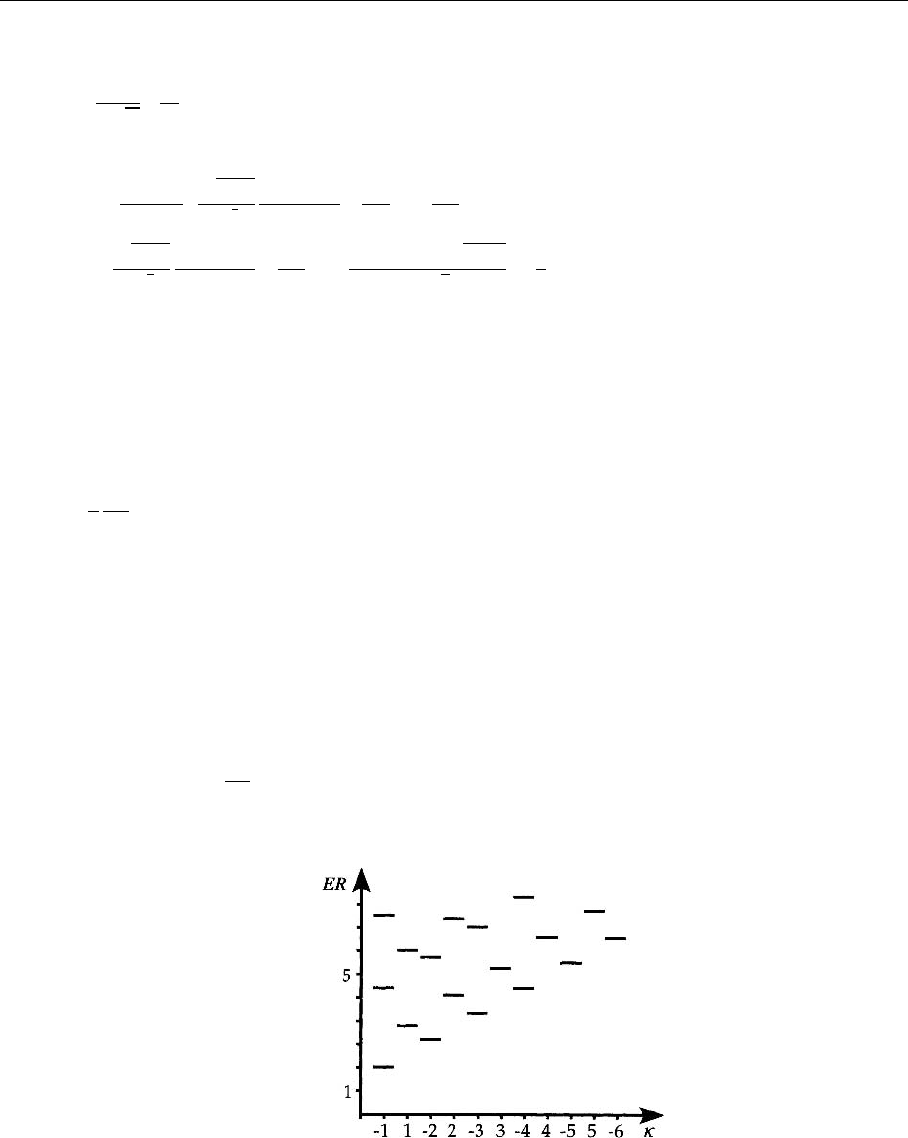
be seen in Fig. 3.14, which shows the lowest-lying energy states.

What about the quadratic boundary condition (3.115); can it also be satisﬁed?

Inserting (3.128) into (3.115), we obtain

B =−







R)χ

(θ, φ)

− j



R)χ

−κ

(θ, φ)

−κ

(θ, φ)



. (3.136)

Equation (3.136) can only be satisﬁed if the right-hand side no longer depends on

θ and φ. This is only the case for κ =±1, or when for higher κ states the q sum

runs over all µ quantum numbers (shells). As we are primarily interested in q

and qqq systems (mesons and baryons), we can neglect this last possibility. The

quadratic boundary condition can therefore only be satisﬁed for |κ|=1. For this

case, |κ|=1, it holds that

µ+

= χ

µ+

−κ

4π

,κ=1 , (3.137)

Fig. 3.14. The lowest-energy

eigenvalues of the quark

states in the MIT bag. R

denotes the bag radius

134 3. Scattering Reactions and the Internal Structure of Baryons

and (3.136) becomes

B =−

4π







R)E

d(E



− j



R)E

d(E



. (3.138)

When all quarks are in the lowest state with κ =−1, it follows from (3.130) and

from the following properties of the j



s,i.e.

d(z)

(z) =

2l +1



l−1

(z) −(l +1) j

l+1

(z)



l+1

(z) + j

l−1

(z) =

2l +1

(z), (3.139)

that

d(ER)



(ER) =

d(ER)

(ER) =−j

(ER), (3.140a)

d(ER)



(ER) =

d(ER)

(ER) = j

(ER) −

(ER)

= j

(ER) −

(ER)

= j

(ER) −

(ER), (3.140b)

and therefore

B =

4π

κ=−1

2Ej

(ER)



1 −



, (3.141)

where N

indicates the number of quarks in the bag.

In Exercise 3.11, Equation (3), we put m = 0andκ =−1 and obtain for the

normalization constant

κ=−1

(ER−1) j

(ER)

, (3.142)

and consequently

B =

4π

or R

4πB

. (3.143)

Equation (3.136) thus yields for any quark content (for full quark shells only!)

the corresponding bag radius as a function of the bag pressure constant B.

3.3 The MIT Bag Model 135

EXERCISE

3.11 The Bag Wave Function for Massive Quarks

Problem. Show that the bag wave function of massive quarks is

Ψ = N





( pr)χ

E+m

sgn(κ) j



( pr)χ

−κ



−iEt

(1)

with

E =





−κ −1forκ<0

κ for κ>0



−κ for κ<0

κ −1forκ>0

Show, furthermore, that the normalization condition

bag

r Ψ

†

Ψ =1(2)

leads to

N =

√

2E(ER+κ) +m



( pR)|R

, (3)

R being the bag radius.

Solution. Adding the mass term to (3.113) yields

( p/ −m)Ψ =0 . (4)

Correspondingly (3.119) becomes



−i



0 σ



∂

∂r

−



+βm



Ψ = E Ψ (5)

and (3.121) then becomes



−



f(r) −(E −m)g(r) = 0(6a)

and





g(r) +(E +m) f(r) = 0 . (6b)

136 3. Scattering Reactions and the Internal Structure of Baryons

Exercise 3.11

Now we insert g(r) = j



( pr) and f(r) =−[p/(E +m)]sgn(κ) j



( pr) and

evaluate the left-hand sides of (6a) and (6b), respectively:

−p

E +m

sgn(κ)



d(pr)

1 −κ



( pr) −(E −m) j



( pr) = 0(7a)

and



d(pr)

1 +κ



( pr) − p sgn(κ) j



( pr) = 0 . (7b)

With the help of recursion relations (3.127) (c =−sgn(κ)) we then obtain

E +m



( pr) −

E +m



( pr) = 0(8a)

and

p sgn(κ) j



( pr) − p sgn(κ) j



( pr) = 0 . (8b)

This yields the wave function of a massive quark in the MIT bag

Ψ = N



lκ

( pr)χ

E+m

sgn(κ) j

lκ

( pr)χ

−κ



−iEt

which transforms into (3.128) for m → 0. Accordingly we obtain from (3.129)

and (3.130) by substituting sgn(κ) →[p/(E +m)]sgn(κ) the modiﬁed linear

boundary condition

lκ

( pr) =−sgn(κ)

E +m

lκ

( pr). (9)

By means of (9) we then evaluate the normalization integral:

1 =

bag

r Ψ

†

drr





( pr) +

(E +m)



( pr)



. (10)

Employing the differentiation and recursion formulas of the Bessel functions

(see (3.139))



(z) =

(z) − j

n+1

(z),



n−1

(z) =

n −1

n−1

(z) − j

(z),



n+1

(z) =−

n +2

n+1

(z) + j

(z),

n+1

(z) + j

n−1

(z) =

2n +1

(z) (12)