Drake G.W.F. (editor) Handbook of Atomic, Molecular, and Optical Physics
Подождите немного. Документ загружается.

High Precision Calculations for Helium 11.4 Total Energies 213
11.4.2 Asymptotic Expansions
The asymptotic expansion method [11.39, 55] rapidly
increases in accuracy with increasing angular momen-
tum L of the Rydberg electron, and can be used to high
precision for L ≥ 7. The method is based on a model in
which:
1. the Rydberg electron, treated as a distinguishable
particle, moves in the field of the core consisting of
the He nucleus and a tightly bound 1s electron.
2. the core, as characterized by its various multipole
moments, is perturbed by the electric field of the
Rydberg electron.
A systematic perturbation expansion yields an
asymptotic series of the form
ε
nL
0
=−2 −
(Z − 1)
2
2n
2
+ a
0
N
j=4
A
j
r
− j
nL
e
2
a
0
,
(11.56)
where the expectation value r
− j
nL
is calculated
with respect to the hydrogenic nL-electron wave func-
tion [11.56] and the series is truncated at the upper
limit N where the series begins diverging. The leading
coefficients A
j
are
A
4
=−
1
2
α
1
, A
5
= 0 , A
6
=−
1
2
(α
2
− 6β
1
),
where α
k
is the 2
k
-pole polarizability of the hydro-
genic core and β
k
is a nonadiabatic correction. The exact
hydrogenic values are
α
1
=
9a
3
0
2Z
4
,α
2
=
15a
5
0
Z
6
,β
1
=
43a
5
0
8Z
6
.
All terms are known up to A
10
(see [11.39, 55] for de-
tailed results). The expansions for the terms ε
0
, ε
1
,and
ε
2
in (11.6) for helium are
ε
nL
0
=−2 −
1
2n
2
−
9
64
r
−4
+
69
512
r
−6
+
3833
15 360
r
−7
−
55 923
65 536
+
957L(L + 1)
10 240
r
−8
−
908 185
688 128
r
−9
+
3 824 925
1 048 576
+
33 275L(L + 1)
28 672
r
−10
+ e
(1,1)
−
23
20
e
(1,2)
, (11.57)
ε
nL
1
=−
9
32
r
−4
+
249
256
r
−6
+
319
3840
r
−7
−
34 659
16 384
+
957L(L + 1)
5120
r
−8
−
14 419
3072
r
−9
+
6 413 781
262 144
+
24 155L(L + 1)
8192
r
−10
+ 4e
(1,1)
−
53
5
e
(1,2)
, (11.58)
ε
nL
2
=−
1
2n
2
−
45
64
r
−4
+
165
512
r
−6
+
2555
3072
r
−7
−
268 485
32 768
+
957L(L + 1)
2048
r
−8
+
598 909
172 032
r
−9
+
3 907 923
524 288
+
629 515L(L + 1)
114 688
r
−10
+ 14 e
(1,1)
−
251
10
e
(1,2)
. (11.59)
The terms e
(1,1)
and e
(1,2)
are second-order dipole–
dipole and dipole–quadrupole perturbation corrections.
Defining f
L
p
= (L + p)!/(L − p)!, they are given by
e
(i, j )
=−
(2 − δ
j,k
)2
2i+2 j+1
(2L − 2i )!(2L − 2 j )!
n
3
(2L + 2i + 1)!(2L + 2 j + 1)!
×
2
2i+2 j
(2L − 2i − 2 j )!A
(i, j )
n
2i+2 j+2
(2L + 2i + 2 j + 1)!
+
B
(i, j )
n
2i+2 j+1
(11.60)
with
A
(1,1)
= 3n
2
(3n
2
− 2 f
1
)( f
1
− 2)
#
45 + 623 f
L
1
+ 3640 f
L
2
+ 560 f
L
3
$
,
B
(1,1)
=
#
9n
2
− 7 f
L
1
$#
3n
2
− f
L
1
$
,
A
(1,2)
=−21n
6
#
94 500 + 122 850 f
L
1
− 1 126 125 f
L
2
− 18 931 770 f
L
3
− 11 171 160 f
L
4
− 1 029 600 f
L
5
− 18 304 f
L
6
$
− 15n
4
#
94 500 − 444 150 f
L
1
+ 7 747 425 f
L
2
+ 337 931 880 f
L
3
+ 375 290 190 f
L
4
+ 66 518 760 f
L
5
Part B 11.4
214 Part B Atoms
+ 2 880 416 f
L
6
+ 29 568 f
L
7
$
+ 9n
2
f
L
1
#
90 300 − 177 450 f
L
1
+ 1 738 450 f
L
2
+ 133 125 575 f
L
3
+ 160 040 870 f
L
4
+ 29 322 216 f
L
5
+ 1 293 600 f
L
6
+ 13 440 f
L
7
$
+ 2 f
L
1
f
L
2
f
L
3
#
45 + 252 f
L
1
− 1680 f
L
2
− 2240 f
L
3
$
,
B
(1,2)
= 315n
6
+ 125n
4
#
3 − 5 f
L
1
$
− 7n
2
f
L
1
#
43 − 39 f
L
1
$
− 27 f
L
1
f
L
2
.
The accuracy of the expansion for the ε
0
, ε
1
,andε
2
can
be reliably estimated to be one-half of the last r
− j
term
included in the sum. Formulas for the r
− j
are given in
Table 11.10.
The asymptotic formulas for the NFS relativistic
corrections are [11.39, 57]
H
mass
+ H
D
→ −
α
2
Z
4
8
+ h
1
(nL) + χ
1
(nL)
+
(Zα)
2
2
14
3Z
4
r
−4
−
5041
240Z
6
r
−6
(11.61)
H
oo
→
α
2
Z
2
r
−4
+
3(Z − 1)
Z
2
r
−5
−
3
#
f
L
1
+ 8
$
4Z
2
r
−6
,
(11.62)
where
h
1
(nL) =
α
2
(Z − 1)
4
2n
3
3
4n
−
1
L +
1
2
(11.63)
is the leading one-electron Dirac energy and
χ
1
(nL) =
α
2
α
1
2
3
Z − 1
n
2
r
−4
− (Z − 1)
r
−5
−
4(2L − 2)!
(2L + 3)!
×
4
Z − 1
n
6
n +
9n
2
− 5 f
L
1
2L + 1
+(Z − 1)
2
40 f
L
2
+ 70 f
L
1
− 3
2L + 1
r
−4
(11.64)
is the correction due to the dipole perturbation of the
Rydberg electron. The relativistic recoil terms due to
Table 11.10 Formulas for the hydrogenic expectation value
r
− j
≡nl|r
− j
|nl in terms of
G
nl
p
=
2
p
Z
p
(2l − p + 2)!
n
p+1
(2l + p − 1)!
, f
l
p
=
(l + p)!
(l − p)!
.
j
r
− j
(a
0
)
2
1
2
G
nl
2
3 nG
nl
3
4 G
nl
4
#
3n
2
− f
l
1
$
5 2G
nl
5
5n
3
− n
#
3 f
l
1
− 1
$
6 G
nl
6
35n
4
− 5n
2
#
6 f
l
1
− 5
$
+ 3 f
l
2
7 2G
nl
7
63n
5
− 35n
3
#
2 f
l
1
− 3
$
+ n
#
15 f
l
2
− 20 f
l
1
+ 12
$
8 G
nl
8
462n
6
− 210n
4
#
3 f
l
1
− 7
$
+42n
2
#
5 f
l
2
− 15 f
l
1
+ 14
$
− 10 f
l
3
9 2G
nl
9
858n
7
− 462n
5
#
3 f
l
1
− 10
$
+ 42n
3
#
15 f
l
2
− 75 f
l
1
+101
$
− 2n
#
35 f
l
3
− 105 f
l
2
+ 252 f
l
1
− 180
$
10 G
nl
10
6435n
8
− 6006n
6
#
2 f
l
1
− 9
$
+ 1155n
4
#
6 f
l
2
− 44 f
l
1
+81
$
− 6n
2
#
210 f
l
3
− 1365 f
l
2
+ 4648 f
l
1
− 4566
$
+35 f
l
4
mass polarization are
H
mass
+ H
D
RR
→
µ
M
22(Zα)
2
(Z − 1)
9Z
4
r
−4
+ 2(Z − 1)χ
1
(nL)
+
µ
M
2
−
5
12
αZ(Z − 1)
n
2
+ 4h
1
(nL)
,
(11.65)
H
oo
RR
+∆
oo
→−
α
2
µ
M
Z
4
+
(Z − 1)
4
n
3
1
n
−
3
2L + 1
−
25[1 + 13 f(Z)]
16Z
2
r
−4
,
(11.66)
with f(Z) 1 + (Z − 2)/6. The −(5/12)[αZ(Z −
1)/n]
2
term in (11.65) is the dominant contribution in
helium for L ≥ 4. It is included in (11.54)for∆W
n
,
along with the leading 1/n
2
term from (11.59), and the
1/n
4
term from (11.63). The complete relativistic finite
Part B 11.4
High Precision Calculations for Helium 11.5 Radiative Transitions 215
mass correction includes also the mass-scaling terms
−(µ/M)4H
mass
+ 3H
D
+ 3H
oo
obtained by expand-
ing µ/m
e
in (11.47). The δ(r
1
) term is [11.58]
πδ(r
1
)→
Z
3
2
−
31
4Z
3
r
−4
+
1447
32Z
5
r
−7
−
−31(Z − 1)
2Z
3
µ
M
r
−4
+··· .
(11.67)
δ(r
12
) vanishes exponentially as 1/n
2L+4
with increas-
ing L. The complete asymptotic expressions for the FS
matrix elements are summarized by the formulas
nL
3
L
J
|H
FS
|nL
3
L
J
→ T
nL
(J ){Z − 3 + 2S
L
(J ) + 2a
e
[Z − 2
+ (2 + a
e
)S
L
(J )]+(µ/M)[2 − 4S
L
()]} ,
(11.68)
nL
3
L
J
|H
FS
|nL
1
L
J
→ T
nL
(L)(Z + 1 + 2a
e
Z − 2µ/M)
'
L(L + 1),
(11.69)
where
T
nL
(J ) =
−α
2
(L + 1)
r
−3
/4 J = L − 1 ,
−α
2
r
−3
/4 J = L ,
α
2
L
r
−3
/4 J = L + 1 ,
S
L
(J ) =
1 J = L ,
±1/(2J +1) J = L ± 1 .
The asymptotic form for the QED term ∆E
L,1
fol-
lows from (11.38) with the use of (11.67)forδ(r
1
) and
(11.41)forlnk
0
. The electron–electron part is
∆E
L,2
→−
7α
3
6π
r
−3
+
3
Z
2
r
−5
.
(11.70)
With the use of the formulas in this section, the vari-
ationally calculated ionization energies for the K-states
(L = 7) in Table 11.7 can be reproduced to within ±20
Hz. For L > 7, the uncertainty becomes less than 1 Hz,
up to the Casimir-Polder retardation effects which have
not been included.
11.5 Radiative Transitions
11.5.1 Basic Formulation
In a semiclassical picture, the interaction Hamiltonian
with the radiation field is obtained by making the mini-
mal coupling replacements
P
N
→ P
N
−
Ze
c
A(R
N
)
P
i
→ P
i
+
e
c
A
i
(R
i
) (11.71)
in (11.1), where
A(R) = c
2π
ωV
1/2
ˆ
e
ik·R
(11.72)
is the time-independent part of the vector potential
A(r, t) = A(r)e
−iωt
+ c.c for a photon of frequency ω,
wave vector k, and polarization
ˆ
⊥ knormalized to unit
photon energy
ω in volume V. The linear coupling
terms then yield
H
int
=−
Ze
Mc
P
N
· A(R
N
) +
e
m
e
c
2
i=1
P
i
· A(R
i
),
(11.73)
and from Fermi’s Golden Rule, the decay rate for spon-
taneous emission from state γ to γ
is
w
γ,γ
dΩ =
2π
|γ |H
int
|γ
|
2
ρ
f
, (11.74)
where ρ
f
= Vω
2
dΩ/(2πc)
3
is the number of photon
states with polarization
ˆ
per unit energy and solid angle
in the normalization volume V. In the long wavelength
and electric dipole approximations, the factor e
ik·R
in
(11.72) is replaced by unity. After integrating over angles
dΩ and summing over polarizations
ˆ
, the decay rate
reduces to
w
γ,γ
=
4
3
αω
γ,γ
|γ |Q
p
|γ
|
2
, (11.75)
where ω
γ
,γ
is the transition frequency and Q
p
is the
velocity form of the transition operator
Q
p
=−
Z
Mc
P
N
+
1
m
e
c
N
i=1
P
i
(11.76)
for the general case of N electrons. From the commu-
tator [H
0
, Q
r
/ ω
γ,γ
]=Q
p
,whereH
0
is the field-free
Hamiltonian in (11.1), the equivalent length form is
Q
r
=−
i
c
ω
γ,γ
Z R
N
−
N
i=1
R
i
.
(11.77)
Part B 11.5

216 Part B Atoms
After transforming to c.m. plus relative coordinates
in parallel with (11.3), the dipole transition operators
become
Q
p
=
Z
p
m
e
c
N
i=1
p
i
, Q
r
=
iω
γ,γ
c
Z
r
N
i=1
r
i
,
(11.78)
with
Z
p
=
Zm
e
+ M
M
, Z
r
=
Zm
e
+ M
Nm
e
+ M
,
and H
0
now contains the H
mp
term. If (11.3)issolved
exactly for the states |γ and |γ
, then the identity
γ |Q
p
|γ
=γ |Q
r
|γ
(11.79)
is satisfied to all orders in m
e
/M. For a neutral atom,
N = Z and Z
r
= 1. If the oscillator strength is defined
by
f
γ
,γ
=
2m
e
ω
γ
,γ
3
Z
r
Z
p
(
(
(
(
(
)
γ
(
(
(
(
(
N
i=1
r
i
(
(
(
(
(
γ
*
(
(
(
(
(
2
=
2
3m
e
ω
γ
,γ
Z
p
Z
r
(
(
(
(
(
)
γ
(
(
(
(
(
N
i=1
p
i
(
(
(
(
(
γ
*
(
(
(
(
(
2
(11.80)
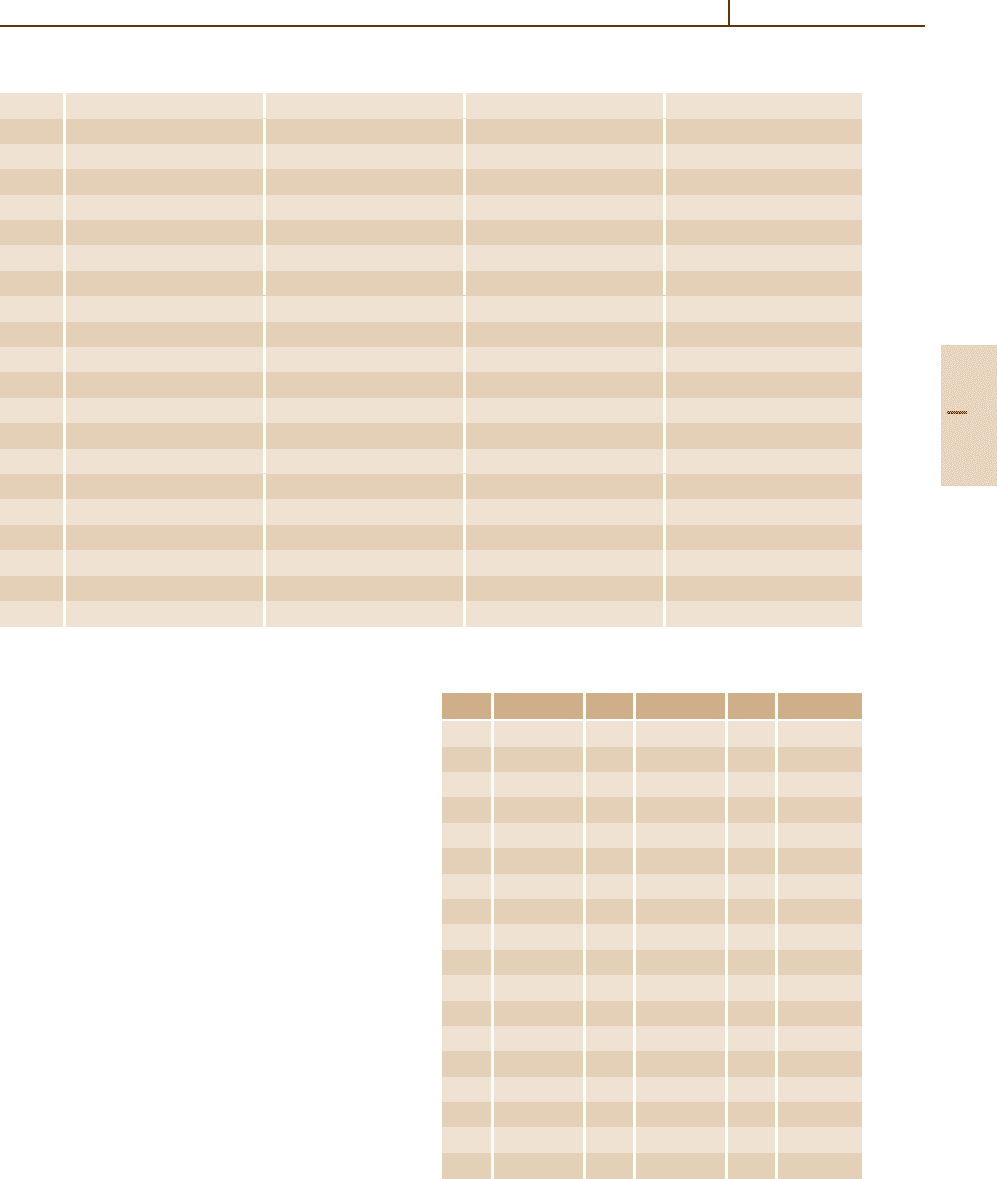
Table 11.11 Oscillator strengths for helium. The factor in brackets gives the finite mass correction, with y = µ/M
1
1
S2
1
S3
1
S4
1
S
2
1
P 0.276 1647(1 − 2.282y) 0.376 4403(1 + 1.255y) − 0.145 4703(1 + 1.351y) − 0.025 8703(1 + 0.885y)
3
1
P 0.073 4349(1 − 1.789y) 0.151 3417(1 − 3.971y) 0.626 1931(1 + 1.234y) − 0.307 5074(1 + 1.097y)
4
1
P 0.029 8629(1 − 1.583y) 0.049 1549(1 − 3.235y) 0.143 8889(1 − 4.650y) 0.858 0214(1 + 1.205y)
5
1
P 0.015 0393(1 − 1.474y) 0.022 3377(1 − 2.967y) 0.050 4714(1 − 3.764y) 0.146 2869(1 − 5.080y)
6
1
P 0.008 6277(1 − 1.407y) 0.012 1340(1 − 2.829y) 0.024 1835(1 − 3.444y) 0.052 7562(1 − 4.105y)
7
1
P 0.005 4054(1 − 1.362y) 0.007 3596(1 − 2.75y) 0.013 6794(1 − 3.279y) 0.025 8918(1 − 3.75y)
2
3
S3
3
S4
3
S5
3
S
2
3
P 0.539 0861(1 − 3.185y) − 0.208 5359(1 − 3.773y) − 0.031 7208(1 − 2.819y) − 0.011 3409(1 − 2.609y)
3
3
P 0.064 4612(1 + 5.552y) 0.890 8513(1 − 2.967y) − 0.435 6711(1 − 3.362y) − 0.067 6073(1 − 2.359y)
4
3
P 0.025 7689(1 + 3.886y) 0.050 0833(1 + 7.505y) 1.215 2630(1 − 2.878y) − 0.668 3003(1 − 3.185y)
5
3
P 0.012 4906(1 + 3.332y) 0.022 9141(1 + 5.209y) 0.044 2305(1 + 9.009y) 1.530 6287(1 − 2.827y)
6
3
P 0.006 9822(1 + 3.063y) 0.011 9933(1 + 4.460y) 0.021 6301(1 + 6.198y) 0.041 5177(1 + 10.215y)
7
3
P 0.004 2990(1 + 2.908y) 0.007 0772(1 + 4.092y) 0.011 7754(1 + 5.292y) 0.021 1003(1 + 6.981y)
2
1
P3
1
P4
1
P5
1
P
3
1
D 0.710 1641(1 − 0.281y) − 0.021 1401(1 + 29.947y) − 0.015 3034(1 − 6.680y) −0.003 1128(1 − 6.27y)
4
1
D 0.120 2704(1 − 1.307y) 0.648 1049(1 + 0.435y) − 0.040 0610(1 + 29.183y) − 0.039 2932(1 − 6.163y)
5
1
D 0.043 2576(1 − 1.681y) 0.141 3027(1 − 0.566y) 0.647 6679(1 + 0.817y) − 0.057 3258(1 + 28.903y)
6
1
D 0.020 9485(1 − 1.866y) 0.056 2766(1 − 0.936y) 0.152 8104(1 − 0.170y) 0.669 8361(1 + 1.056y)
7
1
D 0.011 8970(1 − 1.975y) 0.028 8961(1 − 1.127y) 0.063 5953(1 − 0.538y) 0.163 0272(1 + 0.082y)
8
1
D 0.007 4645(1 − 2.046y) 0.017 0777(1 − 1.241y) 0.033 6403(1 − 0.731y) 0.069 3063(1 − 0.26y)
then the sum rule
γ
f
γ
,γ
= N remains valid,
independent of m
e
/M. The decay rate, summed
over final states and averaged over initial states,
is
¯
w
γ,γ
=−
2α
ω
2
γ,γ
m
e
c
2
Z
p
Z
r
¯
f
γ,γ
, (11.81)
where
¯
f
γ,γ
=−(g
γ
/g
γ
)
¯
f
γ
,γ
is the (negative) oscilla-
tor strength for photon emission, and g
γ
, g
γ
are the
statistical weights of the states.
11.5.2 Oscillator Strength Table
Table 11.11 provides arrays of nonrelativistic oscillator
strengths among various states of helium, including the
effects of finite nuclear mass as a separate factor. In the
absence of mass polarization, the correction factor would
be (1 + µ/M)
−1
1 − µ/M. Mass polarization effects
are particularly strong for P-states, and for transitions
with ∆n = 0.
The largest relativistic correction comes from
singlet–triplet mixing between states with the same n,
L,andJ (e.g. 3
1
D
2
and 3
3
D
2
) due to H
FS
.Thewave
functions obtained by diagonalizing the 2 × 2 matrices
Part B 11.5
High Precision Calculations for Helium 11.5 Radiative Transitions 217
Table 11.11 Oscillator strengths for helium. The factor in brackets gives the finite mass correction, with y = µ/M, cont.
2
3
P3
3
P4
3
P5
3
P
3
3
D 0.610 2252(1 − 2.029y) 0.112 1004(1 + 6.653y) − 0.036 9592(1 + 3.292y) − 0.006 9009(1 + 2.678y)
4
3
D 0.122 8469(1 − 1.001y) 0.477 5938(1 − 3.059y) 0.200 9498(1 + 6.368y) − 0.088 3017(1 + 2.939y)
5
3
D 0.047 0071(1 − 0.631y) 0.124 5532(1 − 2.019y) 0.438 3888(1 − 3.607y) 0.280 0558(1 + 6.225y)
6
3
D 0.023 4692(1 − 0.449y) 0.053 0093(1 − 1.631y) 0.123 9414(1 − 2.555y) 0.429 4411(1 − 3.961y)
7
3
D 0.013 5638(1 − 0.346y) 0.028 1587(1 − 1.432y) 0.055 2332(1 − 2.153y) 0.125 2389(1 − 2.904y)
8
3
D 0.008 6047(1 − 0.280y) 0.016 9809(1 − 1.315y) 0.030 2853(1 − 1.94y) 0.057 0589(1 − 2.498y)
3
1
D4
1
D5
1
D6
1
D
4
1
F 1.015 0829(1 − 1.010y) 0.002 4920(1 + 3.833y) − 0.012 6968(1 − 0.888y) − 0.002 2631(1 − 0.890y)
5
1
F 0.156 8808(1 − 0.993y) 0.886 1343(1 − 1.023y) 0.004 6467(1 + 4.139y) − 0.033 2539(1 − 0.893y)
6
1
F 0.054 0508(1 − 0.984y) 0.186 0576(1 − 1.001y) 0.839 1374(1 − 1.031y) 0.006 6028(1 + 4.302y)
7
1
F 0.025 6799(1 − 0.978y) 0.072 3229(1 − 0.994y) 0.196 3692(1 − 1.014y) 0.826 9464(1 − 1.039y)
8
1
F 0.014 4782(1 − 0.978y) 0.036 6627(1 − 0.987y) 0.080 7847(1 − 1.003y) 0.203 1182(1 − 1.019y)
9
1
F 0.009 0730(1 − 0.977y) 0.021 5401(1 − 0.975y) 0.042 4256(1 − 1.000y) 0.086 0955(1 − 1.01y)
3
3
D4
3
D5
3
D6
3
D
4
3
F 1.014 3389(1 − 0.997y) 0.003 3992(1 − 2.166y) − 0.012 8084(1 − 1.042y) − 0.002 2830(1 − 1.044y)
5
3
F 0.156 9831(1 − 1.004y) 0.884 5767(1 − 0.991y) 0.006 5121(1 − 2.387y) − 0.033 5369(1 − 1.043y)
6
3
F 0.054 1179(1 − 1.006y) 0.186 0264(1 − 1.003y) 0.837 0221(1 − 0.988y) 0.009 3836(1 − 2.499y)
7
3
F 0.025 7201(1 − 1.008y) 0.072 3579(1 − 1.003y) 0.196 2031(1 − 0.996y) 0.824 4031(1 − 0.984y)
8
3
F 0.014 5037(1 − 1.009y) 0.036 6936(1 − 1.004y) 0.080 7712(1 − 1.00y) 0.202 8407(1 − 0.993y)
9
3
F 0.009 0903(1 − 1.008y) 0.021 5632(1 − 1.011y) 0.042 4344(1 − 0.99y) 0.086 0373(1 − 0.99y)
H
0
+ H
NFS
+ H
FS
are then
Ψ(n
1
L
L
) = Ψ
0
(n
1
L
L
) cos θ + Ψ
0
(n
3
L
L
) sin θ
Ψ(n
3
L
L
) =−Ψ
0
(n
1
L
L
) sin θ + Ψ
0
(n
3
L
L
) cos θ.
Val ue s of s in θ are listed in Table 11.12.Thecor-
rected oscillator strengths
˜
f
γ,γ
for the singlet (s) and
triplet (t) components of a γ → γ
transition can then be
calculated from the values in Table 11.11 according to
˜
f
ss
γ,γ
= ω
ss
γ,γ
X
ss
γ,γ
cos θ
γ
cos θ
γ
+X
tt
γ,γ
sin θ
γ
sin θ
γ
2
,
˜
f
tt
γ,γ
= ω
tt
γ,γ
X
ss
γ,γ
sin θ
γ
sin θ
γ
+X
tt
γ,γ
cos θ
γ
cos θ
γ
2
,
˜
f
st
γ,γ
= ω
st
γ,γ
X
ss
γ,γ
cos θ
γ
sin θ
γ
−X
tt
γ,γ
sin θ
γ
cos θ
γ
2
,
˜
f
ts
γ,γ
= ω
ts
γ,γ
X
ss
γ,γ
sin θ
γ
cos θ
γ
−X
tt
γ,γ
cos θ
γ
sin θ
γ
2
,
Table 11.12 Singlet–triplet mixing angles for helium
State sinθ State sinθ State sinθ
2P 0.000 2783
3P 0.000 2558 3D 0.015 6095
4P 0.000 2498 4D 0.011 3960 4F 0.604 1024
5P 0.000 2473 5D 0.010 1143 5F 0.549 9291
6P 0.000 2460 6D 0.009 5289 6F 0.518 0737
7P 0.000 2452 7D 0.009 2067 7F 0.498 4184
8P 0.000 2447 8D 0.009 0087 8F 0.485 5768
9P 0.000 2444 9D 0.008 8777 9F 0.476 7620
10P 0.000 2442 10D 0.008 7862 10F 0.470 4595
5G 0.693 4752
6G 0.693 1996 6H 0.696 2385
7G 0.692 9889 7H 0.696 2377 7I 0.697 9315
8G 0.692 8356 8H 0.696 2372 8I 0.697 9315
9G 0.692 7195 9H 0.696 2374 9I 0.697 9316
10G 0.692 6329 10H 0.696 2353 10I 0.697 9316
8K 0.699 1671
9K 0.699 1671 9L 0.700 1089
10K 0.699 1671 10L 0.700 1089 10M 0.700 8507
Part B 11.5

218 Part B Atoms
where X
ss
γ,γ
= ( f
ss
γ,γ
/ω
ss
γ,γ
)
1/2
, and similarly for X
tt
γ,γ
.
From (11.80), X
γ,γ
is proportional to the dipole length
form of the transition operator, for which there are
no spin-dependent relativistic corrections [11.59, 60].
The mixing corrections are particularly significant for
D–F and F–G transitions, where intermidiate cou-
pling prevails. The two-state approximation becomes
increasingly accurate with increasing L, but for P-states,
where sin θ is small, states with n
= n must also be
included [11.61].
11.6 Future Perspectives
The variational calculations, together with quantum
defect extrapolations for high n and asymptotic ex-
pansions for high L, provide essentially exact results
for the entire singly-excited spectrum of helium. In
this sense, helium joins hydrogen as a fundamental
atomic system. The dominant uncertainties arise from
two-electron QED effects beyond the current realm of
standard atomic physics. Transition frequencies from the
1s2s
1
S
0
state are now known to better than ±0.5MHz
(±1.8 parts in 10
9
) [11.62, 63], and the fine struc-
ture intervals in the 1s2p
3
P state have been measured
to an accuracy exceeding 1 kHz [11.64]. Comparisons
with theory [11.65, 66] hold the promise of determining
an “atomic physics” value for the fine structure con-
stant. Transition frequencies among the n = 10 states are
known even more accurately [11.53]. Recent progress in
the use of isotope the shift to deduce the size of the nu-
cleus from the nuclear volume effect (see Sect. 90.1)has
attracted a great deal of attention, especially for neutron-
rich “halo” nuclei such as
6
He and
11
Li. [11.67, 68]
References
11.1 T. Kato: Commun. Pure Appl. Math. 10,151(1957)
11.2 M. Hoffman-Ostenhoff, T. Hoffmann-Ostenhoff,
H. Stremnitzer: Phys. Rev. Lett. 68, 3857 (1992)
11.3 V. A. Fock: Izv. Akad. Nauk SSSR, Ser. Fiz. 18,161
(1954)
11.4 V. A. Fock: D. Kngl. Norske Videnskab. Selsk. Forh.
31,138(1958)
11.5 V. A. Fock: D. Kngl. Norske Videnskab. Selsk. Forh.
31,145(1958)
11.6 G. B. Sochilin: Int. J. Quantum Chem. 3, 297 (1969)
11.7 C. R. Myers, C. J. Umriger, J. P. Sethna, J. D. Mor-
gan III: Phys. Rev. A 44, 5537 (1991)
11.8 J. E. Gottschalk, E. N. Maslen: J. Phys. A 20,2781
(1987)
11.9 K. McIsaac, E. N. Maslen: Int. J. Quantum Chem. 31,
361 (1987)
11.10 J. H. Macek: Phys. Rev. 160, 170 (1967)
11.11 J. Leray: Trends and Applications of Pure Math-
ematics to Mechanics, Lect. Notes Phys., Vol. 195,
ed. by P. G. Ciarlet, M. Roseau (Springer, Berlin,
Heidelberg 1984) pp. 235–247
11.12 J. D. Morgan III: Theoret. Chem. Acta 69,181(1986)
11.13 P. Deift, W. Hunziker, B. Simon, E. Vock: Commun.
Math. Phys. 64,1(1978)
11.14 J. D. Morgan III: J. Phys. A 10, L91 (1977)
11.15 M. Hoffman-Ostenhoff, T. Hoffmann-Ostenhoff:
Phys. Rev. A 16, 1782 (1977)
11.16 R. Ahlrichs, T. Hoffman-Ostenhoff, M. Hoffmann-
Ostenhoff, J. D. Morgan III: Phys. Rev. A 23, 2107
(1981)
11.17 E. A. Hylleraas, B. Undheim: Z. Phys. 65, 759 (1930)
11.18 J. K. L. MacDonald: Phys. Rev. 43, 830 (1933)
11.19 E. A. Hylleraas: Z. Phys. 48, 469 (1928)
11.20 E. A. Hylleraas: Z. Phys. 54, 347 (1929)
11.21 Y. Accad, C. L. Pekeris, B. Schiff: Phys. Rev. A 4,516
(1971)
11.22 K. Frankowski, C. L. Pekeris: Phys. Rev. 146,46
(1966)
11.23 K. Frankowski, C. L. Pekeris: Phys. Rev. 150, 366(E)
(1966)
11.24 D. E. Freund, B. D. Huxtable, J. D. Morgan III: Phys.
Rev. A 29,980(1984)
11.25 A. J. Thakkar, T. Koga: Phys. Rev. A 50, 854 (1994)
11.26 G. W. F. Drake: Nucl. Instrum. Methods Phys. Res.
Sect B 31, 7 (1988)
11.27 G. W. F. Drake, Z.-C. Yan: Phys. Rev. A 46, 2378
(1992)
11.28 G. W. F. Drake: Long Range Casimir Forces: Theory
and Recent Experiments in Atomic Systems,ed.by
F. S. Levin, D. A. Micha (Plenum Press, New York
1993) p. 107
11.29 G. W. F. Drake, Z.-C. Yan: Chem. Phys. Lett. 229,486
(1994)
11.30 A. M. Frolov, V. H. Smith: J. Phys. B 37, 2917 (2004)
11.31 V. Korobov: Phys. Rev. A 69, 0545012 (2004) The
Bethe logarithms calculated by Korobov include an
additional ln Z
2
in their definition
11.32 G. W. F. Drake: Phys. Rev. A 18, 820 (1978)
11.33 Z.-C. Yan, G. W. F. Drake: Can. J. Phys. 72, 822
(1994)
Part B 11

High Precision Calculations for Helium References 219
11.34 J. Ackermann: Phys. Rev. A 52, 1968 (1995) and
earlier references therein
11.35 C.-Y. Hu, A. A. Kvitsinsky, J. S. Cohen: J. Phys. B 28,
3629 (1995) and earlier references therein
11.36 R. Krivec, V. B. Mandelzweig, K. Varga: Phys. Rev.
61, 062503 (2000) and earlier references therein
11.37 A. H. Wapstra, G. Audi: Nucl. Phys. A 432,1(1985)
11.38 A. Dalgarno, N. Lynn: Proc. Phys. Soc. (London) A
70, 802 (1957)
11.39 G. W. F. Drake: Adv. At. Mol. Opt. Phys. 31, 1 (1993)
11.40 G. W. F. Drake, S. P. Goldman: Can. J. Phys. 77,835
(1999)
11.41 S. P. Goldman, G. W. F. Drake: Phys. Rev. Lett. 68,
1683 (1992)
11.42 G. W. F. Drake: Phys. Scr. T95, 22 (2001)
11.43 G. W. F. Drake, R. A. Swainson: Phys. Rev. A 41,1243
(1990)
11.44 A. P. Stone: Proc. Phys. Soc. (London); 77,786
(1961)
11.45 A. P. Stone: Proc. Phys. Soc. (London); 81,868
(1963)
11.46 G. W. F. Drake: Phys. Rev. Lett. 74, 4791 (1995)
11.47 K. Pachucki: Phys. Rev. Lett. 84, 4561 (2000)
11.48 V. Korobov, A. Yelkhovsky: Phys. Rev. Lett. 87,
193003 (2001)
11.49 G. W. F. Drake, I. B. Khriplovich, A. I. Milstein,
A. S. Yelkhovsky: Phys. Rev. A 48, R15 (1993)
11.50 J. F. Babb, L. Spruch: Phys. Rev. A 38, 13 (1988)
11.51 C.-K. Au: Phys. Rev. A 39, 2789 (1989)
11.52 C.-K. Au, M. A. Mesa: Phys. Rev. A 41, 2848 (1990)
11.53 C. H. Storry, N. E. Rothery, E. A. Hessels: Phys.
Rev. Lett. 75, 3249 (1995) and earlier references
therein
11.54 G. W. F. Drake: Adv. At. Mol. Opt. Phys. 32, 93 (1994)
11.55 R. J. Drachman: Phys. Rev. A 47, 694 (1993)
11.56 G. W. F. Drake, R. A. Swainson: Phys. Rev. A 42, 1123
(1990)
11.57 E. A. Hessels: Phys. Rev. A 46, 5389 (1992)
11.58 G. W. F. Drake: Phys. Rev. A 45, 70 (1992)
11.59 G. W. F. Drake: Phys. Rev. A; J. Phys. B 5, 1979 (1972)
11.60 G. W. F. Drake: J. Phys. B 9, L169 (1976)
11.61 G. W. F. Drake: Phys. Rev. A 19, 1387 (1979)
11.62 C. J. Sansonetti, J. D. Gillaspy: Phys. Rev. A 45,R1
(1992)
11.63 W. Lichten, D. Shiner, Z.-X. Zhou: Phys. Rev. A 43,
1663 (1991)
11.64 M. C. George, L. D. Lombardi, E. A. Hessels: Phys.
Rev. Lett. 87, 173002 (2001)
11.65 G. W. F. Drake: Can. J. Phys. 80, 1195 (2002)
11.66 K. Panchucki, J. Sapirstein: J. Phys. B 36, 803 (2003)
and earlier references therein
11.67 L.-B. Wang, P. Müller, K. Bailey, G. W. F. Drake,
J. P. Greene, D. Henderson, R. J. Holt, R. V. F. Jans-
sens, c. L. Jiang, Z.-T. Lu, T. P. O’Connor, R. C. Pardo,
M. Paul, K. E. Rehm, J. P. Schiffer, X. D. Tang: Phys.
Rev. Lett. 93, 142501 (2004)
11.68 G. Ewald, W. Nörtershäuser, A. Dax, S. Göte,
R. Kirchner, H.J. Kluge, Th. Kühl, R. Sanchez, A. Wo-
jtaszek, B. A. Bushaw, G. W. F. Drake, Z.-C. Yan,
C. Zimmermann: Phys. Rev. Lett. 93, 113002 (2004)
Part B 11
221
Atomic Multip
12. Atomic Multipoles
Often symmetries in the experiment limit
the number of nonvanishing multipoles, and
frequently only populations proportional to
diagonal elements of the density matrix are
significant. This chapter studies the physical and
geometrical significance of simple multipoles and
examines whether symmetries allow a complete
characterization of the ensemble with state
populations. More thorough treatments can be
found elsewhere [12.1].
12.1 Polarization and Multipoles.................. 222
12.2 The Density Matrix in Liouville Space ..... 222
12.3 Diagonal Representation:
State Populations ................................ 224
12.4 Interaction with Light.......................... 224
12.5 Extensions .......................................... 225
References .................................................. 226
A typical atomic experiment involves the preparation
of an atomic or molecular ensemble, its perturbation
by a combination of collisions and external fields, and
the characterization of the perturbed system through de-
tection of emitted or scattered particles or quanta. The
ensemble can be described by its density matrix, whose
full specification generally requires elements between
every pair of states in a complete basis set, and whose
elements generally depend on spatial position, velocity,
and time. The time development of such an ensemble
may depend on interactions among the atoms of the en-
semble with each other, with external fields, and with
external perturbers. Most problems burdened by this
much detail are intractable, but fortunately, significant
simplifications can usually be made.
In this chapter, only ensembles of homogeneously
excited, independent atoms are considered. The spatial
and velocity distribution of the atoms is thus assumed
to be independent of the quantum-state distribution, and
the total density matrix can be factored into the prod-
uct of a phase-space distribution with a state density
matrix that is independent of position and velocity. The
assumption is severe and prevents us from treating cases
of radiation transfer (see Chapt. 19) where the degree of
excitation varies as a function of position or problems of
velocity-selective laser excitation. Nevertheless, many
experiments in atomic physics do use ensembles that
are well-described by our assumption, and even in more
complex cases, our model can often serve as a starting
point for analysis. Typical of the experiments for which
our approach is well suited are collisionally induced po-
larization relaxation experiments in a gas cell, either
of an excited state or of an optically pumped ground
state.
In addition, we shall for the most part focus on a few
isolated manifolds, usually a ground-state manifold and
one or two excited-state manifolds. Each manifold, we
assume, can be described by a given eigenvalue J of the
total angular momentum. The qualifier “isolated” means
that coherences between manifolds oscillate rapidly in
time compared to other processes and can be ignored.
These assumptions permit a great simplification and fre-
quently allow a decoupling of the equations of motion, as
shown below. The density matrix within each state mani-
fold can be described by multipole moments, which are
coefficients of the expansion of the density matrix in irre-
ducible tensor operators, as described in Chapt. 7. Each
state multipole is associated with a physical electric or
magnetic moment in the atom.
The amount of information which can be im-
parted to, or obtained from, an atomic ensemble is
limited by the finite number of accessible multipole mo-
ments.The nature of that information may depend on
the time evolution of the multipoles in external fields
and their relaxation through collisions. The ideal ex-
periment is a complete measurement that determines
all the multipoles. Often over-complete experiments can
be designed, but there is little reason for determining
redundant information unless consistency checks are
important.
Part B 12
222 Part B Atoms
12.1 Polarization and Multipoles
An ensemble is said to be polarized if there is a non-
statistical distribution of atoms in magnetic sublevels.
The type of polarization can be described by the multi-
poles that exist. In many experiments, symmetries in
the preparation or detection of the ensemble limit
the detectable polarizations to ones caused by differ-
ent sublevel populations. One can then employ the
relatively simple population (or diagonal) representa-
tion. The more general case is discussed in the next
section.
As an introduction to the concepts, consider an
atomic manifold of total angular momentum 3/2, for
example an excited alkali P
3/2
state. There are four mag-
netic sublevels
|
m
with m =±3/2, ±1/2sothatstate
populations are given by a four-dimensional population–
density vector
N =
N
3/2
N
1/2
N
−1/2
N
−3/2
,
(12.1)
where N
m
is the density of atoms in state
|
m
.Ifthere
are no external fields, the time evolution of N is given
by
˙
N =S −ΓN −γ · N ,
(12.2)
where S is the source vector and gives the excitation
rate to the various sublevels, Γ is the radiative decay
rate, assumed the same for all levels, and γ is the colli-
sional matrix, whose elements −γ
mn
give the collisional
transition rate from n to m.
The equation of motion is most easily solved if
the basis used for the state space exploits the sym-
metry. Define the “spherical” orthonormal basis of
vectors
ˆ
T
0
=
1
2
1
1
1
1
,
ˆ
T
1
=
1
√
20
3
1
−1
−3
,
(12.3)
ˆ
T
2
=
1
2
1
−1
−1
1
,
ˆ
T
3
=
1
√
20
1
−3
3
−1
.
(12.4)
(These relations follow from the T
LM
with M = 0. See
Sects. 12.2 and 12.3). The population vector N can be
expanded
N =
4
L=0
n
L
ˆ
T
L
, (12.5)
where the coefficients n
L
= N·
ˆ
T
L
contain all the
knowable information about the population distribu-
tion. The coefficient n
L
is called the 2L multipole
moment of the ensemble. In particular, n
0
=
1
2
m
N
m
is twice the population of the entire ensemble; n
1
=
5
−1/2
m
mN
m
=2n
0
J
z
/
√
5 is the “orientation” (as-
sociated with a physical magnetic-dipole moment) of the
system, n
2
=
1
3
n
0
3J
2
z
− J
2
is the “alignment” (electric
quadrupole moment), and n
3
= (2n
0
/
√
45)J
z
(5J
z
+
1 −3J
2
) is the octupole moment of N. The source
vector S is readily expanded in the same basis.
If the collisions are isotropic, as is usually ap-
proximately the case in gas-cell experiments, then the
collision matrix γ is invariant under rotations and
γ ·
ˆ
T
L
= γ
L
ˆ
T
L
. (12.6)
The equation of motion (12.2) is separated into four
uncoupled scalar equations:
˙
n
L
= S
L
−Γn
L
−γ
L
n
L
. (12.7)
If the source term S
L
= S ·
ˆ
T
L
is constant, (12.7)have
the simple solutions
n
L
(
t
)
= n
L
(
∞
)
+
[
n
L
(
0
)
−n
L
(
∞
)
]
exp
[
−
(
Γ +γ
L
)
t
]
,
(12.8)
with the steady-state value
n
L
(
∞
)
= S
L
/
(
Γ +γ
L
)
.
(12.9)
The detection of such multipoles is discussed at the end
of Sect. 12.4.
12.2 The Density Matrix in Liouville Space
If in the example of the last section the state basis is
changed, say by rotating the axis of quantization, then
populations are generally no longer sufficient to char-
acterize the ensemble. In the new basis, the ensemble
will have been prepared in a coherent superposition of
states. An adequate description for the quantum state of
an ensemble in a manifold of n quantum states requires
an n× n density matrix ρ(t) as discussed in Chapt. 7.
Part B 12.2
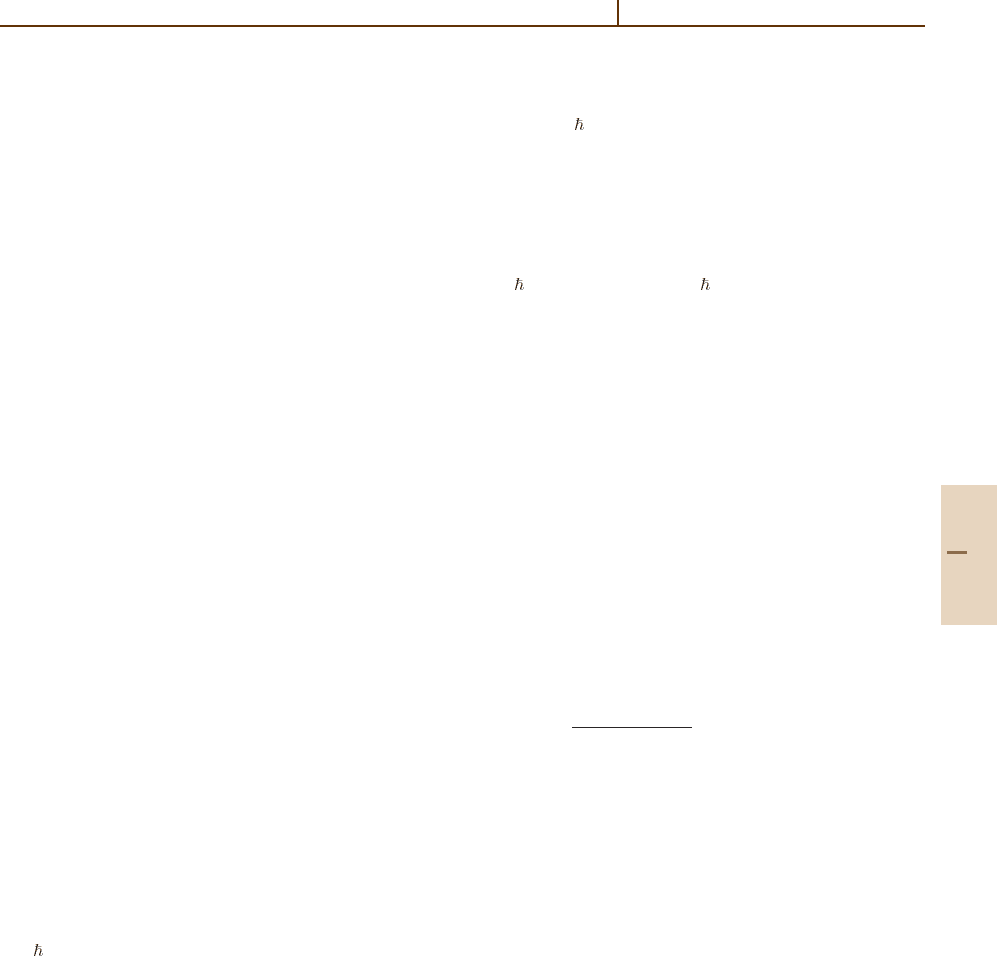
Atomic Multipoles 12.2 The Density Matrix in Liouville Space 223
The density matrix may be considered a vector in
a space, called Liouville space,ofn
2
dimensions. A con-
venient set of complex orthonormal basis vectors in
this space is given by the irreducible tensor operators
T
LM
:
T
∗
LM
· T
L
M
:= Tr
T
†
LM
T
L
M
= δ
LL
δ
MM
,
(12.10)
where the trace (Tr) expression is appropriate for the
usual n × n matrix representations of the T
LM
.Every
quantum operator on the manifold can be expanded in
the basis
T
LM
, and in particular the density matrix
has an expansion
ρ
(
t
)
=
LM
ρ
LM
(
t
)
T
LM
,
(12.11)
with time-dependent coefficients
ρ
LM
(
t
)
= T
∗
LM
· ρ
(
t
)
:= Tr
T
†
LM
ρ
(
t
)
= N
T
†
LM
(12.12)
that are identified with the multipole moments of the
ensemble. Here, ···indicates the average value over
the ensemble and N = Tr{ρ} is the normalization of the
density matrix ρ, which is often set equal to unity but
is sometimes more conveniently set equal to the total
density of atoms in the manifold.
The T
LM
are defined to transform under rotations
as spherical harmonics Y
LM
[12.2, 3]. The expansion
(12.11) splits the density matrix into basis vectors
T
LM
which contain the geometric information and
scalar coefficients (the multipole moments) ρ
LM
(t)
which contain the physical information about state
distributions.
The time dependence of ρ(t) isgivenbythe
Schrödinger equation and in the interaction picture takes
the form
i
˙
ρ =
[
V, ρ
]
,
(12.13)
where V is the interaction due to external fields (in-
cluding radiation) and collisions. When V is expanded
and second-order perturbation theory is applied, the time
evolution (12.13) can be expressed in a form analogous
to (12.2) but with an extra term [12.1]:
ρ =S −Γ · ρ −γ · ρ −iL
0
· ρ , (12.14)
where L
0
is the Liouvilleoperator arising from the inter-
action V
0
with slowly varying external fields. Its matrix
elements with ρ are
(
L
0
· ρ
)
mn
=
−1
[
V
0
, ρ
]
mn
=
(
ω
m
−ω
n
)
ρ
mn
,
(12.15)
where ω
m
−ω
n
are the field-induced frequency splittings
between the states.
For example, in a weak magnetic field B
0
ˆ
z oriented
along the quantization axis
ˆ
z, V
0
= ω
0
J
z
where ω
0
=
g
j
µ
B
B
0
/ .Since
J
z
, T
LM
= M T
LM
(see Chapt. 7),
then assuming that the collisions are isotropic and that
the radiative decay rate is the same for all states of the
manifold, the expansion of ρ in T
LM
decouples the
equation of motion (12.14) into multipoles:
˙
ρ
LM
= S
LM
−Γρ
LM
−γ
L
ρ
LM
−iMω
0
ρ
LM
,
(12.16)
with the solution
ρ
LM
(
t
)
= ρ
LM
(
∞
)
+
ρ
LM
(
0
)
−ρ
LM
(
∞
)
×exp
[
−
(
Γ +γ
L
+iMω
0
)
t
]
(12.17)
comprising a transient part that decays as it precesses at
the angular rate ω
0
plus a steady-state part that predicts
the collisionally broadened Hanle effect (see Chapt. 17):
ρ
LM
(
∞
)
=
S
LM
Γ +γ
L
+iMω
0
. (12.18)
If M = 0, the solutions reduce to the field-independent
ones given in (12.9). On the other hand, any stray
field that is not aligned with an induced multipole
moment can rotate the moment and possibly cause
systematic measurement errors if not taken into ac-
count. Measurements of γ
L
in a gas cell at temperature
T are frequently used to determine thermally aver-
aged multipole-relaxation cross sections Q
L
,defined
by
γ
L
= N
¯
vQ
L
, (12.19)
where N is the density of perturbers, and the mean
relative velocity is
¯
v = 2π
−1/2
(
2kT/µ
)
1/2
, with µ the
reduced mass of the polarized-atom/perturber system.
Many semiclassical and full quantum calculations of
such cross sections have been made, both for atoms
[12.1, 4] and for molecules [12.5].
Part B 12.2
