Drake G.W.F. (editor) Handbook of Atomic, Molecular, and Optical Physics

Подождите немного. Документ загружается.

High Precision Calculations for Helium 11.2 Computational Methods 203

Table 11.1 Formulas for the radial integrals I

(a, b, c; α, β) =r

−αr

−βr



rad

and I

log

(a, b, c; α, β) =r

ln r

−αr

−βr



rad

; ψ(n) =−γ +



n−1

k=1

−1

is the digamma function,

(a, b; c; z) is the hypergeometric function, and

s = a + b + c + 5. Except as noted, the formulas apply for a ≥−1, b ≥−1, c ≥−1

1. I

(−2, −2, −1; α, β) =



α + β





α + β



2. I

(a, b, c; α, β) =

c + 2

[(c+1)/2]



i=0



c + 2

2i + 1



a+2i+2, b+c−2i+2

(α, β) + F

b+2i+2, a+c−2i+2

(β, α)]

(c ≥−1, s ≥ 0)

where F

p,q

(α, β) =











(α + β)

p+1

q+1



j=0

( p + j)!



α + β



q ≥ 0, p ≥ 0

p+q+2

∞



j= p+q+1

( j − q)!



α + β



j+1

q < 0, p ≥ 0

p < 0

3. I

(a, b, c; α, α) =

c+3

(c + 2)(2α)

s+1





a+1



j=0



a + 1



(b + 1)!



( j + b + 2)!

−

( j + c + 2)!

( j + b + c + 4)!



+ (a ↔ b)





5. I

(a, b, −2; α, β) =

(a + 1)!

a+2

a+1



j=0

(b + 1 + j)!



(α + β)

b+2+ j

−

(−α)

(β − α)

b+2+ j







2α

α + β



− ψ(a + 2 − j) + ψ(1)





a ↔ b

α ↔ β



6. I

(a, b, −2; α, α) =

2s!(a + 1)!(b + 1)!

(2α)

s+1





a+1



j=0

ψ(s + 1 − j)− ψ(a + 2 − j)

j!(s − j)!

+ (a ↔ b)





7. I

(−1, −1, −3; α, β) =

2(β ln β − α ln α)

− β

2[ψ(2) − ln ]

α + β

8. I

(a, b, −3; α, β) =





(a + 1)!

a+1

(α + β)

b+2



j=0

(b + 1 + j)!

j!(a + 1 − j)



α + β





a ↔ b

α ↔ β







−



ln(αβ

) − 2ψ(2)



(α + β)

s+1

−

(a + 1)!(b + 1)!

(s + 1)α

a+2

b+1



a + 2, 1; s + 2;

α−β



, a ≥−1, b ≥−1

9. I

log

(−1, −1, c; α, β) =

2(c + 1)!

− β



ln α − ψ(c + 2)

c+2

−

ln β − ψ(c + 2)

c+2



10. I

log

(a, b, c; α, β) =

(a + 1)!

(c + 2)α

a+c+4

a+1



j=0

(b + 1 + j)!(a + c + 3 − j)!

j!(a + 1 − j)!



(α + β)

b+2+ j

−

(−α)

(β − α)

b+2+ j





−ψ(a + c + 4 − j) +

c + 2

+ ln α





a ↔ b

α ↔ β



11. I

log

(a, b, c; α, α) =

c+3

s!(b + 1)!

(c + 2)(2α)

s+1

a+1



j=0



a + 1



( j + b + 2)!

−

( j + c + 2)!

( j + b + c + 4)!



ψ(s + 1) − ln α −

c + 2



(a + c + 3 − j)!

(s + 1 − j)!

[

ψ(s + 1 − j)− ψ(a + c + 4 − j)

]

+ (a ↔ b)

Terms with p < 0 represent divergent parts which cancel from convergent differences between integrals with the same α and β

 is the radius of an inﬁnitesimal sphere about r

= 0 which is omitted from the range of integration

Part B 11.2

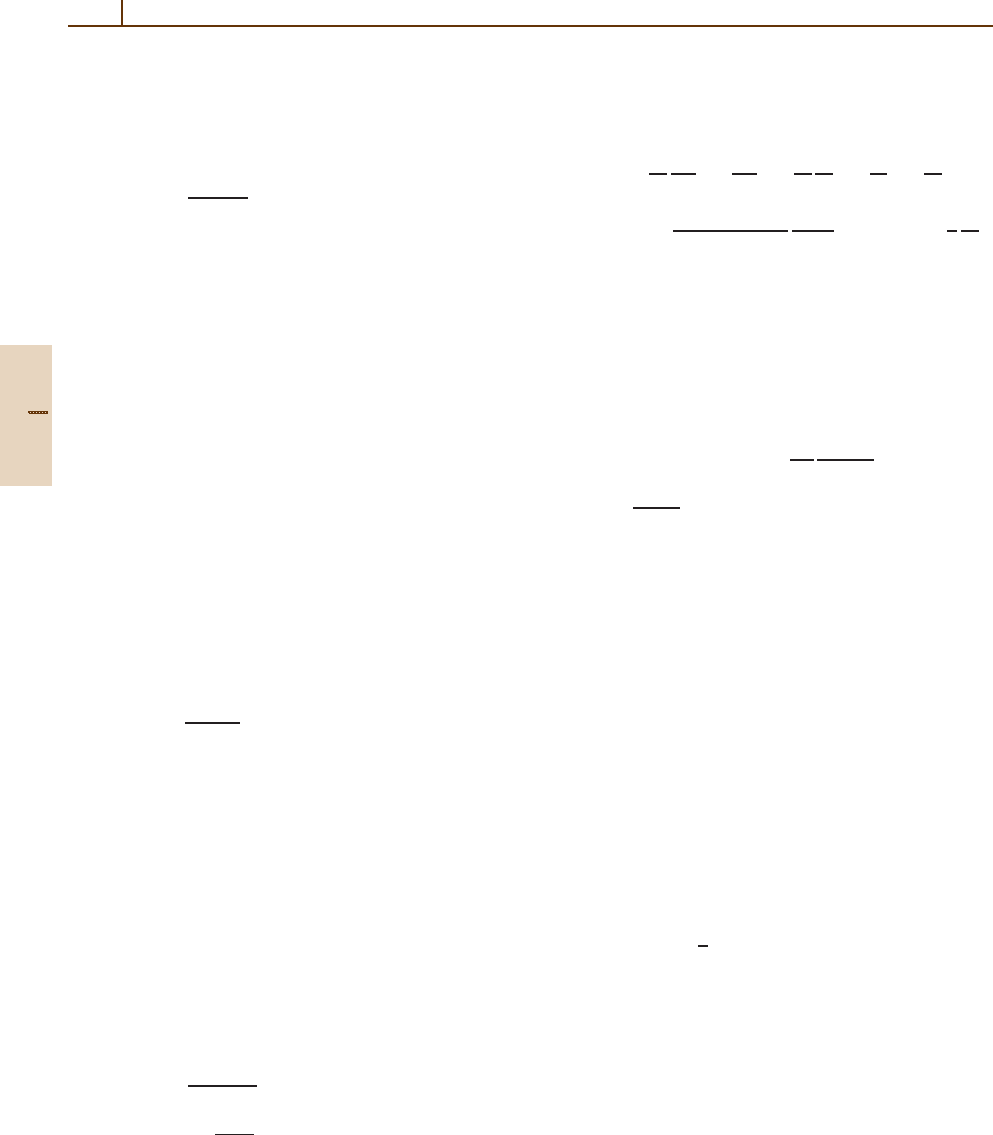
204 Part B Atoms

techniques for these are discussed in [11.33]. Other cases

can be derived by use of the formula

−1

f(r

) e

−αr

−βr

rad

− β

∞



−βr

− e

−αr

rf(r) dr ,

(11.25)

and then differentiating or integrating with respect to

α or β to raise or lower the powers of r

and r

Total Integral

The angular integral (11.23) combined with the radial

integrals from Table 11.1 yields the total integral

M∗



f(a, b, c; α, β )



(a, b, c; α, β) , (11.26)

where

(a, b, c; α, β) =f(a, b, c; α, β)P

(cos θ)

rad

f(a, b, c; α, β ) = r

−αr

−βr

Starting from I

and I

, the general I

can be efﬁciently

calculated from the recursion relations [11.32]

Λ+1

(a, b, c; α, β)

2Λ + 1

c + 2

(a − 1, b − 1, c + 2; α, β)

+ I

Λ−1

(a, b, c; α, β), c =−2 (11.27)

Λ+1

(a, b, −2; α, β)

= (2Λ + 1)I

log

(a − 1, b − 1, 0; α, β)

+ I

Λ−1

(a, b, −2; α, β), c =−2 (11.28)

where

log

(a, b, c; α, β)

=f(a, b, c,α,β)ln r

(cos θ)

rad

The I

log

integrals follow the recursion relation

log

Λ+1

(a, b, c; α, β)

(2Λ + 1)

c + 2



log

(a − 1, b − 1, c + 2; α, β)

−

c + 2

(a − 1, b − 1, c + 2; α, β)



+ I

log

Λ−1

(a, b, c; α, β) . (11.29)

Hamiltonian Matrix Elements

The general form of the Laplacian operator in terms of

, r

variables is

∇

∂

∂r



∂

∂r



∂

∂r



∂

∂r



−

2(r

−r

cos θ)

∂

∂r

− 2



∇

· r



∂

∂r

(11.30)

and similarly for ∇

with subscripts 1 and 2 inter-

changed. The term ∇

is understood to act only on

the Y

(

) part of the wave function. This term

in ∇

can be easily evaluated by means of the effective

operator replacement



M∗



∇

· r



ang

∂g(r

)

∂r

→

g(r

)



(cos θ) (11.31)

for the angular part of the total integral, where

=[l



+ 1) − l

+ 1) − Λ(Λ + 1)]C

(11.32)

The replacement (11.31) becomes an equality after ra-

dial integration with any function g(r

) in the integrand.

The matrix elements of H between arbitrary basis func-

tions deﬁned by

χ = r

−αr

−βr

(



= r



−α



−β



(

can then be written in the explicitly Hermitian form (for

inﬁnite nuclear mass)

χ



|H|χ=



i=0



(1)

× I

− i, b

, c

; α

,β

)

+ A

(2)

, b

− i, c

; α

,β

)

+ A

(3)

, b

, c

− i; α

,β

)



(11.33)

where a

= a



± a, α

= α



± α etc., and

(1)

=−α

− α

−

+ 2α

−

Part B 11.2

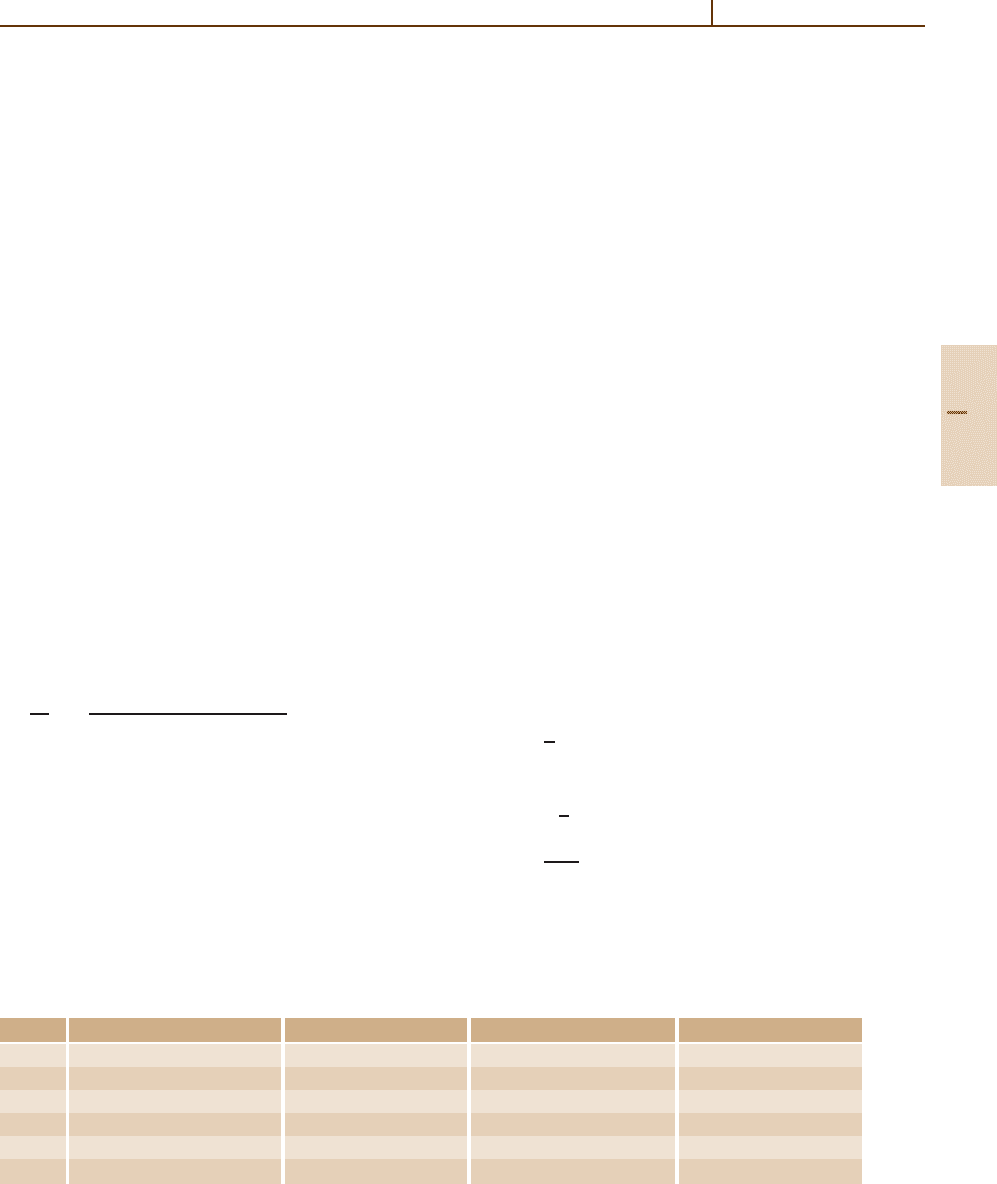
High Precision Calculations for Helium 11.3 Variational Eigenvalues 205

(1)

= 2{α

+ 2) + α

−

−[α

−

+ α

−

+ 2)](c

−

)}−8Z ,

(1)

=−a

− a

−

− 2a

+ 2a

−

+ 1)(c

−

)

+ 2l

+ 1)(1 − c

−

)

+ 2l



+ 1)(1 + c

−

(3)

= 0 , A

(3)

= 8 ,

(3)

= 2(c

− c

−

with (c

−

) = 0forc

= 0. The A

(2)

are deﬁned sim-

ilarly to A

(1)

with the replacements a → b, α → β,

→ l

. The overlap integral is

χ



|χ=



, b

, c

; α

,β

). (11.34)

11.2.4 Other Computational Methods

Although not yet at the same level of accuracy as

variational methods, certain nonvariational methods,

such as ﬁnite element methods [11.34], solutions to

the Faddeev equations [11.35], and the correlated-

function hyperspherical-harmonic method [11.36], have

their own advantages of ﬂexibility and/or general-

ity. A characteristic feature of these methods is that

they provide direct numerical solutions to the three-

body problem which in principle converge pointwise

to the exact solution, rather than depending upon

a globally optimized solution. Other methods particu-

larly suited to doubly-excited states are discussed in

Chapt. 25

11.3 Variational Eigenvalues

High precision variational eigenvalues are available for

all states of helium up to n = 10 and L = 7 [11.27–29].

The nonrelativistic values of ε

, ε

and ε

[see (11.6)]

are listed in Table 11.2 and Table 11.3.Theε

are the

eigenvalues for inﬁnite nuclear mass, and ε

and ε

together with (11.6) give the ﬁnite mass corrections for

the isotopes

He and

He. The values of µ/M can be

calculated from



5.485 799 110 (12) ×10

−4

− N + 1



−1

(11.35)

where M

is the atomic mass (in amu, see [11.37]

for a tabulation. For high precision work, the helium

electronic binding energy of 8.48 × 10

−8

amu should be

added to M

.) and N is the number of electrons. For

He, one can use directly the accurately known value

of m

to calculate µ/M = 1/(m

+ 1).Values

of µ/M for the ﬁrst several isotopes are listed in Ta-

ble 11.4, and the corresponding energy coefﬁcients for

the 1s

S ground state are given in Table 11.5.

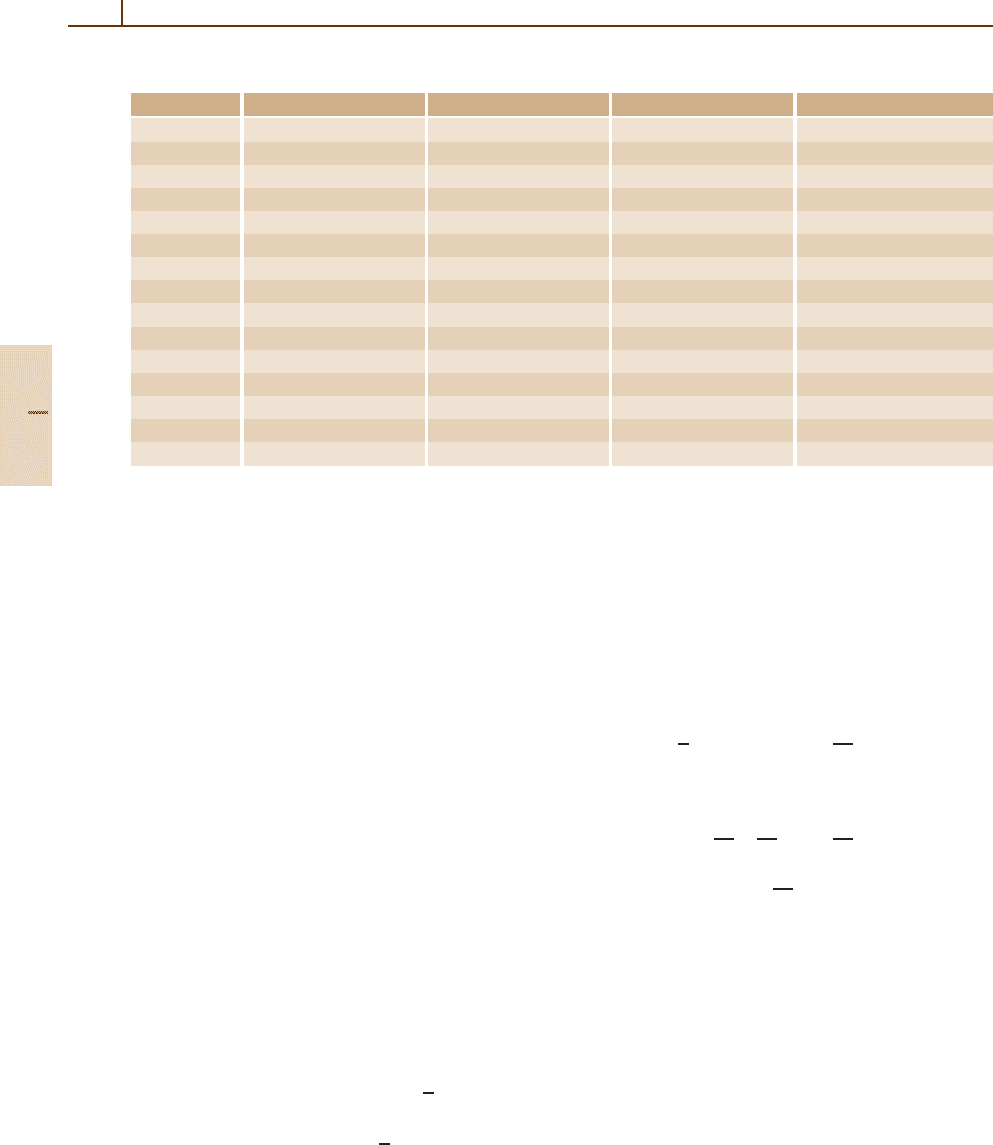
Table 11.2 Nonrelativistic eigenvalue coefﬁcients ε

and ε

for helium

State ε

L) ε

1S − 2.903 724 377 034 1195 0.159 069 475 085 84 − −

2S − 2.145 974 046 054 419(6) 0.009 503 864 419 28 − 2.175 229 378 236 791 30 0.007 442 130 706 04

2P − 2.123 843 086 498 093(2) 0.046 044 524 937(1) − 2.133 164 190 779 273(5) − 0.064 572 425 024(4)

3S − 2.061 271 989 740 911(5) 0.002 630 567 0977(1) − 2.068 689 067 472 457 19 0.001 896 211 617 81

3P − 2.055 146 362 091 94(3) 0.014 548 047 097(1) − 2.058 081 084 274 28(4) − 0.018 369 001 636(2)

3D − 2.055 620 732 852 246(6) − 0.000 249 399 9921(1) − 2.055 636 309 453 261(4) 0.000 025 322 839(1)

11.3.1 Expectation Values of Operators

and Sum Rules

Expectation values for various powers of the radial co-

ordinates, together with operators appearing in the Breit

interaction, are listed in Table 11.6 for the ground state

of helium and He-like ions. Included are all terms re-

quired to calculate V

, and the oscillator strength sum

rules [11.38]

S(−1) =



)



(11.36a)

S(0) = 2 , (11.36b)

S(1) =−

(ε

− ε

), (11.36c)

S(2) =

2πZ



δ(r

) + δ(r

)



, (11.36d)

where S(k) =



[ε

P ) − ε

S )]

, with ener-

gies in a.u., and f

is the 1

S − n

P oscillator strength

(see Sect. 11.5.1).

Part B 11.3

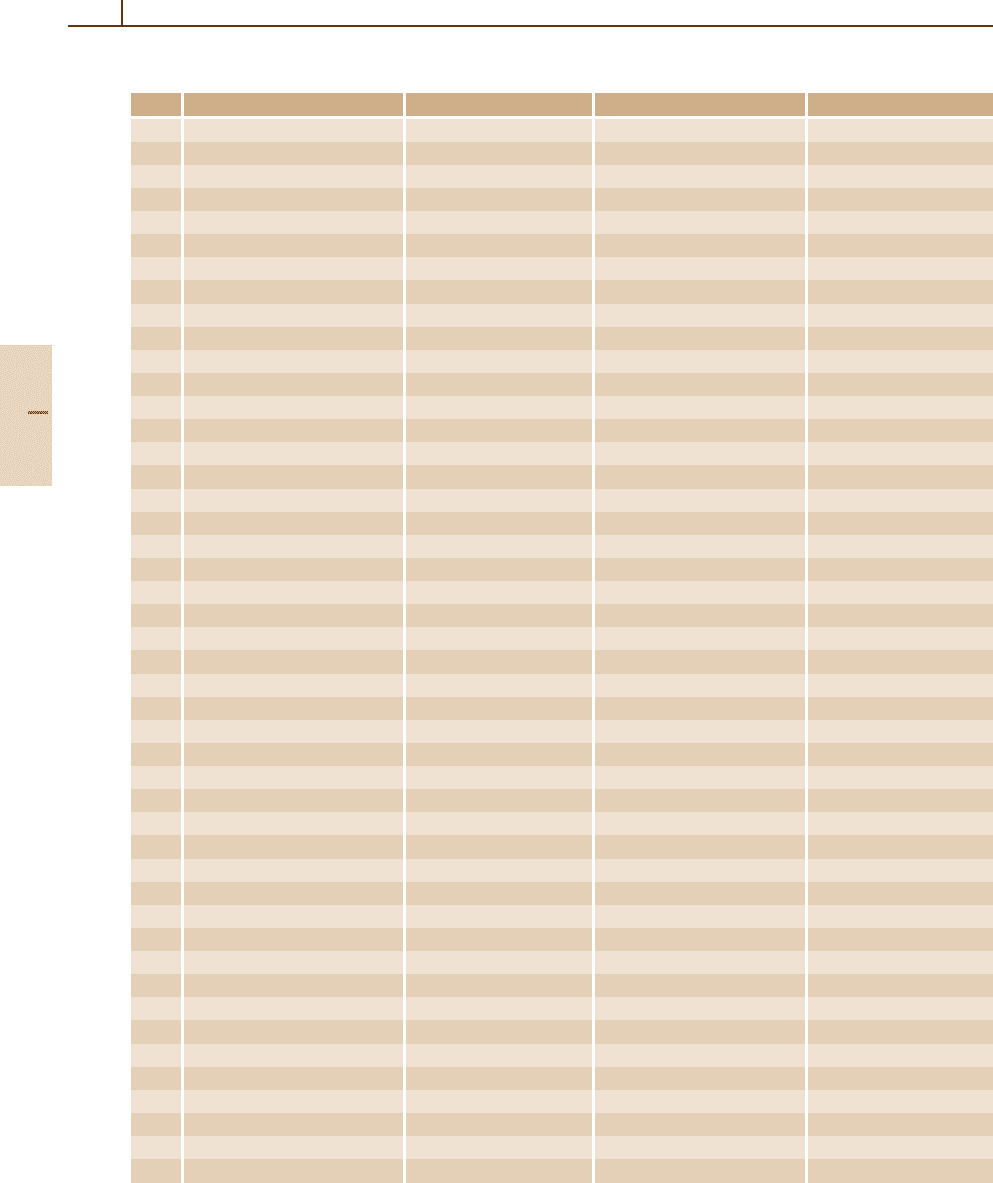
206 Part B Atoms

Table 11.2 Nonrelativistic eigenvalue coefﬁcients ε

and ε

for helium, cont.

State ε

L) ε

4S − 2.033 586 717 030 72(1) 0.001 073 641 2266(1) − 2.036 512 083 098 236 30(2) 0.000 742 661 516 18

4P − 2.031 069 650 450 24(3) 0.006 254 923 5543(1) − 2.032 324 354 296 62(2) − 0.007 555 178 98(1)

4D − 2.031 279 846 178 687(7) − 0.000 129 175 1887(8) − 2.031 288 847 501 795(3) 0.000 029 442 651(2)

4F − 2.031 255 144 381 749(1) − 0.000 010 024 2694(2) − 2.031 255 168 403 2456(6) − 0.000 009 669 6396

5S − 2.021 176 851 574 363(5) 0.000 538 860 3605(1) −2.022 618 872 302 312 27(1) 0.000 363 697 136 49

5P − 2.019 905 989 900 83(2) 0.003 230 021 84(2) − 2.020 551 187 256 25(1) − 0.003 810 911 035(1)

5D − 2.020 015 836 159 984(4) − 0.000 071 883 131(6) − 2.020 021 027 446 911(5) 0.000 019 568 85(1)

5F − 2.020 002 937 158 7427(5) − 0.000 005 704 2946(4) − 2.020 002 957 377 3694(4) − 0.000 005 406 4900(5)

5G − 2.020 000 710 898 584 71(1) − 0.000 001 404 4136 − 2.020 000 710 925 343 92(1) − 0.000 001 404 0013

6S − 2.014 563 098 446 60(1) 0.000 307 704 277(1) − 2.015 377 452 992 862 19(3) 0.000 204 329 479 10

6P − 2.013 833 979 671 73(2) 0.001 878 058 536(1) − 2.014 207 958 773 74(1) − 0.002 184 346 463(1)

6D − 2.013 898 227 424 286(5) − 0.000 043 412 2689(9) − 2.013 901 415 453 792(7) 0.000 012 742 22(3)

6F − 2.013 890 683 815 5497(3) − 0.000 003 482 257(7) − 2.013 890 698 348 5320(2) − 0.000 003 268 4586(8)

6G − 2.013 889 345 387 313 22(3) − 0.000 000 898 5799(7) − 2.013 889 345 416 952 96(3) − 0.000 000 898 1237(7)

6H − 2.013 889 034 754 279 72 − 0.000 000 290 3471 − 2.013 889 034 754 301 55 −0.000 000 290 3467

7S − 2.010 625 776 210 87(2) 0.000 191 925 025(1) − 2.011 129 919 527 626 21(4) 0.000 125 981 736 89

7P − 2.010 169 314 529 35(2) 0.001 186 152 30(1) − 2.010 404 960 007 94(2) − 0.001 366 5008(3)

7D − 2.010 210 028 457 98(1) − 0.000 028 027 840(2) − 2.010 212 105 955 595(2) 0.000 008 563 121(3)

7F − 2.010 205 248 074 013(1) − 0.000 002 262 00(4) − 2.010 205 258 374 865(1) −0.000 002 110 58(3)

7G − 2.010 204 386 224 772 55(7) − 0.000 000 598 3963(3) − 2.010 204 386 250 217 93(6) − 0.000 000 598 005(1)

7H − 2.010 204 182 806 482 04(2) − 0.000 000 201 0978 − 2.010 204 182 806 512 04(1) − 0.000 000 201 0973

7I − 2.010 204 120 606 191 32 − 0.000 000 077 7755 − 2.010 204 120 606 191 340 − 0.000 000 077 7755

8S − 2.008 093 622 105 61(4) 0.000 127 650 436(1) − 2.008 427 122 064 721 42(6) 0.000 083 070 552 34

8P − 2.007 789 127 133 22(2) 0.000 796 195 83(5) − 2.007 947 013 771 12(1) − 0.000 911 0535(3)

8D − 2.007 816 512 563 811(7) − 0.000 019 076 181(1) − 2.007 817 934 711 706(3) 0.000 005 971 1234(3)

8F − 2.007 813 297 115 0141(6) − 0.000 001 545 48(1) − 2.007 813 304 535 0908(5) − 0.000 001 436 452(2)

8G − 2.007 812 711 494 0241(1) −0.000 000 415 0040(1) − 2.007 812 711 514 424 82(9) − 0.000 000 414 6904

8H − 2.007 812 571 828 655 81(1) − 0.000 000 142 6492(3) − 2.007 812 571 828 685 73(1) − 0.000 000 142 6487(2)

8I − 2.007 812 528 549 584 59 − 0.000 000 056 9359 − 2.007 812 528 549 584 61 − 0.000 000 056 9359

8K − 2.007 812 512 570 229 31 − 0.000 000 025 1113 − 2.007 812 512 570 229 306 − 0.000 000 025 1113

9S − 2.006 369 553 107 85(3) 0.000 089 149 6387(7) − 2.006 601 516 715 010 67(3) 0.000 057 628 311 52

9P − 2.006 156 384 652 86(5) 0.000 559 978 028(2) − 2.006 267 267 366 41(4) − 0.000 637 531 359(6)

9D − 2.006 175 671 437 641(6) − 0.000 013 542 185(3) − 2.006 176 684 884 697(2) 0.000 004 306 538(6)

9F − 2.006 173 406 897 3246(8) − 0.000 001 099 9671(3) − 2.006 173 412 365 0430(7) − 0.000 001 019 651(2)

9G − 2.006 172 991 627 5863(2) −0.000 000 298 2672(1) − 2.006 172 991 643 6650(3) − 0.000 000 298 0198(1)

9H − 2.006 172 891 903 619 14(2) − 0.000 000 104 0022 − 2.006 172 891 903 645 88(2) − 0.000 000 104 0019

9I − 2.006 172 860 732 382 57 − 0.000 000 042 3136 − 2.006 172 860 732 382 60 − 0.000 000 042 3136(1)

9K − 2.006 172 849 096 329 78 − 0.000 000 019 1516 − 2.006 172 849 096 329 780 − 0.000 000 019 1516

10S − 2.005 142 991 748 00(8) 0.000 064 697 214(3) − 2.005 310 794 915 6113(2) 0.000 041 598 811 52

10P − 2.004 987 983 802 22(4) 0.000 408 649 4263 − 2.005 068 805 4978(1) − 0.000 463 433 718(8)

10D − 2.005 002 071 654 250(6) − 0.000 009 947 5060(6) − 2.005 002 818 080 232(8) 0.000 003 198 298(8)

10F − 2.005 000 417 564 6682(9) −0.000 000 809 442(9) − 2.005 000 421 686 6036(7) − 0.000 000 748 9264(2)

10G − 2.005 000 112 764 3180(3) −0.000 000 220 982(2) − 2.005 000 112 777 0031(4) − 0.000 000 220 785(3)

10H − 2.005 000 039 214 394 52(2) − 0.000 000 077 8067 − 2.005 000 039 214 417 41(2) − 0.000 000 077 8062

10I − 2.005 000 016 086 516 19 − 0.000 000 032 0590(1) − 2.005 000 016 086 516 22 − 0.000 000 032 0589(2)

10K − 2.005 000 007 388 375 88 − 0.000 000 014 7514 − 2.005 000 007 388 375 88 −0.000 000 014 7514

Part B 11.3

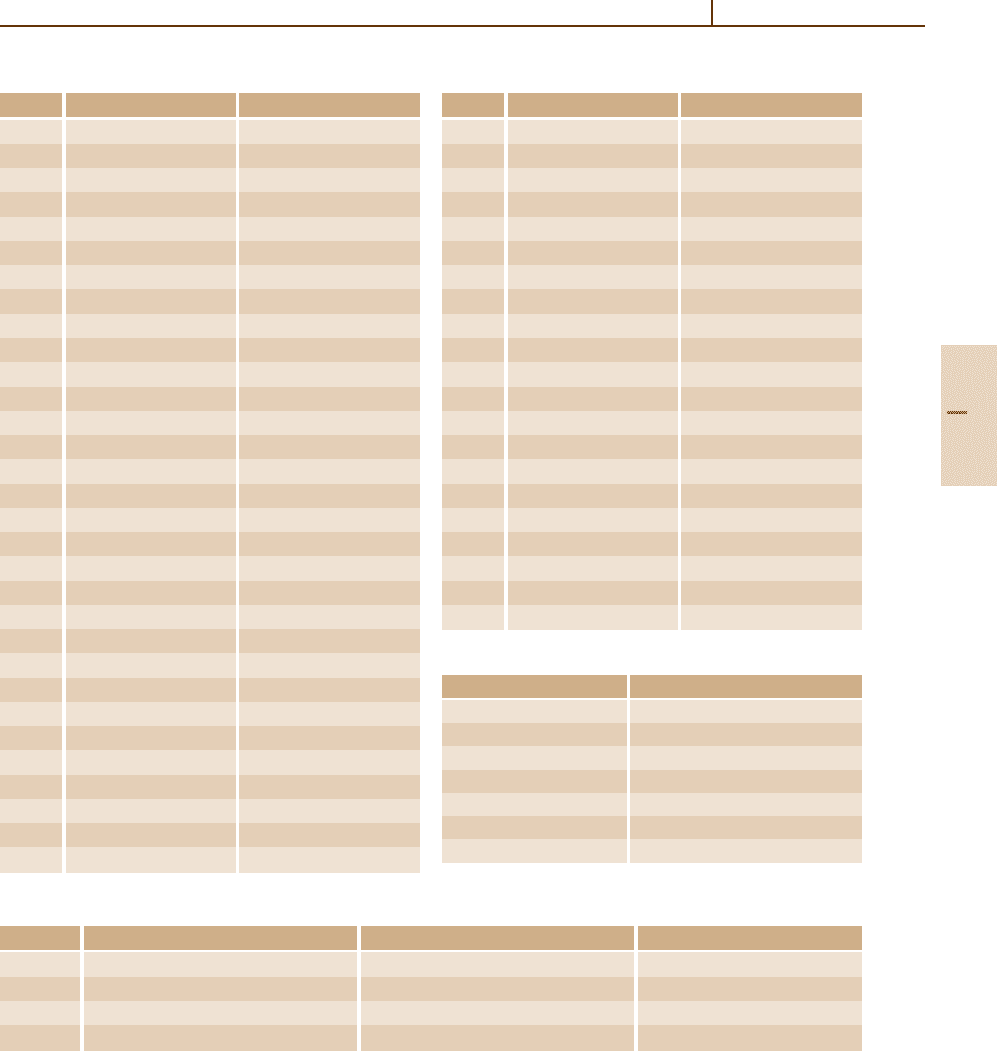
High Precision Calculations for Helium 11.3 Variational Eigenvalues 207

Table 11.3 Eigenvalue coefﬁcients ε

for helium

State ε

L) ε

1S − 0.470 391 870(1) −

2S − 0.135 276 864(1) − 0.057 495 8479(2)

2P − 0.168 271 22(7) − 0.204 959 88(1)

3S − 0.058 599 3124(4) − 0.040 455 8505(5)

3P − 0.066 047 859(3) − 0.070 292 710(2)

3D − 0.057 201 299(9) − 0.054 737 73(1)

4S − 0.032 522 293(2) − 0.025 628 6338(1)

4P − 0.035 159 71(6) − 0.036 129 973(2)

4D − 0.032 150 91(2) − 0.030 747 891(7)

4F − 0.031 274 336(4) − 0.031 277 9921(3)

5S − 0.020 647 26(9) − 0.017 322 734 96

5P − 0.021 8476(3) − 0.022 166 61(9)

5D − 0.020 5101(2) − 0.019 7062(2)

5F − 0.020 013 498(6) − 0.020 016 561(4)

5G − 0.020 003 5608 − 0.020 003 5646

6S − 0.014 261 796(4) − 0.012 411 3991(3)

6P − 0.014 902 86(9) − 0.015 033 58(5)

6D − 0.014 1994(2) − 0.013 707 27(1)

6F − 0.013 896 984(2) − 0.013 899 22(3)

6G − 0.013 891 179(6) − 0.013 891 184(8)

6H − 0.013 889 6191 − 0.013 889 6190

7S − 0.010 4382(2) − 0.009 304 4433(3)

7P − 0.010 8186(2) − 0.010 879(2)

7D − 0.010 405 09(3) − 0.010 085 212(1)

7F − 0.010 2092(3) − 0.010 2107(3)

7G − 0.010 205 61(5) − 0.010 205 61(5)

7H − 0.010 204 590(2) − 0.010 204 587(2)

7I − 0.010 204 2767 − 0.010 204 2768

8S − 0.007 968 944(3) − 0.007 224 7705(3)

8P − 0.008 2117(5) − 0.008 2487(6)

8D − 0.007 9507(4) − 0.007 731 59(2)

Table 11.4 Values of the reduced electron mass ratio µ/M

Table 11.5 Nonrelativistic eigenvalues E = ε

+ (µ/M)ε

+ (µ/M)

for helium-like ions (in units of e

)

Atom ε

S ) ε

S )

−

− 0.527 751 016 544 377 0.032 879 781 852 30 −0.059 779 492 64(1)

He − 2.903 724 377 034 1195 0.159 069 475 085 84 − 0.470 391 870(1)

− 7.279 913 412 669 3059 0.288 975 786 393 99 −1.277 369 3776(2)

− 13.655 566 238 423 5867 0.420 520 303 439 44 − 2.491 572 8581(1)

Table 11.3 Eigenvalue coefﬁcients ε

for helium, cont.

State ε

L) ε

8F − 0.007 8159(3) − 0.007 8170(2)

8G − 0.007 813 563(1) − 0.007 813 568(3)

8H − 0.007 812 855(4) − 0.007 812 859(5)

8I − 0.007 812 6429 − 0.007 812 6429

8K − 0.007 812 5630 − 0.007 812 5630

9S − 0.006 282 5136(1) − 0.005 768 0285(1)

9P − 0.006 4457(2) − 0.006 464 9369(1)

9D − 0.006 270 99(7) − 0.006 1152(1)

9F − 0.006 175 20(1) − 0.006 176 0254(7)

9G − 0.006 173 5796(1) − 0.006 173 592(4)

9H − 0.006 173 104(2) − 0.006 173 101(2)

9I − 0.006 172 9459(1) − 0.006 172 9460(2)

9K − 0.006 172 8876 − 0.006 172 8876

10S − 0.005 079 8362(8) − 0.004 709 4530(1)

10P − 0.005 197(1) − 0.005 2067(1)

10D − 0.005 0724(4) − 0.004 9580(8)

10F − 0.005 001 76(2) − 0.005 002 386(2)

10G − 0.005 000 55(2) − 0.005 000 55(2)

10H − 0.005 000 1935(2) − 0.005 000 1935(1)

10I − 0.005 000 0803(4) − 0.005 000 081(1)

10K − 0.005 000 0369 − 0.005 000 0368

Isotope µ/M×10

H 5.443 205 771(12)

D 2.723 695 064(6)

He 1.819 212 075(4)

He 1.370 745 641(3)

Li 0.912 167 61(8)

Li 0.782 020 21(6)

Be 0.608 820 45(3)

Part B 11.3

208 Part B Atoms

Table 11.6 Expectation values of various operators for He-like ions for the case M =∞(in a.u.)

Quantity H

−

He Li

r

 11.913 699 678 05(6) 1.193 482 995 019 0.446 279 011 201 0.232 067 315 531

r

 25.202 025 2912(1) 2.516 439 312 833 0.927 064 803 063 0.477 946 525 143

r

· r

 − 0.687 312 967 569 − 0.064 736 661 398 − 0.017 253 390 330 − 0.006 905 947 040

r

 2.710 178 278 444(1) 0.929 472 294 874 0.572 774 149 971 0.414 283 328 006

r

 4.412 694 497 992(2) 1.422 070 255 566 0.862 315 375 456 0.618 756 314 066

1/r

 0.683 261 767 652 1.688 316 800 717 2.687 924 397 413 3.687 750 406 344

1/r

 0.311 021 502 214 0.945 818 448 800 1.567 719 559 137 2.190 870 773 906

1/r

 1.116 662 8246(1) 6.017 408 8670(1) 14.927 623 7214(2) 27.840 105 671 33(2)

1/r

 0.155 104 152 58(3) 1.464 770 923 350(1) 4.082 232 787 55(2) 8.028 801 781 824(1)

1/r

 0.382 627 890 340 2.708 655 474 480 7.011 874 111 824(1) 13.313 954 940 144(1)

1/r

 0.253 077 567 065 1.920 943 921 900 5.069 790 932 379 9.717 071 116 528

δ(r

) 0.164 552 872 86(3) 1.810 429 318 49(3) 6.852 009 4344(1) 17.198 172 544 74(3)

δ(r

) 0.002 737 9923(3) 0.106 345 3712(2) 0.533 722 5371(9) 1.522 895 3541(2)

p

 2.462 558 614(3) 54.088 067 230(2) 310.547 150 179(6) 1047.278 491 476(2)

H

/α

− 0.008 875 022 10(1) − 0.139 094 690 556(1) − 0.427 991 611 178(9) −0.878 768 694 709(1)

11.4 Total Energies

As discussed in Chapts. 21 and 27, relativistic and QED

corrections must be added to the nonrelativistic eigen-

values of Sect. 11.3 before a meaningful comparison

with measured transition frequencies can be made. The

corrections are discussed in detail in [11.27, 28, 39].

The terms in order of decreasing size are:

1. Relativistic corrections of O(α

)

rel

= H

NFS

+ H

NFS

= H

mass

+ H

ssc

+ H

= H

+ H

soo

+ H

The various nonﬁne-structure (NFS)andﬁne-

structure (FS) terms are deﬁned in Sect. 21.1.The

off-diagonal matrix elements of H

mix states

of different spin and cause a break-down of LS-

coupling.

2. Anomalous magnetic moment corrections of O(α

)

The general FS matrix elements between states with

spins S and S



due to the anomalous magnetic mo-

ment a

are (see Sect. 27.4)

γS|H

anom

|γ



=2a

γS|H

S,S



soo



1 +



|γ



(11.37)

where a

= (g

− 2)/2 = α/(2π) − 0.328 479α

+···.

3. QED corrections of O(α

)

The lowest order QED corrections (including NFS

anomalous magnetic moment terms) can be written

in the form ∆

L,1

+ ∆

L,2

,where

∆E

L,1

Zα



ln(Zα)

−2

− ln k



× δ(r

) + δ(r

) (11.38)

∆E

L,2

= α



ln α −

· s



× δ(r

)−

Q , (11.39)

ln k

is the two-electron Bethe logarithm de-

ﬁned by (27.86)andQ is the matrix ele-

ment deﬁned by (27.83). For a highly excited

1snl state, ∆E

L,2

→ 0, δ(r

) + δ(r

)→Z

/π,

ln k

→ ln k

(1s) = 2.984 128 555, and ∆E

L,1

re-

duces to the Lamb shift of the 1s core

state (see Sects. 28.3.4 and 28.3.5). Thus

∆E

L,1

represents the electron–nucleus part of

the QED shift with the factor of Z

/π re-

placed by the correct electron density at the

nucleus. Accurate values of ln k

for two-

electron atoms and ions are tabulated in [11.40].

Part B 11.4

High Precision Calculations for Helium 11.4 Total Energies 209

For the low-lying S-states and P-states of he-

lium [11.31],

ln k

S ) = 2.983 865 861 , (11.40a)

ln k

S ) = 2.980 118 365 , (11.40b)

ln k

S ) = 2.977 742 459 , (11.40c)

ln k

P ) = 2.983 803 377 , (11.40d)

ln k

P ) = 2.983 690 995 . (11.40e)

For a 1snl state with large l, the asymptotic expan-

sion [11.41, 42]

ln k

(1snl) ≈ ln k

(1s) +



Z − 1



ln k

(nl)

+ 0.316 205(6)Z

−6



−4



+ ∆β(1snl) (11.41)

becomes essentially exact. Here ln k

(nl) is the one-

electron Bethe logarithm [11.43]and



−4



16(Z − 1)

[3n

−l(l + 1)]

(2l − 1)2l(2l + 1)(2l + 2)(2l + 3)

(11.42)

The correction ∆β(1snl) for higher order terms is

∆β(1snl

L) = 95.8(8)



−6



− 845(19)



−7



+ 1406(50)



−8



(11.43)

∆β(1snl

L) = 95.1(9)



−6



− 841(23)



−7



+ 1584(60)



−8



(11.44)

For example, for the 1s4f

F state, β(4

F ) =

2.984 127 1493(3).

For higher Z,1/Z expansions of [11.40] should be

used.

4. Relativistic ﬁnite mass corrections of O(α

µ/M)

Relativistic ﬁnite mass corrections come from two

sources. First, a transformation to relative coordi-

natesasin(11.3) is applied to the pairwise Breit

interactions among the three particles, generating

the new terms [11.44, 45]

∆ = ∆

+ ∆

+ 2

where

∆

−Zα



i, j



· p



(11.45)

∆

Zα



i= j

× p

· s

. (11.46)

Second, the mass polarization term H

in (11.3)

generates second-order cross-terms between H

and H

rel

. If the wave functions are calculated by

solving (11.4) in scaled atomic units, the H

cor-

rection is then automatically included to all orders

and the mass-corrected relativistic energy shift is (in

units of e

)

∆E

rel





%



mass

+ H

ssc

+ H

+ ∆



1 +



+ H

soo

+ H

+ ∆

(11.47)

with µ/m

= 1 − µ/M. The difference ∆E

rel

−

H

rel



∞

calculated for inﬁnite nuclear mass is the

relativistic ﬁnite mass correction.

5. Higher-order corrections

Spin-dependent terms of O(α

) are known in

their entirety, and have recently been calculated to

high precision [11.46]. Nonrelativistic operators for

the spin-independent part have recently been de-

rived and calculated for the 1s2s

state [11.47]

and 1s

state [11.48]. The dominant electron–

nucleus part is known from the one-electron Lamb

shift to be

∆E



L,1

= Zα



πZα



427

− 2ln2



+ 0.538 931



× δ(r

) + δ(r

)+O

(11.48)

and the electron–electron logarithmic part is [11.49]

∆E



L,2

= πα

ln α

−1

δ(r

) . (11.49)

As an example, ∆ E



L,1

contributes −50.336 MHz

and −88.267 MHz to the 2

P–2

Sand2

–2

transition frequencies respectively, while the differ-

ences between theory and experiment are ≈1MHz

and ≈−7 MHz for the two cases (see [11.39]). Thus,

two-electron corrections (for example, relativistic

corrections of relative order Zα to ∆ E

L,2

)areevi-

dently small.

Table 11.7 lists the calculated ionization energies

for all states of helium up to n = 10 and L = 7.

For the D-states and beyond, the uncertainties are

sufﬁciently small that these states can be taken

as known points of reference in the interpretation

of experimental transition frequencies. However,

Part B 11.4

210 Part B Atoms

long-range Casimir–Polder corrections [11.50–52]

are not included since they still lack experimen-

tal conﬁrmation [11.53]. The QED shifts are the

largest for the S- and P-states. The contributions

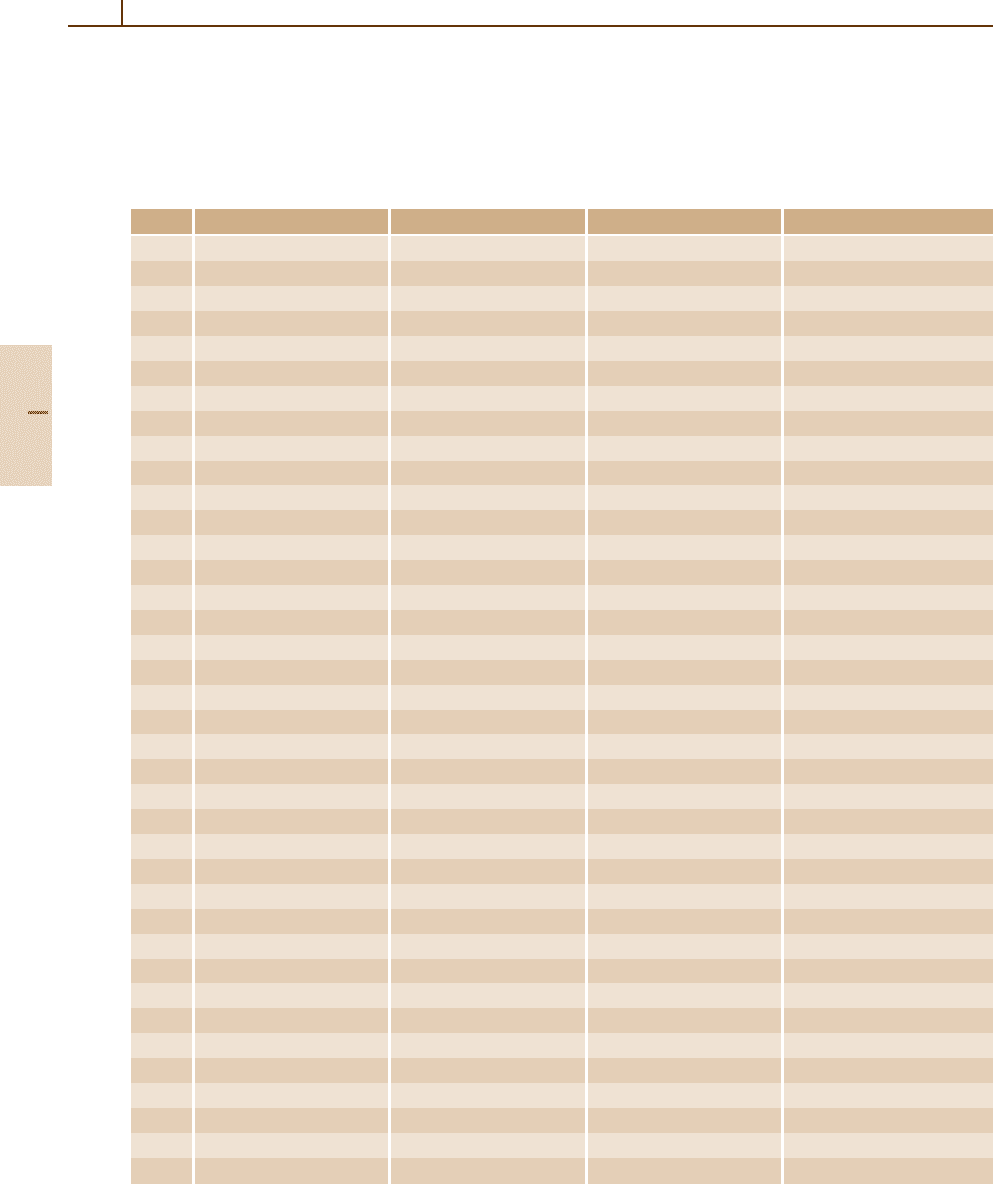
Table 11.7 Total ionization energies for

He, calculated with R

= 3 289 391 006.715 MHz

State E(n

) E(n

L−1

) E(n

L+1

)

1S 5 945 204 223.(91)

2S 960 332 041.(25) 1 152 842 741.2(6)

2P 814 709 150.(9) 876 078 642.(17) 876 108 265.(17) 876 110 558.(17)

3S 403 096 132.(8) 451 903 472.(8)

3P 362 787 968.(3) 382 109 902.(6) 382 118 017.(6) 382 118 676.(6)

3D 365 917 749.018(5) 366 018 892.97(1) 366 020 218.086(8) 366 020 293.415(2)

4S 220 960 311.(3) 240 210 377.(3)

4P 204 397 211.(1) 212 658 040.(3) 212 661 348.(2) 212 661 617.7(4)

4D 205 783 935.816(3) 205 842 547.918(6) 205 843 103.149(4) 205 843 139.171(1)

4F 205 620 797.145 205 621 029.602(1) 205 621 502.019(1) 205 621 287.974

5S 139 318 258.(2) 148 807 312.(2)

5P 130 955 541.8(7) 135 203 443.(2) 135 205 105.(1) 135 205 240.8(2)

5D 131 680 211.938(2) 131 714 043.938(3) 131 714 327.498(2) 131 714 346.719(1)

5F 131 595 041.501 131 595 195.235(1) 131 595 419.741(1) 131 595 327.454

5G 131 580 320.1329(1) 131 580 370.9465(2) 131 580 529.5188(2) 131 580 446.4606(1)

6S 95 807 682.0(9) 101 166 442.3(9)

6P 91 009 810.5(4) 93 472 041.5(9) 93 472 992.5(7) 93 473 070.0(1)

6D 91 433 655.841(1) 91 454 440.605(2) 91 454 604.486(1) 91 454 615.8316(5)

6F 91 383 852.0310(2) 91 383 954.3008(5) 91 384 078.8996(5) 91 384 030.7936(2)

6G 91 374 997.961 01(7) 91 375 027.4177(2) 91 375 119.1361(2) 91 375 071.113 69(7)

6H 91 372 940.612 32(3) 91 372 961.813 55(7) 91 373 021.530 29(7) 91 372 990.226 74(3)

7S 69 904 819.7(6) 73 222 269.3(6)

7P 66 901 127.5(2) 68 452 586.6(6) 68 453 180.9(4) 68 453 229.30(8)

7D 67 169 717.1562(6) 67 183 264.590(1) 67 183 367.7091(9) 67 183 374.9339(3)

7F 67 138 158.5571(1) 67 138 228.5582(3) 67 138 305.0654(3) 67 138 276.7195(1)

7G 67 132 455.947 62(6) 67 132 474.5216(1) 67 132 532.2572(1) 67 132 502.036 82(5)

7H 67 131 109.015 31(2) 67 131 122.366 81(5) 67 131 159.972 34(5) 67 131 140.259 24(2)

7I 67 130 692.480 04(1) 67 130 702.489 54(2) 67 130 728.915 04(2) 67 130 715.088 42(1)

8S 53 246 283.1(4) 55 440 834.1(4)

8P 51 242 587.4(2) 52 282 092.0(4) 52 282 488.0(3) 52 282 520.19(5)

8D 51 423 248.1412(4) 51 432 523.2471(8) 51 432 592.2921(6) 51 432 597.1660(2)

8F 51 402 021.6289(1) 51 402 071.1099(2) 51 402 121.5341(2) 51 402 103.3700(1)

8G 51 398 146.238 04(6) 51 398 158.6930(1) 51 398 197.3600(1) 51 398 177.125 22(7)

8H 51 397 221.579 33(2) 51 397 230.523 93(4) 51 397 255.716 51(4) 51 397 242.510 26(2)

8I 51 396 931.943 12(1) 51 396 938.648 74(2) 51 396 956.351 73(2) 51 396 947.088 95(1)

8K 51 396 822.734 66 51 396 827.929 60(1) 51 396 841.052 96(1) 51 396 834.203 39

9S 41 903 979.2(3) 43 430 382.9(3)

9P 40 501 246.4(1) 41 231 283.2(3) 41 231 560.2(2) 41 231 582.71(4)

from ∆ E

L,1

+ ∆E



L,1

and ∆ E

L,2

+ ∆E



L,2

for these

states are listed separately in Table 11.8. Appli-

cations to isotope shifts and measurements of the

nuclear radius are discussed in Sects. 16.2 and 90.1

Part B 11.4

High Precision Calculations for Helium 11.4 Total Energies 211

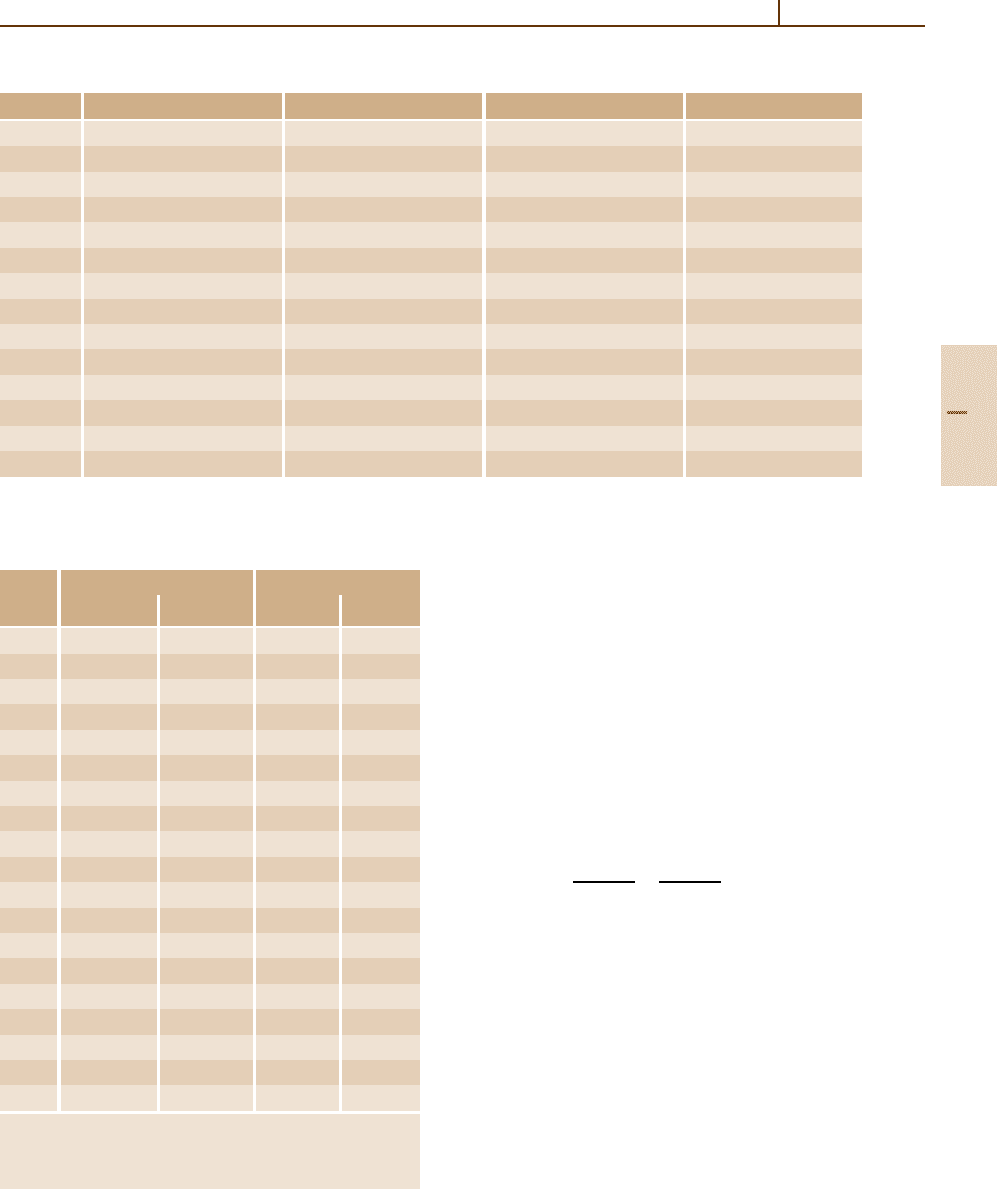
Table 11.7 Total ionization energies for

He, calculated with R

= 3 289 391 006.715 MHz, cont.

State E(n

) E(n

L−1

) E(n

L+1

)

9D 40 628 480.2670(3) 40 635 090.4472(6) 40 635 138.9215(4) 40 635 142.3606(2)

9F 40 613 531.5089(1) 40 613 567.5551(2) 40 613 602.5777(2) 40 613 590.2103(1)

9G 40 610 783.198 70(5) 40 610 791.952 31(8) 40 610 819.103 53(8) 40 610 804.897 45(5)

9H 40 610 123.035 88(2) 40 610 129.318 02(3) 40 610 147.011 47(3) 40 610 137.736 30(2)

9I 40 609 914.516 79(1) 40 609 919.226 38(2) 40 609 931.659 73(2) 40 609 925.154 18(1)

9K 40 609 835.097 59 40 609 838.746 17(1) 40 609 847.963 12(1) 40 609 843.152 44

10S 33 834 679.6(2) 34 938 883.9(2)

10P 32 814 665.30(8) 33 346 784.3(2) 33 346 985.6(1) 33 347 001.97(3)

10D 32 907 601.9150(2) 32 912 470.7559(4) 32 912 506.0839(3) 32 912 508.5992(1)

10F 32 896 683.0965(1) 32 896 710.0670(1) 32 896 735.3970(1) 32 896 726.5815(1)

10G 32 894 665.770 94(3) 32 894 672.155 70(5) 32 894 691.945 68(5) 32 894 681.592 48(3)

10H 32 894 178.909 63(1) 32 894 183.489 34(2) 32 894 196.387 81(2) 32 894 189.626 22(1)

10I 32 894 024.241 08(1) 32 894 027.674 38(1) 32 894 036.738 28(1) 32 894 031.995 73(1)

10K 32 893 964.927 04 32 893 967.586 86(1) 32 893 974.306 01(1) 32 893 970.799 02

Table 11.8 QED corrections to the ionization energy in-

cluded in Table 11.7 for the S- and P-states of helium (in

MHz)

E

L,1

+ E



L,1

E

L,2

+ E



L,2

State Singlet Triplet Singlet Triplet

1S −45 409. 4173.

2S −3134.4 − 4098.7 327.865 39.883

3S − 858.34 − 1030.29 91.258 8.468

4S − 349.09 − 402.29 37.303 3.203

5S − 174.93 − 196.80 18.735 1.544

6S − 99.807 − 110.505 10.702 0.861

7S − 62.221 − 68.113 6.677 0.528

8S − 41.369 − 44.904 4.441 0.347

9S − 28.885 − 31.147 3.102 0.240

10S − 20.959 − 22.482 2.251 0.173

2P − 103.6 1208.7 62.608 45.502

3P − 35.13 344.96 19.559 12.376

4P − 15.15 142.33 8.413 5.035

5P − 7.816 71.911 4.348 2.529

6P − 4.540 41.256 2.529 1.446

7P − 2.866 25.824 1.598 0.904

8P − 1.923 17.223 1.073 0.602

9P − 1.352 12.055 0.755 0.421

10P − 0.987 8.764 0.551 0.306

Includes additional contributions of −4MHz for the 1

state [11.48] and 3.00(1) MHz for the 2

S state [11.47] due

to electron–electron terms of O

∞

11.4.1 Quantum Defect Extrapolations

As discussed in Sect. 14.1, the ionization energies of an

isolated Rydberg series of states can be expressed in the

form

= R

(Z − 1)

∗2

, (11.50)

where Z − 1 is the screened nuclear charge and n

∗

the effective principal quantum number deﬁned by an

iterative solution to the equation

∗

= n − δ(n

∗

), (11.51)

where δ(n

∗

) is the quantum defect deﬁned by the Ritz

expansion

δ(n

∗

) = δ

(n − δ)

+··· (11.52)

with constant coefﬁcients δ

. The absence of odd terms

in this series is a special property of the eigenvalues of

Hamiltonians of the form H

+ V ,whereH

is a pure

one-electron Coulomb Hamiltonian, and V is a local,

short-range, spherically symmetric potential of arbitrary

strength (see [11.54] for further discussion). For the Ry-

dberg states of helium, odd terms must be included in

the Ritz expansion (11.52) due to relativistic and mass

polarization corrections, but they can be removed again

by ﬁrst adjusting the energies according to



= W

− ∆W

, (11.53)

Part B 11.4

212 Part B Atoms

where, to sufﬁcient accuracy [11.54] [see discussion

following (11.66)]

∆W

= R



−3α

(Z − 1)





(Z − 1)



1 +

(αZ)



(11.54)

Table 11.9 Quantum defects for the total energies of helium with the ∆W

term subtracted (11.54)

Value

0.139 718 064 86(21) 0.296 656 487 71(75)

0.027 835 737(18) 0.038 296 666(59)

0.016 792 29(41) 0.007 5131(12)

− 0.001 4590(31) − 0.004 5476(79)

0.002 9227(65) 0.002 180(14)

− 0.012 141 803 603(64) 0.068 328 002 51(27) 0.068 357 857 65(27) 0.068 360 283 79(23)

0.007 519 0804(59) − 0.018 641 975(24) −0.018 630 462(24) − 0.018 629 228(21)

0.013 977 80(15) − 0.012 331 65(57) − 0.012 330 40(57) − 0.012 332 75(51)

0.004 8373(12) − 0.007 9515(45) − 0.007 9512(45) − 0.007 9527(41)

0.001 2283(29) − 0.005 448(10) − 0.005 450(10) − 0.005 451(9)

0.002 113 378 464(49) 0.002 885 580 281(22) 0.002 890 941 493(25) 0.002 891 328 825(26)

− 0.003 090 0510(58) − 0.006 357 6012(27) − 0.006 357 1836(30) − 0.006 357 7040(33)

0.000 008 27(22) 0.000 336 67(11) 0.000 337 77(11) 0.000 336 70(13)

− 0.000 3094(31) 0.000 8394(16) 0.000 8392(16) 0.000 8395(18)

− 0.000 401(14) 0.000 3798(72) 0.000 4323(75) 0.000 3811(83)

0.000 440 294 26(62) 0.000 444 869 89(22) 0.000 448 594 83(28) 0.000 447 379 27(21)

− 0.001 689 446(65) − 0.001 739 275(24) − 0.001 727 232(30) − 0.001 739 217(23)

− 0.000 1183(20) 0.000 104 76(76) 0.000 1524(9) 0.000 104 78(71)

0.000 326(18) 0.000 0337(69) − 0.000 2486(83) 0.000 0331(64)

0.000 124 734 490(79) 0.000 125 707 43(12) 0.000 128 713 16(10) 0.000 127 141 67(11)

− 0.000 796 230(12) − 0.000 796 498(19) − 0.000 796 246(15) − 0.000 796 484(17)

− 0.000 012 05(53) − 0.000 009 80(81) − 0.000 011 89(66) − 0.000 009 85(75)

− 0.000 0136(69) − 0.000 019(11) − 0.000 0141(85) − 0.000 019(10)

0.000 047 100 899(61) 0.000 047 797 067(43) 0.000 049 757 614(51) 0.000 048 729 846(45)

− 0.000 433 2277(84) − 0.000 433 2322(55) − 0.000 433 2274(65) − 0.000 433 2281(57)

− 0.000 008 14(26) − 0.000 008 07(16) − 0.000 008 13(19) − 0.000 008 10(16)

0.000 021 868 881(17) 0.000 022 390 759(20) 0.000 023 768 483(14) 0.000 023 047 609(26)

− 0.000 261 0673(22) − 0.000 261 0680(28) − 0.000 261 0662(18) − 0.000 261 0672(35)

− 0.000 004 048(67) − 0.000 004 042(87) − 0.000 004 076(58) − 0.000 004 04(11)

with Z = 2 for helium. The quantum defect

parameters listed in Table 11.9 provide accu-

rate extrapolations to higher-lying Rydberg states,

with

= R

∗2

+ ∆W

. (11.55)

Part B 11.4