Drake G.W.F. (editor) Handbook of Atomic, Molecular, and Optical Physics

Подождите немного. Документ загружается.

Atomic Spectroscopy 10.11 Zeeman Effect 183

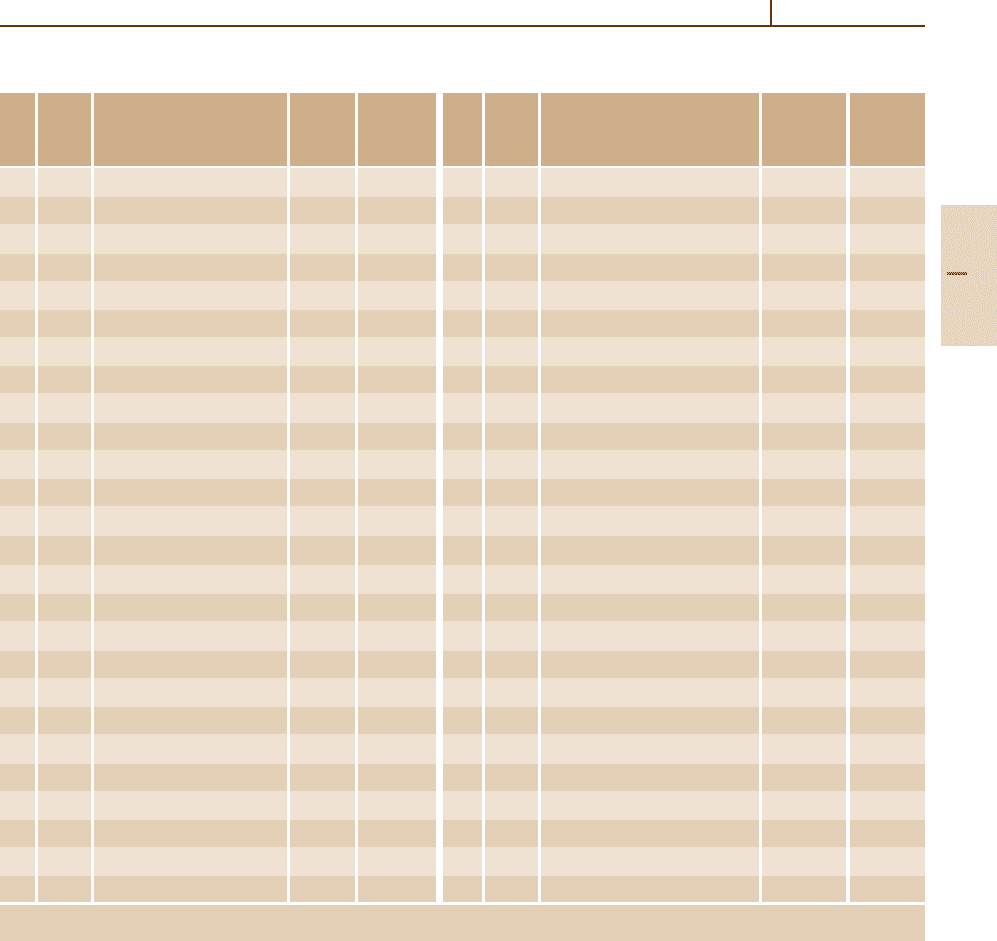
Table 10.3 Ground levels and ionization energies for the neutral atoms, cont.

Ionization Ionization

Elem- Ground energy Elem- Ground energy

Z ent Ground conﬁguration

level (eV) Z ent Ground conﬁguration

level (eV)

53 I [Kr] 4d

5 2

◦

3/2

10.4513 79 Au [Xe] 4f

1/2

9.2255

54 Xe [Kr] 4d

6 1

12.1298 80 Hg [Xe] 4f

2 1

10.4375

55 Cs [Xe] 6s

1/2

3.8939 81 Tl [Xe] 4f

◦

1/2

6.1082

56 Ba [Xe] 6s

2 1

5.2117 82 Pb [Xe] 4f

2 3

7.4167

57 La [Xe] 5d 6s

2 2

3/2

5.5769 83 Bi [Xe] 4f

3 4

◦

3/2

7.2855

58 Ce [Xe] 4f 5d 6s

2 1

◦

5.5387 84 Po [Xe] 4f

4 3

8.414

59 Pr [Xe] 4f

2 4

◦

9/2

5.473 85 At [Xe] 4f

5 2

◦

3/2

60 Nd [Xe] 4f

2 5

5.5250 86 Rn [Xe] 4f

6 1

10.7485

61 Pm [Xe] 4f

2 6

◦

5/2

5.582 87 Fr [Rn] 7s

1/2

4.0727

62 Sm [Xe] 4f

2 7

5.6437 88 Ra [Rn] 7s

2 1

5.2784

63 Eu [Xe] 4f

2 8

◦

7/2

5.6704 89 Ac [Rn] 6d 7s

2 2

3/2

5.17

64 Gd [Xe] 4f

5d 6s

2 9

◦

6.1498 90 Th [Rn] 6d

2 3

6.3067

65 Tb [Xe] 4f

2 6

◦

15/2

5.8638 91 Pa [Rn] 5f

(

) 6d 7s

(4, 3/2)

11/2

5.89

66 Dy [Xe] 4f

2 5

5.9389 92 U [Rn] 5f

(

◦

9/2

) 6d 7s

(9/2, 3/2)

◦

6.1941

67 Ho [Xe] 4f

2 4

◦

15/2

6.0215 93 Np [Rn] 5f

(

) 6d 7s

(4, 3/2)

11/2

6.2657

68 Er [Xe] 4f

2 3

6.1077 94 Pu [Rn] 5f

2 7

6.0260

69 Tm [Xe] 4f

2 2

◦

7/2

6.1843 95 Am [Rn] 5f

2 8

◦

7/2

5.9738

70 Yb [Xe] 4f

2 1

6.2542 96 Cm [Rn] 5f

6d 7s

2 9

◦

5.9914

71 Lu [Xe] 4f

5d 6s

2 2

3/2

5.4259 97 Bk [Rn] 5f

2 6

◦

15/2

6.1979

72 Hf [Xe] 4f

2 3

6.8251 98 Cf [Rn] 5f

2 5

6.2817

73 Ta [Xe] 4f

2 4

3/2

7.5496 99 Es [Rn] 5f

2 4

◦

15/2

6.42

74 W [Xe] 4f

2 5

7.8640 100 Fm [Rn] 5f

2 3

6.50

75 Re [Xe] 4f

2 6

5/2

7.8335 101 Md [Rn] 5f

2 2

◦

7/2

6.58

76 Os [Xe] 4f

2 5

8.28 102 No [Rn] 5f

2 1

6.65

77 Ir [Xe] 4f

2 4

9/2

9.02 103 Lr [Rn] 5f

7p?

◦

1/2

? 4.9?

78 Pt [Xe] 4f

8.9588 104 Rf [Rn] 5f

? 6.0?

An element symbol in brackets represents the electrons in the ground conﬁguration of that element

10.11 Zeeman Effect

The Zeeman effect for “weak” magnetic ﬁelds (the

anomalous Zeeman effect) is of special interest because

of the importance of Zeeman data in the analysis and

theoretical interpretation of complex spectra. In a weak

ﬁeld, the J value remains a good quantum number

although in general a level is split into magnetic sub-

levels [10.3]. The g value of such a level may be deﬁned

by the expression for the energy shift of its magnetic

sublevel having magnetic quantum number M,which

has one of the 2J + 1values,−J, −J + 1, ..., J:

∆E = gMµ

B . (10.4)

Part B 10.11

184 Part B Atoms

The magnetic ﬂux density is B and µ

is the Bohr

magneton (µ

= e /2m

The wavenumber shift ∆σ corresponding to this

energy shift is

∆σ = gM(0.466 86B cm

−1

), (10.5)

with B representing the numerical value of the magnetic

ﬂux density in teslas. The quantity in parentheses, the

Lorentz unit, is of the order of 1 or 2 cm

−1

for typical

ﬂux densities used to obtain Zeeman-effect data with

classical spectroscopic methods. Accurate data can be

obtained with much smaller ﬁelds, of course, by using

higher-resolution techniques such as laser spectroscopy.

Most of the g values now available for atomic energy

levels were derived by application of the above formula

(for each of the two combining levels) to measurements

of optical Zeeman patterns. A single transverse-Zeeman-

effect pattern (two polarizations, resolved components,

and sufﬁciently complete) can yield the J value and the

g value for each of the two levels involved.

Neglecting a number of higher-order effects, we can

evaluate the g value of a level β J belonging to a pure

LS-coupling term using the formula

βSL J

= 1+



− 1) (10.6)

J(J + 1) − L(L + 1) + S(S + 1)

2J(J + 1)



The independence of this expression from any other

quantum numbers (represented by β) such as the con-

ﬁguration, etc., is important. The expression is derived

from vector coupling formulas by assuming a g value of

unity for a pure orbital angular momentum and writing

the g value for a pure electron spin as g

[10.15]. A value

of 2 for g

yields the Landé formula. If the anomalous

magnetic moment of the electron is taken into account,

the value of g

is 2.002 3193. “Schwinger” g values ob-

tained with this more accurate value for g

are given for

levels of SL terms in [10.8].

The usefulness of g

SL J

values is enhanced by their

relation to the g values in intermediate coupling. In the

notationusedin(10.2) for the wave function of a level

βJ in intermediate coupling, the corresponding g value

is given by

βJ



γSL

SL J

|c(γSL J)|

, (10.7)

where the summation is over the same set of quantum

numbers as for the wave function. The g

βJ

value is thus

a weighted average of the Landé g

SL J

values,the weight-

ing factors being just the corresponding component

percentages.

Formulas for magnetic splitting factors in the

and J

coupling schemes are given in [10.8]

and [10.15]. Some higher-order effects that must be

included in more accurate Zeeman-effect calculations

are treated by Bethe and Salpeter [10.4] and by

Wybourne [10.15], for example. High precision calcula-

tions for helium are given in [10.16]. See also Chapt. 13

and Chapt. 15.

10.12 Term Series, Quantum Defects, and Spectral-Line Series

The Bohr energy levels for hydrogen or for a hydrogenic

(one-electron) ion are given by

=−

, (10.8)

in units of the Rydberg for the appropriate nuclear mass.

For a multielectron atom, the deviations of a series of

(core)nl levels from hydrogenic E

values may be due

mainly to core penetration by the nl electron (low l-value

series), or core polarization by the nl electron (high

l-value series), or a combination of the two effects. In

either case it can be shown that these deviations can

be approximately represented by a constant quantum

defect δ

in the Rydberg formula,

=−

(n − δ

)

=−

∗

)

, (10.9)

where Z

is the charge of the core and n

∗

= n − δ is the

effective principal quantum number. If the core includes

only closed subshells, the E

values are with respect

to a value of zero for the (core)

level, i. e., the

level is the limit of the (core)nl series. If the quantities in

(10.9) are taken as positive, they represent term values

or ionization energies; the term value of the ground level

of an atom or ion with respect to the ground level of the

next higher ion is thus the principal ionization energy.

If the core has one or more open subshells, the series

limit may be the baricenter of the entire core conﬁgura-

tion, or any appropriate sub-structure of the core, down

to and including a single level. The E

values refer

to the series of corresponding (core)nl structures built

on the particular limit structure. The value of the quan-

tum defect depends to some extent on which (core)nl

structures are represented by the series formula.

Part B 10.12

Atomic Spectroscopy 10.14 Spectral Wavelength Ranges, Dispersion of Air 185

The quantum defect in general also has an energy

dependence that must be taken into account if lower

members of a series are to be accurately represented by

(10.9). For an unperturbed series, this dependence can

be expressed by the extended Ritz formula

δ = n − n

∗

= δ

(n − δ

)

(n − δ

)

+··· , (10.10)

with δ

, a, b ... constants for the series (δ

being

the limit value of the quantum defect for high se-

ries members) [10.17]. The value of a is usually

positive for core-penetration series and negative for

core-polarization series. A discussion of the foundations

of the Ritz expansion and application to high precision

calculations in helium is given in [10.18].

A spectral-line series results from either emission or

absorption transitions involving a common lower level

and a series of successive (core)nl upper levels differ-

ing only in their n values. The principal series of Na i,

1/2

− np

◦

1/2,3/2

(n ≥ 3), is an example. The reg-

ularity of successive upper term values with increasing n

(10.9,10.10) is of course observed in line series; the

intervals between successive lines decrease in a regu-

lar manner towards higher wavenumbers, and the series

of increasing wavenumbers converges towards the term

value of the lower level as a limit.

10.13 Sequences

Several types of sequences of elements and/or ionization

stages are useful because of regularities in the progres-

sive values of parameters relating to structure and other

properties along the sequences. All sequence names may

refer either to the atoms and/or ions of the sequence or

to their spectra.

10.13.1 Isoelectronic Sequence

A neutral atom and those ions of other elements hav-

ing the same number of electrons as the atom comprise

an isoelectronic sequence. (Note that a negative ion

having this number of electrons is a member of the se-

quence.) An isoelectronic sequence is named according

to its neutral member; for example, the Na i isolectronic

sequence.

10.13.2 Isoionic, Isonuclear,

and Homologous Sequences

An isoionic sequence comprises atoms or ions of differ-

ent elements having the same charge. Such sequences

have probably been most useful along the d- and f-shell

rows of the periodic table. Isoionic analyses have also

been carried out along p-shell rows, however, and a ﬁne-

structure regularity covering spectra of the p-shell atoms

throughout the periodic table is known [10.19].

The atom and successive ions of a particular element

comprise the isonuclear sequence for that element.

The elements of a particular column and subgroup

in the periodic table are homologous. Thus the C, Si,

Ge, Sn, and Pb atoms belong to a homologous se-

quence having np

ground conﬁgurations (Table 10.3).

The singly ionized atoms of these elements comprise

another example of a homologous sequence.

10.14 Spectral Wavelength Ranges, Dispersion of Air

The ranges of most interest for optical atomic spec-

troscopy are:

∼ 2–20 µm mid-infrared (ir)

700–2000 nm near ir

400–700 nm visible

200–400 nm near ultraviolet (uv)

100–200 nm vacuum uv or far uv

10–100 nm extreme uv (euv or xuv)

< 10 nm soft X-ray, X-ray

The above correspondence of names to ranges

should not be taken as exact; the variation as to the ex-

tent of some of the named ranges found in the literature

is considerable.

Wavelengths in standard air are often tabulated for

the region longer than 200 nm. These wavelengths can

be related to energy-level differences by conversion to

the corresponding (vacuum) wavenumbers or frequen-

cies [10.20,21].

Part B 10.14

186 Part B Atoms

10.15 Wavelength (Frequency) Standards

In 2001 the Comité International des Poids et

Mesures recommended values for optical frequency

standards from stabilized lasers using various ab-

sorbing atoms, atomic ions, and molecules [10.22].

These frequencies range from 29 054 057 446 579 Hz

(10.318 436 884 460 µm; relative standard uncer-

tainty 1.4×10

−13

) for a transition in OsO

1 267 402 452 889.92 kHz (236.540 853 549 75 nm; rel-

ative standard uncertainty 3.6×10

−13

) for a transition

in the

115

ion [10.22].

Extensive tables of wavenumbers for molecular

transitions in the mid-ir range of 2.3to20.5 µm

are included in a calibration atlas published in

1991 [10.23]. Wavenumbers of Ar i [10.24]and

Ar ii [10.25] emission lines having uncertainties as small

as 0.0003 cm

−1

are included in tables for these spec-

tra covering a broad range from 222 nm to 5.865 µm.

Measurements of U and Th lines (575 to 692 nm)

suitable for wavenumber calibration at uncertainty lev-

els of 0.0003 cm

−1

or 0.0004 cm

−1

were reported

in [10.26]. Comprehensive tables of lines for U [10.27],

Th [10.28], and I

[10.29, 30] are useful for cali-

bration at uncertainty levels of 0.002 to 0.003 cm

−1

the atlas of the Th spectrum extending down to

278 nm.

A 1974 compilation gives reference wavelengths for

some 5400 lines of 38 elements covering the range

1.5nm to 2.5 µm, with most uncertainties between

−5

and 2× 10

−4

nm [10.31]. The wavelengths for

some 1100 Fe lines selected from the Fe/Ne hollow-

cathode spectrum have been recommended for reference

standards over the range 183 nm to 4.2 µm, with

wavenumber uncertainties 0.001 to 0.002 cm

−1

[10.32].

Wavelengths for about 3000 vuv and uv lines (110

to 400 nm) from a Pt/Ne hollow-cathode lamp have

been determined with uncertainties of 0.0002 nm or

less [10.13, 33]. More recent high-accuracy measure-

ments of ultraviolet lines of Fe i,Gei,Krii,andPti, ii

include some wavelengths with uncertainties smaller

than 10

−5

nm [10.34]. The wavelengths tabulated for the

Kr and Pt lines in [10.34] extend from 171 to 315 nm,

and the accuracies of earlier measurements of a num-

ber of spectra useful for wavelength calibration are

discussed.

10.16 Spectral Lines: Selection Rules, Intensities, Transition

Probabilities, f Values, and Line Strengths

The selection rules for discrete transitions are given in

Table 10.4.

10.16.1 Emission Intensities

(Transition Probabilities)

The total power  radiated in a spectral line of frequency

ν per unit source volume and per unit solid angle is



line

= (4π)

−1

hν A

, (10.11)

where A

is the atomic transition probability and N

the number per unit volume (number density) of excited

atoms in the upper (initial) level k. For a homoge-

neous light source of length l and for the optically thin

case, where all radiation escapes, the total emitted line

intensity (SI quantity: radiance) is

line

= 

line

l =

+∞



I(λ)dλ

= (4π)

−1

(hc/λ

) A

l , (10.12)

where I(λ) is the speciﬁc intensity at wavelength λ,and

the wavelength at line center.

10.16.2 Absorption f Values

In absorption, the reduced absorption

W(λ) =[I(λ) − I



(λ)]/I(λ) (10.13)

is used, where I(λ) is the incident intensity at wavelength

λ, e.g., from a source providing a continuous back-

ground, and I



(λ) the intensity after passage through

the absorbing medium. The reduced line intensity from

a homogeneous and optically thin absorbing medium of

length l follows as

+∞



W(λ) dλ =

4

l ,

(10.14)

where f

is the atomic (absorption) oscillator strength

(dimensionless).

Part B 10.16

Atomic Spectroscopy 10.16 Spectral Lines: Selection Rules, Intensities, Transition Probabilities, f Values, and Line Strengths 187

Table 10.4 Selection rules for discrete transitions

Electric dipole (E1) Magnetic dipole (M1) Electric quadrupole (E2)

(“allowed”) (“forbidden”) (“forbidden”)

Rigorous rules 1. ∆J = 0, ±1 ∆J = 0, ±1 ∆J = 0, ±1, ±2

(except 0 ↔ 0) (except 0 ↔ 0) (except 0 ↔ 0, 1/2 ↔ 1/2, 0 ↔ 1)

2. ∆M = 0, ±1 ∆M = 0, ±1 ∆M = 0, ±1, ±2

(except 0 ↔ 0when∆J = 0) (except 0 ↔ 0when∆ J = 0)

3. Parity change No parity change No parity change

With negligible conﬁguration 4. One electron jumping, No change in electron No change in electron

interaction

with ∆l =±1, conﬁguration; i. e., conﬁguration; or one

∆n arbitrary for all electrons, electron jumping with

∆l = 0, ∆n = 0 ∆l = 0, ±2, ∆n arbitrary

For LS coupling only 5. ∆S = 0 ∆S = 0 ∆S = 0

6. ∆L = 0, ±1 ∆L = 0 ∆L = 0, ±1, ±2

(except 0 ↔ 0) ∆ J =±1 (except 0 ↔ 0, 0 ↔ 1)

10.16.3 Line Strengths

and f

are the principal atomic quantities related to

line intensities. In theoretical work, the line strength S

is also widely used (see Chapt. 21):

S = S(i, k) = S(k, i) =|R

, (10.15)

=ψ

|P|ψ

 , (10.16)

where ψ

and ψ

are the initial- and ﬁnal-state wave

functions and R

is the transition matrix element of

the appropriate multipole operator P (R

involves an

integration over spatial and spin coordinates of all

N electrons of the atom or ion).

10.16.4 Relationships Between A, f,andS

The relationships between A, f ,andS for electric dipole

(E1, or allowed) transitions in SI units (A in s

−1

, λ in m,

S in m

)are

2π e

c

16π

3h 

S . (10.17)

Table 10.5 Wavelengths λ, upper energy levels E

, statistical weights g

and g

of lower and upper levels, and transition

probabilities A

for persistent spectral lines of neutral atoms. Many tabulated lines are resonance lines (marked “g”),

where the lower energy level belongs to the ground term

Spectrum λ

Accuracy

(Å) (cm

−1

) g

(10

−1

)

Ag 3280.7g 30 473 2 4 1.4 B

3382.9g 29 552 2 2 1.3 B

5209.1 48 744 2 4 0.75 D

5465.5 48 764 4 6 0.86 D

Numerically, in customary units (A in s

−1

, λ in Å,

S in atomic units),

6.6702×10

2.0261×10

S ,

(10.18)

and for S and ∆E in atomic units,

(∆E/g

) S . (10.19)

and g

are the statistical weights, which are obtained

from the appropriate angular momentum quantum num-

bers. Thus for the lower (upper) level of a spectral

line, g

i(k)

= 2J

i(k)

+ 1 and for the lower (upper) term

of a multiplet,

i(k)



i(k)

(2J

i(k)

+ 1) = (2L

i(k)

+ 1)(2S

i(k)

+ 1).

(10.20)

The A

values for strong lines of selected elements

are given in Table 10.5. For comprehensive numerical

Part B 10.16

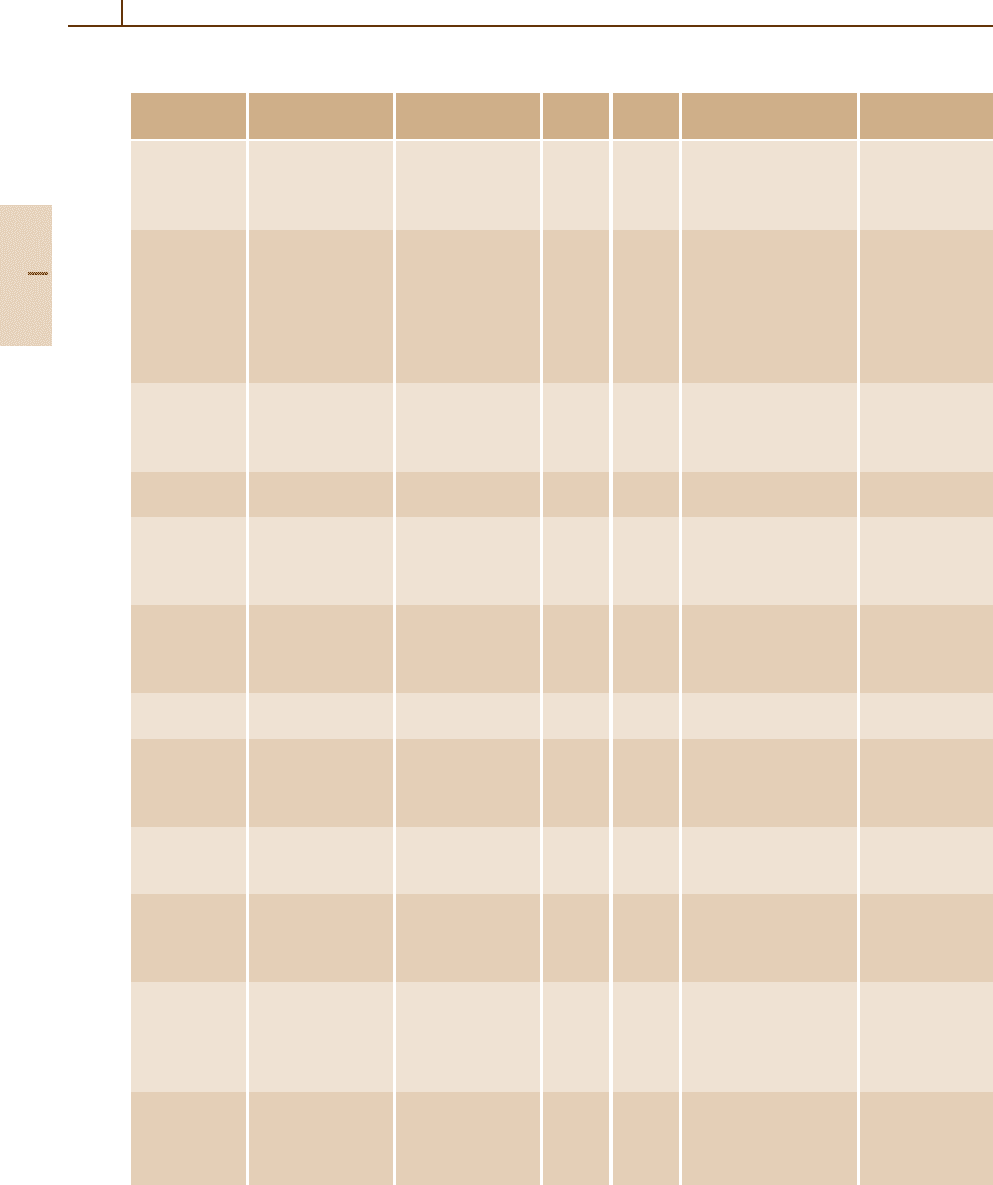
188 Part B Atoms

Table 10.5 Wavelengths λ, upper energy levels E

, statistical weights g

and g

of lower and upper levels, ... , cont.

Spectrum λ

Accuracy

(Å) (cm

−1

) g

(10

−1

)

Al 3082.2g 32 435 2 4 0.63 C

3092.7g 32 437 4 6 0.74 C

3944.0g 25 348 2 2 0.493 C

3961.5g 25 348 4 2 0.98 C

Ar 1048.2g 95 400 1 3 5.32 B

4158.6 117 184 5 5 0.0140 B

3453.5 32 431 10 12 1.1 C+

4259.4 118 871 3 1 0.0398 B

7635.1 106 238 5 5 0.245 C

7948.2 107 132 1 3 0.186 C

8115.3 105 463 5 7 0.331 C

As 1890.4g 52 898 4 6 2.0 D

1937.6g 51 610 4 4 2.0 D

2288.1 54 605 6 4 2.8 D

2349.8 53 136 4 2 3.1 D

Au 2428.0g 41 174 2 4 1.99 B+

2676.0g 37 359 2 2 1.64 B+

B 1825.9g 54 767 2 4 1.76 B

1826.4g 54 767 4 6 2.11 B

2496.8g 40 040 2 2 0.864 C

2497.7g 40 040 4 2 1.73 C

Ba 5535.5g 18 060 1 3 1.19 B

6498.8 24 980 7 7 0.54 D

7059.9 23 757 7 9 0.50 D

7280.3 22 947 5 7 0.32 D

Be 2348.6g 42 565 1 3 5.547 AA

2650.6 59 696 9 9 4.24 AA

Bi 2228.3g 44 865 4 4 0.89 D

2898.0 45 916 4 2 1.53 C

2989.0 44 865 4 4 0.55 C

3067.7g 32 588 4 2 2.07 C

Br 1488.5g 67 184 4 4 1.2 D

1540.7g 64 907 4 4 1.4 D

7348.5 78 512 4 6 0.12 D

C 1561.4g 64 087 5 7 1.18 A

1657.0g 60 393 5 5 2.52 A

1930.9 61 982 5 3 3.51 B+

2478.6 61 982 1 3 0.340 B+

Ca 4226.7g 23 652 1 3 2.18 B+

4302.5 38 552 5 5 1.36 C+

5588.8 38 259 7 7 0.49 D

6162.2 31 539 5 3 0.354 C

6439.1 35 897 7 9 0.53 D

Cd 2288.0g 43 692 1 3 5.3 C

3466.2 59 498 3 5 1.2 D

3610.5 59 516 5 7 1.3 D

5085.8 51 484 5 3 0.56 C

Part B 10.16

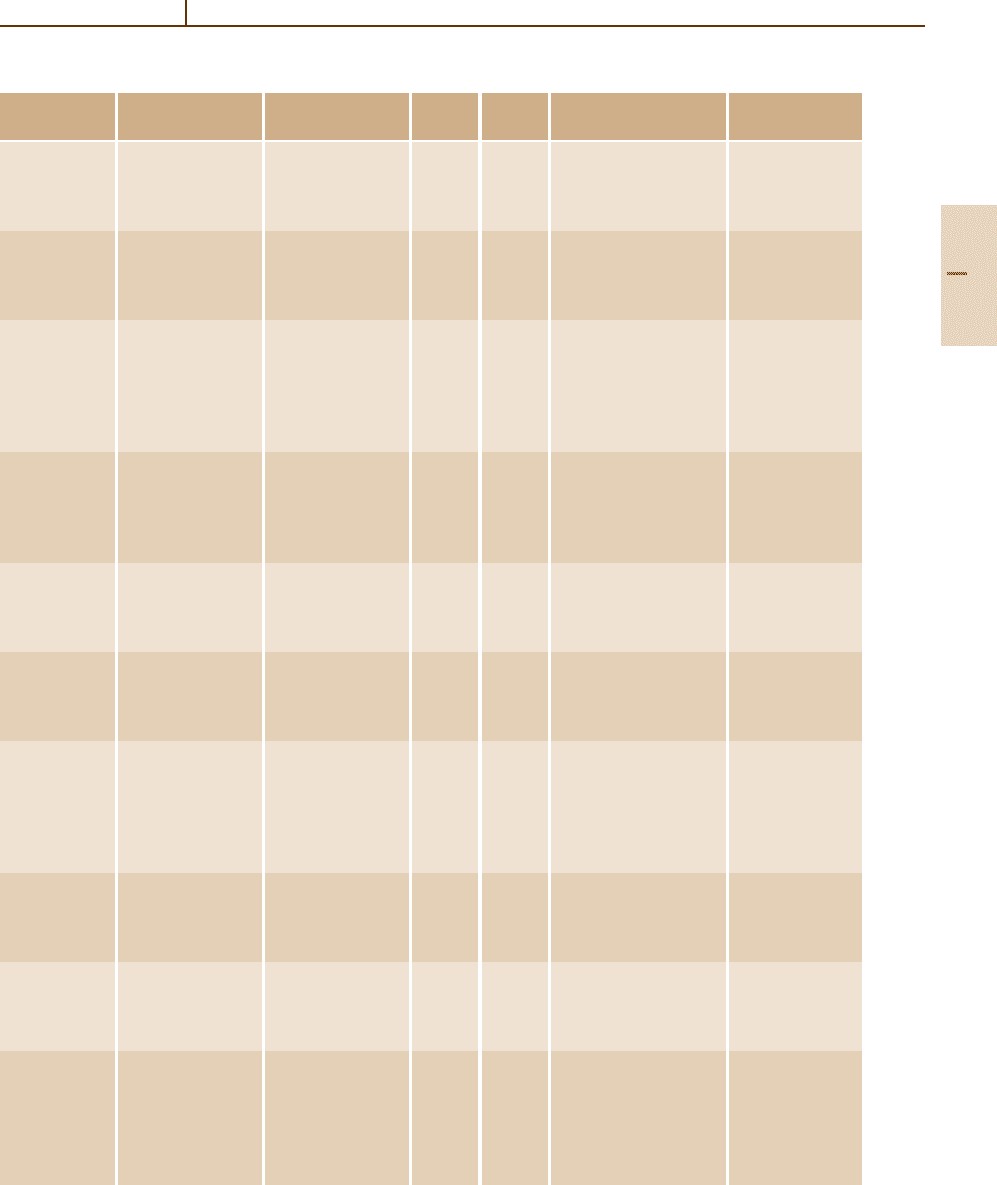
Atomic Spectroscopy 10.16 Spectral Lines: Selection Rules, Intensities, Transition Probabilities, f Values, and Line Strengths 189

Table 10.5 Wavelengths λ, upper energy levels E

, statistical weights g

and g

of lower and upper levels, cont.

Spectrum λ

Accuracy

(Å) (cm

−1

) g

(10

−1

)

Cl 1347.2g 74 226 4 4 4.19 C

1351.7g 74 866 2 2 3.23 C

4526.2 96 313 4 4 0.051 C

7256.6 85 735 6 4 0.15 C

Co 3405.1 32 842 10 10 1.0 C+

3453.5 32 431 10 12 1.1 C+

3502.3 32 028 10 8 0.80 C+

3569.4 35 451 8 8 1.6 C

Cr 3578.7g 27 935 7 9 1.48 B

3593.5g 27 820 7 7 1.50 B

3605.3g 27 729 7 5 1.62 B

4254.3g 23 499 7 9 0.315 B

4274.8g 23 386 7 7 0.307 B

5208.4 26 788 5 7 0.506 B

Cs 3876.1g 25 792 2 4 0.0038 C

4555.3g 21 946 2 4 0.0188 C

4593.2g 21 765 2 2 0.0080 C

8521.1g 11 732 2 4 0.3276 AA

8943.5g 11 178 2 2 0.287 A

Cu 2178.9g 45 879 2 4 0.913 B

3247.5g 30 784 2 4 1.39 B

3274.0g 30 535 2 2 1.37 B

5218.2 49 942 4 6 0.75 C

F 954.83g 104 731 4 4 5.77 C

6856.0 116 987 6 8 0.494 C

7398.7 115 918 6 6 0.285 C+

7754.7 117 623 4 6 0.382 C+

Fe 3581.2 34 844 11 13 1.02 B+

3719.9g 26 875 9 11 0.162 B+

3734.9 33 695 11 11 0.901 B+

3745.6g 27 395 5 7 0.115 B+

3859.9g 25 900 9 9 0.0969 B+

4045.8 36 686 9 9 0.862 B+

Ga 2874.2g 34 782 2 4 1.2 C

2943.6g 34 788 4 6 1.4 C

4033.0g 24 789 2 2 0.49 C

4172.0g 24 789 4 2 0.92 C

Ge 2651.6g 37 702 1 3 0.85 C

2709.6g 37 452 3 1 2.8 C

2754.6g 37 702 5 3 1.1 C

3039.1 40 021 5 3 2.8 C

He 537.03g 186 209 1 3 5.663 AA

584.33g 171 135 1 3 17.99 AA

3888.6 185 565 3 9

0.09475 AA

4026.2 193 917 9 15

0.1160 AA

4471.5 191 445 9 15

0.2458 AA

5875.7 186 102 9 15

0.7070 AA

Part B 10.16

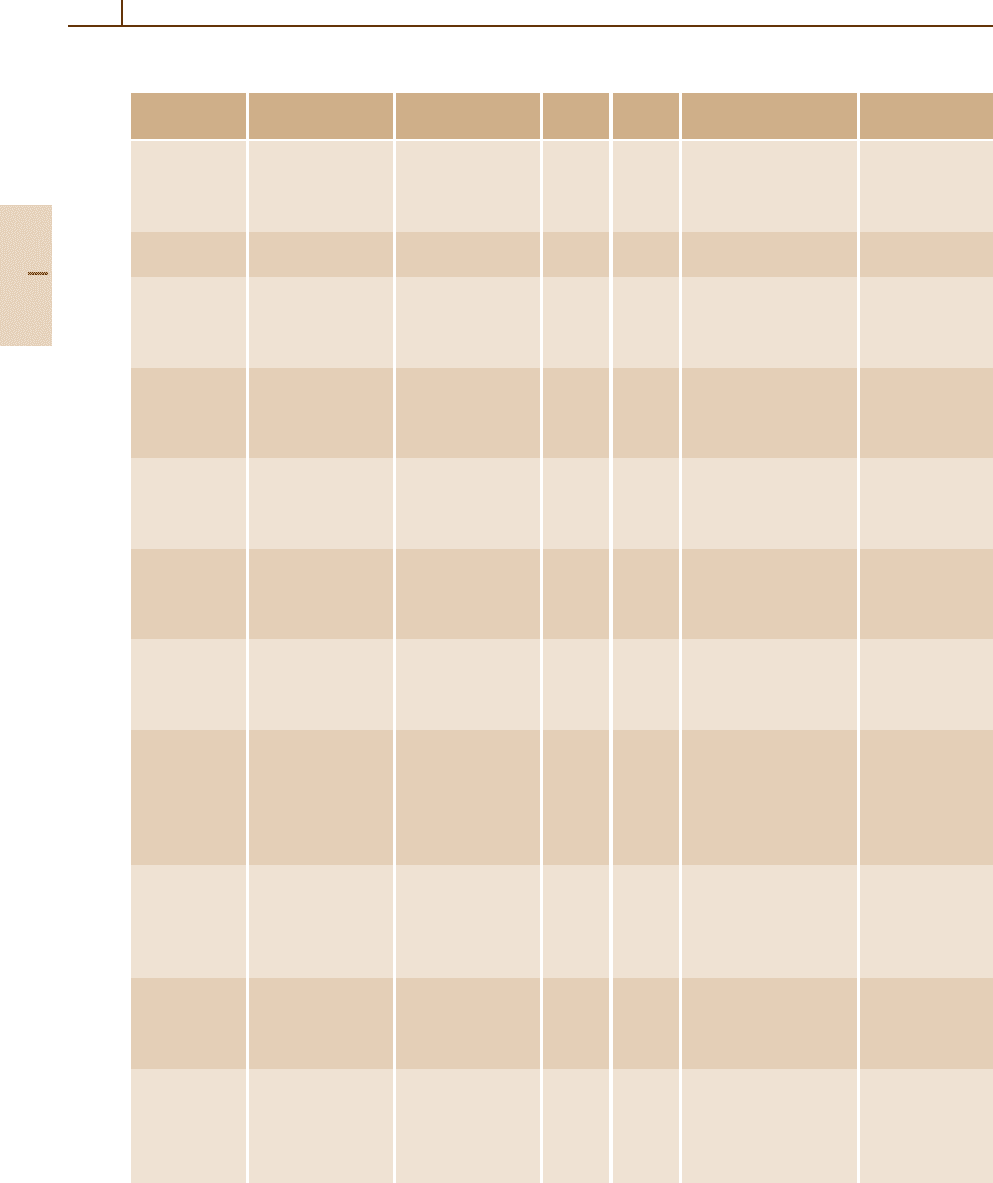
190 Part B Atoms

Table 10.5 Wavelengths λ, upper energy levels E

, statistical weights g

and g

of lower and upper levels, cont.

Spectrum λ

Accuracy

(Å) (cm

−1

) g

(10

−1

)

Hg 2536.5g 39 412 1 3 0.0800 B

3125.7 71 396 3 5 0.656 B

4358.3 62 350 3 3 0.557 B

5460.7 62 350 5 3 0.487 B

I 1782.8g 56 093 4 4 2.71 C

1830.4g 54 633 4 6 0.16 D

In 3039.4g 32 892 2 6 1.3 D

3256.1g 32 915 4 4 1.3 D

4101.8g 24 373 2 2 0.56 C

4511.3g 24 373 4 2 1.02 C

K 4044.1g 24 720 2 4 0.0124 C

4047.2g 24 701 2 2 0.0124 C

7664.9g 13 043 2 4 0.387 B+

7699.0g 12 985 2 2 0.382 B+

Kr 5570.3 97 919 5 3 0.021 D

5870.9 97 945 3 5 0.018 D

7601.5 93 123 5 5 0.31 D

8112.9 92 294 5 7 0.36 D

Li 3232.7g 30 925 2 6

0.01002 A

4602.9 36 623 6 10

0.233 B

6103.6 31 283 6 10

0.6860 AA

6707.8g 14 904 2 6

0.3691 AA

Mg 2025.8g 49 347 1 3 0.84 D

2852.1g 35 051 1 3 4.95 B

4703.0 56 308 3 5 0.255 C

5183.6 41 197 5 3 0.575 B

Mn 2794.8g 35 770 6 8 3.7 C

2798.3g 35 726 6 6 3.6 C

2801.1g 35 690 6 4 3.7 C

4030.8g 24 820 6 8 0.17 C+

4033.1g 24 788 6 6 0.165 C+

4034.4g 24 779 6 4 0.158 C+

N 1199.6g 83 365 4 6 4.01 B+

1492.6 86 221 6 4 3.13 B+

4935.1 106 478 4 2 0.0176 B

7468.3 96 751 6 4 0.193 B+

8216.3 95 532 6 6 0.223 B+

Na 5890.0g 16 973 2 4 0.611 AA

5895.9g 16 956 2 2 0.610 AA

5682.6 34 549 2 4 0.103 C

8183.3 29 173 2 4 0.453 C

Ne 735.90g 135 889 1 3 6.11 B

743.72g 134 459 1 3 0.486 B

5852.5 152 971 3 1 0.682 B

6402.2 149 657 5 7 0.514 B

6074.3 150 917 3 1 0.603 B

Part B 10.16

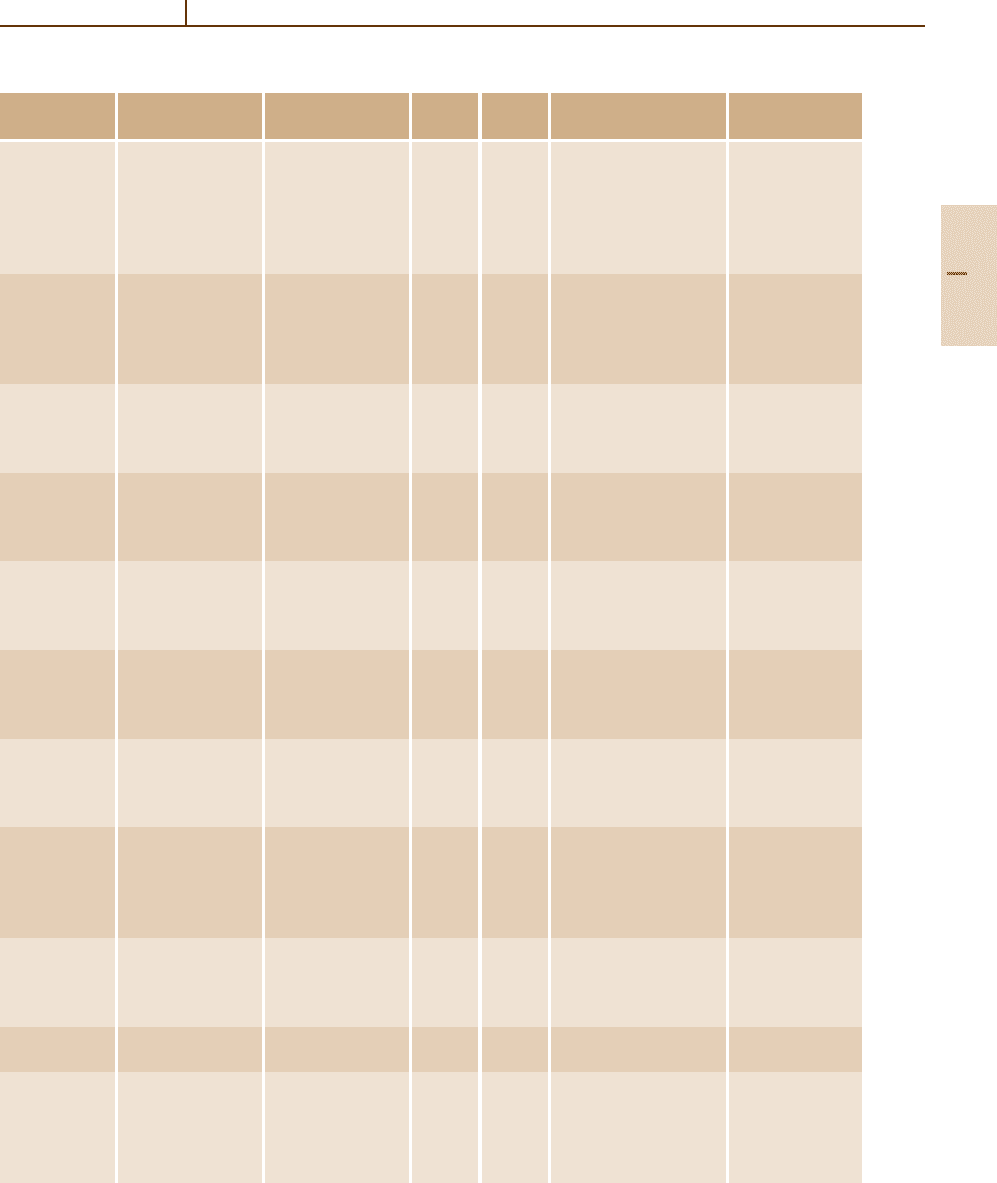
Atomic Spectroscopy 10.16 Spectral Lines: Selection Rules, Intensities, Transition Probabilities, f Values, and Line Strengths 191

Table 10.5 Wavelengths λ, upper energy levels E

, statistical weights g

and g

of lower and upper levels, cont.

Spectrum λ

Accuracy

(Å) (cm

−1

) g

(10

−1

)

Ni 3101.6 33 112 5 7 0.63 C+

3134.1 33 611 3 5 0.73 C+

3369.6g 29 669 9 7 0.18 C

3414.8 29 481 7 9 0.55 C

3524.5 28 569 7 5 1.0 C

3619.4 31 031 5 7 0.66 C

O 1302.2g 76 795 5 3 3.41 A

4368.2 99 681 3 9

0.007 58 B

5436.9 105 019 7 5 0.0180 C+

7156.7 116 631 5 5 0.505 B

7771.9 86 631 5 7 0.369 A

P 1775.0g 56 340 4 6 2.17 C

1782.9g 56 090 4 4 2.14 C

2136.2 58 174 6 4 2.83 C

2535.6 58 174 4 4 0.95 C

Pb 2802.0g 46 329 5 7 1.6 D

2833.1g 35 287 1 3 0.58 D

3683.5g 34 960 3 1 1.5 D

4057.8g 35 287 5 3 0.89 D

Rb 4201.8g 23 793 2 4 0.018 C

4215.5g 23 715 2 2 0.015 C

7800.3g 12 817 2 4 0.370 B

7947.6g 12 579 2 2 0.340 B

S 1474.0g 67 843 5 7 1.6 D

1666.7 69 238 5 5 6.3 C

1807.3g 55 331 5 3 3.8 C

4694.1 73 921 5 7 0.0067 D

Sc 3907.5g 25 585 4 6 1.28 C+

3911.8g 25 725 6 8 1.37 C+

4020.4g 24 866 4 4 1.65 C+

4023.7g 25 014 6 6 1.44 C+

Si 2506.9g 39 955 3 5 0.466 C

2516.1g 39 955 5 5 1.21 C

2881.6 40 992 5 3 1.89 C

5006.1 60 962 3 5 0.028 D

5948.5 57 798 3 5 0.022 D

Sn 2840.0g 38 629 5 5 1.7 D

3034.1g 34 641 3 1 2.0 D

3175.1g 34 914 5 3 1.0 D

3262.3 39 257 5 3 2.7 D

Sr 2428.1g 41 172 1 3 0.17 C

4607.3g 21 698 1 3 2.01 B

Ti 3642.7g 27 615 7 9 0.774 B

3653.5g 27 750 9 11 0.754 C+

3998.6g 25 388 9 9 0.408 B

4981.7 26 911 11 13 0.660 C+

5210.4g 19 574 9 9 0.0357 C+

Part B 10.16

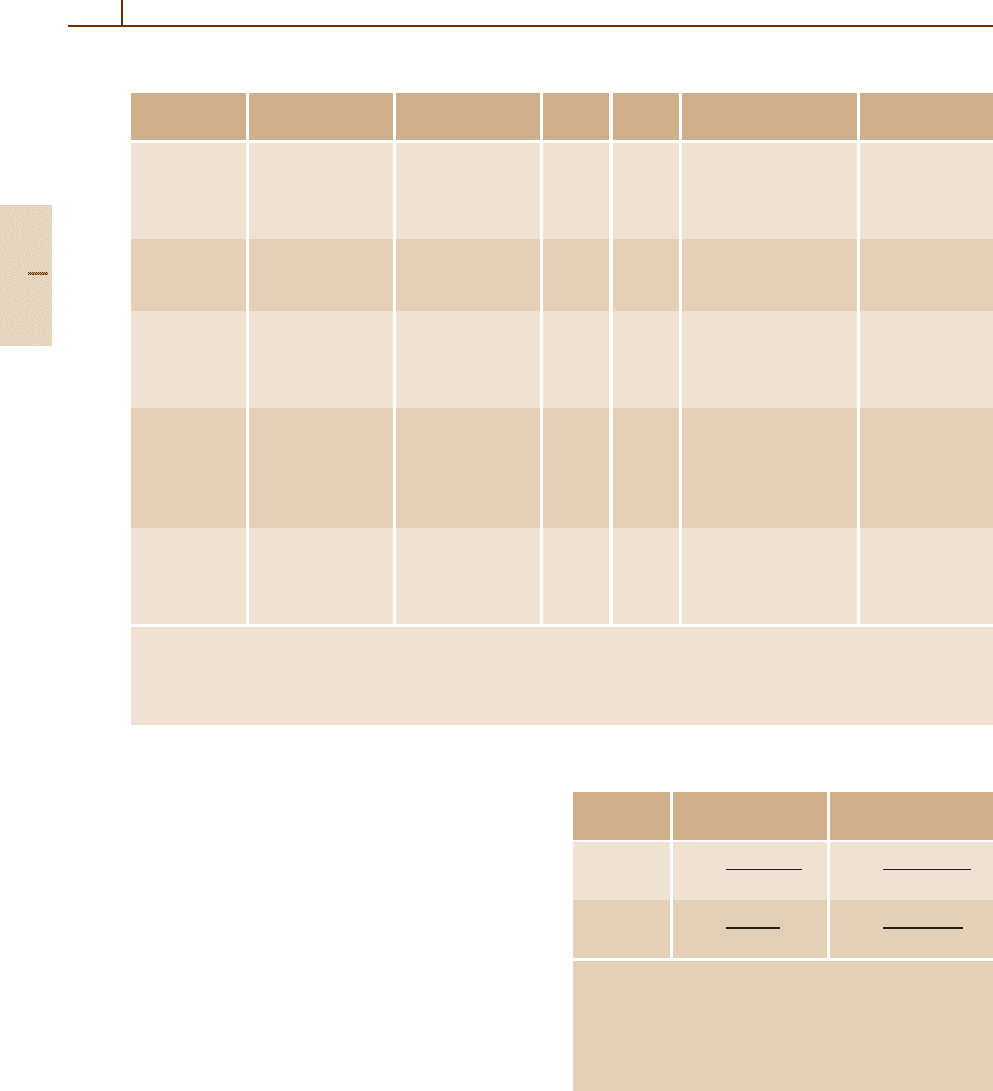
192 Part B Atoms

Table 10.5 Wavelengths λ, upper energy levels E

, statistical weights g

and g

of lower and upper levels, cont.

Spectrum λ

Accuracy

(Å) (cm

−1

) g

(10

−1

)

Tl 2767.9g 36 118 2 4 1.26 C

3519.2g 36 200 4 6 1.24 C

3775.7g 26 478 2 2 0.625 B

5350.5g 26 478 4 2 0.705 B

U 3566.6g 28 650 11 11 0.24 B

3571.6 38 338 17 15 0.13 C

3584.9g 27 887 13 15 0.18 B

V 3183.4g 31 541 6 8 2.4 C+

4111.8 26 738 10 10 1.01 B

4379.2 25 254 10 12 1.1 C

4384.7 25 112 8 10 1.1 C

Xe 1192.0g 83 890 1 3 6.2 C

1295.6g 77 186 1 3 2.5 C

1469.6g 68 046 1 3 2.8 B

4671.2 88 470 5 7 0.010 D

7119.6 92 445 7 9 0.066 D

Zn 2138.6g 46 745 1 3 7.09 B

3302.6 62 772 3 5 1.2 B

3345.0 62 777 5 7 1.7 B

6362.3 62 459 3 5 0.474 C

A “g” following the wavelength indicates that the lower level of the transition belongs to the ground term, i. e., the line is

a resonance line. Wavelengths below 2000 Å are in vacuum, and those above 2000 Å are in air

Accuracy estimates pertain to A

values: AA, uncertainty within 1%; A, within 3%; B, within 10%; C, within 25%; D, within 50%

The A

, λ, g

,andg

are multiplet values; see (10.20) and Sect. 10.16.5

tables of A, f ,andS, including forbidden lines, see

Sect. 10.21.

Experimental and theoretical methods to determine

A, f ,orS values as well as atomic lifetimes are discussed

in Chapts. 17, 18,and21.

Conversion relations between S and A

for the most

common forbidden transitions are given in Table 10.6.

Oscillator strengths f are not used for forbidden tran-

sitions, i. e., magnetic dipole (M1), electric quadrupole

(E2), etc.

[Numerical example: For the 1s2p

◦

− 1s3d

(allowed)transition in He i at 6678.15 Å: g

= 3; g

= 5;

= 6.38×10

−1

; f

= 0.711; S = 46.9 a

Table 10.6 Conversion relations between S and A

for

forbidden transitions

Numerically, in

SI units

customary units

Electric

quadrupole

16π

15h 

S A

1.1199× 10

Magnetic

dipole

16π

3h λ

S A

2.697× 10

A in s

−1

, λ in m. Electric quadrupole: S in m

. Magnetic

dipole: S in J

−2

A in s

−1

, λ in Å. S in atomic units: a

2.013× 10

−79

(electric quadrupole), e

/16π

= 8.601 × 10

−47

−2

(magnetic dipole). µ

is the

Bohr magneton

Part B 10.16