DiBenedetto E. Degenerate Parabolic Equations
Подождите немного. Документ загружается.

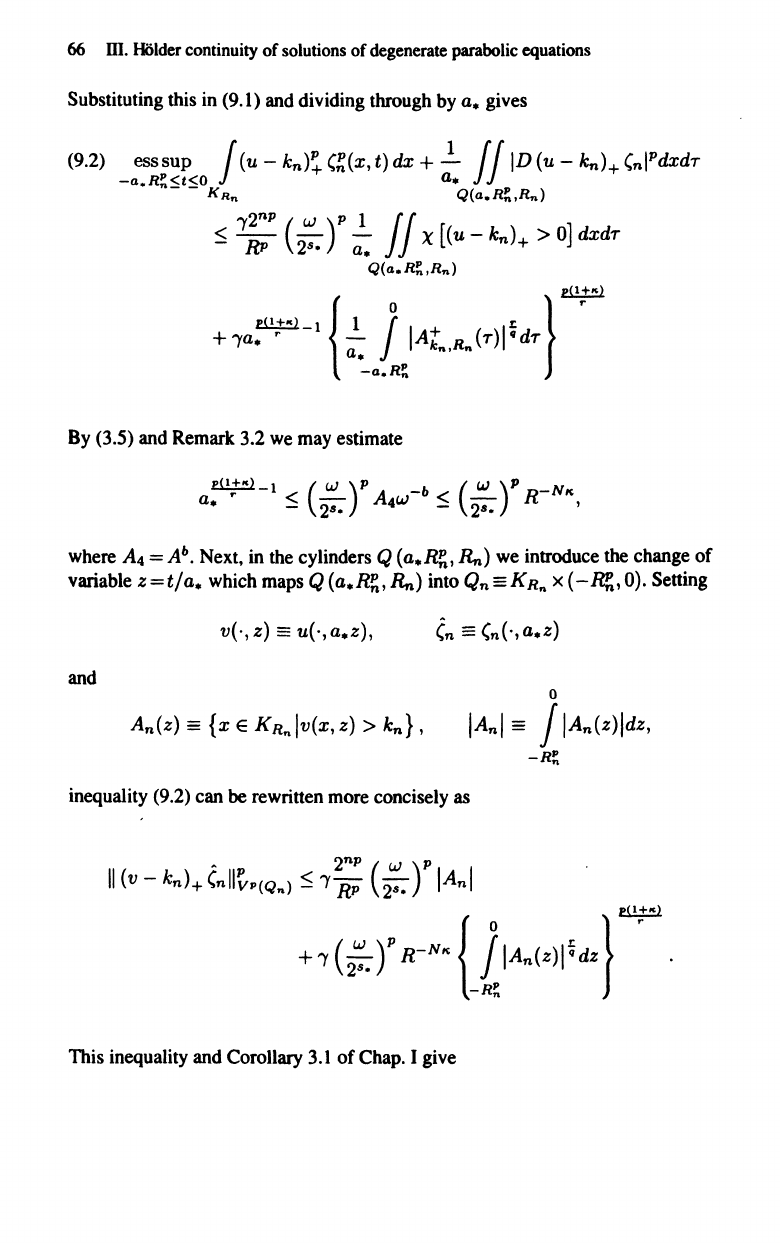
66
m.
HOlder
continuity of solutions of degenerate
parabolic
equations
Substituting this
in
(9.1)
and
dividing through
by
aoo
gives
By
(3.5)
and
Remark
3.2
we
may
estimate
a
p(1:">
-1
<
(.::!.-)P
A
w-"
<
(.::!.-)"
R-
Ntc
• - 2". 4 - 2". '
where
A4
=
A".
Next,
in the
cylinders Q
(a
..
~,
Rn)
we
introduce the change of
variable
z
=tfa
..
which
maps
Q
(a.~,
Rn) into
Qn
=KR"
X
(-~,
0). Setting
v(·,z)
=
u(·,a.z),
and
o
IAnl
= J
IAn(z)ldz,
-R~
inequality
(9.2)
can
be
rewritten
more
concisely
as
This inequality and Corollary
3.1
of
Chap.
I give

9.
The second alternative concluded 67
2-(n+2)p
(~)P
IA
I
2
8
•
n+l
Thus setting
=
(kn+l
-
knt
I(X,
Z)
E
Qn+!
I
V(X,
Z)
>
kn+!1
$
II
(v
- k
n
)+
lI~n+l
$
II
(v
- k
n
)+
(nll~n
$
'YIAn
I
NT;;
II
(V
- kn
)+
(nlltP(Qn)
< '"II
(~)P
2
np
IA
11+NT;;
- I 28 •
Rp
n
ri!.±!!l
+~
(2~'
r
R-N<IAnl*'
tlIAn(Zl!'dz}
·
we have the recursive inequalities
Y
n
+!
$
'Y
4np
{y~+m:;;
+
Yn,.,T,;
Z!+" } ,
Zn+l
$
'Y
4np
{Y
n
+
Z~+"}.
It
follows from these with the aid
of
Lemma
4.2
of
Chap. I that Y
n
and
Zn
tend to
zero as
n -
00
provided
1+"1+
..
Yo
+
Z~+"
$
'Y
-er-
4-
P
er-
==
v.,
where 0
0
=
min
{~;
It}.
The
following proposition summarises the results
of
the second alternative
and it is proved arguing as in the
proof
of
Proposition 6.1
PROPOSITION
9.1. There exists numbers v
o
,
111
E (0,1)
and
A2 » 1 depending
only upon the data
and
independent
of
w
and
R,
such that
if
for
all cylinders
of
the type
[(0,
f)
+ Q (dRP, R)]
\(x,
t) E
[(0,
f) + Q (dRP, R)]
lu(x,
t) >
f.J-+
-
2~o
\
$
(1
- v
o
)
IQ
(dRP,
R)
I,
then either
(9.3)

68
m.
UUder
continuity of solutions of degenerate
parabolic
equations
or
(9.4)
essosc u <
111
W
[Q(a.(
ft.f)]
-
where
b
is
introduced
in
(3.4).
Remark
9.1. The constants
111
E (0,1) and
A2
depend only upon the data and, in
general, also upon the
nonn
lIulloo.nT via the number
So.
If
the lower order tenn
b(x, t,
u,
Du) satisfies the structure condition
(A~)
of
§5
of
Chap. II, we have
So
= 1 and therefore
111,
A,
A2
can be detennined a priori only in tenns
of
the data
and are independent
of
lIulloo.nT'
10.
Proof of Proposition 3.1
The two alternatives just discussed can be combined to prove the main Proposition
3.1. Let us recall that
~
=
(~)P-2.
a
o
A
The concluding statement
of
the first alternative is that, starting from the cylinder
and going down to the smaller cylinder
the essential oscillation
w decreases by a factor
110
E (0,1), unless w
:5
A1R~,
where A 1 is a large constant that can be computed a priori only in tenns
of
the
data and the number
So
is introduced in (3.2). Analogously, the conclusion
of
the
second alternative is that starting from the same cylinder and going down to the
smaller box
Q(!f(ft.f)
,
the number w decreases by a factor
111
E (0, 1), unless
w:5
A2R~
, where
A2
is a
constant that can be computed a priori in tenns
of
the data.
We
combine these two
facts into
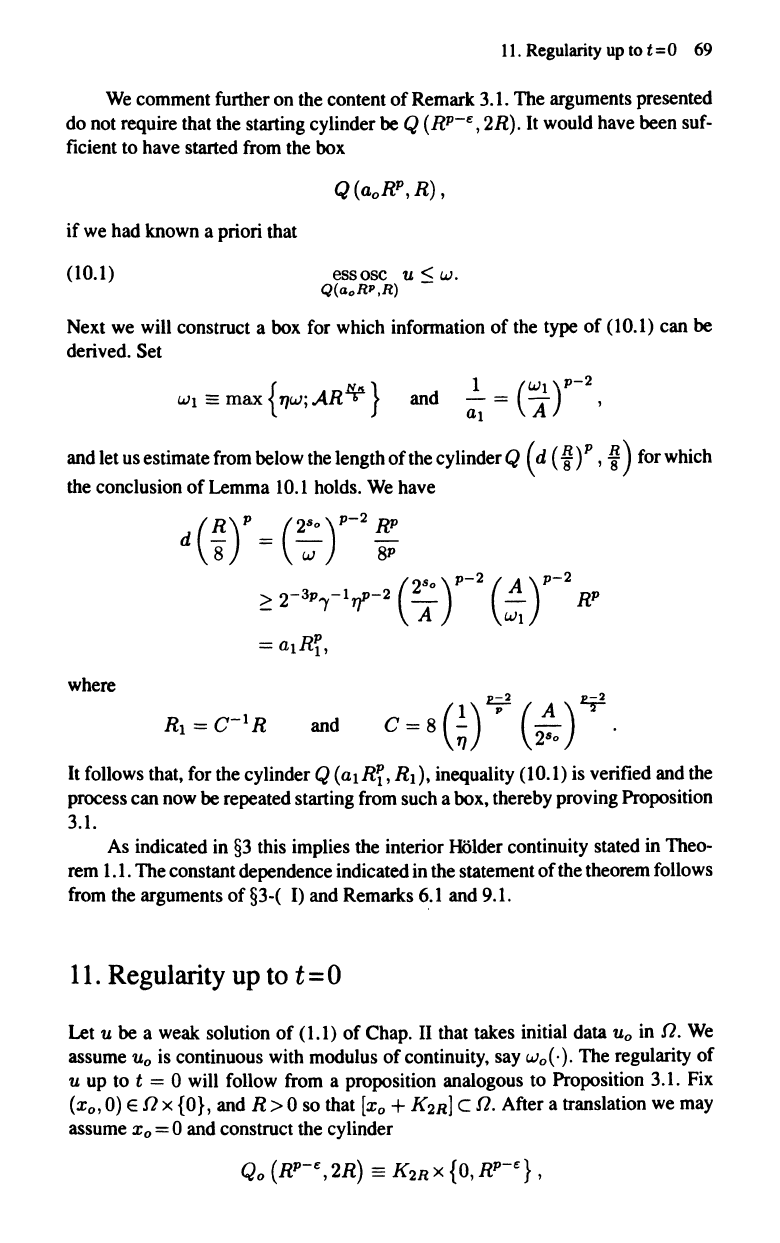
LEMMA
10.1.
There
exist constants
that
can
be
determined a priori
only
in
terms
of
the
data.
such
that
either
w:5.AR~
or
11.
Regularity
up
to
t = 0
69
We
comment further on the content
of
Remark 3.1. The arguments presented
do not require that the starting cylinder be
Q (RP-f:,
2R).
It would have been suf-
ficient to have started from the box
if
we had known a priori that
(l0.1)
essosc U <
w.
Q(aoRP,R}
-
Next we will construct a box for which information
of
the type
of
(l0.1)
can be
derived. Set
WI
==
max
{Tlw;AR~}
and
~
=
(WI)P-2,
al
A
and let us estimate from below the length
of
the cylinder Q ( d (
i)P
, i) for which
the conclusion
of
Lemma 10.1 holds.
We
have
where
and
It
follows that, for the cylinder Q (aIRf, R
I
),
inequality
(l0.1)
is verified and the
process can now
be
repeated starting from such a box, thereby proving Proposition
3.1.
As indicated in
§3
this implies the interior
mlder
continuity stated in Theo-
rem 1.1. The constant dependence indicated in the statement
of
the theorem follows
from the arguments
of
§3-( I) and Remarks 6.1 and 9.1.
11.
Regularity up to t = 0
Let u be a weak solution
of
(1.1)
of
Chap. II that takes initial data U
o
in
n.
We
assume
U
o
is continuous with modulus
of
continuity, say wo('}' The regularity
of
u up to t = 0 will follow from a proposition analogous to Proposition 3.1. Fix
(xo,
0) E n x {OJ, and R > 0 so that
[xo
+
K2RJ
c
n.
After a translation we may
assume
Xo
= 0 and construct the cylinder
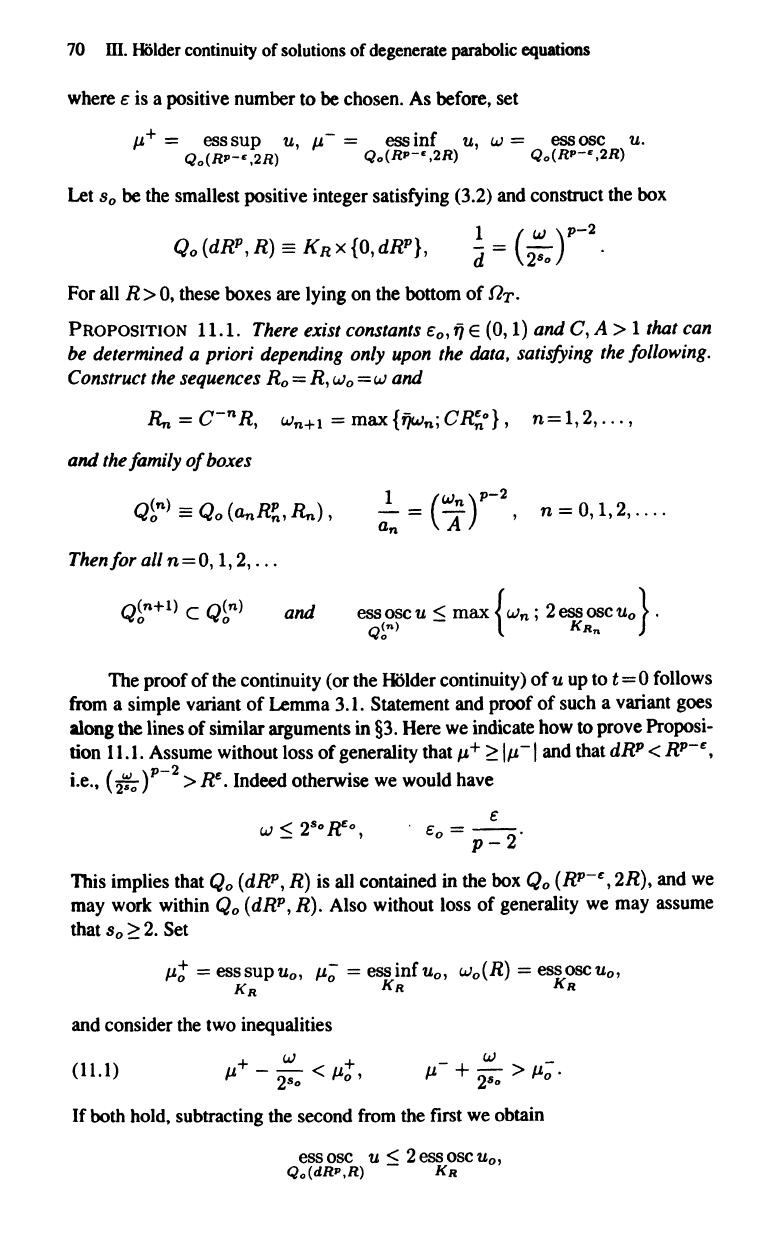
70
m.
HOlder
continuity of solutions
of
degenerate parabolic equations
where c is a positive number
to
be chosen. As before. set
p.+
= esssup u, p.- = essinf u, w = essosc u.
Qo(RP-~
,2R)
Qo(RP-<
,2R)
Qo(RP-~
,2R)
Let So be the smallest positive integer satisfying (3.2) and construct the box
For all
R > 0. these boxes are lying on the bottom
of
aT.
PROPOSITION
11.1. There exist constants
co,
ij
E (0,
1)
and
C, A > 1 that can
be
determined a priori depending only upon the data. satisfying the following.
Construct the sequences
Ro
=
R,
Wo
=W
and
Rn=C-nR,
w
n
+1=max{iiWn;CR:t},
n=I,2,
...
,
and
the family
of
boxes
~
__
(~)P-2,
an A
n = 0,1,2,
....
Thenfor all
n=O,
1,2,
...
and
essoscu:5
max
{w
n
;
2essoscu
o
}.
Q~n)
Kiln
The proof
of
the continuity (or the
HOlder
continuity)
of
u up to t = ° follows
from a simple variant
of
Lemma 3.1. Statement and proof
of
such a variant goes
along
the
lines
of
similar arguments in §3. Here we indicate how
to
prove Proposi-
tion 11.1. Assume without loss
of
generality that
p.+
~
Ip.-I and that dRP <
RP-
e
,
. (
)P-2
I.e..
2":0
>
R£.
Indeed otherwise we would have
E
.
C:
o
=
--.
p-2
This implies that Qo (dRP,
R)
is all contained in the box Qo (RP-e,
2R).
and we
may work within Qo
(dRP,
R).
Also without loss
of
generality we may assume
that
So
~
2. Set
and consider the two inequalities
(11.1)
+ w +
p.
- 2
80
<
P.o
,
_ w _
p.
+ 2
80
>
P.o
•
If both hold, subtracting the second from the first we obtain

11.
Regularity up to t
=0
71
and there is nothing
to
prove.
Let
us
assume, for example, that
the
second of (11.1)
is
violated. Then for all 8
~
8
0
,
the
levels
_ w
k =
Jl.
+ 2
8
'
satisfy
the
second of (4.16) of
Chap.
II.
Therefore
we
may
derive energy
and
log-
arithmic estimates for the truncated functions
(u - k) _. These take
the
form
(11.2) sup
}(u
-
k):(x,
t)(Pdx + f f
ID(u
-
k)-(r
dxdT
O<t<dRP
J J
- -
KR
Qo(dRP,R)
.ei!±cl
$1'
II(U-k)"-'D('PdxdT+1'{jiBk.R(T)'~dT}
r ,
Qo(dRP,R)
0
(11.3) sup
1!li2(Dk",(u-k)-,c)(P(x)dx
O<t<dRp
- -
KR
$
l'
II
!lil!li
u
(Dk",
(u - k)_,c) 1
2
-
P
I
D
(I
P
dxdT
Qo(dRP,R)
+;
(Hln
~')
{fBk.n(fll'df
('"' ,
where
Dt
and
Bt,R
are
defined
as
in
(4.2) and (4.4) of
Chap.
II.
The
proof can
now
be
completed
as
follows. First
by
using the logarithmic estimates (11.3) and
proceeding
as
in
Lemma 5.1, given
any
eo
E (0,
1)
we
can find positive numbers
to
and A
o
,
depending only
upon
the data, such that either
(11.4)
(b
defined
in
(3.4»
or,
for
all
t E
(0,
dRP),
Ix
E
KR/21
u(x, t) < Jl.- +
2~0
I < e
o
IKR/21·
Second, using
the
energy inequalities (11.2) and
the
procedure of
Lemma
6.1,
we
conclude that if (11.4) does not hold, then
(11.5)
.nf W
esSl
u > Jl.- +
--.
Qo(d(fY,f)
2
1
0+1
Changing
the
sign of (11.5) and adding ess
sUPQo(
d(
f
Y,
f)
u
to
the
left hand
side
and
Jl.+
to
the
right hand side
we
obtain
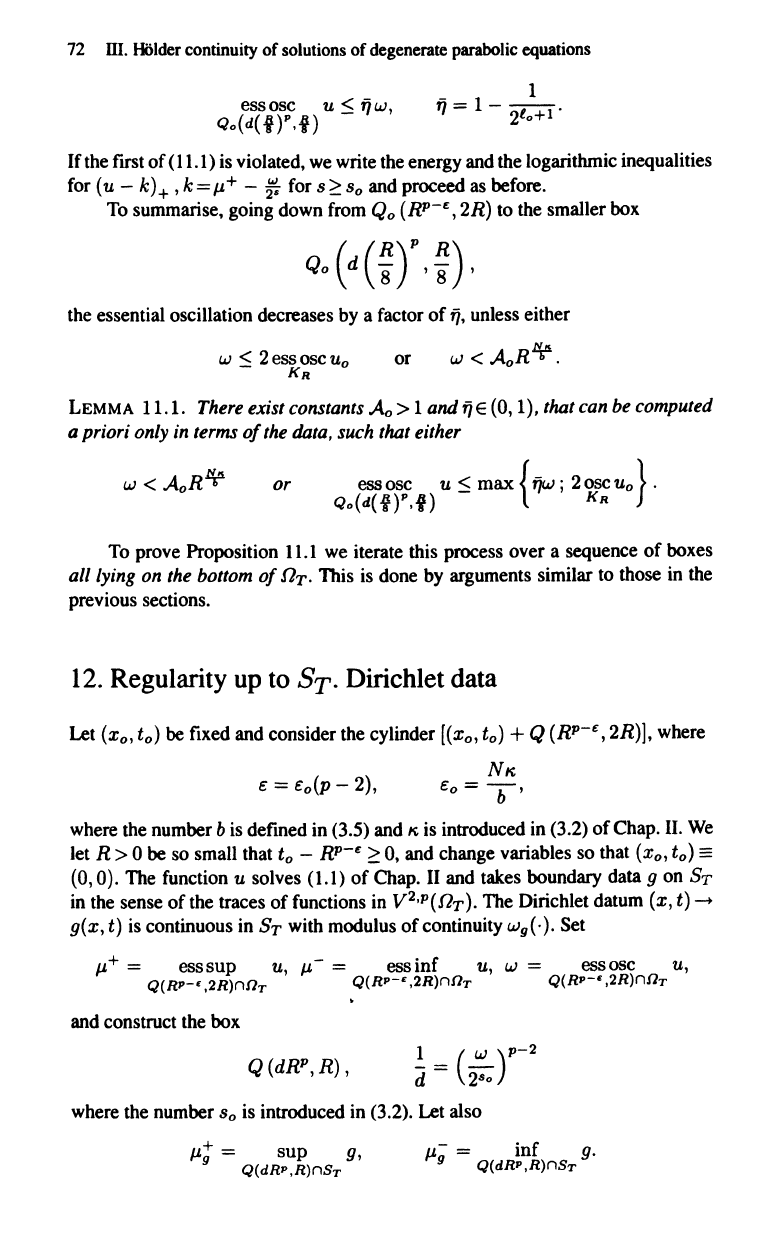
72
ill.
ltilder
continuity
of
solutions
of
degenerate parabolic equations
1
ij
= 1 - 2
1
0+1
.
If
the
frrst
of (11.1)
is
violated,
we
write
the
energy
and
the logarithmic inequalities
for
(u -
k)+,
k=JI.+ -
~
for
s~so
and
proceed
as
before.
To
summarise, going down
from
Qo
(RP-E,
2R)
to
the
smaller
box
the
essential oscillation decreases
by
a factor of ij, unless either
W <
2essoscu
o
-
KR
or
W <
AoR~.
LEMMA
11.1.
There
exist
constants
Ao > 1 and
ijE
(0,1),
that
can
be
computed
a priori
only
in
terms
of
the
data,
such
that
either
or
To
prove Proposition
11.1
we
iterate this process over a sequence of boxes
all
lying
on
the
bottom
of
n
T
•
This
is
done
by
arguments similar
to
those
in the
previous sections.
12. Regularity up to
ST.
Dirichlet data
Let (x
o
,
to)
be
fixed
and
consider
the
cylinder
[(xo,
to)
+ Q (RP-t, 2R)], where
c =
co(P
- 2),
where the number b
is
defined
in
(3.5) and
It
is
introduced
in
(3.2) of Chap. II.
We
let
R>
0
be
so
small that
to
-
RP-E
~
0,
and
change variables
so
that (xo,
to)
==
(0,0).
The function u solves (1.1) of Chap. II and takes boundary data 9
on
ST
in the
sense of
the
traces of functions
in
V
2
,p(nT).
The Dirichlet datum (x, t)--+
g(x, t)
is
continuous
in
ST
with modulus of continuity w
g
(·).
Set
JI.+
= esssup
u,
JI.
= essinf u, W = essosc
u,
Q(RP-<
,2R)nJJT
Q(RP-'
,2R)nJJT
Q(RP-'
,2R)nJJT
and
construct the
box
Q(dRP,R) ,
where the number
So
is
introduced
in
(3.2). Let also
JI.;
= sup g,
Q(dRP,R)nST
JI.-
= inf
g.
9
Q(dRP,R)nST
12. Regularity up to Sr. Dirichlet data 73
If
the two inequalities
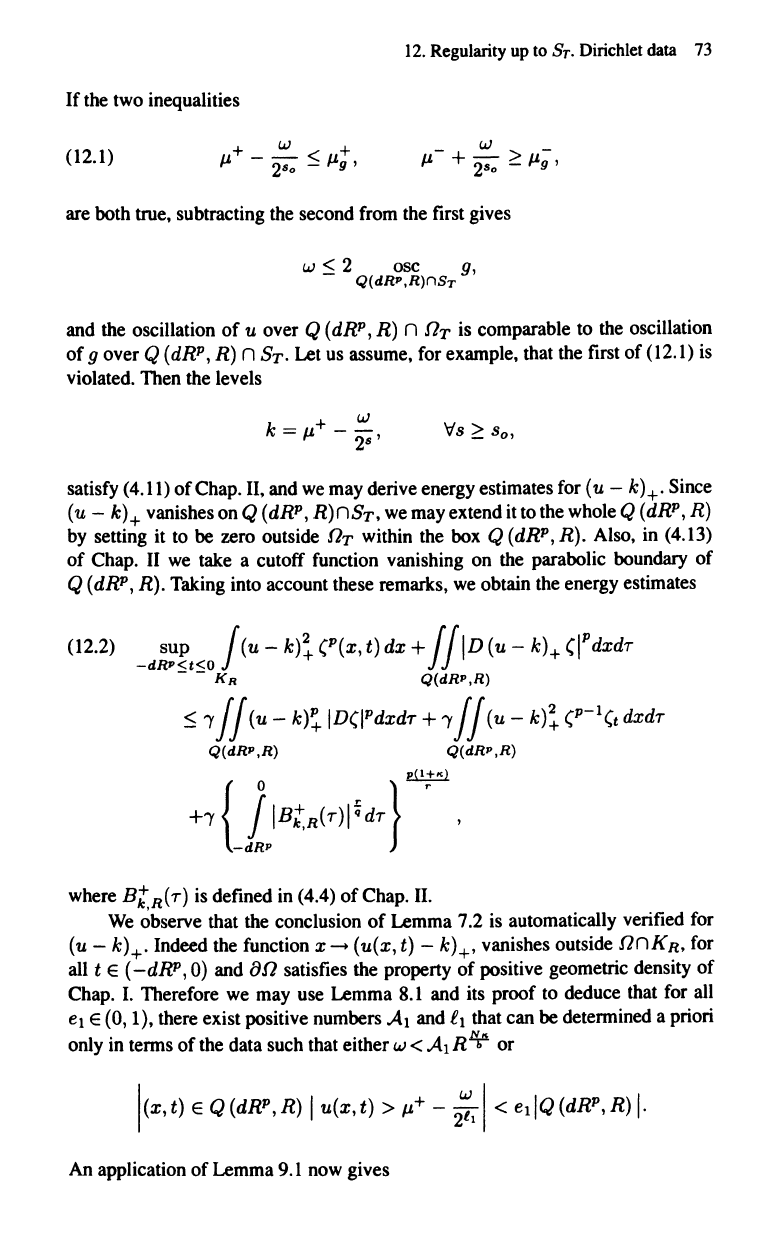
(12.1)
are both true, subtracting the second from the first gives
w
~
2 osc
g,
Q(dRP,R)nST
and the oscillation
of
u over Q
(dJtP,
R)
n
{h
is comparable to the oscillation
of
9 over Q
(dRP,
R)
n
ST.
Let us assume, for example, that the first
of
(12.1) is
violated. Then the levels
satisfy
(4.11)
of
Chap. II, and we may derive energy estimates for
(u
- k)+. Since
(u
-
k)+
vanishes on Q
(dJtP,
R)nST,
wemayextendittothe
wholeQ
(dJtP,
R)
by setting it to be zero outside
[h
within the box Q
(dRP,
R). Also, in (4.13)
of
Chap.
II
we take a cutoff function vanishing on the parabolic boundary
of
Q
(dRP,
R). Taking into account these remarks, we obtain the energy estimates
(12.2)
sup
j(U-k)!(P(x,t)dx+jrrID(u-k)+(IPdxdr
-dRP<t<O
J
- -
KR
Q(dRP,R)
~
'Y
j
j(u
-
k)~
ID(IPdxdr +
'Y
jj(u
-
k)!
(p-l(t
dxdr
Q(dRP,R)
Q(dRP,R)
~
-M
Ll.IBt.R(T)I'dT}
. ,
where Bt,R(r) is defined in (4.4)
of
Chap. II.
We
observe that the conclusion
of
Lemma 7.2 is automatically verified for
(u - k)+. Indeed the function x
-+
(u(x, t) - k)+, vanishes outside
nnKR,
for
all
t E
(-dRP,
0)
and
an
satisfies the property
of
positive geometric density
of
Chap. I. Therefore we may use Lemma
8.1
and its proof to deduce that for all
el E (0,1), there exist positive numbers
Al
and
il
that can be detennined a priori
only in tenns
of
the data such that either w <
Al
RItf
or
I(X,
t) E Q
(dRP,
R) 1
u(x,
t) >
f.L+
-
2~1
I <
ellQ
(dRP,
R)
I·
An application
of
Lemma 9.1 now gives

74
m.
HOlder
continuity
of
solutions
of
degenerate
parabolic
equations
LEMMA
12.1.
There
exist numbers
Al
> 1 and
ijE
(0, 1) that
can
be
computed
a priori only
in
terms
of
the
data
such
that either
w < AIRl!f- or essosc
u:5
max
{ijw;
osc
g}.
Q(d(
~)",~)
Q(dRP,R)nST
The proof of the theorem can
now
be
completed
by
stating a proposition sim-
ilar to Proposition
11.1.
13. Regularity at ST. Variational data
First
we
remark that the proof of interior regularity
is
only based
on
the energy
and logarithmic estimates
of
§3
of
Chap. II. In particular
if
such estimates were
available for some locally bounded function
U E
vt:':(
nT), then the conclusion
of
Theorem
1.1
would hold for u, irrespective of the differential equation u might
satisfy.
Keeping this in mind, one realises that the proof of Theorem
1.3
is
the same
as
that
of
interior
HOlder
continuity, owing to the energy
and
logarithmic inequalities
of
Proposition
4.1
of
Chap.
II.
If (x
o
,
to)
E
ST
is
fixed, after a translation to (x
o
,
to)
==
(0,0) and a local
flattening
of
an,
inequalities (4.6) and (4.7)
of
§4
of
Chap. II, can
be
viewed
as
written over cylinders
of
the type Q+(8,
p),
defined
in
(4.8) of Chap.
II.
The cutoff function x --+
(x,
t) vanishes on the boundary of
Kp
and not
on
the boundary of
K"t.
This affects the proof only in the application
of
the embed-
ding Corollary
3.1
of
Chap.
I.
Such
an
embedding
was
applied, after rescaling, to
functions
v E V"(Qn), where
Qn
==
KR.,.
x
{-~,
O}
(see Lemmas 4.1,6.1,8.1).
Now
for these domains, the ratio T
Ilnl,,/N
is
a constant depending only upon the
dimension
N.
We
also remark that the application of Lemma 2.2
of
Chap.
I,
in
the context
of
half cubes
K"t,
is
possible since such a lemma holds for convex domains (see
Remark 2.2 of Chap.
I).
14. Remarks
on
stability
As
p'\.
2 the equation becomes less degenerate. The proof presented in the previ-
ous sections shows that
"Y
and Q are stable in the sense that
lim
"Y(p)
=
"Y(2)
<
00
and lim
Q(p)
=
Q(2)
E (0,1).
",2
",2
Thus the classical results
of
HOlder
continuity of weak solutions of quasilinear
non-degenerate parabolic equations can be recovered from our results
by
letting
p'\. 2 in the structure conditions
of
§ I of Chap.
II.
15.
Bibliographical notes
75
14-(i).
Continuous
dependence
on
the
operator
A similar stability holds for the local behaviour
of
solutions
of
a family
of
equa-
tions
of
the type
of
(1.1)
of
Chap. II. To be specific, let us consider as an example
the family
of
equations
8 .
lh
u>.
- dIva>. (x,
t,
u>.,
Du>.)
=
b>.
(x,
t,
u>.,
Du>.)
u>.
E
C'oe
(0,
Ti
L~oc(n))nLfoe
(0,
Ti
wl~:(n»)
,
where A ranges over some subset I
of
the real numbers. Assume that for all A E
I,
the functions
satisfy the structure conditions
(At}-(A5)
uniformly in
A,
i.e., for constants Ci
and functions
!Pi,
i = 0,
I,
2, independent
of
A.
Assume moreover that
uniformly in
A.
Then
LEMMA 14.1.
{u>.}
is a family
of
uniformly
HOlder
continuous functions over
compact subsets
of
nT.
Results
of
this kind are referred to in the literature as continuous dependence
of
the solution on the operator. Stability results also hold for a family
of
equations
where also the parameter p ranges over a compact subset
of
[2,00).
15.
Bibliographical
notes
Questions regarding the local behaviour
of
solutions
of
equations
of
the type
of
the
p-Iaplacian
were raised
by
Ladyzenkaja-Solonnikov-Ural'tzeva [67],
Aronson-Serrin [7] and Trudinger [97]. The first results for the elliptic case ap-
pear in Ural'tzeva [100] and Uhlenbeck [99] and, for the parabolic case, in [39].
These results hold also for systems and we will comment further on them in
Chap. VllI. The proof presented here is taken from [36,37]. The structure con-
ditions
(At}-(A5)
are optimal for Theorems 1.1-1.3
to
hold, as pointed out in
[67] in the non-degenerate case p = 2. The iteration technique
of
Lemma 4.1 is
a parabolic version
of
a similar elliptic technique due to DeGiorgi [33]. The new
input regards the
space-time
geometry intrinsically defined by the solution itself.
A fIrst version
of
this technique appears in [38] in a simpler situation. It turns out
that the same idea can be used to establish the local
HOlder
continuity
of
solutions
of
the porous medium equations and its generalisations. Here we mention the con-
tributions
of
[37] and [24]. It can also be used
to
prove the local
HOlder
continuity
