DiBenedetto E. Degenerate Parabolic Equations
Подождите немного. Документ загружается.


36
II.
Weak
solutions
and
local energy
estimates
(4.14) sup
1!li
2
(nt,
(u -
k)±,
c)
(x, t)(P(x)dx
to-9<t<to
[zo+Kp]nn
:5
!
!li
2
(nt,(u-k)±,c)
(x,to-O)(P(x)dx
[zo+Kp]nn
+')'
II
!lil!li
u
(nt,(u-k)±,c)
1
2
-
P
ln(I
P
dxdr
[(Zo,to)+Q(fJ,p)]nnT
~
+
~
(1+
In
Dn
LlIBUT)
It
dT
} ·
where
the
numbers
q,
r,
It
satisfy (3.6)-(3.7).
Local considerations
as
those
in
§3
apply
to
the
present case. In particular
the
Proposition continues
to
hold
for weak
solutions that satisfy the Dirichlet data on
a portion of
ST.
4-(iii). Initial data
Consider a
weak
solution of (1.1) that takes
the
initial datum U
o
in the
sense that
h
(4.15)
k!
u(·,
r)dr
-+
U
o
in
L~oc(fl)
as
h-+O.
o
Thus u could be a solution of either
the
Dirichlet problem (2.1) or the
Neumann
problem (2.1). In either case the assumption
(U
o
)
is
in
force.
Fix
(x
o
, to)
E
flT
and consider the cylinder
[(xo,
to) + Q (0,
p)]
where
0
is
such that to-O=O. Therefore
[(x
o
, to)
+ Q
(0,
p)]lies on
the
bottom
of
the cylin-
drical domain
fl
T
•
Consider a cutoff function ( satisfying (3.1) and
in
addition
(
is
independent of E
(0,
to).
Local energy estimates
for
u near t = ° are derived
by
taking
in
the
weak
formu-
lation (1.5) testing functions
"':
= ±(Uh - k)±(P,
integrating over (0, t), t E (0, to)' and letting h-+O.
The
fllSt
term
in
(1.5) gives
~
!(u
h
-
k)l(x,t)(Pdx
-
~
!(U
h
-
k)l(x,O)(Pdx.
~+K~ ~+K~
If k
is
chosen so that
k~sUP[zo+Kp]
u
o
,
then
in
view
of (4.15)
we
have

4.
Energy
estimates
near the
boundary
37
j
(Uh(X,
0)
-
k)!
<Pdx
-+
0
as
h -
o.
[xo+Kp]
Also
from
the
definition (3.12) of
the
function
lli(·).
it
follows
that
lli
(Dt,
(u - k)±,
c)
= 0 whenever (u - k)± =
o.
j
lli
2
(Dt,
(Uh
-
k)+,
c)
(x,O)<P(x)dx
-+
0
as
h -
O.
[xo+Kp]
Analogous
considerations
hold
for
(Uh
-
k)
_
<p.
We
summarise
PROPOSITION
4.3. There exist constants
'Y
and
6
0
that can be determined a pri-
ori only in terms
of
the data such that for every
(xo,
to)
E
{h,for
every cylinder
[(xo,
to)
+ Q
(8,
p)]
such that t
o
-8
= 0
andfor
every level k satisfying (4.2)for
6 $ 6
0
and in addition
(4.16)
{k
~
sUP[xo+Kp] U
o
k $
inf[xo+K
p
]
U
o
the following inequalities hold:
for the function
(u -
k)+
for the function
(u
-
k)
_,
(4.17) sup
j(u
-
k)~(x,
t)<P(x)
dx +
jr
flD(u -
k)±<IPdxdT
to-9<t<to
J
[xo+Kp]
[(xo,t
o
)+Q(9,p)]
~
$
'Y
j j (u -
k)'±ID<IPdxdT
+
'Y
{JIB~/T)lidT}
r
[(x
o
,t
o
)+Q(9,p)]nnT
0
Moreover
(4.18) sup
jlli2
(Dt,(u-
k)±,c) (x,t)<P(x)dx
to-9<t<to
[xo+Kp]nn
+
'Y
j j
llillliu
(Dt,
(u
- k)±, c) 1
2
-
P
ID<I
P
dxdT
[(X
o
,t
o
)+Q(9,p)]
li!±.!tl
~
J
(1+
In
D!)
tl.IB~)T)lidT}
·
where the numbers q, r,
K,
satisfy (3.6)-(3.7).
Remark
4.3.
Local
considerations
apply
to
this
case
along
the
lines
of similar
remarks
in
the
previous
sections.

38
D.
Weak
solutions
and
local
energy
estimates
Remark
4.4. The constant
'Y
on the right hand sides
of
either (4.13)-(4.14)
or
(4.17)-(4.18) is independent
olu.
It is only the levels k that might depend upon
the solution u via (3.11). Moreover
if
"Pi
==
0, i = 0, 1, 2, and C
2
= 0, the levels k
are independent
of
u.
Remark
4.5. We conclude this section by observing that all the energy estimates
as well as logarithmic estimates for ( u -
k)
+ hold true
if
merely u is a subsolution
of
(1.
1)
and for (u - k)_
if
u is a supersolution
of
(1. 1).
5. Restricted structures: the levels k and the constant
'Y
We
will make a few remarks on the dependence
of
the constant
'Y
in the energy
and logarithmic estimates and on the restrictions to be placed on the levels
k.
5-W. About the constant
'Y
For the interior estimates
of
Propositions 3.1 and 3.2, the constant
'Y
depends only
upon the data and it is independent
of
the apriori knowledge
of
lIulloo,nT.
It can
be calculated apriori only in terms
of
the numbers N, p,
r,
It,
the constants
Ci,
i =
0,
1,2,
and the norms
lI"Po,
"Pr
,
"P2114,r;
nT·
1be
same dependence holds for estimates near the parabolic boundary
of
n
T
in
the case
of
Dirichlet data (see §4-(ii) and §4-(iii».
In the case
of
variational data,
'Y
depends also upon the structure
of
an (see
§
1,
Chap. I), and the norms
5-(;;). Restricted structures
The choices (3.11) and (4.2)
of
6
0
impose a restriction on the levels
k.
Such a re-
striction is needed to handle the lower order terms
b(
x, t,
u,
Du) in (1.1).
It
follows
from (3.10) and (3.10)' that the choice (3.11)
of
6
0
permits the absorption
of
the
term
C2
jjlD(U - k):l:I"(u -
k):l:C"dxdr
:5
6
0
C2
j
jID(U
- k):l:I"C"dxdr
~
q
into the tenns generated by the principal part
of
the operator in (1.1). Also. the
coefficient
of
the integral involving "P2 depends only upon the data (i.e.,
Co,
C
2
),
if
the levels k are chosen according
to
(3.11).

5.
Restricted structures: the levels k and the constant
'Y
39
Such a choice of
~o
impose~
on k
to
be
close
to
either the supremum or the
infimum
ofuin
Q(6, p). Thus,
in
particular, the apriori knowledge of
li
u
lioo,Q(8,p),
is
required.
We
will introduce structure conditions on (1.1) that yield energy
and
logarith-
mic
estimates analogous
to
(3.8) and (3.14) for the truncated functions (u - k)±.
with
no
restriction on the levels k.
We
will limit ourselves
to
the
interior estimates
of Propositions
3.1
and 3.2.
First, it
is
obvious
from
the
remarks above that Propositions
3.1
and
3.2
con-
tinue
to
hold for all the levels k if b(x, t, u, Du)
==
O.
A
more
general condition
is
(A
3
)
where
(A~)
and
q,
r satisfy (As) and
(As-i)-(As-iii).
The structure condition (A3) implies
(A3)' The
source
term
'{J2
is
required
to
be
more
integrable than the corresponding
source tenn
in
(A3).
Let
us
consider local weak solutions u
of
(1.1)
with
the
structure conditions
(Ad,
(A2), (A
3
),
(A4),
(A~),
(As) and
(As-i)-(As-iii).
We
do
not
require
that u
be locally bounded.
To
derive local energy and logarithmic estimates
for
u
we
proceed
as
in the proof of Propositions
3.1
and 3.2. The lower order tenns
in
(3.10) are
now
estimated
by
repeated use of the Young's inequality
as
follows.
!
!lb(X,
r, u, Du)(u - k)±("ldxdr
Q&
~
~o
!
!ID(U
- k)±(I"dxdr +
'Y
!!
'{J2(U
-
k)±("dxdr
Q& Q&
+
'Y
!
!(u
-
k)~
max {ID(I" ;
("}
dxdr.
Qt
We
conclude that, for such solutions, inequalities (3.8)-(3.14) hold true
for
every
level k,
with
a constant
'Y
independent
of
u, provided the integral
!
j(U
-
k)~ID(I"dxdr
[(x
o
,t
o
)+Q(8.p)]
in (3.8)
is
replaced
by
and the integral
j
j(U
-
k)~
max {ID(I"j
("}
dxdr
[(x
o
,t
o
)+Q(8,p)]
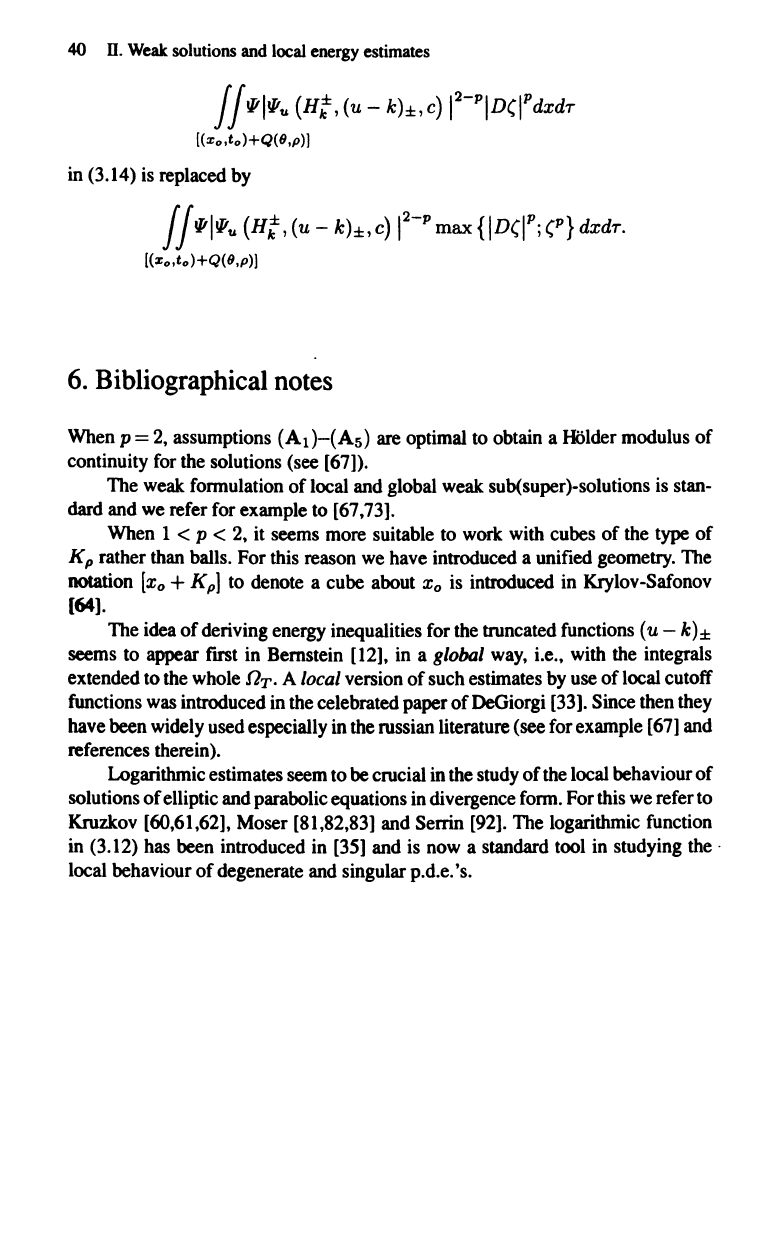
40
II. Weak solutions and local energy estimates
J J
!lil!li
u
(Ht,
(u - k)±,
c)
1
2
-"ID(I"
dxdr
[(xo,t
o
)+Q(9,p))
in
(3.14)
is
replaced
by
J J
!lil!li
u
(Ht,
(u - k)±, c) 1
2
-"
max
{ID(I";
("}
dxdr.
[(x
o
,t
o
)+Q(9,p))
6. Bibliographical notes
When p =
2,
assumptions (A
d-(
As)
are
optimal
to
obtain a
HOlder
modulus of
continuity
for
the solutions (see [67]).
The
weak
fonnulation of local and global
weak
sub(super)-solutions
is
stan-
dard and
we
refer for example
to
[67,73].
When 1
< p <
2,
it seems
more
suitable
to
work
with cubes of the type of
K p rather
than
balls. For this reason
we
have introduced a unified
geometry.
The
notation
[xo
+
Kp]
to denote a cube about
Xo
is
introduced
in
Krylov-Safonov
(64).
The
idea of deriving energy inequalities
for
the truncated functions (u -
k)
±
seems to appear fust
in
Bernstein
(12),
in
a global
way,
i.e., with the integrals
extended
to
the whole n
T
•
A local version of such estimates
by
use
of local cutoff
functions
was
introduced
in
the celebrated paper
of
DeGiorgi
(33).
Since then they
have been widely used
eSJ)C':ially
in
the russian literature (see for example
(67)
and
references therein).
Logarithmic estimates
seem
to
be
crucial
in
the study of
the
local behaviour of
solutions of elliptic and parabolic equations
in
divergence fonn. For this
we
refer
to
Kruzkov [60,61,62), Moser [81,82,83] and Senin
(92).
The logarithmic function
in (3.12)
has
been
introduced
in
(35)
and
is
now
a standard tool
in
studying
the
-
local behaviour of degenerate
and
singular p.d.e. 's.

III
Holder
continuity
of solutions
of
degenerate
parabolic
equations
1.
The regularity theorem
Consider solutions u
of
(1.1) or
of
Chap. II for the case P >
2.
The equation
is
degenerate since the modulus
of
ellipticity vanishes when I
Dul
=
O.
We
will prove
that if
u E
L~(nT),
then it
is
HOlder
continuous within its domain of defini-
tion. It will shown in Chap. V that local weak solutions
of
such degenerate equa-
tions are indeed locally bounded.
To
simplify the presentation
we
will assume that
u E L
00
(nT
).
If
u
is
only locally bounded, it will suffice to
work:
within a fixed
compact subset
of
nT.
In
the theorems below, the statement that a constant
'Y
de-
pends upon the data means that it can
be
determined a priori only
in
terms
of
the
norm
lIulloo,oT'
the constants C
i
,
i=O,
1,2, and the norms
IIr,oo,
r,or,
r,02l1ii,r;OT
appearing in the structure conditions (A 1
)-(
A3)'
We
let
/C
denote a compact sub-
set
of
n
T
and let p - dist
(/C
j
r)
be the intrinsic parabolic distance from
/C
to
the
parabolic boundary
of
nT, i.e.,
(1.1)
(
~
)
p - dist
(/Cj
rjp)
==
inf
Ix
-
yl
+
II
ull
00"
0
It
- sll/p .
(.,.t)elC
' T
(1I
••
)er
l-(i). Interior
HOlder
continuity
THEOREM
1.1. Let u
be
a bounded local weak solution
of
(1.1)
of
Chap. 1/ in
n
T
.
Then (x, t) -
u(x,
t) is locally Holder continuous in nT. Moreover there

42
m.
mlder
continuity
of
solutions of degenerate parabolic equations
for every pair
of
points (x},
h),
(X2'
t2)
EK-.lfthelowerordertermsb(x, t, u, Du)
satisfy
(A~)
of§5
of
Chap.
II.
then
'Y
and a
are
independent ofllulloo.UT'
1-(ii). Boundary regularity (Dirichlet data)
THEOREM
1.2. Let u
be
a bounded
weak
solution
of
the
Dirichlet
problem
(2,/ )
of
Chap.
II
and
let
(D) and
(U
o
)
hold.
The
boundary
an
is
assumed
to
satisfy
the
property
of
positive geometric density
(1./)
of
Chap.
I.
Then
uEC
caT)'
and
there
exists a continuous positive non-decreasing function 8
-+
W(
8)
: R +
-+
R + •
such
that
IU(XI'
tl)
-
U(X2'
t2)1
:5
W
(ixi
-
x21
+
It
I -
t2li)
,
for every pair
of
points (Xl,
tl),
(X2'
t2)
E
nT.ln
particular if
the
boundary
datum
9
is
Holder
continuous
in
ST
with
exponent say a
g
•
and if
the
initial
datum
U
o
is
Holder
continuous
in
n
with
exponent
say
Quo
•
then
u
is
Holder
continuous
in
nT
alul
there
exist constants
'Y>
1 and a E (0,
1)
such
that
lu(xt, tt} -
U(X2'
t2)1
~
'Yllulloo,u
T
~Xl
-
x21
+
lIulI~Tltl
- t211/Pr '
for every pair
of
points (Xl. tt},
(X2'
t2)
E
nT.
The
constants
'Y
and a depend only
upon
the
data.
Moreover
the
constant a
depends
also
upon
the
HOlder
exponents a
g
,
Quo
of
9 and U
o
respectively.
If
the
lower
order
terms
b(x, t,
u,
Du) satisfy
(A~)
of§5
of
Chap.
II.
then
'Y
and a
are
independent
of
lIulloo,u
T
.
Even though
we
have
stated
the
Theorem
in
a global
way
the proof has a
local
thrust. For example the boundary datum 9 could be continuous or
HOlder
continuous only on a open portion
of
ST (open
in
the
relative topology
of
ST),
say
E. Then the solution u
of
the Dirichlet problem would be continuous (respectively
HOlder
continuous)
up
to
every compact subset of E.
Analogous remarks hold
in
the
case U
o
is
only locally continuous or locally
HOlder
continuous.
In
particular
to
establish
the
continuity
(HOlder
continuity
re-
spectively) of u
up
to
nx
{O},
no
reference
is
needed
to
the
Dirichlet problem or
any
boundary value problem.

2. Preliminaries
43
1-0;;). Boundary regularity (Variational data)
To
stress such a locality
we
state our next theorem
as
if
no
information
were
avail-
able on the initial datum u
O
•
THEOREM
1.3.
Let
u be a weak solution
of
the Neumann problem (2.7)
of
Chap. II, satisfying
u E
LaO
(fi X
[E,
TJ)
,
E E (0, T).
Assume that
an
is
of
class
Cl,~
and let (N) and
(N
- i) hold. Then u is Holder
continuous in
n x
[Eb
T],for
all E <
El
<
T,
and
there exist constants
"y
and Q
such that
IU(Xb
td
-
U(X2,
t2)
I
~
'Yllulloo,iix[€,T]
(IXl -
x21
+ lIull.:rox(€,T)ltl -
t211/P)
Q ,
for
every pair
of
points
(Xl,
tl),
(X2,
t2)
E n x
[El,
T]. The constants
'Y
> 1 and
Q depend only upon
E,
lIulloo,iix(€,T]
and the data, including the structure
of
an
and the norms
IItPl,
tPrr IIq,r;UT appearing in (N - i). In addition the constant
'Y
depends upon the distance
(El
-
E).
If
the Neumann data are homogeneous, i.e., if
tPo
==
tPl
==
0,
and
if in addition
the lower order terms
b(x,
t, u,
Du)
satisfy (Aa)
of§5
of
Chap. II, then
'Y
and Q
are independentofllulloo,iix(€,T]'
Remark
1.1. The continuity of u can be claimed
up
to
t = 0 provided
(U
o
)
of
Chap.
II
holds. Also, if U
o
is
HOlder
continuous
in
n then u
is
HOlder
continuous
innT.
2.
Preliminaries
The
HOlder
continuity of u, either
in
the interior of n
T
or
at
the parabolic
boundary,
will be, heuristically, a consequence of the following
fact.
The function (x, t)
-+
u( x, t) can be modified
in
a set of measure zero
to
yield a continuous representative
out of
the
equivalence class u E
Vj!:(n
T
),
if for every
(xo,
to)
E nT there exist
a family
of
nested and shrinking cylinders
[(xo,
to)
+ Q (6
n
,
Pn)]
with
the
same
vertex such that the essential oscillation
Wn
of u
in
[(xo,
to)
+ Q
(6
n
,
Pn)]
tends
to
zero
as
n
-+
00
in
a
way
quantitatively determined
by
the structure conditions
(AD!-(A6).
The
key
idea of
the
proof
is
to
work with cylinders whose dimensions
are
suitably rescaled
to
reflect the degeneracy exhibited
by
the
equation.
To
make
this
precise,
fix
(xo,
to)
E n
T
and
construct the cylinder
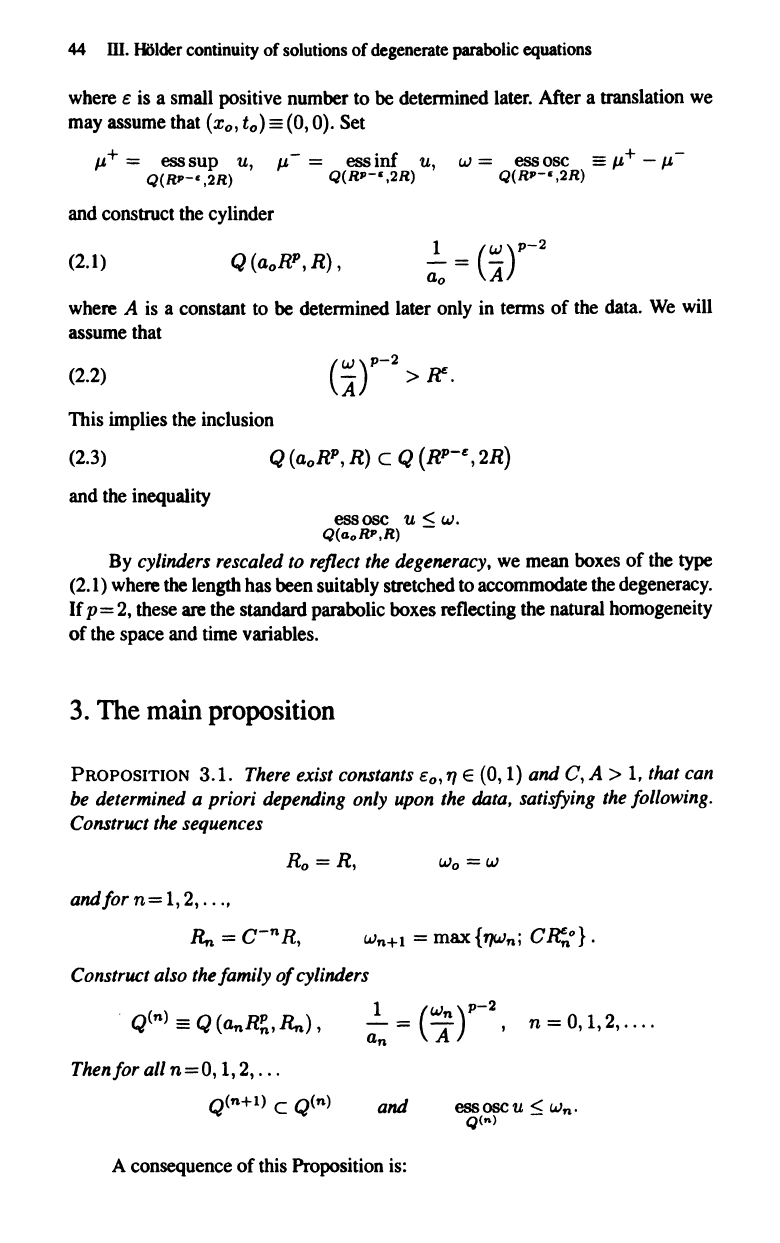
44
m.
ltilder continuity of solutions of degenerate
parabolic
equations
where E is a small positive number to
be
determined later. After a translation
we
may assume that
(xo,
to)
= (0, 0). Set
p.+ = esssup u,
Q(RP-',2R)
and construct the cylinder
p.-
= ess inf u,
Q(RP-',2R)
+ -
W=
essosc
=p.
-p.
Q(RP-e,2R)
(2.1)
.!.
=
(~)"-2
a
o
A
where A
is
a constant to
be
determined later only
in
terms
of
the data.
We
will
assume that
(2.2)
(
W)"-2
A >
[lE.
This implies the inclusion
(2.3)
and the inequality
essosc u <
w.
Q(aoRP,R)
-
By
cylinders rescaled to rejlectthe degeneracy,
we
mean boxes
of
the type
(2.1) where the length has been suitably stretched to accommodate the degeneracy.
If
p=
2,
these are the standard parabolic boxes reflecting the natural homogeneity
of
the space and time variables.
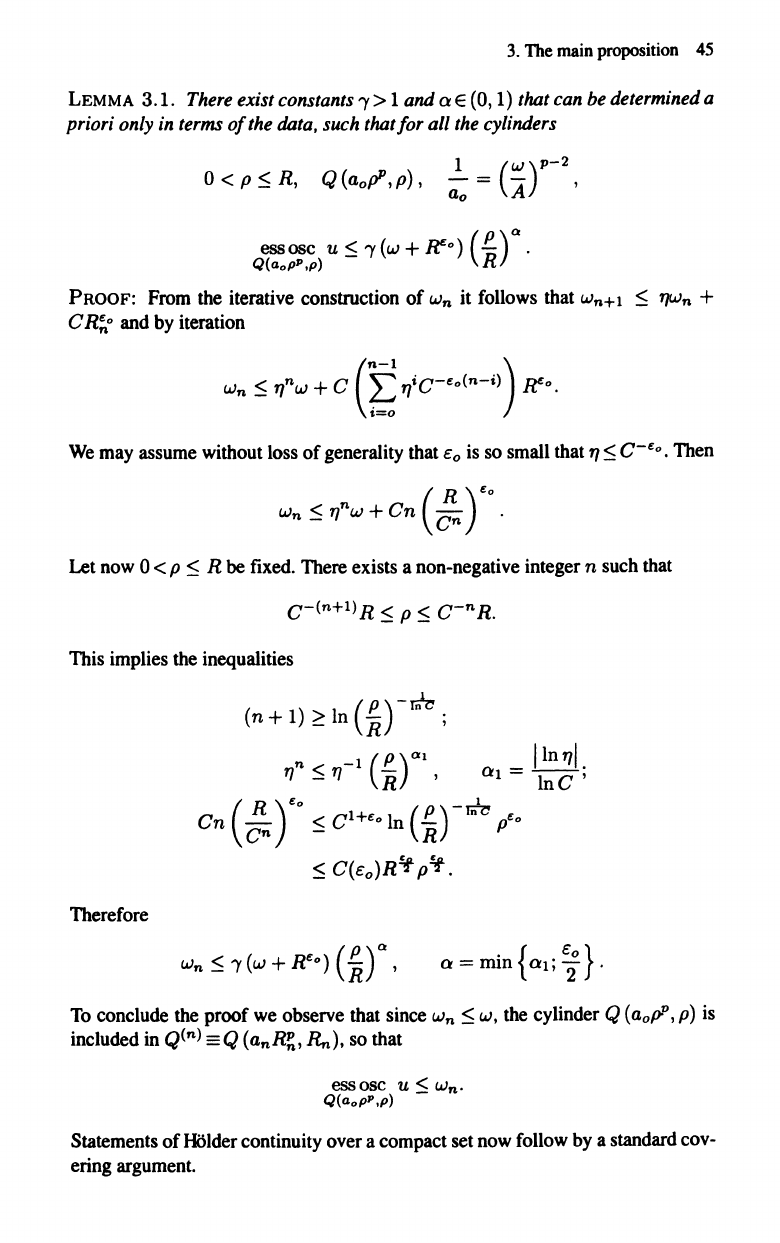
3. The main proposition
PROPOSITION
3.1. There exist constants
Eo,
" E
(0,
1)
and C, A >
1,
that can
be determined a priori depending only upon the data, satisfying the following.
Construct the sequences
Ro=R,
wo=w
andfor
n=
1,
2,
...
,
R".
=
C-nR,
Construct also the family
of
cylinders
~
__
(W
n
),,-2,
an A
n=0,1,2,
....
Thenfor all n=O, 1,2,
...
Q(n+l)
c
Q(n)
and
A consequence
of
this Proposition
is:
3.
The main proposition
45
LEMMA
3.1.
There
exist constants
'Y
> 1 and 0 E (0, 1) that
can
be
determined a
priori only
in
terms
of
the
data.
such
that for all
the
cylinders
o < p
5:
R,
Q
(aopp,
p)
,
~
=
(~)P-2,
a
o
A
essosc u
5:
'Y
(w
+
~o)
(RP)O
.
Q(aopP,p)
PROOF:
From
the
iterative construction
of
Wn
it follows that
Wn+1
5:
7JWn
+
C
R~o
and
by
iteration
We
may
assume
without loss of generality that
eo
is
so
small
that"
5:
C-Eo. Then
Let
now
0 < P
5:
R
be
fixed.
There
exists a non-negative integer n
such
that
This implies
the
inequalities
Therefore
. {
eo}
o=mm
0
1
;"2
.
To
conclude
the
proof
we
observe that since
Wn
5:
w.
the
cylinder Q
(aopp
,
p)
is
included
in
Q(n)
=Q
(an~'
Rn).
so
that
essosc u
5:
W
n
.
Q(aopp,p)
Statements of
HOlder
continuity over a compact set
now
follow
by
a standard
cov-
ering
argument.
