DiBenedetto E. Degenerate Parabolic Equations
Подождите немного. Документ загружается.


II
Weak
solutions
and
local
energy
estimates
1.
Quasilinear degenerate or singular equations
We
introduce a class
of
quasilinear parabolic equations with the principal part in
divergence fonn, that are either degenerate
or
singular due to the vanishing
of
the
gradient
IDul
of
their solutions.
(l.l)
Ut
-
div
a(x,
t,
u,
Du) = b(x, t,
u,
Du) in
1)'
(nT)'
The functions a : n
T
x R N +
1
-+
RN
and b : n
T
x
RN
+1
-+
R are only assumed
to
be
measurable and satisfying the structure conditions
a(x,t,u,Du)·Du
~
ColDul
P
-
!Po(x,t),
la(x,
t,
u,
Du)1
S C
1
1Dul
p
-
1
+
!PI
(x, t),
Ib(x,
t, u,
Du)1
s C
2
1Dui
P
+
!P2(X,
t)
for p > 1 and a.e. (x, t) E n
T
•
Here C
i
,
i = 0,
1,2,
are given positive constants
and
!Pi,
i = 0,
1,2,
are given non-negative functions, defined in n
T
and subject to
the condition
where
q,
r
~
1 satisfy
(As)
and
-'!.-
• •
p-I
E Lq,r(rl )
<Po.
!PI
.!P2
UT
1 N .
-=
+
--:::
= 1 - 1\:1.
r
pq
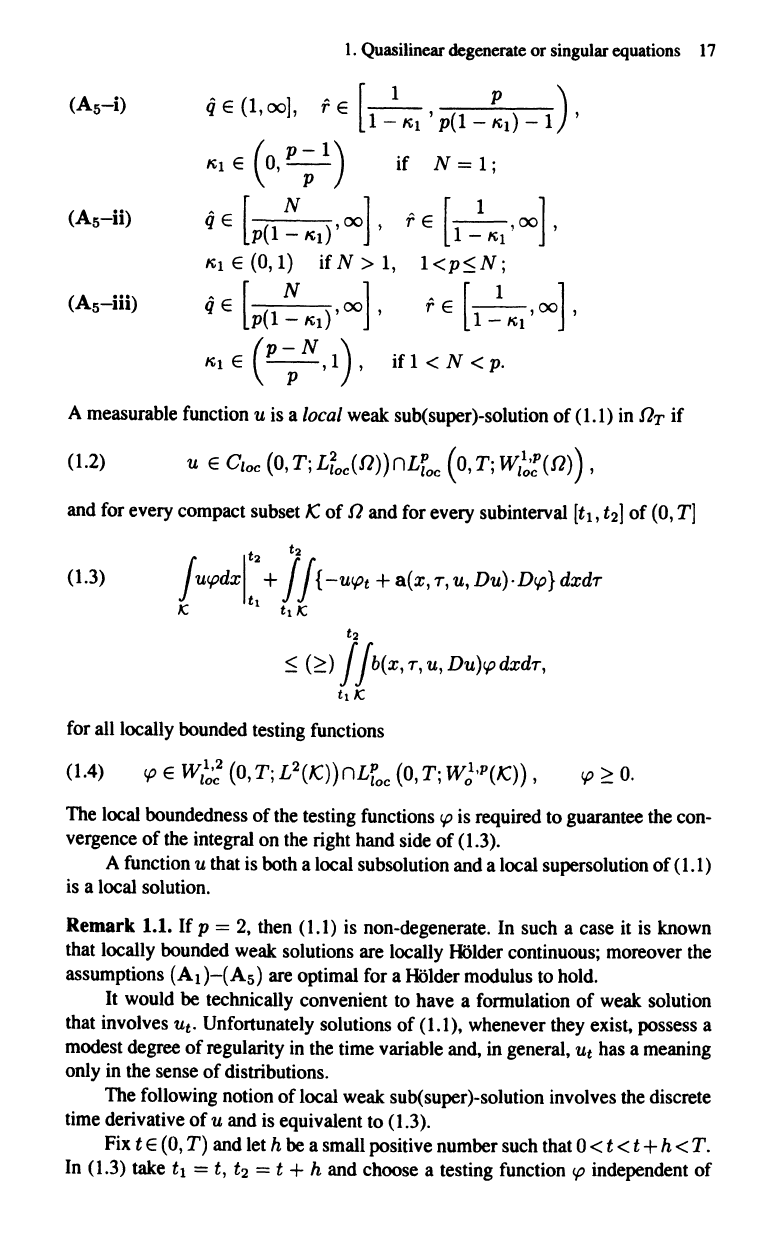
1.
Quasilinear degenerate or singular equations
17
(A5-
i
)
(A5-
ii
)
(A
5
-iii)
A measurable function u is a local weak sub(super)-solution
of
(Ll)
in n
T
if
(1.2) u E
Gloc
(0,
T;
L~oc(n»nLfoc
(0,
T;
wl~:(n))
,
and for every compact subset
JC
of
n and for every subinterval [h,
t2J
of
(0,
TJ
t t2
0.3)
fUrpdXlt2
+ f
f{
-urpt + a(x,
T,
u, Du)·Drp}
dxdT
IC
1
tllC
t2
~
(~)
f fb(x,T,
U,
Du)rpdxdT,
tllC
for all locally bounded testing functions
(1.4)
rp
~
O.
The local boundedness
of
the testing functions
rp
is required
to
guarantee the con-
vergence
of
the integral
on
the right hand side
of
(1.3).
A function u that is both a local subsolution and a local supersolution
of
(1.1)
is a local solution.
Remark
1.1.
If
p = 2, then (1.1) is non-degenerate. In such a case it is known
that locally bounded
weak:
solutions are locally
mlder
continuous; moreover the
assumptions
(AI
)-(
A5) are optimal for a
mlder
modulus to hold.
It would
be
technically convenient to have a formulation
of
weak solution
that involves
Ut.
Unfortunately solutions
of
(1.1), whenever they exist, possess a
modest degree
of
regularity in the time variable and, in general,
Ut
has a meaning
only in the sense
of
distributions.
The following notion
of
local
weak:
sub(super)-solution involves the discrete
time derivative
of
u and is equivalent to (1.3).
Fix t E (0,
T)
and let h be a small positive number such that 0 < t < t + h < T.
In (1.3) take
tl
= t,
t2
= t + h and choose a testing function
rp
independent
of

18
ll. Weak solutions and local energy estimates
the
variable T E (t, t + h). Dividing
by
h and recalling
the
definition of Steklov
averages
we
obtain
(1.5) J
{Uh,tCP
+ la(x,
T,
u,
Du)Jh
·Dcp
-Ib(x,
T,
U,
DU)]h
cp}
dx
::;;
(~)O,
K:x{t}
for
all 0 < t < T - h and
for
all
cp
E
W~,p(~)nL~c(n),
cp
~
O.
To
recover (1.3),
fix
a subinterval
O<tl
<
t2
<T,choose h so small thatt2+h
::;;
T
and
in
(1.5)
take
a testing function
as
in
(1.4). Such a choice
is
admissible, since
the testing functions
in
(1.5)
are
independent of
the
variable T E (t, t + h) but
may
be
dependent
upon
t.
Integrating over
It
I ,t2J and letting h
-+
0
with
the aid of
Lemma
3.2 of Chap. I gives (1.3).
1-(i). Subsolutions and parabolic equations
Tbe structure conditions
(AI)-(As)
are
not sufficient
to
characterize parabolic
p.d.e. 's. For example the
'principal part'
a(x, t,
u,
Du} = Du - Du/IDul
satisfies
(Ad-(As)
with
p=
2.
However its
'modulus
of
ellipticity' changes type
at
IDul =
1.
In
what follows
we
assume that (1.1)
is
weakly
parabolic
in
the sense
that it satisfies
(AI
)-(
As) and
in
addition, whenever u
is
a
weak
solution of ( 1.1),
(A6) for all k E R
the
truncated functions (u -
k)±
are
weak
subsolutions of (1.1)
in the
sense of (1.3).
with
a(x, t, u, Du) replaced
by
±a(x,
t, k ± (u -
k)±,
±D(u
-
k)±)
and
b(x, t, u, Du} replaced
by
±b(x, t, k ± (u -
kh,
±D(u
-
k)±).
To
clarify the connection between subsolutions
and
parabolic structures,
we
derive
some
sufficient conditions
on
a(x, t, u, Du)
for
(A6) to
be
verified.
Let
u
be
a local
weak
solution of (1.1), and
in
(1.5) take
the
testing function
e>O
and
cp
satisfying (1.4).
We
integrate
in
dt over
It
I ,
t2J
C
(0,
T)
and let first h
-+
0 and then e
-+
0
to
obtain

(1.6)
1.
Quasilinear
degenerate
or singular
equations
19
t2
ju+C{)(x,t)I:: + fj{-u+C{)t+a(x,t,u+,DU+).DCP} dxdr
n
tIn
t2
= f f b(x, t,
u+,
Du+)
cpdxdr
tIn
LEMMA
1.1.
Assume
that
a(x,t,u,11)·11
~
0,
Then
(1.1)
is
weakly
parabolic.
PROOF:
It suffices to verify (A6)
for
(u - k)+ and k =
0.
This
is
the
content of
(1.6).
A more general condition
for
(As)
to
hold
can
be
given
by
the
notion of
monotonicity.
We
say that
11-+a(X,
t, u,
11)
is
monotone
i£<l)
(1.7) (a(x,t,u,11d - a(x, t,u,
112),111
-
"12)
~
0,
V11i
ERN,
i=I,2.
LEMMA
1.2.
Assume
that
11-+a(x,
t, u,
11)
is
monotone
and
(1.8)
Then
(A6)
holds.
PROOF:
Write
the last integral
on
the right hand side of (1.6)
as
t2
fy
[(a(X,t,u,DU+)
-a(x,t,u,O)).Du+l
d d
e
()2
cp
X r
U++e
tl
n
t2
-
f}diV
a(x, t, u,
0)
~
cp
dxdr
U++e
tl
n
t2
-
f"fa(x,t,u,O).Dcp~dxdr.
J
U++e
tIn
We
let e
'\.
° and discard the
non
- negative contribution of the first integral. The
sum
of the last
two
terms tends
to
zero since
(1)
The
monotonicity assumption (1.7)
is
natural
in
the
existence theory.
It
permits one
to
apply Minty's Lemma
[78]
to
identify
the
weak limit of
the
principal
part
of
the
p.d.e.
when (1.1) is approximated by a sequence
of
regularised problems.

20
n.
Weak
solutions
and
local
energy
estimates
(1.9)
t2
= j j div a(x, t,
u+,
O)<pdxdT
tin
t,
= - jja(x,t,u+,O)'D<PdxdT.
tin
One
checks that the assumptions
of
the lemma are verified for example by
equations with principal part
where
1/Jo
is bounded, non-negative and
.p;,x;
E
Ll(nT)
and the matrix (ai;) is
only measurable
and
positive definite.
Remark
1.1. The 'regularity' assumption (1.8) is only needed
to
justify the limit
in (1.9). It can be dispensed with when working with a sequence
of
approximating
solutions.
2.
Boundary value problems
We will give regularity results for weak solutions
of
(1.1)
up
to
the lateral boundary
ST,
provided u satisfies appropriate Dirichlet
or
Neumann boundary conditions.
We also prove that weak solutions are HBlder continuous
up
to
t = °
if
the initial
datum is HBlder continuous.
Since the arguments are local in nature, for these results
to
hold, the
pre-
scribed boundary
data
have
to
be
taken
only locally. However, for simplicity
of
presentation we will state them globally, in
tenns
of
boundary value problems.
2-(i). The Dirichlet problem
Consider fonnally the Dirichlet problem
(2.1)
{
u
t
.-
div
a~,
t,.u, Du) = b(x, t, u, Du),
u( ,t)18n -
g(
,t),
u(',O) =
uoO,
in nT,
a.e. t E (0, T),
where the structure conditions
(Al)-(As)
are retained.
On
the Dirichlet data 9
and U
o
we
assume
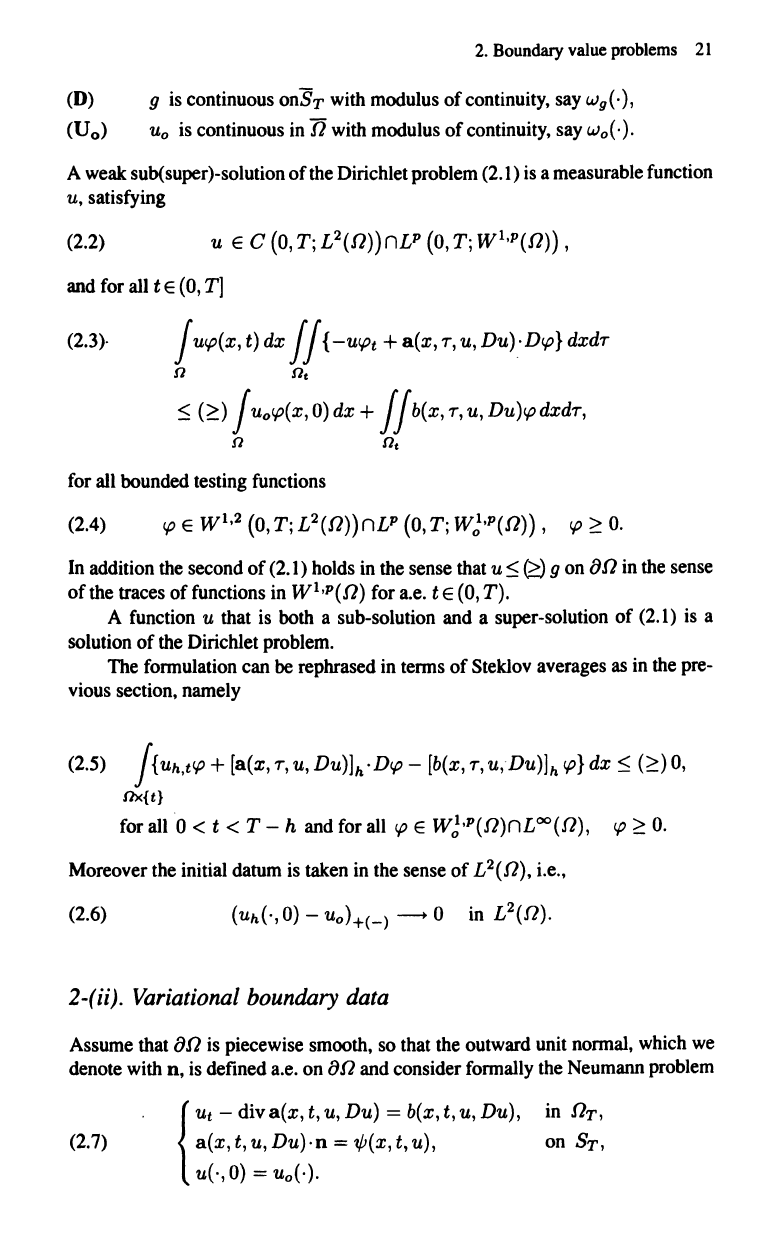
2.
Boundary
value
problems
21
(D)
9
is
continuous onST with modulus of continuity,
say
w
g
(·),
(U
o
)
U
o
is
continuous
in
n with modulus of continuity, say
woO.
A
weak:
sub(super)-solution
of
the
Dirichlet problem (2.1)
is
a measurable function
u, satisfying
(2.2)
and for all
te
(0,
T]
(2.3)· I uY'(x, t)
dx
II
{-uY't
+ a(x,
T,
u,
Du)·DY'} dxdT
fI
fie
~
(~)
luoY'(X,
0)
dx
+
Ilb(X,
T,U,
DU)Y'dxdT,
fI
fie
for all bounded testing functions
In
addition
the
second of (2.1) holds
in
the sense that u
~
~
9 on
an
in the
sense
of
the traces offunctions in W1,p(n) for
a.e.
t e
(0,
T).
A function u that
is
both
a sub-solution and a super-solution of (2.1)
is
a
solution of
the
Dirichlet problem.
The formulation can
be rephrased
in
terms of Steldov averages
as
in
the pre-
vious section, namely
(2.5)
l{uh,tY'
+ [a(x,
T,
u, Du)lh ·DY' -
[b(x,
T,
u,Du)lh
Y'}
dx
~
(~)
0,
!1x{t}
for all 0 < t < T - h and for all
Y'
e w!,p(n)nLOO(n),
Y'
~
o.
Moreover the initial datum
is
taken
in
the sense of L
2
(n), i.e.,
(2.6)
2-(ii).
Variational
boundary
data
Assume that
an
is
piecewise smooth,
so
that the outward unit normal,
which
we
denote with n,
is
defined
a.e.
on
an
and consider formally the
Neumann
problem
{
Ut
- div a(x,
t,
u, Du) = b(x, t, u, Du), in nT,
(2.7) a(x,
t,
u,
Du)·n
=
1/J(x,
t, u), on
ST,
u(·,O) = u
o
(-).

22 ll.
Weak
solutions
and
local energy estimates
We
retain the structure conditions
(AIHAs)
and the assumption (U
o
)
on
the
initial datum.
We
assume that
tP(·,
t, u(·, t)) admits, for a.e. t E (0, T),
an
extension
into
n which
we
denote
by
1fi(.,
t, u( ,t)), such that
(N)
{
11fi1
~
tPou
+
tPlt
a.e. nT,
l1fiul
~
tPo,
l1fiz.1
~
tPl.
i =
1,
2,
...
,N,
where
tPI,
tPo
are given non-negative functions satisfying
(N-i)
1/Jr,1/Jrr
E Ltl,r(nT), whereq and f satisfy (As).
To
give a notion
of
weak sub(super)-solution
we
let
/C
be
an
arbitrary compact
subset
of
RN
and consider testing functions
(2.8)
cp
~
o.
A function u,
(2.9)
is a weak sub(super)-solution
of
the Neumann problem (2.7) if for every compact
subset
/C
of
RN and for every subinterval [tl'
t2J
of
[0,
T]
t
t2
(2.10) j
UCPdXlt2
+ j j{-uCPt + a(x,T,U,Du)·Dcp}dxdT
ICnn 1
tllCnn
~
~
~
(~)
/ /b(x,T,u,Du)cpdXdT + / /
tP(x,
t,
u)cpdudT,
tllCnn
tl
ICnan
where du denotes the surface measure on
an.
We
remark that the testing functions
cp
vanish,
in
the sense on the traces,
on
the boundary
of
/C
and not on the boundary
of
n.
The variational datum
is
reflected in the boundary integral on the right hand
side
of
(2.10). The formulation in terms
of
Steklov averages
is
(2.11) /
{Uh,tCP
+ [a(x,
T,
u,
Du)J
h
·Dcp
-
Ibex,
T,
u,
Du)J
h
cp}
dx
(ICnn]x{t}
~
(~)
j
[1/J(x,
t,
u)Jh
cp
du,
(ICnan]x{ t}
for all 0 < t < T - h and for all
cp
E
W~'P(/C),
cp
~
0,
and the initial datum
is
taken in the sense
of
(2.6).
3. Local integral inequalities
We
will derive some integral inequalities in the interior
of
nT. which will be the
main tools
in
establishing local
HOlder
estimates for the solutions. Analogous es-
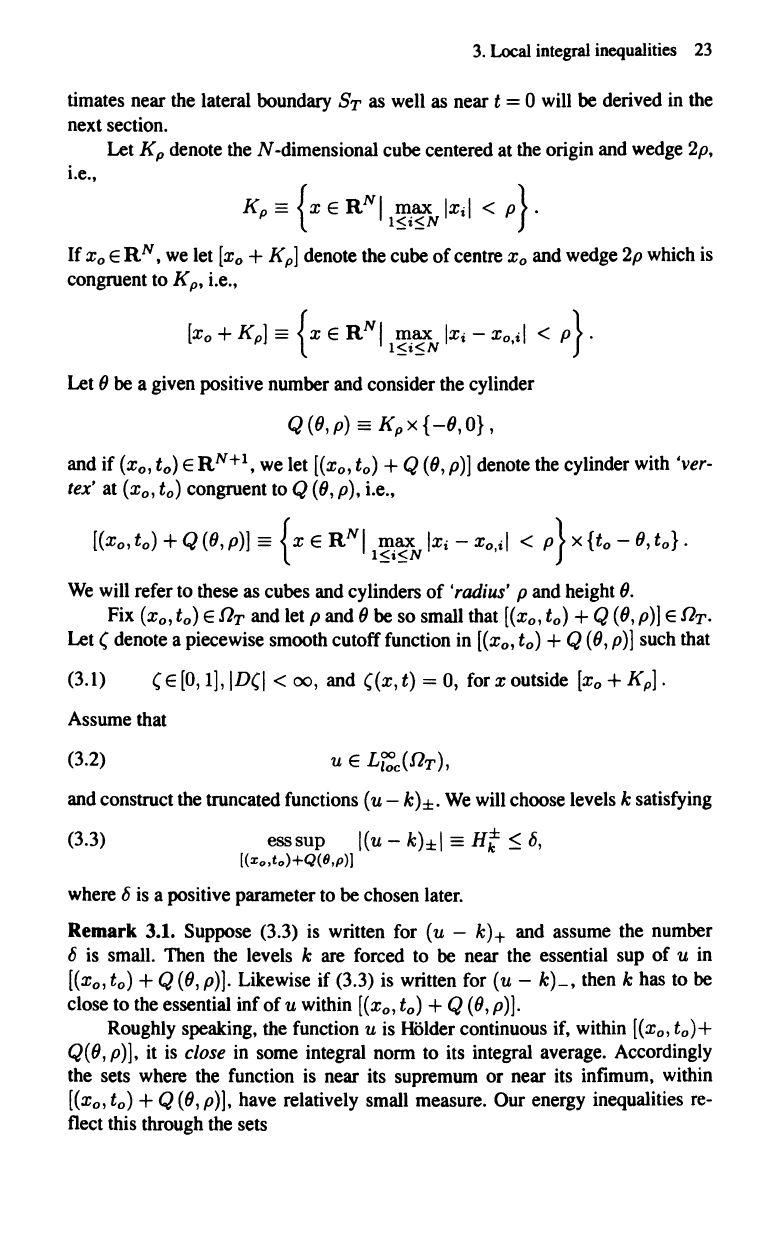
3.
Local integral inequalities
23
timates near the lateral boundary
ST
as well as near t = 0 will be derived in the
next section.
Let
K p denote the N-dimensional cube centered at the origin and wedge
2p.
i.e
.•
If
Xo
E RN. we let
[xo
+ Kp] denote the cube
of
centre
Xo
and wedge
2p
which is
congruent
to
Kp. i.e
.•
[xo
+ Kp]
==
{x
E
RNll~~N
IXi
-
xo.il
<
p}.
Let 6 be a given positive number and consider the cylinder
Q (6,
p)
==
Kp x
{-6,
O}
,
and
if
(xo,
to)
ERN+!.
we let
[(xo,
to)
+ Q
(6,
p)]
denote the cylinder with
'ver-
tex'
at
(xo,
to)
congruent to Q
(6,
p).
i.e
.•
[(xo,
to)
+ Q
(6,p)]
==
{x
E
RNll~~N
IXi
-
xo,il
<
p}
x
{to
- 6, to}.
We
will refer to these as cubes and cylinders
of
'radius' p and height 6.
Fix
(xo,
to)
E n
T
and let p and 6 be so small that
[(xo,
to)
+ Q (6,
p)]
E
nT.
Let
(denote
a piecewise smooth cutoff function in
[(xo,
to)
+ Q (6,
p)]
such that
(3.1)
(E
[0,1],
ID(I
<
00,
and ((x, t) = 0, for x outside
[xo
+
Kp].
Assume that
(3.2)
and construct the truncated functions (u - k)±. We will choose levels k satisfying
esssup I(u -
k)±1
==
Ht
~
0,
[(z",t,,)+Q(II,p»)
(3.3)
where 0 is a positive parameter to be chosen later.
Remark
3.1. Suppose (3.3) is written for (u -
k)+
and assume the number
o is small. Then the levels k are forced
to
be near the essential sup
of
u in
[(xo,
to)
+ Q
(6,
p)].
Likewise
if
(3.3) is written for (u - k)_. then k has to be
close
to
the essential inf
of
u within
[(xo,
to)
+ Q (6,
p)].
Roughly speaking. the function u is HOlder continuous if. within
[(xo,
t
o
)+
Q(6, p)]. it is
close
in some integral norm to its integral average. Accordingly
the sets where the function is near its supremum
or
near its infimum. within
[(xo,
to)
+ Q
(6,
p)].
have relatively small measure.
Our
energy inequalities re-
flect this through the sets
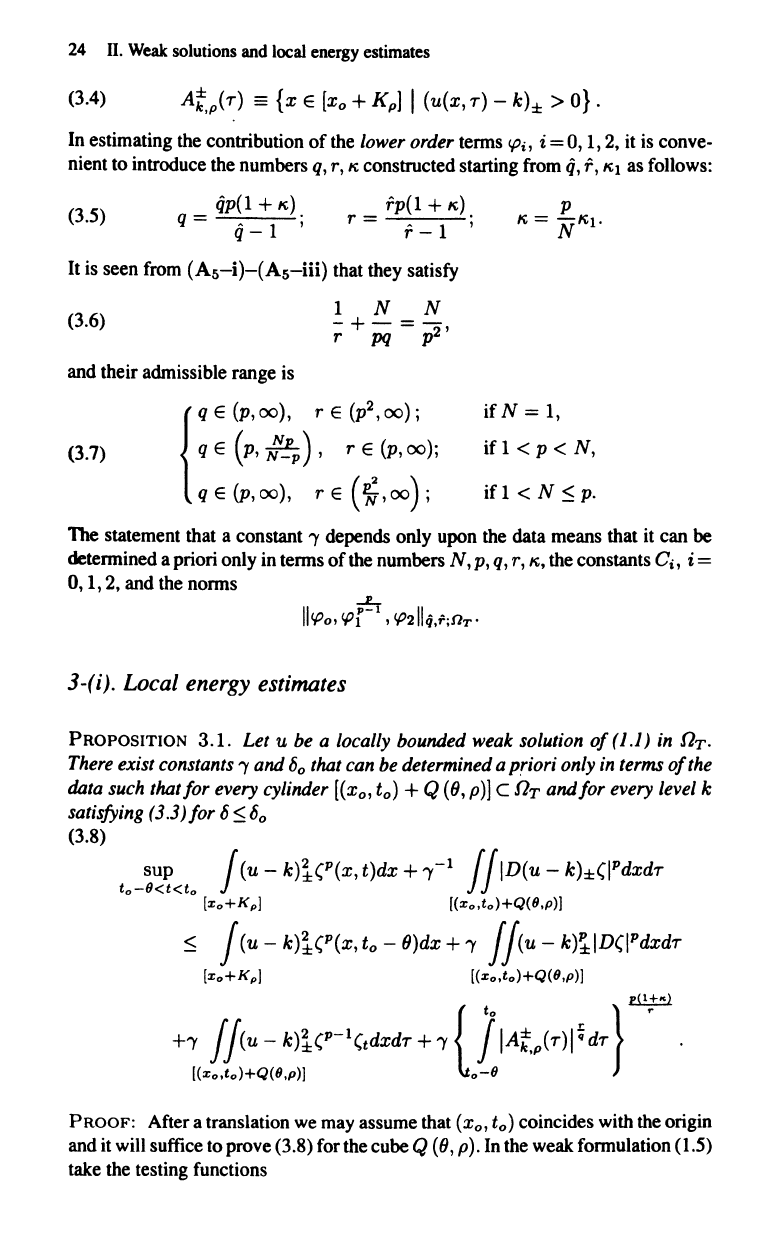
24
II.
Weak
solutions and local energy estimates
(3.4)
At)r)
==
{x
E
[xo
+
Kp]
I
(u(x,r)
- k)± >
O}.
In
estimating
the
contribution of
the
lower order
terms
!.pi, i = 0,
1,
2,
it
is
conve-
nient to introduce the numbers
q,
r,'"
constructed starting
from
q,
r,"'1
as
follows:
(3.5)
qp(1 +
",)
q =
AI;
q-
rp(1 +
",)
r =
AI;
r-
It
is
seen
from
(As-i)-(As-iii)
that they satisfy
1 N N
;+pq=p2'
(3.6)
and their admissible range
is
{
qE(P'OO)'
rE(p2,oo);
q E
(p,
:!p),
r E (p,oo);
qE(P,OO),
rE(~'OO);
(3.7)
if N =
1,
if 1 < p < N,
if!
< N
'5:
p.
1be
statement that a constant
"'I
depends only
upon
the data means that it can
be
determined a priori only
in
terms of the numbers N, p,
q,
r,
"',
the constants C
i
,
i =
0,1,2, and the norms
3-(i). Local energy estimates
PROPOSITION
3.1. Let u
be
a locally bounded weak solution
of
(1.1)
in
n
T
.
There
exist constants
"'I
and 0
0
that
can
be
determined a priori only
in
terms
of
the
data
such that for every cylinder
[(xo,
to)
+ Q
(0,
p)]
c
nT
andfor every level k
satisfying
(3.3)
for 0
'5:
0
0
(3.8)
sup
I(u
-
k)~(P(x,
t)dx +
"'1-
1
fr
flD(u
- k)±(IPdxdr
to-8<t<to
J
[xo+Kp]
[(X
o
,t
o
)+Q(8,p)]
'5:
I(U
-
k)~(P(x,to
-
O)dx
+
"'I
IJ(U
-
k)~ID(IPdxdr
[xo+Kp]
[(Xo,t
o
)+Q(8,p)]
~
+"'1
I
J(u
-
k)~(P-1(tdxdr
+
"'I
{J
IAt,(T)l
i
dT}
·
[(x
o
,t
o
)+Q(8,p)]
0-
8
PROOF:
After a translation
we
may
assume that (xo,
to)
coincides with
the
origin
and
it will suffice to prove (3.8) for the cube Q
(0,
p).
In
the weak formulation (1.5)
take
the
testing functions

3.
Local integral inequalities
25
"P
= ±(Uh -
k)::I::(P
and integrate over (-e, t), t E (-e, 0). Estimating the various terms separately
we have ftrSt
Therefore integrating by
parts and letting h - 0 with the aid
of
Lemma 3.2
of
Chap.
I,
~
j(u
-
k)~(P(x,
t)
dx
-
~
j(u
-
k)~(P(x,
-e)
dx
Kp Kp
t
-
~
I
fiu
-
k)~(p-l(t
dxd-r.
-8K
p
In estimating the remaining parts we first let h _ 0 and then use the structure
conditions
(AI)-(As). To simplify the notation, set
tE(-e,O).
Then
(3.9)
± j
j[a(x,
t, u, DU)]h·D
((Uh
-
k)::I::(P)
dxd-r
~
Q'
II
a(x, t, u,
Du)·
[±D(u
-
k)::I::(P
± p(u -
kh(P-1
D(] dxd-r
Q'
~
Co
IIID(U
-
khlP(PdxdT
-
II
"Po(P
X
[(u
-
k)::I::
>
0]
dxd-r
Q' Q'
- pCI
IIID(U
-
k)::I::lp-I(U
- k)::I::(P-IID(ldxd-r
Q'
- p I j
"PI(U
- k)::I::(P-IID(ldxdT,
Q'
where
x(E)
denotes the characteristic function
of
the set E.
By
Young's inequality
