DiBenedetto E. Degenerate Parabolic Equations
Подождите немного. Документ загружается.

6
I.
Notation and furiction spaces
PROOF:
We
fust prove (2.9) for p =
1.
For every z E e and x
En,
Iz-zl
Iv(x)1
=
Iv(x)
-
v(z)1
= I j :p
v(z+,:::,p)
dpl
o
Iz-zl
$ j
IVV(Z+RP)I
dp.
o
Multiply this inequality
by
cp(x)
and integrate
in
dx over n and in
dz
over
e.
This
gives
Iz-zl
(2.10)
lei
j
cplvldx
$ j
dz
jdX j
<P(x)IDV(z+,::=,p)ldp.
n E n 0
By
virtue
of
the assumption (2.8)
cp(x)IVv(z+,::=,'p)1
$
cpjDvl(z+,:::,p).
We
put this inequality in (2.10) and compute the integral in dx on the right hand
side, in polar coordinates with pole at
z and radial variable r =
Ix
- zl.
We
let w
be
the angular variables and denote
by
R(w) the polar representation
of
an
with
pole at z.
Next, for all
x
En,
and for all
6>
0,
dz
j
dz
Ix
- zlN-1 +
Ix
- zlN-1
En{lz-zl~6}
Minimising with respect to the parameter 6 gives
(2.11)
3.
Parabolic spaces and embeddings 7
for a constant
1'=1'(N,p).
By replacing v with
Ivl
P
in (2.11), we obtain
J
(diamfl)N
J
cplvl
P
dx
<
P'Y
cplvlp-1lDvl
dx
-
1t:11--k
n n
<
~/cplvIPdx
+
1'(P)
[(diam~NlP
/CPIDvIPdx.
- 2
1t:1
1
-
n n
Remark 2.4. Inequality (2.7) follows by applying (2.9) with
cp
==
1 and p = 1 to
the function
w = {:n{v,l} - k
By Corollary 2.2 such a function is in
W1,1(fl).
3. Parabolic spaces and embeddings
if
v>
k
if
v
~
k.
We introduce spaces
of
functions, depending
on
(x, t) E flT, that exhibit differ-
ent regularity in the space and time variables. These are spaces where typically
solutions
of
parabolic equations in divergence fonn are found.
Let
m,
p
~
1 and consider the Banach spaces
and
Vom,P(flT)
==
VlO
(0,
Tj
Lm(fl))nV
(0,
Tj
W;'P(fl)) ,
both equipped with the nonn. v E
Vm,P(
flT
).
When
m=p.
we set V!,P(fl
T
)
==
V!(flT)
and VP,P(fl
T
)
==VP(flT).
Both
spaces are embedded in
Lq
(flT ) for some q > p. In a precise way
we
have
PROPOSITION
3.1.
There exists a constant
l'
depending only upon
N,
p, m such
that
for
every v E
Vom,p(
fl
T
)
(3.1) //lv(x,t)lqdxdt
nT
,;
~,
([/
IDu(x,
tll'dxdt )
(
esSSUP/
Iv(x,
t)lmdX)
."
,
O<t<T
n
where
8 I.
Notation
and
function
spaces
Moreover
(3.2)
N+m
q=p--.
N
The
multiplicative inequality (3.1) and
the
embedding
(3.2) continue
to
hold for
functions v
E V
m
,,,(
n
T
)
such
that
J v(x, t)dx = 0,
n
fora.e. tE(O,T),
provided
8n
is
piecewise
smooth.
In
such
a
case
the
constant
"y
depends
also
on
the
structure
of
8n.
PROPOSITION
3.2. Assume that
8n
is
piecewise
smooth.
There
exists a constant
"y
depending
only
upon
N,
p,
m and
the
structure
of
8n,
such
that for every v E
Vm,"(n
T
),
(3.3)
N+m
q=p--.
N
PROOF
OF
PROPOSITION
3.1: Assume first that
N(p-m)+mp
>
O.
Write
the
embedding inequality (2.1) for the function x
-+
v(x, t) for a.e. t E
(0,
T) and for
the choice
of
the parameters s = m and
N+m
q=p---
j
N
N(P - m) + mp>O.
Taking the qthpower in the resulting inequality and then integrating over
(0,
T)
proves (3.1).
If
N
(p
- m) +
mp
::5
0, we must have p <
N.
Therefore applying
Corollary 2.1,
T
J J
Ivl
q
dx dt = J J Ivl"lvl
mN
dx dt
nT
0 n
T
~
N
$ !
(j
Ivl~dz
)
(j
IV1mdz)
~
$
([f
IDvIPdz
~)
(~~",\'
[ Iv(x,
t)lmdz
r
To prove (3.2), we rewrite (3.1) as
3.
Parabolic spaces
and
embeddings 9
and
apply Young's inequality.
PROOF
OF
PROPOSITION
3.2: IfvEVm,p(n
T
),
consider
the
function
w(·, t) = v(·, t) -
I~I
J v(x, t)dx,
a.e.
t E (0, T),
n
which
has
zero integral average over n
for
a.e.
t E (0, T).
By
Remark 2.1,
x-+
w(x, t) satisfies the embedding inequality (2.1) for
a.e.
t E (0,
T]
and
with
constant
C depending also
upon
the structure of
an.
Proceeding
as
before,
we
arrive at (3.2)
for
w.
For
a.e.
t E (0,
T)
,
IIDwllp,nT
= IIDvllp,nT'
IIw("
t)IIm,n
:::;
211v(·,
t)IIm,n.
Moreover
1
IIwll
•.
a.
"
II-II
•.
",.
- 1!llh!.
(I
U
1_lmdx)
;1.
<it
) •
Therefore
.
The
last term
is
majorised
by
1
(
N(pT
m)+m
p
)
9 esssup
IIv("
t)IIm,n,
Inl
Nm
O<t<T
and
the proposition follows.
We
will
use
the following Corollaries obtained
from
the previous Propositions
by
taking m = p and
by
applying
the
HOlder
inequality.
COROLLARY 3.1.
Let
p >
1.
There exists a constant
'Y
depending only upon N
and
p, such that
for
every v E
Vo"(
n
T
).
(3.4) IIvll:,nT
:::;
'Yllvl
>
OI~IIvll~p(nT)'
COROLLARY 3.2.
Letp>
1.
There exists a constant'Y depending only upon
N,p
and
the structure
of
an.
such that
for
every v E V
P
(
n
T
).
(3.5)
(
T)ih
~
IIvll:,nT
:5
'Y
1 +
Inl
N
Ilvl
>
01
p
IIvll~p(nT)'
The next
two
Propositions hold
in the
case m = p.
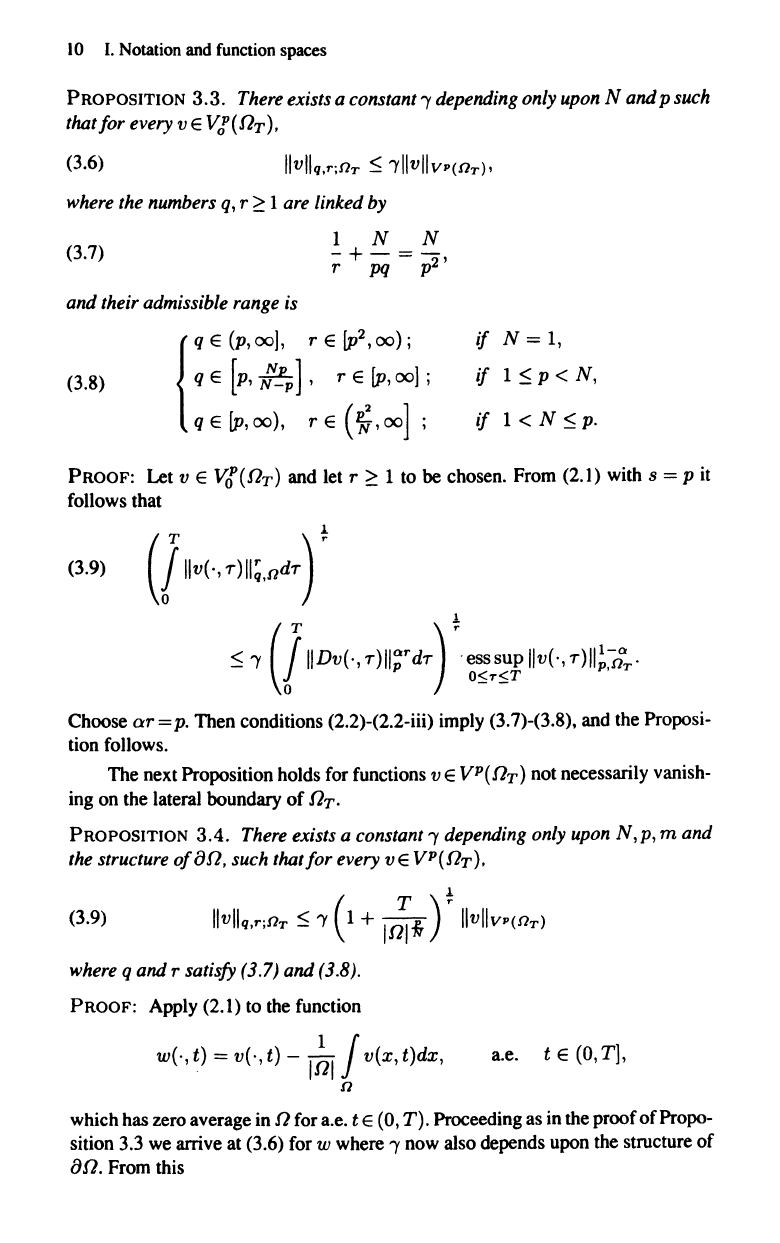
10
I. Notation
and
function spaces
PROPOSITION
3.3.
There
exists a constant
'Y
depending only upon N and p such
that for every v
E
V/(
n
T
),
(3.6)
where
the numbers
q,
r
~
1
are
linked
by
(3.7)
1
N N
-+-=-
r
pq
p'
and their admissible range
is
{
q
E
(p,ool,
r E
[P2,00)
;
q E
[p,
:!p],
r E
[P,ool
;
q E
[P,oo),
r E
(~,oo]
;
(3.8)
if N =
1,
if 1 S p <
N,
if 1 < N S p.
PROOF:
Let v E vt(n
T
)
and let r
~
1 to be chosen. From (2.1) with s =
pit
follows that
Choose
or=p.
Then conditions (2.2)-(2.2-iii) imply (3.7)-(3.8), and the Proposi-
tion follows.
The next Proposition holds for functions
v E V
P
(
n
T
)
not necessarily vanish-
ing
on
the lateral boundary
of
nT.
PROPOSITION
3.4.
There
exists a constant
'Y
depending only
upon
N,
p,
m and
the
structure
of
an,
such that for every
vE
VP(nT),
(3.9)
IIvll
q
,r;S1
T
S
'Y
(1
+
1;11
) t
IIvllvP(S1T)
where
q and r satisfy (3.7) and (3.8).
PROOF:
Apply (2.1) to the function
w(·, t) = v(·, t) -
I~I
J
v(x,
t)dx,
S1
a.e. t E
(0,
TI,
which has zero average in n for a.e. t E (0, T). Proceeding as in the proof
of
Propo-
sition 3.3 we arrive at (3.6) for
w where
'Y
now also depends upon the structure
of
an.
From this
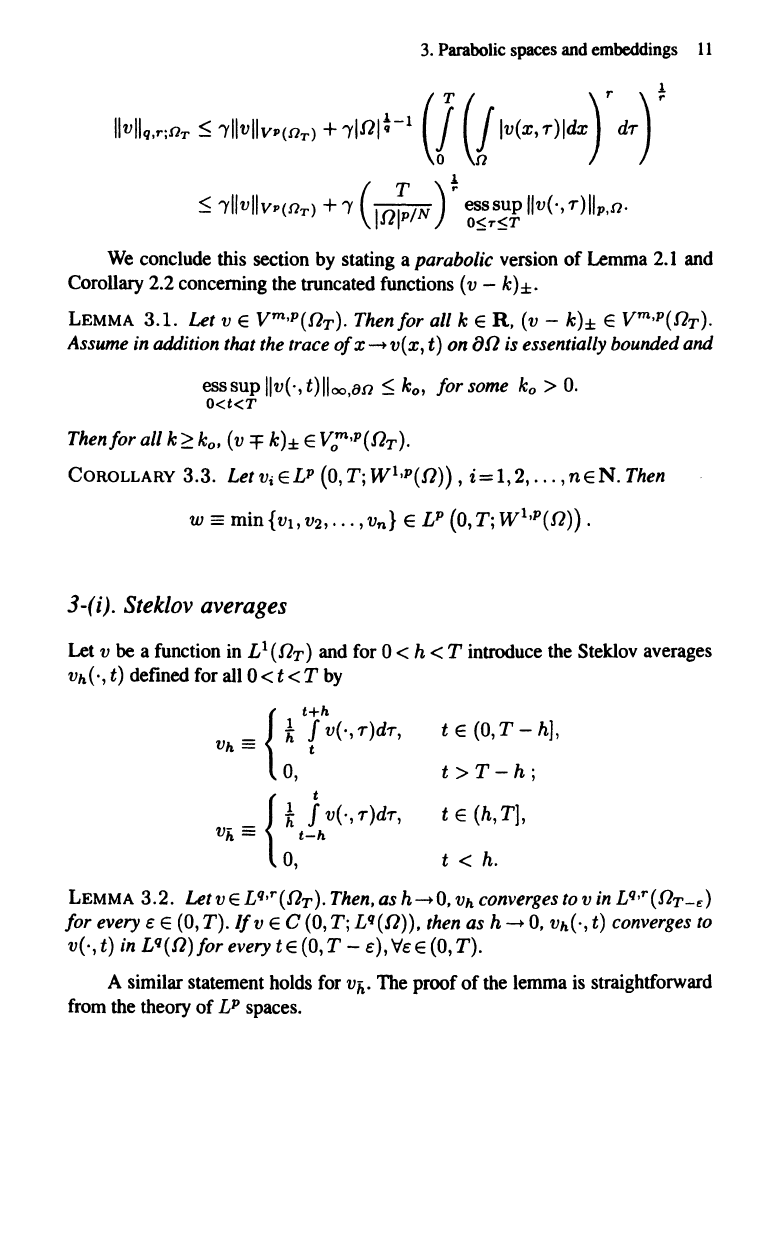
3.
Parabolic spaces and embeddings
II
IIvll
•.•
,,,.
~
~lIvllv.(".)
+
~Inlt-l
(1
U Iv(x.
T)ldx)
·
dT)
1
:$1'lI
v
llvP(I1
T ) +1'
C!1~/N);
~:~~lIv(·,'T)lIp,I1'
We
conclude this section by stating a parabolic version of
Lemma
2.1
and
Corollary
2.2
concerning
the
truncated functions
(v
- k)±.
LEMMA
3.1. Let v E V
m
,P(IlT)' Then/or all k E
R,
(v
- k)± E V
m
,P(!1T)'
Assume
in
addition
that
the
trace
0/
x - v( x, t)
on
all
is
essentially
bounded
and
esssupllv(·,t)lIoo,al1:$ k
o
, /orsome
ko
>
0.
O<t<T
Then/or all k?, k
o
•
(v
=t=
k)± E V
o
m
,P(!1
T
).
COROLLARY
3.3. Let
Vi
ELP
(0,
Tj W
1
,P(Il)),
i=1,2,
...
,nEN.
Then
w
==
min{v1,v2,
...
,v
n
}
E
LP
(0,TjW
1
,P(Il)).
3-(i). Steklovaverages
Let
v
be
a
function
in
L1
(!1T)
and
for
° < h < T introduce
the
Steklov
averages
Vh(-,
t)
defined
for
all
0<
t < T by
{
t+h
_ * J
v(·,'T)d'T,
Vh
= t
0,
t E (O,T -
h},
t>T-hj
_
{*
j
v(','T)d'T,
vii.
=
t-h
0,
t E (h,T],
t <
h.
LEMMA 3.2. LetvE Lq,r(Il
T
).
Then,
as
h-O,
Vh
converges
to
v
in
Lq,r(Il
T
_t:)
for
every
e E
(0,
T).lfv
E C (0, Tj Lq(f1)).
then
as
h -
0,
Vh(-,
t)
converges
to
v(·, t)
in
Lq(Il)/or
every
tE
(0, T - e),
'VeE
(0, T).
A similar statement
holds
for
vii..
The
proof of
the
lemma
is
straightforward
from
the
theory
of
LP
spaces.

12
I.
Notation
and
function
spaces
4. Auxiliary lemmas
4-(;).
Fast
geometric
convergence
We
state and prove two lemmas concerning the geometric convergence
of
se-
quences
of
numbers.
LEMMA
4.1.
Let
{Yn},
n=O,
1,2,
...
,
be
a
sequence
of
positive
numbers,
sat-
isfying
the
recursive
inequalities
(4.1)
where
C,
b,>
1 and a > 0
are
given
numbers.
If
(4.2)
then
{Y
n
}
converges
to
zero
as
n-oo.
The proof is by induction.
LEMMA
4.2. Let
{Y
n
}
and
{Zn},
n=O,
1,2,
...
,
be
sequences
of
positive
num-
bers, satisfying
the
recursive
inequalities
(4.3)
{
Yn+l
:5
Cb
n
(Y,t+a +
Z~+"Yna)
,
Zn+1
:5
Cb
n
(Y
n
+
Z~+,,)
where
C,
b> 1 and
It,
a > 0
are
given
numbers.
If
(4.4)
1
!±!!
1+"
Y.
+ Z
+"
<
(2C)-
"
b---;r
o 0 _ ,
where
u =
min{lt;
a},
then
{Y
n
}
and
{Zn}
tend
to
zero
as
n-oo.
PROOF:
Set Mn = Y
n
+
Z~+"
and rewrite the second
of
(4.3) as
(4.5)
Consider the tetm in braces in the
fU'St
of
(4.3).
If
Z!+"
:5
Y
n
•
such a term is
majorised by
2M~+a.
If
Z~+"
~
Y
n
• then the same term can be majorised by
y~+a
+
(Z~+,,)l+a
:5
M~+a.
Combining this with (4.5) we deduce that in either case
M
<
2C
1
+K.b(1+K.)nM1+min{K.,a}
n+l _
n'
The proof is concluded by induction as in Lemma 4.1.

4.
Auxiliary lemmas
13
4-(ii).
An
interpolation lemma
LEMMA
4.3. Let
{Y
n
} ,
n =
0,1,2
...
, be a sequence
of
equibounded positive
numbers satisfying the recursive inequalities
(4.6)
where
C,
b>
1 and a E
(0,
1)
are given constants. Then
(4.7)
(
2C
)±
Yo
~
bl-~
Remark
4.1.
The Lemma turns the qualitative infonnation
of
equiboundedness
of
the sequence
{Y
n
}
into a quantitative apriori estimate for
Yo.
PROOF
OF
LEMMA
4.3:
From (4.6), by Young's inequality
VeE
(0,1),
n=O,1,2,
....
By iteration
(
C
)~
n-l
.
Yo
~
enYn
+ e
1
-
a
Q
~
(b±e)'.
Choose
b±
e = !
so
that the sum
on
the right hand side can be majorised with a
series convergent to 2. Letting
n
-+
00
proves the Lemma.
4-(iii).
An
algebraic lemma
We
conclude this section by recording two algebraic inequalities needed in what
follows.
LEMMA
4.4.
Letp~2.ThenVa,bERm,mEN
(4.8)
where
'Yo
depends only upon p, m.
Let
1<p<2.
Then Va,
bERm
(4.9)
where
'Yl
depends only upon p, m.

14
I. Notation and function
spaces
PROOF:
J(p) =
(laI
P
-
2
a -
Ibl
p
-
2
b,
a -
b)
1
~
(/
!
I"
+
(1
-
8)bl'-'(80
+
(1
-
8)b)da,
0 -
b)
1
= !
Isa
+
(1
-
s)bI
P
-
2
Ia
-
bl
2
ds
o
1
+
(p
- 2) !
Isa
+
(1
-
s)bI
P
-
4
1(sa
+
(1
-
s)b,
a -
b)1
2
ds.
o
1
Ifp~2.
J(p)
~
la
-
bl
2
J
Isa
+
(1
-
s)bI
P
-
2
ds.
If
lal
~
Ib
-
al.
we have
o
Isa
+
(1
-
s)bl
~
lial-
(1
- s)la -
bll
~
sla
-
bl
and
(4.8) follows.
If
lal
<
Ib
-
al.
1
la
-
bl
2
!
Isa
+
(1
-
s)bI
P
-
2
dx
o
1 2
/2
>
la
_ b
12
!
(Isa
+
(1
-
s)bl
)P
ds
- (2 - s)la -
bl
2
o
(
1
)P/2
~
~
/
Isa
+
(1
-
s)bl
2
ds
=
~
::/2
(la1
2
+
Ibl
2
+
(a,
b)
)P/2
~
'iol
a
-
bjP.
Remark
4.2. The reverse inequality
to
(4.8) is. in general. false. Indeed. if a E R + •
b=a+1
J(p)
=
(p
-
1)~p-2;
for some
~
E
(a,
a + 1).
Letting a
-+
00
shows that the inequality
J(p)
:5
"rIa
-
bl
P
cannot hold with
"r
independent
of
a and
~.
Next.
if!
<p<2.
1
J(p)
:5
(p
-
1)la
-
bl
2
!
Isa
+
(1
-
s)bI
P
-
2
ds.
o
If
lal
~
la
-
bl.
since p<2.
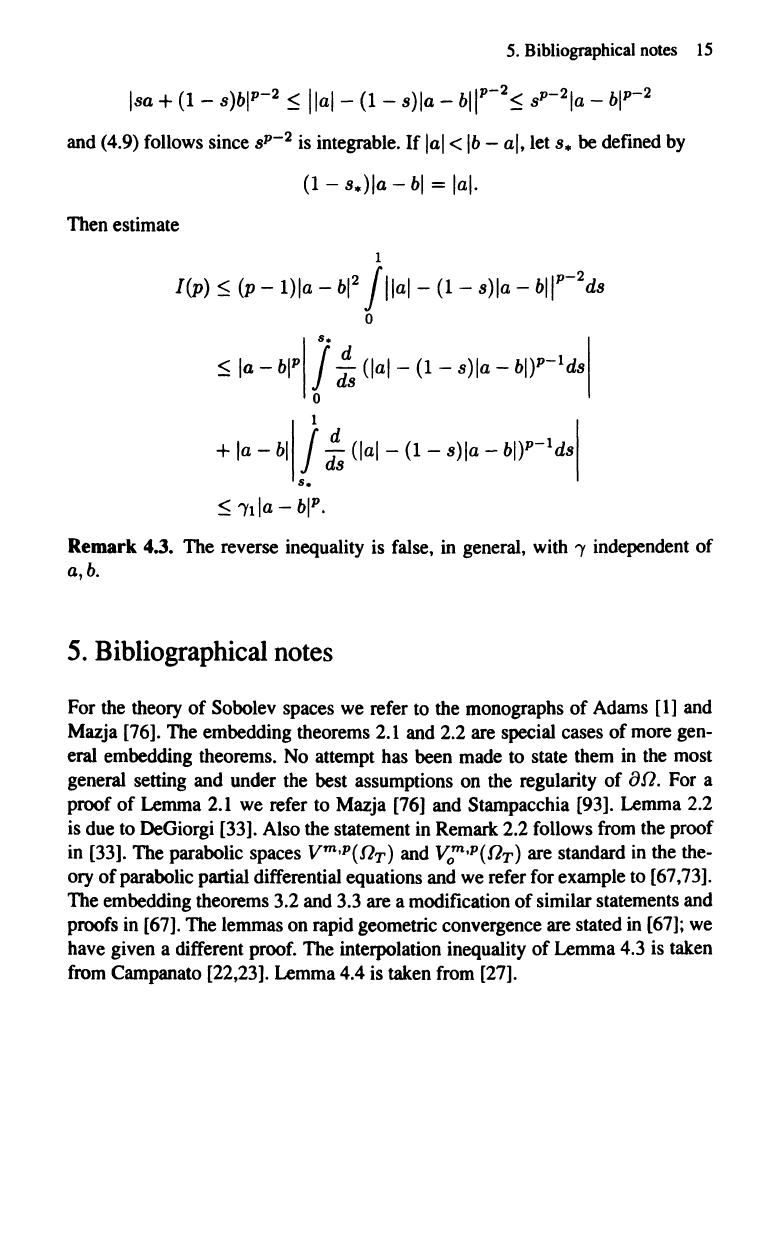
5.
Bibliographical notes
15
Isa
+
(1-
s)bI
P
-
2
~
Ilal-
(1-
s)la -
bIlP-2~
sp-
2
la
-
bl
p
-
2
and (4.9) follows since sp-2 is integrable.
If
lal
<
Ib
-
ai,
let
s.
be defined by
(1
- s.)la -
bl
=
lal.
Then estimate
1
/(P)
~
(p
-I)la -
bl
2
/lIa
l
-
(1-
s)la -
b!lP-2
ds
o
8.
~
la
-
bl
P
/
:s
(lal
-
(1
- s)la - bDp-1ds
o
1
+
la
-
bl
/ !
(Ial
-
(1
- s)la - bl)p-1ds
s.
Remark
4.3. The reverse inequality is false, in general, with
'Y
independent
of
a,b.
5.
Bibliographical notes
For the theory
of
Sobolev spaces we refer to the monographs
of
Adams
[I]
and
Mazja [76]. The embedding theorems 2.1 and 2.2 are special cases
of
more gen-
eral
embedding theorems. No attempt has been made to state them in the most
general setting and under the best assumptions on the regularity
of
afJ. For a
proof
of
Lemma 2.1 we refer to Mazja [76] and Stampacchia [93]. Lemma 2.2
is due to DeGiorgi [33]. Also the statement in Remark 2.2 follows from the proof
in [33]. The parabolic spaces
Vm,P(fJ
T
) and
Vom,P(fJ
T
)
are standard in the the-
ory
of
parabolic partial differential equations and we refer for example to [67,73].
The embedding theorems 3.2 and 3.3 are a modification
of
similar statements and
proofs in [67]. The lemmas on rapid geometric convergence are stated in [67]; we
have given a different proof. The interpolation inequality
of
Lemma 4.3 is taken
from Campanato [22,23]. Lemma 4.4 is taken from [27].
