DiBenedetto E. Degenerate Parabolic Equations

76

m.

HOlder

continuity of solutions of degenerate parabolic equations

of

doubly degenerate equations.

To

be specific consider the p.d.e. (1.1)

of

Chap.

II

satisfying the structure conditions

(AI)

a(x, t,

u,

Du)·Du

~

Co~(Iul)IDuIP

-

IPo(x,

t),

(A2) la(x,t,u,Du)1

$

Cl~(luI)IDulp-l

+~~(U)IP1(X,t),

(A3)

Ib(x,

t,

u,

Du)1

$

C2~(lul)IDuIP

+

IP2(X,

t).

The non-negative functions

IPi,

i=O,

1,2, satisfy (A4)-(A5) of§1

of

Chap. II. The

function

~(

.)

is degenerate near the origin in the sense that there exists a number

eT

o

> ° such that

for given positive constants

° <

1'1

$

1'2

and ° $

f32

$

/31,

This behaviour has to

hold only near the degeneracy, i.e., for

s near zero. For s >

eT

o

it will suffice that

~(s)

be bounded above and below

by

given positive constants, i.e., for example,

We

require that

u E

Cloc

(O,TjL~oc(.a»),

~;6(u)IDul

E

Lfoc(!1r).

Let F(.) denote a primitive of

~;6

(.). Then the p.d.e. can be interpreted weakly

by requiring that

F(u)

E

Lfoc

(O,T;w,!:(n»).

If~(s)

=

1,

'Vs

>0,

then (1.1) is

of

the p-Iaplacian type.

Ifp=2

and

~(s)

=sm-l

for some m >

0,

then

0.1)

exhibits a degeneracy (m >

1),

or singularity (0 < m <

1)

of

the type

of

porous medium equation.

In

the latter case a

weak.

solution is required

to satisfy

lul

m

E

L~oc

(O,T;WI!:(nT»).

The

HOlder

continuity

of

solutions of such doubly degenerate equations can be

proved by methods similar to the ones presented here and

has

been established

independently

by

Porzio-

Vespri

[88]

and Ivanov [52]. The technique is also flex-

ible enough to handle equations bearing a power-like degeneracy at two values

of

the solutions. These arise in the flow

of

immiscible fluids in a porous medium and

have

as

a prototype

Ut

=

Llu(

1 - u) =

0,

Results on continuous dependence appear in

[9]

in a different context.

IV

Holder continuity

of

solutions

of

singular parabolic equations

1.

Singular equations

and

the

regularity theorems

Evolution equations of the type of (1.1) of Chap.

II

for 1 < p < 2 are singular since

their modulus of ellipticity becomes unbounded

when

IDul

=0.

We

will lay out a

theory of local and global

HOlder

continuity of solutions u of such singular p.d.e.

'so

We

assume that u E L

00

(n

T

).

If

u

is

only locally bounded it will suffice to

work

within compact subsets

K.

of

nT.

The intrinsic p-distance dist

(K.j

rjp)

from

K.

to

the

parabolic boundary of

nT

is

defined

as

in

(1.1) of Chap. ID.

In

the theorems

below,

the

statement that a constant

'Y

depends

upon

the

data means that

it

can

be

determined a priori only

in

terms of

lIuli

OO,nT'

the

constants G

i

,

i=O,

1,

2,

and

the

norms

II

'Po

,

'Pr

,'P2I1q,r;nT

appearing in

the

structure conditions (Ad-(A5). For

the

singular range 1 <p<

2,

let

,p-

dist

(K.j

r)

denote the intrinsic parabolic

distance

from

K.

to the parabolic boundary of

nT,

i.e,

(

Cz

1)

P - dist

(K;j

r)

==

inf

lIuli

oo

P

n

Ix

-

yl

+

It

-

sip

.

(

..

,t)elC

' T

(1I,.)er

1-(i).

HOlder

continuity

in

the

interior

THEOREM

1.1.

Let

u be a bounded local weak solution

of

(1.1)

of

Chap,

11

and

let (Ad-(A5) hold. Then u is locally HOlder continuous in n

T

•

and

there exists

constants

'Y> 1

and

a:

E (0,

1)

depending only upon the data. such that

YK.

c

nT.

78

IV.

HBlder

continuity

of

solutions

of

singular parabolic equations

/oreverypairo/points (Xl,

td,

(X2,

t2)

E

IC.

I/the

lower

order

terms

b(x, t, u, Du)

satisfy

(A~)

0/§5 o/Chap.lI,

then

'Y

and a

are

independento/llulloo.o

r

.

1-(ii). Boundary regularity (Dirichlet data)

THEOREM

1.2. Let u

be

a bounded

weak

solution o/the Dirichlet

problem

(2.1)

o/Chap.

II

and

let

(D)

and

(U

o

)

hold.

Assume

also

that

the

boundDry

an

has

the

property 0/ positive

geometric

density (1.1) 0/

Chap.

I.

Then

u E C (liT) and

there

exists a continuous non-decreasingfunction s

-+

w(s) : R+

-+

R+,

such

that w(O);:O and

lu(XI. tI) -

U(X2.

t2)1

:s

w

(ixi

-

x21

+

It

I - t21;) ,

/orevery pairo/points

(XI.

fI),

(X2,

t2)

E nT.lnparticular,

if

the

boundDrydatum

9

is

Holder

continuous

in

ST

with

exponent

say

a

g

,

and

if

the

initial

datum

U

o

is

Holder

continuous

in

Ii

with

exponent

say

auo'

then

(x.

t)

-+

u(x,

t)

is

Holder

continuous

in

nT

and

there

exist constants

'Y

> 1

and

a E (0,

1)

such

that

for every pair o/points (Xl,

til,

(X2'

t2)

E liT,

The

constants

'Y

and a depend

only

upon

the

data

and

the

number

a*

0/

(1.1) o/Chap.l.

Moreover

the

constant a also

depends

upon

the

Holder

exponents

a

g

,

auo

0/ 9 and U

o

respectively.

I/the

lower

order

terms

b(x,

t,

u, Du) satisfy

(A~)

0/§5 o/Chap.lI,

then

'Y

and a

are

independent

o/llulloo.o

r

.

1-(iii). Boundary regularity (variational data)

THEOREM

1.3. Let u

be

a

bounded

weak

solution

o/the

Neumann

problem

(2.7)

o/Chap.1I

and

let

(N) and

(N

- i)

hold.

Assume

that

the

boundary

an

is

0/

class

CI.~.

Then

u

is

Holder

continuous

in

nT

and

there

exist constants

'Y

and a

such

that

for

every

pair o/points

(XI.

td,

(X2,

t2)

E

nT.

2.

The main proposition

79

The

constants

'Y

> 1 antfo:

only

depend

upon

lIulloo.i'iT

and

the

data,

including

the

structure

of

afl

and

the

norms

IItP1,

tPf=r

IIq.r;,aT

appearing

in

the

assumptions

(N) -

i.

If

the

Neumann

data

are

homogeneous.

i.e.,

iftPo=tP1

=0,

and ifin

addition

the

lower

order

terms

b(x, t, u, Du) satisfy (Aa)

of§5

of

Chap.

II.

then

'Y

and

0:

are

independent

ofllulloo.i'iT.

l-(iv). Some comments

The last two Theorems have been stated

in

a global

way.

The

proof however

uses

only local arguments so that they could be stated within any compact portion,

say

/(,

of fl. Accordingly, the hypotheses on the boundary data need only

to

hold

within

/(,.

For example,

in

the case of Dirichlet data, the boundary datum 9 could be con-

tinuous or

HOlder

continuous only on a open portion of

ST

(open in the relative

topology

of

ST), say

E.

Then the solution u of the Dirichlet problem would

be

continuous (respectively

HOlder

continuous)

up

to

every compact subset of E.

Analogous considerations can

be

made

for Neumann data satisfying (N)-(N-i)

on

relatively open portions

of

ST.

Similar remarks hold if U

o

is

only locally continuous or locally

HOlder

con-

tinuous. In particular, to establish the continuity (lli)lder continuity respectively)

of u

up

to

fl

x

{O},

no

reference

is

needed

to

any

boundary data on

ST.

Finally

we

comment on the assumption that u

be

locally bounded.

It

will

be

shown

in the

next Chapter, that

when

p >

2,

solutions of (1.1)

are

locally bounded.

This

is

no

longer true, in general, if 1

<p<

2.

A weak solutions

of

u of (1.1)

is

in

L~(flT)'

only

if

u E

L1oc(fl

T

)

for

some r

~

1 satisfying

N(p

-

2)

+ rp>O

and

such a condition

is

sharp. Thus, unlike

the

degenerate case,

when

p

is

near one,

the

local boundedness

is

not implicit into the notion of

weak

solution and must be

obtained

by

other information such

as

boundary data.

We

refer

to

Chap.

V for a

systematic study of local and global boundedness.

2.

The main proposition

The

lli)lder continuity of u, either in the interior of flT or

at

the parabolic

boundary,

will be, heuristically, a consequence of

the

following

fact.

The function (x, t)

-+

u(

x, t) can

be

modified

in

a set of measure zero

to

yield a continuous representative

out of the equivalence class

uE

Vi!;;(fl

T

),

if for every (xo,

to)

E flT

there

exist a

family

of nested and shrinking cylinders

[(xo,

to)

+ Q

(On'

Pn)]

with

same

vertex

such that the essential oscillation

Wn

of u

in

[(xo,

to)

+ Q

(On,

Pn)]

tends

to

zero

as

80

IV.

HOlder

continuity of solutions

of

singular parabolic equations

n -

00

in a way that

can

be quantitatively detennined by the structure conditions

(AlH

A

5). \

To begin the

proof

of

Theorem

1.1

we

introduce a

space-time

configuration

that reflects the singularity exhibited by the p.d.e. Fix

(xo,

to)

E n

T

and construct

the cylinder

[(X

o

,

to)

+ Q

(RP,

R

l

-

e

)]

C nT,

where e is a small positive number to be detennined later. After a translation one

may assume that

(xo,

to)

==

(0, 0) and set

1'+ =

esssup

u,

1'-

= ess

inf

u,

W=

essosc

u==J.L+

-

1'-.

Q(RP,RI-C)

Q(RP,Rl-<)

Consider the box

E=!

Q

(RP,

CoR)

, where Co =

(~)

P

(2.1)

and where

A is a constant to be detennined later only in

tenns

of

the data.

If

we

assume that

(2.2)

then we have

and

essosc

u <

w.

Q(RP,coR)

-

Cylinders

of

the type

of

(2.1) have the space variables stretched by a factor

(w

/

A)

~

, which is intrisically detennined by the solution.

If

p = 2 these are the

standard parabolic cylinders with the natural homogeneity

of

the space and time

variables.

PROPOSITION

2.1.

There

exist constants eo," E (0,1) and

C,A,A

>

1,

that

can

be

determined a priori

depending

only

upon

the

data,

satisfying

the

following.

Construct

the

sequences

Ro

= R,

WO

=w

Rn

=

c-nR,

Wn+1

=

max{1JWn;.A~t},

n=I,2,

...

,

and

the

boxes

Q(n)

==

Q

(R",

enR,,),

en

=

(:;)

~,

n=O, 1,2,

....

Then

for all n=O, 1,2,

...

A consequence

of

this proposition is

3.

Preliminaries

81

LEMMA 2.1.

There

exist constants

'Y>

1 and

aE

(0, 1) that

can

be

determined a

priori only

in

terms

of

the

data,

such

that for all

the

cylinders

0<

p

~

R,

essosc

U~'Y(w+JtEO)(RP)Q.

Q(pP,cop)

This

is

the

analog of Lemma

3.1

of Chap. III. The proof is

the

same

and it

implies the

HOlder

continuity of u over compact subsets of

{}T

via a covering

argument.

Remark

2.1. The proof of Proposition

2.1

will show that it would suffice to

work

with the number w and the cylinder Q (IV',

CoR)

linked

by

(2.3)

essosc

u <

w.

Q(RP,coR)

-

This fact.

is

in

general not verifiable for a given

box

since

its

dimensions

would

have

to

be

intrinsically dermed

in

terms of the essential oscillation of u within

it.

The reason for introducing the cylinder Q

(RP,

R1-E)

and assuming (2.2)

is

that (2.3) holds true for the constructed

box

Q

(RP,

coR).

It

will

be

part of

the

proof

of Proposition

2.1

to show that at each step the cylinders

Q(n)

and

the

essential

oscillation of

u within them satisfy the intrinsic geometry dictated

by

(2.3).

Remark

2.2. Such a geometry

is

not the only possible. For example,

one

could in-

troduce a scaling with different parameters in

the

space

and

time variables.

Exam-

ples of such mixed scalings will occur along the proof of Proposition

2.1.

Here

we

mention that the proof could

be

structured

by

introducing the boxes Q

(RP-E

,

2R)

and Q

(aoIV',

R)

formally identical to those of

§2

of Chap.

III

and rephrasing

the

Proposition

2.1

in terms

of

such a geometry.

3. Preliminaries

Inside Q

(RP,

CoR)

consider subcylinder: of smaller

size

constructed

as

follows.

The number

w being fixed, let So be the smallest positive integer such that

(3.1)

w

- <fJ

2

80

_

0'

where

fJ

o

is

introduced

in

(3.11) of Chap.

II.

Then construct cylinders

(3.2)

[(ii,O) + Q

(RP,

doR)1

,

E.::l

d =

(~)

P

o 2

80

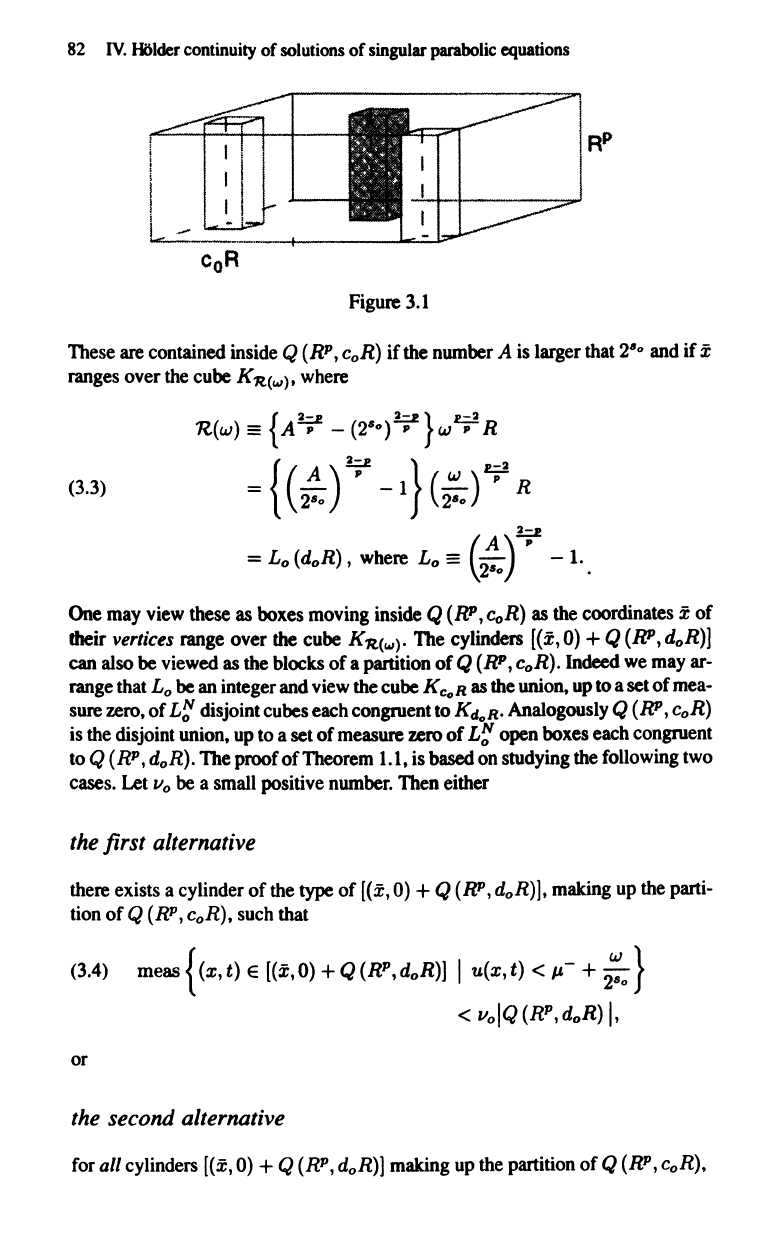
82

IV.

ltiider continuity of

solutions

of

singular

parabolic

equations

....

Figure 3.1

These are contained inside Q

(RP,

coR)

if

the

number A

is

larger that 2

60

and

if x

ranges

over the cube

K'R.(w),

where

(3.3)

!=J!

=

Lo

(doR)

,

where

Lo

==

(:'0)"

-

1.

One

may

view

these

as

boxes moving inside Q

(RP,

CoR)

as

the coordinates x of

dleir

vertices

range over

the

cube

K'R.(w)'

The

cylinders

[(x,

0) + Q

(JlP

,

doR)

I

can also

be

viewed

as

the

blocks

of a partition

of

Q

(RP,

coR).

Indeed

we

may

ar-

range that

Lo

be

an

integer and

view

the

cube

KeoR

as

the union,

up

to

a set of

mea-

sure zero, of

L:

disjoint cubes each congruent

to

KdoR. Analogously Q

(JlP,

coR)

is

the

disjoint union,

up

to a set of measure

zero

of

L:

open

boxes

each congruent

to

Q

(W,

doR).

The proof of Theorem 1.1, is based

on

studying

the

following

two

cases. Let

110

be

a small positive

number.

Then either

the first alternative

there exists a cylinder of

the

type of

[(x,

0) + Q

(W,

doR)],

making

up

the

parti-

tion of

Q

(W

,

coR),

such that

(3.4) meas { (x,

t)

E

[(x,O)

+ Q

(JlP,

doR)]

I u(x,

t)

<

Jr

+

2~0

}

< lIoIQ(RP,doR)

I,

or

the second alternative

for

all cylinders

[(x,O)

+ Q

(RP,

doR)]

making

up

the

partition

of

Q

(W,

CoR),

3.

Preliminaries

83

(3.S) meas { (x, t) E

[(x,

0)

+ Q (R",

doR)]

1 u(x, t) <

p-

+

2~0

}

~

volQ

(R",

doR)

I·

In

either

case

the

conclusion

is

that

the

oscillation of u

in

a smaller cylinder

with

vertex at the origin, decreases

in

a

way

that

can

be

quantitatively

measured.

In

the

arguments

to

follow

we

assume

(2.2)

holds.

Indeed if

not,

pE

eo

=

(2

_ p)

and

the

fll'St

iterative

step

of Proposition

2.1

would

be

trivial.

Remark 3.1.

Along

the proof

we

will encounter quantities of

the

type

i=I,2,

...

,IEN,

where

Ai

are

constants that

can

be

determined a priori only

in

terms

of

the

data

and

(3.6)

b

o

= 2 +

N(p

_ 2)

(~

_ 1 :

K.)

.

From

the

range

of

K.

and

q

as

defmed in

(3.S)-(3.7) of

Chap.

II,

one

checks

that

b

o

~

p.

We

may

assume that

(3.7)

Indeed

if

not,

we

would

have

w $ A'wo

for

the

choices

and

Remark 3.2. The proof

shows

that

the

numbers

e

and

eo

can

be

taken

as

eo(2-p)

e=

.

p

3-(i). About the dependence on

lIull

oo

,I1T

In

the

arguments

below

we

will

use the

energy

and

logarithmic estimates of

Propo-

sitions

3.1

and

3.2

of

Chap.

II,

for

the

truncated

functions

(u-

k)±,

over cylinders

contained

in

Q (R",

CoR).

When

working

with

(u - k)_

we

will

use

the

levels

_ w

k=p

+-2

+.,

8

0

,

for

some

i

~

0,

and

when

working

with

(u - k) +

we

will take

for

some

i

~

o.

84

IV.

Holder continuity

of

solutions

of

singular parabolic equations

These are admissible since

W

11(1.£

- k)±lIoo.Q(RJ>.coR)

::5

2

80

+

i

::5

Do·

Let us fix

Do

as in (3.11)

of

Chap. II. Then, since

w::5

2I11.£ll

oo

.nT' (3.1) holds true,

within

any

subdomain

of

nT,

if

we choose

So

so large that

(3.8)

4

P

+

8

C

2

80

=

Co

1I1.£lloo.nT·

The a priori knowledge

of

the nonn

1I1.£lloo.nT

is required through the number

So.

If

the lower

ordertenns

b(x,

t,

1.£,

D1.£)

in (1.3) satisfy

(A;)

of§5

of

Chap. II, then, as

remarked there, the energy and logarithmic inequalities hold true for the truncated

functions

(1.£

- k)± with

no

restriction

on

the levels k. Thus in such a case,

So

can

be taken to be one and no a priori knowledge

of

1I1.£lloo.nT

is needed.

The numbers

A and

Ai

introduced in (3.7) will be chosen to be larger than

2

80

• In the proof below we will choose them

of

the type

i=1,2,

...

,

where

li

~

0 will be independent

of

1I1.£lloo.nT'

We

have just remarked that

if

the

lower order tenns

b(x, t,

1.£,

D1.£)

satisfy

(A;)

of

§5

of

Chap. II, then

So

can be

taken to be one.

We

conclude that for equations with such a structure the numbers

Ai

can be detennined a priori only in tenns

of

the

data

and independent

of

the

nonn

1I1.£lloo,nT'

4. Rescaled iterations

The following rescaled iteration technique applies to any subcylinder

of

fh

and

it is crucial in both alternatives. Let m

> 0 be given by

and consider the cube

and the box

KdlR

==

{ max

IXil

<

dIR}

,

I$;i$;N

QR

(mb

m2)

==

KdlR

x

{_2

m2

(P-2)

RP,

o}.

Fix (x, t) E

fh,

and let R > 0 be so small that

[(x,

t) +

QR

(mb

m2)] C

nT·

Remark

4.1.

If

(x, t)

==

(0, 0) and 2

ml

= A, m2

=0,

then the cylinder

[(x,

t) +

QR

(mI'

m2)] coincides with Q

(RP,

coR).

Analogously,

ifm2

=0,

mi

=

So

and l = 0, then, for a suitable choice

of

x the cylinder

[(x,

t) +

QR

(mI'

m2)]

coincides with one

of

the boxes making up the partition

of

Q

(RP,

CoR).

4.

Rescaled iterations

85

LEMMA 4.1. There exists a number

Vo

that can be determined a priori only in

terms

o/the

data and independent

o/w,

R and

mt,

m2

such that:

(I).I/u

is a super-solution

0/(13)

in

[(x,

l)

+

QR

{m},

m2)]

satisfying

and

essosc u < w

[(f,l)H2R(mlo

m2)] -

meas

{(x,

t)

E

[(x,

l)

+

QR

(mll

m2)]

I u{x,

t)

<

J.t-

+

2:

}

:::;

v

o

IQR{mllm2)

I,

then either

or

_ w

u{x, t)

~

J.t

+ 2

m

+

1

'

where b

o

is defined in (3.6) and Ao is a constant depending only upon the data

and the numbers

m},

m2.

Analogously

(ll).I/u

is a sub-solution

0/(13)

in

[(x,

t)

+

QR

(m},

m2)]

satisfying

and

essosc u < w

[(f,l)+QR(ml,m2)]

-

meas { (x, t) E

[(x,

l)

+

QR

(mb

m2)]

I u(x, t) >

J.t+

-

2:

}

:::;

volQR

(mt,

m2)

I,

then either

or

u(x, t)

:::;

J.t+

-

2:::+

1

'

PROOF:

We

only prove the statement regarding super-solutions. Assume (x,

t)

==

(0,0)

and construct the decreasing sequences

of

numbers

w w

kn =

J.t-

+ 2

m

+!

+ 2

m

+}+n'

n=O, 1,2,

...

,

and the families

of

nested cubes and cylinders

DiBenedetto E. Degenerate Parabolic Equations

Подождите немного. Документ загружается.