DiBenedetto E. Degenerate Parabolic Equations

286

IX.

Parabolic

p-systems:

fR)lder

continuity of

Du

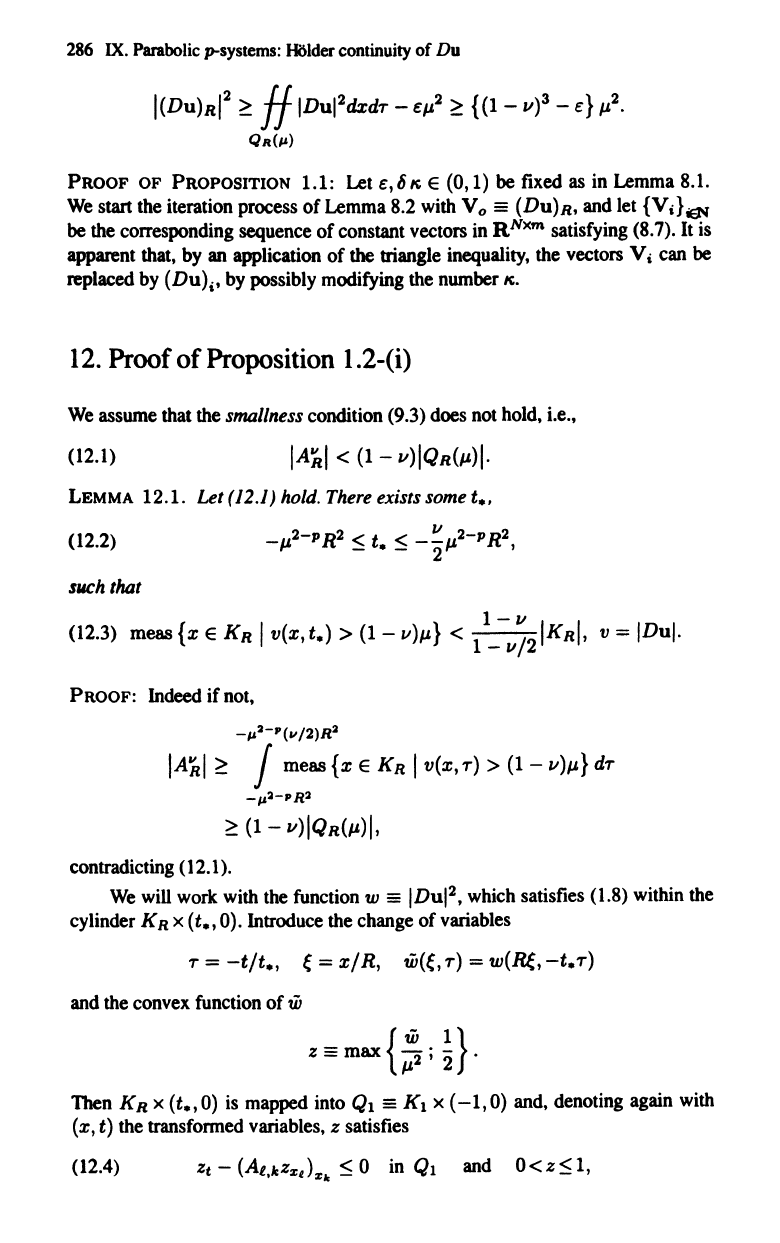
I(Du)RI

2

~

H

IDul

2

dxdT

-

EJ.l.2

~

{(I - v)3 - E}

J.l.

2

•

QR(")

PROOF

OF

PROPOSITION

1.1:

Let E, 6 Ie E

(0,1)

be fixed as in Lemma 8.1.

We

start the iteration process

of

Lemma

8.2

with

Vo

==

(DU)R,

and let {Vi}

~

be the corresponding sequence

of

constant vectors in RNxm satisfying (8.7). It is

apparent that, by

an

application

of

the

triangle inequality, the vectors Vi can be

replaced by

(DU)i' by possibly modifying the number Ie.

12.

Proof of Proposition 1.2-(i)

We assume that the smallness condition (9.3) does not hold, i.e.,

(12.1)

LEMMA

12.1.

Let (12.1)

hold.

There

existssome t ••

(12.2)

such

tMt

I-v

(12.3)

mess

{x

E

KR

! v(x,

t.)

>

(1

-

v)J.I.}

< 1 _ vI2IKR!, v =

IDul·

PROOF:

Indeed

if

not,

_,,2-P(~/2)R2

IARI

~

f

mess

{x

E

KR

I

V(X,T)

>

(1-

v)J.I.}

dT

_,,2-PR2

~

(1

-

V)IQR(J.I.)I,

contradicting (12.1).

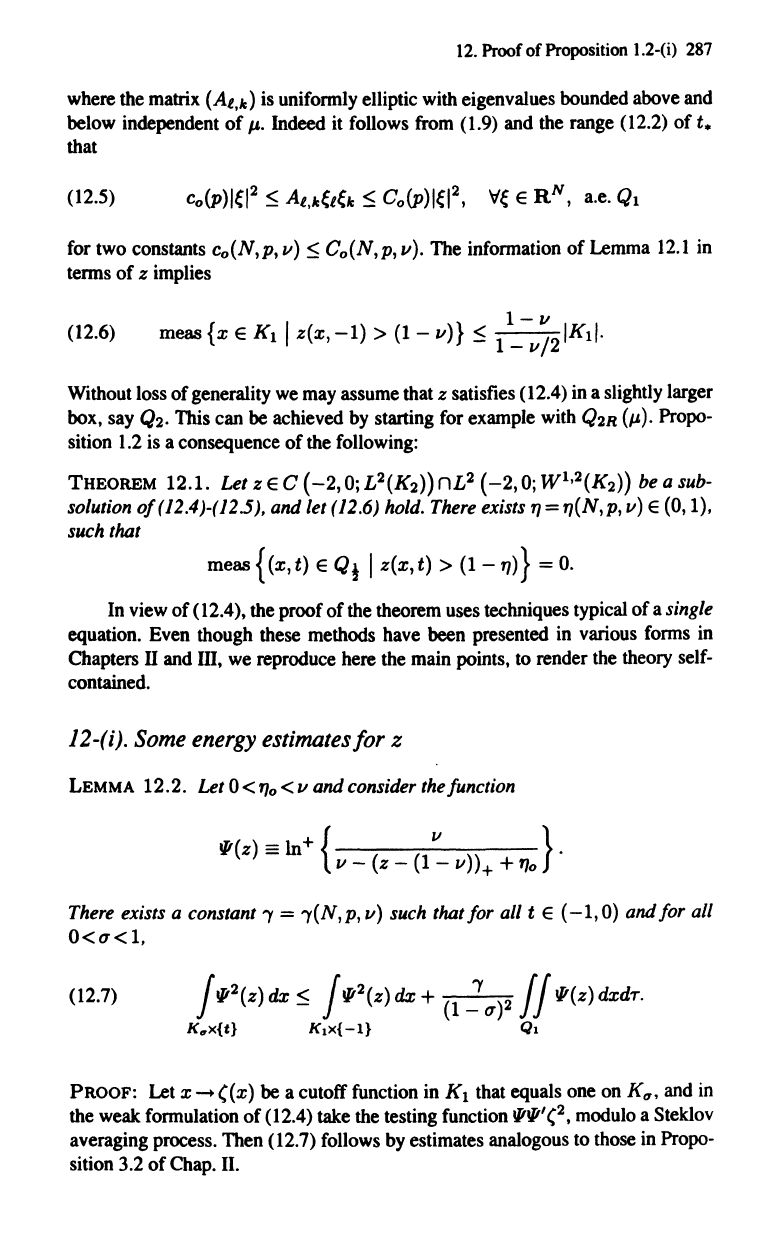
We will work with the function w

==

IDuI

2

,

which satisfies (1.8) within the

cylinder

KR

x (t., 0). Introduce the change

of

variables

T =

-tit.,

e = xl

R,

wee,

T)

=

weRe,

-t.T)

and the convex function

of

w

{

w

I}

%

==

max

J.l.2

i

'2

.

Then

KR

x (t., 0) is mapped into

Ql

==

Kl

X

(-1,0)

and, denoting again with

(x,

t) the transformed variables, % satisfies

(12.4) %t-(At,k%Zt)o;,,:50 in

Ql

and

0<%:51,

12. Proof

of

Proposition 1.2-(i) 287

where the matrix

(At,Al)

is uniformly elliptic

with

eigenvalues bounded above

and

below independent

of

1-'.

Indeed it follows

from

(1.9)

and

the

range (12.2) of

t.

that

for two constants

co(N,p, v)

~

Co(N,p, v). The information of

Lemma

12.1

in

terms of z implies

(12.6)

I-v

meas

{x

E

Kl

I z(x,

-1)

>

(1

- v)}

~

1 _

1I/2IK11.

Without loss of generality

we

may

assume that z satisfies (12.4)

in

a slightly larger

box, say

Q2.

This can be achieved

by

starting for example

with

Q2R

(1-').

Propo-

sition

1.2

is

a consequence of the following:

THEOREM

12.1. Let z E C

(-2,Oi

L

2

(K2»)

nL2

(-2,0;

W

1

,2(K2))

be

a

sub-

solution

off

12.4)-(12.5), and let (12.6)

hold.

There

exists

11

=lI(N,p,

II)

E (0, I),

such

that

meas

{(x,t)

E Q! I z(x,t) >

(1-1I)}

=

O.

In view of (12.4), the proof of

the

theorem uses techniques typical of a single

equation. Even though these methods have been presented

in

various

forms

in

Chapters n and

m,

we

reproduce here the main points,

to

render

the

theory self-

contained.

12-(i).

Some

energy

estimates/or z

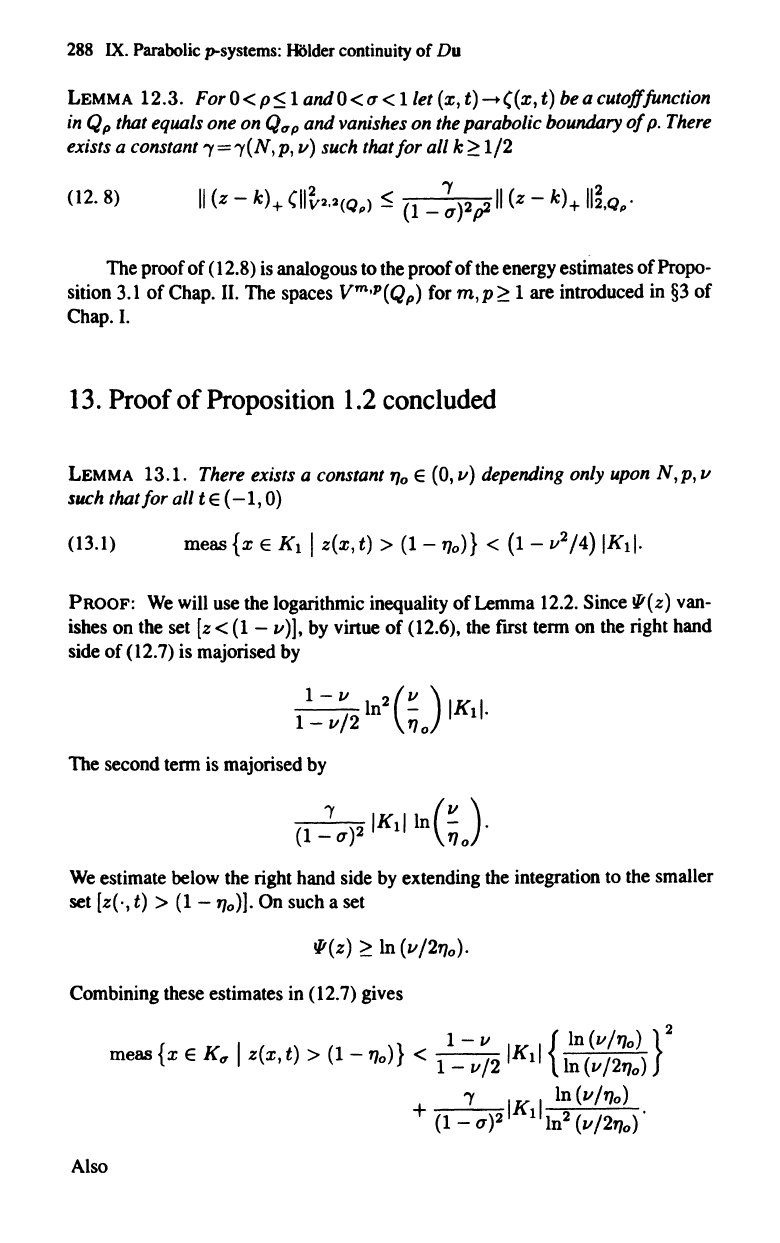
LEMMA

12.2. Let 0 <

110

< v and consider

the

function

!li(z) = In+ {

II

}

-

II

- (z -

(1

-

11»+

+

110

•

There

exists a constant"( = ,,(N,p, v)

such

that for all t E

(-1,0)

andfor all

0<0'<

1,

(12.7) J !li2(z)

dx

~

f !li2(z)

dx

+

(I!

0")2

J J !li(z)

dxdT.

K"x{t}

KIX{

-I}

Ql

PROOF:

Let x -

(x)

be a cutoff function

in

Kl

that equals one

on

KiT,

and

in

the weak formulation of (12.4) take

the

testing function !lilli' (2, modulo a Steklov

averaging process. Then (12.7) follows

by

estimates analogous

to

those

in

Propo-

sition 3.2 of

Chap.

II.

288 IX.

Parabolic

p-systems:

fR)lder continuity of

Du

LEMMA

12.3.

ForO<p$1

andO<u<

lIet

(x,

t)-((x,

t) beacutofffunction

in

Q p that equals one on

Qap

and vanishes on the parabolic

boundJJry

of

p.

There

exists a constant 'Y='Y(N,p, v) such that for all k

~

1/2

(12.8)

The proof of (12.8)

is

analogous

to

the proof of

the

energy estimates of Propo-

sition

3.1

of Chap.

II.

The

spaces

Vm,P(

Q

p)

for

m, p

~

1

are

introduced

in

§3

of

Chap.

I.

13. Proof

of

Proposition 1.2 concluded

LEMMA

13.1.

There

exists a constant

Tio

E (0, v) depending only upon

N,p,v

such that for all t E (

-1,0)

(13.1)

meas

{x

E Kl I z(x, t) >

(1

-

Tio)}

<

(1

-

1.1

2

/4)

IKll·

PROOF:

We

will

use

the logarithmic inequality

of

Lemma

12.2.

Since

!P'(z)

van-

ishes on the set

[z

<

(1

- v)],

by

virtue of (12.6),

the

first

term

on

the

right hand

side of (12.7) is majorised

by

I-v

2(1.1)

1-

1.1/2

In

~o

IKll·

The second term is majorised

by

We

estimate below the right hand

side

by

extending

the

integration

to

the

smaller

set

[z(·,

t) >

(1

-

Tio)].

On

such a set

!P'(z)

~

In

(v/2Tio).

Combining these estimates

in

(12.7) gives

Also

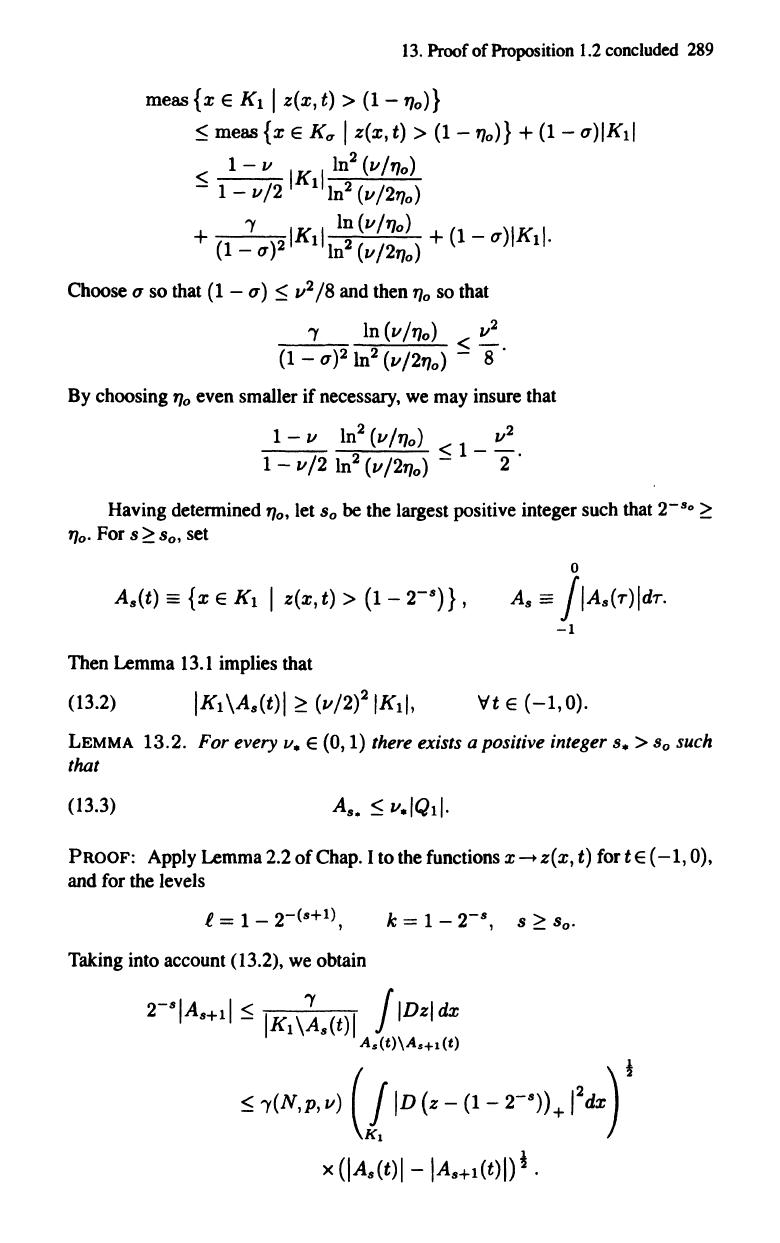
13.

Proof of

Proposition

1.2

concluded

289

meas

{x

E

KI

I z(x,

t)

>

(1

-

'10)}

~

meas

{x

E Ku I z(x, t) >

(1

-

'10)}

+

(1

- O')IKII

< 1 - v IKllln2 (v/'1o)

- 1 -

v/2

In

2

(v/2'10)

'"t

In

(v/'1o)

+

(1

_ 0')21Kllln2 (v/2'10) +

(1

- O')IKII·

Choose

0'

so that

(1

-

0')

~

v

2

/8

and then

'10

so that

'"t

In

(11/'10)

<

112.

(1

- 0')2ln

2

(11/2'10)

- 8

By choosing

'10

even smaller

if

necessary. we may insure that

1 -

II

In

2

(11/'10)

< 1 _

112.

1-

11/2

ln

2

(11/2'10)

- 2

Having determined

'10.

let

So

be the largest positive integer such

that2-

So

~

'10'

For s

~

So.

set

o

A.(t)

==

{x

E

KI

I z(x, t) >

(1

-

2-

S

) } ,

As

==

jIAs(T)ldT.

-I

Then Lemma 13.1 implies that

(13.2)

'It

E

(-1,0).

LEMMA

13.2. For every

v.

E (0, 1) there exists a positive integer

s.

>

So

such

that

(13.3)

PROOF:

Apply Lemma 2.2

of

Chap. I to the functions

x-+z(x,

t)

fortE

(-1,0).

and for the levels

l =

1-

2-(·+1),

Taking into account (13.2). we obtain

2-

S

lAsH I

~

IKI

\~s(t)

I j

IDzl

dx

A.(t)\A.+1(t)

"~(N,p,

v)

(j

ID

(z

-

(1-

2-'))+

I'dx)

I

x

(lA.(t)I-IA

s

+1(t)l) i .

290 IX.

Parabolic

p-systems:

Ht;lder continuity of Du

We

square

both

sides

of

this inequality, integrate in dt over

(-1,0)

and estimate

the resulting integral on the right hand side

by

the

energy inequalities (12.8) written

over

the

pair of cylinders

Ql

and Q2. This gives

4-

s

A~H

:5

"Y

4

-

s

(As -

AsH)

.

Divide through

by

4-

s

and

add

these inequalities for

8=80'

8

0

+ 1,

...

,8.

- 1

to

obtain

s.

(8. - 8

0

-

I)As.

:5

"Y

E (As -

AsH)

:5

"YIQ11·

Therefore

"Y

As.

:5

( 1)

IQ11·

8.

-

80-

PROOF

OF

THEOREM

12.1: Consider the

family

of nested boxes

and

the

increasing levels

n=O,I,

...

,

and

set

Y

n

==

meas {(x, t) E

Qn

I z(x, t) > k

n

} .

Write

the

energy inequality (12.8) over the boxes Qn

for

the

functions

(z

- k

n

)+,

where (

is

the

standard cutoff function

in

Qn that equals one

on

Qn+l'

By

the

embedding Proposition

3.1

of Chap.

I,

with

m = p =

2,

II

(z

- k

n

)!

dxd-r:5 II [(z - k

n

)+

(]2

dxd-r

Q"+1

Q"

On

the other hand

Therefore

x

(if

[(z - k.l+

<)

if<

dzd_,y-It.

y.wb

:5

,,(z -

kn)+

(1I~2.2(Q")Ynwh

:5

4

S

•

Y~+wh

.

Y

nH

:5

"Y

4n

+

s

•

II

(z

- k

n

)!

dxd-r.

Q"+1

y.

<

4

ny'1+1i1h

n+l

_

"Y

n ,

n=0,I,2,

....

It follows

from

Lemma

4.1

of

Chap.

I that

{Y

n

} -

0

as

n -

00

provided

15.

Bibliographical

notes

291

(13.4)

To

prove

the

theorem

we

have

only to pick

8.

by

the

procedure of

Lemma

13.2 so

that (13.4)

is

satisfied and

then

set

'I

=

2-(8.+1).

14.

General structures

Consider

the

general non-linear

system

(1.10) of

Chap.

VIII subject

to

the

struc-

ture

conditions

(81)-(~).

The

proof of Propositions

1.1

and

1.2

for

these

systems

is

analogous

to

that

in

§§6-11.

The

corresponding 'IiMar'

system

about

a

point

(x

o

,

to)

E

flT

is

For this,

the

linear

analysis of §6

can

be

carried with minor changes.

The

analog

of

the

'algebraic'

lemmas

of

§5

are

a direct consequence of

the

structure conditions

(8

1

)-(~).

In

the

proof of Propositions

1.1

and

1.2,

when

working

within

cylinders

[(x

o

,

to)

+ Q (6,

p)],

the 'perturbation

terms'

<Pi,

i

=0,1,2,

contribute

terms

that

are

infinitesimal

with

p of higher order

with

respect

to

those

generated

by

the

principal part.

This

is

due

to

the

integrability condition (86). Further details

on

the

estimation of

the

lower order

terms

can

be

carried

out

with

an

analysis similar

to

the

estimation of

the

lower order

terms

in

Chaps.

II-V.

15.

Bibliographical notes

The content of this Chapter

is

essentially

taken

from

[36,37].

The

estimation of

the

oscillation of

Du

in

§§2

and

3 builds

on

[37]

but

it

is

essentially

new.

The

algebraic

Lemmas

of

§5

are

scattered

in

the

literature

mainly

without

proofs.

We

have

attempted

to

rephrase

them

in

the

context of p-systems.

The

theory

of

linear

parabolic

systems

of §6

is

taken

from Campanato [23].

The

rest of

the

Chapter

follows

[36,37].

X

Parabolic p-systems: boundary

regularity

1.

Introduction

We

will establish everywhere regularity

up

the

boundary

for

weak

solutions of

the

parabolic

system

(1.1)

{

U::(UlIU2,

..•

,Um),

meN,

UieC

(e,T;

L2(O»nV(e,T;

Wl,P(O» ,

Ui,t

-div

IDulp-2 DUi = Bi(X, t,

U,

Du),

in

0 x

(e,

T),

ee(O,T),

i=I,2,,,.,m,

p>max{liJ~2},

associated

with

Dirichlet

boundary

data

(1.2)

in

the

sense

of

the

traces

on

ao,

of

functions

in

Wl,p(n).

The

basic assumptions

on

ao,

the

boundary

data g

and

the

forcing

term

B

g::

(91t!I2,

..

·

,9m),

are

the

following:

(A

l

)

ao

is

of class

Cl,~

for some

oX

e (0,1),

in

the

sense

of (1.2) of

Chap.

I.

Thus

the

norm

IlaolhH

is

finite.

(A2)

The

functions gi, i = 1,2,

...

, m,

are

restrictions

to

ao

of functions

9i'

dermed

in

the

whole

OT,

and

satisfying

1.

Introduction

293

(1.3)

-

.\-

gi,z;

E C (nT),

gi,t

E

LOO(nT),

i = 1,2,

...

, m , j = 1,2,

...

, N.

We

set(l)

m N

0.4)

IIgli

==

L L

{1I9illoo,nT

+

119i,tlloo,nT

+ [9i,z;t,nT}·

i=l

j=1

(A

3

)

IB(x,t,

u,Du)1

::;

Bo

(1

+

IDuIP-1),

a.e.

in

nT,

for

some

given

constant

Bo.

We

say

that a constant

"'1

=

"'1

(data)

depends

only

upon

the data if

it

can

be

determined a priori only

in

terms

of

(data)

==

(N,

p,

B

o

,

Ilanlll+.\,

IIglI)

.

THEOREM

1.1. Let u

be

a

weak

solution

of

(1.1 )-(1.2)

in

n x

(E,

T), and

let

(A

1

)-(A3)

hold.

Then

fl.i

E C

1

-

0I

(nx

(E,

Tj)

, for

every

aE

(0,1), i = 1,2,

...

,

m.

Moreover

for

every

aE

(0,1) and every EE (0, T),

there

exists a constant

"'1

=

"'1

(a,E,IIDullp,flx(£,T),data) ,

such

that

(1.5)

The

constant

"'1

tends

to

infinity

as

either E

'\.

0 or

as

a

'\.

o.

Remark

1.1.

The

constant

"'1

is

• stable'

as

p

-+

2.

THEOREM

1.2 (HOMOGENEOUS BOUNDARY

DATA).

Letubeaweaksolution

of

(1.1

)-(1.2)

with

g

==

0 and

let

(Ad

- (A

2

)

hold.

For

every

E E (0, T)

there

exist constants

"'1

=

"'1

(E,IIDullp,flx(£,T),data) > 1 and a=a(data) E (0,1)

such

that

[tI.i,Z;JOI,'i1x[£,TJ

::;

"'1,

i = 1,2,

...

, m, j = 1,2,

...

, N.

The

constant

"'1/00

as

E

'\.

O.

We

will only carry

the

proof of

Theorem

1.1.

The

proof of

Theorem

1.2

fol-

lows

exactly

the

same

arguments,

where

in

the various estimates

the

contributions

coming

from

IIgll

are

discarded.

(1)

For a smooth function

r/J,

the

norm

[r/Jh,K

is defined

in

(1.3)

of

Chap. I.

294

X.

Parabolic

p-systems:

boundaJy

regularity

2.

Flattening the boundary

Let

e E

(0,

T)

be

fixed.

We

will

estimate

the

oscillation of

Ui

about

each

point

(x

o

•

to)

E

an

x

(e,

T). For

this

we

first introduce a

change

of coordinates that

maps

a

small

portion of

an

about

(xo,

to)

into

a portion of

an

hyperplane.

After

a translation

we

may

assume

that

(xo,

to)

coincides

with

the

origin.

We

will

work

within

the

cylinder

Q'R,

==

K'R,

x

{-

'R.,

O}

,

2'R.

=

min{po;

e},

where

Po

is

the

number that determines

the

structure of

an

as

in

(1.2) of

Chap.

I.

The portion of

the

boundary

annK'R,

is

represented

by

XN

=

4>(x),

x

==

(Xl,

X2,

•••

,

XN-l)

,

where

4>

is

a

function

of class C

l

,>.

in

the

(N

-1)

-dimensional ball8'R,. satisfying

(2.1)

The last condition can

be

realised

by

taking a smaller

Po

if

necessary.

With

respect

to

the

new

variables

Xi

=

Xi,

i = 1,2,

...

,N-I;

the

portion annK'R, coincides

with

the

portion of

the

hyperplane

XN

=0

within

K'R,.

We

orient

XN

so

that,

say,

nnK'R, C

{XN

>O}

and

set

Q~

==

Q'R,n{XN

>

O}.

Denoting

again

by

X

the

transformed variables x

and

with

Ui,

B

i

,

4>,

etc.,

the

trans-

formed

functions.

the

system

(1.1) takes

the

form

(2.2)

(2.3)

(2.4)

( )

_ (

IN-l

-D4>(X))

A X =

-DcI(x)

(1

+

1D4>12(x))

,

where

IN-l

is

the

(N

-1)

x

(N

-

1)

identity

matrix.

To

reduce (2.2) to a system

with

homogeneous

boundary

data

on

Q'R,n{

X N =

O}

set

Wi=U;-.9i,

i=I,2,

...

,m,

and

rewrite (2.2)

in

the

form

(2.5) !

Wi

- div

Ai

(x, t,

Dw)

=

Bi

+

a~l

A"

in

Q:k,

2.

Flattening

the

boundary

295

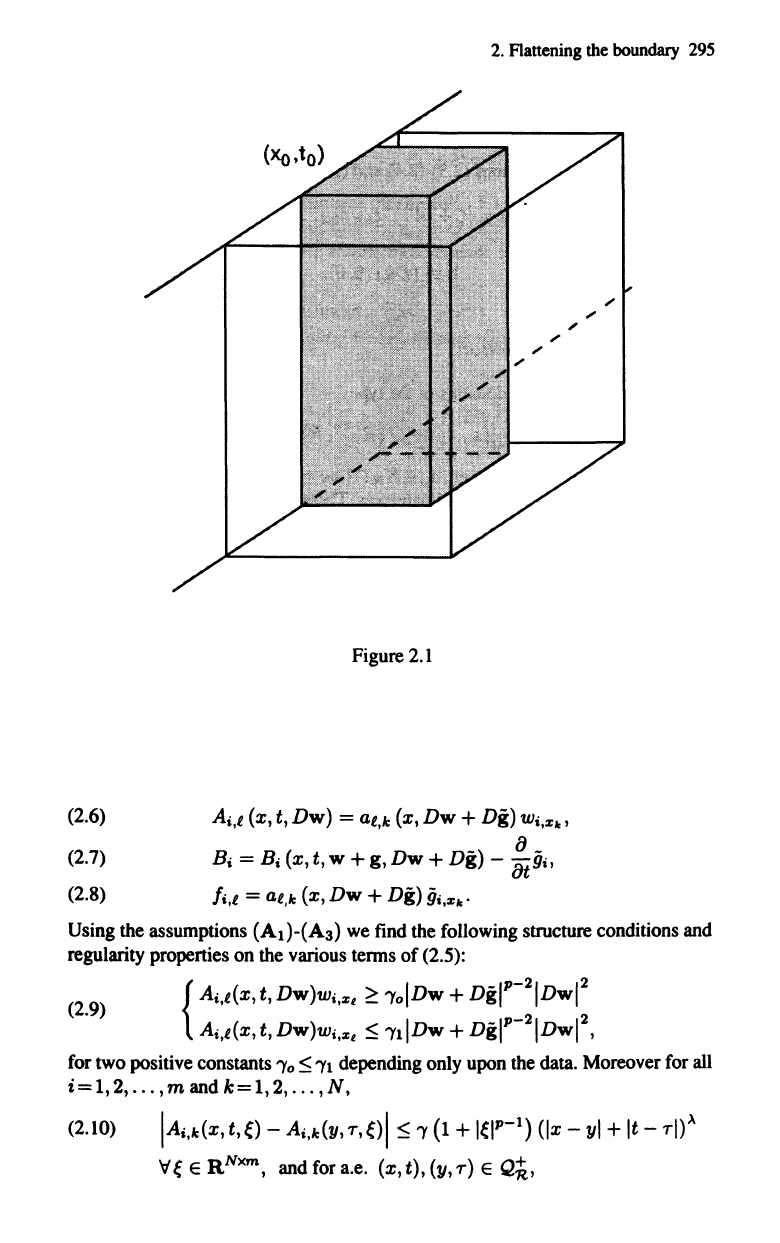
Figure

2.1

(2.6) Ai,t (x, t,

Dw)

= at,k (x,

Dw

+

Di)

Wi,x"

,

(2.7)

Bi=Bdx,t,w+g,Dw+Di)-

!9i,

(2.8) At = at,k (x,

Dw

+

Di)

9i,x".

Using

the

assumptions

(Al)-(A3)

we

find

the

following

structure conditions

and

regularity properties

on

the

various

tenns of

(2.5):

{

Ai,t(x, t,

DW)Wi,XI

~

"YolDw

+ Dil,,-2IDwI2

(2.9)

Ai,t(x,t,Dw)wi,xt

$

"YllDw

+ Dil,,-2I

Dw

I

2

,

for

two

positive constants

"Yo

$

"Yl

depending only

upon

the

data.

Moreover

for

all

i=I,2,

..

.

,m

and

k=I,2,

...

,N,

(2.10)

IAi,k(x,

t,e)

- Ai,k(y,T,e)1 $

"Y

(1

+

lel,,-l)

(Ix

-

yl

+

It

-

TI)~

Ve

E R

Nxm

,

and

for

a.e.

(x,t),

(y,T) E

Q*,

DiBenedetto E. Degenerate Parabolic Equations

Подождите немного. Документ загружается.