Czichos H., Saito T., Smith L.E. (Eds.) Handbook of Metrology and Testing
Подождите немного. Документ загружается.

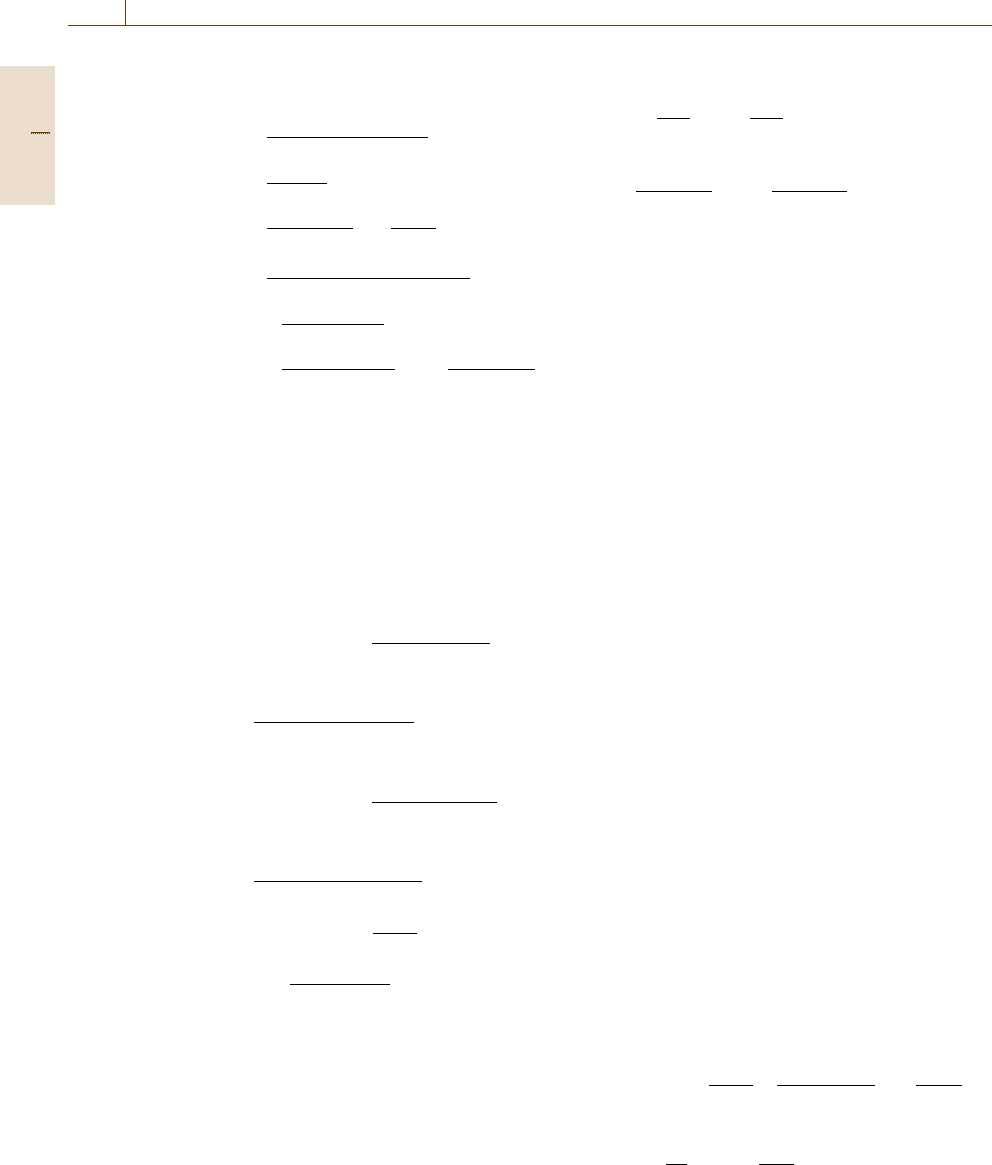
678 Part D Materials Performance Testing
potential E
k
+
=k
0+
exp
−
E
0,A+
−αzFΔφ
RT
=k
0+
exp
αzFΔφ
RT
=k
0+
exp
−αzFΔφ
H
RT
exp
αzFE
RT
, (12.22)
k
−
=k
0−
exp
−
E
0,A−
+(1 −α)zFΔφ
RT
=k
0−
exp −
(1 −α)zFΔφ
RT
=k
0−
exp −
(1 −α)zFΔφ
H
RT
exp −
(1 −α)zFE
RT
.
(12.23)
The introduction of (12.22, 12.23)into(12.19) yields
(12.24). For the conditions of electrochemical equi-
librium, i. e. for E = E
0
and i = 0, one obtains the
expression for the exchange current density i
0
of
(12.25). Introducing the overpotential η = E −E
0
and
(12.25)fori
0
in (12.24) one obtains the Butler–Volmer
equation (12.26)
i =nFk
0+
c(Red) exp
αzF(E +Δφ
H
)
RT
−nFk
0−
c(Ox) exp
−
(1 −α)zF(E +Δφ
H
)
RT
(12.24)
for E = E
0
, i = 0 ,
i
0
=nFk
0+
c(Red) exp
αzF(E
0
+Δφ
H
)
RT
i
0
=nFk
0−
c(Ox) exp
−
(1 −α)zF(E
0
+Δφ
H
)
RT
, (12.25)
i =i
+
+i
−
=i
0
exp
αzFη
RT
−exp
−(1−α)zFη
RT
. (12.26)
For large positive overpotentials η RT/F the ca-
thodic current density may be neglected and for large
negative overpotentials with η RT/F the anodic
current density is vanishingly small. Taking the log-
arithm of (12.26) one obtains the η-log i equations,
(12.27)and(12.28), for both cases. The appropriate
semi-logarithmic presentation yields the so-called Tafel
plot
η =−
RT
αzF
ln i
0
−
RT
αzF
ln i
+
=a +b log i
+
,
(12.27)
η =
RT
(1 −α)zF
ln i
0
−
RT
(1 −α)zF
ln
|
i
−
|
=a +b log
|
i
−
|
. (12.28)
Figure 12.11 gives an example of a dimensionless
log
|
i
|
/i
0
−η presentation for different charge-transfer
coefficients α with an anodic and a cathodic branch.
For α = 0.5 both branches are symmetrical to each
other and for α = 0.5 they are asymmetric. The meas-
ured current densities i deviate from the Tafel lines
for η ≤0.1V and η ≥−0.1 V due to the influence of
the corresponding counter-reaction. Extrapolation of
the Tafel lines meet at the equilibrium potentials with
η = 0, i = i
0
and log
|
i
|
/i
0
=0. The constants a and b
of a Tafel plot are important kinetic parameters for an
electrode process. The b factor contains the value of
α and constant a the exchange current density i
0
.Ac-
cording to (12.22–12.25) i
0
depends on the activation
energy and the electrochemical reaction order for those
species that influence the rate law. The same equations
(12.22–12.28) hold for redox reactions as well as for
anodic metal dissolution and cathodic metal deposition,
when the concentrations of the cations are introduced.
The evaluation of the kinetics of a redox process or
metal dissolution requires Tafel plots, which then yield
data from the slope and the intersection with the ordi-
nate.
An alternative approach requires the determination
of the slope of the polarization curve at E = E
0
only.
For small overpotentials the development of the i-η re-
lation (12.26) in a MacLaurin series with only the linear
terms for η yields (12.29). Its reverse slope for η →0V
yields the charge-transfer resistance R
T
of (12.30). Ac-
cording to this equation only the reciprocal slope of the
polarization curve has to be determined for η →0toget
the exchange current density i
0
. Thus the determination
of the total polarization curve is not required. A similar
situation holds for the rest potential E
R
when two differ-
ent reactions compensate each other, as will be shown
in the following section (12.36, 12.37)
i =i
0
1 +
αzFη
RT
−
[(1 −α)zFη]
RT
=
i
0
zFη
RT
,
(12.29)
R
T
=
dη
di
η→0
=
RT
zFi
0
. (12.30)
Part D 12.2

Corrosion 12.2 Conventional Electrochemical Test Methods 679
12.2.7 Elementary Reaction Steps
in Sequence,
the Hydrogen Evolution Reaction
Electrochemical processes often consist of a sequence
of elementary reaction steps. This situation is treated
similarly to the general rules of chemical kinetics. The
slowest step in a sequence determines the total rate. Any
preceding process is submitted to stationary conditions
or chemical equilibrium may even be established. Any
fast following step does not affect the rate of the over-
all process. In this section the possible mechanisms of
cathodic hydrogen evolution and its influence on the
rate equation and cathodic current density are described.
This relatively complicated electrochemical process is
presented as an example for the treatment of a sequence
of elementary reaction steps. Furthermore it is a very
important reaction for corrosion processes.
Two possible reaction paths are discussed, the
Volmer–Tafel and the Volmer–Heyrovski mechanism.
In both cases the process starts with the oxidation of
hydrogen ions to atoms H
ad
adsorbed at the electrode
surface according to the Volmer reaction of (12.31). The
log | i |/i
0
log | i
–
|/i
0
log i
+
/i
0
(1– a)= 0.75
a =0.75
AnodeCathode
0.5
2
0.15–0.15 –0.1 0.1–0.05 0.05
1
–2
–1
0.5
0.25
0.25
η
D
(V)
Fig. 12.11 Tafel plot for a charge-transfer-controlled reac-
tion and its dependence on the charge-transfer coefficient
α (after [12.35])
following Tafel reaction (12.32a) involves the combina-
tion of two H
ad
to H
2,ad
, which is then finally transferred
to the electrolyte by desorption and diffusion to the
bulk electrolyte. At high negative overvoltages hydro-
gen formation is fast enough to form gas bubbles. An
alternative is the Heyrovski reaction (12.32b), a sec-
ond charge-transfer step which involves the reduction
of a hydrogen ion in the vicinity of H
ad
to from H
2,ad
that then again desorbs and diffuses to the bulk.
Volmer reaction: H
+
+e
−
→H
ad
; (12.31)
Tafel reaction: H
ad
+H
ad
→H
2,ad
; (12.32a)
Heyrovski reaction: H
+
+e
−
+H
ad
→H
2,ad
;
(12.32b)
Desorption: H
2,ad
→H
2
. (12.33)
With the Volmer reaction as a rate-determining step
one obtains for the cathodic current density of hy-
drogen evolution i
H
the Butler–Volmer equation as
described by (12.26). For sufficiently large cathodic
overvoltages i
H
is described by (12.28). A Tafel
plot of (12.28) yields, with z = 1andα = 0.5,
a slope b =
dη
dlog
|
i
H
|
=
RT2.303
(1−α)F
=
0.059
0.5
=−0.120 V. Fig-
ure 12.12 presents the Tafel plot for cathodic hydrogen
evolution at various metals [12.35]. From the slope
1/b of this log
|
i
H
|
–η presentation one obtains for most
metals a factor b =−0.12 V. The Tafel lines for the met-
als are shifted relative to each other, which proves the
strong influence of the kind and surface condition of the
electrode materials on the electrode kinetics. The paral-
lel shift of these lines is caused by the specific values of
the exchange current density i
H,0
for the different met-
als, i. e. of the constant a of (12.28). Pt is a metal with
a small overvoltage whereas Pb and Hg have large over-
voltages. The very fast kinetics on Pt are very useful
for water electrolysis to produce hydrogen as fuel with
optimized energy input. On the contrary Pb and Hg elec-
trodes are well suited to the suppression of hydrogen
evolution when other cathodic reactions are of interest,
such as the reduction of organic compounds or electrol-
ysis of NaCl or KCl solutions, which yield Cl
2
gas at
the anode and K or Na amalgam at the cathode. Hg elec-
trodes are also well suited for polarography. Here again
the large overvoltage suppresses hydrogen evolution
and permits the analysis of cations of very reactive met-
alssuchasZn
2+
and Cd
2+
by their cathodic reduction.
If the Heyrovski reaction of (12.32b) is the rate-
determining step it will lead to the kinetic equa-
tion (12.34). Here Θ
H
is the surface coverage of hydro-
gen atoms which is proportional to their surface concen-
tration. Assuming electrochemical equilibrium for the
Part D 12.2

680 Part D Materials Performance Testing
Current density log
|
i
|
(A/cm
2
)
+2
+1
0
–1
–2
–3
–4
–5
–6
–7
–8
–9
–1.6
Hg
Hg
Hg
Ag
NiNi
Ni
W
Fe
Ag
Hg
Bi
Cd
30
11
1
1
3
15
5
5
1
14
12
31
11
11
30
30
26
26
Hg
Ag
AgHg
κ
Cu
C
Pb
Pb
Rh
Pt (plat.)
Pt
Pt
Pt
Pt
Hg
Hg
2H
+
+2e
–
(2H
2
O+2e
–
H
2
+ 2OH
–
)
–0.4–0.6–0.8–1.0–1.2–1.4 –0.2 0
Overvoltage η (V)
26
H
2
5
7
29
16
36
33
9
Fig. 12.12 Tafel plot of cathodic
hydrogen evolution for different
electrode metals (after [12.35])
preceding Volmer reaction one obtains from the related
Nernst equation (12.35) which allows the replacement
of Θ
H
with (12.36)in(12.34). Equation (12.37)then
contains c
2
(H
+
), i. e. the electrochemical reaction or-
der of H
+
is 2. It also contains a modified exponential
term. Replacing E = E
0
+η one obtains the related
Tafel (12.38) which yields with α =0.5 a slope b =0.40
i
H
=−k
−
Θ
H
c
H
+
exp
−
(1 −α) F
RT
E
,
(12.34)
E = const
+
RT
F
ln
c
H
+
Θ
H
, (12.35)
Θ
H
=const c
H
+
exp
−
F
RT
E
, (12.36)
i
H
=−k
−
c
2
H
+
exp
−
(
2 −α
)
F
RT
E
=−k
−
c
2
H
+
exp
−
(
2−α
)
F
RT
E
0
+η
,
(12.37)
η =
RT2.303
(
2 −α
)
F
log
i
0,H
−
RT2.303
(
2−α
)
F
log
|
i
H
|
=a +b log
|
i
H
|
. (12.38)
Part D 12.2
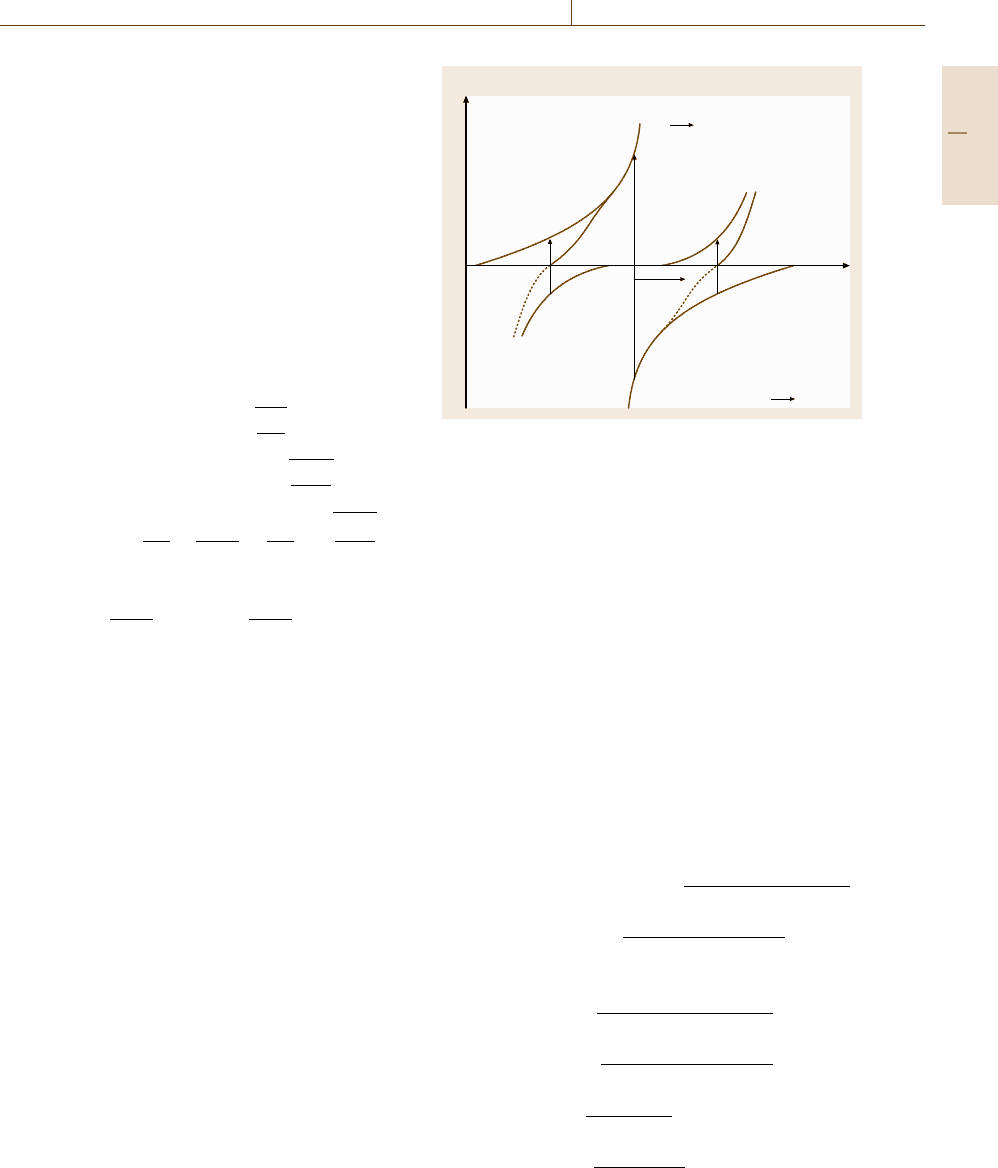
Corrosion 12.2 Conventional Electrochemical Test Methods 681
If the Tafel reaction (12.32a) is the rate-determining
step one starts with (12.39)and(12.40)fori
H
and
Θ
H
and i
0,H
and Θ
H,eq
respectively. Assuming electro-
chemical equilibrium for the Volmer reaction (12.31)
yields the Nernst equation for this charge-transfer step
and consequently (12.41a) for the electrode potential E
and for the overvoltage η. η is thus a consequence of
the varying surface coverage Θ
H
with i
H
. For this dis-
cussion diffusion control of H
+
ions from the bulk
to the electrode surface is excluded and therefore the
same concentration c(H
+
) is present within the bulk
and at the electrode–electrolyte interface. In conse-
quence the Tafel plot according to (12.40) yields a slope
dη/dlogi
H
=b =−0.29 V if the Tafel reaction is the
rate-determining step
|i
H
|=kFΘ
2
H
,Θ
H
=
|i
H
|
kF
, (12.39)
|i
H,0
|=kFΘ
2
H,eq
,Θ
H,eq
=
|i
H,0
|
kF
, (12.40)
E −E
0
=η =
RT
F
ln
Θ
H,eq
Θ
H
=
RT
F
ln
|i
H,0
|
|i
H
|
;
(12.41a)
η =
0.059
2
log |i
H,0
|−
0.059
2
log |i
H
|
=a +b log i
H
. (12.41b)
12.2.8 Two Different Reactions
at One Electrode Surface
The corrosion rate may be ruled by metal dissolution or
by a compensating cathodic reaction of a redox system.
Metal dissolution by hydrogen evolution or oxygen re-
duction are important examples. In acidic electrolytes
hydrogen evolution is the favored counter-reaction for
dissolution of reactive metals such as iron and will be
discussed as an example. The equilibrium potential of
the hydrogen electrode E
0
=−0.059 pH is much more
positive than the standard potential E
0
=−0.409 V for
the Fe/Fe
2+
electrode, which is a necessary require-
ment for an efficient metal dissolution. However in
addition the kinetics of both processes determine the
shape of their polarization curves and thus have a strong
influence on the overall process. Figure 12.13 presents
schematically the anodic and cathodic reactions of both
electrodes in the vicinity of their equilibrium potentials
E
0
(Me/Me
z+
)andE
0
(H
2
/H
+
). The rest potential E
R
is found between both values where anodic metal dis-
solution and cathodic hydrogen evolution compensate
i (A/cm
2
)
0
Me
E (H
2
/H
+
)
E (Me/Me
z+
)
E
R
i
C
i
0, red
i
0, Me
E (V)
π
Me
z+
+ z e
–
2z H
+
+2z e
–
z H
2
Fig. 12.13 Schematic diagram of metal dissolution and hy-
drogen evolution as cathodic counter-reaction with rest
potential E
R
each other. E
R
is sufficiently distant from both equilib-
rium potentials so that only Fe dissolution and hydrogen
evolution have to be taken into account. For a positive
polarization π = E − E
R
> 0 metal dissolution exceeds
hydrogen evolution with a total anodic current i and
for the opposite case hydrogen evolution is larger with
a total cathodic current i. Both partial reactions may be
described by a Butler–Volmer equation and their sum
leads to a similar expression (12.42) as discussed before
when opposite partial reactions of the same electrode
process compensate each other as described by (12.26).
For a vanishing total current density i,i.e.forπ = 0,
one obtains (12.43) for the corrosion current density i
C
.
Its application to (12.42) yields (12.44) which has a sim-
ilar form as (12.26)
i =i
Me
+i
H
=i
0,Me
exp
α
Me
z
Me
F(E −E
0,Me
)
RT
−i
0,H
exp
−
(1 −α
H
)F(E − E
0,H
)
RT
,
(12.42)
i
C
=i
0,Me
exp
α
Me
z
Me
F(E
R
−E
0,Me
)
RT
=i
0,H
exp
−
(1 −α
H
)F(E
R
−E
0,H
)
RT
, (12.43)
i =i
C
exp
α
Me
z
Me
Fπ
RT
−exp
−
(1 −α
H
)Fπ
RT
. (12.44)
Part D 12.2
682 Part D Materials Performance Testing
10
–2
10
–3
10
–4
10
–5
10
–1
10
–6
–0.4–0.5–0.6–0.7–0.8–0.9 –0.3
–0.23
–0.90
1
2
3
4
5
6
31
31
32 33 38 40
0.40
1.63
2.95
pH = 4.0
Electrode potential E (V)
Current density (A/cm
2
)
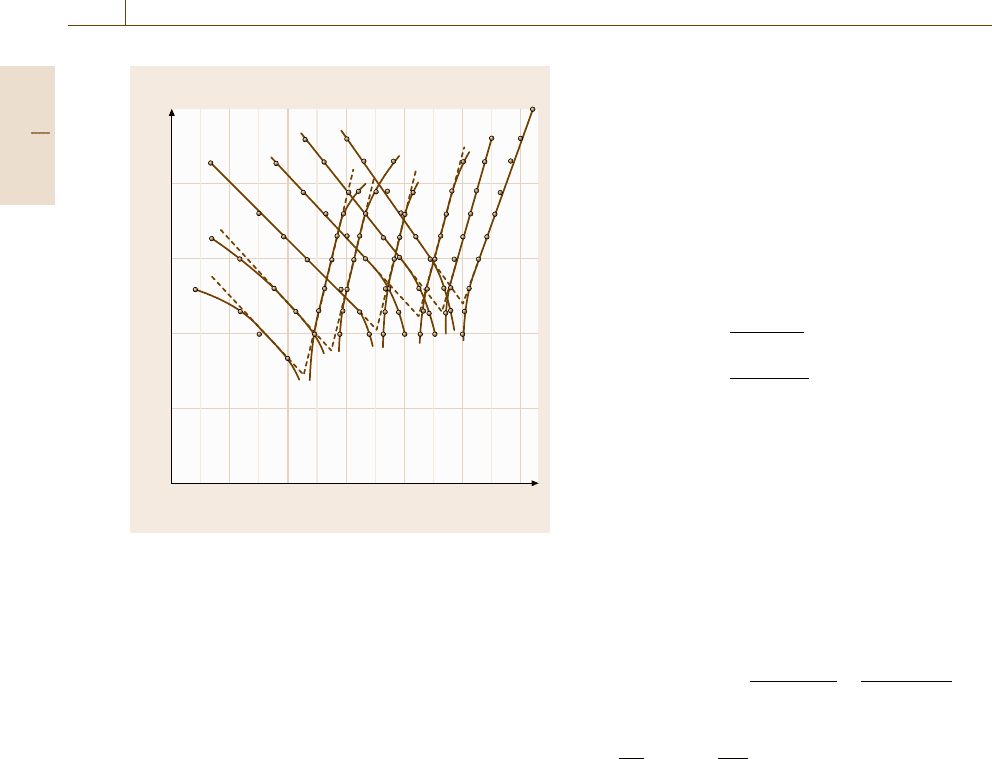
Fig. 12.14 Tafel plot for Fe
2+
dissolution and hy-
drogen evolution of an Fe electrode in solutions
of H
2
SO
4
/Na
2
SO
4
mixtures: 0.482 M/1M pH 4.0,
0.00482 M/1M, pH 2.95, 0.0965 M/0.9M, pH 1.63,
0.75 M/0.75 M, pH 0.40, 2 M/0, pH −0.23, 5 M/0M,pH
−0.90 (after [12.36])
For large polarizations i. e. π RT/α
Me
z
Me
F or
π RT/(1 −α
H
)F the anodic or cathodic current den-
sity dominates the overall process, so that i equals the
first or second term of (12.44), respectively. These con-
ditions yield (12.45)and(12.46), which describe Tafel
plots for metal dissolution and hydrogen evolution. The
extrapolation of the lines intersect at π = 0andE = E
R
with i =i
C
. An example for iron dissolution and hydro-
gen evolution is presented in Fig. 12.14 as a function
of pH [12.36]. With increasing pH the Tafel lines of
hydrogen evolution are shifted to negative potentials.
This is a consequence of the −0.059 pH dependence of
the equilibrium potential of the H
+
/H
2
electrode, but
also of the decrease of the kinetics of H
2
evolution. At
pH 4.0 and 3.0 the start of diffusion control may be seen
by the deviation of the current density from the Tafel
line for large cathodic overvoltages. In addition Fe dis-
solution is pH-dependent; its Tafel lines shift to more
negative potentials with increasing pH. This is a con-
sequence of the aforementioned OH catalysis of iron
dissolution [12.34]. Iron dissolution occurs with a dis-
solution current density log i ∝ 1.5logc(O
−
). Thus the
electrochemical reaction order for OH
−
ions is 1.5, i. e.
log i increases by 1.5 orders when the pH increases by
one unit. Both effects lead to the observed negative shift
of E
R
with increasing pH but they compensate each
other partially with an only moderate decrease of the
corrosion current density i
C
at the rest potential. i
C
may
be determined by the evaluation of the Tafel lines but
also by a direct measurement of metal dissolution. The
related data may be obtained by analysis of the concen-
tration of metal ions within the electrolyte but also by
the weight loss of metal specimens. These independent
data should match each other
log i = log i
C
+
α
Me
z
Me
F
RT
π for π>0 , (12.45)
log i = log i
C
−
(1 −α
H
)F
RT
π for π<0 . (12.46)
A third possibility uses the inverse polarization resis-
tance 1/R
P
=(di/dπ)
π→0
similar to the discussion of
the charge-transfer resistance of (12.30). The develop-
ment of (12.44) by a MacLaurin series for π →0 yields
(12.47)and(12.48) which may serve to determine the
missing kinetic parameters α
Me
and α
H
. With known
charge-transfer coefficients α one may determine i
C
.
Similar relations hold with z
Red
as a factor of the sec-
ond term of (12.47) for the case of other redox systems
such as the reduction of dissolved oxygen or Fe
3+
ions
taking over the counter-reaction for metal dissolution
i = i
C
α
Me
z
Me
Fπ
RT
+
(1 −α
H
)Fπ
RT
,
(12.47)
di
dπ
π→0
=
i
C
F
RT
[α
Me
z
Me
+(1 −α
H
)] . (12.48)
Hydrogen evolution by water decomposition is an
important cathodic process for neutral and alkaline solu-
tions. In these cases the concentration of H
+
ions is too
small to contribute to the cathodic reduction. Hydrogen
evolution by water reduction has a large overpoten-
tial and thus relatively negative potentials are required
to start this reaction. The cathodic polarization curves
for different pH merge at very negative potentials all
in one line because it is not the H
+
but the high and
pH-independent water concentration that enters the rate
equation for these conditions. Figure 12.15 compares
the hydrogen evolution on Fe in HCl solution with 4%
NaCl [12.37, 38]. In acidic electrolytes a linear part
for log i/E is found, corresponding to charge-transfer
control of H
+
reduction. The deviation in the vicin-
ity of E
R
is caused by compensating Fe dissolution.
At large cathodic polarization the current density enters
a plateau which is caused by diffusion control. Its value
Part D 12.2
Corrosion 12.2 Conventional Electrochemical Test Methods 683
Electrode potential E (V)
Current density (A/cm
2
)
–10
–2
–10
–3
–10
–4
–10
–5
–10
–6
–10
–7
–0.5–0.7–0.9–1.1 –0.3
4.11
3.69
2.91
2.69
2.19
1.98
1.42
2.42
pH = 5.26
Fig. 12.15 Hydrogen evolution on Fe in HCl+4% with pH
as indicated (after [12.37,38])
is proportional to c(H
+
). This situation with dominating
diffusion overvoltage will be discussed in Sect. 12.2.11.
Finally all cathodic current densities merge in one line
corresponding to hydrogen evolution by water decom-
position. No limiting current is achieved for very high
cathodic overvoltages due to the high water concen-
tration as reacting species of 55.56 M. For pH > 4no
hydrogen evolution by H
+
reduction is possible due
to its very small bulk concentration and the plateau of
a diffusion-limited process of H
+
reduction is missing.
12.2.9 Local Elements
The above discussion assumes a homogeneous elec-
trode with the same reactivity all over the surface. If
an electrode is composed of physically or chemically
different areas A and B with varying electrochemical
activity their cathodic and anodic reaction rates and re-
lated current densities i
A
and i
B
will follow the same
behavior as described above. i
A
and i
B
are the po-
larization curves that could be measured for the pure
surfaces A and B. They differ from each other in shape
and position on the potential scale and thus have dif-
ferent rest potentials E
R,A
and E
R,B
. The combination
of A and B in one surface causes a potential shift of
A and B to a new value E
R,AB
in between both. At
E
R,AB
the anodic current i
A
on A and a cathodic current
i
B
on B will compensate each other. As a consequence
a net current |i
A
(E
R,AB
) |=|i
B
(E
R,AB
) | will flow from
A to B within the electrolyte and thus will lead to lo-
cal elements at the metal surface. If the electrolyte in
front of the electrode is sufficiently conductive and does
not allow an ohmic drop, A and B will assume the
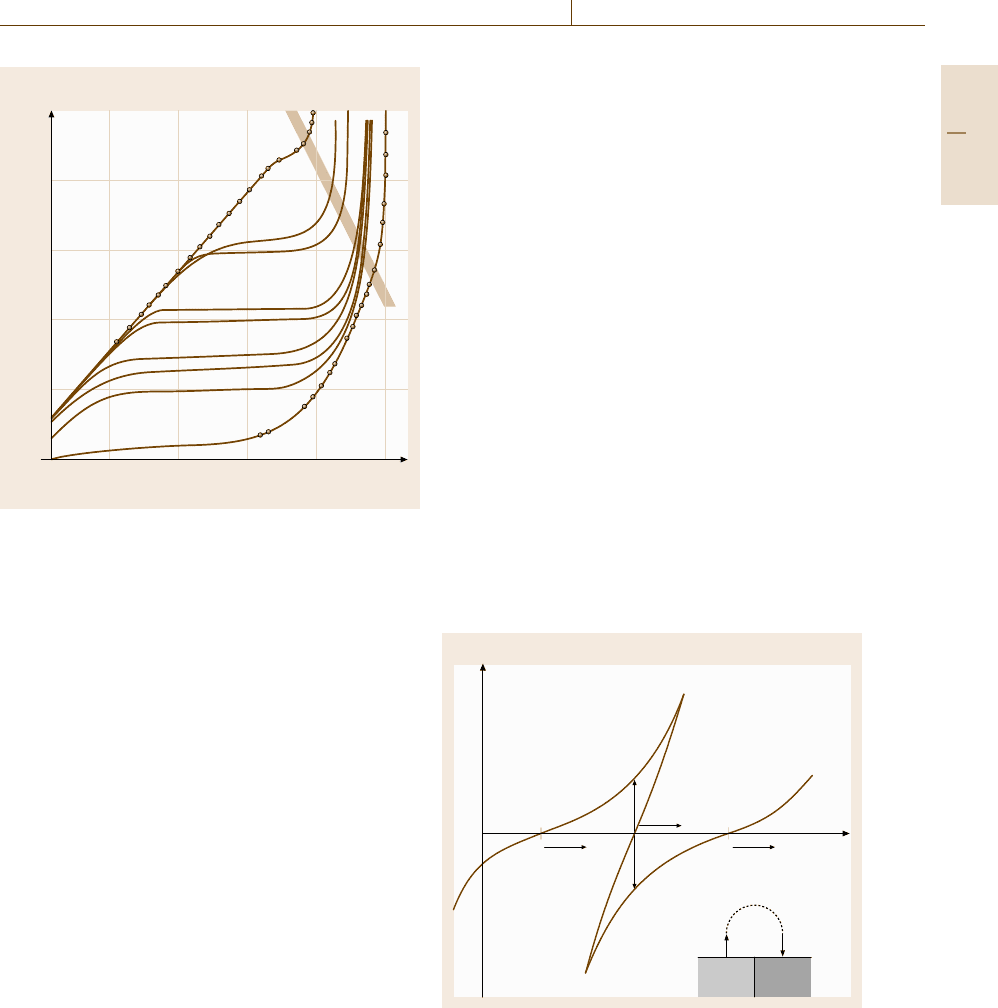
same electrode potential Fig. 12.16. In the example of
Fig. 12.16, A will be dissolved at a higher rate than B
and the reduction of hydrogen will occur at a higher
rate on B. Therefore the A sites are the local anodes
and the B sites are the local cathodes. The application
of a polarization π = E − E
R,AB
via an external sup-
ply, such as a potentiostat, allows the determination of
the polarization curve. One measures the current density
i =i
A
+i
B
as a function of the polarization π of a metal
surface with local anodes A and cathodes B. Low con-
ductivity of the electrolyte may cause a potential drop
in front of the electrode between sites A and B due to
the local current densities between both. This situation
will be discussed in Sects. 12.2.12 and 12.2.13.
These local elements frequently lead to a pro-
nounced increase of the corrosion rate. A well-known
example is the increase of Zn dissolution by small
deposits of copper at its surface for open-circuit condi-
tions. Hydrogen evolution on the Cu deposits occurs at
i (A/cm
2
)
E (V)
0
AB
E
R,AB
E
RB
i
A
i
A
i
A
(E
R,AB
) i
B
(E
R,AB
)
i = i
A
+ i
B
i
B
i
B
π
π
B
E
RA
π
A
Fig. 12.16 Polarization curve of a metal surface with sites
A and B with different electrochemical properties. Rest po-
tentials E
RA
and E
RB
of pure sites and E
R,AB
of mixed
electrode. Polarization π for the sites A and B and mixed
surface AB. i
A
, i
B
are current densities of sites A and B
which compensate each other at E
R,AB
. Inset shows local
elements with current flow from A to B at rest potentials
E
R,AB
Part D 12.2
684 Part D Materials Performance Testing
C
δ
C
B
x
Fig. 12.17 Concentration profile in front of an electrode
with Nernst diffusion layer of thickness δ, maximum cur-
rent density i
D
for c =0
much higher rates, thus promoting zinc dissolution. The
common rest potential is positive when compared to that
of pure zinc. These local elements often cause serious
corrosion damage. Another well-known example is the
corrosion of Cu-containing commercial aluminum. Cu
inclusions prevent the total protection of the Al surface
by a passivating Al
2
O
3
film. Cu acts as a local cath-
ode, which compensates the dissolution of Al by local
oxygen reduction. Well-passivated Al does not permit
the reduction of redox systems such as oxygen due
to the isolating electronic properties of the pure pro-
tecting Al
2
O
3
film. For similar reasons one should not
combine reactive metals such as Fe with Cu in water-
containing supplies. The close vicinity of both metals
and some Cu dissolution and its redeposition on a re-
active metal surface will cause local elements. Effective
oxygen reduction at these Cu deposits causes increased
Fe dissolution, as described above.
12.2.10 Diffusion Control
of Electrode Processes
In many cases metal corrosion and the compensating
redox process may be under diffusion control, which
leads to a diffusion overvoltage η
D
. Diffusion of H
+
ions in weakly acidic electrolytes during Fe dissolution
is one example that was discussed in the last section.
Oxygen diffusion within electrolytes is another. Low
oxygen concentration in solution and thick layers of
electrolyte are a frequent situation that causes diffusion
overvoltage. In all these cases the concentration of the
oxidizing species reduces at the metal surface due to
its intense consumption by the reduction process, lead-
ing to a concentration profile. Similarly high dissolution
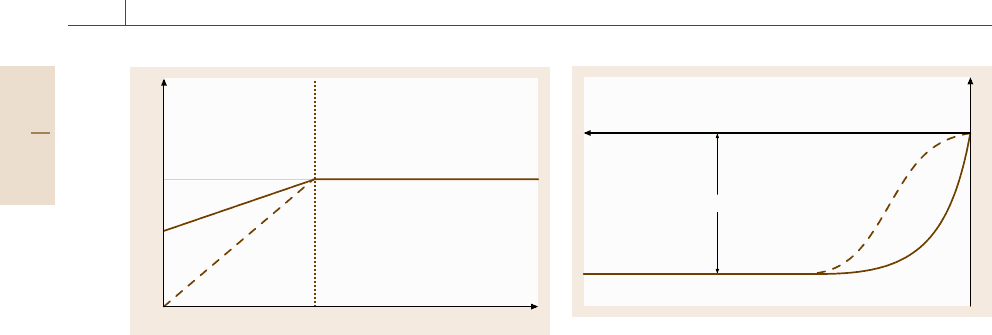
i
η
i
D
Fig. 12.18 i/η dependence for a cathodic reaction un-
der diffusion control without (solid) and with additional
charge-transfer control (dashed line)
rates of a metal cause the accumulation of its cations at
the metal surface and a related increase of its concentra-
tion. As a consequence a concentration profile builds up
which may cause locally saturated or supersaturated so-
lutions and even the precipitation of corrosion products,
such as the formation of salt films.
Figure 12.17 presents the concentration profile of
the oxidized component Ox in front of an electrode
within the Nernst diffusion layer of thickness δ. The
dashed line refers to the maximal concentration gradient
when the concentration of Ox at the surface approaches
zero. For a diffusion-controlled electrode process the
transfer of Ox equals the current density i accord-
ing to (12.49) which is a combination of Fick’s first
diffusion equation and Faraday’s law with Faraday’s
constant F, the diffusion constant D, the concentra-
tion of Ox at the surface c
S
and within the bulk c
B
,
the thickness of the diffusion layer δ and the number
of electrons for the charge-transfer process n. For van-
ishing concentration c
S
= 0 one obtains the maximum
diffusion current density i
D
according to (12.50). The
combination of (12.49)and(12.50) yields (12.51). If
the charge-transfer reaction is fast with respect to diffu-
sion the electrochemical equilibrium is established with
an electrode potential E = E
0
+η
D
. η
D
is given by the
difference of the electrode potentials for the concen-
trations c
S
and c
B
according to the Nernst equation,
which yields (12.52). Introducing the current densities
i and i
D
with (12.49)and(12.50) one obtains (12.53).
Figure 12.19 depicts the change of the current den-
sity with η for a cathodic process which approaches
i
D
for large overvoltages. For small η the reaction be-
comes charge-transfer controlled, which results in the
dashed curve of Fig. 12.18. Similarly metal dissolution
for large positive polarizations becomes diffusion con-
Part D 12.2
Corrosion 12.2 Conventional Electrochemical Test Methods 685
Resin
Electrolyte
Resin
Metal
disc
Fig. 12.19
Rotating disc
electrode with
electrolyte con-
vection in front
trolled and the current density becomes independent
of the electrode potential. For large positive overvolt-
ages the accumulation of cations at the electrode surface
yields precipitation of corrosion products and salt films
may form, which slow down the metal dissolution rate.
In many cases the growth of oxides films at the metal
surface causes a much more pronounced decrease of its
dissolution current density. These processes may lead
to thin passive layers that act as a barrier to the trans-
fer of cations from the metal to the electrolyte, thus
effectively protecting even very reactive metals against
corrosion.
i =−
(c
B
−c
S
) nFD
δ
, (12.49)
i
D
=−
nFDc
B
δ
, (12.50)
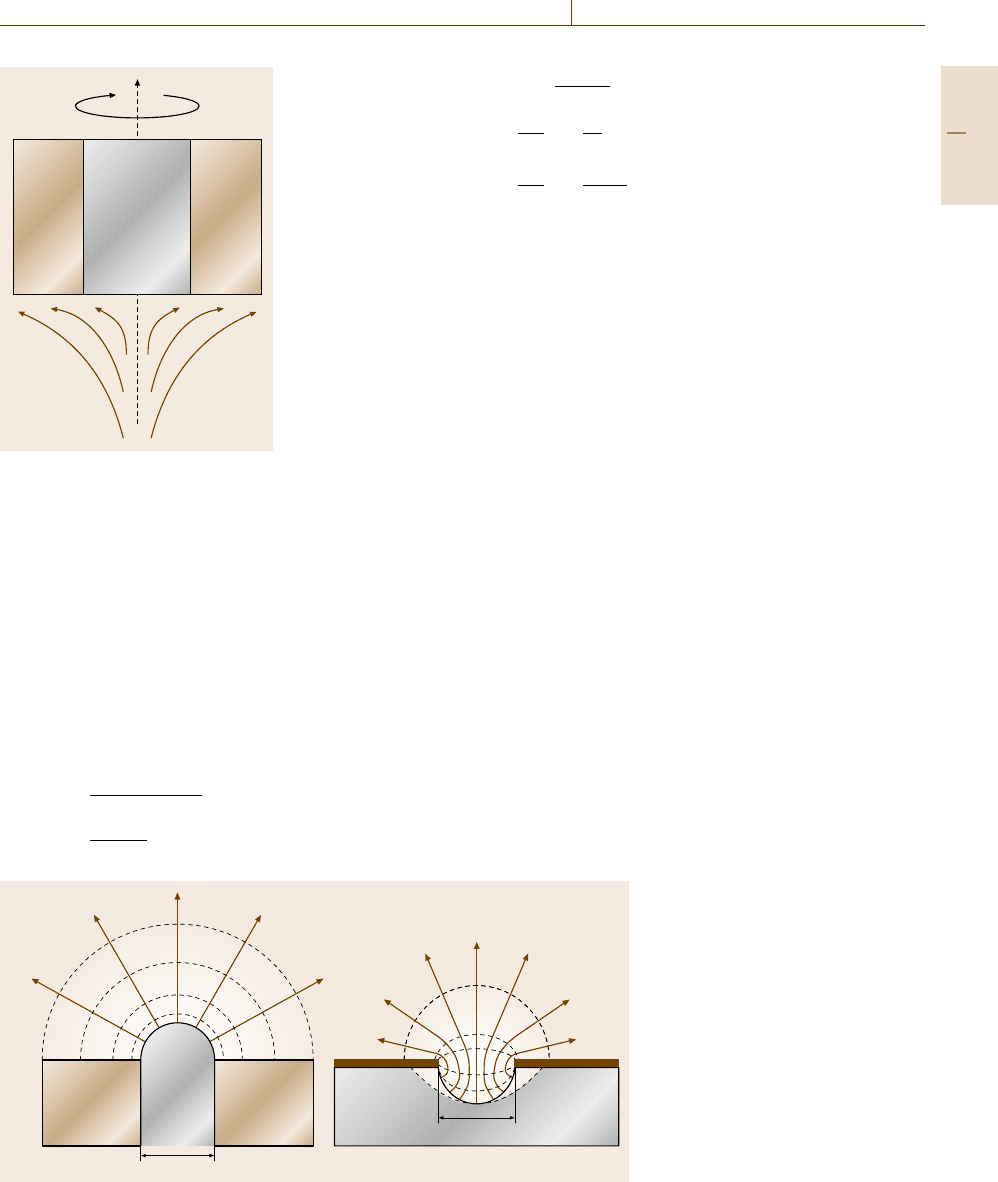
a) b)
Electrolyte
Electrolyte
Metal
Metal
Resin
Passive Passive
2a
2a
Fig. 12.20a,b Cross section
of a convex hemispherical
electrode/electrolyte com-
bination (a) and a concave
hemisphere (b) with diffu-
sion lines or current lines
(solid) and curves of equal
concentration or potential
(dashed) respectively; a is
the radius of the electrode
i =i
D
−
nFDc
S
δ
, (12.51)
η
D
=
RT
nF
ln
c
S
c
B
, (12.52)
η
D
=
RT
nF
ln
i
D
−i
i
D
. (12.53)
Diffusion of oxygen to a corroding metal surface is
frequently the rate-determining step for corrosion pro-
cesses. This is a consequence of its limited dissolution
in water and the presence of thick water layers, which
act as a diffusion barrier. For very thin water layers the
access of oxygen is less hindered and sufficiently fast,
yielding high corrosion rates. This is often the case un-
der atmospheric corrosion. As an estimate i
D
of oxygen
reduction is calculated using the first diffusion law ac-
cording to (12.50) with n = 4 for oxygen reduction to
H
2
O. With a diffusion constant D
O
2
= 10
−5
cm
2
/sthe
thickness of the Nernst diffusion layer δ = 5×10
−3
cm
and a concentration of saturation for oxygen at
25
◦
C c
B
= 2×10
−4
M = 2×10
−7
mol/cm
3
,avalue
of i
D
=1.6×10
−4
A/cm
2
=0.16 mA/cm
2
is obtained.
This value is relatively large and will also disturb
electrochemical studies in the laboratory as a ca-
thodic background current. It may be suppressed
effectively by purging of electrolytes with nitrogen or
argon.
Diffusion may be enhanced by microelectrodes with
a small radius a. According to Fig. 12.20 the diffusion
in front of a hemispherical electrode follows the cur-
rent lines, which follow the direction of its radius. The
concentration gradient is given by the density of the
hemispheres of constant concentration surrounding the
electrode surface (dashed circles). Most variation is lo-
cated close to the surface due to the divergence of the
current lines. According to the hemispherical shape of
Part D 12.2
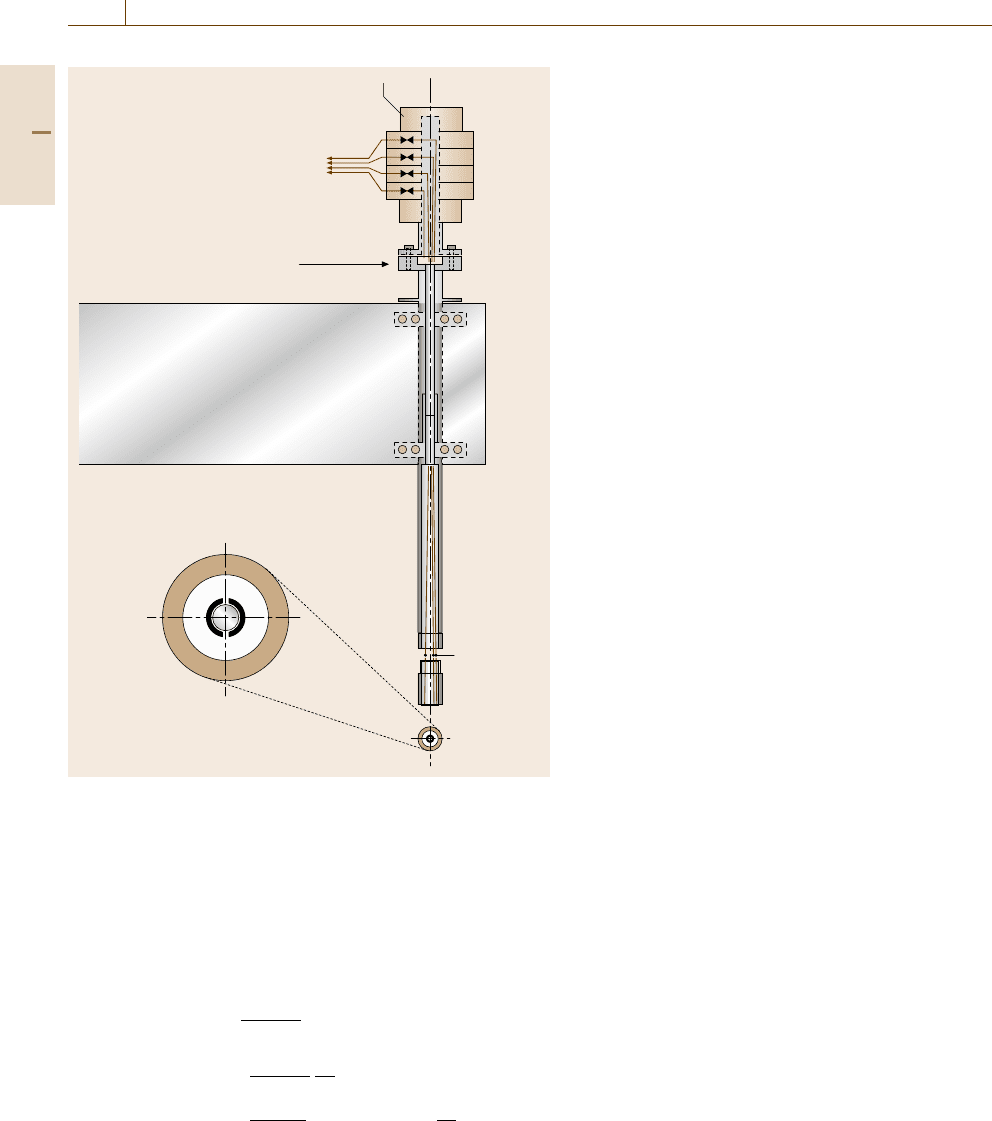
686 Part D Materials Performance Testing
Rotating mercury
contact
Rotator for
split-ring-disc-electrode
Metal disc
Pt-split-ring
Potentiostat
Driving
belt
Contacts
Exchangeable
electrode
Fig. 12.21 Disc rotator with an exchangeable RRDE with a split ring
this transport the current density i(r) decreases with
the distance a +r from the surface according to (12.54)
with the radius a for the metal electrode and the current
density i(a) at its surface. Integration of the first diffu-
sion law (12.55) for these conditions in the range r = 0
to r =∞yields (12.56)fori and the limiting current
density i
D
i(r) =i(a)
a
2
(a +r)
2
, (12.54)
i = nFD
(
a +r
)
2
a
2
dc
dr
, (12.55)
i = nFD
c
S
−c
B
a
; i
D
=−nFD
c
B
a
.
(12.56)
If the radius a becomes small, i
D
may achieve
large values. For c
B
= 1M= 10
−3
mol/cm
3
, n = 1
and D = 5×10
−6
cm
2
/s
1
and an ultramicroelectrode
with a radius a = 1 μm = 10
−4
cm one obtains i
D
=
5A/cm
2
. This value will be even higher for elec-
trodes of nm dimensions. In contrast using (12.50)for
a planar electrode with a well-stirred electrolyte and
a diffusion-layer thickness of δ =5×10
−3
cm, one ob-
tains i
D
= 0.1A/cm
2
. This is one reason why such
small electrodes are used for electroanalytical purposes.
For similar reasons a small radius leads to small ohmic
voltage drops in front of an ultramicroelectrode which
will be discussed in the next section.
Small active areas on a metal surface of this size
may occur during localized corrosion of a passivated
metal surface. In these cases extremely high local corro-
sion current densities may be measured within corrosion
pits of up to several 10 A/cm
2
, whereas the rest of the
surface shows extremely small dissolution currents in
the range of μA/cm
2
. The concentration gradient is
about three times larger in this case due to the con-
cave geometry when compared to a convex hemisphere
(Fig. 12.21b) [12.39]. This more complicated transport
problem has been solved numerically [12.40]. An an-
alytical solution does not exist. Effective hemispherical
transport will delay the accumulation of corrosion prod-
ucts for high dissolution rates, at least for some time
before they precipitate. The precipitation of salt films
will decreases the local current density and change
the shape of growing pits from a polygonal shape to
hemispheres due to the electropolishing effect of the
precipitates [12.29,30, 39].
12.2.11 Rotating Disc Electrode (RDE) and
Rotating Ring-Disc Electrode (RRDE)
Diffusion control of electrode processes has been ap-
plied to various polarographic methods to analyze
qualitatively and quantitatively the composition of elec-
trolytes or the products formed at an electrode surface.
The rotating disc electrode (RDE) and rotating ring-disc
electrode (RRDE) are special arrangements to measure
qualitatively and quantitatively the amount and compo-
sition of corrosion products. A disc that rotates with
moderate speed has a laminar flow of the front elec-
trolyte. Its flow is perpendicular and parallel to the
rotating disc, as shown in Fig. 12.19. On one hand
metal dissolution causes an increase of the concen-
tration of the corrosion products during the outward
flow of the electrolyte film parallel to the surface. On
the other hand the income of fresh electrolyte from
the bulk perpendicular to the surface yields a de-
crease of the concentration. Both effects compensate
Part D 12.2
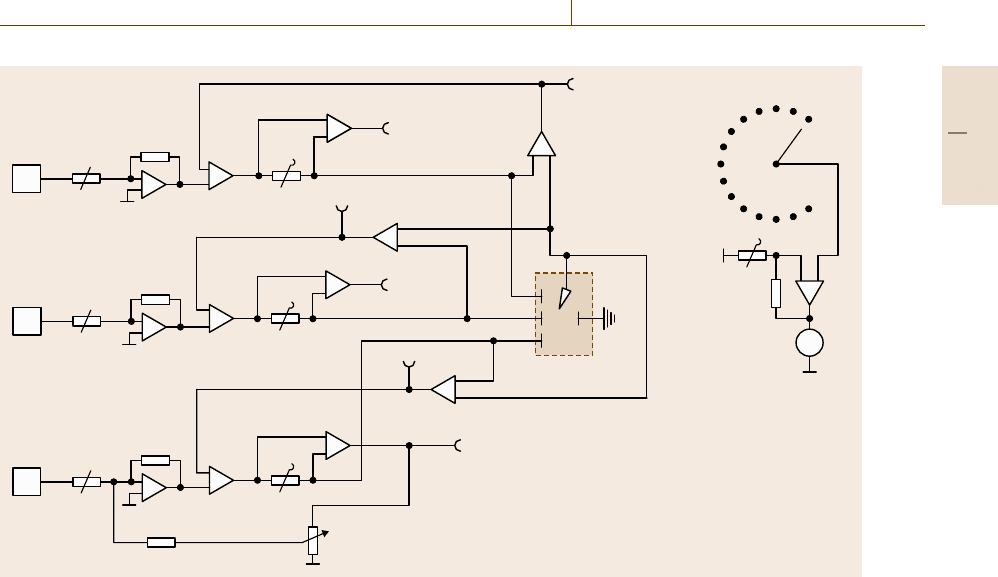
Corrosion 12.2 Conventional Electrochemical Test Methods 687
U
s
WE
1
WE
2
WE
3
Cell
V
Control unit
(zero control
amplifier)
GE
RE
Compensation
of ohmic drop
U
ref 1
U
ref 2
U
ref 3
I
cell 1
I
cell 2
I
cell 3
1
100k
4
7
10
8
9
13
11
100k
Diff
Diff
Diff
Diff
Diff
Diff
Adder
P1
U
s
2
100k
5
14
15
1
12
100k
Adder
P2
U
s
3
100k
6
15
100k
100k
Adder
P3
Fig. 12.22 Tripotentiostat for potentiostatic RRDE studies
each other, so that one may calculate diffusion to the
bulk with a constant thickness of the Nernst diffusion
layer and a constant concentration profile independent
of the location at the disc surface. The quantitative
treatment of this problem yields the Levich equation
(12.57) [12.41] for the thickness of the diffusion layer δ,
with the rotation speed ω =2π f , the frequency f ,
ν =ν
/ρ, i. e. the viscosity ν
divided by the den-
sity ρ of the electrolyte and the diffusion coefficient
D. With ν = 10
−2
cm
2
/sforwater,D =5×10
−6
cm
2
/s
and ω = 63 s
−1
one obtains δ = 1.6×10
−3
cm. Intro-
ducing this value in (12.50) yields with n = 1and
c
B
=10
−3
M for the limiting disc current density of
a species which is consumed at the disc surface
i
D
=300 μA/cm
2
. Another example is i
D
ofarotating
iron disc in 0.5MH
2
SO
4
. With a saturation concentra-
tion of c = 0.56 M for FeSO
4
and its bulk concentration
c
B
=0 one calculates, for all other conditions the same,
i
D
=0.336 A/cm
2
. Continued Fe dissolution causes ac-
cumulation of corrosion products and leads finally to
a precipitated salt film which restricts the dissolution
to the calculated value. This is close to the experi-
mental maximum i
D
=200 mA/cm
2
of dissolution of
a flat iron electrode with moderate agitation of the elec-
trolyte. A better coincidence cannot be expected due to
the difference in hydrodynamic flow between the two
electrodes
δ = 1.61ω
−1/2
ν
1/6
D
1/3
. (12.57)
The RRDE consist of a central disc with a sur-
rounding analytical ring electrode, usually made of
a noble metal such as gold or platinum (Fig. 12.21).
The products of the central disc are transported par-
allel to the electrode surface and finally pass the ring
surface where they undergo an electrochemical pro-
cess with a diffusion-limited maximum current density.
The transfer efficiency N from the disc to the ring
may be calculated from the dimensions of both elec-
trodes [12.42] or may be determined experimentally.
Val ue s o f N > 30% may be achieved easily. The disc
current I
Di
and the ring current I
R
are correlated by
(12.58), which takes into account the number of ex-
changed charges n
Di
and n
R
at both electrodes. With
(12.58) one may calculate I
Di
and the formation of
soluble products at the ring from measured I
R
values.
A reaction well suited to the experimental calibration
of a RRDE and the determination of N is the re-
dox reaction of [Fe(CN)
6
]
3−/4−
with an oxidation of
[Fe(CN)
6
]
4−
at the disc and the related reduction of
Part D 12.2
