Cohen I.M., Kundu P.K. Fluid Mechanics

Подождите немного. Документ загружается.

272 Gravity Waves

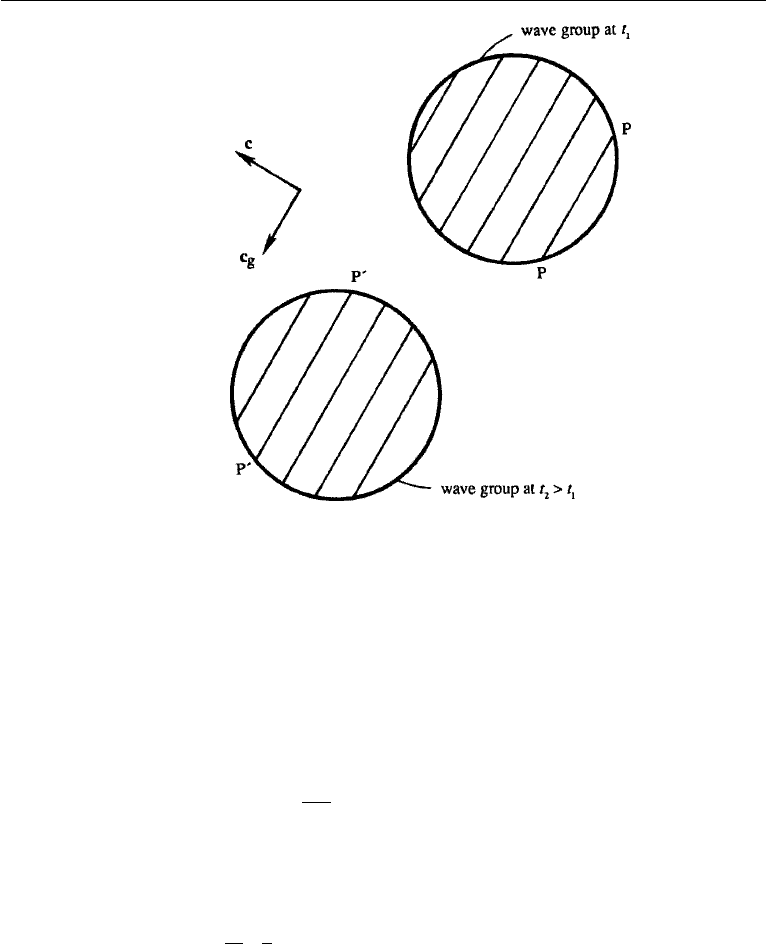
Figure 7.35 Illustration of phase and group propagation in internal waves. Positions of a wave group at

two times are shown. The phase line PP at time t

propagates to P



at t

along four beams that became more vertical as the frequency was increased, which

agrees with cos θ = ω/N.

21. Energy Considerations of Internal Waves in a

Stratiﬁed Fluid

In this section we shall derive the various commonly used expressions for potential

energy of a continuously stratiﬁed ﬂuid, and show that they are equivalent. We then

show that the energy ﬂux



u is c

times the wave energy.

A mechanical energy equation for internal waves can be derived from equa-

tions (7.140)–(7.142) by multiplying the ﬁrst equation by ρ

u, the second by ρ

v, the

third by ρ

w, and summing the results. This gives

∂

∂t



+ v

+ w

)



+ gρ



w + ∇

•



u) = 0. (7.160)

Here the continuity equation has been used to write u∂p



/∂x+v∂p



/∂y+w∂p



/∂z =

∇

•



u), which represents the net work done by pressure forces. Another interpre-

tation is that ∇

•



u) is the divergence of the energy ﬂux p



u, which must change

the wave energy at a point. As the ﬁrst term in equation (7.160) is the rate of change

of kinetic energy, we can anticipate that the second term gρ



w must be the rate of

change of potential energy. This is consistent with the energy principle derived in

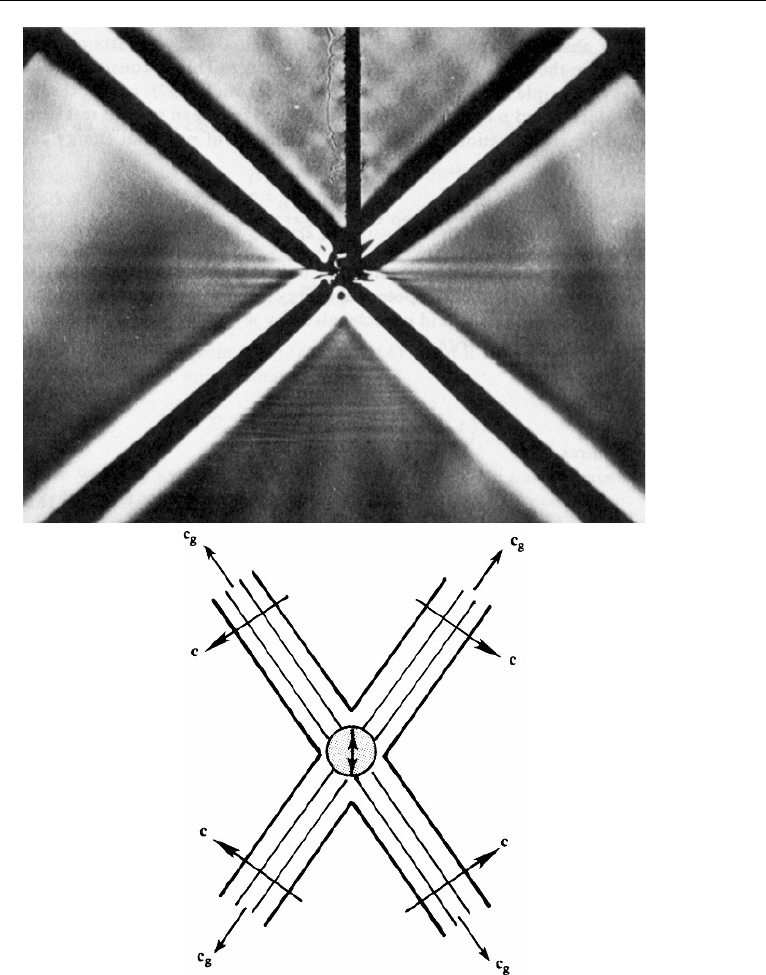
21. Energy Considerations of Internal Waves in a Stratiﬁed Fluid 273

Figure 7.36 Waves generated in a stratiﬁed ﬂuid of uniform buoyancyfrequency N = 1 rad/s. The forcing

agency is a horizontal cylinder, with its axis perpendicular to the plane of the paper, oscillating vertically at

frequency ω = 0.71 rad/s. With ω/N = 0.71 = cos θ , this agrees with the observed angle of θ = 45

◦

made

by the beams with the horizontal. The vertical dark line in the upper half of the photograph is the cylinder

support and should be ignored. The light and dark radial lines represent contours of constant ρ



and are

therefore constant phase lines. The schematic diagram below the photograph shows the directions of c and

for the four beams. Reprinted with the permission of Dr. T. Neil Stevenson, University of Manchester.

274 Gravity Waves

Chapter 4 (see equation (4.62)), except that ρ



and p



replace ρ and p because we

have subtracted the mean state of rest here. Using the density equation (7.143), the

rate of change of potential energy can be written as

∂E

∂t

= gρ



w =

∂

∂t



2

2ρ



, (7.161)

which shows that the potential energy per unit volume must be the positive quan-

tity E

= g

2

/2ρ

. The potential energy can also be expressed in terms of the

displacement ζ of a ﬂuid particle, given by w = ∂ζ /∂t. Using the density equation

(7.143), we can write

∂ρ



∂t

∂ζ

∂t

which requires that



. (7.162)

The potential energy per unit volume is therefore

2

2ρ

. (7.163)

This expression is consistent with our previous result from equation (7.106) for

two inﬁnitely deep ﬂuids, for which the average potential energy of the entire water

column per unit horizontal area was shown to be

(ρ

− ρ

)ga

, (7.164)

where the interface displacement is of the form ζ = a cos(kx −ωt) and (ρ

−ρ

)is

the density discontinuity. To see the consistency, we shall symbolically represent the

buoyancy frequency of a density discontinuity at z = 0as

=−

d ¯ρ

(ρ

− ρ

)δ(z), (7.165)

where δ(z) is the Dirac delta function. (As with other relations involving the delta

function, equation (7.165) is valid in the integral sense, that is, the integral (across the

origin) of the last two terms is equal because



δ(z) dz = 1.) Using equation (7.165),

a vertical integral of equation (7.163), coupled with horizontal averaging over a wave-

length, gives equation (7.164). Note that for surface or interfacial waves E

and E

represent kinetic and potential energies of the entire water column, per unit horizontal

area. In a continuously stratiﬁed ﬂuid, they represent energies per unit volume.

We shall now demonstrate that the average kinetic and potential energies are

equal for internal wave motion. Substitute periodic solutions

[u, w, p



,ρ



]=[ˆu, ˆw, ˆp, ˆρ]e

i(kx+mz−ωt)

21. Energy Considerations of Internal Waves in a Stratiﬁed Fluid 275

Then all variables can be expressed in terms of w:



=−

ωmρ

ˆwe

i(kx+mz−ωt)



ωg

ˆwe

i(kx+mz−ωt)

u =−

ˆwe

i(kx+mz−ωt)

(7.166)

where p



is derived from equation (7.145), ρ



from equation (7.143), and u from

equation (7.140). The average kinetic energy per unit volume is therefore

+ w

) =



+ 1



ˆw

, (7.167)

where we have used the fact that the average of cos

x over a wavelength is 1/2. The

average potential energy per unit volume is

2

2ρ

4ω

ˆw

, (7.168)

where we have used

2

=ˆw

/2ω

, found from equation (7.166) after taking

its real part. Use of the dispersion relation ω

= k

/(k

+ m

) shows that

= E

, (7.169)

which is a general result for small oscillations of a conservative system without

Coriolis forces. The total wave energy is

E = E

+ E



+ 1



ˆw

. (7.170)

Last, we shall show that c

times the wave energy equals the energy ﬂux. The

average energy ﬂux across a unit area can be found from equation (7.166):

F =



u = i



u + i



w =

ωm ˆw



− i



. (7.171)

Using equations (7.157) and (7.170), group velocity times wave energy is

E =

m − i





+ 1



ˆw



which reduces to equation (7.171) on using the dispersion relation (7.151). It follows

that

F = c

(7.172)

276 Gravity Waves

This result also holds for surface or interfacial gravity waves. However, in that case

F represents the ﬂux per unit width perpendicular to the propagation direction (inte-

grated over the entire depth), and E represents the energy per unit horizontal area. In

equation (7.172), on the other hand, F is the ﬂux per unit area, and E is the energy

per unit volume.

Exercises

1. Consider stationary surface gravity waves in a rectangular container of length

L and breadth b, containing water of undisturbed depth H . Show that the velocity

potential

φ = A cos(mπ x/L) cos(nπy/b)cosh k(z + H)e

−iωt

satisﬁes ∇

φ = 0 and the wall boundary conditions, if

(mπ/L)

+ (nπ/b)

= k

Here m and n are integers. To satisfy the free surface boundary condition, show that

the allowable frequencies must be

= gk tanh kH.

[Hint: combine the two boundary conditions (7.27) and (7.32) into a single equation

∂

φ/∂t

=−g∂φ/∂zat z = 0.]

2. This is a continuation of Exercise 1. A lake has the following dimensions

L = 30 km b = 2km H = 100 m.

Suppose the relaxation of wind sets up the mode m = 1 and n = 0. Show that the

period of the oscillation is 31.7 min.

3. Show that the group velocity of pure capillary waves in deep water, for which

the gravitational effects are negligible, is

4. Plot the group velocity of surface gravity waves, including surface tension

σ , as a function of λ. Assuming deep water, show that the group velocity is



1 + 3σk

/ρg



1 + σk

/ρg

Show that this becomes minimum at a wavenumber given by

σk

ρg

√

− 1.

For water at 20

◦

C (ρ = 1000 kg/m

and σ = 0.074 N/m), verify that c

g min

17.8 cm/s.

Literature Cited 277

5. A thermocline is a thin layer in the upper ocean across which temperature

and, consequently, density change rapidly. Suppose the thermocline in a very deep

ocean is at a depth of 100 m from the ocean surface, and that the temperature drops

across it from 30 to 20

◦

C. Show that the reduced gravity is g



= 0.025 m/s

Neglecting Coriolis effects, show that the speed of propagation of long gravity waves

on such a thermocline is 1.58 m/s.

6. Consider internal waves in a continuously stratiﬁed ﬂuid of buoyancy fre-

quency N = 0.02 s

−1

and average density 800 kg/m

. What is the direction of ray

paths if the frequency of oscillation is ω = 0.01 s

−1

? Find the energy ﬂux per unit

area if the amplitude of vertical velocity is ˆw = 1 cm/s and the horizontal wavelength

is π meters.

7. Consider internal waves at a density interface between two inﬁnitely deep

ﬂuids. Using the expressions given in Section 15, show that the average kinetic energy

per unit horizontal area is E

= (ρ

−ρ

)ga

/4. This result was quoted but not proved

in Section 15.

8. Consider waves in a ﬁnite layer overlying an inﬁnitely deep ﬂuid, discussed

in Section 16. Using the constants given in equations (7.116)–(7.119), prove the

dispersion relation (7.120).

9. Solve the equation governing spherical waves ∂

p/∂t

= (c

)(∂/∂r)

∂p/∂r) subject to the initial conditions: p(r, 0) = e

−r

, (∂p/∂t)(r, 0) = 0.

Literature Cited

Gill, A. (1982). Atmosphere–Ocean Dynamics, New York: Academic Press.

Kinsman, B. (1965). Wind Waves, Englewood Cliffs, New Jersey: Prentice-Hall.

LeBlond, P. H. and L. A. Mysak (1978). Waves in the Ocean, Amsterdam: Elsevier Scientiﬁc Publishing.

Liepmann, H. W. and A. Roshko (1957). Elements of Gasdynamics, New York: Wiley.

Lighthill, M. J. (1978). Waves in Fluids, London: Cambridge University Press.

Phillips, O. M. (1977). The Dynamics of the Upper Ocean, London: Cambridge University Press.

Turner, J. S. (1973). Buoyancy Effects in Fluids, London: Cambridge University Press.

Whitham, G. B. (1974). Linear and Nonlinear Waves, New York: Wiley.

This page intentionally left blank

Chapter 8

Dynamic Similarity

1. Introduction ..................... 279

2. Nondimensional Parameters

Determined from Differential

Equations ..................... 280

3. Dimensional Matrix ............. 284

4. Buckingham’s Pi Theorem ....... 285

5. Nondimensional Parameters and

Dynamic Similarity .............. 287

Prediction of Flow Behavior from

Dimensional Considerations . . . 289

6. Comments on Model Testing...... 290

Example 8.1 .................... 290

7. Signiﬁcance of Common

Nondimensional Parameters ..... 292

Reynolds Number ............... 292

Froude Number ................. 292

Internal Froude Number ......... 292

Richardson Number ............. 293

Mach Number ................... 293

Prandtl Number ................. 294

Exercises ........................ 294

Literature Cited .................. 294

Supplemental Reading ............ 294

1. Introduction

Two ﬂows having different values of length scales, ﬂow speeds, or ﬂuid properties

can apparently be different but still “dynamically similar”. Exactly what is meant

by dynamic similarity will be explained later in this chapter. At this point it is only

necessary to know that in a class of dynamically similar ﬂows we can predict ﬂow

properties if we have experimental data on one of them. In this chapter, we shall

determine circumstances under which two ﬂows can be dynamically similar to one

another. We shall see that equality of certain relevant nondimensional parameters is

a requirement for dynamic similarity. What these nondimensional parameters should

be depends on the nature of the problem. For example, one nondimensional parameter

must involve the ﬂuid viscosity if the viscous effects are important in the problem.

The principle of dynamic similarity is at the heart of experimental ﬂuid

mechanics, in which the data should be uniﬁed and presented in terms of nondi-

mensional parameters. The concept of similarity is also indispensable for designing

models in which tests can be conducted for predicting ﬂow properties of full-scale

objects such as aircraft, submarines, and dams. An understanding of dynamic simi-

larity is also important in theoretical ﬂuid mechanics, especially when simpliﬁcations

279

DOI: 10.1016/B978-0-12-381399-2.50008-3

280 Dynamic Similarity

are to be made. Under various limiting situations certain variables can be eliminated

from our consideration, resulting in very useful relationships in which only the con-

stants need to be determined from experiments. Such a procedure is used extensively

in turbulence theory, and leads, for example, to the well-known K

−5/3

spectral law

discussed in Chapter 13. Analogous arguments (applied to a different problem) are

presented in Section 5 of the present chapter.

Nondimensional parameters for a problem can be determined in two ways. They

can be deduced directly from the governing differential equations if these equations

are known; this method is illustrated in the next section. If, on the other hand, the

governing differential equations are unknown, then the nondimensional parameters

can be determined by performing a simple dimensional analysis on the variables

involved. This method is illustrated in Section 4.

The formulation of all problems in ﬂuid mechanics is in terms of the conservation

laws (mass, momentum, and energy), constitutive equations and equations of state

to deﬁne the ﬂuid, and boundary conditions to specify the problem. Most often, the

conservation laws are written as partial differential equations and the conservation

of momentum and energy may include the constitutive equations for stress and heat

ﬂux, respectively. Each term in the various equations has certain dimensions in terms

of units of measurements. Of course, all of the terms in any given equation must have

the same dimensions. Now, dimensions or units of measurement are human con-

structs for our convenience. No system of units has any inherent superiority over any

other, despite the fact that in this text we exhibit a preference for the units ordained

by Napoleon Bonaparte (of France) over those ordained by King Henry VIII (of

England). The point here is that any physical problem must be expressible in com-

pletely dimensionless form. Moreover, the parameters used to render the dependent

and independent variables dimensionless must appear in the equations or boundary

conditions. One cannot deﬁne “reference” quantities that do not appear in the prob-

lem; spurious dimensionless parameters will be the result. If the procedure is done

properly, there will be a reduction in the parametric dependence of the formulation,

generally by the number of independent units. This is described in Sections 3 and 4

in this chapter. The parametric reduction is called a similitude. Similitudes greatly

facilitate correlation of experimental data. In Chapter 9 we will encounter a situation

in which there are no naturally occurring scales for length or time that can be used

to render the formulation of a particular problem dimensionless. As the axiom that

a dimensionless formulation is a physical necessity still holds, we must look for a

dimensionless combination of the independent variables. This results in a contraction

of the dimensionality of the space required for the solution, that is, a reduction by

one in the number of independent varibles. Such a reduction is called a similarity and

results in what is called a similarity solution.

2. Nondimensional Parameters Determined from

Differential Equations

To illustrate the method of determining nondimensional parameters from the gov-

erning differential equations, consider a ﬂow in which both viscosity and gravity

are important. An example of such a ﬂow is the motion of a ship, where the drag

2. Nondimensional Parameters Determined from Differential Equations 281

experienced is caused both by the generation of surface waves and by friction on the

surface of the hull. All other effects such as surface tension and compressibility are

neglected. The governing differential equation is the Navier–Stokes equation

∂w

∂t

∂w

∂x

∂w

∂y

∂w

∂z

=−

∂p

∂z

−g +



∂

∂x

∂

∂y

∂

∂z



, (8.1)

and two other equations for u and v. The equation can be nondimensionalized by

deﬁning a characteristic length scale l and a characteristic velocity scale U . In the

present problem we can take l to be the length of the ship at the waterline and U

to be the free-stream velocity at a large distance from the ship (Figure 8.1). The

choice of these scales is dictated by their appearance in the boundary conditions; U

is the boundary condition on the variable u and l occurs in the shape function of

the ship hull. Dynamic similarity requires that the ﬂows have geometric similarity

of the boundaries, so that all characteristic lengths are proportional; for example,

in Figure 8.1 we must have d/l = d

. Dynamic similarity also requires that the

ﬂows should be kinematically similar, that is, they should have geometrically similar

streamlines. The velocities at the same relative location are therefore proportional;

if the velocity at point P in Figure 8.1a is U/2, then the velocity at the correspond-

ing point P

in Figure 8.1b must be U

/2. All length and velocity scales are then

proportional in a class of dynamically similar ﬂows. (Alternatively, we could take

the characteristic length to be the depth d of the hull under water. Such a choice is,

however, unconventional.) Moreover, a choice of l as the length of the ship makes

the nondimensional distances of interest (that is, the magnitude of x/l in the region

around the ship) of order one. Similarly, a choice of U as the free-stream velocity

makes the maximum value of the nondimensional velocity u/U of order one. For

reasons that will become more apparent in the later chapters, it is of value to have all

dimensionless variables of ﬁnite order. Approximations may then be based on any

extreme size of the dimensionless parameters that will preface some of the terms.

Accordingly, we introduce the following nondimensional variables, denoted by

primes:



p − p

∞

ρU

(8.2)

Figure 8.1 Two geometrically similar ships.