Canale L.C.F., Mesquita R.A., Totten G.E. Failure Analysis of Heat Treated Steel Components

Подождите немного. Документ загружается.

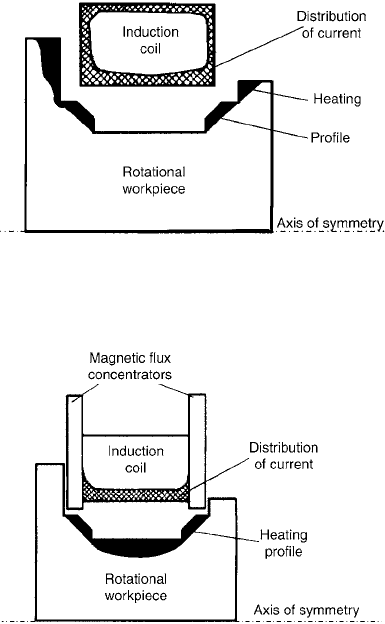
 By placing the conductor into the magnetic

ﬂux concentrator made of powder metals

based on iron, nickel, cobalt, and other

powder materials, it is possible to achieve a

higher density of the magnetic ﬁeld

(Fig. 23c).

The concentrated magnetic ﬁeld results in

localized heating on only those areas that are

meant to be hardened. The magnetic ﬂux con-

centrator surrounds the conductor, so that the

current density on the conductor surface is

redistributed, as is the heating of the workpiece

material.

The effects of the magnetic ﬂux concentrator

depend on:

 Workpiece material and shape of the local

area to which heat is applied

 Workpiece material and concentrator shape

The current density in this case is the highest

in point “A” of the conductor and is considerably

higher than in point “B”. This results in effective

local heating, where the length of the heated area

is only slightly greater than the width of the con-

ductor, but, due to the high density of the mag-

netic ﬂux, a considerably greater depth of the

heated area is achieved.

As for concentrator material, different appli-

cations require the use of different materials.

The material for the concentrator must be chosen

after considering several factors:

 Relative magnetic permeability

 Magnetic reluctance

 Flux density in saturation

 Losses under magnetization

 Resistance to high temperatures

 Cooling abilities

 Resistance to cooling effects of ﬁre extin-

guishers

 Good machinability

 Adjustment to different shapes and sizes of

coils

 Ease of assembly and disassembly

 Manufacturing costs, depending on the kind

of material, induction heating parameters,

and geometry features between the con-

centrator and the workpiece

Figure 24 shows a local surface area with a

complex shape that is induction heated with a

single coil. Current density is in the inductor,

which results from the position of the inductor

with respect to the workpiece. This results in

differences in the current density, which are

reﬂected in the different heating/workpiece

depth proﬁles created by the differences in the

power density distribution (Ref 19, 42).

Figure 25 shows the same area and shape, but

heating was performed with a magnetic ﬂux

concentrator (Ref 19, 42). This is placed on the

left and right sides of the surrounding coil, which

contributes to higher concentration of the mag-

netic ﬂux to prevent heating of the sides of the

workpiece at this place. A redistribution of

power density took place, and a desirable local

heating proﬁle of the workpiece was achieved.

Advantages offered by the magnetic ﬂux

concentrator are:

 Smaller consumption of power

 Improved efﬁciency of heating

 Better exploitation of equipment due to

shorter heating times

 More selective heating of the workpiece

areas

Fig. 24

Heating proﬁle on rotational workpiece with

induction coil. Source: Ref 42

Fig. 25

Heating proﬁle on rotational workpiece with ﬂux

concentrator. Source: Ref 42

438 / Failure Analysis of Heat Treated Steel Components

Name ///sr-nova/Dclabs_wip/Failure_Analysis/5113_417-501.pdf/Chap_13/ 18/8/2008 4:00PM Plate # 0 pg 438

 Achievement of desirable heating proﬁles of

hardened layer proﬁles

 High repeatability of the procedure in terms

of hardened proﬁle size and hardened layer

microstructure

 Efﬁcient protection from unwanted heating

of adjacent areas and successful prevention

of reheating and softening the already hard-

ened layers

 Elimination of detrimental effects on the

operator’s health due to exposure to the

magnetic ﬁeld

 Improved operation lifetime of the heating

equipment and higher productivity

 Less workpiece distortion and lower costs in

straightening and/or ﬁnal grinding sub-

sequent to hardening

 Reduction of the quantity of rejected parts in

terms of required size and shape of the hard-

ened layer and workpiece distortion and

cracking

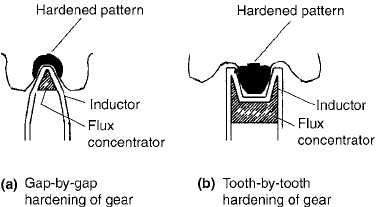
Figure 26 shows two hardening procedures

for tooth proﬁles of gear wheels using appro-

priate induction coils with a ferritic core con-

centrating the magnetic ﬁeld (Ref 19).

The ﬁrst procedure is known as gap-by-gap

hardening (Fig. 26a), because the induction coil

with the ferritic core is moved in the tooth space

so that a suitable gap between a tooth proﬁle and

the inductor is provided. The advantage of using

magnetic ﬂux concentrators rather than the

conventional coil is that, with the same energy

input, heating time is shortened. Unfortunately,

the use of concentrators also shows some deﬁ-

ciencies, since the transition zone between the

hardened and nonhardened microstructures,

which is very important for hardening, is lost due

to rapid heating. This results in less favorable

residual-stress and microhardness proﬁles of the

hardened layer. Thus, a greater risk of failure

is incurred at the point where teeth or other

machine parts are most strongly loaded, which is

at the transition from the hardened subsurface to

the nonhardened core, where an increased stress

concentration will occur. Such heating circum-

stances may be avoided by choosing lower-

energy inputs or power densities. In this case,

however, the case depth is more difﬁcult to con-

trol. If a hardened-and-tempered microstructure

is to be provided at the tooth inside as well,

heating with two frequencies is applied. The

hardened-and-tempered microstructure may be

accomplished with only one frequency in heat

treatment of smaller gear wheels when the induc-

tion coil encompasses the entire gear wheel.

The second procedure is tooth-by-tooth

heating (Fig. 26b). In this case, the induction coil

is moved against a tooth so that an appropriate

gap is provided between the concentrator and the

surface of both ﬂank proﬁles of the same tooth.

The difference between the two techniques is

that in gap-by-gap heating, two adjacent tooth

proﬁles and the root section of a gear wheel are

heated, whereas in tooth-by-tooth heating the

entire gear tooth is heated. Thus, in the ﬁrst

example, only hardened gear wheel tooth pro-

ﬁles are obtained, whereas in the second ex-

ample, hardened tooth proﬁles of the gear wheel

and quenched and tempered tooth inside are

obtained.

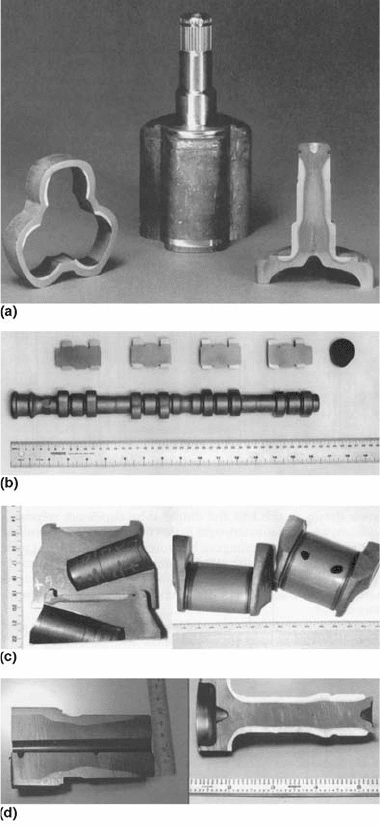
Figures 27(a to d) show various automobile

parts that were induction surface hardened using

the single-shot or scan-hardening technique (Ref

19). They were prepared for macroscopic and

microscopic examinations of the hardened layer.

The segments of the individual specimens of

various characteristic and exacting automobile

parts after grinding, polishing, and macroetch-

ing permit identiﬁcation of the proﬁle of the

hardened pattern, for example, in the cross-

sectional and longitudinal direction of the part,

respectively, as well as an analysis of the micro-

structure and microhardness. Each ﬁgure also

indicates which technique of heating, that is,

single-shot hardening or scan hardening, and

which heating conditions (P, f ), using single-

turn or multiple-turn induction coils, were

employed.

The quality of the surface-hardening process

can often be efﬁciently assessed by measuring

the hardened-layer depth at different locations

on the individual parts. If the achieved depth is

very uniform, regardless of the location of mea-

surement, then the part was not subjected to

distortion. A sufﬁciently high initial hardening

Fig. 26

Gap-by-gap and tooth-by-tooth induction hardening

of gear wheels. Source: Ref 19

Induction Hardening / 439

Name ///sr-nova/Dclabs_wip/Failure_Analysis/5113_417-501.pdf/Chap_13/ 18/8/2008 4:00PM Plate # 0 pg 439

temperature provides an adequate through-depth

microhardness of the hardened proﬁle. The

analysis is considered a destructive method,

because the part must be cut in the longitudinal

and transverse directions respectively, so that

the information required on the hardened pattern

may be obtained. Such methods are appropriate

only for periodical control, particularly statis-

tical control with periodical sampling. Based on

the results obtained for the characteristics of the

selected hardened pattern, the quality of an

article is conﬁrmed or accepted and uninterrup-

ted production provided. These types of non-

destructive testing are long-standing; therefore,

nondestructive methods of testing the material

condition after induction hardening are grad-

ually being introduced. Of all the methods, mag-

netic and magnetic-induction methods have

established themselves because they are very

fast, reliable, and provide reproducible results.

The nondestructive methods of testing these

parts allow on-line supervision of part quality,

since, due to the speed of testing, all parts may be

tested, which is a general tendency in mass

production today.

Conditions in Induction Heating and

Quenching of Machine Parts

Heating of workpieces is done so that a

magnetic ﬁeld is created in the inductor, which is

connected to a high-frequency generator. When

a ferromagnetic material or workpiece is intro-

duced into a magnetic ﬁeld, eddy currents are

induced. The distribution of eddy currents in the

workpiece is speciﬁc, their density being highest

on the surface and decreasing toward the inside.

This phenomenon is known as the surface effect

or skin effect. Due to resistance offered by the

workpiece material, heating takes place mainly

in the thin surface layer, whereas the inner

core remains cold or is only slightly heated

(low-mass workpieces).

The depth of current penetration depends on

workpiece permeability, resistivity, and the

alternating-current frequency. Because the ﬁrst

two factors vary comparatively little, the great-

est variable is frequency. Depth of current

penetration decreases as frequency increases.

High-frequency current generally is used when

shallow heating is desired; intermediate and low

frequencies are used in applications requiring

deeper heating.

Most induction surface-hardening applica-

tions require comparatively high power den-

sities and short heating cycles to restrict heating

Fig. 27

(a) Single-shot inductors used for both track (lobes)

and shaft of this automotive component. The part is

sectioned and acid etched to show the hardness pattern. All tracks

are hardened at the same time using 250 kW/30 kHz; the stem is

hardened using 135 kW/10 kHz. (b) Automotive right and left

cam shafts that have been selectively induction surface hardened.

The cam shaft was forged, heat treated, then ﬁnal ground. No

premachining was necessary. The equipment used a dual-spindle

transfer mechanism; the coil was a four-turn coil that heated four

lobes per spindle at a time. Power was applied for each set of four

lobes: 150 kW, 10 kHz. The result is a 4.2 mm deep case depth

in the base circle of the cam. (c) Hardness patterns on carbon steel

crankshaft journals resulting from the stationary induction-

hardening process. (d) (Left) An unacceptable nonuniform hard-

ness pattern due to the nonuniformity of the workpiece, scanned

with a single-turn inductor. (Right) An acceptable hardness

proﬁle achieved with a single-shot inductor. Source: Ref 19.

Courtesy of Inductoheat, Inc.

440 / Failure Analysis of Heat Treated Steel Components

Name ///sr-nova/Dclabs_wip/Failure_Analysis/5113_417-501.pdf/Chap_13/ 18/8/2008 4:00PM Plate # 0 pg 440

to the surface area. The principal metallurgical

advantages that may be obtained by surface

hardening with induction are the same as for

ﬂame hardening. The drop in magnetic perme-

ability of steels depends on the temperature line,

, where steel transforms from magnetic into

nonmagnetic ferrite. The larger the effect of the

magnetic permeability change on the tempera-

ture line, T

, the smaller the carbon content in

the steel (the larger the proportion of ferrite in

the steel) and vice versa. Due to rapid heating,

phase transformation moves upward toward

higher temperatures. The temperature-time

curves of heating along the depth of a cylindrical

component depend on the kinetics of the mag-

netic transformation, T

, and the effects of other

phase transformations during induction heating.

A thickness of 1.0 to 1.5 mm is reached with a

medium-frequency current. The temperature-

time variation over the cross section of the steel

workpiece is a function of the following factors:

 Penetration depth of eddy currents

 Heat conduction of the material

 Heating rate of the surface

 Initial temperature of the surface

 Size and shape of the workpiece

The depth of penetration of the heat is gov-

erned mainly by the power and frequency em-

ployed. The normal power density is 0.1 to 2 kW/

of the heated surface. The relationship

between depth of penetration and frequency can

be calculated approximately by using simpliﬁed

expressions, which are valid for the temperature

rise in steel up to the hardening temperature

(Ref 16):

ﬃﬃﬃ

cold state (20



500

ﬃﬃﬃ

hot state (800



where d

is the depth of penetration in the cold

state, measured in millimeters; d

is the depth

of penetration in the hot state, measured in

millimeters; and f is the frequency, measured in

hertz.

Due to heat conduction in the material during

heating, the overall depth of penetration is larger.

It is possible to calculate the additional penetra-

tion due to heat conduction from the expression:

=0:2

ﬃﬃ

where t is time, measured in seconds; and d

the depth of penetration for heat conduction,

measured in millimeters.

The total depth of penetration is obviously

= d

. It should be stressed that these

expressions give only a rough estimate of the

depth of penetration, and they have been inclu-

ded here only to show the fundamental effects of

frequency and time.

In ﬂame heating, the temperature achieved on

the surface at equal energy input is considerably

higher than in induction heating, the overheating

and the hardened layer thickness being depen-

dent on the heat conduction of the workpiece

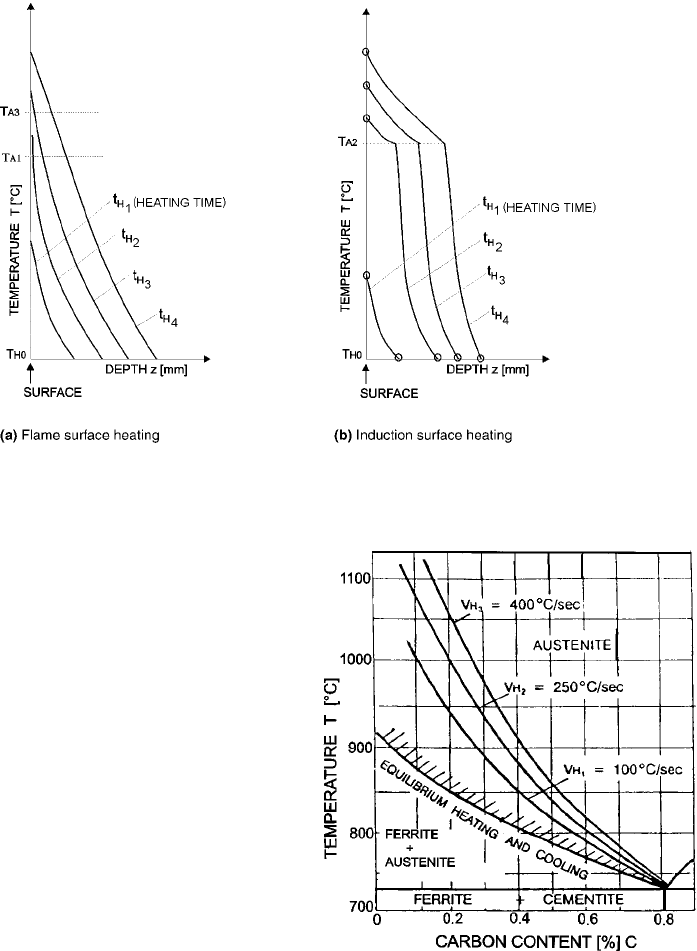
material. Figure 28(a) shows the temperature-

time variation over the cross section of the

workpiece in ﬂame heating (Ref 15). Charac-

teristic of this variation is that the temperature

rapidly changes with time, and therefore, the

conditions for the formation of a homogeneous

austenitic microstructure are not fulﬁlled.

Figure 28(b) shows the temperature–time

variation over the cross section of the workpiece

in induction heating. The temperature variation

is very similar to that in ﬂame heating up to

magnetic transformation, that is, to line A

.At

temperatures higher than line A

, eddy currents

grow characteristically, and the rate of heating

decreases sharply. This slows down the heating

above temperature line A

. A reduced rate of

heating on the surface provides the conditions

for faster heating into the depth of the work-

piece. This ﬁgure shows that a relatively thin

layer is heated up, but the layer has a rather

homogeneous austenitic microstructure. The

temperature-time variation on the workpiece

cross section, or the temperature ﬁeld, depends

on the workpiece size and shape. Thus, in heavy-

mass workpieces, faster heat abduction into the

remaining cold part of the workpiece is achieved,

and that is why the actual variation of tempera-

ture over the cross section is steeper. This means

that in heavy-mass workpieces, a higher surface

temperature than in low-mass workpieces must

be ensured to grant the same penetration depth.

The microstructural changes in induction

hardening depend to a large extent on the rate of

heating and subsequent cooling. The rates of

heating range from one to a few seconds, which

means that the diffusion processes may become

jeopardized. In steel, transformation of pearlite

into austenite takes place in induction heating at

almost the same temperature as in conventional

heating. In subeutectic steels suitable for surface

hardening, it is important that the induction

Induction Hardening / 441

Name ///sr-nova/Dclabs_wip/Failure_Analysis/5113_417-501.pdf/Chap_13/ 18/8/2008 4:00PM Plate # 0 pg 441

heating procedure ensures enough time for the

diffusion of carbon for transformation of ferrite

into homogeneous austenite (Ref 2, 16, 27, 29,

30).

Figure 29 shows the temperature line of

through hardening of subeutectic steels with

different carbon contents versus different heat-

ing rates (Ref 15, 27). The graph shows that

austenitization is clearly inﬂuenced by the

heating rate, especially when carbon con-

centrations are low to medium. Likewise, in the

case of rapid heating for through hardening, a

considerably higher temperature is needed than

for normal hardening. Thus, in surface hard-

ening carbon steels, it is difﬁcult to ensure

enough homogeneous martensite, whereas in the

transition temperature range (T

to T

), a

higher homogenization of martensite is achieved

but with a presence of ferrite. The proportion of

ferrite in the transition temperature range is thus

higher with faster surface heating and smaller

carbon content in the steel.

In surface hardening alloyed steels, a better

homogenization of the austenite is achieved in

the heating phase, and when quenching is com-

pleted, a very homogeneous martensite with a

uniform microhardness along the depth of the

hardened layer is derived. Unfortunately, there

is a transition temperature range with a smaller

ferrite content, which causes a sharp drop in

hardness in the transition area with a hardened

and nonhardened microstructure.

A very important heating requirement in the

hardening procedure, besides the austenitization

temperature, is the time necessary for austeniti-

zation, since both of these heating parameters

Fig. 28

Temperature proﬁle across the workpiece diameter in (a) ﬂame surface heating and (b) induction surface heating. Source:

Ref 15

Fig. 29

Inﬂuence of induction surface heating rate on hard-

ening temperature for subeutectic steels. Source:

Ref 15, 27

442 / Failure Analysis of Heat Treated Steel Components

Name ///sr-nova/Dclabs_wip/Failure_Analysis/5113_417-501.pdf/Chap_13/ 18/8/2008 4:00PM Plate # 0 pg 442

affect the size of austenitic grains. Austenitic

grain size is, on the other hand, dependent on the

martensite formed subsequent to quenching and

is reﬂected in the toughness of the surface layer.

That is why heat treatment conditions are sought

that would ensure the ﬁnest and most homo-

geneous austenitic microstructure in the heating

and overheating phases, leading to the formation

of very ﬁne martensite with the highest possible

toughness of the hardened layer after quenching

(Ref 27, 29). This microstructural condition and

the achieved mechanical properties ensure good

wear resistance of machine components (gears)

and their good response under dynamic loads.

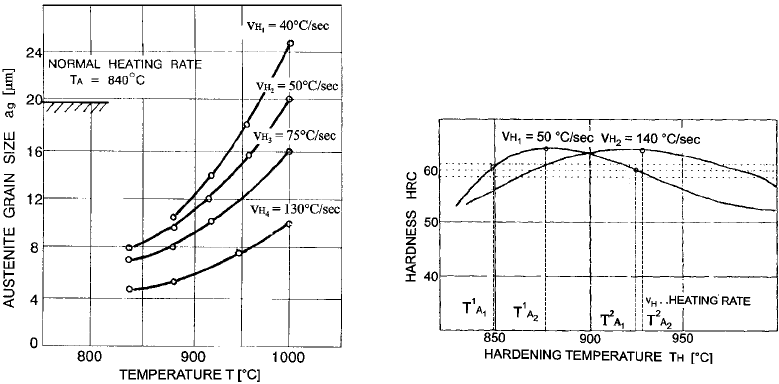
Figure 30 shows the growth of austenitic

grains for different rates of induction heating for

a steel with 0.4% C that can be used for induc-

tion hardening (Ref 15, 27). With increasing

rates of heating, the austenitic transformation

moves toward higher temperatures. A higher

heating temperature creates a higher formation

rate of austenitic crystal and therefore ﬁne grains

of austenite. Subsequent to quenching, these ﬁne

grains of austenite ensure a very ﬁne martensitic

microstructure.

Figure 31 shows the relationship between

the hardened layer hardness and the heating

rate and temperature for a steel with 0.45% C

(Ref 15, 27).

For each steel, there exists a certain tem-

perature range after hardening that yields the

best microstructural condition and thus the best

properties. With higher rates of heating, this

range moves to higher temperatures. This means

that quenching from lower temperatures leads to

imperfect hardening, while higher temperatures

yield medium or rough needles of martensite.

The heating rate of 50



C/s is sufﬁcient to reach

a hardening temperature range between 850 and

925



C. In the case of a higher-energy input that

heats up the thin surface layer with a rate higher

than 140



C/s, the required temperature range

becomes 870 to 970



C. In both cases, a surface

hardness of 60 HRC is reached subsequent to

quenching.

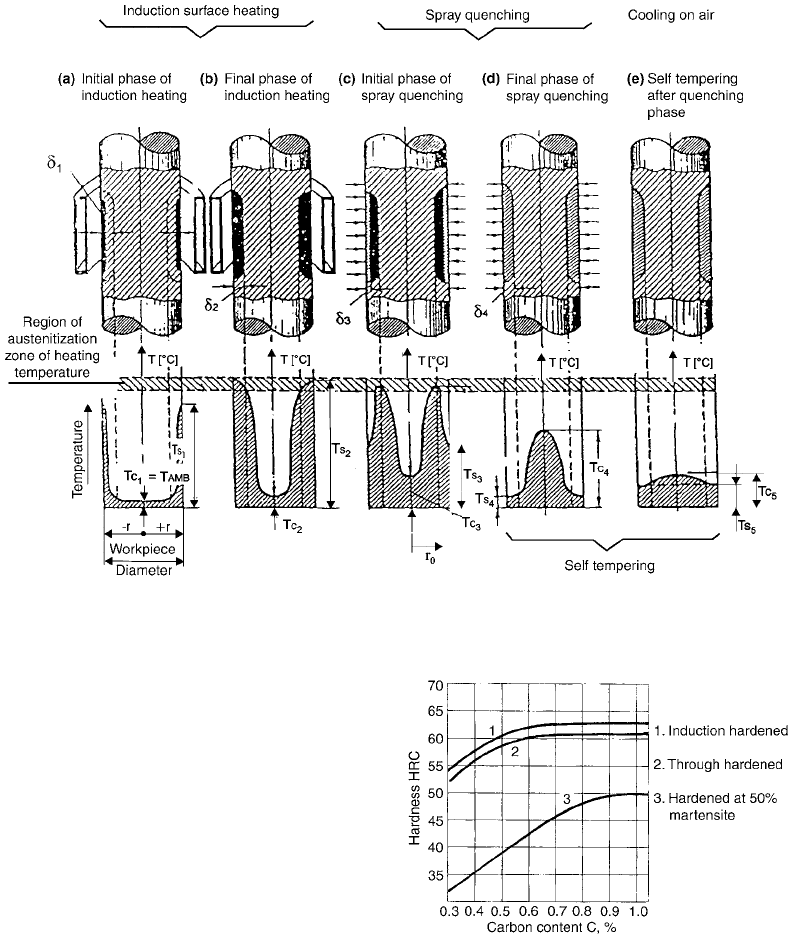
Figure 32(a–e) shows the entire process of

induction hardening a cylindrical component

with a small diameter or cross section, which,

subsequent to hardening, leads to self-tempering

(Ref 15, 27).

Figure 32(a and b) show induction heating of

a thin surface layer to the austenitic temperature

range, ensuring, a sufﬁcient thickness of the

austenite layer d

subsequent to quenching. The

process of quenching or self-tempering is shown

in Fig. 32(c and d), where, due to heat conduc-

tion into the remaining cold part of the work-

piece, the temperature on the workpiece surface

layer increases, and thus, the thickness of the

austenite layer increased to d

or d

, respec-

tively.

Due to a small workpiece cross section or low

workpiece mass causing heat conduction, the

temperature in the middle of the workpiece rose

to a point corresponding to the tempering tem-

perature of the given material. Since the process

of tempering takes place in the workpiece with

the available heat needed for austenitization,

self-tempering of the workpiece is indicated

(Ref 27, 31, 32, 43).

Speciﬁc properties of the hardened layer can

be described by analyzing the microstructure

Fig. 30

Inﬂuence of surface heating rate on austenitic grain

size. Source: Ref 15, 27

Fig. 31

Hardness reached after induction surface hardening

at various heating rates in steel with 0.45% C.

Source: Ref 15, 27

Induction Hardening / 443

Name ///sr-nova/Dclabs_wip/Failure_Analysis/5113_417-501.pdf/Chap_13/ 18/8/2008 4:00PM Plate # 0 pg 443

with hardness measurements on the surface or

microhardness measurements in the cross sec-

tion of the hardened layer, and by measuring

residual stresses (Ref 27, 44, 45).

Figure 33 shows the hardness proﬁle in the

hardened layer subsequent to induction surface

hardening (line 1) as a function of different

carbon content in the steel after conventional

hardening (line 2) (Ref 15, 27). Induction

surface-hardened layers normally have on the

average 3 HRC higher hardness on the surface

than that achieved in the same kind of steel after

conventional hardening. This is primarily due to

a ﬁner martensite and compressive residual

stresses present in the induction surface-

hardened layer (Ref 2, 27, 46).

Time-Temperature Dependence in

Induction Heating

The time variation of temperature in induction

heating of a thin surface layer depends on the

type and shape of the induction coil used, the

alternating-current frequency, f (Hz), the and

power density Q (W/cm

). Power density is

deﬁned by the selected power of the high-

frequency generator and the surface layer of

the workpiece. Surface heating depends on the

coupling between the induction coil and the

workpiece. Figure 34 shows the inﬂuence of

Fig. 32

Individual phases in induction heating and spray quenching in the workpiece surface layer and corresponding temperature-

diameter diagrams. Source: Ref 15, 27

Fig. 33

Inﬂuence of carbon content on steel hardness after

various heat treatments. Source: Ref 15, 27

444 / Failure Analysis of Heat Treated Steel Components

Name ///sr-nova/Dclabs_wip/Failure_Analysis/5113_417-501.pdf/Chap_13/ 18/8/2008 4:00PM Plate # 0 pg 444

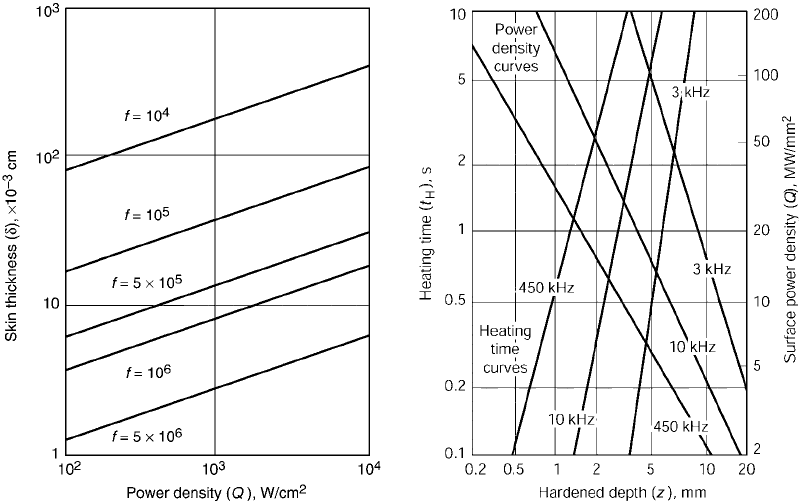
the selected power density and frequency on the

reference depth of the skin effect in a ferro-

magnetic material (Ref 2, 20).

A higher power density results in a greater

reference depth of the skin effect and a greater

depth of the heated layer with the same maxi-

mum temperature obtained at the workpiece

surface. Figure 35 shows the interdependence

between the heating parameters, that is, power

density and generator frequency, as a function of

the speciﬁed depth of the hardened layer and the

heating time required for single-shot techniques

of surface induction hardening (Ref 2, 20).

The data supplied by the diagram can have a

character of information only, but they make the

selection of an optimal surface induction con-

dition easier. With the scanning technique of

surface induction hardening, however, the speed

of the workpiece movement, rather than time,

assures the depth of the required hardened layer

and should be deﬁned. Generally, longer heating

times are required with smaller power densities

and vice versa. For the same depth of hardened

layer, longer heating times with lower current

frequencies are also required. With regard to the

depth of hardened layer selected between 0.5

and 10.0 mm, generator frequencies of 450, 10,

and 3 kHz can be selected in the single-shot

surface-hardening technique, in which case

appropriate power densities between 2 and

50 MW/m

are obtained. With lower high fre-

quencies, such as 10 kHz, the same depth of

surface-hardened layer, that is, 2.0 mm, can be

ensured only when the power density is changed

to 50 MW/m

. The lowest generator frequency,

3 MW/m

, shown in Fig. 35 cannot ensure the

depth of a hardened layer smaller than 2.5 mm.

Immediately after tempering, an intensive in-

verse heat ﬂow is expected as well.

The power density and frequency used in

induction hardening are usually based on the

shape and size of the machine part to be surface

hardened; the case depth is speciﬁed also. In

addition to the depth of hardening, the case

pattern along the entire length of the machine

part is important.

Regardless of the complexity of a workpiece

shape, case depth and transition that are as uni-

form as possible as well as regular-shaped hard-

ened ends should be provided. Inadequate

pattern transitions may produce high stress

concentrations related to the given loads in its

vicinity, so the material cannot resist dynami-

cally loaded parts.

Fig. 34

Reference depth of skin effect as a function of power

density and selected generator frequency for ferro-

magnetic steel. Source: Ref 2, 20

Fig. 35

Inﬂuence of high-frequency generator on selection

of power density and heating time with given thick-

ness of surface induction-hardened layer. Source: Ref 2, 20

Induction Hardening / 445

Name ///sr-nova/Dclabs_wip/Failure_Analysis/5113_417-501.pdf/Chap_13/ 18/8/2008 4:01PM Plate # 0 pg 445

Various heating conditions that are deﬁned by

the power density and frequency provide dif-

ferent pattern depths. The third inﬂuencing

parameter is heating time. Thus, different

hardness and residual-stress proﬁles may be

achieved. The steel grade to be hardened, the

loop shape, and the gap size between the

inductor and the workpiece should be con-

sidered. The data found in the diagram apply

only to stationary hardening; therefore, with

scanning surface hardening, these values

should be suitably corrected. For progressive

hardening, the hardening conditions should

be slightly corrected to allow for the loop

movement.

With the surface heat treatment processes,

studies are often conducted on the inﬂuence of

the selected heating and quenching conditions

on the depth of the hardened layer and the size of

the transition zone between the hardened and

nonhardened microstructures. One simple and

practical procedure to control surface heat

treatment is to measure the time variation of

temperature from the beginning to the end of the

heating process and also from the beginning to

the end of the quenching process. The heat

process may be changed by changing the power

density and the generator frequency, whereas the

quenching process may be changed by the

selection of different quenching agents and

quenching processes.

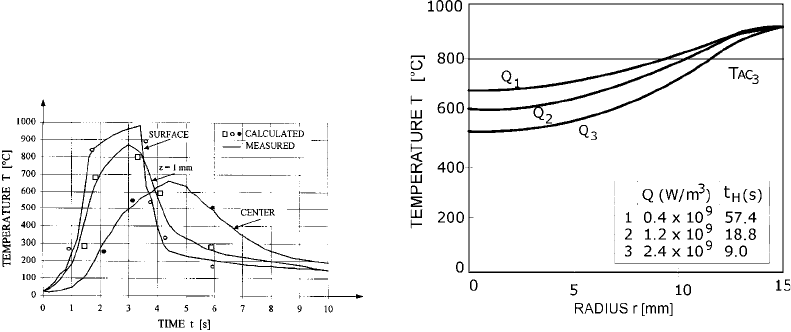
Figure 36 shows the measured and calculated

temperature cycles for the surface, the core, and

in a radius, r, of 7 mm in a depth of 1 mm in a

cylindrical specimen (Ref 47).

A comparison of the temperature cycles

shows that in surface induction hardening, a

thermal ﬂow of 3 MW/mm

in heating and

5.8 MW/mm

in quenching was selected. Under

such heating conditions, a maximum tempera-

ture of nearly 1000



C was attained, while

heating above a temperature of 800



C was

somewhat slowed down. The data in the diagram

show that the time required for heating the speci-

men from the ambient temperature to 800



Cis

equal to the time required for heating from the

latter to the maximum temperature obtained at

the surface, that is, 1.6 s. A temperature cycle at

the surface takes 3.2 to 3.3 s. The temperature

differences between the surface and the core in a

given moment are the greatest during the heating

process, that is, DT

max

ﬃ600



C. During the

quenching process, however, they can reach up

to 360



C. Temperature gradient changes are

much stronger in heating than in quenching. In

material heating, there is also a great difference

in yield stress of the material, which can produce

plastic deformation. Another very important

ﬁnding (Ref 47) is that the theoretical model

is appropriate, since the results obtained

were conﬁrmed by the standard experimental

methods such as temperature measurement with

thermocouples, diamond pyramid hardness test,

and measurement of residual stresses with x-ray

diffraction. The difference between the mea-

sured and the calculated temperature cycles is

very small. It occurs mainly in heating and

reaches up to 60



C at maximum, not taking into

account the losses due to eddy currents.

Figure 37 shows the variation of temperature

from the surface toward the core with various

volume power densities, Q, that is, 0.4 ·

, 1.2 · 10

, and 2.4 · 10

W/m

(Ref 48).

Fig. 36

Comparison of variations of calculated and mea-

sured temperature cycles for a cylindrical specimen

16 mm in diameter. Source: Ref 47

Fig. 37

Calculated variation of temperature through speci-

men cross section in induction heating up to

hardening temperature with different volume power densities.

Source: Ref 48

446 / Failure Analysis of Heat Treated Steel Components

Name ///sr-nova/Dclabs_wip/Failure_Analysis/5113_417-501.pdf/Chap_13/ 18/8/2008 4:01PM Plate # 0 pg 446

With smaller volume power densities, the maxi-

mum temperature differences between the sur-

face and the core also become smaller and the

austenitizing times longer. Because of higher

temperatures attained in the core, the inverse heat

ﬂow may even be so high as to produce a change

in microstructure and, consequently, a reduction

of hardness in the surface layer.

The induction heating industry has standar-

dized power supply frequencies, and probably

99% of the power used will be at the frequencies

listed in Table 2. Also included in Table 2 is the

type of equipment used to a change 50 Hz (60 in

some countries) to a higher frequency and the

conversion efﬁciency (Ref 23).

Melander (Ref 49, 50) ﬁrst treated single-shot

surface induction hardening of low-alloy steel

with 0.4% C, 0.7% Mn, and 1.1% Cr for tem-

pering and hardening as well as surface hard-

ening. For an analysis, a representative-sized

machine part that is most often used, that is,

a cylindrical specimen 40 mm in diameter,

was chosen. Induction-heating conditions were

selected so that the temperature of the diameter,

, was exceeded to a depth of 5.0 mm. This

means that a change of microstructure and hard-

ness was expected even to the depth of 5.0 mm,

where only partial austenitization was obtained.

Induction-heating conditions were chosen that

subjected the surface layer to heating for up to

35 s. Time variations of temperature at the sur-

face of the cylindrical specimen and in its sub-

surface at depths of 2.0, 4.0, and 10.0 mm are

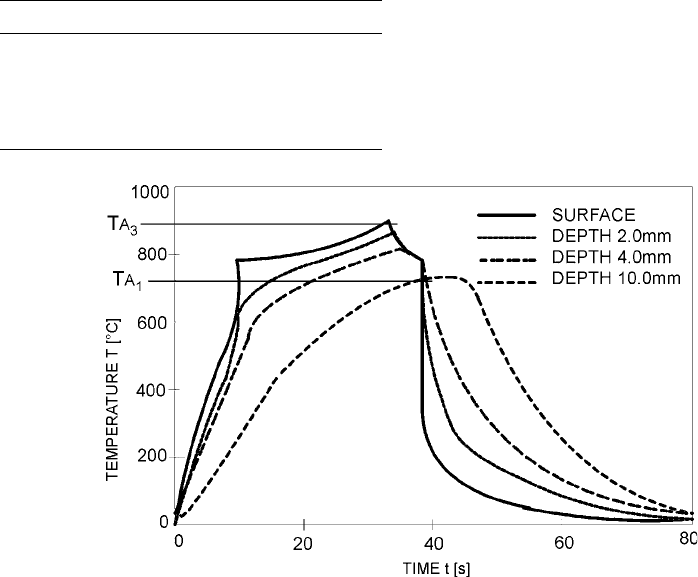
shown in Fig. 38 (Ref 49, 50). Time-temperature

diagrams differ from the previous ones, since a

distinctive deviation occurs in heating the speci-

men material when the temperature of magnetic

domain, T

, is reached and exceeded.

The course of surface heating indicates that

the transformation temperature T

was ob-

tained in 10 s. In spite of the same power den-

sity, further heating of the surface up to a

temperature of 850



C was very slow due to the

nonmagnetic character of the steel surface layer;

it took another 28 s. It is difﬁcult to assess which

models of induction heating are more suitable

than others. However, with the heating process

suggested, it is possible, after quenching, to

ensure a homogeneous, ﬁne austenitic micro-

structure exhibiting the ﬁnest martensite with

the highest possible hardness of the given steel.

Due to the presence of alloying elements and

high cooling rates of the surface layer, in addi-

tion to ﬁne martensite, up to 3% residual auste-

nite also appears. Based on the time-temperature

variation of heating, the depth of the hardened

layer ranges between 2.0 and 4.0 mm. From the

Table 2 Power sources, frequencies, efﬁciency,

and power for induction heating equipment

Type

Power (P),

Efﬁciency (g),

Frequency

( f ), kHz

Vacuum tube

oscillators

5–600 50–60 200–450

Motor generators 7.5–500 75–80 1, 3, 10

Frequency multipliers 100–1000 90–95 180 and 540

Frequency inverters 50–1500 85–95 0.5, 1, 3, 10

Source: Ref 23

Fig. 38 Time-temperature cycle during single-shot surface induction heating and quenching. Source: Ref 49, 50

Induction Hardening / 447

Name ///sr-nova/Dclabs_wip/Failure_Analysis/5113_417-501.pdf/Chap_13/ 18/8/2008 4:01PM Plate # 0 pg 447