Blank B.E., Krantz S.G. Calculus: Single Variable

Подождите немного. Документ загружается.

of φ. Each of these signals is less than 1.5. For what value

of φ is the magnitude of the superposed wave at x 5 2as

large as it can be?

75. Suppose that ν

and ν

are both large compared with

their absolute difference jν

2ν

j. The superposition

t/cos (ν

t) 1 cos (ν

t) might be described as a high-

frequency cosine wave in which the beats have low-

frequency amplitude modulation. Use Exercise 49 to

express the superposition in the form A(t) cos (ω t)

where the frequency ω is about the same size as the

individual frequencies ν

and ν

and where the amplitude

A(t) of the beats varies in time according to a low-

frequency cosine wave. Illustrate with the graph of the

superposed wave for ν

5 8 and ν

5 6. What is ω in this

case? Add the graphs of 62cos (t) to your ﬁgure. What is

the frequency of the modulated amplitude?

76. An amplitude modulation (AM) radio transmitter

broadcasts an audio tone (or baseband signal) cos (ν t)

by modulating the amplitude of a very high-frequency

carrier signal A cos (ω t) by a positive factor

(1 1 m cos (ν t)), where the modulation index m is less

than 1. Thus the emitted signal has the form

SðtÞ5 A ð1 1 m cosð ν  tÞÞcosðω  tÞ:

a. Use the identity from Exercise 49 to express S(t)asa

superposition of three cosine waves. The largest and

smallest frequencies of these three waves are called

sidebands.

b. Set A 5 1, m 5 1/2, ν 5 2, and ω 5 8. Graph the signal

S(t) and envelopes 6(1 1 m cos (ν t))

for 0 # t # 2π.

Explain how the sidebands can be determined visually

from your graph.

c. Suppose that ν

max

, ω is the highest baseband fre-

quency. The largest and smallest frequencies broad-

cast are called the upper and lower sidebands. What

are they in terms of ω and ν

max

? Their difference is

called the bandwidth.

d. Carrier frequencies for AM radio range from 550 kHz

(kilohertz), or 550 3 10

cycles per second, to

1610 kHz. Typically, ν

max

can be as high as 15 kHz. An

AM receiver works by recovering the audio source

cos (ν t) from the received signal S(t), a process known

as demodulation. Demodulation requires that the

bandwidths of different radio stations that broadcast to

the same location not overlap. What must the mini-

mum frequency separation between the carrier signals

of two such stations be?

77. Snell’s inequality, discovered in 1621, states that

t # tan





1 2sin





;

for 0 , t , 3π2. An even older inequality,

3sinðtÞ

2 1 cosðtÞ

, t;

for all t . 0, was obtained in 1458 by Nicholas Cusa

(14011464). Graphically illustrate each inequality for

0 , t , 0.01.

Summary of Key Topics in Chapter 1

Number Systems

(Section 1.1)

In calculus, we deal with the three types of real numbers:

Terminating decimal expansions

2. Nonterminating decimal expansions that, after a ﬁnite number of terms, repeat a

single block of terms endlessly

3. Nonterminating, nonrepeating decimal expansions

The ﬁrst two types are rational numbers, and the third type consists of irra-

tional numbers.

Sets of Real Numbers

(Section 1.1)

Sets of numbers are usually described with the notation fx : P(x)gan

d are graphed on

a number line. Often a set is described by inequalities that must ﬁrst be solved before

the set can be easily sketched. The most frequently encountered sets are intervals:

ða; bÞ5 fx : a , x , bg

½a; b5 fx : a # x # bg

½a; bÞ5 fx : a # x , bg

Summary of Key Topics 75

ða; b5 fx : a , x # bg

ða; NÞ5 fx : a , xg

½a; NÞ5 fx : a # xg

ð2N; aÞ5 fx : x , ag

ð2N; a5 fx : x # ag

ð2N; NÞ5 fx : 2N , x , Ng

Points and Loci in the

Plane

(Section 1.2)

In the plane, points are located using ordered pairs of real numbers, (x, y).

The

distance between two points (a

, b

) and (a

, b

) is given by the formula

d 5

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

ða

1 ðb

In general , we are interest ed in graphing sets of points that are described by

equations. Such a graph is called the locus of the equation. For a given equation, a

point (x, y) is on the graph if and only if the numbers x and y satisfy the equation

simultaneously. An important instance is given by equations of the form

ðx2hÞ

1 ðy2kÞ

5 r

with r . 0. Such an equation describes a circle with center (h, k) and radius r.

Lines and Their Slopes

(Section 1.3)

If ‘ is

a line in the plane, and if (x

, y

)and(x

, y

) are points on ‘, then the slope of

‘ is given by the expression

2 y

2 x

Parallel lines have the same slopes; perpendicular lines have slopes that are

negative reciprocals. It takes two piece s of data to describe a line in the plane: a

point through which the line passes and the slope, or two points through which the

line passes, or two intercepts, or the slope and an intercept.

The equation for a line (except a vertical one) can always be obtained by

ﬁnding two expressions for the slope and equating them. The following are four

particularly useful forms of equations for lines.

1. The Point-Slope Form A line with slope m and passing through the point (x

, y

)

has equation

y 5 mðx 2 x

Þ1 y

2. The Two-point Form A line passing through the points (x

, y

) and (x

, y

)has

equation

y 5



2 y

2 x



ðx 2 x

Þ1 y

3. The Slope-Intercept Form A line with slope m and y-intercept (0, b) has equation

y 5 mx 1 b:

76 Chapter 1 Basics

4. The Intercept Form A line with intercepts (a, 0) and (0, b) where a and b are both

nonzero has equation

5 1:

5. The general linear equation Every line, vertical lines included, has equation

Ax 1 By 5 D.

Functions and Their

Graphs

(Section 1.4)

If S and T are

sets, then a function is a rule that assigns to each element of S a

unique element of T. We call S the domain of the function and T the range of the

function. In calculus, S and T are usually sets of real numbers, and functions are

usually described by formulas . Frequently, the formula is given, and it is up to

the reader to infer the domain and range of the function. The graph of a

function consists of all points (x, y) in the plane such that x is in the domain of f,

and y 5 f (x).

Combining Functions

(Section 1.5)

Most functions that we encounter are built up from simple functions by using

operations,

such as addition, subtraction, multiplication, division, and composition,

on functions. Composition is the most subtle. If f and g are functions, and if the

domain of g contains the range of f, then we deﬁne ( g 3 f )(x) 5 g( f (x)). Care should

be taken not to confuse composition with multiplication.

A function f : S - T is onto if for every t in T, there is an s in S such that f (s) 5 t.

A function f : S - T is one-to-one if for every t in T, there is no more than one s in

S such that f (s) 5 t.Iff is both one-to-one and onto, then there is a unique

function f

with domain T and range S such that f ( f

(t)) 5 t for every t in T and

( f (s)) 5 s for every s in S. In this case, we say that f is invertible. The function

is the inverse of f (and, symmetrically, f is the inverse of f

Trigonometric

Functions

(Section 1.6)

Radian measure of an angle is related to the degree measure of an angle by the

formula

x radians 5

180x

degrees:

If θ is a real number, let P (θ) be the point of the unit circle cut off by rotating the

positive x-axis by θ radians. The rotation is counterclockwise for θ . 0 and clock-

wise for θ , 0. We deﬁne cos ( x ) and sin (x) to be the abscissa and ordinate,

respectively, of P (θ ). The other trigonometric functions are deﬁned as quotients:

tan (x) 5 sin (x)/cos (x ), cot(x) 5 cos (x)/sin (x), csc (x) 5 1/sin (x), and sec (x) 5 1/

cos (x). There are many identities among the trigonometric functions, and most can

be derived from

cos

ðθÞ1 sin

ðθÞ5 1;

sinðθ 1 φÞ5 sinð θÞcos ðφÞ1 cosðθÞsinðφÞ;

and

cosðθ 1 φÞ5 cosðθÞcos ðφÞ2 sinðθÞsin ðφÞ:

Summary of Key Topics 77

REVIEW EXERCISES for Chapter 1

c In each of Exercises 18, express the given set as an

interval or union of intervals (possibly unbounded). b

1. fx : jx 1 5j, 6g

2. fx : jx23j$ 7g

3. fx : x

23x # 0g

4. fx : jx 1 3j/x . 5g

5. fx :(2x 1 1)/jxj, 4g

6. fx : x

, 6 1 xg

7. fx : x21 # 6/xg

8. fx : j2x 1 1j. jx23jg

9. What is the distance between the points (23, 21) and

(9,26)?

10. What is the distance between the points of intersection of

the line 4x23y 5 12 and the coordinate axes?

11. What is the midpoint of the line segment between the

points (23,21) and (9,26)?

12. What is the distance between the points of intersection of

the line y 5 3x25 and the parabola y 5 x

29?

c In each of Exercises 1316, identify the type of conic

section

whose Cartesian equation is given. Determine its

vertical and horizontal axes of symmetry and, if it is a circle or

parabola, its radius or vertex. b

13. x

1 y

1 2y 5 2x

14. 7/4 1 3x2x

2y 5 0

15. 9x

1 18x 1 4y

216y 5 11

16. y

1 4y 5 4x

c In each of Exercises 1720, ﬁnd the slope-intercept form

of the line ‘ determined by the given data. b

17. ‘ has

slope 3 and x-intercept 2.

18. ‘ is parallel to the line 2x24y 5 9 and passes through

(21,23).

19. ‘ is perpendicularto 2x22y 5 1 and passes through (21,23).

20. ‘ passes through the points (1,21) and (3,25).

c In each of Exercises 2124, use the given point P to

write the

point-slope form of the line ‘ determined by the given data. b

21. ‘ is

parallel to the line x 1 y/6 5 1 and passes through P 5

(22, 1).

22. ‘ is perpendicular to the line 2x 1 y 5 1 and passes

through P 5 (22, 1).

23. ‘ passes through P 5 ( 22, 1) and has y-intercept 5.

24. ‘ passes through P 5 (1, 6) and has x-intercept 2.

c In each of Exercises 2528, ﬁnd the intercept form of the

equation

of the line ‘ determined by the given data. b

25. ‘ passes

through (0,23) and (4, 0).

26. ‘ is parallel to the line y 5 22x and passes through

P 5 (2, 3).

27. ‘ is perpendicular to the line 2y 5 x and passes through

P 5 (1, 1).

28. ‘ passes through (1, 5) and (3, 1).

c In each of Exercises 2932, ﬁnd the point or points of

intersection

of the curves whose equations are given. b

29. 2x2y 5 1

and x 1 y 5 5

30. x 1 2y 5 7 and 3x 1 y 5 1

31. y 5 3x25 and y 5 x

32. y 5 x

23 and y 5 1 1 2x2x

c In each of Exercises 3340, ﬁnd the domain of the func-

tion deﬁned by the given expression. b

33. (x

22x22)/(x

22x 1 2)

34. x/(1 1 1/x)

35. (x

225)/(x25)

36. (42x

)

21/2

37. (8 1 x

)

21/3

38.

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

1 x 2 2

39. 1=ð4 2

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

25 2 x

40.

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

9 2 x

=ð1 1

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

2 1

c In each of Exercises 4146, calculate f

for 1 # n # 5. b

41. f

5 n/(n 1 1)

42. f

5 1 1 (21/2)

43. f

5 2

1 2n

44. f

2 þ 1=n

2  1=n

45. f

5 1 1 2 1 4 1 1 2

n 1 1

46. f

5 1 2 3 ( n 1 2)

c In each of Exercises 4750, a sequence ff

g is deﬁned

recursively. Calculate f

. b

47. f

5 1, f

5 2f

n21

48. f

5 1, f

5 n

n21

49. f

5 0, f

5 2f

n21

1 n

50. f

5 1, f

5 1 1 2/(1 1 1/f

n21

)

c In Exercises 5160, calculate the given expression that is

constructed

from f (x) 5 2x 1 3, g(x) 5 1 1 x

and h(x) 5

(12x)/(1 1 x). b

51. f

(

ﬃﬃﬃ

)

52. ( f

24g)(x)

53. (h 3 g)(x)

54. (g 3 f )( x)

55. ( f 3 g

)(x)

78 Chapter 1 Basics

56. (h 3 (2f ))( x)

57. ( f 3 g

1/2

)

ﬃﬃﬃ

58. (h 3 h)(x)

59.





ðxÞ

60. ( f 3 f 3 f )( x)

c In each of Exercises 6164, f is

an invertible function. The

domain S of f is given, as is the formula for f (s) for s in S. State

the domain T of f

and a formula for f

(t) for t in T. b

61. S 5 [1,

4], f (s) 5 1/(1 1 s)

62. S 5 [1,N), f (s) 5 3s

63. S 5 [0, 1], f (s) 5 s

1 2s 1 2

64. S 5 (0, 1), f (s) 5 (1 1 s)/(2 1 s)

c In each of Exercises 6568, a noninvertible function f is

given.

Determine whether f is onto but not one-to-one, one-

to-one but not onto, or neither one-to-one nor onto. b

65. f :[21,N) - (0,

1], f (s) 5 1/(1 1 s

)

66. f : [1, 100] - [1, 110], f (s) 5 s 1 log

(s)

67. f : [0,N) - [0, 1], f (s) 5 s/(s 1 1)

68. f : [0, 2] - [0, 2], f (s) 5 s

22s 1 2

c In each of Exercises 6972, state how the graph of g may

obtained from the graph of f by a vertical translation, a

horizontal translation, or both. b

69. f (x) 5 x

, g(x) 5 (x 1 2)

70. f (x) 5 ( x 1 1)/(x 1 2), g(x) 5 (x21)/x

71. f (x) 5 x

, g(x) 5 x

26x

72. f (x) 5 x

/(x

1 2), g(x) 5 f (x 1 3) 1 3

c In each of Exercises 7376, a parametric curve with domain

parameterization I is given. Describe and sketch it. b

73. x 5 2t 1 1, y 5 3t 1 2, I 5 [22/3, 21/2]

74. x 5 3

sin (t), y 5 3 cos (t), I 5 [2π/2, π/2]

75. x 5 cos

(t), y 5 sin

(t), I 5 [0, π/2]

76. x 5 jtj1 t, y 5 jtj 2t, I 5 [21, 1]

c Each of Exercises 7780 concerns a right triangle with a

potenuse of length c and legs of lengths a and b.LetA be the

angle opposite to the side of length a. From the given infor-

mation, calculate the six trigonometric functions of A. b

77. a 5 3, b 5 2

78. a 5 12, b 5 5

79. b 5 3, c 5

ﬃﬃﬃﬃ

ﬃ

80. b 5 3, c 5 5

81. Let α be the radian measure of angle A of Exercise 78.

Evaluate the six trigonometric functions at α 1 π/2.

82. Let α be the radian measure of angle A of Exercise 80.

Evaluate the six trigonometric functions at α 1 π.

c In each of Exercises 8386, simplify the given expression. b

83. tan

(π/4)2cot(3π/4)

84. sin (π/6) 1 cos (5π/3)

85. csc (π/6) sec (3π/4)

86. cot (π/3) cot (4π/3)

c In each of Exercises 8790, use the given information

about

the acute angle α to calculate the value of r. b

87. sin

(α) 5 3/4, r 5 sin (2α)

88. tan (α) 5 1/2, r 5 cos (2α)

89. cos (α) 5 3/5, r 5 tan (2α)

90. sec(α) 5 3/2, r 5 cos (2α 1 π/2)

Review Exercises 79

GENESIS

DEVELOPMENT1

In this ﬁrst chapter, we reviewed three fundamental

developments that underlie calculus: the real number

line, the mathematic al notion of a function, and the

principles of analytic geometry.

Irrational Numbers and Successive

Approximation

The history of the real number line be gins in the

Golden Age of ancient Greece. The ﬁrst important step

came with the realization that not every number is

rational. Some time before 410 BCE, the followers of

Pythagoras (ca. 580500 BCE) discovered that

ﬃﬃﬃ

irrational. The elegant argument outlined in Exercise

57, Section 1.1, is the earliest proof that has been pre-

served. It was recorded by Aristotle (384322 BCE)

but is certainly older.

The mathematicians of ancient Greece devised a

number of methods for successively approximating

ﬃﬃﬃ

and other irrational numbers. One of these algorithms

is based on ﬁnding integer solutions to the equation

22y

5 1 (see Exercise 56, Section 1.4). Archimedes

(ca. 287212 BCE) used the same idea to approximate

ﬃﬃﬃ

to six decimal places. He obtained his estimate,

1351/780, from the solution x 5 1351, y 5 780 of the

equation x

23y

5 1.

Equations of the form x

2D y

5 1, with D a

nonsquare integer, are called Pell equations, and

Archimedes seems to have had some expertise in their

solution. In 1773, a copy of a previously unknown

message from Archimedes to the mathematicians of

Alexandria was discovered in a German library. In his

letter, Archimedes posed a riddle concerning the

numbers of eight types of cattle. The key to the cattle

problem, as it has come to be known, is the solution

in integers of the Pell equation x

24729494 y

5 1. In

1880, A. Amthor obtained the least positive integer

solution to this Pell equation:

5 109931986732829734979866232821433543901088049;

5 50549485234315033074477819735540408986340;

which enabled him to show that the solution to the

cattle problem has 206, 545 decimal digits. In 1981, a

Cray I supercomputer at the Lawrence Livermore

National Laboratory calculated this large number,

using about 10 minutes of computing time. The life

span of the computer that solved this ancient problem

was only about a dozen years. Having been purchased

in the late 1970s for $19, 000, 000, it was auctioned off in

1993 for $10, 000.

The Number π

The irrational number π also featured prominently in

the mathematics of the ancient Greeks. Archimedes

proved that, if A and C are the area and circumf erence

of a circle of radius r, then the ratios A/r

and C/2r are

the same constant π. (It is not known who discovered

this fact or even when the discovery was made.)

Archimedes also approximat ed π to two decimal places

by deriving the inequalities 3 1 10/71 , π , 3 1 1/7. The

computation of the digits of π has attracted the interest

of mathematicians ever since. Sir Isaac Newton

(16421727), for example, calculated π to 15 decimal

places. By 1761, 113 digits of π were known. In that

year, the Swiss mathematician Johann Heinrich Lam-

bert (17281777) published a proof that π is irrational.

In 1806, Adrien-Marie Legendre (17521833) proved

the stronger result that π

is irrational, and he con-

jectured even more.

A real number is said to be algebraic if it is a root

of some polynomial with rational coefﬁcients; other-

wise, it is said to be transcendental. Every rati onal

number is algebraic, but an irrational number may be

algebraic or transcendental. For example, the irrational

number

ﬃﬃﬃ

is algebraic because it is a root of x

22. At

the time Legendre ventured his conjecture, mathema-

ticians did not even know if transcendental numbers

existed. It was not until 1844 that Joseph Liouville

(18091882) established the existence of transcenden-

tal numbers. Thirty years later, Georg Cantor’s

(18451918) nov el ideas about inﬁnite sets put the

matter in a totally new light.

Using simple but original ideas, Cantor proved that

algebraic numbers can be put into one-to-one corre-

spondence with natural numbers and that transcen-

dental numbers cannot. We say that the set of algebraic

numbers is countable,ordenumerable, because of this

property and that the set of transcendental numbers is

uncountable,ornondenumerable. In a very real sense,

Cantor proved that there are more transcendental

numbers than algebraic numbers. He did not, however,

show that any one particular number is transcendenta l.

Indeed, it is usually very difﬁcult to show that a

given number is transcendental. In 1882, Carl Louis

Ferdinand von Lindemann (18521939) ﬁnally proved

Legendre’s conjecture that π is transcendental. Mean-

while, the digit hunters have continued their work. In

2002, a supercomputer at the University of Tokyo was

used to calculate more than 1.2 trillion digits of π.

Zeno’s Paradoxes

The fundamental idea of calculus, that which distin-

guishes it from algebra and classical geometry, is the

inﬁnite process. We have mentioned the algorithms by

which Greek mat hematicians created inﬁnite sequen-

ces, which tend to

ﬃﬃﬃ

and

ﬃﬃﬃ

in the limit. We have

discussed the manner in which Archimedes inscribed

an inﬁnite sequence of regular polygons in a circle in

order to successively approximate its area and cir-

cumference. A number of other inﬁnite processes have

been outlined in the exercises of Chapter 1. The idea is

not to actually perform an inﬁn ite number of opera-

tions but to see what results in the limit. That is the

essence of calculus. Although the mathematicians of

ancient Greece who originated these ideas were well

aware of the power of the inﬁnite process, they were

also concerned with the logical difﬁculties that can

ensue.

Zeno of Elea (ca. 496435 BCE) lived in the age of

Pericles. Little is known of Zeno’s life. As for his death,

it is written that Zeno was tortured and executed for his

part in a conspiracy against the state. Although the

original writings of Zeno have not survived, numerous

references to them have. Zeno is best known today for

four paradoxes—the Dichotomy, the Achilles, the

Arrow, and the Stade—that Aristotle retold .

According to the Dichotomy, “There is no motion

because that which is moved must arrive at the middle

before it arrives at the end, and it must traverse the half

of the half before it reaches the middle, and so on ad

inﬁnitum.” Thus if a line is inﬁnitely divisible, motion

cannot begin. The Achilles asserts that, given a head

start, a tortoise will not be overtaken by the runner

Achilles because Achilles must ﬁrst reach the point P

at which the tortoise started. In the time required for

Achilles to arrive at P

, the tortoise will have advanced

to a point P

, which Achilles must reach before over-

taking the tortoise. By the time Achilles has run from

to P

, the tortoise will have moved ahead to a point

, and so on, ad inﬁnitum. The four paradoxes are

“immeasurably subtle and profound,” in the words of

Bertrand Russell. Aristotle called them fallacies but

was not able to refute them. Indeed, it woul d be a long

time before mathematicians created a framework in

which they could undertake the inﬁnite pro cesses of

calculus free from such logical difﬁculties.

Analytic Geometry

After the decline of Greek civilization, mathematical

progress came slowly. The next signiﬁcant contribution

to the foundation of calculus came in the 14th century

when philosophers in England and France attempted to

analyze accelerated motion. In doing so, the Norman

scholar Nicole Oresme (13231382) identiﬁed the real

numbers with a geometric continuum. After another

long hiatus, Franc¸ois Vie

te (15401603) further

demonstrated the power of geometrically interpreting

the real numbers. His work set the stage for the

development of analytic geomet ry by Pierre de Fermat

(16011665) and Ren

e Descartes (15961650).

Analytic geometry, as developed in the 17th cen-

tury by Fermat and Descartes, was the ﬁnal break-

through that permitted the discoveries of calculus. The

crucial idea that the solution set of an equation such as

F(x, y) 5 0 can be identiﬁed with a curve in the plane

appeared in the G

eom

etrie, which Descartes published

in 1637. In the words of Descartes, “I believed that I

could borrow all that was best both in geometrical

analysis and in algebra, and correct all the defects of

the one by help of the other.”

Descartes led something of a fugitive existence. He

left France for Holland, where he lived for most of his

adult life. Changing residence 24 times in 21 years, he

adopted the motto “He who hides well lives well.” In

1649, Descartes accepted an invitation to tutor Swe-

den’s Queen Christina in philosophy, but he died of

pneumonia soon after his arrival. The mortal remains

of Descartes had a macabre afterlife that mirrored his

transient lifestyle. Issues of religious afﬁliation led to an

initial burial in a Stockholm cemetery intended for

unbaptized children. Sixteen years later, a French

delegation was dispatched to repatriate the remains of

the country’s most distinguished philosopher. As the

journey was long, the cofﬁn was short. The bones of

Descartes, minus the skull, returned home to a

sequence of burials in and disinterments from a

museum garden, the courtyard of the Louvre, and four

churches. In a chapel of the Church of St. Germain-des-

Pres, Paris, a marble slab bearing an 1819 inscription

marks the ﬁna l resting spot. The skull of Descartes was

returned to France at a later date and is now in the

collection of the Mus

ee de l’Homme in Paris.

Genesis & Development 81

The Scientiﬁc Revolution

Prior to the 16th century, most scientiﬁc teaching in

Europe consisted of scholars passing a ﬁxed collection

of theories and ideas to their students. These theories

were not to be questioned or analyzed—merely

learned. The method of experimentation and proof had

not yet become a universally accepted part of scientiﬁc

activity. Ancient authority ruled the sciences.

During the Age of Reason, a revo lution took place.

The so-called rationalist movement in philosophy

demanded that new ideas be demonstrated or proved.

Descartes became one of the most inﬂuential expo-

nents of the movement. Today, the rationalist metho-

dology is universally accepted. When a calculus

instructor insists on proving assertions in class, it is

because of a deeply held conviction that students

should not simply learn facts by rote but should truly

understand the concep ts being taught.

If the 16th century had brought a revolution in

scientiﬁc thinking, then the 17th century ushered in the

era of quantitative relationships. In The Assayer, Gali-

leo (15641642) published the manifesto of the new

science:

Philosophy is written in this grand book, the uni-

verse which stands continuall y open to our gaze. But

the book cannot be understood unless one ﬁrst

learns to comprehend the language and read the

letters in which it is composed. It is written in the

language of mathematics and its characters are tri-

angles, circles, and other geometric ﬁgures.

Scientists now looked for functional relationships

between the variables of nature. Galileo determined

the distance an object falls under gravity as a function

of time. Johannes Kepler (15711630) discovered that

the time in which a planet orbits the sun is a function of

its mean distance to the sun (Kepler’s Third Law).

Christiaan Huygens (16291695) determined cen-

trifugal force as a function of radius and velocity,

providing a link between the laws of Kepler and Gali-

leo. Before the end of the 17th century, Newton for-

mulated the law of universal gravitation, demonst rating

that Kepler’s laws and Galileo’s law are but different

aspects of the same general phenomenon.

The realization that natural phenomena could be

expressed through functional relationships was not at

all limited to motion. The Dutch scientist Willebrord

Snell (15801626) found that the angle of refraction of

a ray of light is a function of the angle of incidence

(Snell’s law). Evangelista Torricelli (16081647)

demonstrated that the height of mercury in a vertical

tube is a function of atmospheric pressure. Robert

Boyle (16271 691) postulated the functional relation-

ship among the pressure, volume, and temperature of a

gas. Robert Hooke (16351703) expressed the force

exerted by a spring as a function of its compression or

extension. The age of funct ions had arrived. Before the

end of the 17th century, calculus would be invented as a

tool to study these functions.

The Completeness Property of the Real

Numbers

Although scientiﬁc observation validated the phenom-

ena predicted by calculus, the new discipline quickly

came under serious criticism concerning the foundation

of the entire subject. Just as the ancient Greeks had

opened the door to Zeno’s paradoxes when they

admitted the inﬁnite process, so had the mathematicians

of the 17th century set themselves up for a new set of

paradoxes by their lack of rigor in working with the real

number line. By the 1800s, mathematicians realized that

a deeper understanding of the real number system was

essential. Their focus turned to what we now call the

completeness property of the real numbers, which guar-

antees that there are no holes or gaps in the real number

line. Here is one way to describe the completeness of the

real numbers: If fI

} is a sequence of closed bounded

nonempty real intervals with each interval a subinterval

of its predecessor as in the diagram,

½½½½......

|ﬄﬄﬄ{zﬄﬄﬄ}

nþ1



|ﬄﬄﬄﬄﬄﬄﬄﬄﬄﬄ{zﬄﬄﬄﬄﬄﬄﬄﬄﬄﬄ}



then there is at least one point in R that is in every I

. The

real number system satisﬁes this property and is com-

plete; the rational number system is not. An under-

standing of the role of completeness came about in 1872

thanks to several mathematicians, including Georg

Cantor, Julius Richard Dedekind (18311916), and

Karl Weierstrass (18151897). Although many further

discoveries would be made, by 1872 everything was in

place for a rigorous development of calculus, a subject

that was then already 200 years old.

82 Chapter 1 Basics

CHAPTER 2

Limits

The concept of limit is the basis for a solid understanding of calculus. We will

therefore spend an entire chapter studying limits before proceeding to calculus. The

notion of limit originated in Greece approximately 2500 years ago. It arose then

for many of the same reasons that we study limits in a modern calculus course: to

understand continuous motion and to measure lengths, areas, volumes, and other

physical quantities.

Consider a circle C of radius r. We all learn in geometry that its area A is π r

,and

its circumference C is 2πr, but what exactly do we mean by the area and circumference

of a circle? Precise answers can be understood only in terms of limits.

One approach is to approximate the area and circumference of C by a region

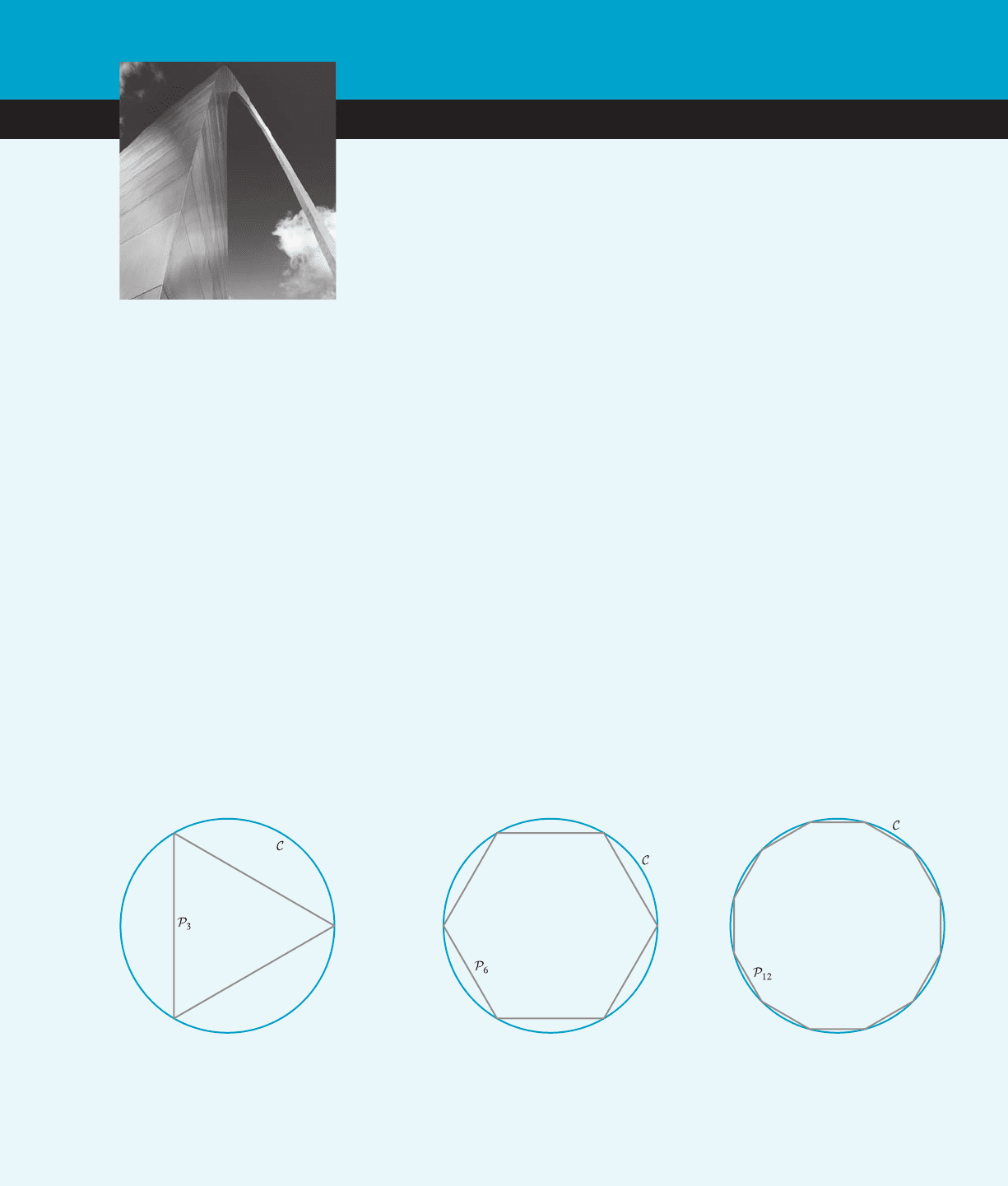
with an area and perimeter that we do know. Figure 1 shows an equilateral triangle

inscribed in C. We know the area and perimeter of the triangle P

, but it is not a

very good approximation to the circle. We add sides, one at a time, to the inscribed

ﬁgure to create inscribed polygons with more and more sides of equal length. It

seems clear that the inscribed polygon is becoming closer and closer to the circle.

Figure 2 shows that the area inside the inscribed 6-gon is fairly close to the area of

the circle, and the area inside the inscribed 12-gon is extremely close to that of the

circle. When the polygon has one billion sides, we could not possibly draw a ﬁgure

illustrating the difference between the inscribed polygon and the circle, but there is

PREVIEW

m Figure 1 An equilateral

triangle inscribed in circle C

m Figure 2a A 6-gon inscribed

in circle C

m Figure 2b A 12-gon inscribed

in circle C

a difference. No matter how small the sides of the polygon become, the polygon

will have many small ﬂat sides of equal length, even though the circle is round. It is

correct to say that, when the side length of the polygon is close to zero (that is,

the number of sides of the polygon is large), the area inside the polygon is close

to the area inside the circle. It is incorrect to say that the area of any approximating

polygon is equal to that of the circle.

The correct mathematical terminology is that, as the number of sides n tends to

inﬁnity, the limit of the area A

enclosed by the n-sided polygon P

equals the area

A inside the circle, and the limit of the perimeter C

of P

equals the circumference

C of the circle. We write these statements as

A 5 lim

n-N

and C 5 lim

n-N

ð2:0:1Þ

For another application of the limit concept, suppose that we view a training ﬁlm of

a runner. The ﬁlm shows elapsed time and distance markers that allow us to measure

the distance s(t) the athlete has run in any given time t. It is easy to compute the average

speed of the runner between any two points: Simply divide the total distance run by the

total elapsed time. However, the speed of the runner will vary from one instant of time

to another. How can the runner’s speed v(c) at a given instant of time c be calculated?

For any value t . c, the average speed of the runner in the small time interval [c, t]is

sðtÞ2 sðcÞ

t 2 c

For t close to c, this will give a good approximation to v(c) because the runner’s

speed does not change much in the small time interval [c, t]. If we press this point

further, then we intuitively arrive at the concept of instantaneous velocity at c:

vðcÞ5 lim

t-c

sðtÞ2 sðcÞ

t 2 c

: ð2:0:2Þ

As we will see, equations (2.0.1) and (2.0.2) actually serve to deﬁne the concepts

of area, circumference, and instantaneous velocity. These examples convey an

important idea: The concept of li mit involves a variable that approaches arbitrarily

close to a number but does not actually reach it. In equation (2.0.1), the natural

number n can get arbitrarily large, but it is never a ctually equal to inﬁnity. In equation

(2.0.2), the number t cannot actuall y be equal to c because that would result in the

meaningless fraction 0/0. The key is ﬁrst to understand exactly what is meant by the

limits in these equations an d then to learn methods for computing them.

The purpose of this chapter is to give a precise meaning to the concept of limit.

That having been done, much of the rest of this textbook concerns ways to calculate

limits. Indeed, the notion of limit is used in a fundamental way in each of the

remaining chapters.