Bird J. Electrical Circuit Theory and Technology
Подождите немного. Документ загружается.

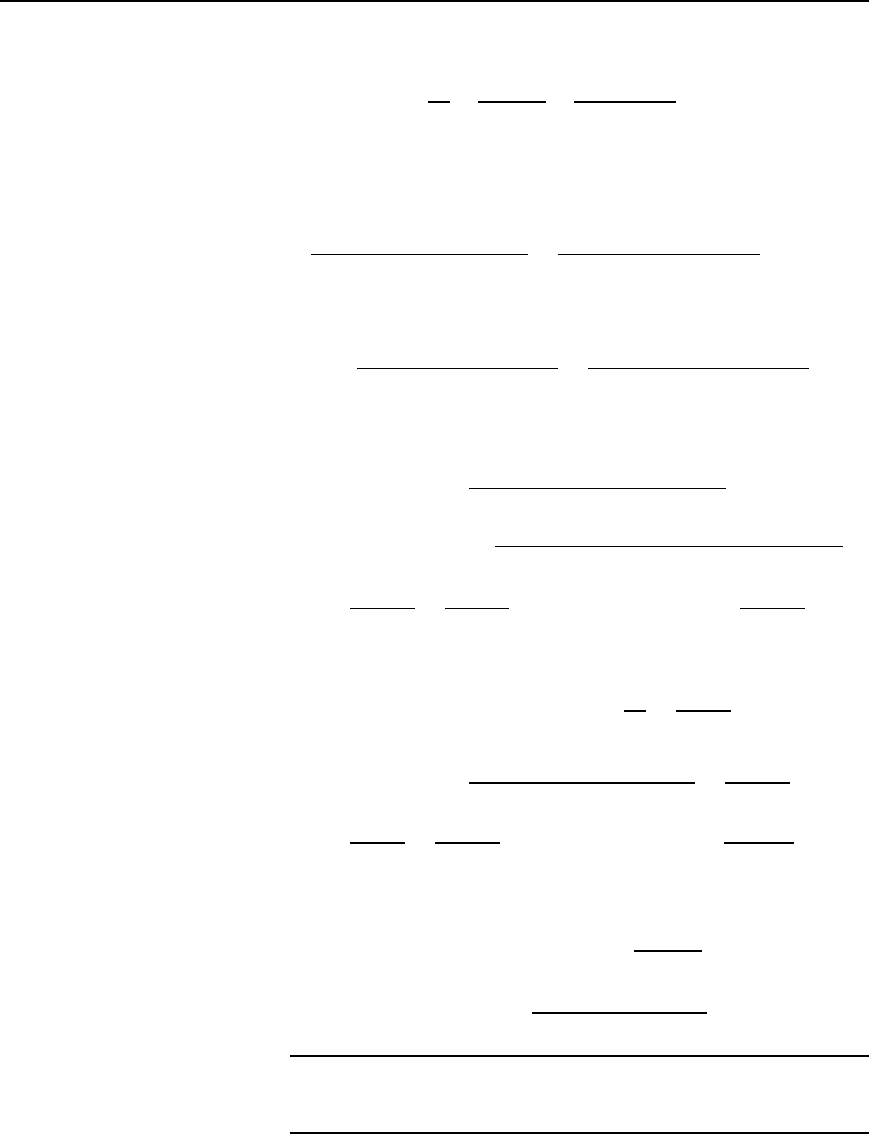
558 Electrical Circuit Theory and Technology
i.e., V
1
C 1.833V
2
0.333V
3
C 0 D 0 2
At node 3,
V
3
4
C
V
3
V
2
3
C
V
3
C 8 V
1
5
D 0
i.e., 0.2V
1
0.333V
2
C 0.783V
3
C 1.6 D 0 3
Equations (1) to (3) can be solved for V
1
,V
2
and V
3
by using
determinants. Hence
V
1
1 0.2 1.6
1.833 0.333 0
0.333 0.783 1.6
D
V
2
1.367 0.2 1.6
1 0.333 0
0.20.783 1.6
D
V
3
1.367 1 1.6
11.833 0
0.2 0.333 1.6
D
1
1.367 1 0.2
11.833 0.333
0.2 0.333 0.783
Solving for V
2
gives:
V
2
1.60.8496 C 1.60.6552
D
1
1.3671.3244 C 10.8496 0.20.6996
hence
V
2
0.31104
D
1
0.82093
from which, voltage,V
2
D
0.31104
0.82093
D 0.3789 V
Thus the current in the 2 Z resistor D
V
2
2
D
0.3789
2
D 0.19 A,
flowing from node 2 to node A.
Solving for V
3
gives:
V
3
1.60.6996 C 1.61.5057
D
1
0.82093
hence
V
3
1.2898
D
1
0.82093
from which, voltage,V
3
D
1.2898
0.82093
D
−1.571 V
Power in the 3 Z resistor D I
3
2
3 D
V
2
V
3
3
2
3
D
0.3789 1.571
2
3
D 1.27 W
Further problems on nodal analysis may be found in Section 31.3
following, problems 10 to 15, page 560.
Mesh-current and nodal analysis 559
31.3 Further problems
on mesh-current and
nodal analysis
Mesh-current analysis
1 Repeat problems 1 to 10, page 542, of Chapter 30 using mesh-
current analysis.
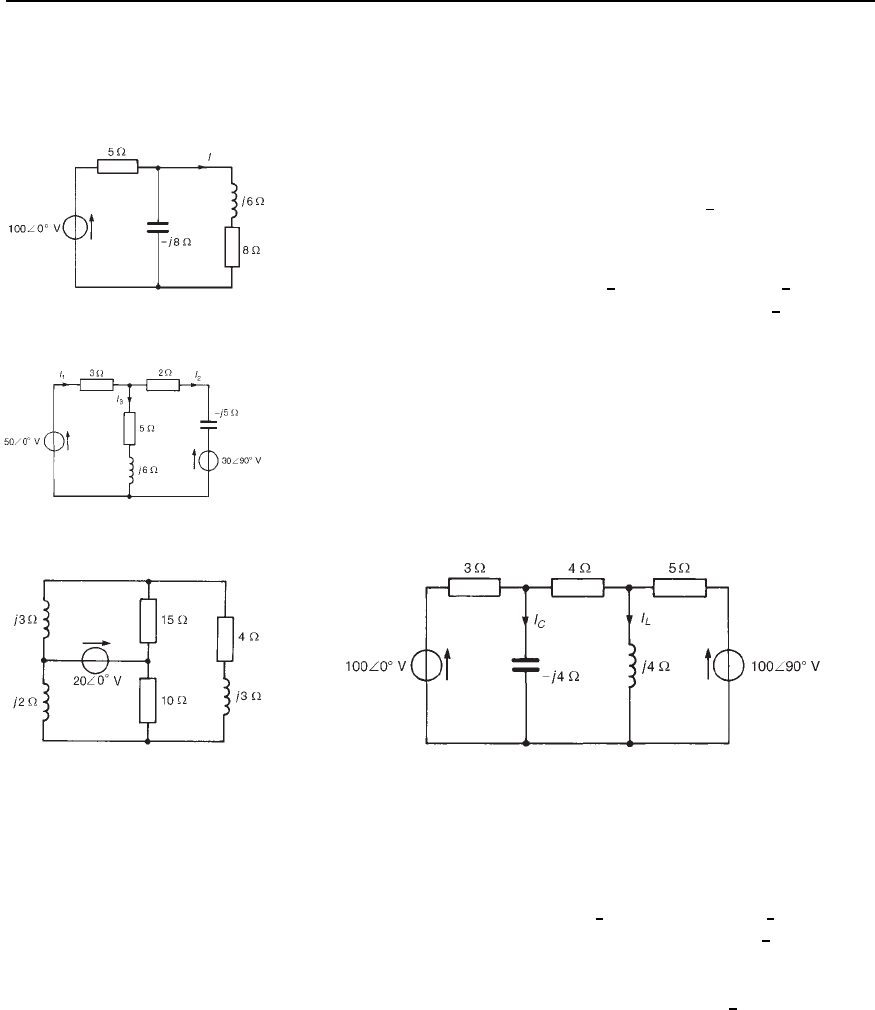
2 For the network shown in Figure 31.14, use mesh-current analysis
to determine the value of current I and the active power output of
the voltage source. [6.96
6
49.94
°
A; 644 W]
3 Use mesh-current analysis to determine currents I
1
,I
2
and I
3
for the
network shown in Figure 31.15.
[I
1
D 8.73
6
1.37
°
A, I
2
D 7.02
6
17.25
°
A,
I
3
D 3.05
6
48.67
°
A]
Figure 31.14
Figure 31.15
4 For the network shown in Figure 31.16, determine the current
flowing in the 4 C j3 impedance. [0]
Figure 31.16
5 For the network shown in Figure 31.17, use mesh-current analysis
to determine (a) the current in the capacitor, I
C
, (b) the current in
the inductance, I
L
, (c) the p.d. across the 4 resistance, and (d) the
total active circuit power.
[(a) 14.5 A (b) 11.5 A (c) 71.8 V (d) 2499 W]
Figure 31.17
6 Determine the value of the currents I
R
,I
Y
and I
B
in the network
shown in Figure 31.18 by using mesh-current analysis.
[I
R
D 7.84
6
71.19
°
AII
Y
D 9.04
6
37.50
°
A;
I
B
D 9.89
6
168.81
°
A]
7 In the network of Figure 31.19, use mesh-current analysis to
determine (a) the current in the capacitor, (b) the current in the 5
resistance, (c) the active power output of the 15
6
0
°
V source, and
(d) the magnitude of the p.d. across the j2 inductance.
[(a) 1.03 A (b) 1.48 A
(c) 16.28 W (d) 3.47 V]
8 A balanced 3-phase delta-connected load is shown in Figure 31.20.
Use mesh-current analysis to determine the values of mesh currents
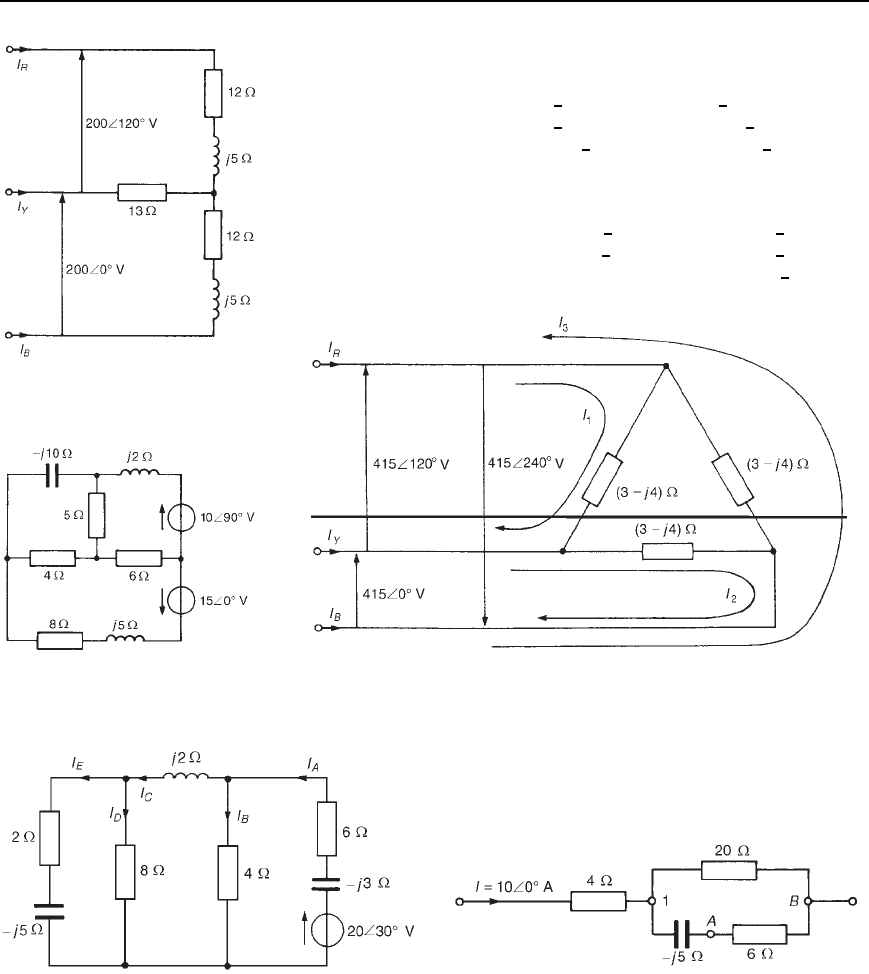
560 Electrical Circuit Theory and Technology
Figure 31.18
I
1
,I
2
and I
3
shown and hence find the line currents I
R
,I
Y
and I
B
.
[I
1
D 83
6
173.13
°
A, I
2
D 83
6
53.13
°
A,
I
3
D 83
6
66.87
°
A I
R
D 143.8
6
143.13
°
A,
I
Y
D 143.8
6
23.13
°
A, I
B
D 143.8
6
96.87
°
A]
9 Use mesh-circuit analysis to determine the value of currents I
A
to I
E
in the circuit shown in Figure 31.21.
[I
A
D 2.40
6
52.52
°
A; I
B
D 1.02
6
46.19
°
A;
I
C
D 1.39
6
57.17
°
A; I
D
D 0.67
6
15.57
°
A;
I
E
D 0.996
6
83.74
°
A]
Figure 31.19
Figure 31.20
Figure 31.21 Figure 31.22
Nodal analysis
10 Repeat problems 1, 2, 5, 8 and 10 on page 542 of Chapter 30, and
problems 2, 3, 5, and 9 above, using nodal analysis.
Mesh-current and nodal analysis 561
Figure 31.23
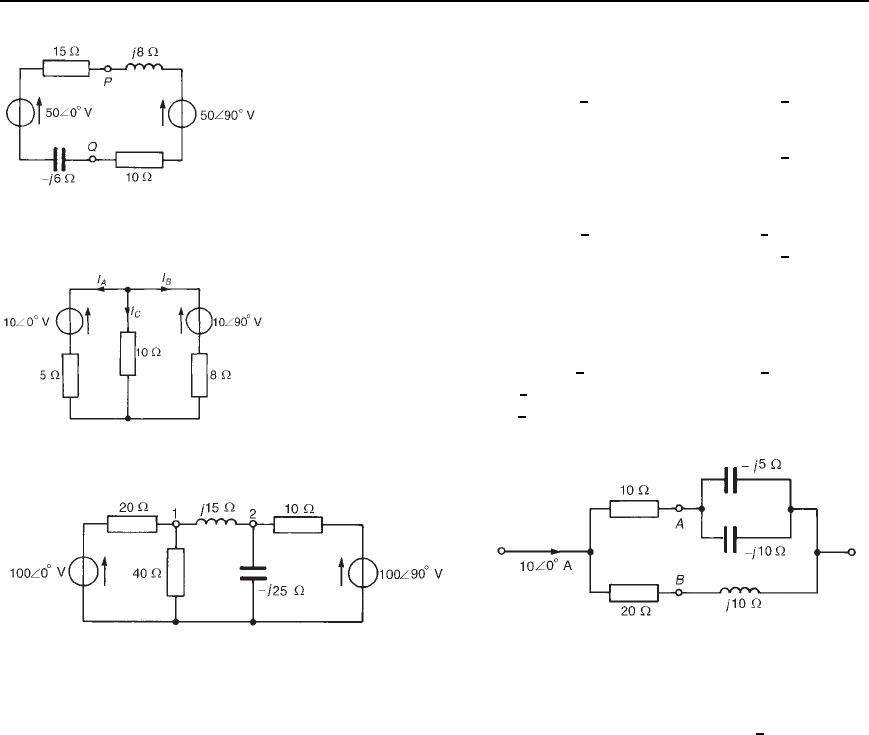
11 Determine for the network shown in Figure 31.22 the voltage at
node 1 and the voltage V
AB
[V
1
D 59.0
6
28.92
°
V; V
AB
D 45.3
6
10.89
°
V]
12 Determine the voltage V
PQ
in the network shown in Figure 31.23.
[V
PQ
D 55.87
6
50.60
°
V]
13 Use nodal analysis to determine the currents I
A
, I
B
and I
C
shown in
the network of Figure 31.24.
[I
A
D 1.21
6
150.96
°
AII
B
D 1.06
6
56.32
°
A;
I
C
D 0.55
6
32.01
°
A]
Figure 31.24
14 For the network shown in Figure 31.25 determine (a) the voltages at
nodes 1 and 2, (b) the current in the 40 resistance, (c) the current
in the 20 resistance, and (d) the magnitude of the active power
dissipated in the 10 resistance
[(a) V
1
D 88.12
6
33.86
°
V, V
2
D 58.72
6
72.28
°
V
(b) 2.20
6
33.86
°
A, away from node 1,
(c) 2.80
6
118.65
°
A, away from node 1, (d) 223 W]
Figure 31.25 Figure 31.26
15 Determine the voltage V
AB
in the network of Figure 31.26, using
nodal analysis. [V
AB
D 54.23
6
102.52
°
V]
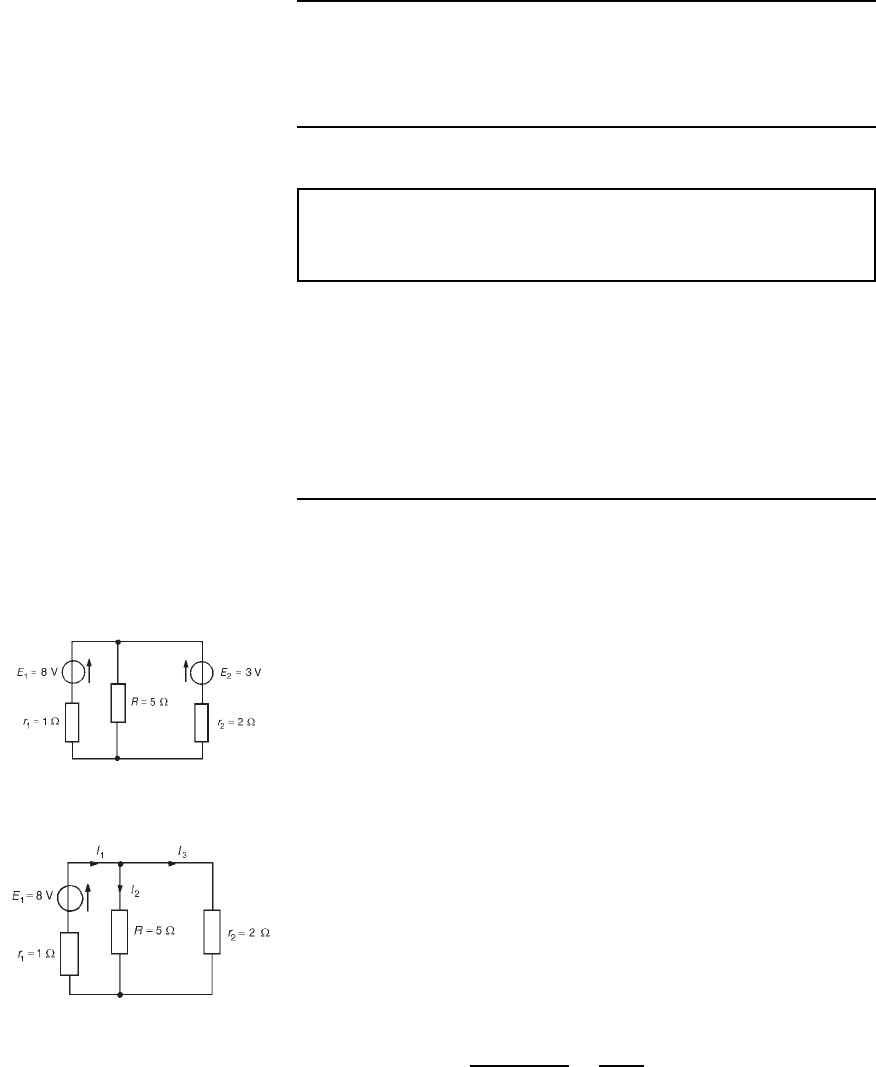
32 The superposition
theorem
At the end of this chapter you should be able to:
ž solve d.c. and a.c. networks using the superposition theorem
32.1 Introduction
The superposition theorem states:
‘In any network made up of linear impedances and containing more than
one source of e.m.f. the resultant current flowing in any branch is the
phasor sum of the currents that would flow in that branch if each source
were considered separately, all other sources being replaced at that time
by their respective internal impedances.’
32.2 Using the
superposition theorem
The superposition theorem, which was introduced in Chapter 13 for d.c.
circuits, may be applied to both d.c. and a.c. networks. A d.c. network
is shown in Figure 32.1 and will serve to demonstrate the principle of
application of the superposition theorem.
To find the current flowing in each branch of the circuit, the following
six-step procedure can be adopted:
(i) Redraw the original network with one of the sources, say E
2
,
removed and replaced by r
2
only, as shown in Figure 32.2.
(ii) Label the current in each branch and its direction as shown
in Figure 32.2, and then determine its value. The choice of
current direction for I
1
depends on the source polarity which,
by convention, is taken as flowing from the positive terminal as
shown.
Figure 32.1
R in parallel with r
2
gives an equivalent resistance of
5 ð 2/5 C 2 D 10/7 D 1.429
as shown in the equivalent network of Figure 32.3. From
Figure 28.3,
current I
1
D
E
1
r
1
C 1.429
D
8
2.429
D 3.294 A
Figure 32.2
The superposition theorem 563
Figure 32.3
From Figure 32.2,
current I
2
D
r
2
R C r
2
I
1
D
2
5 C 2
3.294 D 0.941 A
and current I
3
D
5
5 C 2
3.294 D 2.353 A
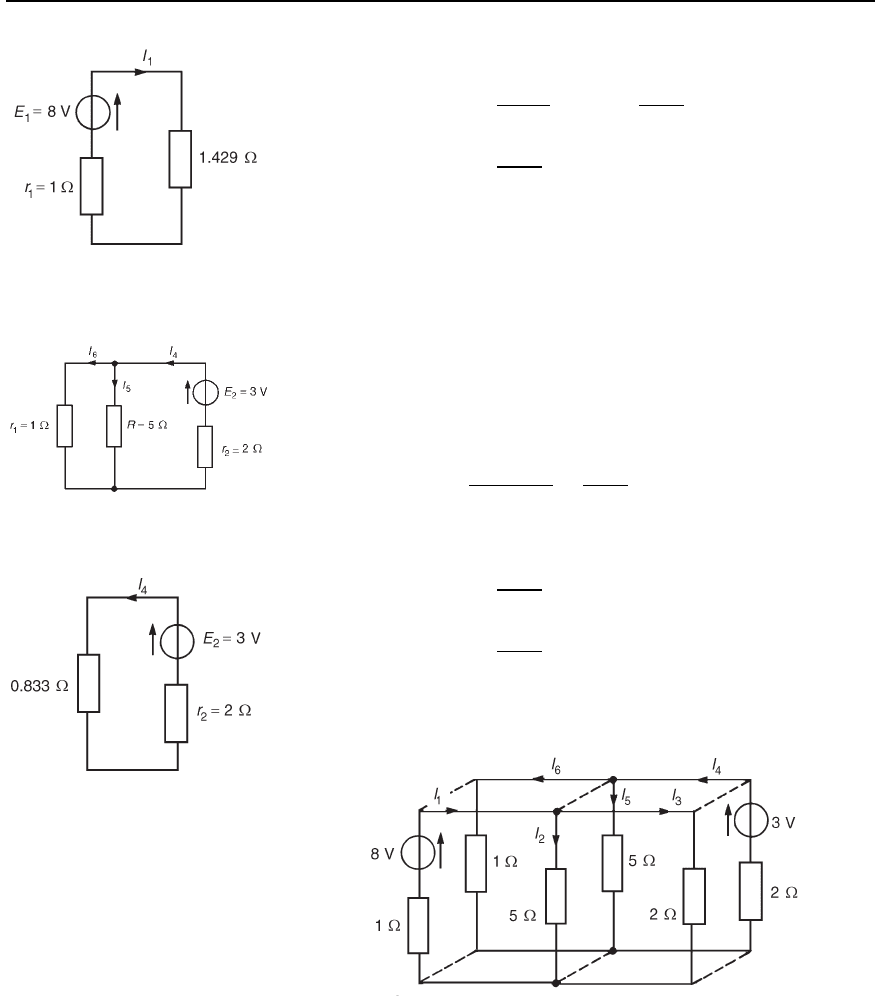
(iii) Redraw the original network with source E
1
removed and replaced
by r
1
only, as shown in Figure 32.4.
(iv) Label the currents in each branch and their directions as shown in
Figure 32.4, and determine their values.
R and r
1
in parallel gives an equivalent resistance of
5 ð 1/5 C 1 D 5/6 or 0.833 ,
Figure 32.4
as shown in the equivalent network of Figure 32.5. From
Figure 32.5,
current I
4
D
E
2
r
2
C 0.833
D
3
2.833
D 1.059 A
From Figure 32.4,
current I
5
D
1
1 C 5
1.059 D 0.177 A
and current I
6
D
5
1 C 5
1.059 D 0.8825 A
Figure 32.5
(v) Superimpose Figure 32.2 on Figure 32.4, as shown in Figure 32.6.
Figure 32.6
(vi) Determine the algebraic sum of the currents flowing in each branch.
(Note that in an a.c. circuit it is the phasor sum of the currents that
is required.)
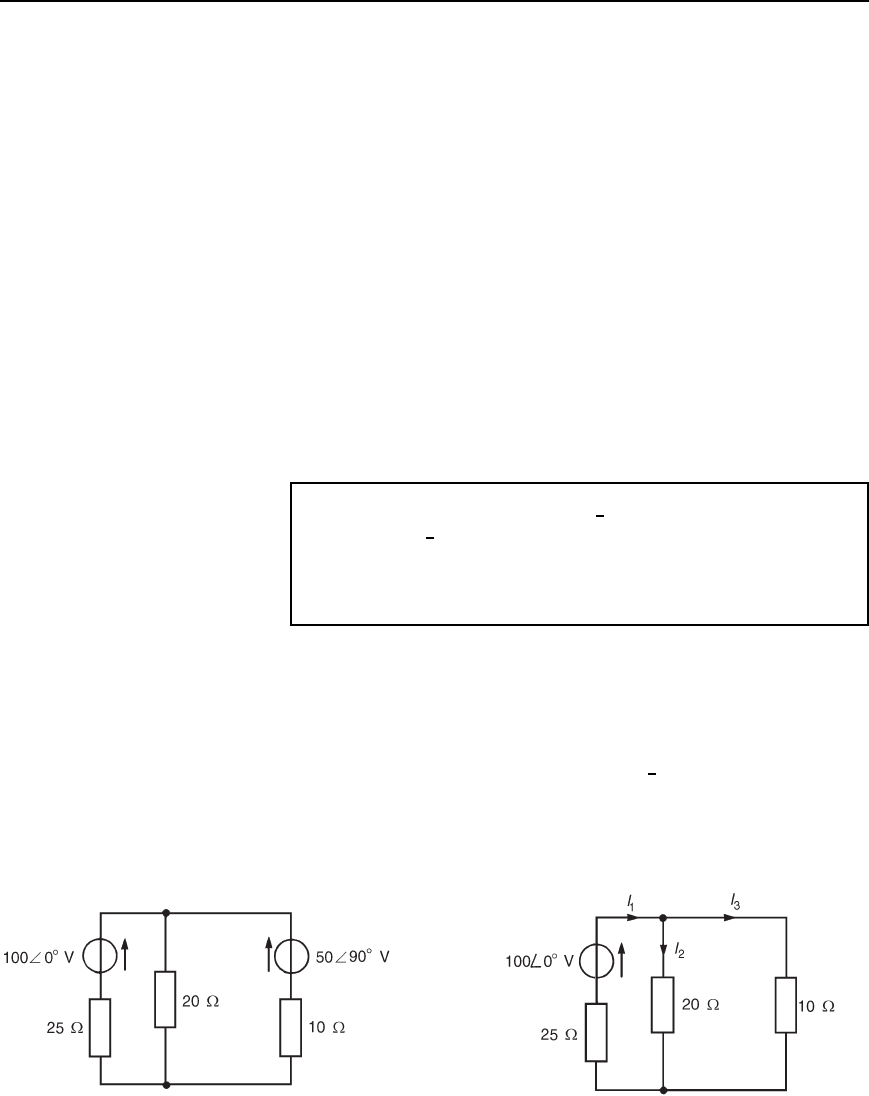
564 Electrical Circuit Theory and Technology
From Figure 32.6, the resultant current flowing through the 8 V
source is given by
I
1
I
6
D 3.294 0.8825 D 2.41 A (discharging, i.e., flowing from
the positive terminal of the source).
The resultant current flowing in th
e 3 V source is given by
I
3
I
4
D 2.353 1.059 D 1.29 A (charging, i.e., flowing into the
positive terminal of the source).
The resultant current flowing in the 5 resistance is given by
I
2
C I
5
D 0.941 C 0.177 D 1.12 A
The values of current are the same as those obtained on page 536
by using Kirchhoff’s laws.
The following problems demonstrate further the use of the superposi-
tion theorem in analysing a.c. as well as d.c. networks. The theorem is
straightforward to apply, but is lengthy. Th
´
evenin’s and Norton’s theo-
rems (described in Chapter 33) produce results more quickly.
Problem 1. A.c. sources of 100
6
0
°
V and internal resistance
25 , and 50
6
90
°
V and internal resistance 10 , are connected
in parallel across a 20 load. Determine using the superposition
theorem, the current in the 20 load and the current in each voltage
source.
(This is the same problem as problem 1 on page 536 and problem 6 on
page 553 and a comparison of methods may be made.)
The circuit diagram is shown in Figure 32.7. Following the above
procedure:
(i) The network is redrawn with the 50
6
90
°
V source removed as
shown in Figure 32.8
(ii) Currents I
1
, I
2
and I
3
are labelled as shown in Figure 32.8.
Figure 32.7 Figure 32.8
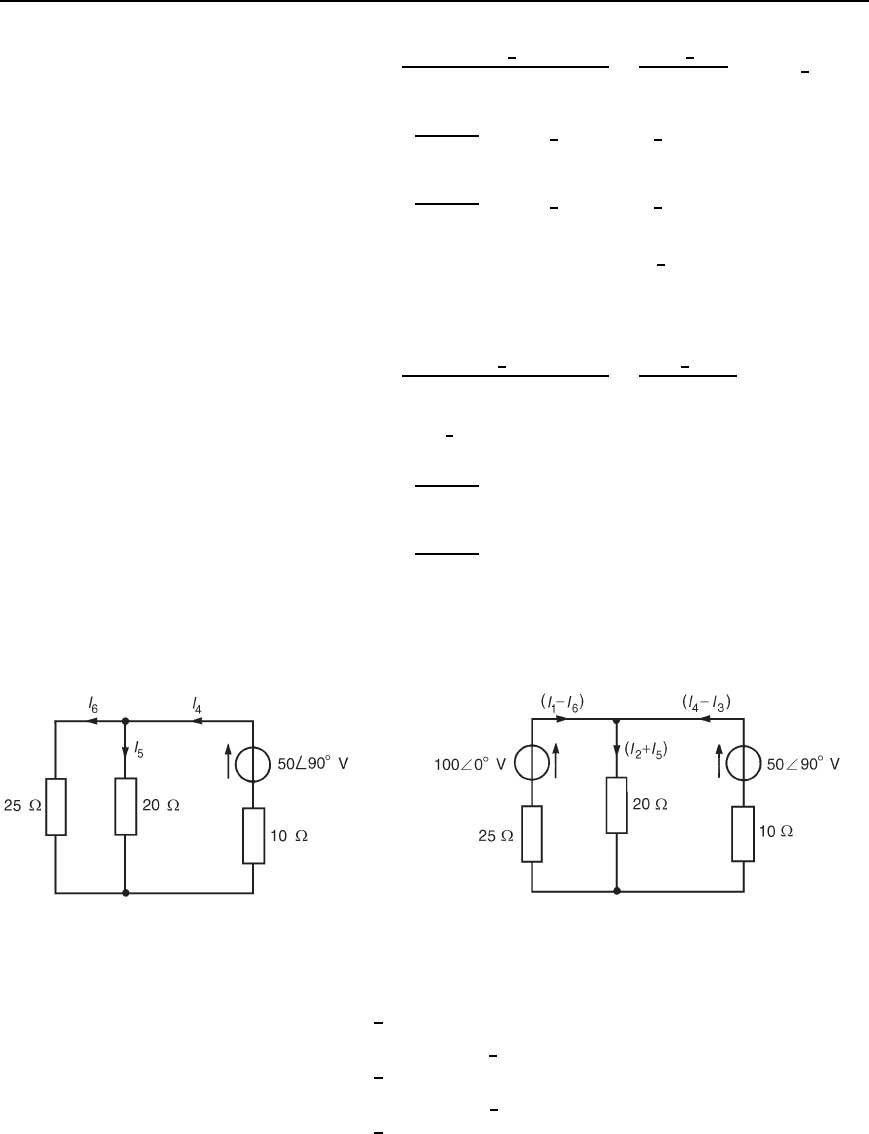
The superposition theorem 565
I
1
D
100
6
0
°
25 C 10 ð 20/10 C 20
D
100
6
0
°
25 C 6.667
D 3.158
6
0
°
A
I
2
D
10
10 C 20
3.158
6
0
°
D 1.053
6
0
°
A
I
3
D
20
10 C 20
3.158
6
0
°
D 2.105
6
0
°
A
(iii) The network is redrawn with the 100
6
0
°
V source removed as
shown in Figure 32.9
(iv) Currents I
4
, I
5
and I
6
are labelled as shown in Figure 32.9.
I
4
D
50
6
90
°
10 C 25 ð 20/25 C 20
D
50
6
90
°
10 C 11.111
D 2.368
6
90
°
Aorj2.368 A
I
5
D
25
20 C 25
j2.368 D j1.316 A
I
6
D
20
20 C 25
j2.368 D j1.052 A
(v) Figure 32.10 shows Figure 32.9 superimposed on Figure 32.8,
giving the currents shown.
Figure 32.9 Figure 32.10
(vi) Current in the 20 load, I
2
C I
5
D 1.053 C j1.316 Aor
1.69
66
51.33
°
A
Current in the 100
6
0
°
V source, I
1
I
6
D 3.158 j1.052 Aor
3.33
66
−18.42
°
A
Current in the 50
6
90
°
V source, I
4
I
3
D j2.368 2.105 or
3.17
66
131.64
°
A
566 Electrical Circuit Theory and Technology
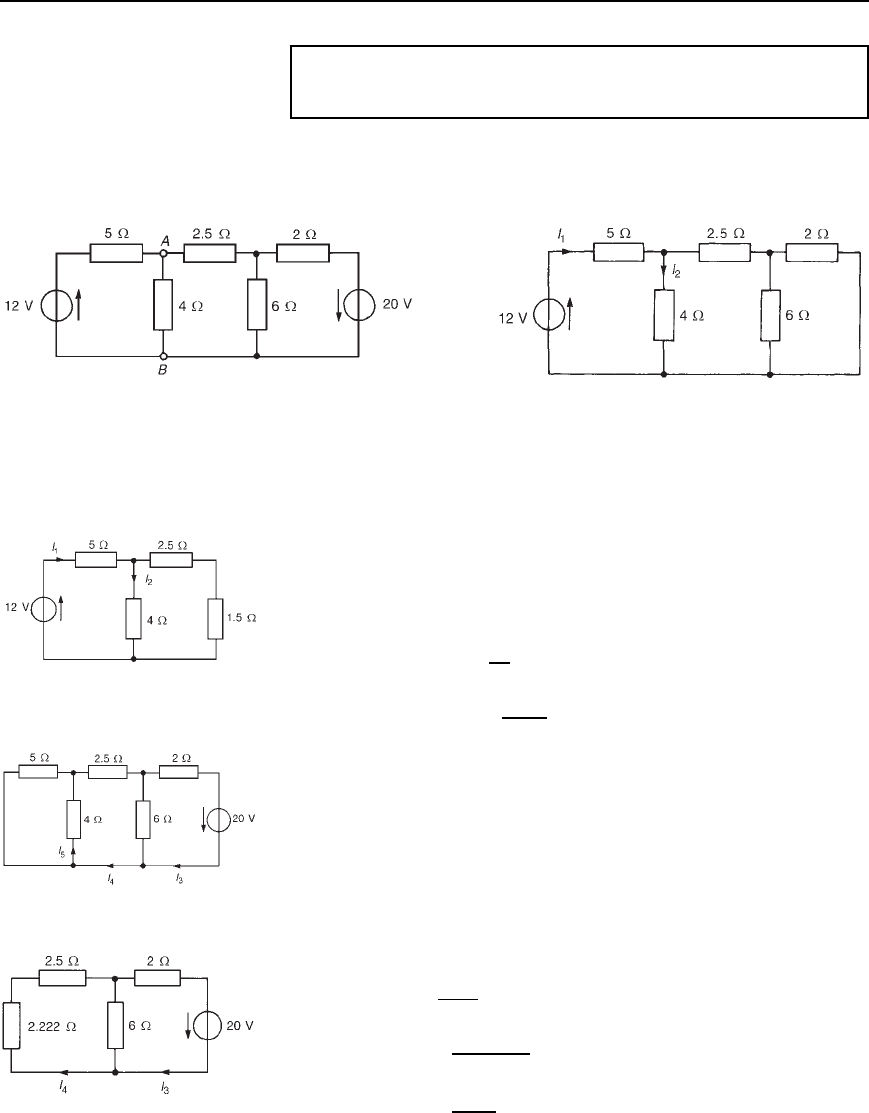
Problem 2. Use the superposition theorem to determine the
current in the 4 resistor of the network shown in Figure 32.11.
(i) Removing the 20 V source gives the network shown in
Figure 32.12.
Figure 32.11 Figure 32.12
(ii) Currents I
1
and I
2
are shown labelled in Figure 32.12. It is unnec-
essary to determine the currents in all the branches since only the
current in the 4 resistance is required.
From Figure 32.12, 6 in parallel with 2 gives
6 ð 2/6 C 2 D 1.5 , as shown in Figure 32.13. 2.5 in series
with 1.5 gives 4 ,4 in parallel with 4 gives 2 , and 2
in series with 5 gives 7 .
Thus current I
1
D
12
7
D 1.714 A and
current I
2
D
4
4 C 4
1.714 D 0.857 A
Figure 32.13
(iii) Removing the 12 V source from the original network gives the
network shown in Figure 32.14.
(iv) Currents I
3
, I
4
and I
5
are shown labelled in Figure 32.14.
Figure 32.14
From Figure 32.14, 5 in parallel with 4 gives
5 ð 4/5 C 4 D 20/9 D 2.222 , as shown in Figure 32.15,
2.222 in series with 2.5 gives 4.722 , 4.722 in parallel
with 6 gives 4.722 ð 6/4.722 C 6 D 2.642 , 2.642 in
series with 2 gives 4.642 .
Hence I
3
D
20
4642
D 4.308 A
I
4
D
6
6 C 4.722
4.308 D 2.411 A, from Figure 32.15
I
5
D
5
4 C 5
2.411 D 1.339 A, from Figure 32.14
Figure 32.15
The superposition theorem 567
(v) Superimposing Figure 32.14 on Figure 32.12 shows that the current
flowing in the 4 resistor is given by I
5
I
2
(vi) I
5
I
2
D 1.339 0.857 D 0.48 A, flowing from B toward A (see
Figure 32.11)
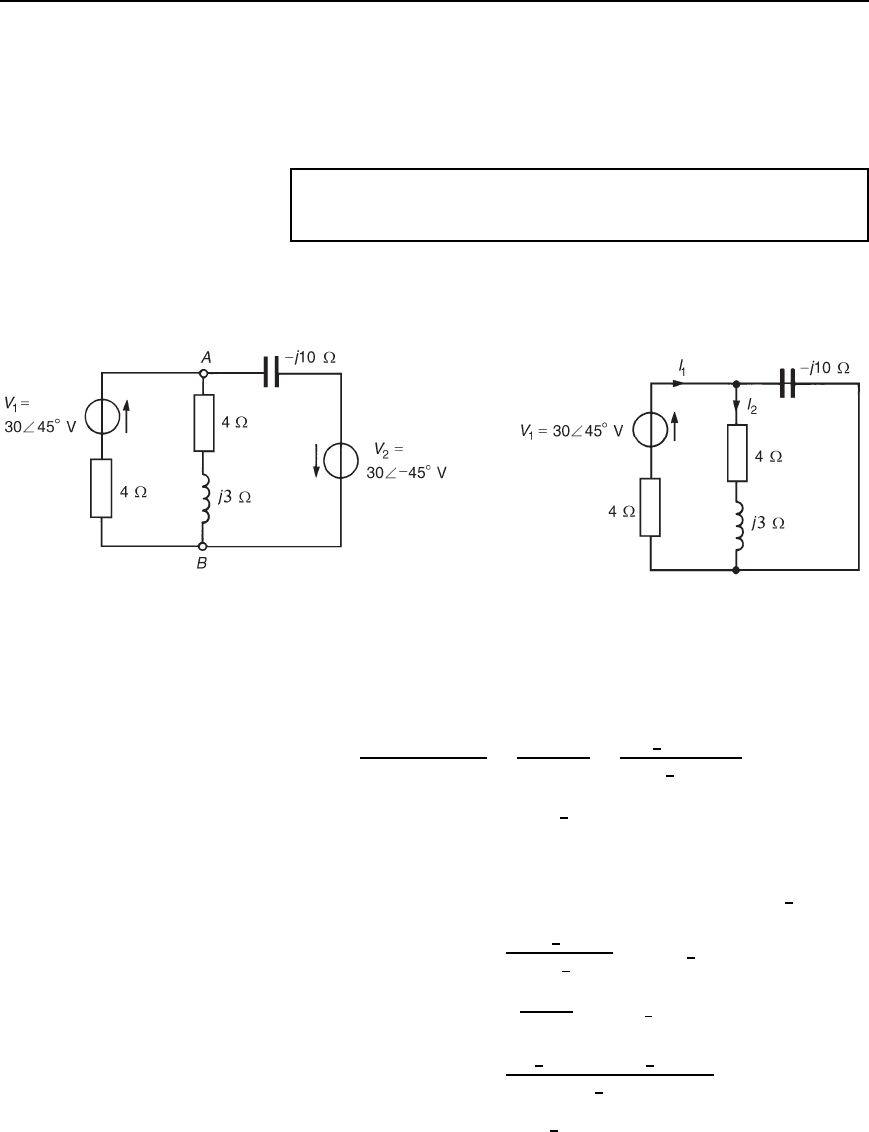
Problem 3. Use the superposition theorem to obtain the current
flowing in the 4 C j3 impedance of Figure 32.16.
(i) The network is redrawn with V
2
removed, as shown in
Figure 32.17.
Figure 32.16 Figure 32.17
(ii) Current I
1
and I
2
are shown in Figure 32.17. From Figure 32.17,
4 C j3 in parallel with j10 gives an equivalent impe-
dance of
4 C j3j10
4 C j3 j10
D
30 j40
4 j7
D
50
6
53.13
°
8.062
6
60.26
°
D 6.202
6
7.13
°
or 6.154 C j0.770
Total impedance of Figure 32.17 is
6.154 C j0.770 C 4 D 10.154 C j0.770 or 10.183
6
4.34
°
Hence current I
1
D
30
6
45
°
10.183
6
4.34
°
D 2.946
6
40.66
°
A
and current I
2
D
j10
4 j7
2.946
6
40.66
°
D
10
6
90
°
2.946
6
40.66
°
8.062
6
60.26
°
D 3.654
6
10.92
°
Aor3.588 Cj0.692A
