Bennemann K.H., Ketterson J.B. Superconductivity: Volume 1: Conventional and Unconventional Superconductors; Volume 2: Novel Superconductors

Подождите немного. Документ загружается.

540 G. Blatter and V.B. Geshkenbein

made in magnetically coupled layered supercon-

ductors where we describe melting within a self-

consistent substrate model. In this system, decou-

pling is replaced by a defect transition, the finite-

field generalization of the Berezinskii–Kosterlitz–

Thouless transition in an individual superconduct-

ing layer, and we discuss this topic in detail. Further

aspects such as the angular dependence of the melt-

ing line, T

− T-scaling, the characterization of the

first-order transition, the entanglement of the vortex

lines in the liquid phase,and the constitutiverelation

B(H), are discussed as well.

12.7.1 Fluctuations in 2D Thin Film and Layered

Superconductors

Superﬂuids and superconductors break the continu-

ous U (1) gauge symmetry and hence cannot develop

an order parameter describing true long-range order

in two dimensions [252–254]. Still, we can define a

local order parameter ¦ = |¦ |exp(i')andasuper-

ﬂuid stiffnessJ describing the low energy excitations

in the film,

F =





∇'



. (12.193)

In a thin film superconductor (of thickness d)we

have

J =



, (12.194)

with a well defined limit e → 0; screening is rele-

vant on the large scale 

eff

=2

/d which we ignore

for the time being, allowing us to replace the gauge

invariant phase difference ˜' by the phase ' of the

superconductor.

Besides the Gaussian phase ﬂuctuations described

by (12.193), the compactness of the phase variable '

allows for vortex excitations which cost an energy

vortex

= J ln(L/R

) , (12.195)

where L denotes the system size and R

is a short

scale cutoff (for a superconductor,R

=  ). The low

temperature phase is characterized by the absence of

free vortices; vortex-pair excitations merely renor-

malize the stiffness J → 

(T)J with 

< 1 [255],

while the Gaussian phase ﬂuctuations produce an al-

gebraic decay of the order parameter correlator,

¦

∗

(R)¦ (0)

['(R)−' (0)]



∼







 =

2

. (12.196)

At high temperatures the entropy T ln L

can com-

pensate for the vortex energy and vortex pairs un-

bind at the Berezinskii–Kosterlitz–Thouless temper-

ature [256,257]

BKT





∞

J , 

∞

= 

BKT

) > 0 . (12.197)

The coefficient 

∞

renormalizing the strength J of

the interaction is not universal and depends on the

short scale properties of the system; for the 2D

XY-model on a square lattice Monte Carlo simula-

tions [258] provide the result 

∞

≈ 0.569 while

typical values 

∞

∼ 0.5−0.9 are found in exper-

iments [259,260]. At high temperatures T > T

BKT

the order parameter correlations (12.196) decay ex-

ponentially with a correlation length



BKT

(T) ∝ exp





BKT

/(T − T

BKT

)



, (12.198)

diverging upon approaching T

BKT

from above. The

parameter b is again non-universal: Monte Carlo

simulations on the 2D square lattice XY-model pro-

vide the value b ≈ 3.17 [261], while typical val-

ues derived from measurements on thin supercon-

ducting films are in the range 2–16 [259, 260]). On

scales R < 

BKT

(T) the vortex pairs are still bound,

whereastheindividualvorticesbecomefreeonscales

R > 

BKT

(T), hence the density of free vortices is

∼ 

−2

BKT

(T) . (12.199)

The presence of free vortices leads to the screening

of the transverse stiffness 

J on the scale 

BKT

,hence



J(K) ∝ [1 + (

BKT

−2

]

−1

and 

J(K =0) = 0.

At the transition the exponent  takes the uni-

versal value (T

BKT

)=1/4 and the superﬂuid den-

sity vanishes with a universal jump [262]. For a

bosonic superﬂuid with superﬂuid density n

and

boson mass m this jump is given by the expres-

sion n

BKT

)/T

BKT

=2m/

. In a superconduct-

ing film the superﬂuid density relates to the or-

der parameter via |¦ |

d = n

d/4=n

/4and

12 Vortex Matter 541

the jump is larger, n

BKT

)/T

BKT

=8m/

;on

the other hand, as the superconductor is charged,

the superﬂuid density is conveniently defined via



=−j

d/A (with j

and A the supercurrent den-

sity and the gauge potential) and we find the jump in



at T

BKT



BKT

)

BKT

8c

. (12.200)

The transition itself is continuous with a singular

part in the free energy density f

sing

(T → T

BKT

) ∝



−2

BKT

and thus all derivatives are continuous.

In a superconducting film we have to account

for screening as well as the underlying mean-field

type temperature dependence of the parameters:

making use of the universal jump relation (12.200)

we find that at T

BKT

screening becomes important

on the large scale 

eff

BKT

)=¥

/16

BKT

≈

2cm/T

BKT

[K]; free vortices only appear beyond this

large distance and hence the rounding of the tran-

sition is weak. The mean-field type temperature de-

pendence mainly enters the discussion via the pa-

rameter "

d ≈ "

d(1 −T/T

) ≈ 2"

(0)d(1 −T/T

with T

the mean-field transition temperature de-

scribing the onset of pairing and the appearance of

a local order parameter |¦ | > 0(here,"

is the

Ginzburg–Landau expression for "

extrapolated to

T → 0, cf. (12.12)).The BKT transition temperature

then follows from a self-consistent solution of the

relation 

∞

BKT

)d/2=T

BKT

, cf. (12.197),

BKT

1+2T

/

∞

1+(4/

∞

)Gi

(12.201)

In a weakly ﬂuctuatingfilm the T

-reductionis small

and so is the jump in the superﬂuid density at the

transition, 

BKT

)  

(0); on the other hand,

in the strongly ﬂuctuating case the T

-reduction is

large and 

BKT

) ∼ 

(0).

The second place where the underlying mean-

field dependence shows up is the correlation length



BKT

;replacingT

BKT

in (12.198) by the tempera-

ture dependent expression (

∞

d/2)(1 − T/T

) ≡

(1 − t), cf. (12.197), we find

BKT

T − T

BKT

→

x(1 − t)

t − x(1 − t)

1+x

1−t

t − x/(1 + x)

≈

− T

T − T

BKT

, (12.202)

wherewehaveusedthatT

BKT

= x/(1 + x)re-

mains close to unity in the last expression.As a result

we find the following modified form for the coher-

ence length,



BKT

(T) ≈ (T

BKT

)exp





b(T

− T)/(T − T

BKT

)



(12.203)

with (T) the Ginzburg–Landau coherence length

[263].

In layered superconductors the Josephson cou-

pling between the superconducting planes intro-

duces a finite anisotropy parameter "

= m/M

and defines the new length scale  = d/".We

then have to compare the crossover parameter 

2

(0)/

defining the 3D ﬂuctuation regimearound

with the 2D ﬂuctuation parameter 

≈ Gi

For a strongly coupled material with 

 

the

crossover from 3D-bulk to 2D-layered behavior can

be discussed within a mean-field description. Here,

we are interested in weakly coupled material with



 

where the transition is strongly inﬂuenced

by the 2D-ﬂuctuations [70, 264]. Whereas the con-

ventionalsuperconductorsaswellastheYBCOcom-

pound belong to the first class of materials, the lay-

ered Bi- and Tl-based materials are members of the

second group with 

∼ 10

−3

Starting from decoupled layers where ﬂuctuations

are governed by vortex pairs with logarithmic inter-

action, the coupling between the layers introduces a

linear confinement potential on scales R > ,see

(12.136), leading to a cutoff in the BKT-behavior of

the system.The 3D-bulktransitionat T

occurswhen

the BKT-coherence length becomes of the order of ,



BKT

(T) ≈ ˛, hence

≈ T

BKT

b(T

− T

BKT

)

{ln[˛/(T

BKT

)]}

, (12.204)

and the width ıT

of the 3D-ﬂuctuational

regime can be estimated from the condition [265]

ıT





BKT



|≈˛,

542 G. Blatter and V.B. Geshkenbein

ıT

≈

2(T

− T

BKT

)

ln[˛/(T

BKT

)]

2b(T

− T

BKT

)

{ln[˛/(T

BKT

)]}

(12.205)

Below the bulk transition at T

and outside the

3D-ﬂuctuation regime the system still develops

strong 2D ﬂuctuations on length scales R < ˛.

With the 2D phase correlator ['(R)−'(0)]



≈

(T/

∞

d)ln(R/) we find that strong phase ﬂuctu-

ations ([' (˛)−'(0)]



∼ 1) appear within a

temperature regime

ıT

≈ T



∞

ln(˛/(T

BKT

)) (12.206)

below T

. This result for the 2D phase ﬂuctuations is

larger than the usual 2D Ginzburg criterion [233],cf.

(12.190).

The numerical ˛ determining the length scale

˛ where the interlayer coupling becomes impor-

tant can be estimated from Monte Carlo simula-

tions of the 3D anisotropic XY-model [266]: Com-

paring the relative T

-shift (T

− T

BKT

)/T

BKT

≈ 0.28

obtained numerically for a system with anisotropy

= J

⊥



=0.02 in the couplings J

⊥,

with the the-

oretical value b/[ln(˛/")]

expected from the con-

dition 

BKT

) ≈ ˛a/" one arrives at the estimate

˛ ≈ 4 (here, a denotes the lattice constant and we

use a value b ≈ 3.2). Numerical estimates for the

various temperatures in the layered BiSCCO super-

conductor then take the values (we choose parame-

ters T

≈ 100 K, d ≈ 15 Å, 

≈ 18 Å, 

≈ 1400

Å, " ≈ 1/100, resulting in "

d ≈ 1500 K, and we

choose 

∞

=0.57,b =3.2,˛ =4):T

−T

BKT

≈ 20 K,

− T

BKT

≈ 2.5K,ıT

≈ 1K,ıT

≈ 60 K.We see

that ﬂuctuationsstrongly (by 20 K) reduce the mean

field transition temperature T

as we go to the thin

film geometry, while the small coupling between the

layers induces only a small upward shift (by 2.5 K)

of T

, hence BiSCCO indeed is a “strongly 2D-like”

material.

An interesting proposal regarding the transition

of a layered material into its bulk superconduct-

ing state goes back to Friedel [245], who suggested

that the proliferation of low-energy in-plane Joseph-

son vortex-loop excitations should lead to a decou-

pling of the layers above some critical temperature

= T

loop

< T

BKT

, producing a system of electro-

magnetically coupled 2D superconducting layers.

However, as shown by Korshunov [246] this interest-

ing scenario is never realized as the loop transition

temperature always appears above the Berezinskii–

Kosterlitz–Thouless transition temperature, T

loop

d =8T

BKT

(see also [82]). We will return to this

issue below, see Sect. 12.7.5.

12.7.2 Lindemann Analysis of Vortex Lattice Melting

The theoretical analysis of melting is a difficult sub-

ject and consistent melting scenarios are known in

few special situations only, notably the dislocation-

mediated melting scheme in two dimensions dis-

cussed in Sect. 12.7.4 below, or the self-consistent

stability analysis applicable to the pancake-vortex

system in layered superconductors presented in

Sect. 12.7.5. On the other hand, a simple and prac-

tical scheme to locate a first-order melting transi-

tion is given by the Lindemann analysis providing

a stability criterion for the lattice phase: Making

use of the continuum elastic description of the vor-

tex lattice, one calculates the mean-squared thermal

displacement u



. The Lindemann criterion [267]

then states that the crystal will undergo a melting

transition once u



1/2

becomes comparable to the

lattice spacing a

, u



≈ c

.TheLinde-

mann number c

is usually chosen to be a constant

of order c

≈ 0.1−0.3. Though not rigorous, the

Lindemann melting scenario has proven very useful

and reasonably accurate in predicting the positions

of first-ordermeltingtransitionsingeneral [268]and

the line shape of the vortex-lattice melting transition

in particular. Below, we first apply the Lindemann

analysis to the vortex lattice melting in 2D thin su-

perconducting films and proceed with the discussion

of magnetically coupled layered systems, before go-

ing over to Josephson coupled [231] and continuous

anisotropic systems [12].

Our main task is the calculation of the mean-

squared thermal displacement

u



≈



(2)



+ c

(k)k

(12.207)

(k)K

+ c

(k)k



12 Vortex Matter 543

with the shear modulus c

(cf. (12.73)) and the

dispersive tilt and compression moduli c

(k)and

(k), cf. (12.68) and (12.75) (the appearance of two

modes in (12.208) accounts for the vectorial charac-

ter of the displacement field u involving two com-

ponents u

and u

). Here, we concentrate on the

first term in (12.208) involving transverse shear/tilt

modes and neglect the second term adding a contri-

bution from compression modes which is smaller

than the shear/tilt term (although comparable in

size). In anisotropic material, the tilt modulus in-

volves the bulk term (12.83) and the single vortex

contribution (12.84). The second term in the single

vortex tilt c

(cf. (12.84)) is due to the electromag-

netic coupling between thelayers and is the only term

in c

surviving the limit " → 0(decoupledlayers).

The electromagnetic contributionto the tilt modulus

is strongly dispersive and produces the large stiffness

≈ "

/2 of the vortex lines in the long-wave-length

limit k

< 1/. With increasing k

the electromag-

netic stiffness decays ∝ 1/

and the line tension

crosses over to the well known result "

≈ "

for

the anisotropic superconductor as k

increases be-

yond 1/" (note that this residual line tension is due

to the Josephson coupling and is relevant only for

" > d,whered denotes the layer separation). The

expression given in (12.84) is valid for small displace-

ments, in the elastic regime. For large displacements

> 1 the logarithm in the second term of (12.84)

should be cut on 2/u rather than k

[65]. In our

analysis below we replace the logarithm by the fac-

tor 

/(1 + ˇ

)withˇ =1/ln(1 + 4

)

producing a smooth interpolation between the hard

and soft tilt modes at large and small wave-lengths,

respectively.

2D Superconducting Films

We start with the Lindemann analysis of the vor-

tex lattice melting in an individual superconducting

layer. In calculating the mean squared displacement

(12.208) we can drop the tilt energy ∝ c

and the

integral over k

provides a factor 2/d.Theintegra-

tion over K is log-divergent — as first-order melting

is expected to involve short scales we cut the integra-

tion on a few lattice spacings ∼ ˛a

and obtain the

ratio

u



≈

ln ˛

2

. (12.208)

The resulting melting temperature is independent of

the magnetic field,

≈



2ln˛

d ≈

≈

140

2Gi

, (12.209)

where we have chosen a cutoff parameter ˛ ≈ 2and

a Lindemann number c

≈ 0.08 in the last two ex-

pressions. These values are compatible with results

from Monte Carlo simulations on the classical one-

component Coulomb plasma with logarithmic inter-

actions V(R)=−e

ln(R/L) in two dimensions [269].

The latter is characterized by the dimensionless cou-

pling parameter  = e

/T measuring the ratio of

the potential energy to the temperature T in the low-

temperature solid. Simulation of the vortex liquid

phase and subsequent comparison of the free ener-

gies of both solid and liquid phases disclose a first-

order melting transition at 

≈ 140 characterized

by an entropy jump S ≈ 0.4 k

(we go over from

the charge plasma to vortices via the replacement

→ 2"

d). Similar results have been found in a

direct simulation of the transition [270], 

≈ 142

and S ≈ 0.17 k

. The (weak) first-order nature of

this melting phenomenon found through numerical

simulations substantiates the use of the Lindemann

criterion in describing the transition.

On the other hand, in two dimensions the

above first-order melting scenario competes with the

dislocation-mediated melting scheme for which an

analytic description is available,see Sect.12.7.4.This

dislocation-mediated melting scenario predicts a

smooth two-step melting transition with an interme-

diate hexatic liquid phase between the quasi-ordered

solid and the fully disordered liquid. The melting

transition at T

≈ Aa

√

3 ≈ 0.023 A "

has the same parametric dependence as the Linde-

mann result (12.209) (the parameter A describes the

renormalization of the shear stiffness at T

). The

dislocation-mediated melting scenario is realized in

the limit of a small density of dislocations, while a

first-order melting transition (involving the collapse

of the two smooth transitions intoa single sharp one)

is expected for the situation where the dislocation

544 G. Blatter and V.B. Geshkenbein

density is largeat the transition,cf.[271].The numer-

icalsimulationsonthe2D Coulomb plasma [269,272]

as well as further resultsbased on the lowest-Landau-

level approximation describing the situation in high

magnetic fields [273–275] indicate that the 2D vortex

crystal melts in a first-order transition. However,the

numerical simulations accurately reproduce various

characteristics of the melting transition as predicted

by the dislocation-mediated melting scenario, such

as the positionof T

,the jump in the shear modulus

at the transition, and the exponent  describing

the decay of the quasi-long range crystalline order.

In layered superconductors,the field-independent

result (12.209) describes well the situation in high

magnetic fields where the in-plane shear interaction

outweighs the tilt interaction, see Fig. 12.11; using

parameters typical for the layered high-T

’s we find

≈ 10–15 K.

Electromagnetically Coupled Layered Superconductors

Next we include the electromagnetic coupling be-

tween the layers while keeping " = 0 (no Josephson

coupling yet). In the high-field regime (a

< )the

shear term in (12.208) dominates over the tilt en-

ergy and we recover the field-independent 2D-result

(12.209).For small fields with a

>  the tilt energy

becomes relevant; here, the electromagnetic contri-

bution in (12.84) is the dominant one and we obtain

the Lindemann criterion in the form

u



≈ c

≈



(12.210)



4ı

ln(1 + 4ıˇ)+

(4ı)

1/2



with ı =2c



= 

/2a

;thefirsttermorig-

inates from the soft tilt modes with k

 > 1, while

the second term involves the long wave-length modes

hardened by the electromagnetic coupling and be-

comes relevant only at very small fields a

/ >

2ln(/d), where the shear modulus is exponentially

small, cf. (12.72). Here, we concentrate on the first

term, see below for a discussion of the second term

producing a reentrant melting line at very low mag-

netic fields.At high fields B  B



= ¥

/

the shear

interactiondominates and we recover the 2D melting

result (12.209) (using (12.211) the logarithmic diver-

gence is cut on large distances by the tilt stiffness

providing an effective substrate potential; as melt-

ing involves short distances we should cut the di-

vergence at a small distance of the order of a lattice

constant a

, equivalent to calculating the quantity

[u(R ≈ a

)−u(0)]



For low fields B < B



the short wave-length tilt

modes dominate and push the melting line to high

temperatures,see Fig.12.11; expanding the logarithm

in (12.211) we find the melting line in the form

(T) ≈

2ˇ



(12.211)

≈

8ˇGi

(0)



1−



wherewemakeuseoftheinterpolationformula



(T)=(0)

/(1 − T

) in the last relation.

Josephson Coupled Layered Superconductors

We now include the Josephson coupling between the

layers which produces a finite anisotropy parameter

" > 0 and a new length scale  = d/". This addi-

tional coupling becomes relevant when a

,  > 

and favors the solid phase, thus pushing the melting

line further towards high temperatures and fields.

Accountingforthe additional single vortex elasticity

in the tilt modulus c

(k)weevaluatetheLinde-

mann criterion in the low-field regime (a

> )and

recover the previous result (12.211) with the modifi-

cation that soft tilt modes are cut on 1/"



ˇ instead

of /d, leading to the replacement of ln(...)/4ı

by (d



ˇ/")[ln(...)/4ˇı + 1]. The melting line

then takes the new form

em,J

(T) ≈

c





(12.212)

≈

c



ˇGi

(0)



(0)



1−



3/2

For (0) <  the line B

em,J

goes over into the electro-

magnetic melting line B

as the temperature drops

below

≈ T

[1 − ˇ((0)/)

]

1/2

(12.213)

12 Vortex Matter 545

(we make use of the interpolation formula 

(T)=

(0)

/(1 − T

)). For the opposite case with

strongly coupled layers, i.e.  < (0), the electro-

magnetic melting line B

is completely hidden.

Continuous Anisotropic Superconductors

As the melting line increases beyond B



the tilt en-

ergies are dominated by the dispersive bulk term

≈ 4"

(see (12.83)) and we arrive at

the result for the melting line in the continuous

anisotropic superconductor, Fig. 12.11,

(T) ≈ 4c



(12.214)



(0)



1−



At large fields (a

<  < (0)) the 2D result (12.209)

is recovered. Comparing (12.192) and (12.215) we

find a Lindemann number c

≈ 0.17; note, however,

that several approximations (e.g., we have dropped

the compression term in (12.208) and have simpli-

fied integrals) have been made in the calculation of

the result (12.215) and hence the numerical value of

the above Lindemann number should not be overin-

terpreted.

With a temperature dependence ∝ (1 − T/T

)

and ˛ > 1 the melting line is located far below the

upper critical field line H

∝ (1 − T/T

) for T close

to T

. For strongly coupled layers, e.g., in YBCO, the

melting line rises steeply at low temperatures and the

suppression of the order parameter close to H

has

to be taken into account (in BiSCCO the melting line

remains far below H

and there is no suppression of

the order parameter). The melting transition of the

vortex lattice under high field conditions has been

studied within the lowest-Landau-level approxima-

tion [276,277]: Using a high-temperature expansion

(up to 9th order) and a subsequent analysis based on

Pad´eand Borel–Pad´e summation techniques,the free

energy of the liquid phase has been calculated and

comparedto theresultfor theAbrikosov lattice phase

[278].Thetwo lines cross (with a finiteangle,indicat-

ing a first-ordertransition)at a reduced temperature

y ≡ −[1 − T/T

− B/H

(0)]/Gi(B) ≈ −7.0, where

Gi(B) is the field dependent width of the ﬂuctua-

tion region,Gi(B)=(Gi/2)

1/3

[TB/T

(0)]

2/3

 Gi

(B  GiH

(0) within the field regime considered

here). The resulting melting line takes the shape

(T) ≈ 0.08

(0)

√



1−

−

(0)



3/2

(12.215)

and is located at an appreciable distance away from

the critical ﬂuctuation regime.Assuming that B

(T)

is closeto H

(T) we obtain the scaling result 1−b

∼

(Gi t

/(1 − t)]

1/3

,whereb = B/H

(T)andt = T/T

denote the reduced field and temperature. This result

is easily reproducedwithin the Lindemann approach

by noting that B

∝ "

; taking into account the

renormalization of the elastic constants due to the

suppression of the order parameter close to H

(see

(12.4.1),"

∝ (1 − b)(1 − t)andc

∝ (1 − b)

(1 − t))

we find that B

∼ (H

(0)/Gi)[(1 −t)/t]

(1−b)

and

assuming B

(T) ≈ H

(T) we recover the above scal-

ing formula. In addition, we can obtain an estimate

for the Lindemann number c

≈ (2/7



)

1/4

≈ 0.15.

Discussion

The bulk melting line (12.215) cuts the B



line at

1−T/T

≈ Gi/c



; for YBCO this parameter is

of order 10

−4

andallthemeltinglineisgivenbythe

bulk result (12.215). The situation is quite different

in weakly coupled layered material such as BiSCCO

with (0) < : at high temperatures the melting

line is strongly suppressed to fields below B



and is

given by the expression (12.213) with its characteris-

tic(1−T

)

3/2

power law behavior; this 3/2power-

law is valid provided that /



ˇ <  < a

and

sensitively depends on the values of the various pa-

rameters (e.g., assuming (0) ≈ 2000 Å, " ∼ 1/300,

and ˇ ≈ 0.2wefindthatT

≈ 0.8 T

).Note that the

results (12.212), (12.213), and (12.215) not only dif-

fer in their temperature scaling but also in their de-

pendence on the anisotropy parameter ", B

∝ "

em,J

∝ "

,andB

∝ "

. This implies that the ex-

traction of a numerical value for the anisotropy pa-

rameter " from experimental data on the melting

line [162,279] is problematic as it is aprioriunclear

to which shape of the melting line the data should

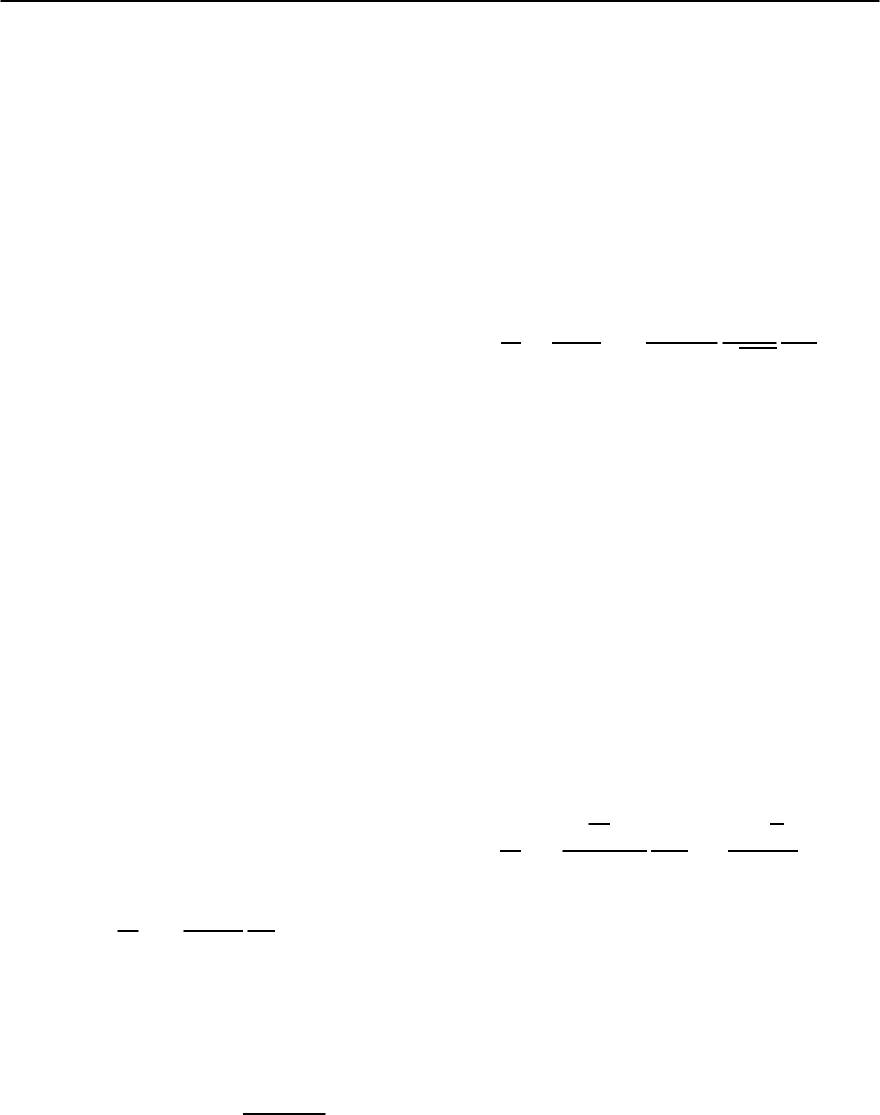
546 G. Blatter and V.B. Geshkenbein

be compared to.The analysis of the melting line can

provide a reliable estimate for the anisotropy param-

eter only if " is large enough, such that either the

bulk result(12.215) is valid or the mixed electromag-

netic/Josephson result (12.213) can be identified via

its particular line shape. On the other hand, if the

anisotropy parameter is very small, say " < 1/500,

the melting line is dominated by the electromagnetic

coupling and no anisotropy parameter can be ex-

tracted.

Reentrant Melting

Up to now we have considered melting driven by in-

creasing thermal ﬂuctuations. However, the vortex

crystal is not a generic crystal as obtained from com-

peting attractive and repulsive interactions produc-

ing a potential minimum and hence a well defined

lattice constant. Rather, the interaction between the

vortices (so far) is purely repulsive and the lattice

constant is determined by the pressure of the exter-

nal H field(but cf.Sect.12.7.8).Decreasing H,thedis-

tance between vortices increases and screening leads

to an exponentially weak interaction ∝ exp(−a

/);

correspondingly the shear modulus vanishes expo-

nentially fast, cf. (12.72), and any finite temperature

melts the crystal at sufficiently low fields.As a result,

the vortex crystal can melt upon decreasing the mag-

netic field and we obtain a reentrant melting line, cf.

Fig. 12.11.

Using the result for the mean-squared displace-

ment (12.208) and the Lindemann criterion we ob-

tain the melting condition (12.211). At low fields the

parameter ı ∝ exp(−a

/) is exponentially small,

the second term in (12.211) dominates, and we ob-

tain the reentrant branch of the melting line,

(T) ≈







4c

(3)

1/4





−2

. (12.216)

Note that this result involves the long wave length

tilt modulus "

∼ "

and hence is independent of

the anisotropy parameter ". Choosing typical val-

ues for the Lindemann number and for the pen-

etrationdepthwehave4c

/(3)

1/4

≈ 0.2and

(0)(0) ∼ 10

K, resulting in a reentrant melting at

(T) ≈ B



/[2 ln(2. 10



1−T

/T)]

with a

maximum B

max

of the order of a few Gauss realized

at a temperature T

max

≈ T

/3. We conclude that the

low-field reentrance is a rather delicate phenomenon

appearing at very low magnetic fields of the order of

a few Gauss. Traces of a dilute liquidphase have been

observed in Bitter decoration experiments on BiS-

CCO single crystals [280].

The high and low-field branches of the melting

line cut in the vicinity of T

.Fortheextremecase

of an electromagnetically coupled system the two

branches merge at

1−

≈

ˇGi





(2ˇ)

1/2

(3)

1/4

√

(0)



−2

(12.217)

and no solid phase can exist at high temperatures

beyond T

. Using typical parameters for the layered

high-T

materials and adopting a value c

≈ 0.17

for the Lindemann number (cf.Fig.12.11) we find T

close to T

,1−T

≈ 0.05 (note that even a small

but finite parameter " pushes T

up close to T

,see

below and Fig. 12.11). The reentrance at T

ends up

in the critical region close to T

;whileourapproach

does account for the ﬂuctuations of the phase-field

via the thermal motion of vortices,itneglects ﬂuctu-

ations in the amplitude of the order parameter and

hence our analysis breaks down in this regime.

Including a finite Josephson coupling " > 0the

melting line B

em,J

(see (12.213)) and the crossing

point of the lower and upper branch of the melt-

ing line are shifted towards higher temperatures, cf.

Fig. 12.11,

1−

≈





2ˇGi

c



(0)







(3)

1/4



−2



(12.218)

Cutting the lower branch of the melting line with

the bulk melting temperature (12.215), we find a

result deep in the ﬂuctuation regime, 1 − T

≈

(Gi/8c



)/[ln(...)]

A similar reentrance also appears in a 2D vor-

tex system: in this case the low-field limit (a



eff

=2

/d) of the shear modulus decays al-

gebraically [281] rather than exponentially, c

≈

0.46 "



eff

∝ B

3/2

, and we obtain the low-field

branch of the 2D melting line in the form

12 Vortex Matter 547

Fig. 12.11. Low-field phase diagram of anisotropic/layered

superconductors. Reduced units b = B/(¥

/(0)

)and

t = T/T

havebeen used (c

=0.17 and parameters (0) ≈

2000 Å, d ≈ 15 Å, T

≈ 100 K). The dashed line shows the

result for the isolated 2D layer. The solid lines illustrate the

3D bulk results for anisotropy parameters " = 0 (only elec-

tromagnetic coupling), " =1/1000, 1/300, 1/100, and 1/8

(data derived from numerical evaluation of (12.208) and

application of the Lindemann criterion u



= c

). The

dotted line traces b



(t)=B/[¥

/

(t)]

≈

◦



eff



37 T



. (12.219)

The result for the melting line of an isolated layer is

illustrated in Fig.12.11 (dashed line).

The reentrant melting lines defined by (12.212),

(12.213), (12.215), and (12.216) are illustrated in

Fig. 12.11. Combining the various elements in the

above discussion, we can easily understand the char-

acteristic “nose”-like shape of the melting line of a

3D vortex crystal: The melting lines of most conven-

tional 3D crystals comewith a positive slope @

p > 0,

with the notable exception of the ice–water transi-

tion which involves a retrograded melting line with

p < 0 (in this comparison between vortex and

conventional crystals we have to identify the induc-

tion H with the pressure p). A soft 2D vortex crystal

with c

∝ a

−2

exhibits an upright melting line with

independent of H over a large portion of the

phase diagram. Going over to a 3D vortex crystal via

electromagnetic coupling of superconducting layers,

the presence of the vortex crystals in the other layers

stabilizes the solid phase; considering one 2D vor-

tex crystal in a particular layer, the vortex lattices in

the other layers provide a stabilizing substrate po-

tential which pushes the melting transition tohigher

temperatures.Finally,theJosephson coupling further

enhances the interaction between the layers and the

melting line is pushed up towards the mean-field

line as the pancake-vortex stacks transform into

increasingly stiffer vortex lines. While the upward

shift of the melting line with decreasing anisotropy

is a consequence of an increase in the layer coupling

and the establishment of vortex lines, the reentrant

part of the low-field melting line is a consequence

of the collapse of the vortex-vortex interaction due

to screening, rendering the vortex crystal super-soft

at low fields with c

∝ exp(−a

/). In summary,

the coupling of the soft 2D pancake-vortex crystals

into a 3D crystal of vortex lines leads to an increas-

ingly steeper retrograded melting line and the 3D

vortex crystal melts like ice, resulting in a vortex liq-

uid phase which is denser than the crystal.

Angular Dependence of Melting

In anisotropic superconductors an additional degree

of freedom in setting up the system is the angle 

between the magnetic field and the c-axis of the ma-

terial (alternatively we may use the angle # = /2−

with respect to the planes).In continuous anisotropic

material the upper part of the melting line involves

fields B > B



and the characteristic scale of the melt-

ing process is small, a

< .Inthissituationwecan

use the scaling rule (12.170) and obtain the angle

dependence of the melting line,

(T, # )=

(T, /2) . (12.220)

The prediction (12.220) is in excellent agreement

with experiments on YBCO crystals [282,283].

In weakly coupled layered superconductors the

most interesting part of the melting line appears at

low fields B ∼ B



and the vortex lattice is expected to

rearrange into a crossed lattice,see Sect.12.5.5,hence

simple anisotropic scaling may fail. Indeed, experi-

ments [218–221] show that the c-axis component H

548 G. Blatter and V.B. Geshkenbein

of the melting field decreases linearly with the in-

plane component H

. Such a behavior can be under-

stood on the basis of the discussion in Sect. 12.5.5,

see [210]: We start from the thermodynamic condi-

tion for melting (at fixed magnetic field H),

)+ıg

, H

)

= g

)+ıg

, H

) , (12.221)

with g

)andg

) the Gibbs free energy densi-

ties of the crystal and liquid statesfor a fieldarranged

along the c-axis; the corrections ıg

, H

)and

ıg

, H

) account for the effect of the parallel

field H

. Expanding H

)=H

(0) + ıH

and

the Gibbs free energies (we use @

g =−B/4)

)−g

(0) + ıH

)−g

(0) + ıH

) ≈

(0)) − B

ıH

4

− g

(0)) + B

ıH

4

we obtain the shift (note thatg

(0))−g

(0))

=0)

ıH

≈ −4g(H

(0), H

)/B , (12.222)

with

g(H

(0), H

)=ıg

(0), H

)−ıg

(0), H

)

and the jump B = B

− B

> 0 in the induction.In

the vortex crystal the correction ıg

, H

) ≈ f

is given by the energy density (12.156)of the Joseph-

son vortex lattice; assuming an efficient layer decou-

pling upon melting the Josephson term in (12.156)

vanishes and the correction ıg

, H

)involves

the field energy alone, hence g ≈ f

− H

/8 ≈

/2¥

)

√

g ""

ln("¥

√

) and we arrive at

the linear (in H

)shift

ıH

≈ "B



4B



c(T)B



4H







1/2

(12.223)

The factor c(T) ≡cos(¥

n,n+1

)

accounts for the

suppression of the Josephson coupling due to the

thermal ﬂuctuations of the pancake vortices, cf.

[210]; at the (H ˆz) melting transition c ≈ 0.64

[25,284].

Comparison Theory–Exp eriment

How well do the above theoretical considerations on

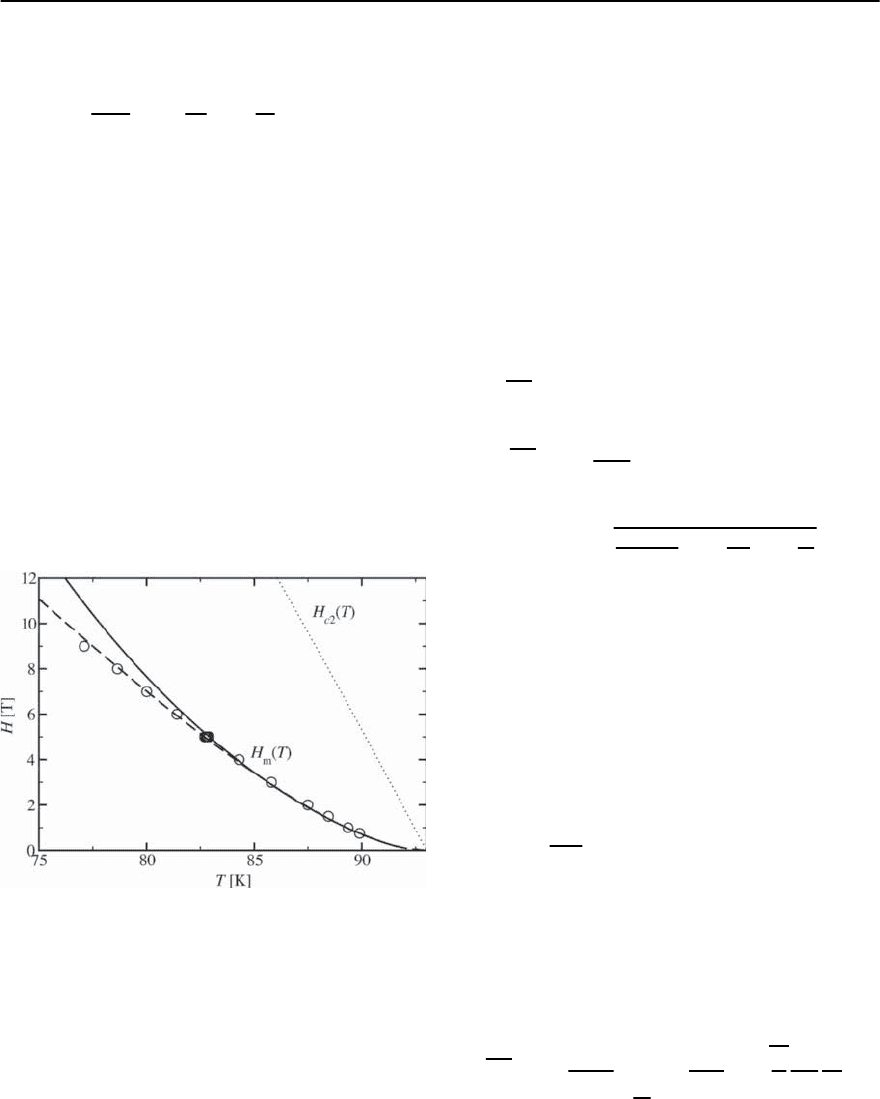
vortex lattice melting agree with experiments? A di-

rect comparison can be carried out for continuous

anisotropic superconductorssuch asYBCO — in this

situation the scaling analysis combined with results

from numerical simulations provide us with the the-

oretical prediction (12.192) which contains no un-

known parameters. On the experimental side, pen-

etration depth measurements (surface impedance)

by Kamal et al. [285] provide us with the result

(T) ≈ .895 (0)(1−T/T

)

−1/3

valid at high tempera-

tures T > 80 K,with (0) = 1400 Å and T

=92.5K.

The accurate position of the melting line is known

from experiments by Schilling et al. [42]. The com-

parison between the theoretical expression (12.192)

and the experimental data points is given in Fig.12.12

[286]: choosing a standard value " ≈ 1/8 for the

anisotropy close to T

we find excellent agreement

between theory and experiment for fields below 5

Tesla; unfortunately, the measurement of (T)and

of the melting line have not been carried out on the

same sample, which may be responsible for the de-

viations observed at higher field values. This com-

parison illustrates the consistency we have reached

in our understanding of vortex lattice melting: once

the material anisotropy and the temperature depen-

dence of the superﬂuid density are known we can

accurately predict the location of the melting line.

The penetration depth measurements of Kamal

et al. [285] on YBCO single crystals report to find a



−2

∝ (1 − T/T

)

2/3

scaling over a region of order T

itself, while in the discussion above we have usually

assumed a mean-field type scaling of the superﬂuid

density with 

−2

∝ n

∝ (1 − T/T

). Indeed, close

to T

one expects that ﬂuctuations change character

and exhibitcriticalscaling:with¦ (r)=

√

exp(i')

a two-component order parameter field, the criti-

cal ﬂuctuations belong to the 3D–XY universality

class [16] and the superﬂuid density is expected to

scale as a power law with an exponent close to 2/3,

hence 

−2

∝ n

∝ (1 − T/T

)

2/3

(note that  ∝ 

hence  ∝ 1/ → 0, the charge e →∞becomes

relevant, and the system seems to approach a type I

behaviorvery close to T

).Such a criticalscaling then

12 Vortex Matter 549

implies a bulk melting line which scales with a 4/3

power law,

≈



(0)



1−



4/3

. (12.224)

The dispute then is about the range where critical

ﬂuctuations should be expected: the usual Ginzburg

criterion which estimates the width of the specific

heat transition predicts a rather narrow critical re-

gion of the order of 1 Kelvin.On the other hand,other

quantities may exhibit a different critical range, cf.

equation (12.206) which predicts a larger critical re-

gion in terms of 2D phase ﬂuctuations than expected

from the conventional Ginzburg criterion.

Note that the mean-field based power law (12.215)

predicts a melting line which is too steep when com-

pared to typical experiments on YBCO [42]; taking

into account the suppression of the order parame-

ter at high fields close to H

, cf. (12.215),produces a

ﬂatter melting line also within the mean-field scaling

scheme, see Fig. 12.12.

Fig. 12.12. Melting line in YBCO. The experimental data

points (circles) are taken from [42].The full line shows the

theoretical result (12.192) with a penetration depth (T)

taken from experimental data [285] and an anisotropy pa-

rameter " =1/8 for YBCO close to T

.Thedashed line is a

fit to the melting line using the result from a London model

(with (T

−T)

1/2

-scaling and additional fitting parameters)

taking into account the suppression of the order parameter

at high fields close to H

12.7.3 Lindemann Analysis of Layer Decoupling

In layered systems an additional thermodynamic

transition takes the 3D bulk system into a system of

decoupled 2D layers [72]. The loss of inter-layer co-

herence is due to strong thermal ﬂuctuations of the

pancake vortices within the individual layers and the

transitionlinecan be estimated within a Lindemann-

typeapproach[72,231].A convenientstarting pointis

the free energy (12.154) describingthe phase degrees

of freedom in the presence of pancake vortices — the

mutual locking of the phase between layers is due to

the electromagnetic and Josephson coupling terms.

Using the equipartition theorem we determine the

mean squared thermal ﬂuctuation amplitude of the

phase

ı' within one layer,



ı'



≈



dK (12.225)

16(1+g)

1+g



andfindthelineintheB-T diagram where this quan-

tity becomes of order unity. The calculation of the

integral in (12.226)is trivial but involves many cases:

below we distinguish between the two cases of weak

(

/ <  < ) and strong ( <  < 

/)

interlayer coupling. We will use the estimate g ≈

ln(a

/d)/4

≈ B

/B for the parameter g,cf.

(12.150).

At high fields, the shear term ∝ K

is dominant

and g is small, 1 + g ≈ 1: the shear term domi-

nates over the Josephson and electromagnetic terms

if K > 1/

√

 and K > 1/, respectively (we drop

numericals in this qualitative discussion). Also, we

can disregard the electromagnetic energy as com-

pared to the Josephson energy if K < /a

.Asa

result, we can ignore the electromagnetic contribu-

tion to the integral (12.226) if the high-field condi-

tion a

< 

/ is satisfied and we obtain



ı'



≈

4T

4/a



√

2/a



≈





(12.226)