Bennemann K.H., Ketterson J.B. Superconductivity: Volume 1: Conventional and Unconventional Superconductors; Volume 2: Novel Superconductors
Подождите немного. Документ загружается.


510 G. Blatter and V.B. Geshkenbein
with
c
l
= ¥
2
0
/2c
2
2
n
the viscous drag coefficient
for a vortex aligned with the c-axis of the crystal (
n
the usual in-plane resistivity).
A material with a parameter !
0
r
1 is classi-
fied as belonging to the“superclean”limit; in this case
the level broadening /
r
duetoquasi-particlescat-
tering is smaller than the level separation !
0
.The
vortex equation of motion then is determined by the
compensation of the Lorentz force f
L
= n
s
v
s
∧ˆz
and the transverse force f
⊥
=−nv
L
∧ˆz.Wesplit
the transverse force into two terms involving the
superfluid density n
s
and the normal component
n
n
= n − n
s
, f
⊥
=−n
s
v
L
∧ˆz − n
n
v
L
∧ˆz,and
combine the term ∝ n
s
with the Lorentz force into
the Magnus force f
M
= n
s
[v
s
− v
L
] ∧ˆz.Thesec-
ond term is identified as the Iordanskii force [491]
f
I
= n
n
[v
n
−v
L
] ∧ˆz and we obtain the equation of
motion in the superclean limit
f
M
+ f
I
= n
s
[v
s
− v
L
] ∧ˆz + n
n
[v
n
− v
L
] ∧ˆz =0
(12.47)
(in our derivation above we have assumed a zero ve-
locity v
n
= 0 for the normal component).As a result,
the vortex line is dragged along with the center of
mass motion of the liquid, as is usually the case in
bosonic superfluids [136].In such superclean mate-
rial the Hall field E =[B∧v
L
]/c ⊥ j is expected
to be large as the vortices move along the direction
of the current; assuming the normal fluid is at rest,
v
n
= 0, the transverse flux-flow conductivity takes
the form
⊥FF
= enc /B.
The transverse force f
⊥
acting on a vortex is the
result of a subtle addition/cancellation of various
terms: when dragging the vortex line with a velocity
v
L
through the fluid which is at rest in the labora-
tory frame of reference (v
s
=0=v
n
)thetransverse
force −nv
L
∧ˆz actingonthevortexcombinescom-
ponents of the Magnus and Iordanskii forces and
involves the total density n; on the other hand, as-
suming the normal component to be at rest in the
lab frame (v
n
= 0), the Lorentz force acting on a
vortex line at rest is given by n
s
v
s
∧ˆz and in-
volves only the superfluid component n
s
.Further-
more, when quasi-particles in the vortex core are
scattered they transfer momentum to the underlying
lattice. On the one hand, this produces the dissipa-
tive force
l
v
L
,butatthesametimethetransverse
force f
sf
= n[1/(1 + !
2
0
2
r
)]v
L
∧ˆz is generated,
the so-called spectral-flow force [137]. Hence the
scattering of quasi-particles in the vortex core not
only produces dissipation but is also responsible for
the cancelation of the Magnus force; the vanishing
of the Hall component ∝ ˛
l
in the equation of mo-
tion (12.44) in dirty and clean (but not superclean)
superconductors then is the consequence of the mu-
tual cancelation of the hydrodynamic Magnus force
and the spectral-flow force due to quasi-particle scat-
tering. Therefore,the conventional situation in type-
II superconductors involves a large dissipative and
only a small Hall component in the vortex motion,
resulting in a small Hall angle
tan
Hall
=
v
L
v
⊥
L
=
˛
l
l
, (12.48)
where v
L
and v
⊥
L
denote the vortex velocity compo-
nents parallel and perpendicular to the current den-
sity j.
The Hall component is substantially modified
when particle-hole symmetry is broken [138, 139].
Then the quasi-particle density n
c
in the core is dif-
ferent from the asymptotic density n.Breakingthe
particle-holesymmetry througha non-constantden-
sity of states with @
E
N(E) = 0 produces a (small)
vortex charge [140–142] of order ek
F
(
TF
/)
2
(
TF
denotes the Thomas Fermi screening length) and
a correction to the Hall coefficient ˛
l
which might
take either sign, a circumstance which may be re-
lated to a peculiar sign change in the Hall effect
observed in some conventional and most high tem-
perature superconductors [143–145].The correction
to the Hall coefficient ˛
l
is easily derived via the
above Drude-like analysis based on the force equa-
tion (12.41) [146]: in this case the transport current
density j has to be evaluated in the vortex co-moving
system,
˜
j = en
c
˜v
c
where
˜
j = j − env
L
and ˜v
c
= v
c
−v
L
.
The additional term ∝ ın = n
c
− n in the expression
for the transport current density
j = en
c
v
c
− eınv
L
(12.49)
then compensates for the asymptotic density n in
the electric field force,see (12.41), replacing it by the
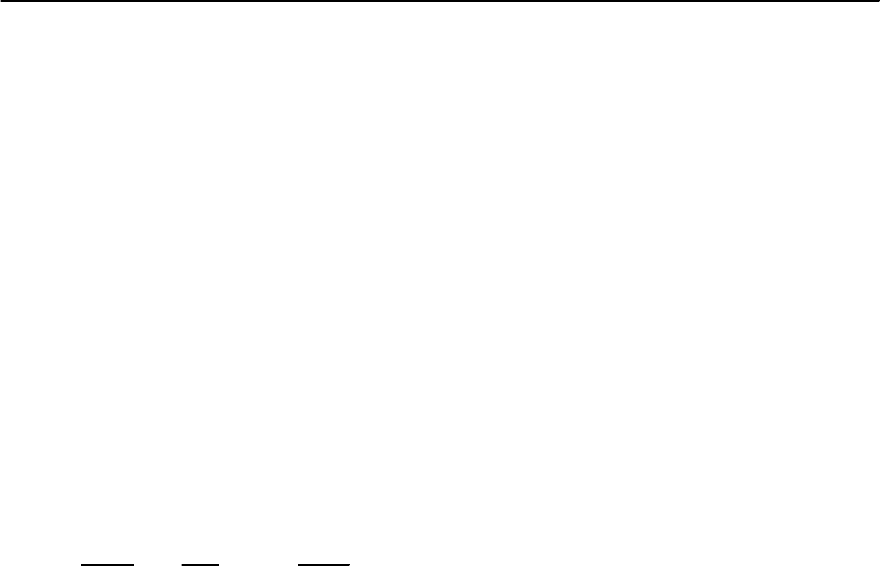
12 Vortex Matter 511
density n
c
in the core. As a result, the force density
equation (12.41) involves only the core density n
c
and the velocity relation (12.43) remains unchanged.
Substituting (12.43) into (12.49) and taking again
the cross product with (¥
0
/c)ˆz (note the substitu-
tion n → n
c
) we recover the force equation (12.44)
with two modifications:i) the relevant density enter-
ing the dynamical coefficients
l
and ˛
l
now is the
density n
c
in the core, and ii) the correction term
∝ ın = n
c
− n in the expression for the trans-
port current density j adds to the Hall coefficient,
˛
l
= n
c
[!
2
0
2
r
/(1 + !
2
0
2
r
)−ın/n
c
]. Hence, de-
pending on the sign of the density modulation ın in
the vortex core,the Hall coefficient˛
l
may reverse its
sign. Note that it is the unscreened density modula-
tion ın /n ∝
2
/
2
F
which enters the expression for
the Hall coefficient [146].
Let us then have a closer look at the origin of the
density modulation ın: Starting from microscopic
(weak coupling) BCS theory, ın is related to the
particle-hole asymmetry parameter @
E
N(E)via
ın ∼ −
dN(E)
dE
2
ln
!
D
T
c
∼ −N
2
@ ln T
c
@
(12.50)
and is of order n
2
/
2
F
(the Debye frequency !
D
pro-
vides the high energy cutoff, N(E) denotes the nor-
mal electron density of states). Here, we have used
the relation @
E
N ln(!
D
/T
c
)=N@
ln T
c
deriving
from the BCS expression T
c
∼ !
D
exp(−1/NV )
in order to express the result through the phe-
nomenological parameter @
T
c
.Alternatively,the
density modulation ın can be found using phe-
nomenological considerations via the appropriate
derivative of the free energy density, ın =−@
f |
∞
0
with f =−˛
0
(T
c
− T)|¦ |
2
+ ...,henceın =
−(H
2
c
/4)@
T
c
=−N
2
@
ln T
c
, and we recover
the result (12.50). The phenomenological parame-
ter @
T
c
also enters the derivation of the Hall con-
ductivity via the time-dependent Ginzburg–Landau
(TDGL) approach [138, 139]: The non-dissipative
dynamical term can be derived from the free en-
ergy functional by exploiting the dependence of
T
c
( + e¥ ) on the local electro-chemical potential
+ e¥ ; we write the free energy density in the form
f =−˛
0
(T
c
−T +e¥@
T
c
)|¦ |
2
+... and going over to
the gauge invariant combination e¥ → e¥ −i@
t
/2
the variation ıf /ı¦
∗
produces the dynamical term
i
@
t
¦ with
=−(˛
0
/2)@
T
c
= ın/n [147].The
first-order time derivative @
t
¦ then involves both
a real and an imaginary part =
+i
govern-
ing the dissipative and the Hall part of the vortex
motion. The corresponding Hall conductivity as de-
rived via the TDGL approach then coincides with
the Drude typederivation discussed above combined
with the weak coupling BCS result (12.50) for the
density modulation ın in the vortex core.
A word of caution is appropriate regarding the
mutual interrelation between parameters: on a phe-
nomenological level we deal with the dependence of
T
c
on the chemical potential ; the quantity @
T
c
enters the expression for the vortex charge, the Hall
dynamics of the vortex,and is related to the particle-
hole symmetry breaking parameter @
E
N,atleast
within weak coupling BCS theory. The question then
arises, whether the knowledge of the doping depen-
dence of T
c
or of the bandstructure allows for the
determination of the vortex charge and of the vor-
tex Hall dynamics — the answer is “no”, in general,
as the critical temperature T
c
may depend separately
on the Fermi energy "
F
≈ and on the particle den-
sity n. In this situation the gauge invariant extension
T
c
("
F
−i@
t
/2, n) involves only the dependence via
the Fermi energy "
F
and the vortex charge and Hall
conductivity may involve different parameters [148].
Hence the full derivative dT
c
/d determining the
vortex charge is different from the partial derivative
@T
c
/@ determining the Hall coefficient
and the
partial derivative @T
c
/@n describing the doping de-
pendence of T
c
.
An attempt to explain the doping dependence of
the sign change in the high-T
c
cuprates through the
behavior of the parameters @
E
N (as measured in
photoemission and tunneling experiments) or @
n
T
c
(doping dependence) predicts a behavior different
from what is found in the experiments; the latter
show a change of sign in the underdoped region and
no such Hall anomaly in overdoped samples (these
are experiments on Y-,Bi-,La-, and Tl-based sam-
ples [144,145]).Deriving ın from @
E
N, the hole-like
Fermi-surface geometry predicts no sign change at
all.On the other hand,extracting ın from the doping
dependence of T
c
, a Hall anomaly is predicted in the

512 G. Blatter and V.B. Geshkenbein
overdoped regime, while none should occur in un-
derdoped samples— this is exactly theopposite from
what is found in the experiment [145].However, the
discussion on the interrelation of various parameters
given above warns us that in general we cannot hope
to extract ın directly from @
E
N or @
n
T
c
.A consistent
explanation of the Hall anomaly has been attempted
in [148] on the basis of a microscopic mechanism
involving preformed pairs, with negatively charged
Cooper pairs; this theory can explain the observed
change in sign of the Hall conductivity in the un-
derdoped regime [148]. On the other hand, the over-
doped regime is expected to behave more regularly,
in which case the hole-like Fermi surface @
E
N cor-
rectly predicts the absence of a Hall anomaly.
12.3.3 Green’s Functions
Completing the free energy (12.30) with a force term
−f (z) ·u(z), a variation with respect to the displace-
mentfield u(z)providesuswiththedifferentialequa-
tion "
l
@
2
z
u =−f and the static Green’s function G,
after Fourier transformation, G(q)=1/"
l
q
2
.Asdis-
cussed above, the vortex dynamical response in type
II superconductors is usually dissipative and the full
dynamical Green’s function takes the form
G(q, !)=
1
"
l
q
2
−i!
l
. (12.51)
12.3.4 Free Energy and Action
When dealing with classical or quantum statistical
aspects of individual vortices we need appropriate
expressions for the free energy and for the imag-
inary time Euclidean action describing the vortex
line. While the former has been derived above, see
(12.30),the latter follows via completion with an ap-
propriate dynamical term. The vortex dynamics in
type II superconductors is usually dominated by the
dissipative term producing a non-local in time con-
tributionto the (Euclidean) action [149],
S
E
=
ddz
"
l
2
@u(z, )
@z
2
(12.52)
+
l
4
d
u(z, )−u(z,
)
−
2
,
where the displacement field u now additionally
depends on the imaginary time argument .The
generalization to the anisotropic case is straightfor-
ward [150].
12.4 Vortex Lattice
Increasing the external field beyond H
c
1
, see (12.22)
and (12.27), the formation of vortices turns energet-
ically favorable and we enter the Shubnikov phase
[3,4,96,108,109]. The pairwise interaction between
parallel straight flux lines is repulsive, V(R > )=
2"
0
K
0
(R/), with the MacDonald function K
0
de-
scribing a 2D Coulomb interaction screened on a
scale . The Ginzburg–Landau free energy is min-
imized by a triangular lattice with a lattice constant
a
=(2/
√
3)
1/2
(¥
0
/B)
1/2
and (planar) lattice coordi-
nates ( =(m, n))
R
=
√
3
2
na
,
2m + n
2
a
,
K
=2
2n − m
√
3a
,
m
a
, (12.53)
in real and reciprocal space (the Abrikosov parame-
ter takes thevalueˇ
A
≡|¦ |
4
/|¦ |
2
2
=1.1595953).
The constitutive relation B(H) is determined by the
free energy density (the term f
s0
=−H
2
c
/4 accounts
for the condensation energy)
f (B) ≈
⎧
⎪
⎪
⎪
⎪
⎪
⎪
⎪
⎪
⎨
⎪
⎪
⎪
⎪
⎪
⎪
⎪
⎪
⎩
f
s0
+
B
¥
0
l
+6"
0
2a
0
1/2
e
−a/
, H
c
1
≤ H ,
f
s0
+
B
2
8
+
B
2¥
0
"
0
ln
˛H
c
2
B
, H
c
1
H H
c
2
,
B
2
8
−
1
8
(H
c
2
− B)
2
1+(2
2
−1)ˇ
A
, H ≤ H
c
2
,
(12.54)
via the derivative H =4@
B
f and the relation
B = H +4M,
B(H → H
c
1
)=
2¥
0
√
3
2
1
{ln[3¥
0
/4
2
(H − H
c
1
)]}
2
,
(12.55)
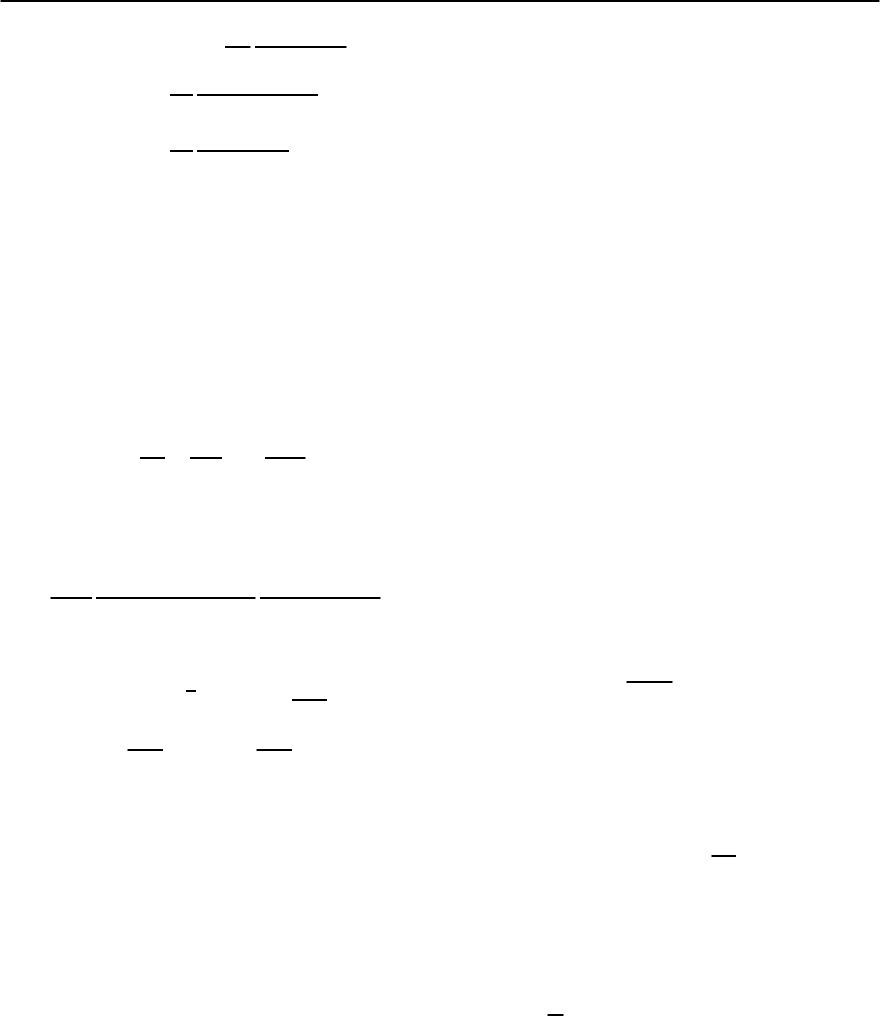
12 Vortex Matter 513
M (H
c
1
H H
c
2
)=−
H
c
1
4
ln(˛H
c
2
/H)
2ln
, (12.56)
M (H → H
c
2
)=−
1
4
H
c
2
− B
1+(2
2
−1)ˇ
A
=
= −
1
4
H
c
2
− H
(2
2
−1)ˇ
A
. (12.57)
The numerical ˛ is of order unity, ˛ ≈ 0.35 as cal-
culated theoretically in [151] and ˛ ≈ 1.2-1.5 when
comparing to data [152, 153]. Thermal fluctuations
and quenched disorder modify these results (see
Sect. 12.7.8); in anisotropic superconductors the H
c
1
transition may be driven first-order at low tempera-
tures.
In anisotropic superconductors the free energy
density picks up an additional dependence on the
angle # ,
f (# , B) ≈
B
2
8
+
B"
#
2¥
0
"
0
ln
˛H
c
2
"
#
B
. (12.58)
Asa result,the angles# and #
H
of B andH differfrom
one another (see (12.28)) and the sample is exposed
to a torque T = M ∧ H,
T =
HH
c
c
1
4
(1 − "
2
)sin# cos #
"
#
ln(˛H
c
c
2
(# )/B)
2ln(/)
;
(12.59)
measuring the maximum torque at
#
max
=
⎧
⎪
⎪
⎨
⎪
⎪
⎩
√
" , 1 " ln
˛H
c
c
2
"B
, " 1 ,
"
ln
˛H
c
c
2
"B
1/2
, " ln
˛H
c
c
2
"B
1 ,
(12.60)
allows for the determination of the anisotropy pa-
rameter " [154].
Fermi surface effects in the underlying crystal
may distort the hexagonal vortex lattice, e.g., the
strong Fermi surface anisotropy in the Borocarbides
produces a square vortex lattice [155, 156] and a
hexagonal-to-square vortex lattice transition with
decreasing magnetic field [157]; these effects can be
understood when non-local correctionsare included
in the London model [158]. Similarly, the nodes in
the d-wave order parameter of the copper-oxides fa-
vor a square vortex lattice [159, 160]: at low mag-
netic fields the repulsive interaction between vor-
tices dominates and the vortex lattice exhibits the
usual hexagonal symmetry, as observed in numerous
Bitter decoration experiments [161] and in small an-
gle neutron scattering experiments [162] (note that
both Bitterdecoration [163] and small angle neutron
scattering [164] experiments measure the magnetic
field distribution and thus are limited to low mag-
netic fields B < ¥
0
/
2
). On the other hand, scan-
ning tunneling experiments on YBCO carried out at
large fields (6 Tesla; this technique allows to mea-
sure the vortex cores and hence is not limited to
low fields) reveal an oblique lattice [165] which is
compatible with a square lattice [166] distorted due
to the (small) ab-anisotropy within the supercon-
ducting planes [167,168]. Note, that twin boundary
pinning is a competing mechanism also favoring a
square lattice [169,170].
12.4.1 Elasticity
In general, the vortex lattice is not in its equilibrium
configuration but in some distorted state character-
ized by the two-component displacement field u
(z)
or its Fourier transform u(k)[r
≡ (R
, z)],
u(k)=a
2
0
dz
e
−ikr
u
(z),
u
(z)=
BZ
d
3
k
(2)
3
e
ikr
u(k), (12.61)
where the integrationruns overthe two-dimensional
Brillouin zone (BZ) of the vortex lattice and the in-
tegration along k
z
is cut off at the inverse core ra-
dius, |k
z
| < / (a convenient approximation is to
replace the hexagonal Brillouin zone by a circular
geometry with radius K
BZ
=
√
4/a
0
). The London
free energy of a distorted configuration with vortex
positions s
= r
+ u
takes the form [171,173] (cf.
(12.34); here, i, j ∈{x, y, z}; see [10,172] for an anal-
ysis describing the situation close to H
c
2
)
F
L
[{s
}]=
"
0
2
,
ds
i,
ds
j,
V
ij
(s
− s
) ; (12.62)
in the isotropic situation the interaction potential
V
ij
(r)=ı
ij
V(r) between vortex segments is given by
(12.34).Note that the sum over , in (12.62)should
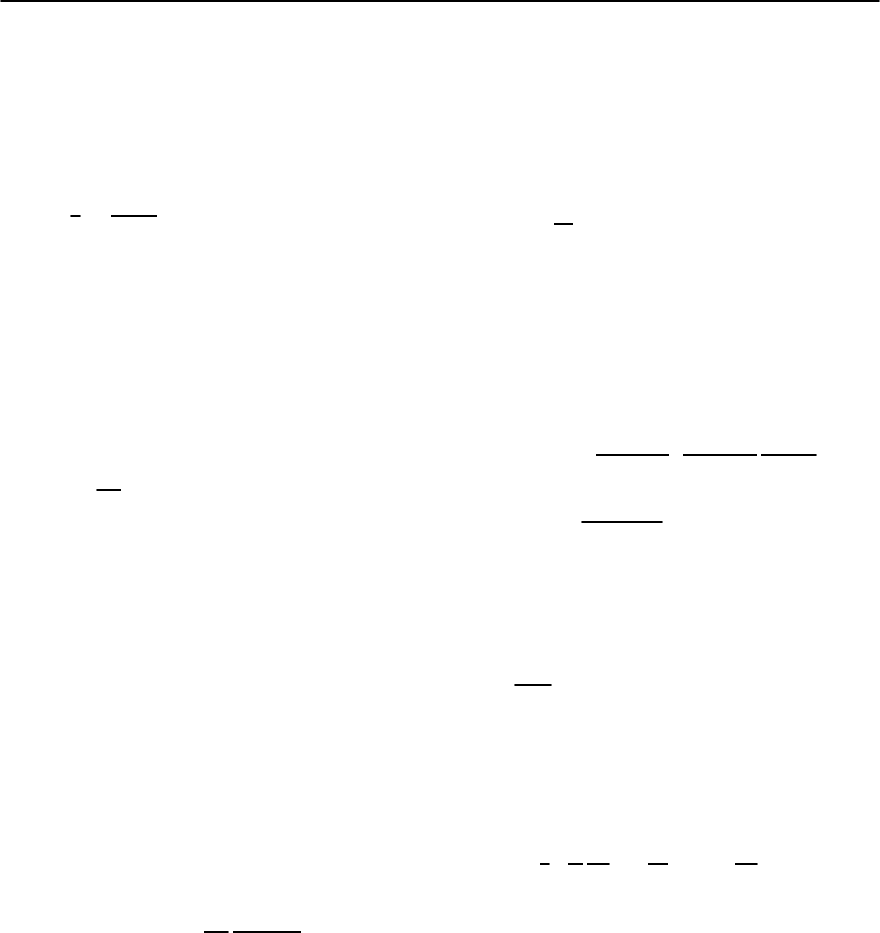
514 G. Blatter and V.B. Geshkenbein
be understood as a sum over segments; for an in-
dividual vortex line the integral goes over all pairs
of line segments ds
and ds
, cf. (12.34).Expanding
(12.62) to second order in the displacement field u
provides us with the elastic energy (indices appear-
ing twice are summed over)
F[u]=
1
2
BZ
d
3
k
(2)
3
u
˛
(k)¥
˛ˇ
(k)u
ˇ
(−k)
. (12.63)
The elastic matrix ¥
˛ˇ
(k) of the vortex lattice is char-
acterized by the symmetries
¥
˛ˇ
(k)=¥
ˇ˛
(k)=¥
∗
˛ˇ
(k)
= ¥
∗
˛ˇ
(−k)=¥
∗
˛ˇ
(k + K
) (12.64)
and relates to the interaction potential V(k)via
¥
˛ˇ
(k)=
B
2
4
f
˛ˇ
(k + K
)−f
˛ˇ
(K
)
, (12.65)
f
˛ˇ
(k)=(k
˛
k
ˇ
+ ı
˛ˇ
k
2
z
)V(k) . (12.66)
Within the nonlocal continuum limit the elastic ma-
trix ¥
˛ˇ
(k) takes the form
¥
˛ˇ
(k)=
[
c
11
(k)−c
66
]
K
˛
K
ˇ
+ ı
˛ˇ
c
66
K
2
+ c
44
(k) k
2
z
, (12.67)
with c
11
(k)andc
44
(k)denotingthedispersive com-
pression and tilt moduli and c
66
is the non-dispersive
shear modulus.
Within the continuum isotropic approximation we
restrict the sum over reciprocal lattice vectors in
(12.65) to the = 0 term and obtain for the com-
pression and tilt moduli
c
11
(k) ≈ c
44
(k) ≈
B
2
4
1
1+
2
k
2
, (12.68)
while the shear modulus c
66
≈ 0 vanishes (within
this rough approximation we cannot decide whether
to write c
11
or c
11
− c
66
in (12.68); the accurate analy-
sis[13,173]shows thatc
11
−c
66
≈ (B
2
/4)/(1+
2
k
2
)).
The same result can be derived in a hydrodynamic
description and hence the expressions (12.68) de-
scribe well the vortex-liquidphase [174].At the same
time the relations (12.68)provide convenient expres-
sions for the compression and tilt moduli in the vor-
tex solid phase, while the calculation of the lattice
shear modulus requires summation of all the terms
= 0 in (12.65),a somewhat non-trivial task: we ex-
press the elastic matrix ¥
˛ˇ
through the real-space
sum
¥
˛ˇ
(K)=
1
a
2
0
1−exp(iK · R
)
@
˛
@
ˇ
V(R
) ,
(12.69)
with the interaction potential V(R)=2"
0
K
0
(R/)
acting between a pair of (straight) vortex lines.
Thelongwavelengthlimitofthetransversepro-
jection defines the shear modulus, c
66
=(ı
˛ˇ
−
K
˛
K
ˇ
/K
2
)¥
˛ˇ
(K)/K
2
|
K→0
. Expanding the exponen-
tial in (12.69) to order K
2
one arrives at
c
66
=
=0
(K · R
)
2
2a
2
0
K
2
(K · R
)
2
K
2
R
2
V
(R
)
R
+
(K ∧ R
)
2
K
2
R
2
V
(R
)
. (12.70)
Using the symmetry of the triangular lattice, we per-
form a partial resummation over stars {R
}
6
1
and
thereby eliminate the dependence on the direction
K/K,
c
66
=
1
16a
2
0
=0
3R
V
(R
)+R
2
V
(R
)
. (12.71)
Inthe dilute limitB < H
c
1
/ ln the vortex separation
increases beyond the screening length; the sum in
(12.71)is dominatedbythesixnearestneighbors and
the shear modulus is exponentially small [18,175],
c
66
=
3
2
6
a
1/2
"
0
2
exp
−
a
. (12.72)
The dense limit with a
0
is puzzling: at short
distances we can approximate V(R) ≈ −2"
0
ln(R/);
with 3RV
(R)=−6"
0
and R
2
V
(R)=2"
0
we find
that each “shell” in (12.71) contributes the negative
value −4"
0
. On the other hand,large distances R >
contribute with a positive sign and eventually over-
compensate the negative terms from small distances
R < . Technically, the final result is obtained by re-
placing the sum by an integral while subtracting the
contribution from the origin; the integral vanishes
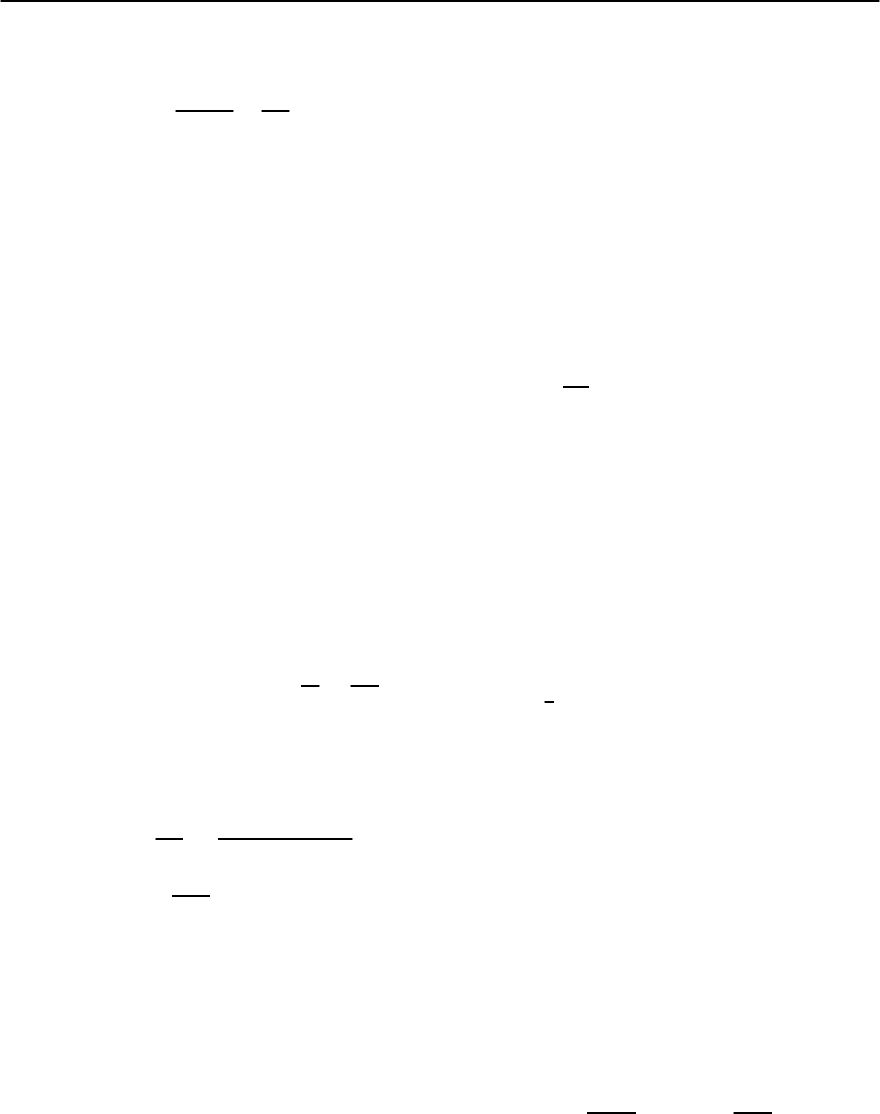
12 Vortex Matter 515
(after partial integration) and the remaining term
provides the desired answer
c
66
≈
¥
0
B
(8)
2
=
"
0
4a
2
0
. (12.73)
The shear modulus of the dense vortex lattice
appears as a subtle cancelation of contributions
from small and large distances. Similar results
are obtained when calculating the “cage potential”
V
cage
(u)=
=0
V(R
− u) acting on the vortex at
the origin and produced by all other vortices in its
environment: choosing an unscreened interaction
V(R)=−2"
0
ln(R/L)(withL denoting the system
size) the cage potential vanishes, while the screened
potential V
s
(R)=2"
0
K
0
(R/) results in a finite cage
potential V
cage
(u) ≈ "
0
(u/a
0
)
2
.
A similar addition of terms = 0 in (12.65) pro-
duces(non-isotropic) correctionsto thetiltand com-
pression moduli important at the BZ boundary and
reflecting the hexagonal symmetry of the vortex lat-
tice (this“geometric”dispersion depends only on the
product a
0
k and thus is weaker than the “essential”
dispersion originating from the =0termand
involving the parameter k). Furthermore, close to
the Brillouin zone boundary the tilt modulus c
44
(k)
crosses over to the single vortex result [173]
c
44
(K > K
BZ
/[ln(a
0
/)]
1/2
, k
z
) ≈
"
0
a
2
0
ln
1
k
z
.
(12.74)
A convenient interpolation formula is given by
c
44
(k)=c
0
44
(k)+c
c
44
(k)withc
0
44
(k) given by (12.68)
and
c
c
44
(k
z
) ≈
"
0
2a
2
0
ln
2
1+
2
(K
2
BZ
+ k
2
z
)
+
1
k
2
z
2
ln(1 +
2
k
2
z
)
. (12.75)
The k
z
→ 0 limit of this expression takes the form
c
c
44
(0) = −BM = B(H − B)/4 (cf. (12.56)) and we
obtain the correct expression c
44
(0) = BH/4 forthe
uniformtilt modulus as determined fromthermody-
namic considerations [7].
At small field values B < H
c
1
/ ln the compres-
sion modulus becomes exponentially small [18,175],
c
11
=3c
66
, (12.76)
whereas the tilt modulus goes over into the single
vortex expression "
l
/a
2
0
with "
l
given by (12.36). In
theoppositesituationoflargeinductionstherenor-
malization of the length scales due to the suppres-
sion of the order parameter should be taken into
account. The results for the compression and tilt
moduli (12.68) are obtained through the substitu-
tion →
= /(1 − b)
1/2
with the reduced field
b = B/H
c
2
(T) [171]; the suppression of the shear
modulus (12.73) near to the upper critical field H
c
2
is more pronounced and involves the factor (1 − b)
2
,
c
66
≈ "
0
(1 − b)
2
/4a
2
0
.
The dispersive behavior of the compression and
tilt moduli c
11
(k)andc
44
(k) leads to a considerable
softening of the vortex lattice. Near the BZ bound-
ary K
BZ
≈
√
4/a
0
a suppression factor (K
BZ
)
2
≈
B ln /H
c
1
is obtained with respect to the value at
k = 0 describing a uniform distortion. On the other
hand, the shear modulus is essentially free of dis-
persion and always small, in fact, c
66
≈ c
11
(K
BZ
)/4.
The physical origin of the dispersion in c
11
and in
c
44
is found in the long-range interaction potential
(12.34): For fields B > H
c
1
/ ln the nearest neigh-
bor distance a
0
drops below the screening length
and the vortex-vortex interactionextends beyondthe
nearest neighbors. The local limit described by the
functional
F[u]=
1
2
d
3
r [c
11
(0) (∇·u)
2
+ c
66
(∇
⊥
· u)
2
+ c
44
(0) (@
z
u)
2
] , (12.77)
with ∇
⊥
=(@
y
, −@
x
)andc
11
(0) ≈ c
44
(0) ≈ B
2
/4,
should therefore beused with caution.Since c
11
(0)
c
66
the functional (12.77) essentially describes an in-
compressible solid.
The generalization of the theory of elasticity to
anisotropic superconductors is somewhat cumber-
some; we summarize the main results below and add
a few remarks on a simplified derivation via the scal-
ing approach [176,177] in the end. As the (internal)
magnetic field is tiltedaway from the c-axis the (equi-
lateral) triangular lattice defining the equilibrium
configuration is deformed and the new basis vec-
tors (in the vortex frame of reference, see Fig. 12.4;
we remind that =(m, n)) become [178]
R
= a
n
3/4"
#
, (2m + n)
"
#
/4
, (12.78)
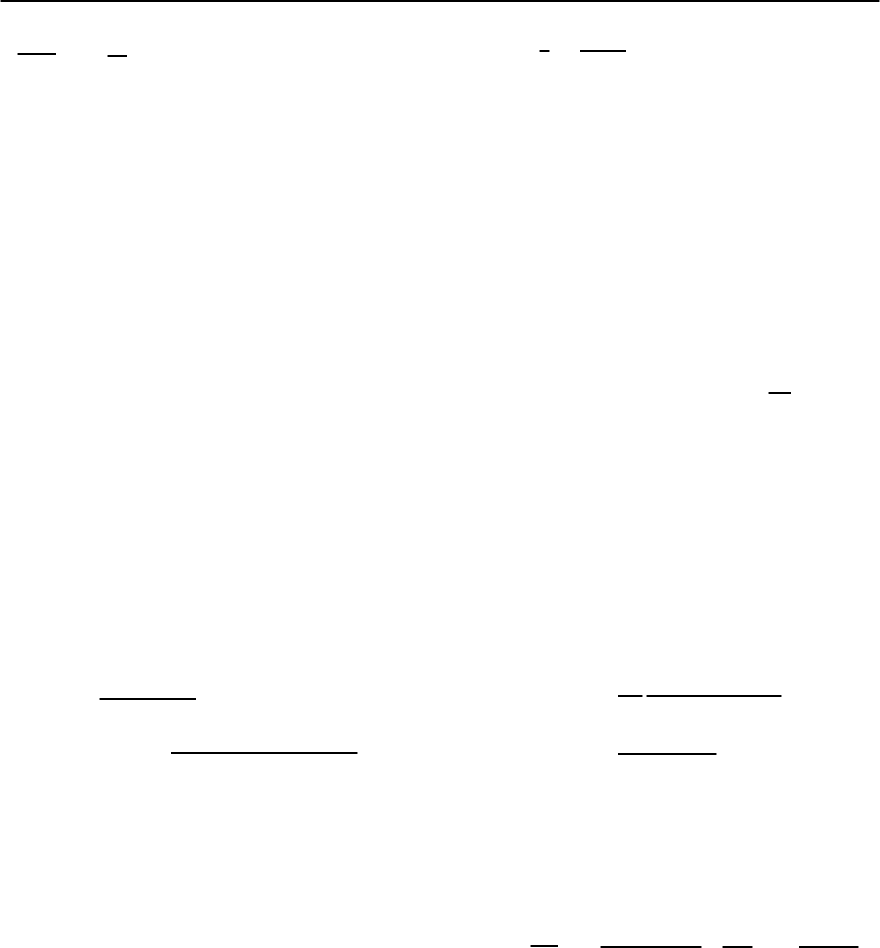
516 G. Blatter and V.B. Geshkenbein
in reciprocal space, K
a
/2 = [(2n − m)
√
"
#
/3, m/
√
"
#
, with the angle-dependent
anisotropy parameter (12.26). Note that the equilib-
rium lattice configuration is characterized by a short
lattice vector R
(1,0)
pointing along the y
-axis and the
planar rotational degeneracy present for H c is
removed as the field is tilted away from the c-axis.
The configuration (12.78) describes the equilibrium
state of the vortex lattice at large magnetic fields
H > H
c
c
1
/"
#
; for small fields H < H
c
c
1
/"
#
the equi-
librium state for the tilted lattice is realized by the so
called chain state [179–181] where the vortex lines
rearrange themselves into parallel running chains
defining planes of high flux density oriented parallel
to the yz-plane containing the c-axis and the mag-
netic field vector. This new state is a consequence of
the attractive vortex-vortex interaction experienced
by the tilted vortices in anisotropic superconduc-
tors [179,182,183] and has been observed via Bitter
decoration experiments on YBCO surfaces [184].
The elastic properties of the vortex lattice derive
from the elastic matrix ¥
˛ˇ
(k), see (12.63). Within
London theory we have to replace the expression
for the potential (12.34) and (12.66) by (i, j ∈{x =
x
, y
, z
}; ˛, ˇ ∈{x = x
, y
})
V
ij
(k)=
e
−(
2
K
2
+
2
c
k
2
z
)
1+
2
k
2
(12.79)
×
ı
ij
−
(
2
c
−
2
) K
⊥i
K
⊥j
1+
2
k
2
+(
2
c
−
2
)K
2
,
f
˛ˇ
(k)=k
˛
k
ˇ
V
z
z
(k)
+ k
2
z
V
˛ˇ
(k)−2k
z
k
ˇ
V
z
˛
(k) , (12.80)
with K =(k
x
, k
y
), K
⊥
= k ∧ˆc and ˆc the unit vector
along the c-axis (as seen from the vortex frame of
reference; we remind the definitions
c
= ",the
coherence length along the c-axis, and
c
= /",
the screening length for currents flowing parallel to
the c-axis).Inananisotropicmaterialthenumberof
elastic moduli increases dramatically: the linear elas-
tic energy within the nonlocal continuum approxi-
mation takes the form
F =
1
2
d
3
k
(2)
3
c
11
(k)(k
x
u
x
)
2
+ c
⊥
11
(k)(k
y
u
y
)
2
+2c
,⊥
11
(k)(k
x
u
x
k
y
u
y
)
+ c
44
(k)(k
z
u
x
)
2
+ c
⊥
44
(k)(k
z
u
y
)
2
+2c
⊥
14
(k)(k
y
k
z
u
2
y
) (12.81)
+2c
,⊥
14
(k)(k
x
u
x
k
z
u
y
)+c
66
(k
y
u
x
)
2
+ c
⊥
66
(k
x
u
y
)
2
+2c
64
(k
y
k
z
u
2
x
)
.
In addition, the vortex lattice in the anisotropic ma-
terial exhibits rotational modes [185]. The shear de-
formationsinvolve the softin-plane and hard out-of-
plane moduli [185]
c
66
(# )=c
66
"
3
#
, c
⊥
66
(# )=
c
66
"
#
, (12.82)
with c
66
= ¥
0
B/(8)
2
the isotropic result evalu-
ated with the planar screening parameter .Thetilt
moduli c
44
and c
⊥
44
forthe(soft)in-planeandforthe
(hard) out-of-plane tilt modes can be written in a
form c
44
(k)=c
0
44
(k)+c
c
44
(k), with c
0
44
(k)thestrongly
dispersive contribution arising from the term =0
in (12.65) and c
c
44
(k) denoting the correction due to
the remaining terms in the sum [12,71,186,187] (note
that = /2−# , "
2
= "
2
sin
2
# +cos
2
# ),
c
0,
44
(k)=
B
2
4
1
1+
2
c
K
2
+
2
k
2
z
,
c
0,⊥
44
(k)=
1+("
c
k)
2
1+
2
k
2
c
0,
44
(k) . (12.83)
The correction term c
c
44
(k) becomes important at
large k-vectors and describes the crossover from the
lattice modulus to the (dispersive) single vortex line
tension as the interaction between neighboring vor-
tices becomes small, for B ˆz,
c
c
44
(k
z
)≈
"
0
2a
2
0
"
2
ln
2
c
1+
2
c
K
2
BZ
+
2
k
2
z
+
1
2
k
2
z
ln
1+
2
k
2
z
1+
2
K
2
BZ
,
(12.84)
where
c
= /". In layered material, the first term in
(12.84) is due to the Josephson coupling between the
superconducting planes; it is only weakly dispersive,
depends strongly on the anisotropy ",andvanishes in
the limit " → 0. The second term is due to the elec-
tromagnetic interaction and is strongly dispersive
12 Vortex Matter 517
but independent of the anisotropy parameter " and
hence always finite.A particularly interesting limitis
thesinglevortexlinetensionwhichbecomesstrongly
dispersive as a consequence of the anisotropy,
"
l
(k
z
) ≈ "
2
"
0
ln
2
c
1+
2
k
2
z
1/2
+ "
0
1
2
k
2
z
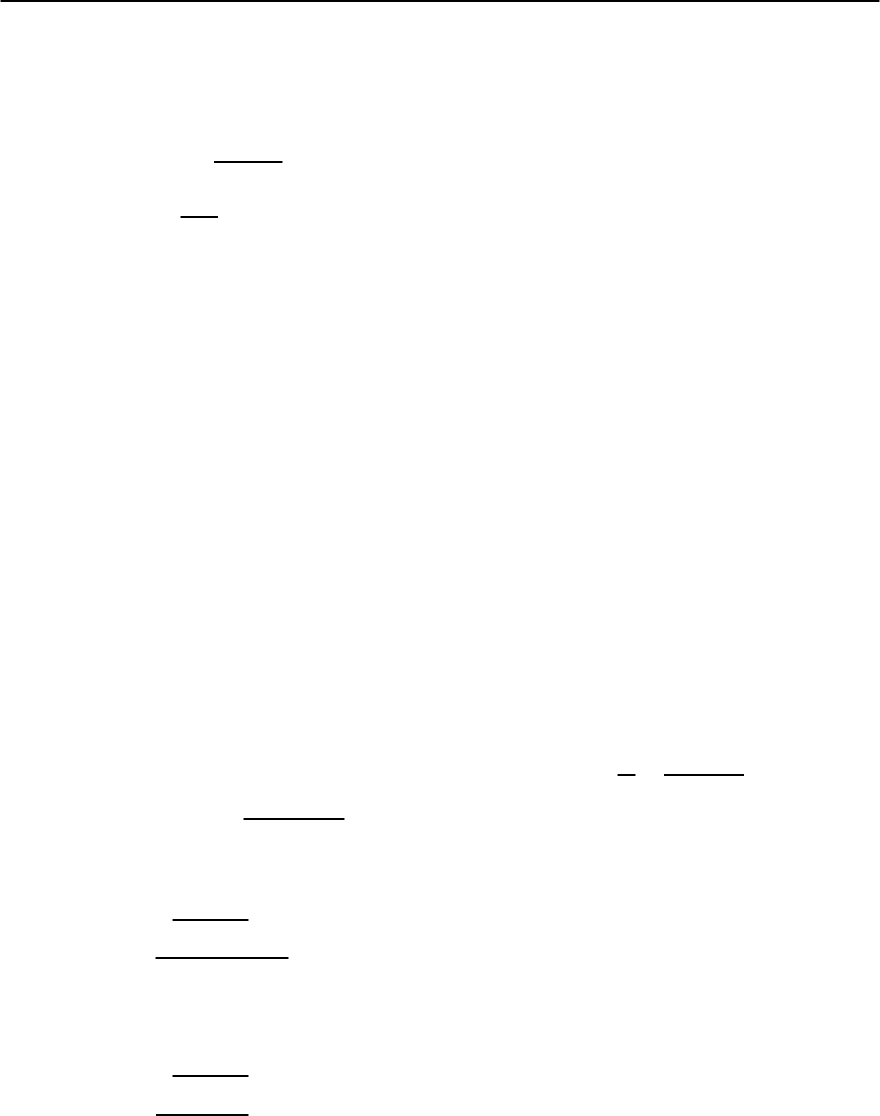
ln(1 + k
2
z
2
)
1/2
. (12.85)
For large wave-vectors k
z
> 1/" the line ten-
sion is small, "
l
∼ "
2
"
0
; in the intermediate regime
1/ < k
z
< 1/" the line tension rapidly increases,
"
l
∼ "
0
/k
2
z
2
, and reaches the long-wavelength limit
"
l
∼ "
0
for k
z
< 1/. This separation into a small
line tension due to the Josephson coupling and a
strongly dispersive component due to the electro-
magnetic couplingis specific to the anisotropic situ-
ation. In the isotropic situation there is no suppres-
sion of the first term and the dispersion is always
weak. Note that the expression (12.85) is valid in the
elastic regime; for large displacements uk
z
> 1the
logarithm in the second term of (12.85) should be
cut on 2/u rather than k
z
[65].
It is interesting to note that the ratio ofthe bulk tilt
moduli c
0,⊥
44
/c
0,
44
≈ "
2
/"
2
shows a different angular
dependence as compared to the ratio "
⊥
l
/"
l
≈ 1/"
2
#
of the single vortex tilt moduli, see (12.38); this dif-
ference is due to the admixing of the bulk modulus
to the tilt mode c
0,⊥
44
.
The compression moduli c
11
, c
⊥
11
,andc
,⊥
11
take the
form [12,186,187]
c
11
(k)=c
⊥
11
(k)=c
,⊥
11
(k)=
1+("
#
c
k)
2
1+
2
k
2
c
0,
44
(k)
(12.86)
andthemixedcompression-tiltmodulic
⊥
14
and c
,⊥
14
read [187]
c
14
(k)=c
⊥
14
(k)=
"
2
#
"
2
− "
2
2
c
k
2
1+
2
k
2
c
0,
44
(k) . (12.87)
The mixed shear-tilt modulus c
64
arises from terms
= 0 in the sum (12.65)and has been obtained using
anisotropic scaling theory [177],
c
64
(k)=
"
2
#
"
2
− "
2
"
2
#
c
66
. (12.88)
In addition, corrections to the above moduli due to
the admixing of the shear mode to the tilt and com-
pression modes can only be obtained by considering
terms = 0 in the sum over reciprocal lattice vec-
tors in (12.65).The complete analysis of these terms
has not yet been done. Using the anisotropic scal-
ing approach (cf. Sect. 12.6 and [176]) the above re-
sults are easily rederived provided that the condition
˜
k > 1, with
˜
k the rescaled k-vector modulus in the
isotropized system, is satisfied [177].
The elastic theory for anisotropic superconduc-
tors for angles # away from the main axes is quite
cumbersome since the elastic energy is not any more
diagonal in the vortex frame of reference; a more
convenient approach is to use the anisotropic scaling
theory (see [176] and the summary in Sect.12.6) and
study the problem within the isotropized system in-
stead of carrying out the analysis in the anisotropic
vortex frame of reference where the elastic energy is
not diagonal.
12.4.2 Dynamics
The dynamical response of the vortex lattice follows
trivially from that of the individual vortex lines, see
Sect. 12.3.2: In type II superconductors the vortex
dynamics is usually dissipative and the viscous drag
coefficient for the lattice follows from the Bardeen
Stephen expression (12.40) by going over to the ap-
propriate density,
≡
l
a
2
0
=
¥
0
B
2c
2
2
n
. (12.89)
12.4.3 Green’s Functions
The Green’s function for the vortex lattice is derived
from the free energy (12.63) completed with a force
term −f (k) ·u(−k). The variation with respect to the
displacement field u(−k) provides us with the differ-
ential equation
[¥
˛ˇ
(k)−i!ı
˛ˇ
] u
˛
(k)=f
ˇ
(k) , (12.90)
where we have already completed the expression
with the dissipative dynamical term −i!u(k). The
Green’s function for the vortex lattice takes the form
G
˛ˇ
(k, !)=[¥ (k)−i!1]
−1
˛ˇ
. (12.91)

518 G. Blatter and V.B. Geshkenbein
Expressing the elastic matrix ¥ through the elastic
moduli with the help of (12.67) the Green’s function
takes the form
G
˛ˇ
(k, !)=
P
˛ˇ
(K)
c
11
(k)K
2
+ c
44
(k)k
2
z
−i!
+
P
⊥
˛ˇ
(K)
c
66
K
2
+ c
44
(k)k
2
z
−i!
(12.92)
with the projection operators
P
˛ˇ
(K)=
K
˛
K
ˇ
K
2
, P
⊥
˛ˇ
(K)=ı
˛ˇ
−
K
˛
K
ˇ
K
2
.
(12.93)
12.4.4 Free Energy and Action
The statistical physics of Vortex Matter including
thermal and quantum fluctuations is determined by
the appropriate partition function requiring know-
ledge of the free energy and the imaginary time Eu-
clidean action of the vortex lattice. The fully non-
local expression for the free energy takes the form
F =
1
2
d
3
k
(2)
3
c
11
(k)|K · u(k)|
2
(12.94)
+ c
66
|K
⊥
· u(k)|
2
+ c
44
(k)|k
z
u(k)|
2
,
with K
⊥
=(k
y
, −k
x
), while completion with a non-
local in time dynamical term describing the viscous
motion of the vortex lattice provides us with the Eu-
clidean action (in Fourier space; f denotes the free
energy density in (12.95))
S
E
=
1
2
d
3
k
(2)
3
d!
2
(12.95)
×
f
u(k, !)
+ |!||u(k, !)|
2
.
12.5 Layered Materials
The most extreme limit of an anisotropic supercon-
ductor is a layered material made from a stack of
uncoupled individual superconducting planes; the
phenomenology of such a material exhibits interest-
ing properties due to the electromagnetic coupling
between the layers. A finite Josephson coupling be-
tween the layers establishes a bulk superconducting
response and increasing the Josephson coupling one
may scan all values of the anisotropy parameter ",
thus covering the physics of layered and continuous
anisotropic up to isotropic materials. Many super-
conductors fall into the class of layered materials:
the oxide superconductors are layered compounds
with building blocks made from conducting (metal-
lic) Cu-O planes separated by buffer layers which
serve as charge reservoirs. The transport proper-
ties are roughly uniaxial, with a large anisotropy
between the c-axis and the ab-planes due to the
layered structure and essentially isotropic behavior
within the Cu-O planes. The layered superconduc-
tors of the BEDT-TTF family [113] are other candi-
dates requiring a discrete description and the most
extreme members of this class of materials are the ar-
tificially grown multi-layer structures, among them
the (Y/Pr)Ba
2
Cu
3
O
7−y
[188] and Mo
x
Ge
1−x
/Ge [189]
systems.While for not too large anisotropy a descrip-
tion in terms of a continuous anisotropic Ginzburg–
Landau or London theory is applicable,for very large
anisotropy the discreteness of the structure becomes
relevant and a description in terms of a set of weakly
coupled superconducting layers, the basic starting
point of the discrete Lawrence–Doniach model [58],
is more appropriate.
The criterion defining the crossover from a con-
tinuous anisotropic to a discrete layered description
depends on the specific physical question at hand.
Examples for such criteria are known from the con-
text of criticalfluctuations or thebehavior ofthe par-
allel upper criticalfield:in bothcasesthe 2Dbehavior
is established when the coherence length
c
(T)along
the c-axis drops below the layer separation d with de-
creasing temperature. The precise criterion involves
the dimensionless ratio
cr
=2
2
c
(0)/d
2
: for a large
coherence length
c
(0), i.e.,
cr
1, the continuous
anisotropic description is always appropriate.On the
other hand, for small
cr
1 a crossover will take
placeatatemperature T
cr
=(1−
cr
) T
c
< T
c
;for tem-
peratures T < T
cr
the fluctuations in the magnetic
and electric response behave 2D-like [190] and the
parallel upper critical field is only limited through
pair breaking via Pauli paramagnetism or spin-orbit
coupling [191].Other physical phenomena,however,
involve different criteria: e.g., vortex lattice melting
12 Vortex Matter 519
involves the length scale "
√
¥
0
/B, while pinning is
characterized by the pinning length "
4/3
L
c
as deter-
mined by the roughness of the disorder landscape (cf.
Sect. 12.9.2); the 2D–3D crossover takes place when
these lengths drop below the interlayer distance d.
Among the important class of copper oxide based
materials a continuous anisotropic description is
usually applicable to YBa
2
Cu
3
O
7−ı
[192],whereas the
more strongly layered Bi
2
Sr
2
CaCu
2
O
8+x
compound
belongstotheclassofmaterialswith
cr
1and
hence2D-like behavior isabundant;examples are the
thermodynamic properties of the superfluid or of the
vortex lattice which more closely resemble those of
a 2D superconducting film rather than of a 3D bulk
material. Still,the continuous anisotropic Ginzburg–
Landau or London based analysis often can provide a
rather good description of the physics of layered ma-
terials. For example, this is the case in the discussion
of the elastic properties of the vortex lattice within a
wide angular regime, the reason being that the non-
linear termin theLawrence–Doniachmodeldepend-
ing on the gauge invariant phase difference between
the layers can be linearized in many situations [193].
12.5.1 Lawrence–Doniach Model
The basis for the phenomenological description of
layered superconductors is given by the Lawrence-
Doniach model [58]. The free energy functional de-
scribes a discrete set of superconducting layers with
order parameters ¦
n
, separated by a distance d and
coupled via a Josephson term,
F[¦
n
, A]=d
d
2
R
n
˛|¦
n
|
2
+
ˇ
2
|¦
n
|
4
+
2
2m
∇
(2)
+
2i
¥
0
A
(2)
¦
n
2
+
2
2Md
2
(12.96)
¦
n+1
e
2i
¥
0
(n+1)d
nd
dzA
z
− ¦
n
2
+
d
3
r
B
2
8
.
The above formulation allows to make direct contact
with the continuous-anisotropic Ginzburg-Landau
functional (12.1) upon approximating the discrete
(non-linear) coupling term as a derivative along z;
the effective masses along the c-axis and in the ab-
planes are denoted by M and m, respectively, and
"
2
= m/M is the usual anisotropy parameter.The full
functional(12.97) canbetreatedina Londonapprox-
imation assuming a constantmodulus |¦
n
|within the
planes and allowing only for phase ('
n
) degrees of
freedom,
F['
n
, A]=
d
2
R
"
0
d
2
n
∇
(2)
'
n
+
2
¥
0
A
(2)
2
+
2m
Md
2
1−cos¥
n+1,n
+
d
3
r
B
2
8
,
(12.97)
with
2
|¦
n
|
2
/2m = "
0
/2 and ¥
n+1,n
the gauge in-
variant phase difference between the layers n and
n +1,
¥
n+1,n
= '
n+1
− '
n
+
2
¥
0
(n+1)d
nd
dzA
z
; (12.98)
here, we express the (superconducting) properties of
the layers by the period d of the layer structure and
the planar bulk penetration depth .Analternative
description is based on the thickness d
s
of the su-
perconducting layers combined with the penetration
depth
s
of the planes — the two descriptions are
related through the equality d/
2
= d
s
/
2
s
,guaran-
teeing equal superfluid sheet densities.
The variation of (12.97) with respect to the vector
potential A and the phases '
n
define the differential
equations describing spatial variations of A and '
n
,
2
A
(2)
= d
n
ı(z − nd )
A
(2)
+
¥
0
2
∇
(2)
'
n
,
(12.99)
A
z
=
4
c
j
J
sin ¥
n+1,n
, (12.100)
2
(2)
'
n
+
2
¥
0
∇
(2)
A
(2)
= sin ¥
n,n−1
−sin¥
n+1,n
, (12.101)
with ¥
n+1,n
the gauge invariant phase difference be-
tween the layers n and n +1andj
J
the Josephson
current density coupling the layers,
j
J
=
c¥
0
"
8
2
2
≈ j
0
"
. (12.102)
The parameter = d/" denotes the in-plane screen-
ing length where non-linearities in the coupling be-
tween the layers are important (we have used a gauge
