Barber J.R. Intermediate Mechanics of Materials
Подождите немного. Документ загружается.


8
Membrane Stresses in Axisymmetric Shells
A shell is any structure or part of a structure in which one local dimension is much
smaller than the other two. Shells abound in all branches of engineering and in the
natural world. Examples include gas tanks, thin-walled pipes and air ducts, grain
hoppers, car bodies, aircraft wings and fuselages, window panes, eggshells, wine-
glasses, concrete roofs and the human skull.
A shell which is plane in the undeformed state is known as a plate. Plates are
generally much less efficient than curved shells as structural elements, being more
flexible and less capable of transmitting transverse loads (i.e. loads normal to the
plane) without failure. You can verify this by pushing against a plate glass window or
the plane side of a metal filing cabinet. You will find that it is quite easy to produce an
observable deflection. The same material formed into a curved shell (e.g. a car body
panel or a drinking glass) will sustain an equal transverse load without observable
deformation.
The basic idea behind the analysis of shells is that the stresses can’t vary much
across the thin dimension and hence we can approximate the stress distribution
across the thickness by a Taylor series. When the shell is very thin, only the first
term of this series will be important — this is the ‘membrane theory’, in which the
stresses are assumed to be uniform through the thickness. In many problems — par-
ticularly those involving shells with discontinuities or built-in boundary conditions
— it is necessary to include both the first and second terms of the series. This con-
stitutes the ‘bending theory of shells’.
It might be helpful to draw an analogy with beams, which of course are structures
in which two dimensions are much smaller than the third. The ‘membrane theory of
beams’ assumes that the axial stress
σ
zz
is uniform across the section and hence
corresponds only to the transmission of an axial force passing through the centroid.
The addition of one extra term in the Taylor series permits the stress to vary linearly
across the section and defines the bending theory of beams.
In this chapter, we shall restrict attention to the membrane theory of shells, which
therefore implies that bending moments are everwhere negligible. It follows that
derivative of the bending moment must also be everywhere negligible and hence
J.R. Barber, Intermediate Mechanics of Materials, Solid Mechanics and Its Applications 175,
2nd ed., DOI 10.1007/978-94-007-0295-0_8, © Springer Science+Business Media B.V. 2011
386 8 Membrane Stresses in Axisymmetric Shells
there can be no shear force or transverse shear stress, by extension of equation (6.1).
In other words, the only non-zero stresses are in the local (tangential) plane of the
shell and are uniform through the thickness.
Despite these restrictions, we shall find that the curvature of a membrane shell
permits it to transmit a wider range of loads than either a straight beam or a plate un-
der similar restrictions, making the shell a useful and versatile lightweight structural
member. We shall illustrate this here for the particular case of axisymmetric shells
under axisymmetric loading.
8.1 The meridional stress
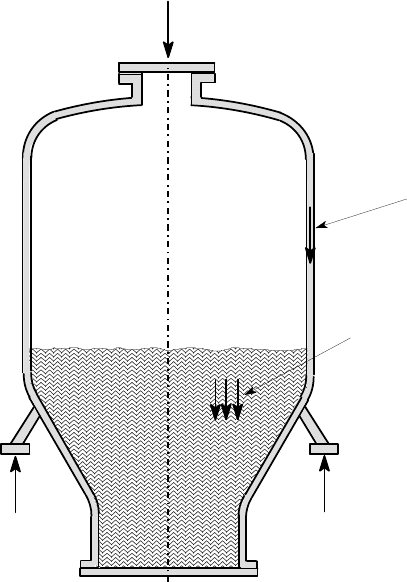
Figure 8.1 shows the cross section of an axisymmetric shell subjected to various
kinds of axisymmetric loads. In particular, the shell may contain a liquid of density
ρ
l
and a gas at pressure p, there may be resultant axial forces F from the attached
ductwork and reactions R at the support, and the density
ρ
s
of the shell material itself
may cause significant loading.
F
RR
gas
pressure
p
ρ g per unit volume
l
ρ g per unit volume
s
Figure 8.1: Loading of an axisymmetric shell
8.1 The meridional stress 387
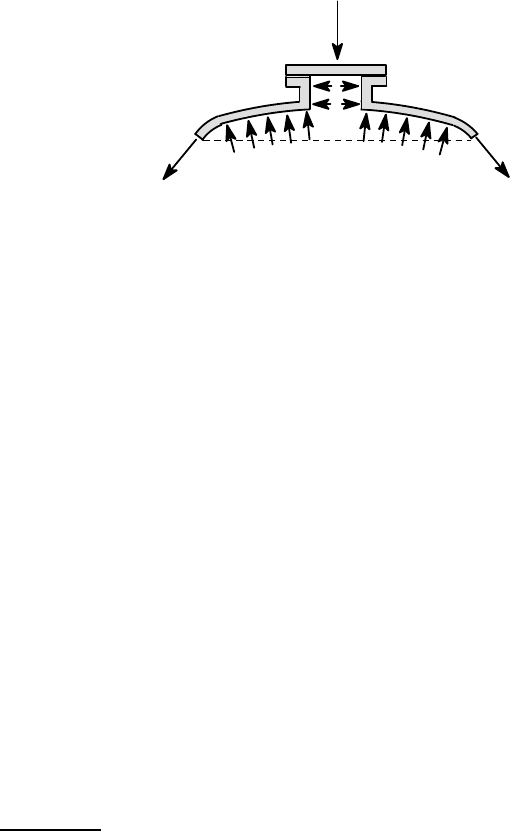
Suppose we now separate the shell into two parts by making a cut perpendicular
to the axis of symmetry. The free body diagram of one such part is shown in Figure
8.2. We anticipate a membrane stress
σ
1
at the cut as shown. Notice that the cut is
made perpendicular to the shell wall,
1
so as to expose the membrane stress which is
tangential to the wall.
F
p
1
σ
1
σ
Figure 8.2: Free body diagram exposing the meridional stress
σ
1
The segment of shell in Figure 8.2 must be in equilibrium under the action of the
forces shown and we can obtain an equilibrium equation by summing the forces in
the axial direction. If the shell and the applied loads are axisymmetric, the membrane
stress
σ
1
will be the same all around the cut, so this equation will be sufficient to
determine
σ
1
, which is known as the meridional stress or the longitudinal stress.
2
Example 8. 1
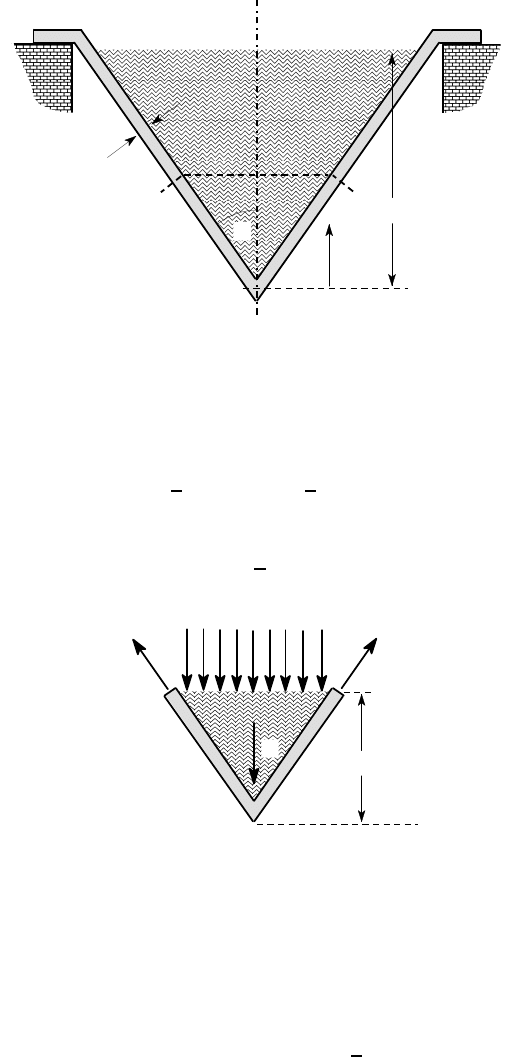
Figure 8.3 shows a conical tank, supported at the top and filled with water of density
ρ
to a height h above the vertex. The cone has semi-angle
α
and the wall thickness
is t. Find the meridional stress
σ
1
as a function of z and identify the location and
magnitude of the maximum value. The weight of the shell may be neglected.
To find
σ
1
at height z, we make the cut A−A shown in Figure 8.3, taking care to
go through the shell at right angles. The simplest procedure is to ‘cut’ through the
water as well, leaving the free-body diagram of Figure 8.4. Three forces act on the
system shown in this figure, resulting from:-
(i) the meridional stress
σ
1
acting on the cut shell surface;
(ii) the hydrostatic pressure
ρ
g(h−z) due to the head of water above A−A acting on
the water surface A−A; and
(iii) the weight W of the cone of water left below A−A and shown in Figure 8.4.
1
One way to remember this is to imagine actually making the cut with a hacksaw. The cut
to make is the one that requires the least effort, because it goes through the minimum wall
thickness.
2
Because on a globe regarded as a shell,
σ
1
would act in the direction of a line of longitude,
also known as a meridian.
388 8 Membrane Stresses in Axisymmetric Shells
Figure 8.3: The conical tank
To determine W , we note that the volume of a cone of height b and base radius a
is V =
π
a
2
b/3. Here, b=z and a =ztan
α
, giving
V =
1
3
π
(ztan
α
)
2
z =
1
3
π
z
3
tan
2
α
and
W =
ρ
gV =
1
3
πρ
gz
3
tan
2
α
.
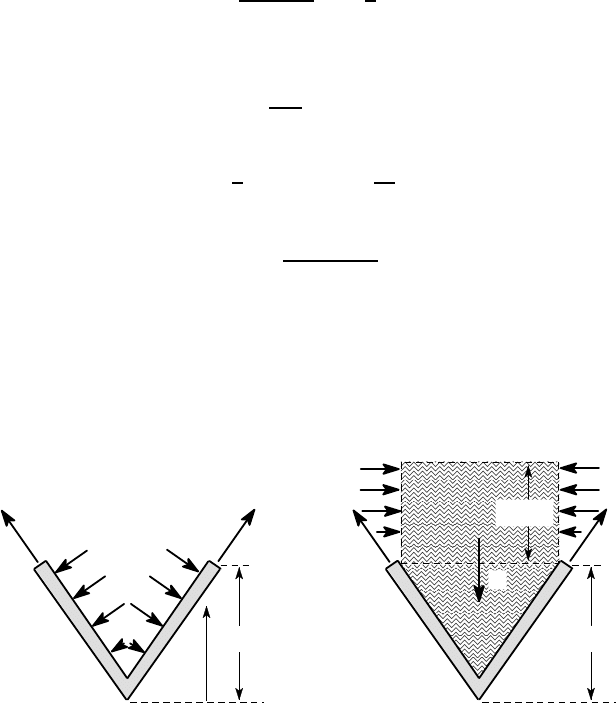
A
A
z
W
ρg (h - z)
σ
1
σ
1
Figure 8.4: Free-body diagram of the system below the cut A−A
The stress
σ
1
acts on the area of the cut through the shell wall, which is of
thickness t and circumferential length 2
π
a = 2
π
ztan
α
. Finally, the water pressure
ρ
g(h−z) acts over a circular area at A−A of radius a = z tan
α
whose area is there-
fore
π
(ztan
α
)
2
.
The three forces must sum to zero and hence
σ
1
(2
π
zt tan
α
)cos
α
−
ρ
g(h −z)
π
(ztan
α
)
2
−
1
3
πρ
gz
3
tan
2
α
= 0 , (8.1)
h
α
t
A
A
z
8.1 The meridional stress 389
where the factor cos
α
in the first term results from resolution of the force due to
σ
1
into the vertical (axial) direction.
Solving this equation for the meridional stress
σ
1
, we obtain
σ
1
=
ρ
gztan
α
2t cos
α
h −
2
3
z
.
We note that
σ
1
is zero at the vertex z = 0 and elsewhere is always positive. The
maximum value occurs when
d
σ
1
dz
= 0
which requires
h −
4
3
z = 0 or z =
3h
4
.
At this point, we have
σ
max
1
=
3
ρ
gh
2
tan
α
16t cos
α
.
8.1.1 Choice of cut
In Example 8.1, we made the cut in such a way as to leave a conical volume of water
in the free-body diagram of Figure 8.4, but any other axisymmetric cut could have
been used, two alternatives being illustrated in Figure 8.5 (a,b).
A
A
z
σ
1
σ
1
ρg (h - ζ)
ζ
(a) (b)
Figure 8.5: Alternative cuts for determining
σ
1
In Figure 8.5 (a), we show the shell segment only, implying a cut which passes
between the water and the shell surface. In this case, the pressure exerted by the
water on the shell must be shown, since it results in an external force on the shell
segment. The resultant axial force due to this pressure can be found by considering
the element of shell surface between height
ζ
and
ζ
+
δζ
above the vertex, multi-
plying by the local pressure
ρ
g(h−
ζ
), resolving the resulting elemental force into
A
A
z
W
σ
1
σ
1
(h - z)
B
B

390 8 Membrane Stresses in Axisymmetric Shells
the axial direction and summing by integration. The final result will be found to be
equal to the sum of terms (ii) and (iii) in the original derivation, but the calculation
is significantly more tedious, which is why we used the cut of Figure 8.4.
Another alternative cut, shown in Figure 8.5 (b), passes through the shell and then
goes vertically upwards to the water surface, so as to include an additional cylindri-
cal volume of water above the plane AA in the free-body diagram. This cylinder is
subjected to pressure from the rest of the water acting on the cylindrical surface AB,
but since these forces are horizontal, they do not feature in the vertical equilibrium
equation. Thus, the only two terms in the equilibrum equation are those due to
σ
1
and to the weight of water in the free-body diagram [(i) and (iii) of the preceding
solution]. However, the weight of water W is now increased by the weight of the
cylinder AB and it is easily verified that this is equal to the missing term (ii). The
volume of the cylinder is
V
cyl
=
π
(ztan
α
)
2
(h −z) ,
so its weight is
W
cyl
=
πρ
g(ztan
α
)
2
(h −z) ,
which is exactly equal to the second term in the equilibrium equation (8.1).
In general, any axisymmetric cut can be used to determine the meridional stress,
provided it cuts through the shell at only one location. All cuts will yield the same
equilibrium equation, but a careful choice will sometimes simplify the calculations.
A good ‘default option’ is to make a plane cut perpendicular to the axis,
3
as in Figure
8.4.
The three cuts defined by the free-body diagrams of Figure 8.4 and Figure
8.5 (a,b) yield the same final equilibrium equation because the volumes of water
involved — i.e. the conical volume in Figure 8.4 and in the cone and the cylinder
in Figure 8.5 (b) — are themselves in equilibrium under the action of gravity and
pressure forces.
In the limiting case where the density and hence the weight of the contents of a
pressure vessel is negligible, the pressure will be the same everywhere and we can
conclude that the resultant of a uniform pressure acting on a general curved surface
is the same for all surfaces terminating on the same closed curve.
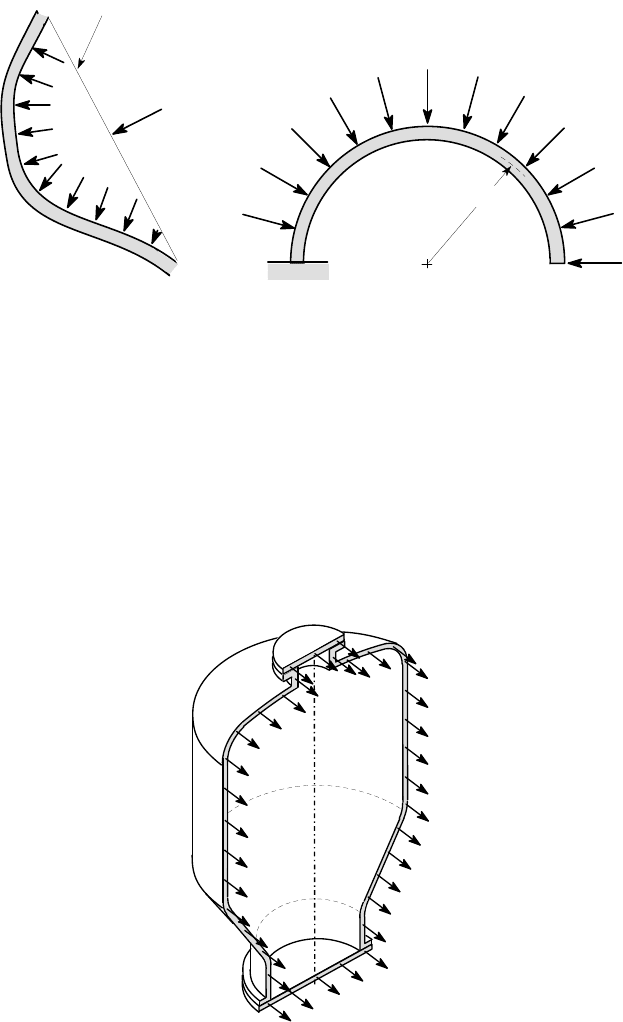
In particular, the uniform pressure p acting on the shell surface of Figure 8.6 (a) is
statically equivalent to a force pA
c
, where A
c
is the area of the plane surface A−A and
the line of action of this force passes through the centroid of A
c
and is perpendicular
to the plane A−A. This result can be useful outside the context of the membrane
theory of shells. For example, we can use it to deduce that the distributed load on the
curved beam of Figure 8.6 (b) is statically equivalent to a vertical force 2w
0
R acting
through the centre O.
3
Except in passing through the shell itself, where the cut must be normal to the local shell
surface.
8.2 The circumferential stress 391
A
A
p
area
A
c
A
c
p
A
R
O
0
w per unit length
(a) (b)
Figure 8.6: (a) uniform pressure on a general shell segment, (b) uniform normal
loading on a curved beam
8.2 The circumferential stress
Since the system is axisymmetric, there can be no shear stresses on the meridional
surface and hence
σ
1
is a principal stress. The other principal stress
σ
2
in the local
tangent plane to the shell is known as the circumferential stress or the hoop stress.
On a globe, it would act in the direction of a line of lattitude — i.e. in the East-West
direction.
2
σ
2
σ
Figure 8.7: The circumferential stress
σ
2
392 8 Membrane Stresses in Axisymmetric Shells
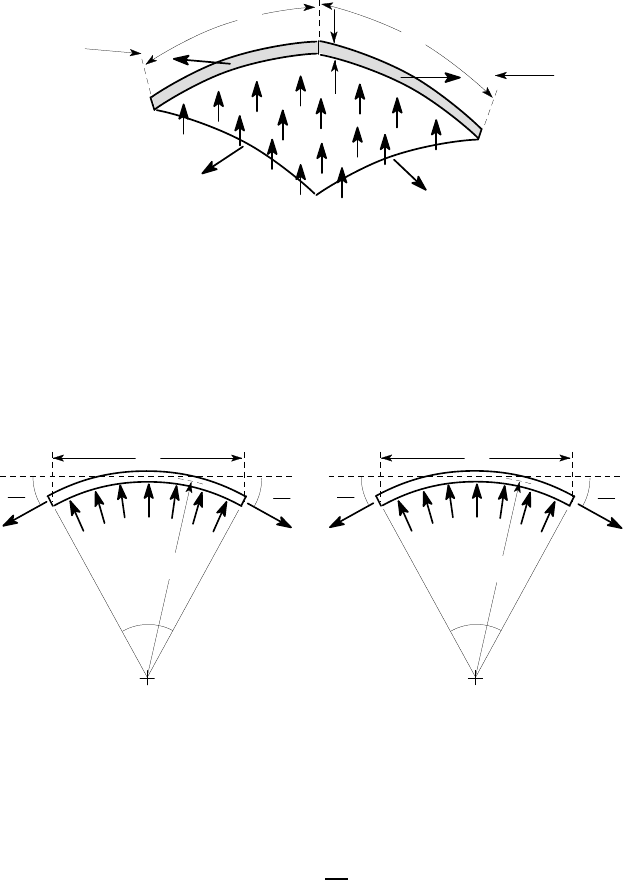
We can expose
σ
2
by making any plane cut that includes the axis of symmetry, as
shown in Figure 8.7, but we cannot generally determine it from such a cut because
σ
2
is not necessarily uniform over the cut surface. However, we can find it by con-
sidering the equilibrium in the through-thickness direction of the small rectangular
element of shell shown in Figure 8.8.
A
B
p
t
δy
δx
σ
1
σ
1
σ
2
σ
2
Figure 8.8: Equilibrium of a small rectangular element of shell
We suppose that the shell element is loaded only by an internal pressure p, acting
normal to the shell, and the membrane stresses
σ
1
,
σ
2
. The pressure exerts a force
p
δ
x
δ
y in the direction normal to the shell. To determine the forces due to
σ
1
,
σ
2
, we
first draw edge views of the element looking in directions A, B, as shown in Figure
8.9 (a,b), respectively. In general, the curvature of the shell will be different in the
two views, so we denote the corresponding radii by R
1
,R
2
, respectively.
R
1
δθ
p
σ t δy
1
σ t δy
1
δθ
2
δθ
2
δx
p
δφ
2
δφ
2
δφ
2
σ t δx
2
σ t δx
δy
R
2
(a) (b)
Figure 8.9: View of the shell element in (a) direction A and (b) direction B
As a result of the curvature, the length
δ
x in Figure 8.9 (a) subtends an angle
δθ
=
δ
x
R
1
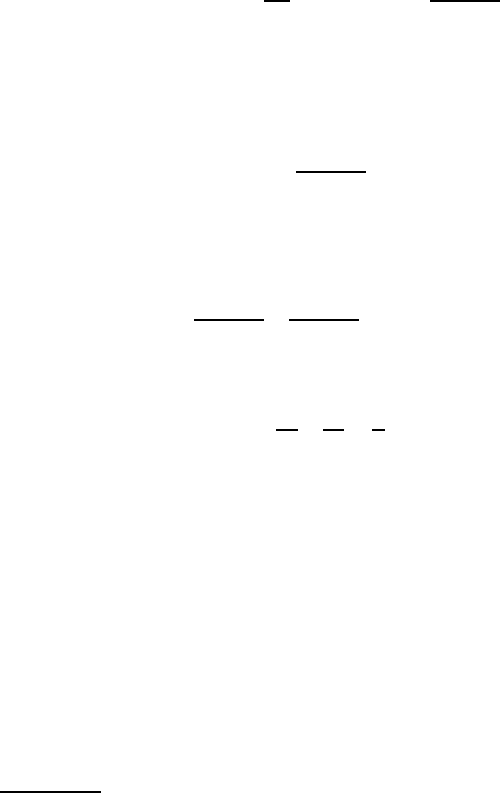
8.2 The circumferential stress 393
at the centre of curvature. The stress
σ
1
acting on the edges of the element cor-
responds to forces
σ
1
t
δ
y on each edge in Figure 8.9 (a) and these forces are not
horizontal in the figure because of the curvature of the shell. They therefore have
a resultant in the direction normal to the shell (vertically downwards in the figure)
equal to
2
σ
1
t
δ
y sin
δθ
2
≈
σ
1
t
δ
y
δθ
=
σ
1
t
δ
x
δ
y
R
1
.
The approximation involved in replacing sin(
δθ
/2) by
δθ
/2 will be exact in
the limit
δ
x →0. A similar argument applied to Figure 8.9 (b) shows that the forces
σ
2
t
δ
x due to stress
σ
2
have a resultant in the same direction (normal to the shell) of
σ
2
t
δ
x
δ
y
R
2
.
Summing the forces along the common normal for the element
δ
x
δ
y, we there-
fore obtain
σ
1
t
δ
x
δ
y
R
1
+
σ
2
t
δ
x
δ
y
R
2
− p
δ
x
δ
y = 0 ,
and hence
σ
1
R
1
+
σ
2
R
2
=
p
t
. (8.2)
The meridional stress
σ
1
can always be calculated by using axial equilibrium
arguments, as in §8.1, so equation (8.2) can be used to determine the circumferential
stress
σ
2
.
8.2.1 The ra dii of curvature
Notice that the shell element has two radii of curvature
4
R
1
,R
2
, which appear in
the two orthogonal views on A and B respectively (see Figure 8.9). These radii are
generally different. For example, for the cylindrical shell of Figure 8.10 (a), the cor-
responding element [Figure 8.10 (b)] will appear curved when looking in direction B
along the axis, giving R
2
=a. However, it will look straight when looking in direction
A, so R
1
=∞.
4
Even a general (non-axisymmetric) element of shell has two orthogonal principal curva-
tures and corresponding principal radii R
1
,R
2
. This can be established by a transformation
of coordinates mathematically analogous to those used in §2.1.1 and §4.4.1. For the ax-
isymmetric shell, the principal directions must always align with the circumferential and
axial directions.
394 8 Membrane Stresses in Axisymmetric Shells
a
σ
1
σ
2
σ
1
σ
2
A
B
(a) (b)
Figure 8.10: (a) A cylindrical shell and (b) the corresponding rectangular shell ele-
ment
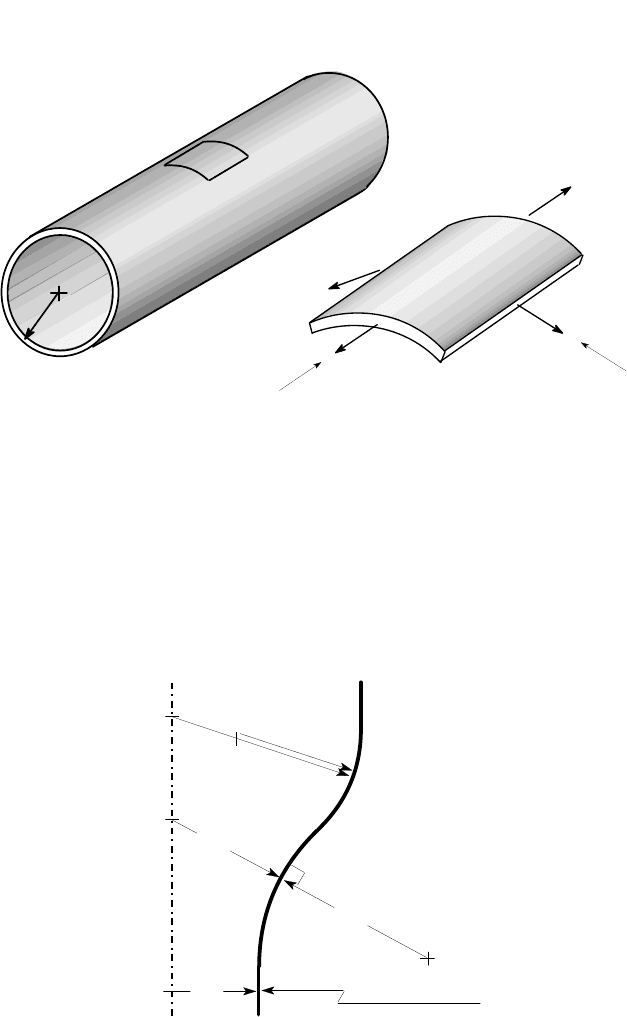
More generally, the radius R
1
is that which is seen in a cross-sectional view of
an axisymmetric shell — i.e. when the shell is sectioned on a plane including the
axis of symmetry. To determine the other radius R
2
, we first note that both centres
of curvature must lie on the common normal to the local piece of shell. Also, the
axisymmetry demands that the centre O
2
must lie on the axis of symmetry. To find
O
2
and hence R
2
, we therefore extend the normal until it cuts the axis, as shown for
various cases in Figure 8.11.
R
2
R
2
O
1
O
2
8
R
1
=
R
1
R
1
O
2
O
1
O
2
R
2
Figure 8.11: Radii of curvature for the shell
Take care not to confuse R
2
with the local cross-sectional radius r, shown in
Figure 8.12 (a). In determining the meridional stress, r will be the radius of the cross-
