Barber J.R. Intermediate Mechanics of Materials
Подождите немного. Документ загружается.

Problems 225
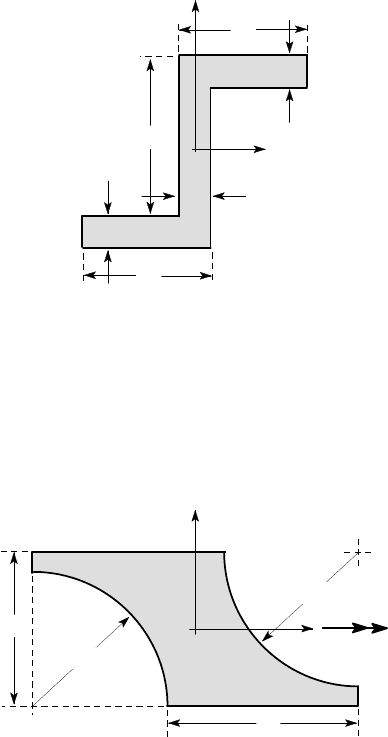
4.8. The Z-section of Figure P4.8 has second moments of area I
x
=560, 000 mm
4
, I
y
=
290, 000 mm
4
, I
xy
=300,000 mm
4
. It is used for a beam of length 2m which is simply
supported at its ends and loaded by a uniformly distributed vertical load of 1000 N/m.
Find the location and magnitude of the maximum tensile stress.
x
y
10
10
10
O
40
40
50
all dimensions in mm.
Figure P4.8
4.9. The beam section shown in Figure P4.9 has second moments of area I
x
=317, 600
mm
4
, I
y
= 791,700 mm
4
, I
xy
= −298,500 mm
4
. The beam is subjected to a bending
moment M
0
= 1000 Nm about the x-axis as shown. Find the equation defining the
neutral axis and hence the location and magnitude of the maximum tensile stress.
35
35
40
50
x
y
O
M
0
all dimensions in mm.
Figure P4.9
Section 4.2
4.10. Find the maximum horizontal and vertical components of the displacement for
the beam of Problem 4.8, if the beam is made of steel for which E = 210 GPa.
226 4 Unsymmetrical Bending
4.11. A beam with the triangular cross section of Figure P4.7 is of length L and made
of material with Young’s modulus E. It is built in at one end and loaded by a force F
in the x-direction at the other end. Find the two components of displacement at the
loaded end.
4.12. Figure P4.12 shows an L-section steel beam (E = 30×10
6
psi) with I
x
= 69.6
in
4
, I
y
= 11.6 in
4
, I
xy
= −16.2 in
4
. The beam is 12 feet long, is simply supported at
its ends and is loaded by a vertical force of 1000 lbs as shown. Find the horizontal
displacement at the point of application of the load.
1000 lb
6 ft
6 ft
Figure P4.12
4.13*. Figure P4.13 shows a beam with the cross section of Figure P4.9 loaded by a
uniformly distributed horizontal load of 1 kN/m. It is built in at A and the end B is
free to move horizontally, but is restrained from moving vertically by a frictionless
support. Find the vertical reaction and the horizontal displacement at B if the beam
is made of aluminium alloy for which E =70 GPa.
1 kN/m
A
B
2.2 m
Figure P4.13
Problems 227
Section 4.3
4.14. Calculate I
x
,I
y
,I
xy
for the symmetric
8
Z-section of Figure P4.8 above.
4.15. Calculate I
x
,I
y
,I
xy
for the section of Figure P4.9 above. You can use the results
from Example B.1.
4.16. Calculate I
x
,I
y
,I
xy
for the L-section shown in Figure P4.16.
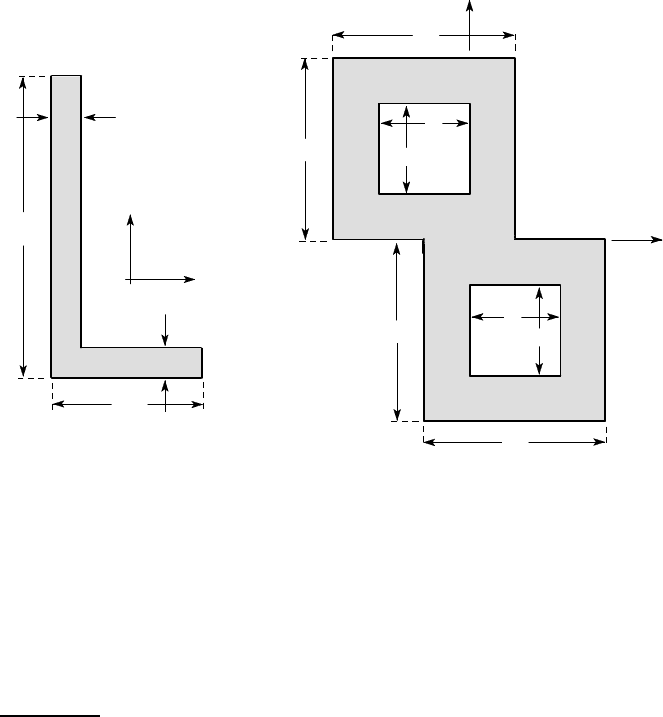
x
y
O
20
20
200
100
x
y
O
.
a
a
a
a
2a
2a
2a
2a
all dimensions in mm.
Figure P4.16 Figure P4.17
4.17. Find the product inertia I
xy
for the beam section shown in Figure P4.17. Notice
that the centroid must be at O by symmetry.
8
Notice that this category of symmetry (reflection through the origin) does not guarantee
I
xy
= 0, by the argument of Figure 4.6, since symmetric points have equal and opposite
values of both x and y coordinates, resulting in contributions to I
xy
of the same sign.
228 4 Unsymmetrical Bending
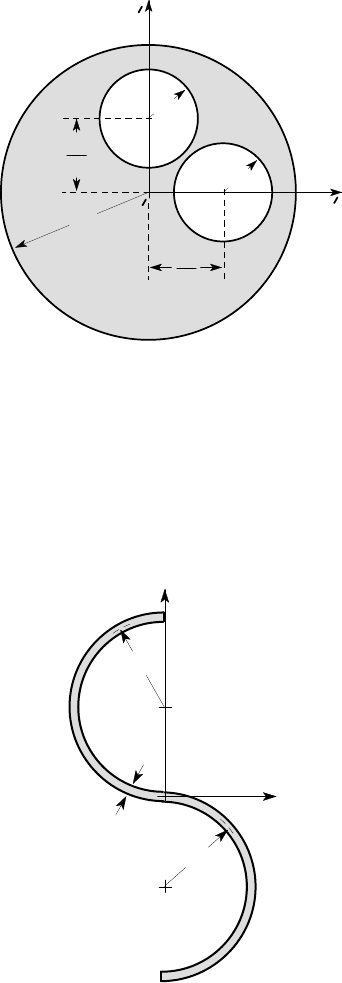
4.18. A beam of circular cross section, radius 3a has two eccentric circular holes of
radius a as shown in Figure P4.18.
2
3a
2
3a
O
3a
x
y
a
a
Figure P4.18
Find the centroid of the section and the second moments of area I
x
,I
y
,I
xy
about
centroidal axes.
Section 4.3.3
4.19.* Calculate I
x
,I
y
,I
xy
for the S-section of Figure P4.19, using the ‘t ≪a’ approx-
imation. Note: You will need to use the integration method of Appendix B.
O
a
a
x
y
t
Figure P4.19
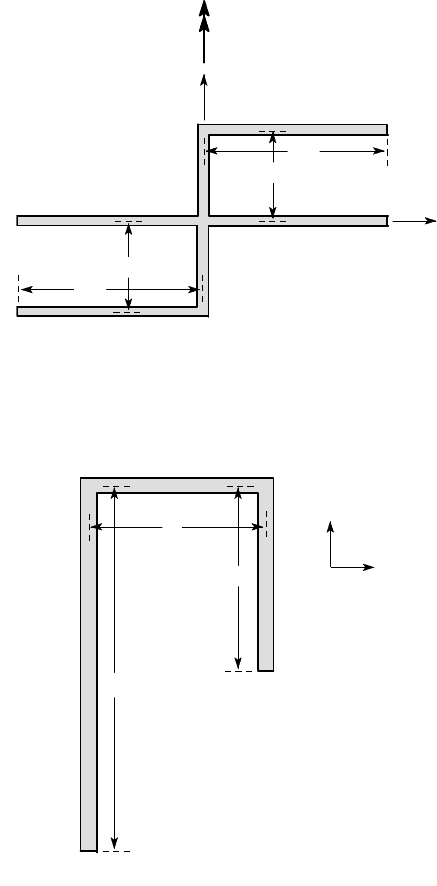
Problems 229
4.20. Calculate I
x
,I
y
,I
xy
for the section of Figure 4.15, using the ‘t ≪a’ approxima-
tion and describing the section by the mean line. Which of the three second moments
is the most in error by this method and by what percentage?
4.21. A beam of the section shown in Figure P4.21 is loaded by a bending moment
M
0
about the y-axis. Calculate I
x
,I
y
,I
xy
using the ‘t ≪a’ approximation and hence
determine the inclination of the neutral axis.
x
O
.
a
a
2a
2a
y
M
0
all thicknesses = t
Figure P4.21
4.22. Find the location of the centroid and the second moments of area I
x
,I
y
,I
xy
for
the thin-walled section of Figure P4.22.
x
y
all thicknesses = t
a
a
2a
Figure P4.22

230 4 Unsymmetrical Bending
4.23. Find the location of the centroid and the second moments of area I
x
,I
y
,I
xy
for
the section of Figure P4.23.
x
y
2
1
1
1.6
20
8
6
all dimensions in mm.
Figure P4.23
Section 4.4
4.24. For the cross section of Figure P4.21, with a = 20 mm, t = 3 mm, we obtain
I
x
=160×10
3
mm
4
, I
y
=256×10
3
mm
4
, I
xy
=96×10
3
mm
4
. Sketch the Mohr’s circle
of second moments of area and hence determine the magnitudes of the principal
second moments and the inclination of the principal axes to the x and y axes. Draw
in the principal axes on a copy of the figure and identify the stiff and flexible axes as
‘1’, ‘2’, respectively.
4.25. Sketch Mohr’s circle of second moments of area for the section of Figure P4.6,
using the results for I
x
,I
y
,I
xy
given in problem 4.6. Hence determine the magnitudes
of the principal second moments and the inclination of the principal axes to the x and
y axes. Draw in the principal axes on a copy of the figure and identify the stiff and
flexible axes as ‘1’, ‘2’, respectively.
4.26. Sketch Mohr’s circle for second moments of area for the section of Figure P4.8,
using the results for I
x
,I
y
,I
xy
given in problem 4.8. Hence determine the magnitudes
of the principal second moments and the inclination of the principal axes to the x and
y axes. Draw in the principal axes on a copy of the figure and identify the stiff and
flexible axes as ‘1’, ‘2’, respectively.
4.27. Using the results for I
x
,I
y
,I
xy
given in problem 4.9, determine the magnitudes of
the principal second moments and the inclination of the principal axes for the section
of Figure P4.9. Draw in the principal axes on a copy of the figure and identify the
stiff and flexible axes as ‘1’, ‘2’, respectively.
4.28. The second moments of area for the section of Figure P4.28 are
I
x
= 26a
3
t ; I
y
=
40a
3
t
3
; I
xy
= −14a
3
t .
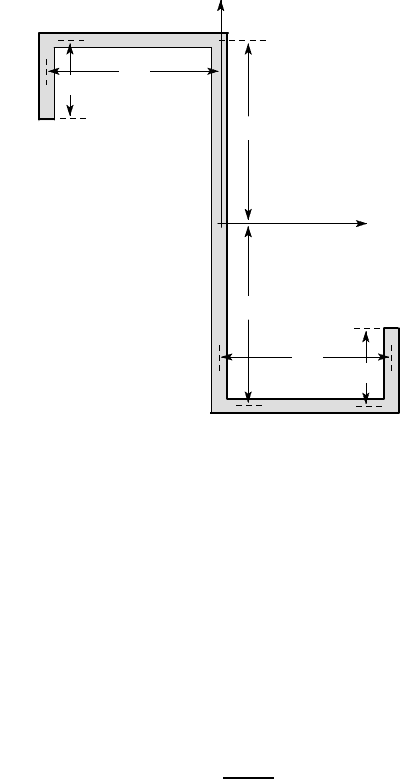
Problems 231
y
2a
2a
x
O
2a
a
a
2a
all thicknesses = t
Figure P4.28
Find the principal second moments of area and the inclination of the principal
axes. Draw in the principal axes on a copy of the figure and identify the stiff and
flexible axes as ‘1’, ‘2’, respectively.
4.29*. A beam with second moments of area I
x
,I
y
,I
xy
is loaded by a bending moment,
M
0
about the x-axis. Find an expression for the angle
ψ
between the resulting neutral
axis and the x-axis.
Devise a geometrical construction for the angle
ψ
on the Mohr’s circle of Figure
4.21 and hence show that for a given section, the maximum deviation between the
moment axis and the neutral axis is
ψ
max
= sin
−1
I
1
−I
2
I
1
+ I
2
.
Find the angle between the moment axis and the stiff axis (1) for this maximum
deviation to occur.
Section 4.4.3
4.30. Figure P4.30 shows the cross section of an unequal angle beam for which the
principal second moments of area are I
1
= 23.95×10
6
mm
4
, I
2
= 2.53×10
6
mm
4
.
The location of the centroid and the inclination of the principal axes are as shown.
The beam is subjected to a bending moment of 10 kNm about the x-axis. Find the
location and magnitude of the maximum tensile and compressive stress.
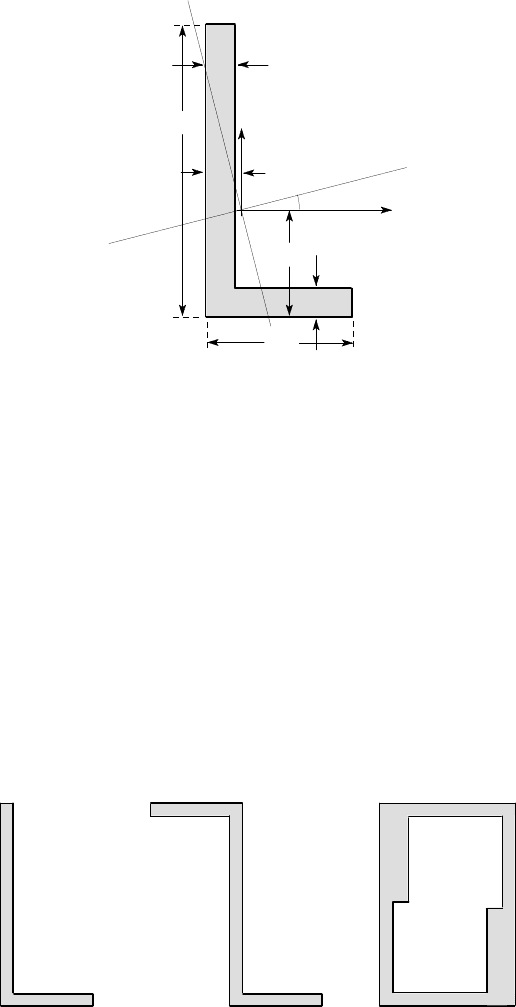
232 4 Unsymmetrical Bending
x
y
O
20
20
100
1
2
200
24.3
14.34
o
74.3
all dimensions in mm.
Figure P4.30
4.31. The beam of Problem 4.30 is subjected to a bending moment of 6 kNm about
the y-axis. Find the location and magnitude of the maximum tensile and compressive
stress.
4.32. The unequal Z-section of Figure 4.17 (a) has principal second moments I
1
=
5.255a
3
t,I
2
=0.761a
3
t and the inclination of the principal axes are as shown in Fig-
ure 4.23. The beam is loaded by a bending moment M
0
about the x-axis. Find the
location and magnitude of the maximum tensile stress.
Section 4.4.4
4.33. Make a copy of the three beam sections in Figure P4.33 and use the ‘least
squares fit’ method to estimate the location of the principal axes on each section.
Label the stiff and flexible axes ‘1’ and ‘2’ respectively.
(a) (b) (c)
Figure P4.33
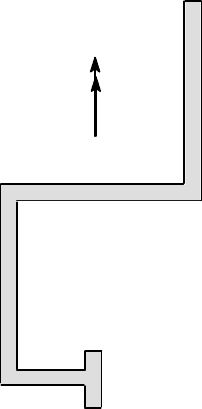
Problems 233
4.34. Make a copy of the beam section of Figure P4.17 and use the ‘least squares fit’
method to estimate the location of the principal axes on each section. Label the stiff
and flexible axes ‘1’ and ‘2’ respectively.
4.35. Make a copy of the beam section of Figure 4.15 and use the ‘least squares fit’
method to estimate the location of the principal axes on each section. Label the stiff
and flexible axes ‘1’ and ‘2’ respectively.
4.36. Make a copy of the beam section of Figure P4.22 and use the ‘least squares fit’
method to estimate the location of the principal axes on each section. Label the stiff
and flexible axes ‘1’ and ‘2’ respectively.
4.37. A beam with the cross section of Figure P4.37 is loaded by a bending moment
about the vertical axis as shown. Show on the figure your best estimate of (i) the
location of the principal axes, (ii) the location of the neutral axis and (iii) the point P
where the maximum tensile stress will occur.
M
Figure P4.37
4.38. A beam with the S-section of Figure P4.19 is loaded by a bending moment
about the x-axis. Show on the figure your best estimate of (i) the location of the
principal axes, (ii) the location of the neutral axis and (iii) the point P where the
maximum tensile stress will occur.
4.39. Estimate the location of the principal axes for the beam section of Figure P4.6
using the ‘least squares fit’ method and hence find the approximate location of the
234 4 Unsymmetrical Bending
neutral axis when the beam is loaded by a bending moment about the x-axis. If you
previously solved Problem 4.6, compare your estimate for the inclination of the neu-
tral axis with the exact calculation.
4.40. Estimate the location of the principal axes for the beam section of Figure P4.9
using the ‘least squares fit’ method and hence find the approximate location of the
neutral axis when the beam is loaded by a bending moment about the x-axis. If you
previously solved Problem 4.9, compare your estimate for the inclination of the neu-
tral axis with the exact calculation.
Section 4.4.5
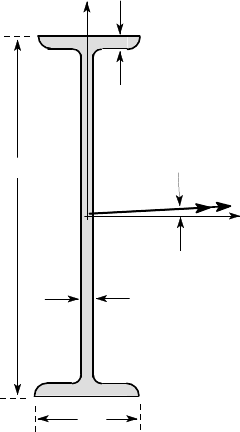
4.41. The I-beam shown in Figure P4.41 is subjected to a bending moment which is
intended to be aligned with the axis Ox, but which is actually inclined at 1
o
towards
Oy because of a manufacturing error.
O
x
y
4
4
180
40
M
0
o
1
all dimensions in mm.
Figure P4.41
Find I
x
,I
y
and hence the direction of the neutral axis, (which is also the axis about
which bending occurs).
4.42. An I-beam section is to be used for a cantilever beam to support an end load
whose direction may deviate from the vertical by ±0.5
o
due to manufacturing and
assembly errors. Determine the maximum permissible ratio I
1
/I
2
if the horizontal
displacement is not to exceed 10% of the vertical displacement.
